Association of Incident Hip Fracture with the Estimated Femoral Strength by Finite Element Analysis of DXA Scans in the Osteoporotic Fractures in Men (MrOS) Study

Abstract

Purpose

Bone fractures only when it is loaded beyond its ultimate strength. The goal of this study was to determine the association of femoral strength, as estimated by finite element (FE) analysis of DXA scans, with incident hip fracture as a single condition or with femoral neck (FN) and trochanter (TR) fractures separately in older men.

Methods

This prospective case-cohort study included 91 FN and 64 TR fracture cases and a random sample of 500 men (14 had a hip fracture) from the MrOS study during a mean±SD follow-up of 7.7±2.2 yrs. We analysed the baseline DXA scans of the hip using a validated plane-stress, linear-elastic FE model of the proximal femur and estimated the femoral strength during a sideways fall.

Results

The estimated strength was significantly (P<0.05) associated with hip fracture independent of the TR and total hip (TH) BMDs but not FN BMD, and combining the strength with BMD did not improve the hip fracture prediction. The strength estimate was associated with FN fractures independent of the FN, TR and TH BMDs, the age-BMI-BMD adjusted hazard ratio (95% CI) per SD decrease of the strength were 1.68 (1.07–2.64), 2.38 (1.57, 3.61) and 2.04 (1.34, 3.11) respectively. This association with FN fracture was as strong as FN BMD (Harrell’s C index for the strength 0.81 v. FN BMD 0.81) and stronger than TR and TH BMDs (0.8 v. 0.78 and 0.81 v. 0.79). The strength’s association with TR fracture was not independent of hip BMD.

Conclusions

Although the strength estimate provided additional information over the hip BMDs, its improvement in predictive ability over the hip BMDs was confined to FN fracture only and limited.

SUMMARY

Finite element model can estimate bone strength better than BMD. This study used such a model to determine its association with hip fracture risk and found that the strength estimate provided limited improvement over the hip BMDs in predicting FN fracture risk only.

INTRODUCTION

Many factors in combination affect hip fracture risk, but a major contributor is reduced mechanical strength of the proximal femur since fracture occurs only when bone strength is too low to sustain mechanical loads upon it. Low areal bone mineral density (BMD), as measured by dual-energy absorptiometry (DXA), is significantly correlated with femoral strength in cadaver specimen experiments^{[1, 2]} and highly associated with clinical risk of hip fracture ^[3]. The accuracy of areal BMD in predicting individual fracture risk is limited: as many as 54% hip fractures occur in postmenopausal women without osteoporosis by WHO definition (BMD T-score <= −2.5) ^[4]. BMD as a bone strength determinant is limited by the facts that DXA is a two-dimensional projection of a 3-dimensional bone and, in addition to BMD, bone geometry, density spatial distribution and bone material properties contribute to bone strength^[5].

Patient-specific finite element (FE) models of the proximal femur based on DXA or quantitative computer tomography (QCT) integrate the bone density distribution, geometry, material mechanical properties and loading conditions of sideways fall to estimate the bone strength non-invasively. Such estimates of femoral strength from QCT-based FE models have been reported to discriminate between no fracture and incident hip fracture ^[6–8] or prevalent hip fracture ^{[9, 10]} in men and women. These estimates have also been used to investigate age- and gender-related differences ^{[7, 11]}, to examine effects of drug therapy for osteoporosis ^[12–15]. On the other hand, few DXA-based FE models have been evaluated in clinical studies and all of them in women ^[16–20].

The purpose of this study was to determine whether the estimated bone strength from a DXA-based FE model of the proximal femur can predict hip fracture, as a single condition or as femoral neck (FN) and trochanteric (TR) fractures separately, independently of hip BMD in older men.

MATERIALS AND METHODS

Study population and case-cohort selection

Details of design and recruitment of the MrOS study have been published ^[21]. Briefly, the MrOS study recruited 5994 community-dwelling, ambulatory men at least 65 yr of age at six U.S. cities from March 2000 to April 2002. Men were not enrolled if they were unable to walk without assistance, had a life-threatening medical condition, or had undergone previous bilateral hip replacements. All participants completed the baseline self-administered questionnaire and attended the baseline visit during which the hip DXA scans were performed. Hip DXA scans were performed using scanners of the same type and same manufacturer (QDR 4500, Hologic Inc, Waltham, USA). Questionnaires were sent to men tri-annually to report any fractures. Medical records were used to verify reported all fractures, and the fractures were confirmed by blinded central adjudicators. Pathologic fractures were excluded. The location of the hip fractures was identified as femoral neck, intertrochanteric, subtrochanteric or other hip fracture. All participants provided written informed consent, and the study was approved by the Institutional Review Board at each site.

This prospective case-cohort study included a random sample of 500 men and 170 incident hip fracture cases (16 in the random sample) during a mean±SD follow-up of 7.7±2.2 yrs from the MrOS study. Among the incident hip fractures, 91 were identified as femoral neck (FN), 64 as trochanteric (TR, intertrochanteric or subtrochanteric) and 15 as neither FN nor TR.

Finite element analysis of DXA scans

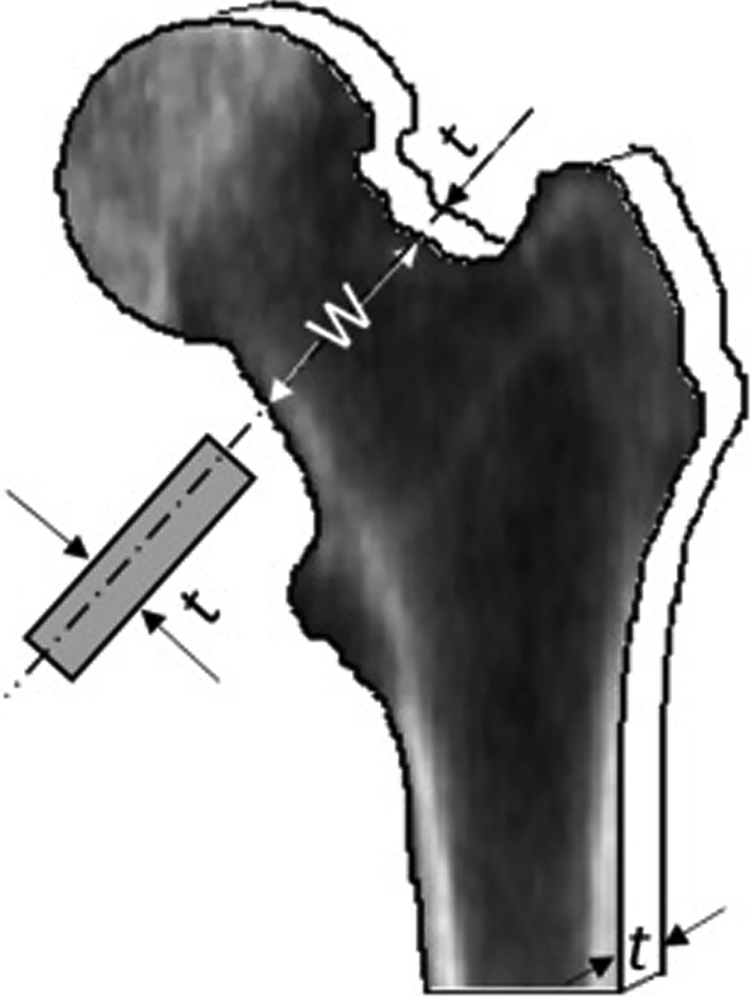
The procedures for performing a linear-elastic FE analysis of DXA scans has been described in detail previously ^{[18, 19, 22]}. A special program provided by Hologic Inc (Hologic Inc, Bedford, USA) was used to extract a pixel-by-pixel BMD map from each DXA scan. The proximal femur was segmented from each bone map and an FE model generated with each femoral pixel (width 0.51 mm by height 0.46 mm) converted into a brick element with 4 nodes at each corner. We took the following approach to assigning mechanical properties to each element. We assumed that the proximal femur was a plate with a patient-specific constant thickness of t=3.5πW/16 where W is the mean width of the middle third cross-sections of the femoral neck on the BMD map (Figure 1). We imposed a condition that the cross-sectional areas and moments of inertia were as close as possible to the plate’s rectangular and the assumed anatomical circular cross-sections. Areal BMD ρ_a was converted to volumetric BMD ρ_v=ρ_a/t, then to apparent density ρ_app= ρ_v /(1.14×0.598)^[23], which was used to derive bone modulus of elasticity using the empirical equations of Morgan et al ^[24] (quoted in ^[22]):

$$\mathit{\text{Modulus}}\mathit{\text{of}}\mathit{\text{Elasticity}}(MPa)=\left\{\begin{array}{c}15010{\rho }_{app}^{2.18}if{\rho }_{app}\le 0.280g/c{m}^3\\ 6850{\rho }_{app}^{1.49}if{\rho }_{app}>0.280g/c{m}^3\end{array}\right\}$$

Figure 1.

The plate model of the proximal femur with a constant thickness t

The above material properties were increased by a factor of 1.28 to account for the side-artefact errors in biomechanical testing of cadaveric trabecular specimen to estimate the relationship between bone density and material properties ^[25]. The Poisson’s ratio was assigned to 0.35. A sideways fall was simulated with the femoral shaft at 30 degrees against the ground; a force of 500 N applied to the greater trochanter vertically, medial surface of the femoral head constrained in the vertical direction and the most distal femoral shaft constrained in the horizontal direction. We performed linear-elastic analysis without considering the post-yield behaviour since the proximal femur behaves linearly elastic until failure ^[26].

Our previous experimental validation^[22] showed that the best failure criteria to define the femoral strength using our FE model was the principal compressive yield strain ε_pc:

$${\epsilon }_{pc}=\frac{{\epsilon }_x+{\epsilon }_y}{2}-\sqrt{{\left(\frac{{\epsilon }_x-{\epsilon }_y}{2}\right)}^2+\frac{{\gamma }_{xy}^2}{4}}$$

where ε_x and ε_y are element normal strain along the x and y directions and γ_xy element shear strain. A compressive yield strain ε_yield of 1.04% for femoral trabecular bone ^[27] was used to calculate strain ratio of ε_pc to ε_yield for each element. A contiguous area of 9 mm² (about 45 elements), within an anatomical region bounded proximally by the sub capital line and distally by a transverse line passing through the distal end of the lesser trochanter, was identified that contains the highest strain ratio, in which hip fracture would occur or initiate. The femoral strength was calculated by dividing the applied force by the minimum strain ratio in that area.

The FE analysis was performed blind to fracture status in Sheffield.

Statistical Analysis

All statistical analyses were performed at UCSF using SAS (version 9). Cox proportional hazard regression with Prentice weighting method and robust variance estimation ^[28] was performed to model the time to first incident hip fracture for total hip (TH), trochanter (TR) and femoral neck (FN) BMDs and the estimated strength and for the combinations of the strength estimate and each hip BMD. Hazard ratios (HR), adjusted for age and BMI, were expressed per one SD decrease of the hip BMDs and estimated strength. Since the estimated strength was highly correlated to hip BMDs predictive abilities of different models were compared using the Harrell’s C index, a concordance measure for survival data analogous to the area under a receiver operating characteristic curve (AUC). With clinical translation in mind, we performed linear regression between the estimated strength and FN BMD and defined strength intervention threshold as the strength corresponding to FN BMD T-score=−2.5 (based on the NHANES 2005–2008 data for both sexes). Sensitivity and specificity were calculated for three classifiers: FN BMD T-score < −2.5, the estimated strength < strength intervention threshold, and either of the above two. The above analyses were repeated treating FN and TR fractures separately. Statistical significance was set at P<0.05.

RESULTS

The baseline characteristics of men in the random cohort of this case-cohort study were similar to the remaining men in the MrOS population (Table 1).

Table 1. Baseline characteristics of men in different cohorts.

	Random Cohort (n= 500)	Not selected (n=5457)	Whole Cohort (n= 5957)	p
Age (yrs)	74.2 (6.1)	73.6 (5.8)	73.6 (5.9)	0.0224
Weight (kg)	81.6 (12.6)	81.3 (12.8)	81.3 (12.8)	0.6539
Height (cm)	174 (7)	174 (7)	174 (7)	0.4708
BMI (kg/m2)	26.8 (3.5)	26.8 (3.7)	26.8 (3.7)	0.5862
Femoral neck BMD (g/cm2)	0.78 (0.12)	0.78 (0.13)	0.78 (0.13)	0.6264
Trochanter BMD (g/cm2)	0.76 (0.12)	0.77 (0.13)	0.77 (0.13)	0.3752
Total Hip BMD (g/cm2)	0.95 (0.14)	0.96 (0.14)	0.96 (0.14)	0.4749

Data are presented as mean (SD)

p values are t, test results comparing random v. not selected cohorts

Table 2 shows the baseline characteristics by fracture status. Compared with men without incident hip fracture, men with any incident all hip, or men with FN and TR fractures separately, were significantly older and had lower weight, hip BMD, and estimated femoral strength. Compared with men with incident FN fracture, men with incident TR fracture had significantly lower TH BMD and TR BMD.

Table 2. Baseline characteristics of men with and without incident hip fractures.

	Non fracture	Femoral neck (FN) fracture	Trochanter (TR) fracture	Neither FN nor TR fractures	Any hip fracture
n	486	91	64	14	170
Age (yrs)	74.1 (6.1)	78.7 (6.1)*	77.1 (6.2)*	77.8 (5.8)	78.0 (6.1)*
Weight (kg)	83.3 (13.2)	80.2 (13.3)*	78.6 (11.2)*	77.0 (13.6)	82.3 (13.2)*
Height (cm)	175 (11)	173 (6)	174 (6)	172 (6)	173 (6)
BMI (kg/m2)	27.3 (3.9)	26.8 (4.1)	26.0 (3.6)*	25.9 (3.5)	26.4 (3.9)*
Femoral neck BMD (g/cm2)	0.79 (0.12)	0.67 (0.11)*	0.65 (0.10)*	0.66 (0.15)	0.66 (0.11)*
Trochanter BMD (g/cm2)	0.76 (0.12)	0.68 (0.11)*#	0.62 (0.10)*	0.63 (0.18)	0.65 (0.11)*
Total Hip BMD (g/cm2)	0.96 (0.13)	0.84 (0.13)*#	0.79 (0.11)*	0.81 (0.19)	0.82 (0.13)*
Strength (N)	4185 (982)	3381 (831)*	3529 (824)*	3415 (854)	3440 (828)*

Data are presented as mean (SD)

^*and

^#indicate that the mean is significantly different from non-fractures and TR fracture at P<0.05, respectively.

Table 3 shows the HRs for hip fractures associated with each SD decreases in hip BMDs and estimated strength. All hip BMDs (TH, TR and FN) and estimated strength were associated with a significantly increased age- and BMI-adjusted risk of hip fracture, either as a single condition or separately as FN and TR fractures. The strength estimate was still associated with all hip fracture after further adjustment for TH and TR BMDs but not for FN BMD. After further adjustment for hip BMDs, the estimated strength was associated with FN but not with TR fractures.

Table 3. Hazard ratio (95% CI) of new hip fracture associated with 1 SD decrease in variable values.

	Adjusted for
	age BMI	Age BMI BMD
Non-fracture (n=486) & hip fracture (n=170)
FN BMD	4.33 (2.95, 6.33)
TR BMD	3.20 (2.34, 4.37)
TH BMD	3.99 (2.87, 5.54)
FE strength	2.37 (1.71, 3.27)	n1.32 (0.89, 1.95)
		t1.70 (1.21, 2.38)
		h1.56 (1.10, 2.21)
Non-fracture (n=486) & FN fracture (n=91)
FN BMD	3.81 (2.46, 5.89)
TR BMD	2.23 (1.60, 3.10)
TH BMD	3.10 (2.12, 4.54)
FE strength	2.83 (1.92, 4.17)	n1.68 (1.07, 2.64)
		t2.38 (1.57, 3.61)
		h2.04 (1.34, 3.11)
Non-fracture (n=486) & TR fracture (n=64)
FN BMD	5.48 (3.22, 9.33)
TR BMD	5.80 (3.34, 10.06)
TH BMD	5.87 (3.65, 9.43)
FE strength	2.26 (1.53, 3.33)	n0.90 (0.52, 1.57)
		t1.03 (0.64, 1.66)
		h1.05 (0.65, 1.71)

Superscripts n, t and h indicate that the hazard ratio was adjusted for FN, TR and TH BMD, respectively.

Table 4 shows the Harrell’s C-indices (AUC for survival data) that demonstrate the ability of various Cox regression models to predict all hip or FN and TR fractures. In combination with age and BMI, the estimated strength performed significantly better than TR or TH BMD but similar to FN BMD in predicting FN fractures. For predicting all hip fracture and TR fractures, hip BMDs performed better than estimated strength. Combining hip BMDs and FE estimates resulted in significant increases in the C-indices only for FN fracture prediction.

Table 4. Harrell’s C-indices showing ability of Cox regression models to predict new hip fractures.

	Plus
	age BMI	Age BMI BMD
Non-fracture (n=486) and hip fracture (n=170)
FN BMD	0.79 (0.75, 0.81)*
TR BMD	0.77 (0.72, 0.80)
TH BMD	0.79 (0.75, 0.81)*
FE strength	0.76 (0.71, 0.79)	n0.79 (0.75, 0.81)
		t0.78 (0.74, 0.81)*
		h0.79 (0.75, 0.82)
Non-fracture (n=486) and FN fracture (n=91)
FN BMD	0.81 (0.74, 0.84)
TR BMD	0.78 (0.73, 0.81)
TH BMD	0.79 (0.75, 0.83)
FE strength	0.80 (0.74, 0.83)	n0.81 (0.76, 0.85)
		t0.80 (0.76, 0.84)*
		h0.81 (0.77, 0.84)*
Non-fracture (n=486) and TR fracture (n=64)
FN BMD	0.81 (0.74, 0.85)*	0.80 (0.74, 0.84)
TR BMD	0.82 (0.77, 0.85)*	0.81 (0.76, 0.85)
TH BMD	0.84 (0.80, 0.87)*	0.82 (0.79, 0.85)
		n0.82 (0.77, 0.86)
FE strength	0.75 (0.68, 0.80)	t0.82 (0.77, 0.85)
		h0.84 (0.79, 0.86)

Superscripts n, t and h indicate that the C-index was compared with that of FN, TR and TH BMD, respectively.

Superscripts

^*indicate that there are significant differences in C-indices between the FE strength and the corresponding hip BMD.

The estimated strength intervention thresholds corresponding to the NHANES 2005–2008 male and female FN BMD T-scores equal to −2.5 were 3318 N and 3103 N, respectively. Table 5 shows sensitivity and specificity of predicting all hip, FN and TR fractures. The sensitivity was higher but the specificity lower when using the male vs. female reference data. For predicting the FN fractures, the FN BMD T-score < −2.5 threshold had lowest sensitivity and highest specificity, followed by the estimated strength below the intervention threshold and then either of the two (i.e. the presence of either FN BMD T-Score < −2.5 or estimated strength below the intervention threshold). For predicting the TR and all hip fractures, the estimated strength intervention threshold had the lowest sensitivity and highest specificity, followed by the FN BMD T-score < −2.5 and then either of the two.

Table 5. Sensitivity (specificity) of various classifiers for hip fractures.

			All hip fracture	Femoral neck (FN) fracture	Trochanteric (TR) fracture
Using NHANES 2005–2008 male FN BMD reference 0.948 (±0.124) g/cm2
T-Score < −2.5			0.46 (0.91)	0.45 (0.91)	0.48 (0.91)
FE strength < 3318 N			0.45 (0.82)	0.49 (0.82)	0.39 (0.82)
T-score < −2.5 or FE strength < 3318 N			0.57 (0.79)	0.62 (0.79)	0.53 (0.79)
Using NHANES 2005–2008 female FN BMD reference 0.884 (±0.113) g/cm2
T-Score < −2.5			0.32 (0.95)	0.31 (0.95)	0.36 (0.95)
FE strength < 3103 N			0.36 (0.88)	0.41 (0.88)	0.30 (0.88)
T-score < −2.5 or FE strength < 3103 N			0.47 (0.86)	0.51 (0.86)	0.45 (0.86)

DISCUSSION

QCT-based FE models of the proximal femur have been evaluated in many clinical studies ^[6–15], whereas evaluations of DXA-based models are much fewer and are limited to women ^[16–19]. This study is the first prospective evaluation of the association of estimated femoral strength with any hip as well as FN and TR fracture risk in men. A central question in such studies is whether the FE strength provides additional information beyond hip BMD to improve hip fracture discrimination/prediction. This is especially true for DXA-based FE analysis since both hip BMD and FE strength are derived from the same scan. In this study, the estimated femoral strength was significantly associated with incident hip fracture independent of TH and TR BMDs but not of FN BMD, whereas adding the estimated strength to hip BMDs did not improve the predictive ability. Considering FN and TR fractures separately, the estimated strength was independently associated with FN fracture only, and the predictive ability for FN fractures, as judged by sensitivity of the intervention thresholds of the estimated strength (< 3318 N) and FN BMD T-score (< −2.5), was enhanced moderately when either of the thresholds was satisfied. The estimated strength did not perform better than hip BMDs for TR fractures. It appears that, since the FE model integrates the bone geometry, density distribution and impact force in sideway falls, the estimated strength derived from the FE model does provide additional information on the bone fragility, but its contribution to fracture prediction improvement in men is moderate at best and limited to FN fractures only.

The moderately large number of hip fracture cases in this study enabled us to investigate FN and TR fractures separately, which lead to some interesting findings. The estimated strengths in men (control: 4185 N; fracture: 3440 N) were much higher than those found in women in previous studies^[19] (control: 2614^[18] and 3027^[19] N; fracture: 1820^[18] and 2236^[19] N), which is most likely due to higher BMD and larger bone size in men, whereas there was no significant difference in the estimated strength between FN and TR fractures for both men and women. Although a lower estimated strength was associated with both FN and TR fractures in men and women, the estimated strength was an independent discriminator for both FN and TR fractures in women but for FN fracture only in men. Significantly lower hip BMDs in TR fractures than in FN fractures dominated the fracture risk in men and may partially explain this difference. Alternatively, lifestyle differences between men and women that result in men being exposed to greater levels of trauma^[29] might also account for part of this difference.

Other subtle differences between FN and TR fractures in bone density distribution and structure have been reported, mostly in women. Hip structure analysis of DXA and radiograph images reveal that the current BMD-based clinical assessment procedure is adequate to predict TR fracture ^[30] but may underestimate FN fracture risk ^[31], since women with FN fracture tend to have a much more complex risk profile such as longer femoral neck length, wider neck-shaft angle and narrower neck width than in the control or patient with TR fracture ^[32–35]. Three-dimensional analyses based on QCT ^[36–41] showed that, in addition to reduced cortical and trabecular vBMD, reduced cortical thickness was also associated with hip fracture in both women and men. Bousson et al ^[37] found that a regression model combining trochanteric BMD and mean trochanteric cortical thickness discriminated TR fractures best whereas femoral head BMD discriminated FN fracture best. Johannesdottir et al ^[38] reported that cortical thickness at the inferior-anterior quadrant of the femoral neck was significantly lower in women with TR fractures than those with FN fractures whereas a significant difference was found in the inferior-posterior quadrant for men. Yang et al ^[40] reported that cortical thickness was important for FN but not for TR fractures in women.

It appeared that the DXA FE performed somewhat better in association studies of hip fracture in women than in men. In a case-cohort sample of the Study of Osteoporotic Fractures, which included the largest number of cases (n=668) and longest follow-up period (mean 12.8 yrs) among similar studies ^[19], we found that the estimated strength had the similar age-BMI-adjusted HR to this study (2.21 vs. 2.37) and was independent of FN BMD with age-BMI-FN BMD-adjusted HR of 1.71 whereas it was not independent in this study. It is interesting to notice that the age-BMI-adjusted HR for FN BMD in the SOF study are much lower than in this study (2.04 vs. 4.33), which seems to suggest that hip fracture in men is more strongly related to FN BMD than in women and therefore more difficult to improve the prediction using DXA FE.

In a case-cohort study of 40 hip fractures and 210 non-cases from the MrOS study (with a mean follow-up period of 5.6 yrs) ^[6], the estimated strength from QCT FE in the control group (5939±1919 N) was significantly higher than this study (4185±982 N), but the strength in the fractured group (3782±1563 N) was similar to this study (3440±828 N), whereas the age-BMI-adjusted hazard ratio was much higher than this study (6.5 v. 2.37). Similar comparison can be made in another QCT FE study of fracture association which included a male hip fracture arm of 63 hip fracture and 377 controls ^[8], the estimated strengths (cases 3860±940, controls 5140±1200 N) were similar to the QCT FE results in MrOS, higher than this study for controls and similar to this study for cases. However, the age-BMI-adjusted odds ratio (OR) for hip fracture due to one SD decrease in the estimated strength was 3.7, much lower than the QCT FE analyses in MrOS and again higher than this study. These difference may be caused in part by the characteristics of participants in the different study cohorts, but certainly by the differences in imaging modalities. Comparing with QCT, the 2D nature of DXA has many inherent limitations in imaging bone geometry, density distribution and structure as discussed in the next paragraph. Multiple-orientation DXA has been tried to address the limitations directly [42]. Several other studies have showed that the 3D information lost in DXA can be partially restored using statistical models based on training QCT scans ^[43–45], and the technique was able to explain 85% of the QCT-estimated femoral strength^[44] and improve fracture discrimination over BMD ^[45]. Combining such statistical models with multiple-orientation DXA may enhance the methodology further. More studies are required to validate the above techniques with prospective fracture cohorts. Further direct comparisons of DXA and QCT in a same cohort are required to compare the above techniques and examine which aspects of bone fragility can be detected by QCT but not by DXA. We would encourage the use of 3D approaches and active shape and appearance models to build on our work with DXA-FE.

Like other DXA-based models, our FE model has inherent limitations related to 2-dimensional DXA scanning that ignores variation of geometry, bone density and loading conditions in the anterior-posterior direction and may have specific limitations for sideway falls in directions different from the DXA projection plane. Since DXA scans project cortical and trabecular bones on top of each other, the material properties of this cortical/trabecular bone mix is difficult to model and may differ from the trabecular bone properties used in this study. We did not consider the different yield stresses of bone in tension and in compression, which may over-estimate the femoral strength since bone is stronger in compression than in tension and the superior-lateral aspect of the femoral neck, where hip fractures usually initiate, is under compression in sideways fall. Since the human proximal femur behaves linear-elastically up to failure ⁽²⁴⁾, we only performed linear elastic analysis without considering post-yield behaviour. We applied fall impact force directly onto the greater trochanter and did not consider the attenuation and diffusion effects of the peri-trochanter soft tissue ^[46]. We also did not consider any muscle forces in our model. We derived the estimated strength intervention threshold from the MrOS cohort that does not include younger men, and large studies with wide age ranges are needed to derive and evaluate the thresholds separately for women and men. In the MrOS study only left hip DXA scans were performed, but we included incident fractures that occurred on both sides. Conflicting results have been reported on the importance of side-differences in hip BMD ^{[47, 48]} and only one study reported a significant yet small intra-subject asymmetry in femoral geometry (mainly in the infero-medial cortex)^[49]. Further studies are required to ascertain side-differences in FE-derived femoral strength and its effects on fracture risk assessment. Hologic QDR 4500 scanners used in the MrOS study were fan-beam scanners, which introduces a magnification problem and we did not correct for it. Due to the study population size, we only adjusted for the strongest determinants of hip fracture risk (age, BMI and BMD) and did not consider other risk factors such as fall history, muscle strength, vitamin D status and etc. It is possible to use an empirical equation relating a patient-specific impact force to BMI or patient height and weight based on dynamics of sideway falls. Such impact force can be used to calculate a load to strength ratio which may improve the fracture risk prediction over the strength alone.

In conclusion, the results of this study suggest that the femoral strength estimated from the FE analysis of DXA scans is an independent risk factor for hip and FN fractures but not TR factures, and provides, in combination to hip BMD, a limited improvement in the predictive ability for FN fracture only.