Establishing the V̇o₂ versus constant-work-rate relationship from ramp-incremental exercise: simple strategies for an unsolved problem

Abstract

The dissociation between constant work rate of O₂ uptake (V̇o₂) and ramp V̇o₂ at a given work rate might be mitigated during slowly increasing ramp protocols. This study characterized the V̇o₂ dynamics in response to five different ramp protocols and constant-work-rate trials at the maximal metabolic steady state (MMSS) to characterize 1) the V̇o₂ gain (G) in the moderate, heavy, and severe domains, 2) the mean response time of V̇o₂ (MRT), and 3) the work rates at lactate threshold (LT) and respiratory compensation point (RCP). Eleven young individuals performed five ramp tests (5, 10, 15, 25, and 30 W/min), four to five time-to-exhaustions for critical power estimation, and two to three constant-work-rate trials for confirmation of the work rate at MMSS. G was greatest during the slowest ramp and progressively decreased with increasing ramp slopes (from ~12 to ~8 ml·min⁻¹·W⁻¹, P < 0.05). The MRT was smallest during the slowest ramp slopes and progressively increased with faster ramp slopes (1 ± 1, 2 ± 1, 5 ± 3, and 10 ± 4, 15 ± 6 W, P < 0.05). After “left shifting” the ramp V̇o₂ by the MRT, the work rate at LT was constant regardless of the ramp slope (~150 W, P > 0.05). The work rate at MMSS was 215 ± 55 W and was similar and highly correlated with the work rate at RCP during the 5 W/min ramp (P > 0.05, r = 0.99; Lin’s concordance coefficient = 0.99; bias = −3 W; root mean square error = 6 W). Findings showed that the dynamics of V̇o₂ (i.e., G) during ramp exercise explain the apparent dichotomy existing with constant-work-rate exercise. When these dynamics are appropriately “resolved”, LT is constant regardless of the ramp slope of choice, and RCP and MMSS display minimal variations between each other.

NEW & NOTEWORTHY This study demonstrates that the dynamics of V̇o₂ during ramp-incremental exercise are dependent on the characteristics of the increments in work rate, such that during slow-incrementing ramp protocols the magnitude of the dissociation between ramp V̇o₂ and constant V̇o₂ at a given work rate is reduced. Accurately accounting for these dynamics ensures correct characterizations of the V̇o₂ kinetics at ramp onset and allows appropriate comparisons between ramp and constant-work-rate exercise-derived indexes of exercise intensity.

INTRODUCTION

With step transitions to work rates within the moderate [below the lactate threshold (LT)]-, heavy [above LT but below the “maximal metabolic steady state” (MMSS)]-, and severe (above MMSS)-exercise-intensity domains, the phase II time constant (τ) characterizing the rate of O₂ uptake (V̇o₂) and total gain (G; i.e., ∆V̇o₂/∆work rate) of the V̇o₂ response become progressively greater, until steady-state V̇o₂ can no longer be attained (i.e., within the severe intensity domain; see Ref. 36). With ramp-incremental exercise, these intensity domain-specific V̇o₂ response characteristics (varying τ and G) yield a “linear” rise in V̇o₂ (22, 38, 44). Knowledge of this phenomenon is important because V̇o₂ is the gold standard of aerobic exercise intensity, and ramp protocols are the primary tool used to determine a work rate that will elicit a target V̇o₂ (42). However, it is often assumed that any specified V̇o₂ can accurately be targeted selecting a work rate by interpolating the ramp V̇o₂ response (12). This supposition, of course, neglects that V̇o₂ lags increasingly the changes in work rate during ramp exercise, particularly at intensities exceeding LT, and that, when performed constantly, any work rate will inevitably elicit a greater V̇o₂ than expected based on the ramp V̇o₂ response (25). Interestingly, ramp V̇o₂ responses computed from varying τ and G have predicted that, compared with faster ramp protocols (i.e., 30 W/min), slow ramp protocols (i.e., ≤15 W/min) would reduce the “gap” between steady-state V̇o₂ and ramp V̇o₂ at any given work rate (22, 44). However, empirical data supporting these hypotheses over a wide range of ramp protocols are still needed.

Irrespective of ramp slope, another factor to consider when establishing the V̇o₂-to-constant-work-rate relationship are the V̇o₂ kinetics at the onset of ramp exercise (4). After ramp onset, the muscle-mouth circulatory transit time and the muscle V̇o₂ kinetics delay the beginning of the linear rise in V̇o₂, such that V̇o₂ is “right shifted,” or offset, from its corresponding work rate (25). To nullify the effect of this time delay, ramp V̇o₂ data are typically aligned with their corresponding work rate by computing and correcting for the mean response time (MRT; see Refs. 4 and 13). For 30 W/min ramp protocols, we have recently shown that the MRT is best calculated by measuring the steady-state V̇o₂ during a 6-min bout of moderate exercise performed before ramp exercise, which, projected on the ramp V̇o₂-to-work rate relationship, provides an accurate estimate of time (s or W) by which to “left shift” the V̇o₂ data (19). Given that G in the moderate domain should be greater in response to slower ramp protocols (22, 40, 44), it is hypothesized that the difference in seconds between the ramp and constant-work-rate V̇o₂ (i.e., the MRT) would be reduced progressively from faster to slower ramp protocols, necessitating less of a correction to achieve the same work rate at LT across different ramp slopes.

Finally, a disadvantage of traditional ramp protocols that is yet to be overcome is that V̇o₂ and work rate associated with MMSS cannot be identified (25). The V̇o₂ at the respiratory compensation point (RCP) is consistently similar to that at MMSS (16, 17, 23, 39), but the high variability in work rate between these two markers has led to the interpretation that the RCP is not a suitable surrogate (7, 9, 11, 30). This interpretation, however, does not consider that the work rate at RCP is a function of the characteristics of the ramp slope (25, 26, 30, 39). If MMSS and RCP represent the same physiological event, then strategies that 1) provide sufficient time for the V̇o₂ control system to develop its kinetics during ramp exercise and 2) apply accurate “left shifting” of the V̇o₂ data should result in similar work rates at MMSS and RCP that are less variable than previously reported (30).

Therefore, the present study characterized the V̇o₂ response to five ramp protocols of varying slope (5, 10, 15, 25, and 30 W/min increments). Thereafter, the discrepancy between constant-work rate and ramp work rate at a given moderate V̇o₂ (i.e., MRT) was determined to identify and compare between ramp protocols the work rates corresponding to LT and RCP. It was hypothesized that the dissociation between V̇o₂ and work rate during ramp exercise would be explained by the characteristics of work rate increase (i.e., greater ramp slopes would result in greater dissociations between the V̇o₂ response and its corresponding work rate) and would be reduced, or even abolished, with slower ramp protocols. Based on this idea, it was also hypothesized that the work rate at RCP and MMSS would be the same when using the slowest ramp protocols.

METHODS

Participants

Eleven recreationally trained participants (6 men and 5 women; mean ± SD, age = 28 ± 7 yr; height = 174 ± 9 cm; weight = 66 ± 8 kg) completed the study. Participants were not undergoing any medical treatment that could potentially alter their cardiorespiratory and metabolic responses to exercise. All participants signed an informed consent, and all procedures were approved by the Conjoint Health Research Ethics Board of the University of Calgary in compliance with the latest version of the Declaration of Helsinki.

Experimental Protocol

The study encompassed the following three phases: 1) five ramp-incremental tests to exhaustion, 2) four to five time-to-exhaustion trials for critical power (CP) estimation, and 3) two to three constant-work-rate trials to measure/confirm the physiological variables elicited by the work rate at the estimated CP. All testing sessions were performed on an electromagnetically braked cycle ergometer (Velotron; RacerMate, Seattle, WA). Participants performed the tests on separate days, with 6 ± 1 day interval between the ramp tests and a minimum of 48-h and a maximum of 72-h interval between the time-to-exhaustions and between the constant-work-rate trials. All testing sessions were performed at the same time of the day (±30 min). The preferred cadence was self-selected by the participants during their first laboratory visit, recorded, and maintained the same for subsequent visits. During both ramp and time-to-exhaustion tests, the tests were terminated when participants could no longer maintain the self-selected cadence by 10 rpm for more than 10 s or at volitional exhaustion despite strong verbal encouragement. In all conditions, participants were blinded to the work rate and elapsed time but received visual feedback on their cadence.

Ramp tests.

The ramp slopes were 5, 10, 15, 25, and 30 W/min increment rates and were initiated from a 50-W baseline cycling of 4 min. The 25 W/min ramp was always performed first; thereafter, the remaining ramp tests were performed in a randomized order. Each ramp test was preceded by a step-transition protocol within the moderate-intensity domain. This transition, which consisted of 6 min at 20 W followed by 6 min at 100 W, served the purpose of calculating the ramp MRT of V̇o₂, as previously described (19; also see Data Analyses). The use of a 100-W fixed work rate for the moderate-intensity transition in this study was based on the prediction and subsequent confirmation that this work rate would be large enough to increase the amplitude without exceeding the work rate at LT.

Time-to-exhaustion trials.

For the estimation of CP through the power-duration relationship, the work rates for the four to five time-to-exhaustion trials were selected to elicit exhaustion times between ~1.5 and 20 min, with the objective of generating an even distribution curve between the trials (32, 35). With this logic, percent work rate ranging from 70 to 110% of the peak work rate achieved during the 25 W/min were chosen (33). The order of these trials was randomized, and each consisted of a 4-min baseline cycling at 50 W, which was followed by the square-wave transition to the predetermined work rate.

Constant-work-rate trials.

Participants performed additional constant-work-rate trials at the estimated CP of 30 min of duration, or to exhaustion (whichever occurred earlier), to measure steady-state physiological responses [stability of V̇o₂ and blood lactate concentration ([La⁻]_b)] such that attainment of true maximal metabolic steady state could be confirmed. In circumstances where participants could not complete the 30 min of exercise, or V̇o₂ and/or [La⁻]_b were unstable, another trial was performed at a lower work rate (−10 W). Vice versa, if V̇o₂ and [La⁻]_b were stable at CP, the following ride was performed at a higher work rate (+10 W). A steady state was established as no further appreciable change in V̇o₂ (≤100 mL/min; see Ref. 24) in concomitance with stable [La⁻]_b responses (≤1 mM; see Ref. 14) after 15 min within the trial (between minutes 15 and 30). [La⁻]_b measurements were taken at baseline and every 5 min after the work rate was instantaneously increased to the predetermined work rate. At “sensitive” time points (15, 30 min), [La⁻]_b measures were taken in triplicate, and the average of the two closest measures was used for further analysis.

Data Collection

Gas exchange and ventilatory variables were measured breath-by-breath with a metabolic cart (CPET; Cosmed, Rome, Italy). The system consisted of a low-dead-space turbine and O₂ and CO₂ gas analyzers; these were calibrated with a syringe of known volume (3 L) and a gas mixture of known concentration (16% O₂-5% CO₂-balance N₂), respectively. Capillary blood samples were drawn from a finger prick and immediately analyzed for [La⁻]_b (Biosen C-Line; EKF Diagnostics, Barleben, Germany).

Data Analyses

The parameters CP and the amount of work in joules that can be done above CP (W') were estimated using the three-parameter hyperbolic model using nonlinear least-squares regression analysis (35), as follows:

$$t=\text{W}\prime /\left(\text{P}-\text{CP}\right)+\text{W}\prime /\left(\text{CP}-{\text{P}}_{\text{max}}\right)$$

where t is time to exhaustion (s), CP is the critical power in watts, and P_max is the maximal “instantaneous” power. The “goodness of fit” for the hyperbolic model was determined as the 95% confidence interval for CP. V̇o₂ responses during the constant-work-rate 30-min trials at CP were averaged into 2-min bins so that responses across time could be examined. The V̇o₂ corresponding to MMSS was calculated as the average of the last 5 min of the 30-min trial.

The raw ventilatory and gas exchange data for each participant were scrutinized by two experts to identify the V̇o₂ corresponding to LT and RCP. In the circumstances of a disagreement of >100 mL/min, the experimenters reevaluated together the profiles until an agreement was reached. Briefly, the LT was estimated by identifying the point at which V̇co₂ began to increase out of proportion in relation to V̇o₂, coincidental with a systematic rise in the V̇_E-to-V̇o₂ relation and end-tidal Po₂, whereas the ventilatory equivalent of V̇co₂ (V̇_E/V̇co₂) and end-tidal Pco₂ were stable (2). RCP was identified as the point corresponding to the systemic fall in end-tidal Pco₂ after a period of isocapnic buffering (43). This point was confirmed by examining the V̇_E/V̇co₂ and V̇_E/V̇o₂ relationships as well as by identifying the second breakpoint in the V̇_E-to-V̇o₂ relation.

V̇o₂ data during the moderate-step-transition, ramp, time-to-exhaustion, and constant-work-rate trials were edited on an individual basis by removing aberrant data that lay 3 SD from the local mean (29). Subsequently, all trials were interpolated on a second-by-second basis, with time 0 representing the onset of the step transitions and/or ramp portion of the trials.

The MRT of V̇o₂ for each ramp exercise trial was calculated, as previously described (19). Briefly, a linear fit was used to fit the ramp V̇o₂ data from the onset of its systematic increase up to the previously established LT. Next, the average V̇o₂ of the last 2 min of the moderate step transition was used to linearly identify the corresponding work rate during the ramp exercise. The difference in watts between the work rate of the moderate step transition and the identified ramp work rate at the common V̇o₂ corresponded to the steady-state MRT (MRT_SS) and was used to align the ramp V̇o₂ with the corresponding ramp work rate (19). Thereafter, the work rates at LT and RCP during each ramp protocol (RCP₅, RCP₁₀, RCP₁₅, RCP₂₅, and RCP₃₀) were linearly identified from the aligned V̇o₂-to-work-rate profiles.

Additionally, the MRT was also calculated with the commonly used “back-extrapolation” technique (5). Briefly, a piecewise equation that included two linear segments was used:

$$f=\text{if\hspace{0.17em}}\left[t<\text{\hspace{0.17em}MRT\hspace{0.17em}use\hspace{0.17em}}g\left(t\right),\text{else\hspace{0.17em}}h\left(t\right)\right];\hspace{0.17em}g\left(t\right)=i_1+s_{1}t;i_2=i_1+s_{1}t;h\left(t\right)=i_2+s_{2}t–\text{MRT}$$

where f is the piecewise function, t is time, and g and h are V̇o₂, MRT is the time corresponding to the intersection of the two regression lines (MRT_LIN), i₁ and i₂ are the intercepts of the first and second linear function, respectively, and s₁ and s₂ are the slopes. The s₁ parameter was fixed at “zero.” The two linear segments were fitted from the last 3 min of the preramp baseline (t = −180 s) up to the LT (25).

After averaging the V̇o₂ data in response to the different ramp protocols in 5-s bins, each V̇o₂ profile was partitioned in three domains of intensity demarcated by the previously established V̇o₂ at LT and MMSS. Thereafter, G during each ramp exercise was calculated in the moderate (G_MOD, below LT), heavy (G_HVY, between LT and MMSS), and severe (G_SVR, above MMSS) domains and expressed as milliliters per minute per watt (ml·min^–1·W^–1). A linear-regression fit of the V̇o₂ responses as a function of work rate was used to compute G in each domain. In the moderate-intensity domain, the linear fit was initiated after the lag phase of the V̇o₂ (computed from the back-extrapolation approach previously described), whereas in the severe domain the linear fit was terminated before the (potential) onset of the plateau in V̇o₂ (identified as any data points falling below and outside the extrapolated 95% confidence interval of the liner regression fit; see Ref. 37). All data editing and fitting were performed with customized functions of a commercially available statistical software package (Origin; OriginLab, Northampton, MA).

Statistical Analysis

Data are presented as means ± SD. Repeated-measures ANOVA were performed to detect possible differences in response to the different ramp protocols for: peak work rate, V̇o_2max, G (within and between ramp protocols and domains), MRT_SS, MRT_LIN, LT, and RCP (with either one approach for left shifting). Repeated-measures ANOVA were also performed to compare the V̇o₂ at the RCP values and at MMSS and to compare the work rate at the RCP, MMSS, and CP. Bland-Altman plots were performed to calculate the agreement (e.g., bias and limits of agreement) between markers of intensity. Pearson’s index, root mean square error (RMSE), and Lin’s concordance coefficient (CCC) were used to calculate correlation, typical error, and concordance, respectively, between the intensity markers. As previously described (30), the CCC was interpreted as follow: almost perfect agreement = CCC > 0.99, substantial agreement = 0.95 > CCC < 0.99, moderate agreement = 0.90 > CCC < 0.95, and poor agreement = CCC < 0.90 (34). Where appropriate, a post hoc analysis was performed. Statistical significance was set at a α value of <0.05. All statistics were performed using SPSS version 23 (SPSS; IBM, Chicago, IL).

RESULTS

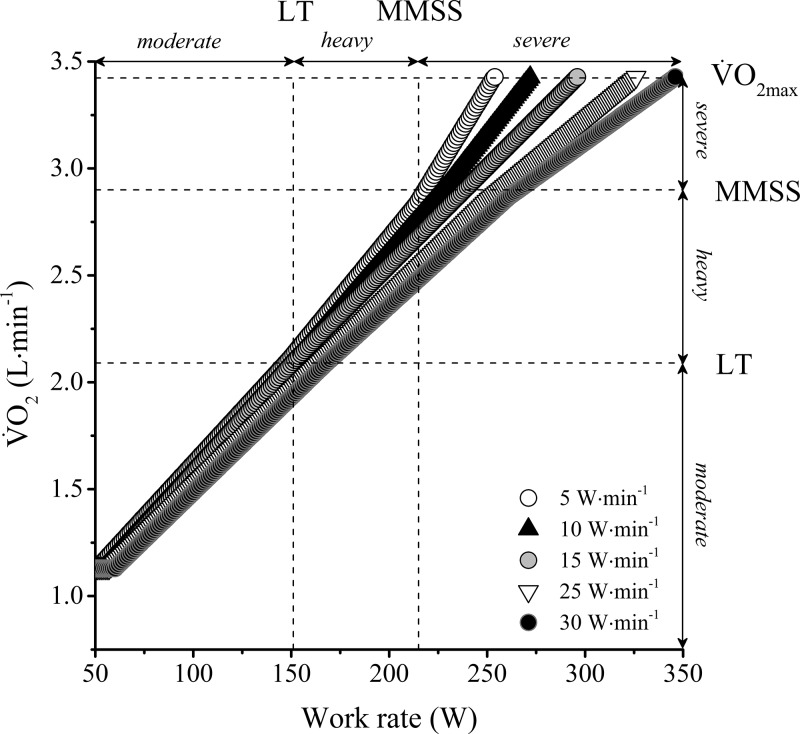
While peak work rate progressively increased (P < 0.05), V̇o_2max was not different with increasing ramp slopes (P > 0.05, Table 1). G was progressively smaller with increasing ramp slopes regardless of the exercise intensity domain (for locus of significant differences see Table 1). Figure 1 displays the average G for each ramp protocol modeled within each intensity domain as function of work rate.

Table 1.

Peak and submaximal physiological parameters and responses of aerobic performance during different ramp protocols

	Ramp Slope, W/min
	5	10	15	25	30	CV
Peak work rate, W	262 ± 55§	291 ± 59§	310 ± 63§	340 ± 66§	353 ± 69§
V̇o2max, L/min	3.35 ± 0.68	3.44 ± 0.67	3.44 ± 0.69	3.44 ± 0.74	3.44 ± 0.72	0.02 ± 0.01
[La−]b, mM	10.6 ± 2.3	11.2 ± 1.9	11.9 ± 2.4	12.4 ± 2.7	12.5 ± 2.0	0.20 ± 0.03
LT
L/min	2.10 ± 0.36	2.08 ± 0.33	2.09 ± 0.35	2.10 ± 0.33	2.10 ± 0.36	0.03 ± 0.02
W	147 ± 27	150 ± 31	150 ± 34	155 ± 29	152 ± 33	0.07 ± 0.02
RCP
L/min	2.83 ± 0.65	2.84 ± 0.59	2.82 ± 0.61	2.86 ± 0.60	2.86 ± 0.61	0.02 ± 0.01
W	212 ± 54§	221 ± 53§	231 ± 55§	242 ± 56§	247 ± 58§
GMOD, ml·min−1·W−1	10.0 ± 1.a	9.9 ± 0.6b	10.1 ± 0.9	9.0 ± 0.7^	8.9 ± 1.3b,^
GHVY, ml·min−1·W−1	11.2 ± 1.2a	10.6 ± 1.6	9.2 ± 1.2*	8.8 ± 0.9*,#	8.3 ± 1.4c,*,#
GSVR, ml·min−1·W−1	15.0 ± 3.9a	11.8 ± 1.9	9.2 ± 1.7†	7.6 ± 2.0†	6.6 ± 1.5†,‡

Data are expressed as means ± SD. CV, coefficient of variation; V̇o_2max, maximal rate of O₂ uptake; [La⁻]_b, blood lactate concentration; LT, lactate threshold; RCP, respiratory compensation point; G_MOD, rate of O₂ uptake (V̇o₂) gain in the moderate domain; G_HVY, V̇o₂ gain in the heavy domain; G_SVR, V̇o₂ gain in the severe domain.

^§Significant differences across all ramp protocols.

^aSignificant differences within the same domain.

^bSignificant difference between G_MOD and G_SVR.

^cSignificant difference between G_HVY and G_SVR.

^{^}Significant differences from 10 and 15 W/min ramp.

^*Significant differences from 5 W/min ramp.

^#Significant differences from 10 W/min ramp.

^†Significant differences from 5 and 10 W/min ramp.

^‡Significant differences from 15 W/min ramp.

Fig. 1.

Functional gain of the rate of O₂ uptake (V̇o₂) as a function of work rate during each ramp protocol. Lactate threshold (LT) and maximal metabolic steady state (MMSS) demarcate the moderate-, heavy-, and severe-intensity domains. Of note are the “upward” and “downward” curvilinear increases occurring with slower and faster ramp protocols, respectively.

The MRT_SS of V̇o₂ expressed in seconds and watts (computed from the moderate step transitions preceding the ramp protocols) in response to 5, 10, 15, 25, and 30 W/min ramp tests was 7 ± 6, 14 ± 9, 21 ± 12, 24 ± 10, and 29 ± 11 s and 1 ± 1, 2 ± 1, 5 ± 3, 10 ± 4, and 15 ± 6 W, respectively, and these were all different from each other (P < 0.05, Fig. 2). The MRT_LIN (computed from the backextrapolation approach) of V̇o₂ expressed in seconds in response to 5, 10, 15, 25, and 30 W/min ramp tests was 38 ± 44, 69 ± 50, 42 ± 23, 18 ± 14, and 21 ± 11 s, and these were not different from each other (P > 0.05). When expressed on the basis of work rate, MRT_LIN values (from the slowest to the fastest ramp slope) were 3 ± 4, 12 ± 8, 11 ± 6, 8 ± 6, and 11 ± 5 W. The MRT_LIN from the 5 W/min ramp was different from all the others (P < 0.05), with the remaining values not different from each other (P < 0.05).

Fig. 2.

Steady-state mean response time (MRT_SS) of rate of O₂ uptake (V̇o₂) in response to each ramp protocol. *Significant from the previous symbol(s).

The LT and RCP (on the basis of both V̇o₂ and work rate) are shown in Table 1. There was no difference across the ramp exercise protocols for the V̇o₂ at either LT or RCP (P > 0.05). However, in response to the different ramp slopes and after left shifting the ramp V̇o₂ data (with MRT_SS), whereas the work rate associated with the estimated LT was not different (P > 0.05), the work rate at RCP progressively increased with faster ramp slopes (P < 0.05, Fig. 3, A and B). When using MRT_LIN for left shifting the ramp V̇o₂, the work rate at the LT was greater during 25 and 30 W/min compared with the other ramp protocols (P < 0.05).

Fig. 3.

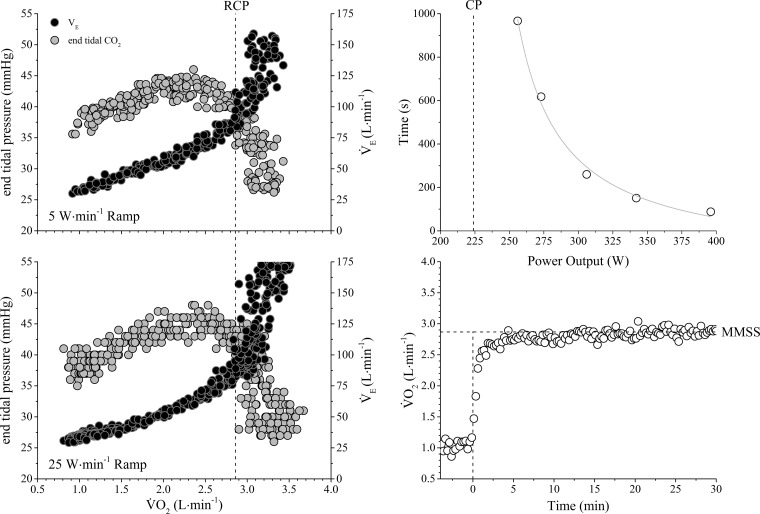
Gas exchange and ventilatory profiles during 5 and 25 W/min ramp protocols, power duration relationship for critical power (CP) estimation, and rate of O₂ uptake (V̇o₂) response during 30-min constant-work-rate trial at CP in a representative subject. V̇e, minute ventilation; RCP, respiratory compensation point. For clarity, the data from the 5 W/min ramp protocols were averaged into 5-s bins.

Time-to-exhaustion during the severe domain trials for CP estimation were, on average (from the shortest to the longest), 1.8 ± 0.8, 3.5 ± 1.5, 6.7 ± 1.2, 11.6 ± 1.5, and 16.2 ± 2.1 min (the longest trial was required in seven participants) and corresponded, on average, to 110 ± 3, 93 ± 3, 82 ± 10, 75 ± 5, and 71 ± 5% peak work rate of the 25 W/min ramp. The group mean average of the CP and W' parameter estimates from the hyperbolic model were 214 ± 59 W and 32.3 ± 12.5 kJ, respectively. The R² and the standard error of the estimate were 0.99 ± 0.01 and 6.3 ± 5.0 W, respectively. In three participants, metabolic stability was possible at work rates greater than the one predicted by CP. One participant could not complete the 30-min trial at CP. In this participant, and in two others who were able to complete the trial, end-trial V̇o₂ was within 95% of V̇o_2max, and [La⁻]_b values were similar to those recorded at the end of the ramp tests. In the remaining five participants, the work rate at the predicted CP conformed to MMSS. There was no difference between the work rate at CP and the confirmed work rate at MMSS (215 ± 55 W), which corresponded to 63 ± 5% of peak work rate recorded at the end of the 25 W/min ramp. The group average of V̇o₂ at the end of the 30-min trial at MMSS was 2.90 ± 0.67 (L/min), which corresponded to 81.9 ± 5.9% of V̇o_2max, and was not different from any of the V̇o₂ values at RCP measured during the different ramp protocols (range bias = 0.03–0.07 L/min; P > 0.05) and highly correlated to the V̇o₂ values at RCP (range r = 0.987–0.990; P < 0.05). Group average [La⁻]_b values at the end of the 30-min trial at MMSS were 5.6 ± 2.0 mM. The end-trial group average V̇o₂ and [La⁻]_b values at 10 W above MMSS were 3.17 ± 0.69 (L/min), which corresponded to 91.9 ± 4.9% of V̇o_2max, and 8.2 ± 2.4 mM, respectively. Figure 3 depicts the profiles of end-tidal pressure of CO₂ (mmHg) and V̇e (L/min) during the 5 and 25 W/min ramp test, power-duration relationship for CP estimation, and V̇o₂ response during the constant-work-rate trial at the estimated CP for a representative participant.

Table 2 displays the comparison between the work rate at MMSS and at CP and RCP from each ramp protocol. The agreement was nearly perfect between MMSS and RCP₅ (P > 0.05). This comparison was characterized by small bias, limits of agreement, and RMSE and high concordance (Fig. 4, C and D). With faster ramp slopes, this agreement worsened, and the work rate at MMSS was lower than RCP in all circumstances (P < 0.05). The comparison between estimated CP and RCP showed that both RCP₅ and RCP₁₀ are not different from estimated CP (P > 0.05). However, these comparisons were characterized by large limits of agreement and RMSE. With increasing ramp slopes, then, the agreement between estimated CP and RCP worsened, with significant differences detected between the two indexes (P < 0.05).

Table 2.

Comparison on the basis of work rate between MMSS and RCP (corrected by MRT_SS) and between CP and RCP (corrected by MRT_LIN)

	Ramp Slope, W/min
	5	10	15	25	30
MMSS vs. RCP (corrected by MRTSS)
Bias, W	−3 (−14 to 9)	6*# (−12 to 25)	16*# (−4 to 36)	28*# (9–47)	32*# (10–55)
r	0.99	0.99	0.98	0.98	0.98
RMSE, W	6	11	19	29	34
CCC	0.99 (0.97–1.00)	0.98 (0.92–0.99)	0.94 (0.83–0.98)	0.87 (0.69–0.95)	0.83 (0.62–0.93)
CP versus RCP (corrected by MRTLIN)
Bias, W	−6 (−36 to 23)	−4 (−46 to 39)	10* (−23 to 43)	29*# (−7 to 65)	35*# (0–71)
r	0.97	0.93	0.96	0.95	0.95
RMSE, W	16	21	19	34	39
CCC	0.96 (0.86–0.99)	0.92 (0.75–0.98)	0.94 (0.81–0.98)	0.83 (0.59–0.94)	0.80 (0.54–0.92)

Bias is calculated from the Bland&Altman plot (lower and upper limits of agreement). MMSS, maximal metabolic steady state; RCP, respiratory compensation point; MRT, mean response time of V̇o₂ corresponding to the intersection of the two regression lines (LIN) and at steady state (SS); r, Pearson’s coefficient of correlation; RMSE, typical root mean square error between two indexes; CCC, Lin’s concordance correlation coefficient (95% confidence interval); CP, critical power.

^*Bias is different from 0.

^#Significant difference between MMSS or CP from RCP (P < 0.05).

Fig. 4.

Rate of O₂ uptake (V̇o₂, L/min) and work rate (W) (A and B, respectively) at the lactate threshold (LT) and respiratory compensation point (RCP). Broken line represents the aggregated V̇o₂ and/or work rate at LT and the average V̇o₂ and/or work rate at the maximal metabolic steady state (MMSS). C and D: correlation and bias (with limits of agreement), respectively, between the work rate at RCP during the 5 W/min ramp protocol (RCP₅) and MMSS.

DISCUSSION

This study demonstrated that the dissociation between constant V̇o₂ and ramp V̇o₂ at a given work rate is progressively reduced (or abolished) with slower ramp protocols. The implications are threefold: 1) the V̇o₂ data in response to ramp exercise need to be “left shifted” by less watts (or seconds) when performing slow compared with fast ramp protocols, 2) a moderate step transition performed before the ramp test ensures appropriate left shifting of the V̇o₂ response, regardless of the ramp slope such that the work rate at LT is constant, and 3) the discrepancy between the work rates at ramp-identified RCP and constant-work-rate-identified MMSS disappears if MRT-corrected slow ramp protocols are used.

V̇o₂ in Response to Ramp Exercise Using Different Slopes

Compared with the 15 W/min ramp protocol, the overall V̇o₂ versus work rate response reflected “upward” and “downward” curvilinear increases during the slowest (i.e., 5 and 10 W/min ramps) and the fastest (i.e., 25 and 30 W/min ramps) ramp protocols, respectively (see Fig. 1). In line with previous investigations (22, 40, 44), total G was greatest with the slowest ramp protocol (i.e., 5 W/min) and progressively decreased as ramp slope increased. Nonlinear dynamics of V̇o₂ (1, 27, 41) were most evident during the 5 and 10 W/min ramp protocols (see Fig. 1), where G increased from ~10 (G_MOD) to ~15 ml·min⁻¹·W⁻¹ (G_HVY). Compared with G_MOD and G_HVY, G_SVR was greater with the slowest ramp protocols (i.e., 5 and 10 W/min ramps), not different (~10 ml·min⁻¹·W⁻¹) with the 15 W/min ramp protocols, and smaller (~7 ml·min⁻¹·W⁻¹) during the 25 and 30 W/min ramp protocols. Although other mechanisms have also been proposed (28), these different V̇o₂ dynamics during ramp exercise may be attributed to the notion that, with increasing work rate, motor units characterized by slower V̇o₂ kinetics and lower efficiency are progressively recruited to synthesize energy from O₂ (6). Therefore, while during slow ramp protocols the newly recruited motor units are provided more time to dynamically adjust to the change in work rate and contribute to the observable increase in V̇o₂ gain, the reverse occurs during faster ramp protocols (22, 44).

The result of these different V̇o₂ dynamics is that, with slower ramp protocols, the magnitude of the dissociation between constant V̇o₂ and ramp V̇o₂ at a given work rate (i.e., 100 W) was reduced, such that the MRT_SS became progressively smaller; thus, a progressively smaller number of watts was required to left shift the V̇o₂ response. Furthermore, the work rate at LT was not different and varied minimally among ramp slopes, lending further support to the use of a “step-ramp” protocol for accurate and valid quantification of MRT. As discussed elsewhere (19), this approach is unaffected by between-day variability in the V̇o₂ parameters or noise associated with the breath-by-breath data. More susceptible to these factors, however, is the back-extrapolation approach (4, 15, 31). Moreover, an “artificial” prolongation of the MRT can be seen with slower ramp protocols when using this method. Indeed, MRT_LIN was greater on average with slower (5, 10, and 15 W/min) compared with faster ramp protocols likely because G_MOD increases (Table 1 and Refs. 15 and 19) and breath-by-breath noise at baseline reduces certainty of detecting a rise in V̇o₂ following ramp onset (5, 24, 40). For these reasons, estimations of the work rate at LT based on MRT_LIN-corrected ramp data were not uniform (smaller with slower ramps) but were identical when a MRT_SS correction was applied (see Fig. 3B).

Transitioning from the Heavy to the Severe Domain

The V̇o₂ at RCP was constant regardless of the ramp protocol adopted and was not different from the V̇o₂ at MMSS (Fig. 3, C and D), corroborating the observation that the RCP is an invariant parameter of the ramp exercise (39). Although the characteristics of the hyperventilatory compensatory response can be modified by the ramp slope of choice, the overall dynamics of the gas exchange and ventilatory patterns are typically unaltered (39), with the RCP occurring at very similar V̇o₂ regardless of the ramp protocol (30, 39). This is because the range of intensities (i.e., metabolic rates) at which oxidative processes can account for most of the energy required by the task is constant regardless of the forcing function. During exercise sustained above this “critical metabolic rate,” the need to buffer the accumulation of hydrogen concentration originating from an accelerated reliance on substrate-level phosphorylation initiates a potent compensatory hyperventilation. That the V̇o₂ at RCP is similar to the V̇o₂ at MMSS is consistent with previous studies (16, 17, 23). For example, it was demonstrated that the V̇o₂ associated with these markers changed concordantly in well-trained cyclists over the course of a competitive season (21).

In addition to this, also the work rate at RCP during the 5 W/min ramp protocol (after left shifting) was similar and highly concordant with the work rate at MMSS (Fig. 3B). This close agreement shows that the variability among these indexes is no longer present when the V̇o₂ dynamics during ramp exercise are appropriately considered and reinforces the idea that the V̇o₂ at RCP represents the metabolic rate associated with the transition from heavy- to severe-intensity exercise during ramp exercise (20, 23). These findings are in contrast to Leo et al. (30) who concluded that the RCP should not be used to separate the heavy from the severe domain based on their observation of a high variability between the work rates at RCP and CP. Although we agree with their conclusion that, in most instances the work rate at the RCP should not be used to demarcate the heavy to severe boundary, it is important to consider that this study found a near perfect agreement between the work rates at MMSS and RCP with the MRT_SS-corrected 5 W/min ramp protocol (CCC > 0.99). It is reasoned that these robust findings stem from 1) an accurate estimation of the MRT and 2) an accurate prediction and, subsequently, confirmation of metabolic stability at the work rate estimated by the CP model. For example, the concordance between RCP₅ and MMSS is nearly perfect when RCP₅ is corrected by MRT_SS, but more variable when RCP₅ is corrected by MRT_LIN and the work rate at the estimated CP is accepted without physiological confirmation (see Table 2). Thus, these data provide strong evidence that the RCP may be used as a surrogate of the heavy-severe boundary, provided that slow ramp protocols (i.e., 5 W/min) are performed and the V̇o₂ dynamics at ramp onset are accurately quantified.

Implications for Exercise Intensity Prescription

The ramp-incremental test has become the gold standard protocol to quantify important parameters of aerobic function that are linked to performance and health (10, 42). Erroneously, however, this test has been also used to select the intensity, through linear interpolation of the V̇o₂-work rate relationship, for constant-work-rate exercise (12, 25). This practice, by failing to recognize the nonlinear and domain-specific dynamics of V̇o₂ that are obscured during a rapidly incremented ramp exercise (e.g., 25–30 W/min; see Refs. 22 and 44), inevitably leads to inaccurate prescriptions of exercise intensity (18). In this context, the findings of the present study have important implications for the use and the interpretation of ramp-derived data. 1) Neglecting to or inaccurately left shifting ramp V̇o₂ data jeopardizes the accurate identification of the work rate associated with LT and incorrectly establishes the boundary between moderate and heavy domains, with this having important implications for metabolic responses and exercise performance (3). The adoption of the “step-ramp” method to quantify the MRT (i.e., MRT_SS; see Ref. 19) appears to be a simple strategy to ensure the correct identification of the work rate at LT. 2) After correctly accounting for the MRT of V̇o₂, a dissociation between constant V̇o₂ and ramp V̇o₂ at a given work rate remains above LT, unless the 5 W/min ramp protocol is used. If the goal of the ramp exercise is accuracy in the exercise prescription, the 5 W/min ramp protocol could be used to simultaneously determine within a single visit 1) the V̇o₂-to-work rate relationship across the intensity spectrum; 2) the V̇o₂ and work rates not only at LT but also at MMSS (via RCP detection), thus determining the exercise intensity domains; and 3) V̇o_2max. However, the potential length of this ramp protocol (e.g., ~1 h in relatively trained individuals) may hinder the ability to obtain a true V̇o_2max measure in all individuals (8) and might not be well suited for all populations.

Conclusions

The dynamics of V̇o₂ kinetics of ramp exercise are affected by ramp slope. This study provides mechanistic and practical explanations for the issues related to the interpretation of ramp-derived data. With an accurate quantification of MRT and confirmation of maximal metabolic steady state, the work rate at LT is constant regardless of the ramp slope selected, and the variability between RCP and MMSS greatly reduced, respectively. Furthermore, the long-standing problem of identifying from ramp exercise a work rate that will elicit a target V̇o₂ within the heavy intensity domain can be solved by performing a 5 W/min ramp protocol and identifying RCP. Exercise prescriptions derived from ramp-incremental data should carefully consider the specific characteristics of the dynamics of V̇o₂ in response to the ramp protocol of choice.

GRANTS

This study was supported by National Science and Engineering Research Council of Canada (RGPIN-2016-03698) and the Heart & Stroke Foundation of Canada (1047725).

DISCLOSURES

No conflicts of interest, financial or otherwise, are declared by the authors.

AUTHOR CONTRIBUTIONS

D.I., D.A.K., and J.M.M. conceived and designed research; D.I. and R.d.A.A. performed experiments; D.I. analyzed data; D.I., R.d.A.A., D.A.K., and J.M.M. interpreted results of experiments; D.I. prepared figures; D.I. drafted manuscript; D.I., R.d.A.A., D.A.K., and J.M.M. edited and revised manuscript; D.I., R.d.A.A., D.A.K., and J.M.M. approved final version of manuscript.