Abstract
Aim
To determine the Minimal Clinically Important Difference (MCID) for Constant-Murley score (CMS), University of California Los Angeles (UCLA) shoulder score, and Oxford Shoulder Scores (OSS) after arthroscopic rotator cuff (RC) repairs.
Material and methods
306 patients were followed up for 24-month. MCID for CMS, UCLA and OSS were determined using simple linear regression according to patient satisfaction and expectation fulfilment.
Results
The following MCID were identified:
12-month: CMS 6.7, UCLA 3.0, OSS 3.3.
24-month: CMS 6.3, UCLA 2.9, OSS 2.7.
Conclusions
The various MCID could assist in meaningful interpretation of these scores and to power future comparative studies.
Level of evidence
III.
Keywords: Minimal clinically important difference, Oxford shoulder score, Constant murley score, University of California los Angeles shoulder score, Arthroscopy, Rotator cuff
1. Introduction
Arthroscopic rotator cuff (RC) repair is an effective and cost-efficient treatment option for patients with painful and debilitating RC tears.1 Its success rate is also reproducible across a wide range of patient demographics.2
Patient Reported Outcome Measures (PROMs) are important tools for clinicians to quantify, measure, and determine the success of surgical procedures. Furthermore, outcome measures play an important role in today's evidence-based medicine to serve as a standardized outcome that comparative studies can be based on.
While the changes in PROMs are useful for monitoring treatment outcomes, one of the key challenges is determining the significance of any differences observed. Over the recent years, there has been a shift in interest from pathophysiological measurements to measuring patient-perceived health. While change in scores might be statistically significant, this might not necessarily translate to clinical significance in terms of patient outcomes. To draw clinically meaningful interpretation of the changes in measures, the Minimal Clinically Important Difference (MCID) needs to be define. First described by Jaeschke et al., MCID was defined as “the smallest difference in score in the domain of interest which patients perceive as beneficial and which would mandate, in the absence of troublesome side effects and excessive cost, a change in patient's management”.3 This is the minimal change in a scoring measure that would be perceived by a patient to be beneficial or harmful.
The concept of MCID has been gaining increasing attention in the recent years and used in various literatures measuring patient outcomes to quantify the observed change in PROMs.4,5 Various MCID specific to each Orthopaedic procedure has also been developed and reported in recent literatures.6, 7, 8, 9, 10, 11 The Oxford Shoulder Score (OSS), Constant-Murley score (CMS), and University of California Los Angeles (UCLA) shoulder score are widely used for the evaluation of functional and quality of life outcome after arthroscopic rotator cuff repair with good reliability and validity.12, 13, 14 However, there is currently a paucity of literature investigating the MCID of these PROMs after arthroscopic RC repair.
Therefore, the purpose of this study is to determine the various MCID for OSS, CMS, and UCLA shoulder score after arthroscopic RC repairs. The authors hypothesize that OSS, CMS, and UCLA are responsive measures of arthroscopic RC repair outcomes and valid MCID values could be determined for each of these scores.
2. Material and Methods
This is a retrospective study with prospectively collected data. Between 2010 and 2015, the records of 327 patients with partial or full thickness supraspinatus tear who underwent unilateral arthroscopic rotator cuff repair with subacromial decompression surgery by a single fellowship trained shoulder surgeon were extracted from the authors’ institutional joint registry. Patients with bilateral RC repairs, foreign patients who returned to their home country for further follow up, and those who refused additional follow up assessment by physiotherapist were excluded. All patients sustained symptomatic rotator cuff tears proven with either ultrasound or Magnetic Resonance Imaging (MRI), and is deemed unsuitable or have failed conservative treatment.
This study was approved by the hospital's ethics committee. The patients were prospectively followed up for 24 months. They were assessed by an independent senior principal physiotherapist working in the institution's joint registry pre-operatively and at 12 and 24-month post-operatively for PROMs, patient satisfaction, and expectation fulfilment. Patients with incomplete data at 12-month post-operatively were excluded, leaving a total of 306 patients who were included in the final study. The demographics and mean pre-operatively PROMs of the patients who were assessed was comparable to those lost to follow up.
Functional outcome was assessed by the Constant-Murley score (CMS), University of California Los Angeles (UCLA) shoulder score, and the Oxford Shoulder Score (OSS). All three scores are reliable and responsive instruments in assessing improvements after rotator cuff repair and are the primary outcome measures used in the authors institution for observing treatment response.12, 13, 14 Patients completed each PROMs questionnaire unaided during their follow up visits and their response were recorded by an independent physiotherapist.
2.1. Determining the MCID
There are various methods to calculate MCID. An extensive review by Wells et al.15 classified these methods into nine different approaches. Overall, regardless of the larger framework employed, methods to determine MCIDs can be divided into two categories: distribution-based and anchor-based approaches. Distribution-based approaches compare the change in score to some measure of variability such as standard error of measurement (SEM), standard deviation, or effect size, while anchor-based approaches compare the change in a patient-reported outcome with a second, external measure of change, which serves as the anchor.16,17 Distribution-based approach are limited by their ability to define only a minimal value below which a change in outcome score for a given measure may be due to measurement error, which does not provide information on clinical importance. Thus, it is generally agreed that the anchor-based approach is the optimal way to determine MCID because it directly captures the patients’ preferences and values.16, 17, 18, 19, 20
In an anchor-based approach, comparison can be made between difference groups (“cross-sectional method”) or the same group of patients at different follow-up interval (“longitudinal method”).21 In cross-sectional methods, comparison is made between groups that are different in terms of some disease-related criterion, such as severity class of a particular disease, or external non-disease-related criteria which could give an insight to the severity of the disease. However, cross-sectional comparison is limited by the fact that different groups of patients are likely to differ in many other relevant variables which cannot be controlled. The longitudinal method is one of the most commonly used anchor-based approach in which patient-reported outcome is compared to a global rating of change, such as satisfaction and expectation fulfilment, in the same group of patients at different follow-up time points.
Within the longitudinal method, there also exist many different methods of defining the MCID. Some methods define MICD as the score difference between two adjacent levels of a Likert scale on a global rating, such as between “somewhat satisfied” and “unsatisfied” or “better” and “unchanged”.18,22 Another method is to compare the score difference between every level of patient response7,9 by simple linear regression analysis and using the slope of the line for the minimal change according to a global rating scale. This method allows the MCID to reflect a change in patient perception in each level of response instead of merely between two adjacent levels in a Likert scale that could include 6 or 7 different level of response. This method is most likely to reflect the score change required for a patient to perceive a clinical change in outcome regardless of their standing and is the method the authors have chosen for this study.
2.2. Defining the MCID
An anchor-based approach was used to establish the MCID.16,17 Two anchor questions were used to assess the patient satisfaction and expectation fulfilment and assigned to categorical groups according to their response. For the question on satisfaction with surgery, patient was asked “how would you rate the overall results of your treatment?“, and their response recorded on a 6-point Likert scale (1 = Excellent, 2 = Very good, 3 = Good, 4 = Fair, 5 = Poor, 6 = Terrible). For the question on expectation fulfilment, patient was asked “has the surgery met your expectation so far?“, and their response recorded on a 7-point Likert scale (1 = Yes, totally, 2 = Yes, almost totally, 3 = Yes, quite a bit, 4 = More or less, 5 = No, not quite, 6 = No, far from it, 7 = No, not at all). For this study, the last 2 level of response for satisfaction (“poor” and “terrible”) and expectation fulfilment (“no, far from it” and “no, not at all”) were grouped together for statistical analysis in view of the relatively small percentage of patients in these groups.
2.3. Statistical analysis
Statistical analysis was done in consultation with a biostatistician in the authors' institute. The functional scores at 12-month and 24-month post operation were compared with baseline scores using paired t-test and the mean changes were estimated with 95% confidence interval. The change in scores on the questionnaires were calculated by subtracting patient's 12- and 24-month follow up score from their pre-operative score and were compared across levels of satisfaction and expectation of fulfilment of surgery by Analysis of Variance (ANOVA). The MCID was determined using the slope of the line of simple linear regression analysis with the score changes in the various outcome measures as the dependent variables against patient's satisfaction and expectation fulfilment. Statistical analysis was carried out in consultation with a statistician, using R software version 3.4.2 ((R Core Team (2017). R: A language and environment for statistical computing. R Foundation for Statistical Computing, Vienna, Austria. URL https://www.R-project.org/.) and significance was defined as a p-value ≤ 0.05.
3. Results
A total of 327 patients who underwent arthroscopic rotator cuff repair with subacromial decompression and met the inclusion criteria were included in this study. 306 patients had complete follow up at 12-month and 222 patients had complete follow up at 24-month. The mean age of patients with complete follow up at 12-month and 24-month were 60.2 years (±10.3 years) and 60.3 years (±10.0 years) respectively. There were 139 male and 167 female patients in the 12-month follow up group and 101 male and 121 female patients in the 24-month group. The demographics and mean pre-operatively PROMs of the patients who were assessed was comparable to those lost to follow-up.
The mean CMS, UCLA, and OSS pre-operatively as well as on 12-month and 24-month follow-up are shown in Table 1. Patients experienced significant increase in all three PROMs at 12- and 24-month post-operatively.
Table 1.
Mean CMS, UCLA, OSS.
| Score | Pre-op | 12-month | Change in Score | 95% CI |
p-value* | |
|---|---|---|---|---|---|---|
| Lower | Upper | |||||
| Mean CMS (SD) | 39.8 (19.7) | 67.2 (15.6) | 27.1 | 24.8 | 29.5 | <0.001† |
| Mean UCLA (SD) | 15.4 (5.3) | 28.3 (12.8) | 12.8 | 12.1 | 13.6 | <0.001† |
| Mean OSS (SD) |
28.1 (11.2) |
43 (7.4) |
14.9 |
13.5 |
16.2 |
<0.001† |
| Score | Pre-op | 24-month | Change in Score | 95% CI | p-value* | |
|
Lower |
Upper |
|||||
| Mean CMS (SD) | 40.0 (18.9) | 70.9 (13.8) | 30.9 | 28.0 | 33.8 | <0.001† |
| Mean UCLA (SD) | 15.3 (4.9) | 29.8 (5.2) | 14.6 | 13.7 | 15.5 | <0.001† |
| Mean OSS (SD) | 28.4 (10.9) | 44.2 (7) | 16.0 | 14.4 | 17.6 | <0.001† |
*paired t-test.
†Statistically significant.
CMA, Constant-Murley Score; UCLA, University of California Los Angeles shoulder score; OSS, Oxford Shoulder Score.
3.1. At 12-month follow-up
3.1.1. CMS, UCLA, and OSS with level of satisfaction and expectation fulfilment
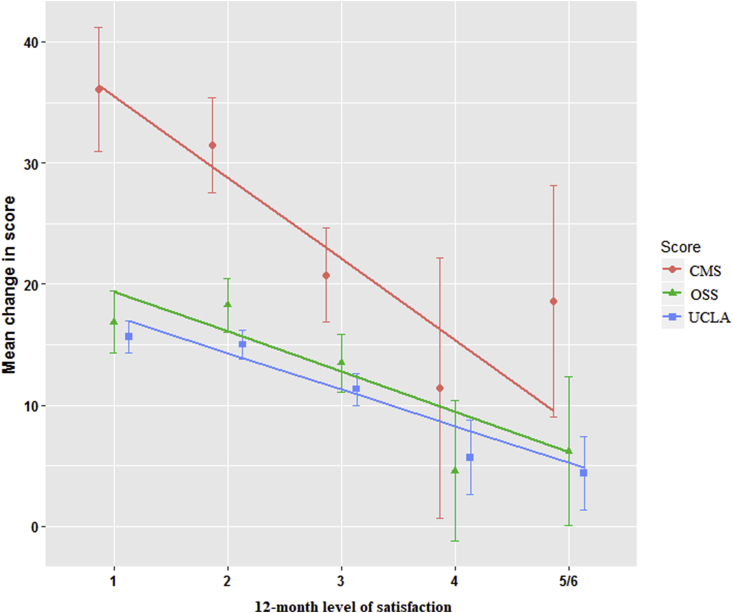
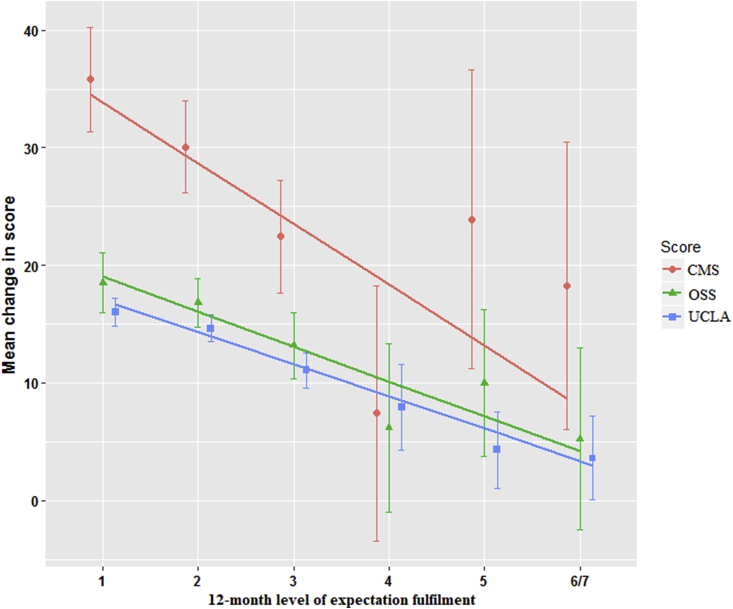
Table 2 shows the distribution of patients and their improvement in CMS, UCLA, and OSS at each level of satisfaction and expectation fulfilment. The improvement in all PROMs over 12-month in each response group was significantly different from each other (p < 0.001). The MCID identified by simple linear regression for satisfaction was: 6.7 (95% CI 4.5–8.9) for CMS, 3.0 (95% CI 2.4–3.7) for UCLA, and 3.3 (95% CI 2.1–4.6) for OSS and for expectation fulfilment was: 5.2 (95% CI 3.3–7.1) for CMS, 2.7 (95% CI 2.2–3.3) for UCLA, and 3.0 (95% CI 1.9–4.4) for OSS (Fig. 1, Fig. 2, Table 3).
Table 2.
12-month change in CMS, UCLA, OSS according to level of satisfaction and expectation fulfilment with surgery.
| Score | All patients | Level of satisfaction |
p-value* | |||||
|---|---|---|---|---|---|---|---|---|
| Excellent | Very good | Good | Fair | Poor/Terrible | ||||
| n | 306 | 72 | 111 | 83 | 25 | 15 | ||
| CMS | 27.4 ± 21.9 | 36.1 ± 21.8 | 31.5 ± 20.9 | 20.7 ± 17.8 | 11.4 ± 26 | 18.6 ± 17.3 | <0.001† | |
| UCLA | 12.9 ± 7 | 15.6 ± 5.6 | 15 ± 6.2 | 11.3 ± 6.1 | 5.7 ± 7.4 | 4.4 ± 5.4 | <0.001† | |
| OSS |
14.9 ± 12.2 |
16.9 ± 10.9 |
18.3 ± 11.7 |
13.5 ± 11.1 |
4.6 ± 14.1 |
6.2 ± 11.1 |
<0.001† |
|
| Score | All patients | Surgery meeting expectation | ||||||
|
Yes, totally |
Yes, almost totally |
Yes, quite a bit |
More or less |
No, not quite |
No, far from it/No, not at all |
|||
| n | 306 | 77 | 113 | 71 | 23 | 10 | 12 | |
| CMS | 27.4 ± 21.9 | 35.8 ± 19.6 | 30.1 ± 21.1 | 22.4 ± 20.2 | 7.4 ± 25.2 | 23.9 ± 17.8 | 18.2 ± 19.3 | <0.001† |
| UCLA | 12.9 ± 7 | 16 ± 5.3 | 14.6 ± 6.2 | 11.1 ± 6.3 | 7.9 ± 8.5 | 4.3 ± 4.5 | 3.6 ± 5.6 | <0.001† |
| OSS | 14.9 ± 12.2 | 18.5 ± 11 | 16.8 ± 10.9 | 13.2 ± 11.9 | 6.2 ± 16.6 | 10 ± 8.7 | 5.2 ± 12.2 | <0.001† |
*One-way ANOVA.
†Statistically significant.
CMA, Constant-Murley Score; UCLA, University of California Los Angeles shoulder score; OSS, Oxford Shoulder Score.
Fig. 1.
12-month improvement in CMS, UCLA and OSS according to level of satisfaction.
Fig. 2.
12-month improvement in CMS, UCLA and OSS according to level of expectation fulfilment.
Table 3.
12-Month minimal clinically important difference for CMS, UCLA, and OSS.
| Satisfaction | ||||
|---|---|---|---|---|
| MCID | 95% CI |
p-value* | ||
| Lower | Upper | |||
| CMS | 6.7 | 4.5 | 8.9 | <0.001† |
| UCLA | 3 | 2.4 | 3.7 | <0.001† |
| OSS |
3.3 |
2.1 |
4.6 |
<0.001† |
|
Expectation | ||||
| MCID | 95% CI | p-value* | ||
|
Lower |
Upper |
|||
| CMS | 5.2 | 3.3 | 7.1 | <0.001† |
| UCLA | 2.7 | 2.2 | 3.3 | <0.001† |
| OSS | 3 | 1.9 | 4 | <0.001† |
*One-way ANOVA.
†Statistically significant.
CMA, Constant-Murley Score; UCLA, University of California Los Angeles shoulder score; OSS, Oxford Shoulder Score.
At 12-month post-operatively, a minimal change of 6.7 for CMS, 3.0 for UCLA, and 3.3 for OSS was needed to constitute a significant change in patient's perceptive of their improvement in satisfaction with surgery and expectation fulfilment.
3.2. At 24-month follow-up
3.2.1. CMS, UCLA, and OSS with level of satisfaction and expectation fulfilment
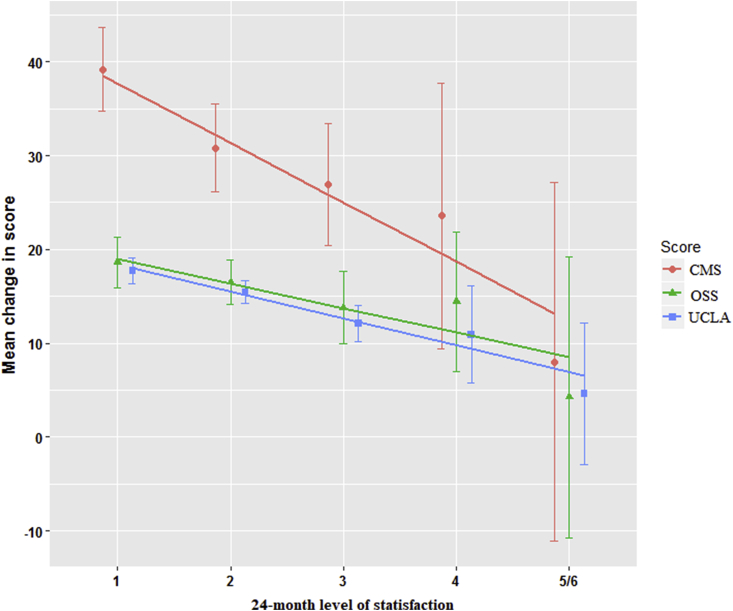
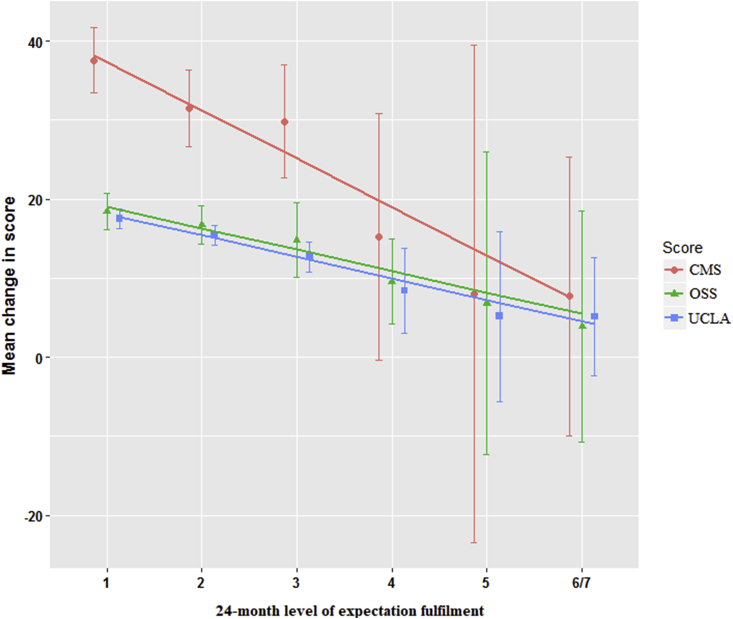
Table 4 shows the distribution of patients and their improvement in CMS, UCLA, and OSS at each level of satisfaction and expectation fulfilment. The improvement in all PROMs over 24-month in each response group was significantly different from each other (p < 0.02). The MCID identified by simple linear regression for satisfaction was: 6.3 (95% CI 3.6–9.1) for CMS, 2.9 (95% CI 2–3.7) for UCLA, and 2.6 (95% CI 1–4.2) for OSS and for expectation fulfilment was: 6.1 (95% CI 3.8–8.4) for CMS, 2.7 (95% CI 2.1–3.4) for UCLA, and 2.7 (95% CI 1.4–4) for OSS (Fig. 3, Fig. 4, Table 5).
Table 4.
24-month change in CMS, UCLA, OSS according to level of satisfaction and expectation fulfilment with surgery.
| Score | All patients | Level of satisfaction |
p-value* | |||||
|---|---|---|---|---|---|---|---|---|
| Excellent | Very good | Good | Fair | Poor/Terrible | ||||
| n | 222 | 62 | 83 | 56 | 13 | 8 | ||
| CMS | 30.9 ± 22.4 | 39.2 ± 17.7 | 30.8 ± 21.6 | 26.9 ± 24.4 | 23.6 ± 23.4 | 8 ± 22.9 | <0.001† | |
| UCLA | 14.6 ± 6.9 | 17.7 ± 5.4 | 15.4 ± 5.5 | 12.1 ± 7.1 | 10.9 ± 8.6 | 4.6 ± 9 | <0.001† | |
| OSS |
15.8 ± 12.3 |
18.6 ± 10.5 |
16.5 ± 10.8 |
13.8 ± 14.4 |
14.4 ± 11.7 |
4.2 ± 17.9 |
0.015† |
|
| Score | All patients | Surgery meeting expectation | ||||||
|
Yes, totally |
Yes, almost totally |
Yes, quite a bit |
More or less |
No, not quite |
No, far from it/No, not at all |
|||
| n | 222 | 77 | 74 | 45 | 13 | 6 | 7 | |
| CMS | 30.9 ± 22.4 | 37.6 ± 18.4 | 31.5 ± 20.8 | 29.8 ± 23.7 | 15.3 ± 25.8 | 8 ± 30 | 7.7 ± 19.1 | <0.001† |
| UCLA | 14.6 ± 6.9 | 17.5 ± 5.4 | 15.4 ± 5.3 | 12.7 ± 6.4 | 8.4 ± 8.9 | 5.2 ± 10.2 | 5.1 ± 8.1 | <0.001† |
| OSS | 15.8 ± 12.3 | 18.4 ± 10.2 | 16.7 ± 10.4 | 14.8 ± 15.8 | 9.9 ± 8.5 | 6.8 ± 18.2 | 3.9 ± 15.8 | 0.004† |
*One-way ANOVA.
†Statistically significant.
CMA, Constant-Murley Score; UCLA, University of California Los Angeles shoulder score; OSS, Oxford Shoulder Score.
Fig. 3.
24-month improvement in CMS, UCLA and OSS according to level of satisfaction.
Fig. 4.
24-month improvement in CMS, UCLA and OSS according to level of expectation fulfilment.
Table 5.
24-Month minimal clinically important difference for CMS, UCLA, and OSS.
| Satisfaction | ||||
|---|---|---|---|---|
| MCID | 95% CI |
p-value* | ||
| Lower | Upper | |||
| CMS | 6.3 | 3.6 | 9.1 | <0.001† |
| UCLA | 2.9 | 2 | 3.7 | <0.001† |
| OSS |
2.6 |
1 |
4.2 |
<0.001† |
|
Expectation | ||||
| MCID | 95% CI | p-value* | ||
|
Lower |
Upper |
|||
| CMS | 6.1 | 3.8 | 8.4 | <0.001† |
| UCLA | 2.7 | 2.1 | 3.4 | <0.001† |
| OSS | 2.7 | 1.4 | 4 | <0.001† |
*One-way ANOVA.
†Statistically significant.
CMA, Constant-Murley Score; UCLA, University of California Los Angeles shoulder score; OSS, Oxford Shoulder Score.
At 24-month post-operatively, a minimal change of 6.3 for CMS, 2.9 for UCLA, and 2.7 for OSS was needed to constitute a significant change in patient's perceptive of their improvement in satisfaction with surgery and expectation fulfilment.
The MCID identified with the two anchor questions and between the two follow-up time points were similar with overlap in 95% Confidence Intervals. Combining the results of both follow-up and taking the higher value as the minimal score required, the MCID necessary to constitute a significant change in patient's perceived outcome is 6.7 for CMS, 3 for UCLA, and 3.3 for OSS.
4. Discussion
The most important findings of this study were the MCID for CMS, UCLA, and OSS in patients who underwent arthroscopic rotator cuff. MCID for American Shoulder and Elbow Surgeon Score (ASES), Simple Shoulder Test Score (SST), and Disabilities of the Arm, Shoulder, and Hand outcome measure (DASH) has been developed and validated in the literature.10,11 CMS, UCLA, and OSS are also common PROMs used in the assessment of patient outcome after arthroscopic RC repair with good reliability and validity, and there is currently a paucity of literature on their MCID. The MCID calculated can be used as a benchmark for the interpretability of a PROM to determine whether the observed change is beneficial to the patient. Furthermore, MCID values can also be used to power future comparative studies as focus on powering a study has shifted from finding statistically significant difference between groups to finding clinically important or relevant differences.23
There are two primary approaches for estimating MCID: distribution-based and anchor-based methods. Distribution-based methods rely on the distribution around the mean scores of the measure of interest. In the anchor-based approach, investigators examine the relation between the target PROM and an independent measure that is itself interpretable – the anchor. In this study, the authors made use of the anchor-based approach. Compared to a distribution-based method in which investigators rely on the distribution around the mean scores of the measure of interest (e.g. Standard Deviation), it is generally agreed that the patient-reported anchor-based approach is the optimal way to determine MCID because it directly captures the patients' preferences and values.15,22 This has also been the method of choice in majority of the MCID literatures published.6, 7, 8, 9, 10, 11 An appropriate anchor need to be relevant to patients and are often global ratings of change.25 The authors made use of 2 anchor questions on patient's satisfaction with surgery and expectation fulfilment as these provide a good overview on patient's perceived treatment success/failure. The use of triangulation, by using 2 anchor questions, is also thought to be a more reliable method of identifying the true MCID and confers greater credibility in the value of MCID determined.6, 7, 8, 9, 10, 11 Furthermore, the question on satisfaction and expectation are 6-points and 7-points Likert based, which allows a more representative linear regression on patient distribution along each level of response. In the study by Kukkonen et al.8 on the MCID of CMS in which a single question was used with 2 possible responses (patients were asked if shoulder is better or worse after operation compared with preoperative state), they found that this pushes patients to decide either better or worse in cases of uncertainty and can affect the quantification of MCID.
Majority of literature on MCID made use of the 12-month post-operative period as the benchmark in calculating MCID. In the study by Clement at al9 on the calculation of Oxford Knee Score and Short-Form 12 Score in Total Knee Arthroplasty in which MCID was calculated at 12-month post-operatively, they concluded that a limitation was the relatively early assessment of patient satisfaction, and potentially some patient's perception might continue to change after this time point, and hence, their level of satisfaction may change. In this study, the authors calculated the MCID at both the 12- and 24-month post-operative follow-up. The MCID calculated at these 2 time points were similar. This method of using two cohort could be seen as a way of validating the values obtained.
4.1. Limitation
The limitation of this study is that all patients were recruited from a single institution and different study population characteristics (age, gender, and ethnicity) may give rise to different scores. Secondly, there is also lack of repeated measurements of the scores within the same patient at each time point of follow up.
5. Conclusion
CMS, UCLA, and OSS are valid tools for calculation of MCID after arthroscopic RC repair and the various MCID calculated could be used as a benchmark for interpretation of these scores and serve as a tool to power future comparative studies.
Funding
No funding was received related to the subject of this article.
Declaration of competing interest
Sheng Xu: The author, their immediate family, and any research foundation with which they are affiliated have not received any financial payments or other benefits from any commercial entity related to the subject of this article.
Jerry Yongqiang Chen: The author, their immediate family, and any research foundation with which they are affiliated have not received any financial payments or other benefits from any commercial entity related to the subject of this article.
Hannah Mei En Lie: The author, their immediate family, and any research foundation with which they are affiliated have not received any financial payments or other benefits from any commercial entity related to the subject of this article.
Ying Hao: The author, their immediate family, and any research foundation with which they are affiliated have not received any financial payments or other benefits from any commercial entity related to the subject of this article.
Denny Tjiauw Tjoen Lie: The author, their immediate family, and any research foundation with which they are affiliated have not received any financial payments or other benefits from any commercial entity related to the subject of this article.
References
- 1.Murphy J., Gray A., Cooper C., Cooper D., Ramsay C., Carr A. Costs, quality of life and cost-effectiveness of arthroscopic and open repair for rotator cuff tears: an economic evaluation alongside the UKUFF trial. The bone & joint journal. 2016;98(12):1648–1655. doi: 10.1302/0301-620X.98B12.BJJ-2016-0121.R1. [DOI] [PubMed] [Google Scholar]
- 2.Miller B.S., Kessler K., Robbins C., Bedi A., Carpenter J., Gagnier J. Does obesity affect functional outcomes after rotator cuff repair? Arthroscopy. 2017;33(10):e85. [Google Scholar]
- 3.Jaeschke R., Singer J., Guyatt G.H. Measurement of health status: ascertaining the minimal clinically important difference. Contr Clin Trials. 1989;10(4):407–415. doi: 10.1016/0197-2456(89)90005-6. [DOI] [PubMed] [Google Scholar]
- 4.Henry P., Wasserstein D., Park S. Arthroscopic repair for chronic massive rotator cuff tears: a systematic review. Arthrosc J Arthrosc Relat Surg. 2015;31(12):2472–2480. doi: 10.1016/j.arthro.2015.06.038. [DOI] [PubMed] [Google Scholar]
- 5.Xu S., Chen J.Y., Lo N.N. The influence of obesity on functional outcome and quality of life after total knee arthroplasty: a ten-year follow-up study. Bone Joint Lett J. 2018;100(5):579–583. doi: 10.1302/0301-620X.100B5.BJJ-2017-1263.R1. [DOI] [PubMed] [Google Scholar]
- 6.Escobar A., Pérez L.G., Herrera-Espiñeira C. Total knee replacement; minimal clinically important differences and responders. Osteoarthr Cartil. 2013;21(12):2006–2012. doi: 10.1016/j.joca.2013.09.009. [DOI] [PubMed] [Google Scholar]
- 7.Lee W.C., Kwan Y.H., Chong H.C., Yeo S.J. The minimal clinically important difference for Knee Society Clinical Rating System after total knee arthroplasty for primary osteoarthritis. Knee Surg Sport Traumatol Arthrosc. 2017;25(11):3354–3359. doi: 10.1007/s00167-016-4208-9. [DOI] [PubMed] [Google Scholar]
- 8.Kukkonen J., Kauko T., Vahlberg T., Joukainen A., Äärimaa V. Investigating minimal clinically important difference for Constant score in patients undergoing rotator cuff surgery. J Shoulder Elb Surg. 2013;22(12):1650–1655. doi: 10.1016/j.jse.2013.05.002. [DOI] [PubMed] [Google Scholar]
- 9.Clement N.D., MacDonald D., Simpson A.H.R.W. The minimal clinically important difference in the Oxford knee score and Short Form 12 score after total knee arthroplasty. Knee Surg Sport Traumatol Arthrosc. 2014;22(8):1933–1939. doi: 10.1007/s00167-013-2776-5. [DOI] [PubMed] [Google Scholar]
- 10.Tashjian R.Z., Deloach J., Green A., Porucznik C.A., Powell A.P. Minimal clinically important differences in ASES and simple shoulder test scores after nonoperative treatment of rotator cuff disease. JBJS. 2010;92(2):296–303. doi: 10.2106/JBJS.H.01296. [DOI] [PubMed] [Google Scholar]
- 11.Franchignoni F., Vercelli S., Giordano A., Sartorio F., Bravini E., Ferriero G. Minimal clinically important difference of the disabilities of the arm, shoulder and hand outcome measure (DASH) and its shortened version (QuickDASH) J Orthop Sport Phys Ther. 2014;44(1):30–39. doi: 10.2519/jospt.2014.4893. [DOI] [PubMed] [Google Scholar]
- 12.Placzek J.D., Lukens S.C., Badalanmenti S. Shoulder outcome measures: a comparison of 6 functional tests. Am J Sports Med. 2004;32(5):1270–1277. doi: 10.1177/0363546503262193. [DOI] [PubMed] [Google Scholar]
- 13.Kirkley A., Griffin S., Dainty K. Scoring systems for the functional assessment of the shoulder. Arthrosc J Arthrosc Relat Surg. 2003;19(10):1109–1120. doi: 10.1016/j.arthro.2003.10.030. [DOI] [PubMed] [Google Scholar]
- 14.Olley L.M., Carr A.J. The use of a patient-based questionnaire (the Oxford Shoulder Score) to assess outcome after rotator cuff repair. Ann R Coll Surg Engl. 2008;90(4):326–331. doi: 10.1308/003588408X285964. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Wells G., Beaton D., Shea B. Minimal clinically important differences: review of methods. J Rheumatol. 2001;28(2):406–412. [PubMed] [Google Scholar]
- 16.Revicki D., Hays R.D., Cella D., Sloan J. Recommended methods for determining responsiveness and minimally important differences for patient-reported outcomes. J Clin Epidemiol. 2008;61(2):102–109. doi: 10.1016/j.jclinepi.2007.03.012. [DOI] [PubMed] [Google Scholar]
- 17.Johnston B.C., Ebrahim S., Carrasco-Labra A. Minimally important difference estimates and methods: a protocol. BMJ open. 2015;5(10) doi: 10.1136/bmjopen-2015-007953. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18.Hägg O., Fritzell P., Nordwall A. The clinical importance of changes in outcome scores after treatment for chronic low back pain. Eur Spine J. 2003;12(1):12–20. doi: 10.1007/s00586-002-0464-0. [DOI] [PubMed] [Google Scholar]
- 19.Musoro ZJ, Hamel JF, Ediebah DE, Cocks K, King MT, Groenvold M, Sprangers MA, Brandberg Y, Velikova G, Maringwa J, Flechtner HH. Establishing anchor-based minimally important differences (MID) with the EORTC quality-of-life measures: a meta-analysis protocol. BMJ open. 2018 Jan 1;8(1):e019117. [DOI] [PMC free article] [PubMed]
- 20.Copay A.G., Subach B.R., Glassman S.D., Polly D.W., Jr., Schuler T.C. Understanding the minimum clinically important difference: a review of concepts and methods. Spine J. 2007;7(5):541–546. doi: 10.1016/j.spinee.2007.01.008. [DOI] [PubMed] [Google Scholar]
- 21.Crosby R.D., Kolotkin R.L., Williams G.R. Defining clinically meaningful change in health-related quality of life. J Clin Epidemiol. 2003;56(5):395–407. doi: 10.1016/s0895-4356(03)00044-1. [DOI] [PubMed] [Google Scholar]
- 22.Stucki G., Liang M.H., Fossel A.H., Katz J.N. Relative responsiveness of condition-specific and generic health status measures in degenerative lumbar spinal stenosis. J Clin Epidemiol. 1995;48(11):1369–1378. doi: 10.1016/0895-4356(95)00054-2. [DOI] [PubMed] [Google Scholar]
- 23.Cadeddu M., Farrokhyar F., Thoma A. Users' guide to the surgical literature: how to assess power and sample size. Can J Surg. 2008;51(6):476. [PMC free article] [PubMed] [Google Scholar]
- 25.King M.T. A point of minimal important difference (MID): a critique of terminology and methods. Expert Rev Pharmacoecon Outcomes Res. 2011;11(2):171–184. doi: 10.1586/erp.11.9. [DOI] [PubMed] [Google Scholar]