The Multi-Scale, Three-Dimensional Nature of Skeletal Muscle Contraction

Abstract

Muscle contraction is a three-dimensional process, as anyone who has observed a bulging muscle knows. Recent studies suggest that the three-dimensional nature of muscle contraction influences its mechanical output. Shape changes and radial forces appear to be important across scales of organization. Muscle architectural gearing is an emerging example of this process.

Introduction

Motion requires an incredibly broad range of mechanical output from our skeletal muscles. A given muscle can contribute to movements that are slow, precise, and delicate or fast, forceful, and powerful. The mechanisms that allow for such a broad functional range are familiar. The independent and precise control of hundreds of motor units within a muscle allows for graded recruitment, with increasing muscle volume recruited for more forceful tasks (14). Individual muscle fibers vary in mechanical and metabolic properties, so, within a muscle, slow, fatigue-resistant fibers can be recruited for long repetitive tasks, whereas fast fibers can be recruited for short bursts of power (29, 41). The topic of this review is another phenomenon that also expands a muscle’s mechanical range: the way it changes shape during a contraction. The motions and forces involved in the radial expansion of muscle fibers during muscle contraction influence force and speed by mechanisms that are not included in most models of muscle function. The organization of muscle fibers and the mechanical interactions of contractile elements, elastic collagenous elements within muscle, and fluid are central determinants of muscle shape change, and thus dynamic muscle architecture may provide a pathway through which disease processes that affect these components influence muscle function.

Muscle Fiber Architecture and Function

Skeletal muscle is often conceptualized as a linear actuator acting to generate tension between two points, the sites of bony attachment that define a muscle’s line of action. In the simplest arrangement, the force-generating fibers (cells) are bundled in parallel, running the length of the muscle, from bone to bone. Some of our muscles are arranged this way, for example, the muscles attached to the hyoid bone that drive motions of chewing and swallowing (22). But in most muscles, the fibers are arranged at some angle to the muscle’s line of action so that the direction in which the fibers generate force and motion is not the same as the direction of whole muscle action. Such muscles are described as “pennate,” with the “pennation angle” describing the angle between the fiber orientation and the muscle line of action. Pennate muscles come in a variety of architectures (15, 29). For example, muscles classified as bipennate resemble a feather, with fibers converging from two sides on a central tendon, whereas, in unipennate muscles, fibers run from one tendinous sheet (aponeurosis) to another (FIGURE 1). Such architectures allow for different kinds of packing of muscle contractile elements within a given muscle volume, and this packing has functional implications. For example, pennation allows for a greater cross-sectional area of muscle within a given volume and therefore allows for higher force per unit muscle mass compared with a parallel arrangement of fibers (1, 15, 29). Pennate muscle architecture is the rule not the exception; the vast majority of limb muscles have some degree of pennation (49).

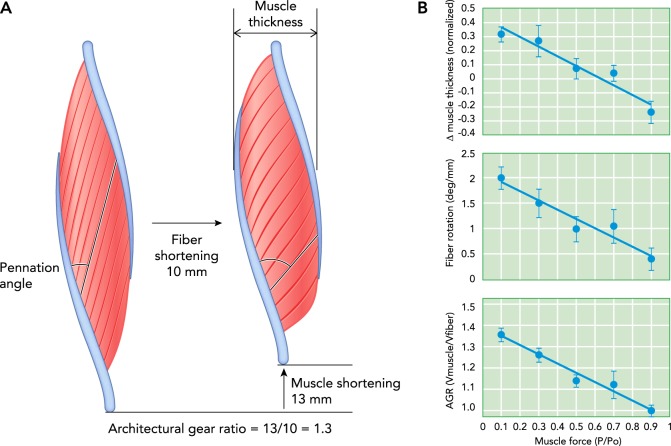
FIGURE 1.

Time points in the contraction of a unipennate muscle and the architectural gear ratio measured for a series of contractions

A: two time points in the contraction of a unipennate muscle show fiber rotation from a lower to a higher angle of pennation. In the example shown, a 10-mm shortening of the muscle fibers is associated with a 13-mm shortening of the muscle. This 1.3-fold greater muscle shortening compared with fiber shortening results from the effects of fiber rotation. B: the architectural gear ratio measured for a series of contractions in turkey gastrocnemius at different levels of force shows that gearing is high for low-force contractions and low for high-force contractions. Measurements of muscle thickness and pennation angle change (i.e., fiber rotation) show that this difference in gearing is due to differences in the way the muscle changes shape. The example given in A represents a low-force, high-gear contraction (from Ref. 2, with permisison from Proceedings of the National Academy of Sciences USA).

A muscle’s fiber architecture is not fixed but changes dynamically during contraction. Modern ultrasound methods can visualize a plane of fibers and the tendinous tissue to which they attach to provide a “movie” of dynamic muscle architecture in vivo. In a study of human gastrocnemius medialis with the ankle in a fixed position, ultrasound measurements showed fiber rotation during a maximum voluntary contraction, from a starting pennation angle of 15.5° to a final angle of 33.6° (35). Such rotation of fibers from lower to higher angles of pennation contributes to muscle shortening and results in a total shortening of the muscle (along the line of action) that is greater than the amount the fibers shorten (FIGURE 1) (36). The term “architectural gear ratio” (AGR) was coined to express this effect and is defined as a ratio of muscle shortening to fiber shortening (8). Measurements on a variety of muscles from various vertebrates show that pennate muscles operate with a gear ratio during shortening typically within a range of ~1–2 (2, 4, 11, 13, 26). Muscle gearing must adhere to the same principle that any gear system (such as that on a bicycle) does: velocity advantages must come at the cost of a reduction in force. In muscles with high gear ratios resulting from fiber rotation, the force the muscle develops at the joint is lower than the total force developed by the fibers. Thus the way in which a muscle changes shape during contraction affects two key mechanical performance metrics of muscle contraction, speed and force.

The AGR of a pennate muscle depends not only on static architecture but also on the way the muscle changes shape in directions orthogonal to shortening (8). Orthogonal shape change (i.e., bulging) is driven by the isovolumetric nature of muscle fibers; when muscle fibers shorten, they must expand radially since they are essentially constant in volume. This dynamic architecture effect is most clear in the segmented body musculature of salamanders and fishes (8) in which angled muscle fibers run between aponeurosis-like sheets of collagen (myosepta). Contraction of the muscle fibers pulls the myosepta toward each other and bends the body laterally for swimming (FIGURE 2). Segmented body musculature differs from pennate muscles in that the aponeuroses translate directly toward each other during muscle shortening (FIGURE 2) rather than sliding or shearing relative to each other as in pennate muscles (FIGURE 1). This difference means that segmented musculature must bulge in one or both of the directions orthogonal to shortening, whereas pennate muscles theoretically can shorten without changing thickness or width because radial muscle fiber expansion can be accommodated by the change in shape of a parallelogram (16).

FIGURE 2.

Schematic of segmented body musculature in a fish or salamander

A: segmented blocks of muscle are arranged along the length of the body, with angled muscle fibers attached to aponeurosis-like collagen sheets (i.e., myosepta). B: simple model of a single fiber in a segment. As the fibers shorten, the segment must bulge dorsoventrally and/or mediolaterally to maintain constant volume. C: the maximum amount of lateral bending (highest AGR) is produced when all bulging is dorsoventral. D: when segments bulge equally in dorsoventral and mediolateral directions, the AGR is lower but still greater than 1. Figure modified from Ref. 8, with permission from Journal of Experimental Biology.

In practice, however, pennate muscles often do change thickness and width during shortening (2, 4, 11, 24, 35, 38). In a simple parallelogram model of pennate muscle, changes in thickness affect gearing (FIGURE 1). Increasing thickness increases the amount of fiber rotation and thereby increases AGR, whereas decreasing thickness decreases fiber rotation and AGR (2). Hence, the AGR of pennate muscles depends on both initial fiber angle and changes in muscle thickness that occur during contraction. This effect of orthogonal muscle shape change was overlooked for a long time because pennate muscles theoretically do not have to bulge when shortening. Early studies of arthropod muscles, in fact, emphasized this feature of pennate muscle, because it was considered an essential feature that allowed muscles to operate within the confined space of an exoskeleton [lobster claw muscles, for example (36)]. In practice, however, many pennate muscles experience bulging during contraction. Studying the segmented musculature of salamanders in which bulging must occur led to an appreciation for how important orthogonal shape change can be in determining dynamic AGR (FIGURE 2) (8).

Dynamic Muscle Architecture and Variable Muscle Gearing

Much of our early understanding of dynamic muscle architecture was developed through theoretical models of how contracting muscle changes shape under a prescribed set of geometric constraints (5, 16, 36). These models predicted features of pennate muscles that have since been measured empirically, including changes in fiber pennation angle during contraction, sliding of opposing aponeuroses, and a decoupling of fascicle length changes from muscle length changes. Over the last few decades, advances in experimental tools have allowed us to build on this theoretical foundation and test some of the predictions. Video analysis of intact but exposed muscles in rats provided the first visualization of the dynamics of pennate muscle contraction (54). These measurements showed changes in pennation angle during a contraction and quantified the velocity differences between the muscle and the fiber (i.e, the AGR) (54). The development of sonomicrometery as an experimental tool for measuring instantaneous muscle fiber lengths during a contraction provided investigators the ability to quantify dimensional changes within a muscle in animal models (23). The experimental toolkit for examining muscle architecture was further expanded through the development of musculoskeletal ultrasound imaging, which allowed investigators to dynamically visualize internal muscle anatomy during contractions in human subjects in vivo (24, 30, 35). These approaches all provide direct measurements of AGR based either on the ratio of length changes in the muscle and the fascicle or on the ratio of the shortening velocity of the muscle and fascicle. Regardless of the experimental approach, measurements of AGR are ideally made during a period of constant force to limit the potential contribution of series elastic elements (which can be substantial in pennate muscles) to the observed length changes. As a result, investigators have either made such measurements during the constant force phase of isotonic contractions (2, 4, 26) or visualized the length changes of elastic structures and accounted for their effects during data analysis (11).

Initially, studies aiming to quantify AGR relied on the predictions of existing models and therefore expected to find that a muscle with a given architecture (i.e., pennation angle) operates with a single relationship between fiber shortening and muscle shortening (8). Implicit in this prediction is the idea that pennation angle changes but that there is always the same fixed (force invariant) relationship between fiber length and pennation angle. Direct measurements of AGR in a number of muscles, however, showed that this is not the case; the relationship between pennation angle and fiber length can vary significantly in the same muscle, depending on the force of contraction (2). It has been established that the way a muscle changes shape during a contraction is influenced by the force of a contraction, which in turn changes the relationship between fiber length and pennation angle (as discussed in the following section). Most interestingly, these findings showed that not only does AGR vary across the range of forces the muscle produces, but also that the variation in AGR provides more force during forceful contractions and more speed during high-velocity, lower-force contractions compared with a muscle with constant AGR. The presence of variable gearing in a pennate muscle will therefore extend the functional range by dynamically changing the gear through which muscle fibers act on a load. Although other mechanisms, such as variation in fiber types and muscle moment arms, allow adjustments in force or speed, such changes simply shift the functional range of a muscle. Variable gearing is a unique feature of muscle in that it provides a mechanism that broadens a muscle’s functional range (2).

Variable gearing has been observed in the pennate muscles of a number of animals, including turkeys, frogs, rats, and humans (2, 11, 26). In most cases, AGR has been quantified during isotonic (constant force) concentric contractions. Results consistently show that pennate muscles operate with the highest gear at low contractile force and lowest gear at low force (12). AGR has also been quantified during eccentric (lengthening) contractions in the frog plantaris, indicating that the underlying mechanism responsible for variable gearing likely works across a broad range of contractile conditions (4). Studies using a highly controlled set of conditions (e.g., isotonic contractions in situ) have been crucial for understanding the underlying mechanism responsible for variable gearing. In a few cases, variable gearing has been measured in vivo during normal activities by using ultrasound (11). Extending such findings to additional natural movements in vivo remains challenging and provides an exciting avenue for further investigation.

Structures and Mechanisms Involved in Muscle Shape Change

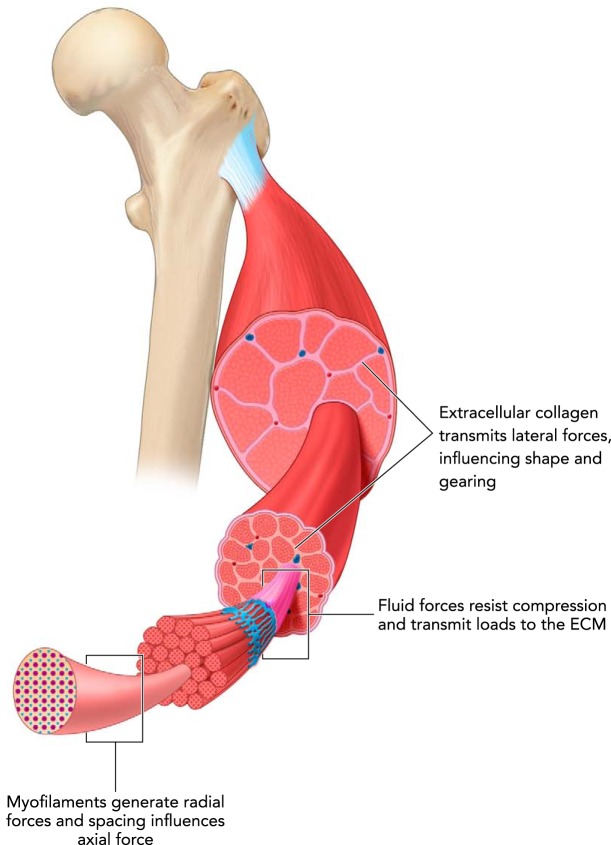
The observation that dynamic shape changes in muscle influence muscle force and speed by determining muscle gearing is, we believe, just one example of a growing understanding of two important but underappreciated phenomena that underlie muscle mechanical performance. First, muscle contraction is a three-dimensional process, and the dominant conceptual model of muscle as a one-dimensional linear actuator may impede our understanding of muscle contraction, and in particular the functional consequences of structural changes that occur in growth and disease. Second, although acto-myosin interactions are the ultimate source of force and power in a muscle contraction, the composite, hierarchical nature of muscle significantly shapes mechanical output (FIGURE 3).

FIGURE 3.

Muscle is a composite, hierarchical structure

Force transmission occurs between different structures, at different levels of organization, and in directions both along the line of action of the muscle and orthogonal to it. The transmission of forces and flow of mechanical energy between elements within muscle determines muscle gearing and also has implications for many aspects of the mechanics and energetics of muscle contraction.

Sarcomeres are considered the functional unit of muscle, and the textbook portrayal of sarcomere function as the interaction of actin and myosin shapes much of our thinking about how muscles work as actuators. Specifically, this model emphasizes a linear, one-dimensional production of force and motion, and it implicitly depicts actin and myosin behavior as the sole determinant of muscle contractile performance. The view of the sarcomere as a one-dimensional linear actuator overlooks the complex three-dimensional motions and forces occurring during a muscular contraction. It has been recognized that in skinned (permeabilized) muscle fibers, changes in lattice spacing (the distance between actin and myosin filaments in the radial dimension) can significantly influence muscle force (33). This behavior has generally been considered an artifact of skinned muscle preparations, where lattice spacing can vary widely with osmotic shrinking or swelling (33). Recently, however, it has been demonstrated through theoretical and experimental approaches that lattice spacing may influence muscle force as the muscle changes length (52). The well-known decline in muscle tension at lengths longer and shorter than optimal is generally attributed to changes in filament overlap in the axial dimension (20), but the effects of filament spacing in the radial dimension may explain 20–30% of the variation in force (52). The flow of elastic strain energy through filaments and cross-bridges has been a central theme of muscle biophysics, and this work has also focused on axial strains and forces. However, evidence from modeling suggests that radial forces between filaments and flows of elastic energy in the radial direction may be of the same order of magnitude as those in the axial (line of action) direction (51).

Three-dimensional transmission of force and motion is also important at levels of organization beyond the sarcomere. Increasingly, there has been an appreciation of geometric variation at the level of the whole muscle affecting contraction mechanics through effects on muscle fiber arrangements and architecture (6, 45, 50). The observation that muscles produce force in the transverse direction, orthogonal to fiber orientation, influencing muscle force (40, 42, 44) and work (3), further highlights the three-dimensional nature of a contraction.

A consideration of the three-dimensional nature of muscle contraction also expands the potential mechanical roles of the various structural components of muscle. Acto-myosin interactions are at the heart of muscle actuation, but the mechanical role of many other proteins is significant, with the emergence of titin as the “third myofilament” being a prominent example (21, 25, 31). Mechanically relevant, non-sarcomeric components of muscle include the many intermediate proteins that span from myofilaments to the sarcolemma (17), the collagenous extracellular matrix that surrounds and links muscle fibers (7, 18, 39), and intracellular and extracellular fluids (19, 46). Studies of the functional roles of the collagenous extracellular matrix have emphasized its importance as a pathway for force transmission from sarcomeres to tendon (27, 48) and as a structure that contributes to passive muscle tension (9, 32, 53). It has also been proposed that the collagenous sleeves surrounding muscle fibers and fascicles have the potential to resist the radial expansion that occurs during shortening, possibly reducing muscle work in fibrotic muscle (3). The reduction in muscle force that occurs with transverse (orthogonal to the line of action) compression of a muscle also suggests a pathway by which ECM might influence muscle function (43, 44).

Forces transmitted by fluid within muscle are likely important in the three-dimensional dynamics of muscle contraction. Intramuscular fluid pressures developed during active muscle contraction can be measured, and under some measurement conditions have been estimated to be proportional to developed muscle force (10). Models and measurements in passive muscle suggest that the resistance of fluid to compression may play an essential role in the contribution of the ECM to passive tension (19, 46). Transmission of force via fluid pressure also likely plays a role in active muscle contraction and in the dynamics of architectural changes in pennate muscles (12).

Mechanical interactions between sarcomere-generated forces, collagenous elements in muscle (ECM, aponeurosis), and fluid are hypothesized to underlie the change in muscle gearing with contractile force (12). Briefly, forces developed by contractile elements that are orthogonal to the muscle line of action tend to compress the muscle belly, and this compression is resisted by collagenous ECM and aponeurosis. Central to this proposed mechanism is the transmission of force via fluid to redirect forces that tend to compress the muscle in thickness (the distance between aponeuroses; FIGURE 1) to forces that act to extend the muscle in width (orthogonal to muscle thickness and length), thus allowing ECM collagenous fibers and aponeurosis to be loaded in tension. Higher contractile forces lead to higher loading of ECM, greater changes in muscle width, and thus lower changes in muscle thickness. Variable gearing results because increases in width tend to reduce muscle fiber rotation, whereas increases in thickness increase fiber rotation. The proposed mechanism may involve substantial flows of energy between sarcomeres and collagenous elastic elements of muscle. This internal cycling of elastic energy in muscle may have significant implications for muscle mechanical and metabolic function, and it is not included in current models of muscle function.

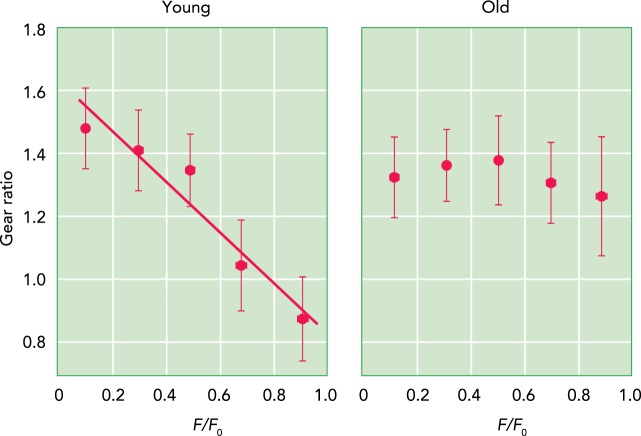
Connective tissues associated with muscle are altered through muscle growth, through disease processes, and with aging (28, 37, 47, 53). Many neuromuscular disorders involve an increase in the passive stiffness of muscle, and evidence suggests that, in some cases, this may be related to a stiffening of the ECM (47). Similarly, a study of aged mice showed a correlation between passive muscle stiffness and both the amount and composition of muscle ECM (53). The idea that collagenous ECM also plays an important role through elastic energy storage and recovery and through an influence on muscle shape change during active contraction points to other mechanisms by which changes in ECM might influence muscle function. Holt and coworkers (26) studied muscle shape change and gearing in aged rats and found that, although young rats showed the variable gearing observed in other species, the muscles of old individuals operated with a constant gear in all muscle contractions (FIGURE 4). The older rats had stiffer aponeuroses, and it was proposed that stiffer aponeurosis and ECM prevented increases in muscle width, thus favoring increases in thickness and the fiber rotation associated with high gearing. These results suggest that connective tissue properties in muscle are “tuned” to allow for variable muscle shape changes and gearing. The reduced range of gearing in older muscles may contribute to reduction in force output with age (26). Direct perturbations to muscle-associated connective tissue have also demonstrated an influence on gearing. In an isolated muscle preparation, incising the aponeurosis to reduce its mechanical integrity leads to a reduction in gearing at high forces (13).

FIGURE 4.

Gearing varies in young but not old rat muscles

Young rats show a pattern of architectural gearing that has been observed in other species, i.e., high-force contractions operate at a lower gear (left). In aged rats (right), there is no change in gear ratio with force; all contractions show the same gear ratio. It is hypothesized that this lack of variable gearing results from stiffening of collagenous components of the ECM and aponeuroses. Force is normalized to the maximum isometric force (F_o).

Summary

The complex physical interaction among the many elements of skeletal muscle shapes its mechanical performance. Acto-myosin interactions are at the heart of this process and have received the most attention, but the mechanical action of many other structures over several scales of organization also influence the force and speed of muscle contraction. Variable gearing in pennate muscles is one example, where the interaction of contractile elements, fluid, and collagenous extracellular matrix can alter muscle speed and force through the effects of variable muscle shape change. A linear, one-dimensional view of muscle contractile mechanics limits our understanding of these complex three-dimensional phenomena. Our comprehension of the functional consequences of structural changes that occur in growth, aging, and disease should benefit from greater understanding of the multi-scale, three-dimensional nature of muscle contractile mechanics.