Sleep duration and mortality – Does weekend sleep matter?

Summary

Previous studies have found a U‐shaped relationship between mortality and (weekday) sleep duration. We here address the association of both weekday and weekend sleep duration with overall mortality. A cohort of 43,880 subjects was followed for 13 years through record‐linkages. Cox proportional hazards regression models with attained age as time‐scale were fitted to estimate multivariable‐adjusted hazard ratios and 95% confidence intervals for mortality; stratified analyses on age (<65 years, ≥65 years) were conducted. Among individuals <65 years old, short sleep (≤5 hr) during weekends at baseline was associated with a 52% higher mortality rate (hazard ratios 1.52; 95% confidence intervals 1.15–2.02) compared with the reference group (7 hr), while no association was observed for long (≥9 hr) weekend sleep. When, instead, different combinations of weekday and weekend sleep durations were analysed, we observed a detrimental association with consistently sleeping ≤5 hr (hazard ratios 1.65; 95% confidence intervals 1.22–2.23) or ≥8 hr (hazard ratios 1.25; 95% confidence intervals 1.05–1.50), compared with consistently sleeping 6–7 hr per day (reference). The mortality rate among participants with short sleep during weekdays, but long sleep during weekends, did not differ from the rate of the reference group. Among individuals ≥65 years old, no association between weekend sleep or weekday/weekend sleep durations and mortality was observed. In conclusion, short, but not long, weekend sleep was associated with an increased mortality in subjects <65 years. In the same age group, short sleep (or long sleep) on both weekdays and weekend showed increased mortality. Possibly, long weekend sleep may compensate for short weekday sleep.

1. INTRODUCTION

The health effects of sleep duration have been addressed in a large number of studies and summarized in several systematic reviews (Cappuccio, D'elia, Strazzullo, & Miller, 2010; Gallicchio & Kalesan, 2009; Silva et al., 2016). They conclude that both short and long sleep is associated with higher mortality. Still, published results are not consistent, as pointed out by Kurina et al. in their critical review (Kurina et al., 2013). Two recent meta‐analyses (Itani, Jike, Watanabe, & Kaneita, 2017; Jike, Itani, Watanabe, Buysse, & Kaneita, 2018) found that short sleep was significantly related not only to increased mortality but also to increased morbidity, with a similar association observed for long sleep. In these two meta‐analyses, age‐subgroup analyses, using 65 years as cut‐off, showed an increased mortality in both age groups among individuals with long sleep, but no significant association between short sleep and mortality in any of the age groups. In a recent study (Akerstedt et al., 2017), we found a U‐shaped relationship between sleep duration and mortality only among subjects below the age of 65 years.

Most studies of sleep duration and its consequences ask for the “usual” or “habitual” duration. It is not clear, however, whether individuals refer only to weekdays or take into account weekends. Because there are 5 weekdays and 2 weekend days, it is likely that self‐reports of typical sleep duration more strongly reflect weekday sleep. Thus, it is of interest to investigate the relationship between weekend sleep duration and mortality, as well as the different patterns of sleep duration between weekday and weekend sleep. If, for example, short weekday sleep increases mortality, long weekend sleep might mitigate this effect. Moreover, short sleep during both weekday and weekend (consistently short sleep) may be particularly detrimental. This issue has not been addressed before, but weekend compensatory sleep seems to counteract obesity in children who have short sleep duration during weekdays (Wing, Li, Li, Zhang, & Kong, 2009), and to counteract hypertension in adults (Hwangbo, Kim, Chu, Yun, & Yang, 2013). The purpose of the present study was to prospectively analyse the association between different sleep duration patterns between weekday and weekend sleep duration and mortality in a large Swedish cohort during 13 years of follow‐up.

2. MATERIALS AND METHODS

2.1. Study population

The Swedish National March Cohort (SNMC) comprises participants who took part in a fundraising event for the Swedish Cancer Society organized in September 1997 at 3,600 sites throughout Sweden (Trolle Lagerros et al., 2017). A total of 43,880 subjects returned the 36‐page questionnaire concerning lifestyle and medical history. The study was approved by the Regional Ethical Review Board of Karolinska Institutet, and all subjects provided informed consent.

Participants with inconsistent answers (n = 4), incorrect national registration number (n = 13), missing data on age (n = 11), age <18 years (n = 1,740), who had emigrated (n = 457) or died (n = 8) before the start of follow‐up, or with missing values on weekday or weekend sleep duration (n = 3,632) were excluded from the analyses. After applying the exclusion criteria, the final cohort consisted of 38,015 subjects.

2.2. Exposure assessment

Sleep duration was self‐reported through two questions at baseline: “How many hours, approximately, do you usually sleep during a workday/weekday night?” and “How many hours, approximately, do you usually sleep per night on days off?” The response alternatives were: <5, 5, 6, 7, 8 or ≥9 hr. Even though days off do not necessarily correspond to the weekend, for simplicity, we will refer to the second question as weekend sleep duration. For the study of weekend sleep we defined short sleep as ≤5 hr, and weekend long sleep as ≥9 hr, with 7 hr being the reference category. The former is a common definition (Cappuccio et al., 2010) and the one used in the largest study (Kripke, Garfinkel, Wingard, Klauber, & Marler, 2002). The definition of long sleep as ≥9 hr is the most common one (Cappuccio et al., 2010), and the one used in the largest study (Kripke et al., 2002). For the analysis of both weekend and weekday sleep duration we collapsed the two highest categories to ensure enough participants with long weekday sleep (which is shorter than weekday sleep).

We further combined some subgroups due to small cell sizes, and formed the following weekday/weekend sleep duration groups: short (SS); medium (MM–reference); or long (LL) sleep on both weekdays and weekend; short weekday and medium or long weekend sleep (SML); medium weekday and long weekend sleep (ML). A final group was composed of those who reported long weekday and short, or medium, weekend sleep or medium weekday and short weekend sleep (LS).

2.3. Confounders

All previous studies of the association between sleep duration and mortality adjust for a large number of potential confounders measured at baseline (Cappuccio et al., 2010; Gallicchio & Kalesan, 2009; Silva et al., 2016). Among them are gender, education, body mass index (BMI), severe disease, use of hypnotics, and lifestyle factors like smoking, alcohol intake, physical activity, coffee intake, employment status (all adjusted for in the present study). Most of these factors are likely to be associated with mortality as well as with sleep.

2.4. Follow‐up and outcome

Participants were followed for overall and cause‐specific mortality from 1 October 1997 until the date of death, emigration or the end of follow‐up on 31 December 2010, whichever occurred first. Through the use of the individually unique National Registration Numbers (NRNs), provided by the participants at baseline, the cohort was linked to the Swedish National Register of Death to obtain information on date of death and the underlying causes of death. Up to the end of follow‐up, 3,234 deaths occurred. The main causes of death were cancer (ICD‐10 codes C00‐C97; n = 1,515) and cardiovascular diseases (CVD‐ICD‐10 codes I00‐I99; n = 1,017).

2.5. Statistical analysis

Baseline characteristics of the study cohort are described according to the six groups of weekday and weekend sleep durations. The distribution of all variables, except age and sex, was directly standardized to the age distribution of the entire study population. We fitted multivariable‐adjusted Cox proportional hazards (PHs) models with attained age as time‐scale to estimate overall mortality hazard ratios (HRs) and 95% confidence intervals (95% CIs) for both weekend sleep duration and the weekday/weekend sleep duration groups (Figure 1); stratified analyses on age (<65 years, ≥65 years) were conducted. Sixty‐five years represents the common retirement age in Sweden; we therefore assumed that weekday sleep duration is no longer restricted by working hours after retirement, and may thus permit extended sleep. The latter should be of importance for interpreting data on sleep duration and mortality. In a previous study the association between long or short sleep duration and mortality decreased with increasing age, and was no longer significant in the ≥65 years group (Akerstedt et al., 2017).

Figure 1.

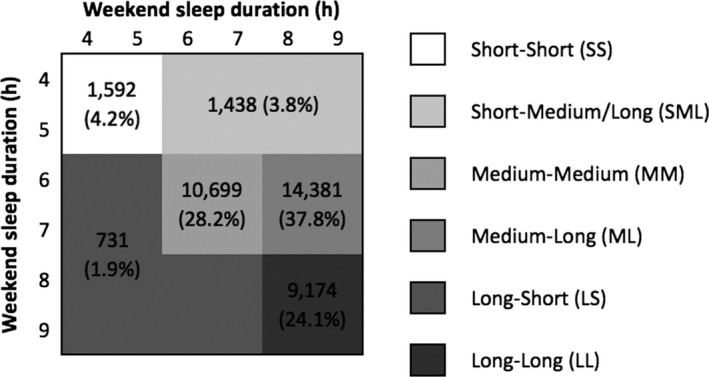
Distribution of weekday/weekend sleep durations

To control for potential confounding, we adjusted for sex, BMI (categorized as <25, 25–30, >30 kg/m²), education (categorized as 9, 11–14, ≥15 years, other), smoking status (categorized as never, former, current), alcohol consumption (g per month), total physical activity (expressed as metabolic energy turnover hours per day [MET‐hr per day], and categorized as low <34.3, medium 34.3–46.4, high >46.4; Ainsworth et al., 1993), shift work (categorized as daytime, shiftwork, no work hours, other) and Charlson's co‐morbidity weighted index based on the National Inpatient Register (Charlson, Pompei, Ales, & Mackenzie, 1987; categorized as 0, ≥1). When weekend sleep duration was the main exposure of interest, we further adjusted for weekday sleep duration categorized as ≤5, 6, 7 and ≥8 hr.

Schoenfeld's residuals (Grambsch & Therneau, 1994) were used to assess whether the underlying PHs assumption of the Cox model was met, both for sleep duration and for the confounders. In case of departures from proportionality, interactions between the time‐scale and the exposures were investigated (Cologne et al., 2012; Lamarca, Alonso, Gomez, & Munoz, 1998). Overall, our exposures were not fulfilling the PH assumption, and time‐varying HRs of the weekday/weekend sleep duration exposure were estimated. The temporal trend was modelled based on restricted cubic splines after having selected 4 knots according to Harrell's recommendations (Harrell, 2001; Heinzl & Kaider, 1997). The corresponding graphs are also reported. Finally, for the predictors who did not fulfill the PH assumption (Charlson's index), stratified Cox models were implemented.

Multivariate Cox models were also fitted to study cancer mortality and CVD mortality. Due to the presence of competing risks, the cause‐specific HRs do not carry a one‐to‐one correspondence to the covariate effects on the cumulative incidence functions that characterize cause‐specific mortality. However, we still carried out these analyses because the alternative choice, the regression analysis on the cumulative incidence functions, has its own drawback – difficulty of interpreting the coefficients (Andersen, Geskus, De Witte, & Putter, 2012). Quantile regression was used to model the 25th, 50th and 75th percentiles of age at death (Bellavia, Akerstedt, Bottai, Wolk, & Orsini, 2014); differences in survival percentiles between the weekday/weekend sleep duration groups are reported. The same set of confounders as in the main analysis was used, to which baseline age is added.

2.6. Sensitivity analysis

In sensitivity analyses we excluded subjects who had less than 2 years of follow‐up time in order to prevent reverse causation and, simultaneously, we left the follow‐up start 2 years later for subjects who contributed for more than 2 years of follow‐up. In addition, we further adjusted our model for sleep‐ and health‐related variables: sleep quality, snoring, self‐reported sleep medication use, napping, feeling rested at awakening, coffee intake, subjective health and high demands at work. Demands at work (Karasek & Theorell, 1990) were assessed by five questions (working fast, working hard, too much efforts, enough time and conflicting demands) and split at the median value.

Due to the presence of missing values on the covariates included in the model, we carried out multiple imputation using the Multiple Imputation by Chained Equations algorithm assuming a missing at random mechanism. The imputation model for each incomplete variable included information on the survival outcomes (Nelson–Aalen cumulative hazard and mortality status), age and the variables appearing in the fully‐adjusted Cox PHs model. Twenty imputed datasets were thus created and analysed. The results were pooled to obtain the HR estimates and the 95% CIs.

Analyses were performed using Stata, version 14 (StataCorp LP, College Station, Texas, USA). All statistical tests were two‐sided, and p‐values less than 0.05 were considered statistically significant.

3. RESULTS

Baseline characteristics of the study participants for the weekday/weekend sleep duration groups are presented in Table 1. Subjects with short‐short sleep (SS) were on average older, less educated and more physically active. Subjects with long‐long sleep (LL) were more likely not to use sleep medications, to be never smokers and to drink less coffee. Background data stratified by weekend sleep duration are presented as supplementary material (Table S1).

Table 1.

Age‐standardized baseline characteristics by categories weekdays/weekend sleep durations in the Swedish National March Cohort

Characteristics	Sleep durationa, hr per day
	Short‐short		Short‐medium/long		Medium‐medium		Medium‐long		Long‐long		Long‐short
	No.	%	No.	%	No.	%	No.	%	No.	%	No.	%
N	1,592		1,438		10,699		14,381		9,174		731
Years at risk, mean (SD)	12.1 (2.8)		12.8 (2.0)		12.6 (2.2)		13.0 (1.5)		12.6 (2.2)		12.4 (2.4)
Deaths, n	323	20.3	85	5.9	1,302	12.2	503	3.5	918	10.0	103	14.1
Age, mean (SD)	61.2 (14.2)		46.1 (14.9)		56.7 (13.7)		44.0 (13.2)		50.2 (16.9)		54.8 (16.5)
Gender, males	587	36.9	604	42.0	4,499	42.1	4,765	33.1	2,972	32.4	260	35.6
BMI, mean (SD)	25.01 (4.0)		25.3 (4.0)		24.7 (3.4)		24.5 (3.5)		24.5 (3.5)		25.1 (3.6)
Education
9 years	912	46.9	476	39.4	4,715	36.6	3,766	33.6	3,460	37.9	351	43.5
11–14 years	431	35.5	569	35.1	3,041	34.8	5,226	33.0	3,057	33.2	241	36.3
15 years	212	17.0	361	24.3	2,739	27.9	5,214	32.9	2,498	28.2	116	18.9
Other	17	0.6	15	1.2	90	0.7	62	0.5	71	0.7	10	1.3
Employment
Employed	373	49.7	895	68.0	4,520	62.4	9,823	70.0	3,713	58.0	256	56.8
Unemployed	38	5.7	29	2.1	197	2.9	218	1.6	262	3.6	23	4.9
Retired	850	24.7	134	20.5	3,999	24.1	638	20.4	2,588	25.3	267	23.9
Sick leave	75	8.2	16	1.3	190	2.0	83	0.7	208	3.2	14	2.4
Other	75	11.8	153	8.1	343	8.6	1,326	8.3	877	9.9	62	12.0
Snoring
Frequent	140	9.5	145	10.4	879	7.6	942	7.7	653	7.3	68	9.3
Infrequent	1,438	90.5	128	89.6	9,760	92.4	13,393	92.3	8,479	92.	657	90.7
Work schedule
Daytime	474	47.8	803	54.0	4,953	57.3	10,553	66.9	4,709	57.8	346	58.6
Shiftwork	106	13.6	352	22.8	946	12.5	1,956	11.3	719	8.9	64	11.5
Other	22	2.1	52	3.2	151	2.1	309	2.3	168	1.8	12	1.7
No work	753	36.5	152	20.0	3,608	28.1	1,073	19.5	2,801	31.5	238	28.2
Smoking
Never	903	59.7	782	61.6	6,075	61.8	8,548	65.6	5,621	64.8	409	59.3
Former	382	26.7	372	27.4	3,043	29.6	3,716	26.8	2,277	28.1	191	29.0
Current	119	13.6	170	11.0	699	8.6	1,212	7.6	575	7.1	68	11.7
Alcohol g per month, mean (SD)	399 (1,302)		380 (919)		339 (730)		312 (518)		292 (473)		346 (663)
Self‐reported health
Very good	233	15.9	296	20.1	2,637	26.7	4,203	27.9	2,531	28.4	177	25.8
Good	749	46.3	748	53.8	5,937	56.3	7,904	56.6	4,940	54.9	382	54.3
Average	410	27.0	290	21.6	1,581	14.2	1,754	13.4	1,216	13.4	130	17.2
Poor	109	9.4	55	3.9	240	2.5	240	1.8	242	2.8	15	2.1
Very poor	14	1.4	8	0.6	25	0.3	32	0.3	39	0.5	5	0.6
Physical activityb
Low	563	34.5	452	34.1	4,207	40.8	5,834	43.2	4,194	49.0	290	43.7
Medium	490	34.7	467	34.5	3,493	35.5	4,951	35.4	2,852	33.1	212	31.6
High	410	30.8	445	31.4	2,278	23.7	2,845	21.4	1,580	17.9	165	24.7
Coffee intake, cups per day
None	122	11.6	177	10.8	915	12.2	2,071	12.4	1,431	14.5	68	10.4
1–3	892	50.4	643	48.1	5,686	51.3	7,060	52.5	4,823	53.6	412	55.5
4–6	491	31.8	478	34.6	3,509	31.9	4,479	31.6	2,520	29.1	214	29.6
≥7	56	6.2	103	6.5	428	4.6	564	3.5	235	2.8	28	4.5
Nap during day	161	8.0	103	9.2	970	7.0	590	6.0	970	9.9	61	6.5
Hypnotics	565	28.1	277	21.7	1,712	13.2	1,065	9.6	884	9.5	114	13.3
Diabetes	90	4.7	23	2.0	291	2.3	220	2.0	228	2.5	31	3.9
Charlson index ≥1	314	13.9	127	10.7	1,337	9.8	963	9.7	1,079	11.3	108	11.6

BMI, body mass index.

^aGroups are given as weekday‐weekend sleep durations, e.g. short‐medium/long group stands for short sleep during the week and medium or long sleep during the weekend.

^bHigh, medium and low total physical activity levels correspond to more than 46.4, 34.3–46.4, and less than 34.3 MET‐hr per day, respectively.

3.1. Weekend sleep and mortality

Among individuals below the age of 65 years at enrolment (Figure 2), short weekend sleep was associated with a significant 52% higher mortality rate (HR 1.52; 95% CI 1.15–2.02) compared with the 7 hr group, while no association was found for long (≥9 hr) weekend sleep. After adjusting for weekday sleep duration, the risk estimate for short sleep decreased, and lost statistical significance (HR 1.40; 95% CI 0.97–2.02). Among individuals ≥65 years, no association was observed. In fully adjusted models, no significant association was detected between weekend sleep duration and cause‐specific hazards for cancer or CVD mortality neither in the younger, nor in the older age group (data not shown).

Figure 2.

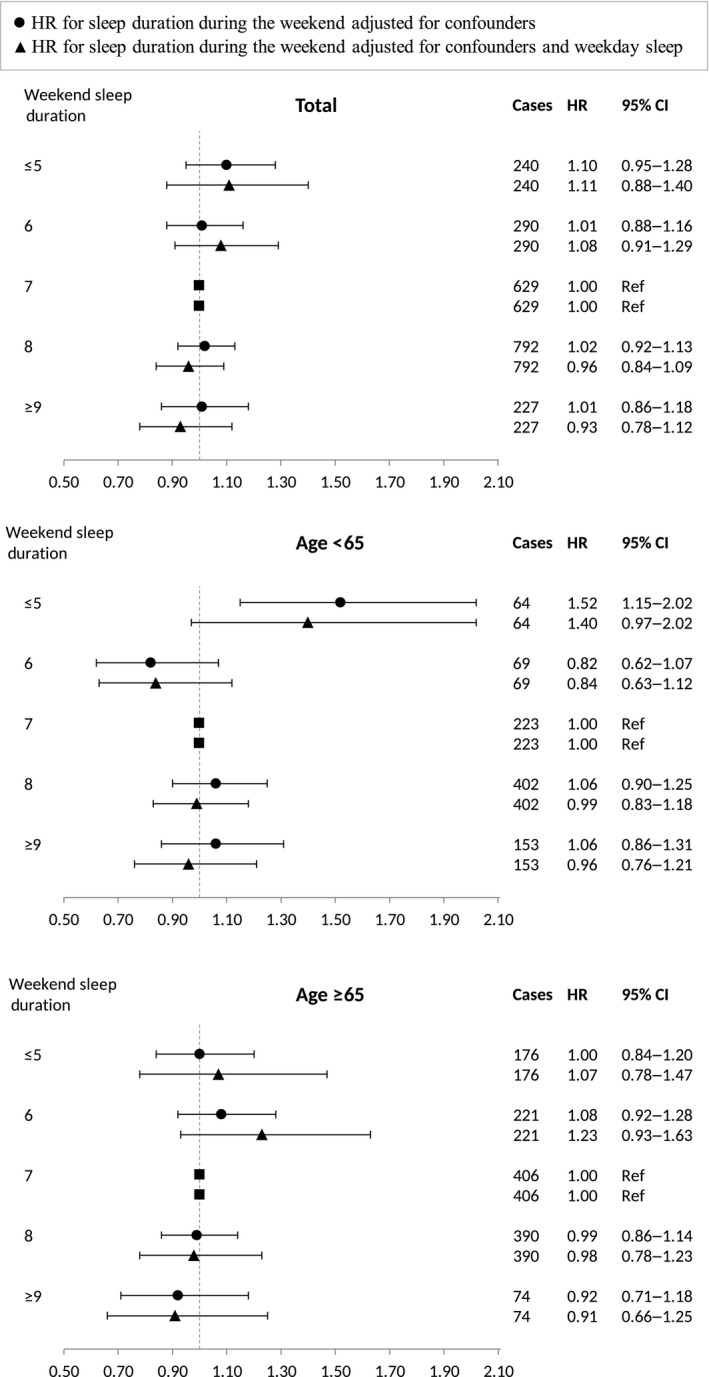
Forest plots showing multivariate Cox regression analysis of the association of weekend sleep duration with mortality – overall and stratified by age. The model was adjusted for sex, body mass index, smoking status, alcohol consumption, educational level, physical activity, shift work and Charlson index

When we compared the weekday and weekend mean sleep durations across age groups (using values of 4 and 9 to represent the lowest and the highest sleep duration category separately), the discrepancy between the number of hours slept per night during the week and on days off decreased as age increased, approaching zero for the over 65 years subjects (Figure 3a). This is due to a gradual decrease in weekend sleep duration with age, compared with a nearly constant pattern for weekday sleep duration. Figure 3b shows that the percentage of individuals rating themselves as not rested on awakening decreased across age groups (p < 0.001). Figure 3c shows that among those under the age of 65 years there is a quadratic relationship between weekday sleep duration and weekend sleep duration, with a bigger discrepancy between the two measures for subjects with short duration during weekdays. The relationship between weekday sleep duration and weekend sleep duration among those aged 65 years or older seemed to be linear. The Pearson correlation between weekday and weekend sleep duration was r = 0.58 (p < 0.001) for the whole cohort, r = 0.53 (p < 0.001) in the youngest group and r = 0.85 (p < 0.001) in the oldest group.

Figure 3.

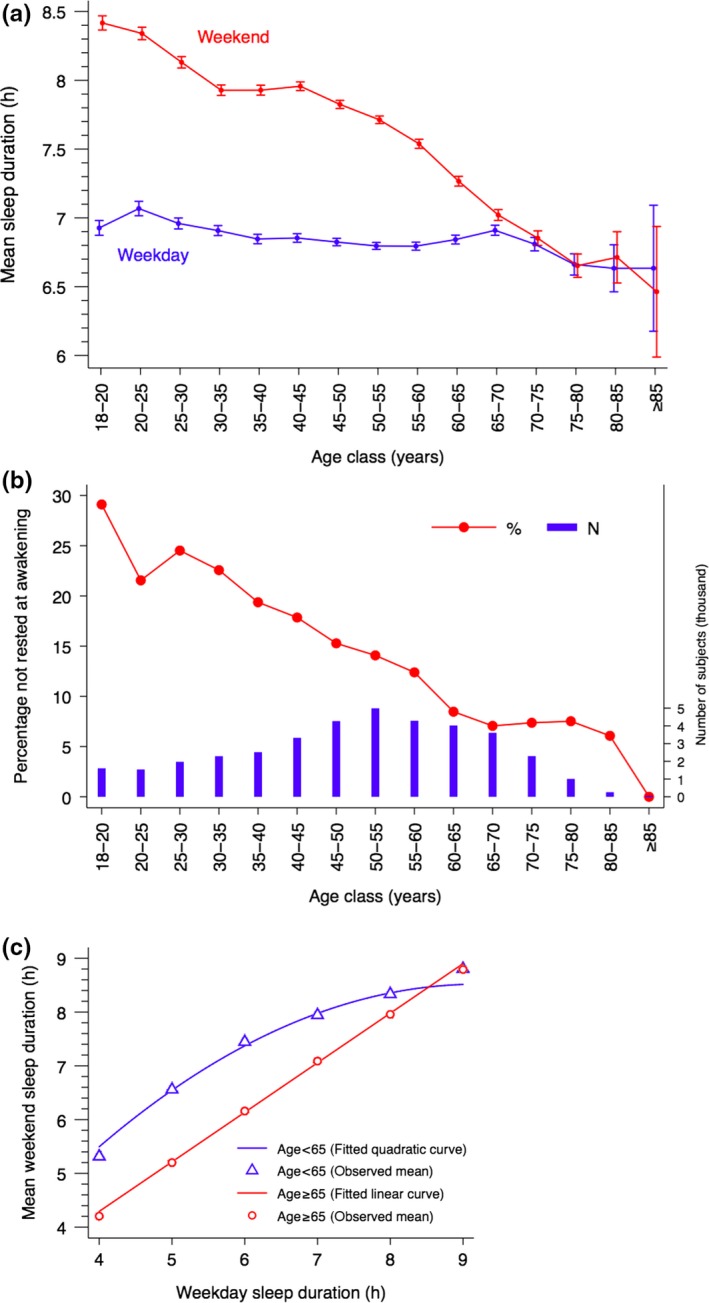
(a) Mean sleep duration (hr) and 95% confidence intervals (CIs) during weekdays and weekend stratified by age groups. (b) Percentage rating themselves as not being rested on awakening stratified by age group. Vertical bars represent the number of subjects in each class of age. (c) Mean weekend sleep duration across levels of weekday sleep duration stratified by age (<65 years, ≥65 years). A linear and a quadratic curve is fitted between the two sleep measures among older and younger subjects, respectively. Due to the high number of subjects and the low number of categories, the CIs are very small and not reported

3.2. Weekday/weekend sleep and mortality

The weekday and weekend sleep duration analysis shows that for age <65 years group sleeping 5 hr or less, both during weekdays and weekend (SS), was associated with a 65% higher mortality rate (HR 1.65; 95% CI 1.22–2.23) compared with those consistently sleeping 6 or 7 hr per night (Figure 4). A higher risk was also observed for individuals with consistently long sleep (LL; HR 1.25; 95% CI 1.05–1.50). The mortality rate of individuals with short sleep during weekdays, but medium or long sleep over weekends (SML), did not differ from the reference group rate. Among individuals above the age of 65 years, no association between sleep durations and mortality was found. When analysing both age groups together, the PH assumption for sleep durations was not met. The estimated time‐varying HRs for the SS and LL groups decreased with increasing age, while no interaction with the time‐scale was observed for the other three groups (Figure S1).

Figure 4.

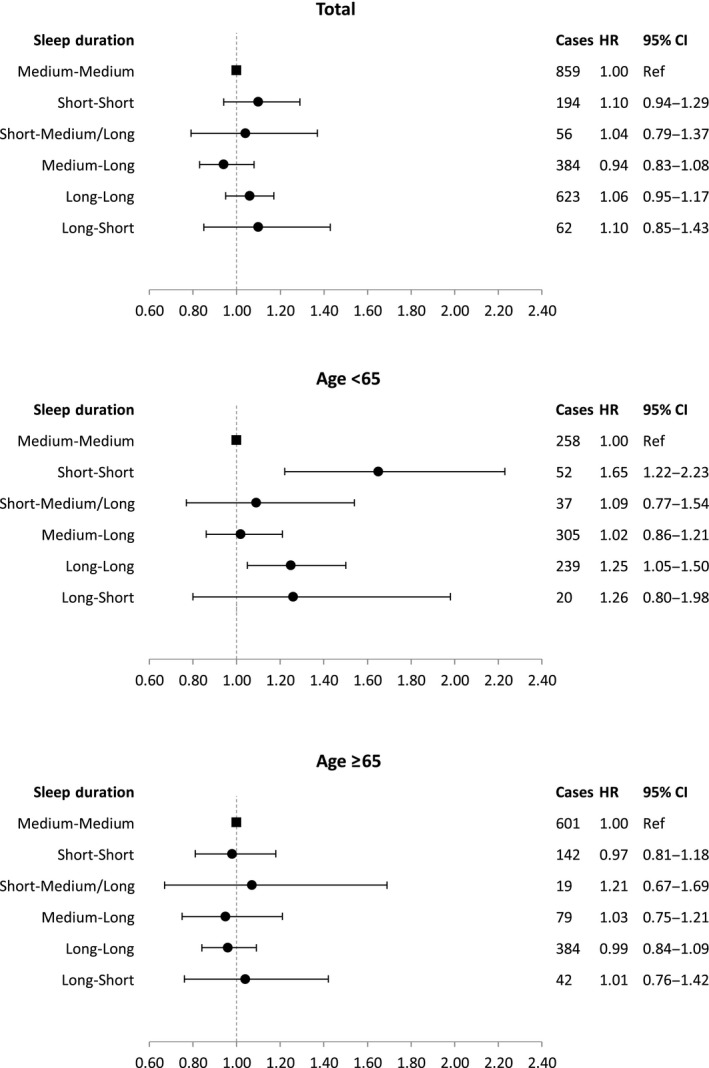
Forest plots showing multivariate Cox regression analysis of the association of weekday/weekend sleep duration groups with mortality – overall and stratified by age. The model was adjusted for sex, body mass index, smoking status, alcohol consumption, educational level, physical activity shift work and Charlson index

When we adjusted our models for sleep quality, snoring, self‐reported sleep medication use, napping, feeling rested at awakening, coffee intake, subjective health and high work demand, the results did not change appreciably. Because of missing values on the newly entered covariates, we lost 421 cases compared with the main model (Table S2).

3.3. Quantile regression

Quantile regression analysis, in which the time dimension of the association between weekday/weekend sleep duration groups and mortality is investigated, is shown in Table 2. Age‐adjusted models showed that at the 25th percentile of age at death subjects with constant short or constant long sleep died approximately 8 months earlier compared with subjects constantly sleeping 6–7 hr. The percentile difference in survival was −5.6 (95% CI −13.0, 1.7) and −5.3 (95% CI −10.1, −0.6) months at the median age at death, respectively. In the fully adjusted model, we observed a significant difference in survival only among short‐short sleepers at the 25th and 50th percentiles of age at death.

Table 2.

Quantile regression analysis for percentile differences (PD) in months in age at death across levels of weekday/weekend sleep duration groups in the Swedish National March Cohort

Sleep duration, hr per day	No. of deaths	Person‐years	25th PD, months		50th PD, months		75th PD, months
			PD	95% CI	PD	95% CI	PD	95% CI
Model 1a
Short‐short	323	19,310	−7.8	−15.4, −0.2	−5.6	−13.0, 1.7	−6.2	−12.7, 0.3
Short‐medium/long	85	18,356	−8.4	−21.3, 4.5	−7.8	−19.5, 4.0	−4.5	−17.1, 8.1
Medium‐medium	1,302	134,434	0.0	Reference	0.0	Reference	0.0	Reference
Medium‐long	503	186,408	5.5	−0.9, 12.0	5.6	−0.7, 11.9	6.5	−0.2, 13.2
Long‐long	918	115,555	−7.7	−13.1, −2.2	−5.3	−10.1, −0.6	−4.7	−9.4, 0.0
Long‐short	103	9,078	−3.9	−15.4, 7.6	−7.9	−17.8, 2.1	−9.6	−19.3, 0.0
Model 2b
Short‐short	194	12,565	−9.2	−18.2, −0.2	−9.7	−18.6, −0.8	−7.5	−16.5, 1.5
Short‐medium/long	56	14,435	−6.1	−19.4, 7.2	−3.4	−20.1, 13.2	−0.1	−15.5, 15.3
Medium‐medium	859	100,901	0.0	Reference	0.0	Reference	0.0	Reference
Medium‐long	384	154,640	3.4	−3.6, 10.5	2.7	−4.5, 9.9	5.4	−2.4, 13.2
Long‐long	623	89,484	−5.9	−12.1, 0.3	−3.6	−9.8, 2.5	−0.3	−6.0, 5.3
Long‐short	62	6,479	−3.6	−16.5, 9.3	−9.3	−22.3, 3.6	−8.2	−22.2, 5.8

CI, confidence interval; PD, percentile differences.

^aEstimates were obtained by fitting a Laplace regression on the 25th, 50th and 75th percentiles of age at death adjusted for age at baseline.

^bThe model was further adjusted for sex, BMI, smoking status, alcohol consumption, educational level, physical activity, shift work and Charlson index.

3.4. Sensitivity analyses

We observed no major changes when the first 2 years of follow‐up were excluded. Among subjects below the age of 65 years, short weekend sleep was associated with a 46% higher mortality rate (HR 1.46; 95% CI 1.08–1.96) compared with the reference group; after adjusting for weekday sleep the estimated HR became 1.34 (95% CI 0.91–1.98). Long weekend sleep, both in the younger and in the older group, as well as short weekend sleep in the older group were not associated with an increased mortality rate compared with the reference group. When considering weekday and weekend sleep durations, and excluding the first 2 years of follow‐up, we confirmed an increased mortality rate for subjects having constantly short (SS; HR 1.56; 95% CI 1.13–2.15) or constantly long sleep duration (LL; HR 1.23, 95% CI 1.02–1.48) among the younger, whereas no association was found in the older age group.

Performing multiple imputations on missing covariates did not alter the results. The estimates found with the imputed data are comparable to those previously reported in the forest plots. Short weekend sleep was associated with mortality only among the youngest (HR 1.48; 95% CI 1.16–1.87), and the estimate remained significant even after adjusting for sleep duration during the week (HR 1.41; 95% CI 1.03–1.92). Long weekend sleep duration was not associated with mortality at any age. Sleeping constantly short or constantly long increased the mortality rate in subjects below the age of 65 years (HR 1.58; 95% CI 1.23–2.04 and HR 1.24, 95% CI 1.07–1.45, respectively). Among the older group, sleeping short during the week and medium or long (SML) during the weekend was associated with a marginally significant higher mortality rate compared with the reference group (HR 1.38; 95% CI 1.00–1.89). Due to the high amount of missingness in the variable occupational status, it was not included in the main analysis. However, it was entered in the model with multiple imputation; the results remained stable.

4. DISCUSSION

In this large prospective cohort study we found that short, but not long, weekend sleep was associated with an increased mortality risk among subjects below the age of 65 years. Short sleep on both weekdays and weekends, as well as consistently long sleep, were associated with higher mortality risk. Extended weekend sleep after weekday short sleep was not associated with increased mortality. No significant associations between sleep duration and death were observed in those aged 65 years or above.

The lack of association between long weekend sleep duration and mortality in the present paper does not appear to align with the meta‐analyses finding a U‐shaped (Cappuccio et al., 2010; Gallicchio & Kalesan, 2009; Silva et al., 2016) or J‐shaped (Liu et al., 2017) relationship. One reason for the difference is probably that previous work has focused on weekday sleep only. The two types of sleep may have quite different causes and consequences, and they clearly differ in duration, with the difference strongly dependent on age. Some of these issues are discussed below.

The link between sleep duration and mortality seems to be easier to understand when considering the analysis of the joint effects of weekday and weekend sleep. Thus, the results imply that short (weekday) sleep is not a risk factor for mortality if it is combined with a medium or long weekend sleep. This suggests that short weekday sleep may be compensated for during the weekend, and that this has implications for mortality. Similar observations have been made for obesity in children (Wing et al., 2009), and hypertension in adults (Hwangbo et al., 2013). An alternative, or complementary, interpretation is that the long weekend sleep after short weekday sleep simply demonstrates a healthy sleep pattern that produces longer sleep when factors restricting sleep duration are removed. The role of extended weekend sleep suggests that some of the inconsistencies in previous results (Cappuccio et al., 2010; Gallicchio & Kalesan, 2009; Kurina et al., 2013; Silva et al., 2016) could be, at least partly, due to a lack of consideration of compensatory sleep.

This interpretation of results on sleep duration in terms of sufficient or insufficient recovery or compensatory sleep is speculative, however. It needs confirmation in studies that link changes in sleep duration between weekend and weekday in a longitudinal approach across many weeks. This would tell us if weekday sleep reduction is linked to weekend sleep extensions and, ideally, the data would be able to extend our conclusion if linked to subsequent mortality. With the present approach, with merely one measure of sleep at baseline, we cannot be sure that longer weekend sleep is a true compensation, rather than appetitive behaviour when the sleep opportunity is increased during the weekend.

Notwithstanding the observation of compensatory sleep, the results also showed a relatively high correlation between weekday and weekend sleep. Thus, most of the long sleepers during weekdays are long sleepers also during weekends. However, we observed a different trend across ages. The older group had a constant sleeping pattern over weekdays and days off, no matter how many hours they slept during the week, whereas the younger had a quadratic relationship. The mean weekend sleep seemed to be 1 hr or more longer among subjects with short sleep, and almost equal among subjects with long sleep. The reason may be that there is no need for compensatory sleep at sleep durations of 8 hr and above, but this needs empirical support from studies with another design.

The gradual reduction of weekend sleep duration as subjects become older might be the reason behind the reduction in HR with increasing age found in our previous study on the same cohort (Akerstedt et al., 2017), and confirmed here. A similar age effect was found for short sleep in relation to hypertension, CVD and obesity in the meta‐analysis by Itani et al. (2017). For the younger participants in the present study, the difference between weekday and weekend sleep was large, probably reflecting a relatively consistent sleep deficit that may accumulate and link to mortality, if not sufficiently compensated for. For the older individuals, weekday and weekend sleep were equally short. This leaves the impression that sleep in that age group has become sufficient. The notion of sufficient sleep among older individuals is supported by the decrease across age groups of the percentage of individuals who feel “not rested” after sleep. This could be an effect of biological aging or of reduced demands in life (work, family, etc.). This issue needs further research.

The mechanism behind the U‐shaped relationship between mortality and sleep duration has been discussed in many previous studies with no convincing conclusions. Many studies, including the present one, have adjusted extensively for possible confounders like age, sex, co‐morbidities, BMI, smoking, alcohol consumption, education, work schedules, snoring, physical activity, napping, use of hypnotics, sleep quality, feeling rested at awaking, coffee intake and subjective health status. Grandner, Patel, Gehrman, Perlis, and Pack (2010) argued that low‐grade inflammation, increased levels of ghrelin, increased lipid levels, or insulin resistance could be involved in this process. Nevertheless, the mechanism still defies understanding.

The strengths of our study include its large size, its prospective design, the length and the completeness of the follow‐up, ascertained through linkages to nationwide registers, the high quality of the baseline data guaranteed by a low level of missing values, and the availability of two separate questions investigating sleep habits during weekdays and weekends/days off.

Among the limitations there is the possibility that individuals not working may have misinterpreted the term “day off”; however, the formulation in the first question on sleep duration emphasizes “workday”/“weekday”. This should have led to the interpretation of the second question as referring to weekend by those not working. Another limitation is that sleep duration was self‐reported and assessed only at baseline; therefore, it is not possible to detect changes in sleep habits over time among the subjects included in the study. Thus, for example, the pattern of sleep duration in different age groups presented in the Results section suggests that one should expect a reduction in weekend sleep during the follow‐up period. Changes in sleep duration over time might occur also due to health, work or life cycle changes occurring after the enrollment into the study. The use of repeated measurements through follow‐up questionnaires would have helped keeping track of sleep habits changes.

Late rising in older individuals was shown to be related to a higher risk of dementia (Bokenberger et al., 2017). Bedtimes and times of rising might also affect mortality, and factors like diurnal type may carry health implications (Roenneberg & Merrow, 2016); however, none of these factors were available in the present material. In addition, due to the low number of subjects in the categories SML and LS, we could not differentiate the effect between short sleep during the week and medium or long sleep during the weekend, or between the groups with medium‐short, long‐short and long‐medium sleep during weekdays and weekend. Finally, the present analysis was of a relatively traditional type with a priori determined combinations of weekday and weekend sleep patterns. There is a possibility that other approaches, for example, cluster analysis, would have provided other groups of sleep patterns. This may be an interesting approach for future studies.

In conclusion, our findings indicate that mortality is increased when both weekday and weekend sleep are short, or when both are long in subjects below the age of 65 years. However, when weekend sleep is extended after short weekday sleep no association with mortality is seen. We suggest that this may reflect positive effects of compensatory sleep. However, this issue needs to be addressed in longitudinal studies of changes in sleep duration and their link to mortality.

DISCLOSURE STATEMENT

No authors have declared any conflicts of interest.

Supporting information

 

Åkerstedt T, Ghilotti F, Grotta A, et al. Sleep duration and mortality – Does weekend sleep matter?. J Sleep Res. 2019;28:e12712 10.1111/jsr.12712