Fragmentation mediates thermal habitat choice in ciliate microcosms

Abstract

Habitat fragmentation is expected to reduce dispersal movements among patches as a result of increased inter-patch distances. Furthermore, since habitat fragmentation is expected to raise the costs of moving among patches in the landscape, it should hamper the ability or tendency of organisms to perform informed dispersal decisions. Here, we used microcosms of the ciliate Tetrahymena thermophila to test experimentally whether habitat fragmentation, manipulated through the length of corridors connecting patches differing in temperature, affects habitat choice. We showed that a twofold increase of inter-patch distance can as expected hamper the ability of organisms to choose their habitat at immigration. Interestingly, it also increased their habitat choice at emigration, suggesting that organisms become choosier in their decision to either stay or leave their patch when obtaining information about neighbouring patches gets harder. This study points out that habitat fragmentation might affect not only dispersal rate but also the level of non-randomness of dispersal, with emigration and immigration decisions differently affected. These consequences of fragmentation might considerably modify ecological and evolutionary dynamics of populations facing environmental changes.

1. Introduction

Natural ecosystems are increasingly converted into agricultural or urban areas for human activities [1,2], turning landscapes into smaller and more distant patches [3,4]. Beyond overall habitat loss, the resulting habitat fragmentation is expected to reduce dispersal movements among patches as a result of increased inter-patch distances [1,4–6]. Dispersal plays a major role in ecological and evolutionary dynamics, in particular by reducing extinction risks through recolonization of empty patches or mitigating the influence of genetic drift [7–13].

Habitat fragmentation and the resulting increased inter-patch distance are expected to lessen dispersal rates because of amplified costs of movement [1,4–6]. Numerous empirical and theoretical studies indeed showed that fragmentation, and hence increased time and/or energy spent during the dispersal movement, selects for a reduced tendency to disperse (e.g. [14–17]; reviewed in [4]). Additionally, fragmentation might lead to reduced dispersal through phenotypic plasticity when organisms reduce their probability to engage or complete a dispersal movement through the matrix [5,17]. Dispersal is indeed increasingly recognized as an informed process in which organisms adjust their decision to engage in a dispersal movement depending on a variety of environmental factors (context-dependent dispersal) and on their own condition (phenotype-dependent dispersal [9,12,18–20]).

Beyond costs of movement per se, dispersal may also incur costs associated with the decision to stay in or join a given habitat. For instance, remaining in a habitat lacking sufficient mating partners, or settling in an environmental context not suitable for an organism given its phenotype are important sources of dispersal costs [19–21]. To mitigate these costs, organisms may adjust their decision to stay or disperse depending on the local environmental context, and then choose among neighbouring patches the one that better suits their phenotype [13,19,20,22]. This process, named habitat choice, has important implications for a wide range of ecological and evolutionary dynamics such as population genetic structure, local adaptation, the evolution of ecological specialization or metapopulation response to habitat fragmentation [4,13,19,20,22–28]. In contrast to random dispersal, informed dispersal leading to habitat choice may especially favour rather than hinder local adaptation even with high dispersal rates [19,22,29], a prediction that has recently been experimentally demonstrated [13].

Informed dispersal decisions during the process of habitat choice require organisms to obtain information about the landscape [18,30]. To do so, organisms may, for instance, perform prospective movements through the landscape, thus gathering information about the presence and density of conspecifics or predators, the quantity of resources or other biotic or abiotic characteristics of the habitats [18,31,32]. However, since habitat fragmentation is expected to raise the costs of moving among patches in the landscape, it should hamper prospective movements and hence the ability of organisms to perform informed dispersal decisions [4,19]. Given how much the effects of dispersal for ecological and evolutionary dynamics differ between habitat choice and random dispersal [13,19,20,22,24,25,27], it is important to quantify the consequences of habitat fragmentation for the ability of organisms to maintain habitat choice.

Here, we used microcosms of a protist, Tetrahymena thermophila, to test experimentally whether habitat fragmentation affects dispersal rate and the ability to perform habitat choice during dispersal. This species is able to adjust its dispersal decisions depending on temperature [13,24], a strategy linked to thermal specialization [24]. We used dispersal systems in which patches differed in their temperature to quantify habitat choice as the ability to adjust dispersal depending on expected fitness, i.e. the growth rate a genotype would reach if choosing a given temperature. To test the consequences of habitat fragmentation on dispersal, we manipulated the length of corridors separating patches with two treatments: a standard corridor length as used in previous studies providing evidence for habitat choice in this species [13,24], and a fragmented treatment with corridors twice as long. We replicated this experiment with six isogenic clonal strains (hereafter called ‘genotypes') kept isolated. First, we predicted that increased inter-patch distance should increase the costs of dispersal and therefore reduce effective dispersal rate. Second, we expected fragmentation to hamper the ability of organisms to perform informed decisions during dispersal and therefore decrease temperature-dependent habitat choice at immigration. Whether such increased costs of dispersal also affect the departure decision (habitat choice at emigration) is an open question.

2. Methods

(a). Culture conditions and population density quantification

The species T. thermophila is a ciliated protist naturally living in North American freshwater ponds and streams [33,34]. In the present experiment, we used six genotypes originally sampled from different locations (D3, D4, D5, D11, D13 and D17; see [24,35,36]), which reproduce clonally in our culture conditions [37,38]. Five of these six genotypes were previously characterized for habitat choice [13,24]: all performed habitat choice at emigration, and we chose two genotypes that also performed habitat choice at immigration (D3 and D11), and three that did not (D4, D13 and D17). We furthermore added a sixth genotype (D5) for which habitat choice was unknown. All cultures and experiments were performed separately for each genotype. Cells were maintained in axenic rich liquid growth media (0.6% Difco proteose peptone, 0.06% Difco yeast extract) at 23°C [13,19,39–43]. All manipulations were performed in sterile conditions under a laminar flow hood to avoid any contamination of cultures by environmental bacteria or fungi.

We used a standardized procedure to measure cell density in T. thermophila cultures: from each culture, we measured five samples (10 µl each) pipetted into one chamber of a multichambered counting slide (Kima precision cell 301890), and took digital pictures under dark-field microscopy [13,19,39–43]. Population density in cultures was quantified based on an automatic analysis of pictures using the IMAGEJ software (v. 1.47, National Institutes of Health, USA, http://imagej.nih.gov/ij; [44]).

(b). Habitat choice experiment

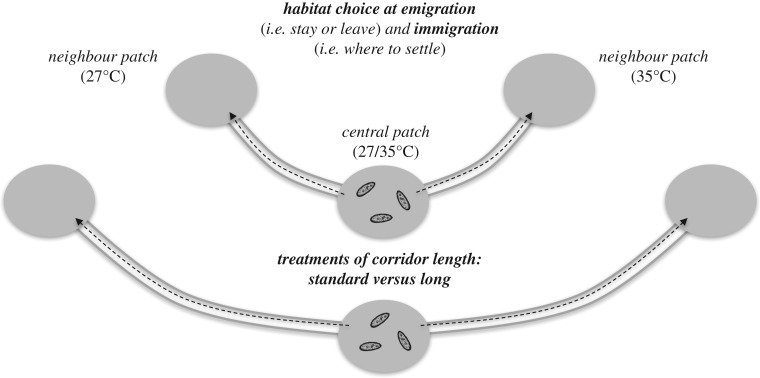
To quantify dispersal rate and habitat choice, we used dispersal systems (figure 1) consisting of three linearly connected patches (5 ml standard Eppendorf tubes), connected by corridors (4 mm internal diameter silicon tube; [13,24,40]). We manipulated habitat fragmentation by using corridors separating habitat patches either of the standard length used in previous studies (hereafter named ‘standard corridors'; 5 cm long, meaning approximately 2500 times the size of cells; [13,24]) or twice as long in the case of the fragmented treatment (figure 1). Corridors separating habitat patches contained no resources (i.e. filled with water), to generate a harsh matrix [45,46]. In a previous experiment, we showed that this method allows maintaining matrix harshness for more than 5 h [45]. We inoculated cells in the central patch of the three-patch systems at standard density (approx. 40 000 cells; six replicates per genotype for each corridor length and central temperature; replicates perfectly randomized among days, with always the same experimenters for each task, standardized procedures to take digital pictures and automatic analysis of these pictures; see above). The central temperature was set at either 27°C or 35°C, connected to one 27°C patch and one 35°C patch, defined according to previous studies to maximize fitness differences between the tested temperatures [13,24]. Temperature in patches was manipulated using dry bath systems (H2O3 Dry Bath Incubator; Coyote Bioscience) placed in incubators (Sanyo MI-554), allowing fine control of the two different temperature levels [13,24].

Figure 1.

Experimental design used to quantify the effects of habitat fragmentation (manipulated through the length of corridors) on temperature-dependent habitat choice at emigration (i.e. stay or leave) and immigration (i.e. where to settle). Six replicates per genotype for each corridor length and central temperature were performed.

After 1 h acclimation in the central patch, corridors were opened for 6 h to allow cells to choose whether to stay or leave the start patch, and if dispersing in which of the two neighbouring patches to settle [24]. Tetrahymena thermophila cells, as most ciliates, are covered with cilia providing high mobility to catch food and move from one location to another [47]. Average movement velocity of T. thermophila cells during routine movements within a patch was about 155 µm s⁻¹ in this study, meaning that cells would theoretically be able to cross the 5 cm long corridors in less than 5 min (assuming unrealistic perfectly straight movements). Cells should thus be able to prospect patches during the timing used in our experiment, as expected given the existence of habitat choice at immigration in this species [13,24]. Importantly however, less than 3 hours is usually not enough to observe dispersers in these systems (SJ 2018, personal observation), and population sizes in this experiment did not homogenize even after 6 h, which would be the result of a very high level of movement. Furthermore, we previously found that the usually observed correlation between cell velocity and dispersal rate (e.g. [36,48,49]) vanishes when harsh corridors are used (i.e. no nutrients; [45]). Dispersal in this experimental system is therefore not a simple diffusion process mediated only by movement ability, but should involve active behavioural decisions.

After 6 h dispersal, we quantified the numbers of cells that remained in the central patch (i.e. residents) and that reached each of the neighbouring patches (dispersers) using the standard procedure described above. Dispersal rate was computed as the proportion of cells in the neighbouring patches after 6 h: N_dispersers/(N_residents + N_dispersers). This species shows a latency time before growth initiation [at 27°C: mean ± s.e. = 17.34 ± 1.80 h; at 35°C: 9.97 ± 2.49 h; [24]), meaning that population growth is negligible during the 6 h of dispersal assay and thus does not affect estimates of dispersal rates as previously shown [36].

(c). Habitat choice characterization

We quantified habitat choice as the relationship between dispersal decisions and the expected fitness at each temperature, following [24]. This means that habitat choice describes how dispersal rate varies depending on the fitness that a genotype would reach if choosing a given temperature [24]. Habitat choice at emigration quantifies how genotypes adjust the decision to remain in the central patch depending on temperature (including the possibility to return after prospecting neighbouring patches), and habitat choice at immigration represents the decision of where to settle if leaving the central patch. To test for effects of corridor length on habitat choice, we here quantified habitat choice of genotypes separately for each fragmentation treatment.

First to quantify the expected fitness, we reconstructed the thermal niche of the six genotypes by quantifying population growth rate from a small number of cells (approx. 100) along a thermal gradient (from 11°C to 39°C). Cells were inoculated in 96-well plates (250 µl wells) filled with growth media, with five replicates per genotype. Population growth was quantified through absorbance measurements at 550 nm performed twice a day until populations reached their maximal density (two to three weeks; greater than 30 generations), using a microplate reader (Synergy H1, BioTek). Population growth rate at each temperature was computed as the maximum slope of population growth using the gcfit function (grofit R-package). The thermal niche of each genotype was computed from the relationship between growth rate and temperature fitted with a generalized additive model, a non-parametric statistical model that does not require any assumption regarding the shape of the curve (gam R-package [50]; see also [24]). From the thermal niches of genotypes, we computed thermal optima as the temperature at maximum growth rate. The expected fitness is defined as the growth rate at each temperature relative to the performance at thermal optimum (i.e. maximal performance over the thermal niche). The expected fitness in the most optimal of the two thermal conditions differed among genotypes, ranging from 0.43 to 0.77 (mean ± s.e. = 0.65 ± 0.27) in the optimal temperatures, and from 0.21 to 0.45 (mean ± s.e. = 0.31 ± 0.13) in the suboptimal temperatures.

To quantify habitat choice at emigration and immigration for each genotype, we quantified the relationship between dispersal decisions (proportion of cells that either stayed in the central patch or joined a neighbouring patch) and the expected fitness in each habitat (two values of growth rate per genotype corresponding to the two temperatures tested: 27°C and 35°C; see above). The expected fitness $\hspace{0.17em}\overline{f}$ in the start patch was used for habitat choice at emigration and expected fitness in neighbouring patches was used for immigration, with habitat choice estimated separately for emigration and immigration.

At the emigration step, we fitted a simple model in which the proportion of cells that left the central patch (D_e) depend on expected fitness $\hspace{0.17em}\overline{f}$ at the central patch, habitat choice ability at emigration (h_e) and dispersal propensity (Dp_e; the tendency to disperse at $\hspace{0.17em}\overline{f}=0.5$) using the function

$$D_e=\hspace{0.17em}D{p}_e-\hspace{0.17em}{h}_e\hspace{0.17em}(\hspace{0.17em}\hspace{0.17em}\overline{f}-0.5),$$

meaning that

$$h_e=\frac{D_{\hspace{0.17em}pe}-\hspace{0.17em}{D}_e}{\hspace{0.17em}f-\hspace{0.17em}0.5}.$$

We fitted the above model using nonlinear regression with the nls function from the stats R-package, with 12 values of D_e for each genotype and fragmentation treatment. The input variables of the model were $\overline{f}$ and D_e, while h_e and Dp_e were estimated using the nls function [24]. h_e is positive when cells preferentially stay at the most optimal temperature, and is negative if cells tend to leave their optimal temperature.

At the immigration step, two proportions of cells joining the neighbouring patches were quantified for each three-patch dispersal system: the proportion of dispersers that moved toward the 27°C patch (D_{i 27°C}) and the proportion that moved toward the 35°C patch (D_i35°C). This resulted in 12 pairs of D_i values for each genotype. Because these two proportions are dependent (they sum to 1), we subtracted the following equations describing habitat choice at immigration (similar to the equation at emigration, with one for immigration towards 27°C and one towards 35°C):

$$D_{i\hspace{0.17em}{27}^{\circ }\mathrm{C}}=\hspace{0.17em}D{p}_i+\hspace{0.17em}{h}_i\hspace{0.17em}({\hspace{0.17em}\overline{f}}_{{27}^{\circ }\mathrm{C}}-0.5)$$

and

$$D_{i\hspace{0.17em}{35}^{\circ }\mathrm{C}}=\hspace{0.17em}D{p}_i+\hspace{0.17em}{h}_i\hspace{0.17em}({\hspace{0.17em}\overline{f}}_{{35}^{\circ }\mathrm{C}}-0.5),$$

leading to

$$D_{i\hspace{0.17em}{27}^{\circ }\mathrm{C}}-\hspace{0.17em}{D}_{i\hspace{0.17em}{35}^{\circ }\mathrm{C}}=\hspace{0.17em}{h}_i\hspace{0.17em}({\hspace{0.17em}\overline{f}}_{{27}^{\circ }\mathrm{C}}-\hspace{0.17em}{\hspace{0.17em}\overline{f}}_{{35}^{\circ }\mathrm{C}})$$

and therefore

$$h_i=\frac{D_{i\hspace{0.17em}{27}^{\circ }\mathrm{C}}-\hspace{0.17em}{D}_{i\hspace{0.17em}{35}^{\circ }\mathrm{C}}}{{\overline{f}}_{{27}^{\circ }\mathrm{C}}-{\overline{f}}_{{35}^{\circ }\mathrm{C}}}.$$

As for emigration, the input variables of the model were $\hspace{0.17em}{\overline{f}}_{{27}^{\circ }\mathrm{C}}$, $\hspace{0.17em}{\overline{f}}_{{35}^{\circ }\mathrm{C}}$, $D_{i\hspace{0.17em}{27}^{\circ }\mathrm{C}}$ and $D_{i\hspace{0.17em}{35}^{\circ }\mathrm{C}}$, while h_i was estimated using the nls function [24]. Note that subtracting the habitat choice equations at immigration allows the parameter Dp_i to be removed from the resulting equation, keeping dispersal propensity only as the tendency of a genotype to emigrate (i.e. Dp_e). As for habitat choice at emigration, h_i is positive when cells preferentially join the neighbouring patch with the most optimal temperature, and is negative if cells tend to join the patch at suboptimal temperature.

The two metrics of habitat choice (h_e and h_i) computed vary between −1 and 1: h = 0 for random dispersal, h > 0 for a preference for optimal habitats (i.e. where expected fitness is higher) and h < 0 for a preference for suboptimal habitats (i.e. where expected fitness is lower). In addition, we computed the absolute value of these habitat choice metrics to denote the intensity of habitat choice ability, irrespective of its direction or adaptive significance.

(d). Statistical analyses

First, to test whether the habitat fragmentation treatment affects effective dispersal rate, we used a linear model with dispersal rate as a dependent variable, habitat fragmentation treatment, genotype and the genotype by fragmentation interaction as explanatory factors, and central temperature as a covariate. Analyses of fragmentation effects within each genotype were performed using lsmeans R-package [51]. Second, we tested whether genotypes perform temperature-dependent habitat choice at emigration and immigration by performing Student's t-tests on the habitat choice metrics (h_e and h_i) compared to 0 (i.e. random dispersal hypothesis). Third, we quantified the effects of increased inter-patch distance on habitat choice metrics by performing Student's t-tests between standard and long corridors.

3. Results

Dispersal rate significantly differed among genotypes (F_5,131 = 9.54; p < 0.001), and corridor length affected dispersal rate differently among genotypes (corridor length by genotype interaction: F_5,131 = 6.26; p < 0.001; main corridor effect: F_1,131 = 0.30; p = 0.58). Analyses within each genotype revealed that two genotypes significantly reduced their dispersal rate when facing longer corridors (D4: estimate ± s.e. = −0.16 ± 0.04; t = 4.19; p < 0.001; D13: estimate ± s.e. = −0.25 ± 0.04; t = 6.49; p < 0.001; all other p > 0.30).

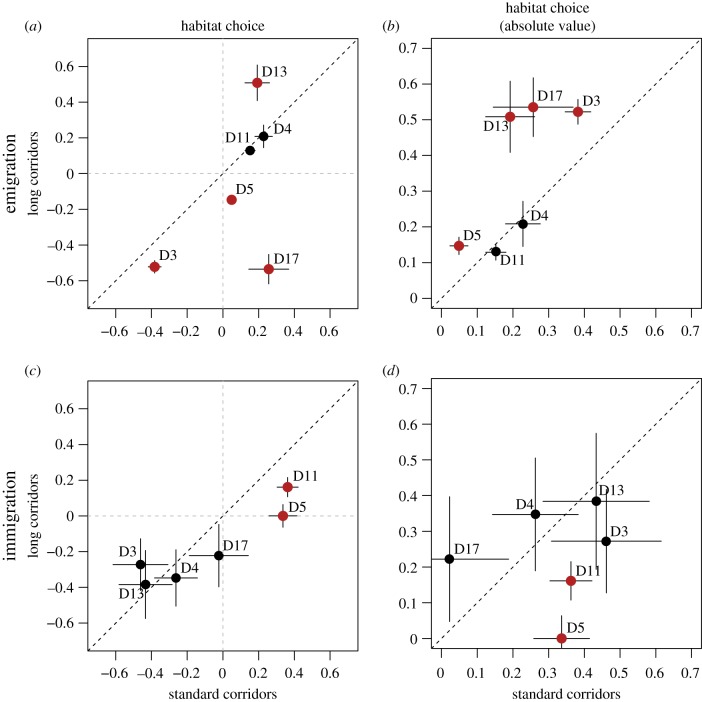
We found evidence for temperature-dependent habitat choice at emigration in five out of six tested genotypes (table 1a). All the genotypes used here except one showed habitat preference for the most optimal temperature (positive habitat choice values; table 1a). Importantly, comparing habitat choice metrics from this study to previously reported values [24] revealed that habitat choice metrics as quantified here are largely repeatable. Doubling corridor length resulted in significant changes in habitat choice at emigration in four out of six genotypes (fragmentation effect in table 1a; red dots in figure 2a,b). Absolute values of habitat choice are furthermore reported in figure 2b to illustrate habitat choice ability irrespective of its adaptive significance. These results show that four genotypes increased their habitat choice (red dots are above the dashed line in figure 2b), meaning that they became choosier at emigration when facing longer corridors. This resulted in three genotypes with a preference for suboptimal habitats in the fragmented treatment, while one genotype with a preference for the more optimal habitat showed increased choosiness in the fragmented treatment (figure 2a).

Table 1.

Effect of corridor length on habitat choice for each genotype at emigration (a) and immigration (b) t-statistics compared to 0 (i.e. random dispersal hypothesis) are shown within each corridor length treatment, and statistics from Student's t-test between standard and long corridors are provided to test for fragmentation effects on habitat choice (N = 12 for each category; ‘.' for p < 0.1; ‘*’ for p < 0.05; ‘**' for p < 0.01; ‘***’ for p < 0.001).

	standard corridors				long corridors				fragmentation effect
genotype	he	SD he	t	p-value	he	SD he	t	p-value	t	p-value
(a) emigration
D3	−0.382	0.119	−10.681	<0.001***	−0.522	0.115	−15.099	<0.001***	2.806	0.011*
D4	0.228	0.161	4.682	0.001***	0.208	0.209	3.300	0.008**	0.252	0.804
D5	0.049	0.084	1.938	0.081.	−0.147	0.080	−6.090	<0.001***	5.604	<0.001***
D11	0.152	0.094	5.382	<0.001***	0.129	0.073	5.863	<0.001***	0.641	0.529
D13	0.192	0.227	2.803	0.019*	0.508	0.330	5.102	<0.001***	2.617	0.018*
D17	0.257	0.371	2.297	0.044*	−0.535	0.273	−6.503	<0.001***	5.703	<0.001***

	hi	SD hi	t	p-value	hi	SD hi	t	p-value	t	p-value
(b) immigration
D3	−0.462	0.721	−2.124	0.060	−0.272	0.678	−1.332	0.213	−0.900	0.373
D4	−0.263	0.562	−1.550	0.152	−0.347	0.74	−1.556	0.151	0.424	0.674
D5	0.336	0.366	3.050	0.012*	0	0.297	0.002	0.998	−3.344	0.002**
D11	0.362	0.276	4.362	0.001**	0.161	0.252	2.124	0.060.	−2.523	0.016*
D13	−0.433	0.697	−2.060	0.066.	−0.384	0.892	−1.427	0.184	−0.203	0.804
D17	−0.023	0.774	−0.098	0.924	−0.222	0.819	−0.900	0.389	0.828	0.412

Figure 2.

Fragmentation affects habitat choice at emigration (a,b) and immigration (c,d). Estimates ± s.e. of habitat choice under long corridors (y-axis) versus standard corridors (x-axis) are shown. Each dot represents one genotype, and s.e. of estimated habitat choice values are illustrated by error bars (table 1). Habitat choice estimates (a & c) vary between −1 and 1: h = 0 for random dispersal (illustrated by grey dashed lines), h > 0 for a preference for optimal habitats and h < 0 for a preference for suboptimal habitats. The black dashed line illustrates the null hypothesis where fragmentation does not affect habitat choice. In addition to basic habitat choice estimates, absolute habitat choice values are provided to illustrate habitat choice ability irrespective of its adaptive value (b,d): dots below the line correspond to a decrease in habitat choice with fragmentation, while genotypes increasing habitat choice with fragmentation are above the line. Red dots are genotypes significantly affected by fragmentation (table 1). (Online version in colour.)

At the immigration step, two genotypes exhibited significant temperature-dependent habitat choice when using standard corridors, preferring to join the most optimal temperatures (i.e. positive habitat choice values; figure 2c and table 1b). Two other genotypes furthermore showed a tendency for negative habitat choice, i.e. preferring suboptimal temperatures as previously shown [24]. As for habitat choice at emigration, the values of habitat choice at immigration observed in this study largely matches those observed previously [24]. When facing longer corridors however, habitat choice mostly vanished, with the two genotypes that performed positive habitat choice in standard conditions showing a significant decrease of their habitat choice degree (red dots are below the dashed line in figure 2c,d; fragmentation effect in table 1b).

4. Discussion

Given the role of dispersal in ecological and evolutionary dynamics, reaching a precise understanding of the consequences of the increasing levels of habitat fragmentation for dispersal movements is a crucial challenge [4,52–56]. Here, we tested experimentally whether habitat choice is affected by an increase of inter-patch distance, a dimension of habitat fragmentation that can mediate its ecological and evolutionary consequences.

Besides an expected reduction of dispersal rate in two out of six genotypes, we found that increased inter-patch distance led to an overall increase of habitat choice at emigration (figure 2b). This result suggests that genotypes become choosier in their decision to either stay or leave their patch when obtaining information about neighbouring patches gets harder. Interestingly, our results suggest that this increased habitat choice at emigration can come with suboptimal decisions: three of the four genotypes that increased their choosiness with fragmentation showed a preference for the suboptimal temperature over the most optimal one (two even switched from preferring the better habitat to preferring the worse habitat; figure 2a). Suboptimal habitat choice has recently been found to be associated with thermal generalism in this species, a strategy that might help generalists to avoid competitive exclusion by fitter specialists [24]. Starting to prefer suboptimal habitats when information becomes more limited might also denote maladaptive strategies [57]. While reduced information availability would limit informed dispersal decisions at immigration as demonstrated here, how it affects the way organisms choose whether to stay or leave their local patch is an important and new question arising from our experiment.

As expected, we found that increasing inter-patch distance can limit habitat choice at immigration (i.e. choosing where to settle during the dispersal movement). The genotypes that performed habitat choice at immigration with standard corridors indeed did not significantly differ from random dispersal when facing twice longer corridors (figure 2b and table 1b). Performing habitat choice at immigration requires efficient information acquisition mechanisms, such as a developed locomotion apparatus coupled with the ability to assess the social and environmental context during prospective movements. In addition to prospecting ability, habitat choice in this species might consist in following environmental gradients through taxic responses (reviewed in [47]). Raising the costs of movements among patches should limit prospective ability and limit the availability of cues about neighbouring habitat patches (e.g. here temperature gradient), therefore decreasing the availability of information required for habitat choice. In addition to the well-considered decrease of dispersal rate following habitat fragmentation, such decrease of habitat choice toward more random movement might fasten extinction dynamics. Whether information acquisition mechanisms involving long distance detection or immigrant-based social information might be less affected than prospecting-based decisions [18,19,58,59], and might therefore be favoured in fragmented landscapes [19], is an interesting trail for future investigation.

Fragmentation had different effects on habitat choice at emigration and immigration in our study, pointing out how dissimilar these two steps of dispersal are (reviewed in [18,19]), especially in terms of information sources (local versus regional) and mechanisms of information acquisition (e.g. prospection, taxic responses). Accordingly, emigration and immigration habitat choice did not significantly correlate in this study, neither with standard nor long corridors (t = 0.67; d.f. = 5; p = 0.54; t = −0.22; d.f. = 5; p = 0.84; resp.; electronic supplementary material, figure S1), the values of habitat choice quantified being highly repeatable (see Results). Furthermore, while only one genotype over the six tested here under standard conditions showed a preference for suboptimal habitats at emigration, four genotypes present negative habitat choice value (although not significant) at immigration (electronic supplementary material, figure S1 and table 1).

The consequences of fragmentation on dispersal decisions might occur through different mechanisms whose consequences for ecological and evolutionary dynamics differ. Especially, a decreased dispersal rate might result from selective pressures against dispersal, as repeatedly suggested ([14,17,60,61]; reviewed in [4]), or from phenotypic plasticity, i.e. when organisms reduce their tendency to engage in costly dispersal movements or increase their return rate when engaging in a fragmented matrix [5]. Furthermore, reduced propensity or ability to cross longer corridors should result in limited habitat choice at immigration, as illustrated here, together with fewer individuals reaching neighbouring patches. Interestingly, the consequences of habitat choice and its context-dependence should depend on the mechanisms underlying these dispersal decisions. Three main categories of habitat choice have been advanced: natal imprinting, direct genetic habitat choice, and matching habitat choice [62]. All three are expected to affect ecological and evolutionary dynamics compared to random dispersal, for instance promoting population divergence and local adaptation [13,19,20,22]. However, they also differ in their expected consequences, with for instance genetic preference that should favour niche conservatism, while matching habitat choice may foster adaptation to niche margins and thus promote niche evolution [23]. In T. thermophila, thermal specialists have been found to prefer habitats where their performance is maximized [24]. Generalists, however, prefer fundamentally suboptimal habitats, supposedly to escape competition with specialists [24]. This preference occurs even in the absence of actual competition with specialists, suggesting that habitat choice might involve a direct genetic preference, and not matching habitat choice or imprinting where a match between preference and realized fitness is expected. Distinguishing between habitat choice mechanisms is a major empirical challenge that should deserve more attention given their importance for ecological and evolutionary dynamics [13,19,20,26,28,62].

Intraspecific variability in habitat choice preferences linked to thermal niche specialization has recently been found in T. thermophila [24]. In addition, we here provided experimental evidence for intraspecific variability in the effects of fragmentation on dispersal rate and habitat choice. Such variability in fragmentation effects on dispersal might be explained by variability among genotypes in the impact of corridor length on movement ability and prospective capabilities. Tetrahymena thermophila genotypes indeed differ in their movement strategies, some moving in a highly sinuous way while others follow very straight trajectories [63]. Alternatively, these results might reveal the existence of a polymorphism in dispersal strategies [18,53,64–66], with some genotypes plastically modifying their dispersal decisions depending on the fragmentation context with potential costs, while others show unconditional dispersal rate.

Habitat fragmentation and associated habitat loss pose serious threats to biodiversity [1–3], including a decrease in functional connectivity resulting from increased inter-patch distance [1,4–6,17]. Here, we provided experimental evidence for decreased dispersal rate and reduced habitat choice at immigration under habitat fragmentation, probably resulting from increased costs of prospecting limiting the ability to perform informed dispersal decisions. Our study, therefore, supports the prediction that habitat choice at immigration might be limited or counter-selected in fragmented landscapes [4,19]. Furthermore, we found that increasing inter-patch distance also modified the decision to either stay or leave the local patch (i.e. habitat choice at emigration), resulting in either increased optimal habitat choice or the rise of a preference for suboptimal habitats. That habitat fragmentation might affect not only dispersal rate but also the level of non-randomness of dispersal can considerably modify ecological and evolutionary dynamics of populations facing environmental changes [4], such as the distribution of specialists and generalists in the landscape, their coexistence and response to environmental changes [24]. Investigating the consequences of habitat fragmentation for populations and communities while accounting for changes in the different facets of dispersal (e.g. dispersal rate, habitat choice, but also for instance dispersal syndromes and distance) is consequently an important next step to understand the dynamics of biodiversity facing current environmental changes.

Data accessibility

The data supporting the findings of this study are available from the Dryad Digital Repository at: https://doi.org/10.5061/dryad.7wm37pvpg [67].

Authors' contributions

S.J. defined and led the project. E.L., N.S. and S.J. designed the experiments. E.L. and S.J. performed the experiments, analysed the data and wrote the manuscript. N.S. contributed to revisions.

Competing interests

We declare we have no competing interests.