The spin Hall effect of Bi-Sb alloys driven by thermally excited Dirac-like electrons

Dirac-like electrons in Bi-Sb alloys are extremely effective in generating spin current and have large spin current mobility.

Abstract

We have studied the charge to spin conversion in Bi_1−xSb_x/CoFeB heterostructures. The spin Hall conductivity (SHC) of the sputter-deposited heterostructures exhibits a high plateau at Bi-rich compositions, corresponding to the topological insulator phase, followed by a decrease of SHC for Sb-richer alloys, in agreement with the calculated intrinsic spin Hall effect of Bi_1−xSb_x. The SHC increases with increasing Bi_1−xSb_x thickness before it saturates, indicating that it is the bulk of the alloy that predominantly contributes to the generation of spin current; the topological surface states, if present, play little role. Unexpectedly, the SHC is found to increase with increasing temperature, following the trend of carrier density. These results suggest that the large SHC at room temperature, with a spin Hall efficiency exceeding 1 and an extremely large spin current mobility, is due to increased number of thermally excited Dirac-like electrons in the L valley of the narrow gap Bi_1−xSb_x alloy.

INTRODUCTION

Generation of spin current or flow of spin angular momentum lies at the heart of modern spintronics. The power consumption of a spintronic device is directly related to its efficiency for converting a charge current that dissipates energy to a dissipative spin-polarized current or a dissipationless spin current (1, 2). A conventional means for creating a flow of spin angular momentum is by passing a charge current across a ferromagnetic metal (FM) that converts to a spin-polarized current. The efficacy of this process is proportional to the spin polarization of the FM. More recently, generation of spin current from a charge current passed along a nonmagnetic metal (NM) (3–5) or interface of materials with strong spin-orbit coupling (6–8) has emerged as an attractive alternative. In particular, the discovery (9) of the giant spin Hall effect (SHE) in 5d transition heavy metals (HMs) has triggered substantial effort in exploiting the spin current to electrically control magnetization of ferromagnets placed nearby. In HM/FM bilayer systems, the magnetization of the FM layer can absorb the orthogonal component of the nonequilibrium spin density originating from the SHE, giving rise to current-induced spin-orbit torque (SOT) at the HM/FM interface (10–12). The SOT in these bilayers enabled current-induced magnetization switching (9, 13), current-driven motion of chiral domain walls and skyrmions (14–16), and magnetoresistance (MR) effect that depends on the SHE, often referred to as the spin Hall MR (17, 18). The figure of merit of the charge to spin conversion in SHE is known as the damping-like spin Hall efficiency ξ_DL that includes nonideal spin transmission across the interface (19, 20). Using ξ_DL, the spin current j_s generated from a charge current j_c passed to an NM layer and entering the FM layer can be expressed as j_s = ξ_DL(ℏ/2e)j_c, where ℏ is the reduced Planck constant and e is the electrical charge. As ξ_DL may depend on the longitudinal conductivity σ_xx of the NM layer, which varies with extrinsic factors such as impurity concentration and film texture, it is customary to use the spin Hall conductivity (SHC) σ_SH, defined through the relation σ_SH = ξ_DL ⋅ σ_xx, to provide a measure of the anomalous transverse velocity the carriers obtain via the SHE (5).

Recent advances in the understanding of topological insulators (21) and Weyl semimetals (22) have attracted great interest in exploiting their unique electronic states for spintronic applications. Giant charge to spin conversion efficiencies were found in heterostructures that consist of a topological insulator and a ferromagnetic/ferrimagnetic layer (23–32). The large charge to spin conversion efficiency observed in these systems was attributed to the current-induced generation of spin density enabled by the spin momentum locked surface states of topological insulators. Ideally, the bulk of a topological insulator should be insulating. In practice, however, it remains as a great challenge to limit the current flow within the bulk of this material class. This is particularly the case for thin-film heterostructures in which imperfect crystal structures and interdiffusion with the adjacent layers may reduce or eliminate the bandgap of the bulk state. To take advantage of the topological surface states in generating spin accumulation, it has been considered detrimental to have current paths in the bulk. In terms of bulk conduction of carriers, the charge to spin conversion efficiency of Bi, a small gap semimetal with large spin-orbit coupling (33, 34) and one of the most used elements in forming topological insulators, has been reported to be extremely small (35, 36) compared to the 5d transition metals. Theoretically, Bi and Bi-Sb alloys have been predicted (37–39) to exhibit considerable SHC due to its unique electronic state.

Here, we show that the charge to spin conversion efficiency that originates from the bulk of Bi_1−xSb_x alloys is significantly larger than that of the 5d transition metals. The SHC of the alloy increases with increasing thickness before it saturates. This thickness dependence of the SHC, together with its facet independence, suggests that a significant amount of spin current is generated from the bulk of the alloy. We find little evidence of spin current generation from the topological surface states, if they were to exist in the sputtered films used here. The damping-like spin Hall efficiency exceeds 1 for the Bi-rich Bi_1−xSb_x alloy and decreases with increasing Sb concentration. The alloy composition dependence of the SHC indicates that the SHE of the alloy has considerable contribution from the so-called intrinsic SHE. Unexpectedly, we find that the SHC and the spin Hall efficiency increase with increasing measurement temperature. We find up to threefold (twofold) enhancement of σ_SH (ξ_DL) upon increasing the temperature from 10 to 300 K. Although thermal fluctuation is typically detrimental for many key parameters of spintronic devices, the SHC of the Bi_1−xSb_x alloy is enhanced at higher temperature due to the increased number of carriers at the valleys with large Berry curvature. Our results suggest that these carriers in Bi_1−xSb_x alloys, particularly in the Bi-rich compositions, have large spin current generation efficiency and equivalent spin current mobility.

RESULTS

Structural characterizations

Thin-film heterostructures with base structure of Sub./seed/[t_Bi Bi∣ t_Sb Sb]_N/t_Bi Bi/t_CoFeB CoFeB/2 MgO/1 Ta (thicknesses in nanometers) were grown by magnetron sputtering at ambient temperature on thermally oxidized Si substrates. N represents the number of repeats of the Bi∣Sb bilayers. The thicknesses of the Bi (t_Bi) and Sb (t_Sb) layers in the repeated structure are set to meet the condition t_Bi + t_Sb ∼ 0.7 nm. The nominal composition of CoFeB is Co:Fe:B = 20:60:20 atomic %. Unless noted otherwise, we use 0.5 nm Ta as the seed layer for Bi|Sb multilayers. The capping layer is always fixed to 2 MgO/1 Ta. We assume that the top 1-nm Ta layer is fully oxidized and does not contribute to the transport properties of the films.

θ − 2θ x-ray diffraction (XRD) spectra of representative films with t_Bi ∼ t_Sb ∼ 0.35 nm, N = 8, 16 are shown in Fig. 1A. The films are polycrystalline, and the peaks are indexed on the basis of the hexagonal representation of the rhombohedral Bi_1−xSb_x (space group $R\overline{3}m$; no. 166) that forms solid solution throughout the composition. Bragg diffraction peaks corresponding to (0003), ($01\overline{1}2$), and ($10\overline{1}4$) crystallographic directions are found. The peak intensities increase with increasing N, reflecting improved crystallinity of the film. Atomic force microscopy (AFM) image of the N = 8 film is shown in Fig. 1B. The root mean square roughness is of the order of 1 nm. Representative cross sectional high-angle annular dark-field scanning transmission electron microscopy (HAADF-STEM) images of the N = 16 film are shown in Fig. 1C. The lower-magnification STEM image at the top panel confirms that the Bi|Sb multilayer is granular and continuous. We find that the average grain size is ∼35 nm. The 2-nm CoFeB and the subsequent capping layers are also continuous and follow the morphology of the multilayer. The bottom panel shows the high-resolution STEM image of the film. The lattice fringes clearly seen in the image reveals the good crystallinity of the Bi∣Sb multilayer. A typical nanobeam diffraction pattern of the Bi|Sb multilayer is shown in the inset of Fig. 1C. The diffraction patterns suggest that the grains are consisted of Bi_1−xSb_x nanocrystallites with random orientations within the film plane. Although alternating Bi and Sb layers were sputtered to form Bi|Sb multilayers, energy-dispersive x-ray spectroscopy (EDS) mapping (see the Supplementary Materials) shows that the two elements intermix to form an alloy rather than a layer-by-layer superlattice.

Fig. 1. Structural characterization of Bi|Sb multilayers.

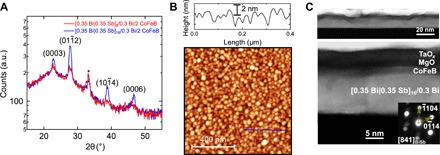
(A) X-ray diffraction (XRD) spectra of 0.5 Ta/[0.35 Bi|0.35 Sb]_N/0.3 Bi/2 CoFeB/2 MgO/1 Ta with N = 8 (blue line) and N = 16 (red line). a.u., arbitrary units. (B) AFM image of the N = 8 film. A line profile along the blue solid line drawn in the bottom image is shown at the top. (C) Cross-sectional HAADF-STEM images of the N = 16 structure. Selected nanobeam diffraction pattern of the Bi|Sb multilayer is shown in the inset.

Experimental setup

Because structural characterization show that the two elements intermix and form an alloy, we denote, hereafter, the Bi|Sb multilayers (i.e., [t_Bi Bi∣t_Sb Sb]_N/t_Bi Bi) as t_BiSb Bi_1−xSb_x using the total thickness of the multilayer (t_BiSb) and the corresponding composition x defined by the relative thickness of the Bi and Sb layers, i.e., $x\equiv \frac{t_{\text{Sb}}}{t_{\text{Bi}}+t_{\text{Sb}}}$. To evaluate the SOT, we pattern Hall bar devices using optical lithography. The nominal channel width w and length L are set to 10 and 25 μm, respectively. Illustration of the Hall bar device and the coordinate system adapted in this work are schematically illustrated in the inset of Fig. 2A. The longitudinal resistance R_xx and the transverse resistance R_xy of the devices were obtained using direct current (DC) transport measurements. Linear fitting to the sheet conductance L/(wR_xx) versus the thickness of one of the layers is used to estimate the conductivity σ_X (X = BiSb, CoFeB, seed layer). The current distribution within the heterostructures is calculated using the thicknesses and conductivities of the conducting layers.

Fig. 2. Bi_0.53Sb_0.47 thickness dependence of SOT and σ_SH.

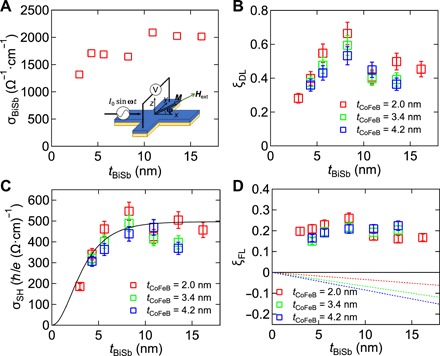
(A) Conductivity σ_BiSb of Bi_0.53Sb_0.47 plotted as a function of its thickness t_BiSb. Inset: Schematic illustration of a Hall bar device and the coordinate system. (B to D) t_BiSb dependence of the damping-like spin Hall efficiency ξ_DL (B), the SHC σ_SH (C), and the field-like spin Hall efficiency ξ_FL (D) of t_BiSb Bi_0.53Sb_0.47/t_CoFeB CoFeB. Dotted lines represent contributions from the Oersted field. All data were obtained at 300 K.

We use the harmonic Hall technique (11, 12, 40–42) to quantify the SOT of in-plane magnetized Bi_1−xSb_x/CoFeB heterostructures. Upon application of an alternating current (AC) I₀ sin ωt with frequency ω/2π and amplitude I₀ along x, an external magnetic field H_ext is applied in the xy plane, while the in-phase first harmonic (V_1ω) and the out-of-phase second harmonic (V_2ω) Hall voltages along y are simultaneously measured. The Hall resistance is obtained by dividing the harmonic voltages with I₀, i.e., $R_{1\mathrm{\omega }(2\mathrm{\omega })}\equiv V_{1\mathrm{\omega }(2\mathrm{\omega })}/(I_0/\sqrt{2})$. R_1ω is dominated by the planar Hall and anomalous Hall resistances, whereas R_2ω contains contributions from the current-induced damping-like spin-orbit effective field (H_DL), the field-like spin-orbit effective field (H_FL), the Oersted field (H_Oe), and thermoelectric effects [anomalous Nernst effect (ANE) of CoFeB, the ordinary Nernst effect (ONE) of Bi_1−xSb_x (43), and the collective action of the spin Seebeck effect (SSE) in CoFeB followed by the inverse SHE (ISHE) in Bi_1−xSb_x (41)]. The magnetic field amplitude dependence of R_2ω allows one to differentiate contributions from each effect (see Materials and Methods for the details). H_DL (H_FL) is related to the damping-like (field-like) spin Hall efficiency via ${\mathrm{\xi }}_{\text{DL}(\text{FL})}=\frac{2e}{\mathit{ħ}}\frac{H_{\text{DL}(\text{FL})}{M}_{\mathrm{s}}{t}_{\text{eff}}}{j_{\text{BiSb}}}$, where j_BiSb is the current density in Bi_1−xSb_x, and M_s and t_eff ≡ t_CoFeB − t_D denote the saturation magnetization and the effective thickness of the CoFeB layer, respectively. t_D is the thickness of the magnetic dead layer (see the Supplementary Materials for details of magnetic properties of the films). From here, we discuss the values of ξ_DL and ξ_FL. To estimate the SHC of Bi_1−xSb_x, we use the relation σ_SH = ξ_DLσ_BiSb, where the spin transmission across the Bi_1−xSb_x/CoFeB interface is assumed transparent (19, 20, 44). Taking into account a nontransparent interface will result in larger ξ_DL (and likely ξ_FL) and therefore results in larger σ_SH.

Bi_1−xSb_x thickness dependence of σ_SH

We first study the layer thickness dependence of the transport properties of heterostructures with nearly equiatomic Bi_0.53Sb_0.47 (t_Bi ∼ t_Sb ∼ 0.35 nm). The conductivity of Bi_0.53Sb_0.47 is plotted against t_BiSb in Fig. 2A. The slight increase of σ_BiSb with t_BiSb may be related to the larger grain size of thicker films that reduces the scattering at grain boundaries. Note that σ_CoFeB takes an average value of ∼5.5 × 10³ Ω⁻¹ cm⁻¹ and shows little dependence on t_BiSb. The t_BiSb dependence of ξ_DL and ξ_FL for Bi_0.53Sb_0.47/CoFeB heterostructures measured at 300 K is shown in Fig. 2, B and D, respectively. For a given t_BiSb, we studied devices with three different t_CoFeB (∼2, 3.4, and 4.3 nm). We find that ξ_DL of Bi_0.53Sb_0.47 has the same sign with that of Pt (45) and is consistent with previous reports on molecular beam epitaxy (MBE)–grown Bi_0.9Sb_0.1 (31) and stoichiometric Bi₂Se₃ (23, 29) topological insulators. At t_BiSb ∼ 8 nm, ξ_DL reaches a maximum of ∼0.65. This value is significantly larger than those found in HMs but lower than some recent reports on Bi-based topological insulators (23, 28, 30, 31). ξ_DL shows little dependence on t_CoFeB, indicating that the CoFeB layer plays little role in setting the SOT of the heterostructures. To take into account the change of σ_BiSb with t_BiSb, we plot the SHC σ_SH = ξ_DLσ_BiSb against t_BiSb in Fig. 2C. σ_SH increases with increasing t_BiSb and tends to saturate beyond t_BiSb of ∼8 nm. This thickness dependence resembles that expected for the bulk SHE in Bi_0.53Sb_0.47 and is inconsistent with the surface state–dominant scenario (23, 46) or with the quantum confinement picture (30). We fit all data using the relation ${\mathrm{\sigma }}_{\text{SH}}={\overline{\mathrm{\sigma }}}_{\text{SH}}[1-\text{sech}(\frac{t_{\text{BiSb}}}{\mathrm{\lambda }}\left)\right]$ with the bulk SHC ${\overline{\mathrm{\sigma }}}_{\text{SH}}$ and the spin diffusion length λ as the fitting parameters (47). We find ${\overline{\mathrm{\sigma }}}_{\text{SH}}=496\pm 26 (\mathit{ħ}/e){\mathrm{\Omega }}^{-1}{\text{cm}}^{-1}$ and λ = 2.3 ± 0.4 nm for Bi_0.53Sb_0.47.

Figure 2D illustrates the t_BiSb dependence of ξ_FL for heterostructures with different CoFeB thicknesses. The contribution from H_Oe, which takes the form of H_Oe/j_BiSb = 2πt_BiSb [10⁻¹ Oe/(A · cm⁻²)] according to Ampère’s law, is shown by the dotted lines in Fig. 2D. Note that H_Oe, which is negative in our convention, is subtracted from the total field-like SOT to calculate ξ_FL. We find that H_FL is opposite to the Oersted field and ξ_FL takes a constant value of ~0.2 throughout the range of t_BiSb studied. The sign of ξ_FL for Bi_0.53Sb_0.47/CoFeB agrees with that of metallic Pt/Co/AlO_x (12), but is opposite to that found in Bi₂Se₃/NiFe (23) and MoS₂/CoFeB (48). The nearly constant ξ_FL against t_BiSb is observed for all structures with different t_CoFeB. The distinct t_BiSb dependence of ξ_FL and ξ_DL suggests that the two orthogonal components of SOT originate from phenomena of different characteristic length scales (11, 49).

Bi_1−xSb_x composition and facet dependence of σ_SH

The bulk Bi_1−xSb_x alloy is known to be a semiconductor with a small bandgap hosting topological surface states for 0.09 ≤ x ≤ 0.22 and is semimetallic for the other compositions (33, 34, 50, 51). In an effort to shed light on the origin of the SOT, we investigate the Sb concentration (x) dependence of σ_SH and related parameters in 10 Bi_1−xSb_x/2 CoFeB heterostructures. To characterize the basic transport properties of the Bi_1−xSb_x alloy, we also deposited and measured stacks without the CoFeB layer (i.e., t_CoFeB = 0). We have excluded pure Bi (x = 0) from this study due to its large sheet resistance (considerably larger than those of the x ≠ 0 alloys) and island-like morphology, which may result in highly nonuniform current flow within the CoFeB layer. For alloys with x > 0, the surface roughness notably improves, as shown in Fig. 1B. Figure 3A shows the x dependence of σ_BiSb: σ_BiSb increases monotonically with increasing Sb concentration. This is consistent with previous report on the transport properties of the bulk Bi_1−xSb_x alloy (50), where it was shown that Bi_1−xSb_x gradually changes from being a semiconductor to a semimetal with increasing Sb concentration. As shown in Fig. 3B, the ordinary Hall coefficient R_H ≡ R_xyt_BiSb/H_z (H_z is the external field along z) also varies monotonically with increasing x. In our convention, R_H > 0 (R_H < 0) corresponds to carrier transport being dominated by electrons (holes). We find that the carriers of Bi-rich alloys are electron dominant, whereas the Sb-rich structures are hole dominant, accompanied by a smooth sign change of R_H at x ∼ 0.55. This reflects the multicarrier nature of the polycrystalline Bi_1−xSb_x films, which have at least one hole and one electron pockets at the Fermi level. We note that this differs from the ternary (Bi_1−xSb_x)₂Te₃ topological insulator for which R_H diverges and abruptly changes sign when traversing the Dirac point (26).

Fig. 3. Bi_1−xSb_x composition dependence of carrier transport and σ_SH.

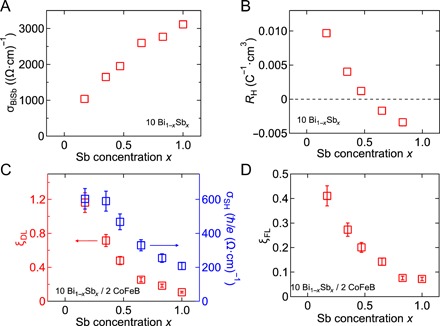
(A and B) Sb concentration x dependence of the conductivity σ_BiSb (A) and the Hall coefficient R_H (B) of Bi_1−xSb_x with t_BiSb = 10 nm. For these studies, heterostructures without the CoFeB layer were used. (C and D) Sb concentration (x) dependence of the damping-like spin Hall efficiency ξ_DL (left axis), the SHC σ_SH (right axis) (C), and the field-like spin Hall efficiency ξ_FL (D) for 10 Bi_1−xSb_x/2 CoFeB heterostructures. All data were collected at 300 K.

ξ_DL, σ_SH, and ξ_FL as a function of x for Bi_1−xSb_x/2 CoFeB heterostructures are presented in Fig. 3 (C and D). ξ_DL and ξ_FL increase with increasing Bi concentration, reaching a maximum of ξ_DL ∼ 1.2 and ξ_FL ∼ 0.41 for structures with x ∼ 0.17, a composition for which bulk Bi_1−xSb_x is commonly classified as a topological insulator. However, we emphasize that the Bi_1−xSb_x thickness dependence in the previous section and the facet dependence of SHE in the next paragraph both suggest the bulk origin of the SHE. The x dependence of σ_SH exhibits similar trend with that of ξ_DL: We find a plateau of ${\mathrm{\sigma }}_{\text{SH}}\sim 600(\mathit{ħ}/e){\mathrm{\Omega }}^{-1}{\text{cm}}^{-1}$ for x < 0.35. This x dependence of σ_SH is in very good agreement with that obtained from tight binding calculations (38), suggesting the dominance of the intrinsic contribution over that of the extrinsic skew scattering and side-jump contributions for the observed SHE in Bi_1−xSb_x.

We have also varied the seed layer of the Bi_1−xSb_x layer to study the facet-dependent SHE. Figure 4A shows the XRD spectra of 8.9-nm-thick Bi_0.53Sb_0.47 films grown on different seed layers, showing that the orientation of Bi_0.53Sb_0.47 nanocrystallites can be tuned from being practically random (Bi_0.53Sb_0.47 on 0.5 Ta seed; see Fig. 1A) to strongly (0003) oriented (0.5 Ta/2 Te seed) or strongly ($01\overline{1}2$) oriented (2 MgO seed). The Sb concentration (x) dependence of the longitudinal conductivity σ_BiSb and the SHC of Bi_1−xSb_x are shown in Fig. 4B. As evident from the inset of Fig. 4B, the difference in the texture causes large changes in σ_BiSb, particularly at smaller x. However, the x dependence of SHC hardly changes upon varying the Bi_1−xSb_x texture and σ_BiSb. We thus infer that topological surface states, which are intimately related to the Bi_1−xSb_x facets (34), are therefore unlikely to be the primary source of the observed SHE. The robustness of SHC against σ_BiSb further consolidates our suggestion that the intrinsic mechanism can account for the observed SHE.

Fig. 4. Facet dependence of σ_SH.

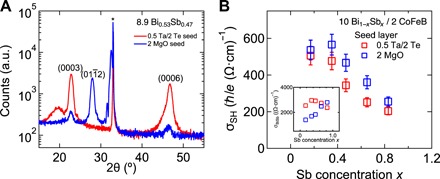
(A) XRD spectra for 8.9 Bi_0.53Sb_0.47 grown on 0.5 Ta/2 Te (red) and 2 MgO (blue) seed layers. Heterostructures without the CoFeB layer were used. (B) Sb concentration (x) dependence of SHC σ_SH for 10 Bi_1−xSb_x/2 CoFeB heterostructures with the two seed layers described in (A). The inset shows the x dependence of σ_BiSb. The measurement temperature is 300 K.

Temperature dependence of σ_SH

Last, we examine the temperature dependence of the transport properties for Bi_1−xSb_x/CoFeB heterostructures with selected x (x ∼ 0.17, 0.47, and 0.65). Here, the seed layer of Bi_1−xSb_x is 0.5 nm Ta. Figure 5A shows σ_BiSb as a function of the measurement temperature. We find that Bi_1−xSb_x has weak and positive temperature coefficient of the conductance, which is typical for a semiconductor. To obtain the variation of the carrier concentration and mobility of Bi_1−xSb_x, we measured temperature dependence of the longitudinal MR ratio ((R_xx(H_z) − R_xx(H_z = 0))/R_xx(H_z = 0)) and R_xy against H_z. Within the framework of a two-band model (52), we define n_h (n_e) as the effective hole (electron) concentration of Bi_1−xSb_x, with an effective mobility μ_h (μ_e). Assuming equal population of the two carriers (n_h = n_e = n) (51), we evaluate these parameters for temperatures ranging from 50 to 300 K, where the MR ratio and R_xy are quadratic and linear, respectively, with H_z up to 8 T (see the Supplementary Materials for details of two-band model analysis). The temperature dependence of the carrier concentration and the mobility are summarized in Fig. 5 (B to D). The carrier concentration increases with increasing temperature, which we infer is caused by the thermal broadening of the Fermi-Dirac distribution. In contrast, μ_h and μ_e both decrease with increasing temperature, obeying a power law that scales with ∼T^−0.5. These results indicate a competition between the impurity-mediated scattering (∝T^1.5) and electron-phonon scattering (∝T^−1.5), with the latter being more dominant. Compared to the carrier concentration and mobility of the majority carrier for bulk single-crystal Bi (n ∼ 4.6 × 10¹⁷ cm⁻³; μ_e ∼ 6 × 10⁵ cm² V⁻¹ s⁻¹) (53) and Sb (n ∼ 3.9 × 10¹⁹ cm⁻³; μ_h ∼ 2 × 10⁴ cm² V⁻¹ s⁻¹) (54) at 77 K, n values of the Bi_1−xSb_x films studied here are one to two orders of magnitude higher, while μ values are orders of magnitude lower. These are expected for sputtered polycrystalline thin films that contain significantly higher defect density compared to that of the bulk samples (36).

Fig. 5. Temperature dependence of carrier transport and σ_SH.

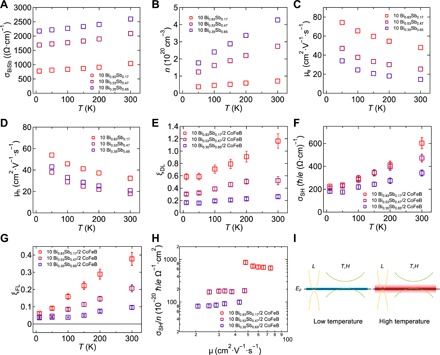
(A to D) Temperature dependence of the conductivity σ_BiSb (A), the effective carrier concentration n (B), the electron mobility μ_e (C), and the hole mobility μ_d (D) for 10 Bi_1−xSb_x. Heterostructures without the CoFeB layer were used. (E to G) Temperature dependence of the damping-like spin Hall efficiency ξ_DL (E), the SHC σ_SH (F), and the field-like spin Hall efficiency ξ_FL (G) for 10 Bi_1−xSb_x/2 CoFeB heterostructures. (H) σ_SH/n as a function of the majority carrier mobility (μ_e for x = 0.17 and 0.47, and μ_h for x = 0.65) for 10 Bi_1−xSb_x/2 CoFeB heterostructures. (I) Schematic illustration of the band structures of the Bi-rich Bi_1−xSb_x alloy with thermal broadening at low and high temperatures.

The temperature dependence of ξ_DL, σ_SH, and ξ_FL for 10 Bi_1−xSb_x/2 CoFeB heterostructures is plotted in Fig. 5, E to G, respectively. Unexpectedly, all these quantities strongly enhance upon increasing the temperature from 10 K to room temperature (300 K), suggesting that this enhancement is a rather generic feature for the Bi_1−xSb_x alloy. Notably, for Bi_0.83Sb_0.17, up to a threefold (twofold) enhancement is observed for σ_SH (ξ_DL) over the investigated temperature interval. While similar increase of ξ_FL with increasing temperature was previously reported in HM/FM heterostructures (49, 55), this strong enhancement of technologically important ξ_DL and σ_SH with increasing temperature has not been observed in metallic systems. We have also studied the temperature dependence of σ_SH for thinner Bi_1−xSb_x films (5.6 Bi_0.53Sb_0.47/2 CoFeB). We find similar temperature dependence of σ_SH compared to that shown in Fig. 5E, which suggests that the temperature dependence of ξ_DL, σ_SH, and ξ_FL is not due to a temperature-dependent spin diffusion length of Bi_1−xSb_x. We have also verified that the CoFeB saturation magnetization hardly changes within this temperature range (see the Supplementary Materials), reassuring that the change of ξ_DL and ξ_FL with temperature is caused by the modulation of the injected spin current.

DISCUSSION

Within the Drude model, the longitudinal conductivity σ_BiSb is proportional to the carrier concentration n and the mobility μ. μ is proportional to the relaxation time τ because μ = eτ/m*, where m* is the effective mass. On varying the temperature, contribution from n surpasses that of μ in Bi_1−xSb_x, resulting in a positive temperature coefficient of the conductance, as shown in Fig. 5A. For the SHC, by definition, the relaxation time dependence of σ_SH provides a measure of the mechanism of the SHE: σ_SH ∼ τ¹ for the extrinsic skew scattering and σ_SH ∼ τ⁰ when the intrinsic or side-jump mechanism dominates (5). With regard to the relation between σ_SH and n, the intrinsic contribution should scale with n if the analogy with the anomalous Hall conductivity applies (2). Calculations suggest that similar scaling between σ_SH and n holds for the extrinsic mechanisms (56). We may thus take the ratio σ_SH/n to eliminate the effect of n on the temperature dependence of σ_SH . We expect σ_SH/n is proportional to μ¹ for the extrinsic skew scattering mechanism and is a constant for the intrinsic/side-jump mechanism. Figure 5H shows σ_SH/n as a function of the mobility μ (here, the mobility of the majority carrier is used, i.e., μ_e for x = 0.17 and 0.47, and μ_h for x = 0.65). We find a relatively weak mobility dependence of σ_SH/n for all alloy compositions studied. The slope of σ_SH/n versus μ tends to increase as the Sb concentration increases. These results indicate that the extrinsic skew scattering contribution is relatively weak for Bi-rich alloys with large intrinsic SHE, but this contribution becomes non-negligible (but still smaller than the intrinsic one) with increasing x (and σ_BiSb). Although this is reminiscent of the crossover from intrinsic to extrinsic SHE for metallic Pt upon tuning the resistivity of the metal (57), we note that σ_BiSb at the crossover is one to two orders of magnitude lower than that of Pt. Alternatively, we consider that this crossover is a consequence of the band structure modification induced by Sb doping. As shown in the schematic band structure of Fig. 5I, the transport in the Bi-rich Bi_1−xSb_x alloy is dominated by the Dirac-like electrons at the L point in the momentum space (33, 34, 37, 50, 51). Upon substituting Bi with Sb, holes from the T and H points with quadratic-like dispersion become increasingly important for the conduction, as shown by the x dependence of R_H in Fig. 3B. Our experimental results indicate that Dirac-like L electrons, in contrast to holes in T and H pockets with quadratic dispersion, are key for achieving large intrinsic SHE in Bi_1−xSb_x. We thus consider that the large enhancement of SHC with temperature is caused by the increased number of L electrons due to thermal broadening of the Fermi-Dirac distribution.

Referring to the relation between the carrier density, mobility, and conductivity that derives from the Drude model, σ_SH/n can be regarded as the equivalent carrier mobility of transverse spin current. To provide reference of the equivalent mobility, we estimate σ_SH/n of a typical transition metal, Pt, which has the highest intrinsic SHC reported thus far [${\mathrm{\sigma }}_{\text{SH}}\approx 2000 (\mathit{ħ}/e){\mathrm{\Omega }}^{-1}{\text{cm}}^{-1}$ at 0 K (58)]. Assuming the carrier density n of Pt is of the order of 10²² cm⁻³, we obtain ${\mathrm{\sigma }}_{\text{SH}}/n\sim 20\times {10}^{-20} (\mathit{ħ}/e){\mathrm{\Omega }}^{-1}{\text{cm}}^2$. This is more than an order of magnitude smaller than that of Bi_0.83SB_0.17 evaluated at room temperature $({\mathrm{\sigma }}_{\text{SH}}/n\sim 800\times {10}^{-20} (\mathit{ħ}/e){\mathrm{\Omega }}^{-1}{\text{cm}}^2)$. The difference is also remarkable within the Bi_1−xSb_x alloy. If we compare Bi_0.83Sb_0.17 with Bi_0.35Sb_0.65, although the SHC σ_SH differs by a factor of 2, the equivalent mobility σ_SH/n is larger for the former by nearly one order of magnitude. These results demonstrate the exceptionally high spin current generation efficiency and mobility of the Dirac-like L electrons in Bi-rich Bi_1−xSb_x alloys compared to the majority holes in Sb-rich Bi_1−xSb_x and the predominantly s-like conduction electrons in Pt.

In summary, we have studied the SOT in sputter-deposited Bi_1−xSb_x/CoFeB heterostructures. The SHC of Bi_1−xSb_x increases with increasing thickness until saturation and is facet independent. These results suggest a dominant contribution from the bulk of the alloy: The effect of the topological surface states, if any, is not evident. The SHC is the largest with Bi-rich composition and decreases with increasing Sb concentration. This trend is in accordance with the intrinsic SHE of Bi_1−xSb_x predicted using tight binding calculations. The SHC and the damping-like spin Hall efficiency increase with increasing temperature. For example, the damping-like spin Hall efficiency of Bi_0.83Sb_0.17 exhibits a twofold enhancement from 5 K to room temperature, reaching ξ_DL ∼ 1.2. We infer that the thermally excited population of the Dirac-like electrons in the L valley of the narrow gap Bi_1−xSb_x is responsible for the temperature-dependent SOT. These results show that the Dirac-like electrons in Bi-rich Bi_1−xSb_x alloys are extremely effective in generating spin current, and their equivalent spin current mobility is more than an order of magnitude larger than that of typical transition metals with strong spin-orbit coupling. The very high spin Hall efficiency of Bi-rich Bi_1−xSb_x makes this material an outstanding candidate for applications involving spin current generation and detection at elevated temperatures. In addition, the lower carrier concentration and therefore a smaller electric field screening length in Bi_1−xSb_x compared to the common HMs allow efficient electric field control of SOT, thus paving a route to multifunctional spin-orbitronic devices that are sensitive to external stimuli such as heat and electric field.

MATERIALS AND METHODS

Sample preparation and characterization

All samples were grown at ambient temperature by magnetron sputtering on Si substrates (10 × 10 mm²) coated with 100-nm-thick thermally oxidized Si layer. AFM was used to characterize the roughness of the surface. θ − 2θ XRD spectra were obtained using a Cu Kα source in parallel beam configuration and with a graphite monochromator on the detector side. The saturation magnetization and the magnetic dead layer thickness of the CoFeB layer in the heterostructures were determined by hysteresis loop measurements using a vibrating sample magnetometer (VSM). AFM, XRD, and VSM studies were performed using unpatterned constant thickness films. HAADF-STEM analysis of cross-sectioned samples was performed using a FEI Titan G2 80-200 TEM with a probe-forming spherical aberration corrector operated at 200 kV. The samples were cross-sectioned from a plain film into thin lamellae by focused ion beam lift-out technique using FEI Helios G4 UX. Hall bars for the transport measurements were pattered from the films using optical lithography and Ar ion etching. The width w and the distance between the two longitudinal voltage probes L are 10 and 25 μm, respectively. Contact pads to the Hall bars, 10 Ta/100 Au (thickness in nanometers), were formed using a standard lift-off process.

SOT measurements

We treat the CoFeB magnetization as a single domain magnet with a magnetization vector M lying in the film plane (xy plane) at equilibrium. The external magnetic field H_ext is applied along the film plane with an angle φ_H with respect to the x axis. We assume that the in-plane magnetic anisotropy of the CoFeB layer is negligible compared to the magnitude of H_ext. Thus, the angle φ between M and the x axis is assumed be equal to φ_H.

When current is passed along x, electrons with their spin direction parallel to y diffuses into the CoFeB layer via the SHE. The impinging spin current exerts spin-transfer torque (or often referred to as the SOT) on the CoFeB magnetization. The torque can be decomposed into two components, the damping-like and field-like torques: The equivalent effective fields are defined as H_DL and H_FL, respectively. Together with the Oersted field H_Oe, H_DL and H_FL cause tilting of the CoFeB layer magnetization. When an AC (amplitude I₀, frequency ω/2π) is applied to the heterostructure, current-induced oscillation of M leads to Hall voltage oscillation via anomalous Hall effect (AHE) and planar Hall effect (PHE). The first harmonic (fundamental) voltage V_1ω represents the magnetization direction at equilibrium, and the out-of-phase second harmonic voltage V_2ω provides information on the current-induced effective fields acting on the magnetization. We define $R_{i\mathrm{\omega }}\equiv V_{i\mathrm{\omega }}/(I_0/\sqrt{2})$ (i = 1, 2) to represent the harmonic signals.

Contributions to R_2ω include five terms. R_DL, R_FL, and R_Oe, which reflect changes in R_2ω caused by H_DL, H_FL, and H_Oe, respectively, decay with increasing H_ext. R_DL is proportional to the x component of the magnetization (cos φ), whereas R_FL + R_Oe scales with cos 2 φ cos φ due to the combined influences of AHE and PHE (40, 41). Current-induced Joule heating and the different thermal conductivity of the substrate and air can lead to an out-of-plane temperature gradient (41) across the heterostructure. With the temperature gradient, the ANE of CoFeB and the collective action of the SSE (59) in CoFeB followed by the ISHE in Bi_1−xSb_x result in a contribution (R_const) that does not depend on the size of H_ext. Applying a field orthogonal to the out-of-plane temperature gradient produces the last term, R_ONE, due to ONE (43). R_ONE scales linearly with H_ext. ONE of CoFeB is negligible compared to that of Bi_1−xSb_x due to the difference in the carrier density. Both R_const and R_ONE scale with cos φ.

Putting together these contributions (and assuming φ ∼ φ_H), R_2ω reads

$$\begin{array}{cc}\hfill R_{2\mathrm{\omega }}& =R_{\text{DL}}+R_{\text{ONE}}+R_{\text{const}}+R_{\text{FL}}+R_{\text{Oe}}\hfill \\ & =(R_{\text{AHE}}\frac{H_{\mathrm{DL}}}{H_{\text{ext}}+H_{\mathrm{K}}}+\frac{\mathrm{N}w\mathrm{\Delta }T}{I_0}{H}_{\text{ext}}+\frac{\mathrm{\alpha }w\mathrm{\Delta }T}{I_0})\text{cos} {\mathrm{\phi }}_H\hfill \\ & \hfill -2R_{\text{PHE}}\frac{H_{\text{FL}}+H_{\text{Oe}}}{H_{\text{ext}}}\text{cos} 2{\mathrm{\phi }}_H\text{cos} {\mathrm{\phi }}_H\hfill \end{array}$$ (1)

where R_AHE is the anomalous Hall resistance, R_PHE is the planar Hall resistance, H_K is the out-of-plane anisotropy field, $\mathrm{N}$ is the ONE coefficient of Bi_1−xSb_x, and α is a coefficient that reflects the size of ANE and the combined action of SSE and ISHE. The distinct H_ext and φ_H dependence of R_2ω for these contributions allows unambiguous separation of each term from the raw R_2ω signal. We first decompose R_2ω into two contributions of different φ_H dependence, i.e., cos φ_H and cos 2φ_H cos φ_H, and define the prefactors of these two parts as A and B, respectively

$$A\equiv R_{\text{AHE}}\frac{H_{\text{DL}}}{H_{\text{ext}}+H_{\mathrm{K}}}+\frac{V_{\text{ONE}}}{I_0}{H}_{\text{ext}}+\frac{V_{\text{const}}}{I_0}$$ (2)

$$B\equiv -2R_{\text{PHE}}\frac{H_{\text{FL}}+H_{\text{Oe}}}{H_{\text{ext}}}$$ (3)

Two parameters V_ONE ≡ $\mathrm{N}$ wΔT and V_const ≡ αwΔT are defined to describe the thermoelectric contributions. The H_ext dependence of A and B is then fitted based on Eqs. 2 and 3, respectively.

Representative R_2ω as a function of φ_H obtained using different H_ext for the 10.9 Bi_0.53Sb_0.47/2 CoFeB heterostructure is shown in Fig. 6A. Solid lines in the figure are fits to the data using Eq. 1. See the Supplementary Materials for the φ_H dependence of R_1ω. All data shown in this article are collected using ω/2π = 17.5 Hz. The current amplitude is typically set to ∼1.5 mA_rms, which corresponds to a current density in the Bi_1−xSb_x layer of ∼1 × 10¹⁰ A/m². We find that R_2ω scales linearly with current. The H_ext dependence of one of the fitting parameters A is shown in Fig. 6B. The best fit to A against H_ext is shown by the solid black line. The colored lines represent decomposition of each contribution following Eq. 2 (see the Supplementary Materials for determination of R_AHE and H_K). In the small H_ext range, R_DL term (red line) dominates, whereas at larger field, A changes sign and is eventually dominated by R_ONE (green line). B is plotted against 1/H_ext in Fig. 6C, with the black solid line showing the best linear fit to the data using Eq. 3. We extract H_DL, H_FL + H_Oe, V_ONE, and V_const from the two fits. We find V_const and V_ONE to be proportional to the square of the current flowing within the CoFeB and Bi_1−xSb_x layer, respectively. These results confirm the thermoelectric origin of these contributions and the validity of the interpretation of R_2ω.

Fig. 6. Representative second harmonic Hall resistances.

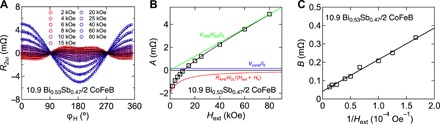
(A) Field angle φ_H dependence of the second harmonic Hall resistance R_2ω for the 10.9 Bi_0.53Sb_0.47/2 CoFeB heterostructure obtained using different H_ext measured at 300 K. (B) H_ext dependence of the fitting parameter A. (C) 1/H_ext dependence of the fitting parameter B. In (B) and (C), the colored lines show contributions from each component described in Eqs. 2 and 3; the black solid line shows the sum of all contributions.

SUPPLEMENTARY MATERIALS

Supplementary material for this article is available at http://advances.sciencemag.org/cgi/content/full/6/10/eaay2324/DC1

Section S1. STEM results of films

Section S2. Magnetic properties of BiSb/CoFeB

Section S3. Anomalous Hall resistance and anisotropy field

Section S4. First harmonic Hall resistance of BiSb/CoFeB

Section S5. Two-band model analysis of BiSb

Section S6. Evaluation of the SOT analysis protocol

Section S7. The efficiency of BiSb SOT

Fig. S1. HAADF-STEM and EDS mapping.

Fig. S2. Saturation magnetization and magnetic dead layer thickness.

Fig. S3. Anomalous Hall resistance and anisotropy field.

Fig. S4. First harmonic Hall resistance.

Fig. S5. Temperature dependence of magnetotransport properties of Bi_1−xSb_x.

Fig. S6. SOT measurements of a standard sample: Pt/CoFeB.

References (60–69)