Local lattice distortions and dynamics in extremely overdoped superconducting YSr₂Cu_2.75Mo_0.25O_7.54

Significance

We show that a second-phase diagram is needed to describe “overdoped” cuprates prepared by high-pressure oxygen methods. The transition temperatures of HPO compounds continue to increase past the values of O stoichiometry and Cu charge where the superconductivity in conventional materials terminates. The Mo and Cu maintain their preferred geometries despite the higher Cu charge via clustering of the Mo. The principal effect of the Mo and excess O is therefore nanophase separation into Mo- and Cu-enriched domains. The high degree of similarity between the HPO and normal structures, including a Cu2-apical O two-site distribution, therefore suggests that the elevated T_c of YSCO–Mo may originate in a combination of this nanoscale heterogeneity and the dynamical properties.

Abstract

A common characteristic of many “overdoped” cuprates prepared with high-pressure oxygen is T_c values ≥ 50 K that often exceed that of optimally doped parent compounds, despite O stoichiometries that place the materials at the edge or outside of the conventional boundary between superconducting and normal Fermi liquid states. X-ray absorption fine-structure (XAFS) measurements at 52 K on samples of high-pressure oxygen (HPO) YSr₂Cu_2.75Mo_0.25O_7.54, T_c = 84 K show that the Mo is in the (VI) valence in an unusually undistorted octahedral geometry with predominantly Mo neighbors that is consistent with its assigned substitution for Cu in the chain sites of the structure. Perturbations of the Cu environments are minimal, although the Cu X-ray absorption near-edge structure (XANES) differs from that in other cuprates. The primary deviation from the crystal structure is therefore nanophase separation into Mo- and Cu-enriched domains. There are, however, indications that the dynamical attributes of the structure are altered relative to YBa₂Cu₃O₇, including a shift of the Cu-apical O two-site distribution from the chain to the plane Cu sites. Another effect that would influence T_c is the possibility of multiple bands at the Fermi surface caused by the presence of the second phase and the lowering of the Fermi level.

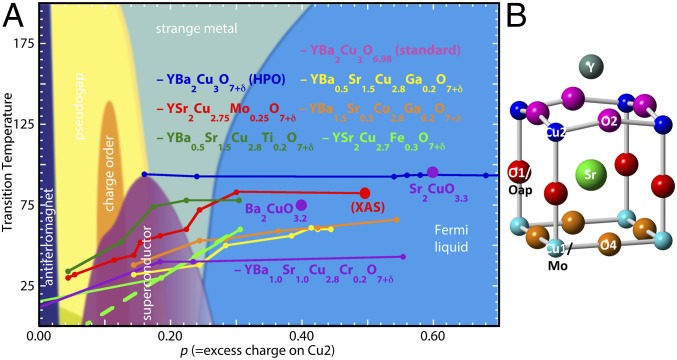
The common, or “conventional,” phase diagram for what we will refer to as “conventional” hole-doped cuprates (1) shows the superconducting dome beginning at p ∼ 0.06, where p is defined as the number of excess holes per CuO₂-type Cu, T_c rising to its maximum at p ∼ 0.16, followed by T_c of “overdoped” materials decreasing rapidly to 0 as they transition to a normal Fermi liquid at p ∼ 0.27. This widely accepted diagram, however, overlooks cuprates synthesized with high-pressure oxygen (HPO) using either chlorate or peroxide compounds (2) that have T_c values greater than 50 K, despite O stoichiometries (3–12) so high that they are outside the superconducting region when made with O₂. In addition, T_c of these substituted HPO compounds can be substantially higher than the parent materials from which they derive, e.g., 84 K for YSr₂Cu_2.75Mo_0.25O_7.54 (YSCO-Mo) (13) vs. 63 K for YSr₂Cu₃O_7+δ (14). One type of HPO materials derives from the La₂CuO_4+δ structure family and consists of Sr₂CuO_3.3 (SCO) (15–18), Ba₂CuO_3.2 (BCO) (15, 19–21), and related compounds with T_c values of 73 to 95 K. The second type is isostructural with YBa₂Cu₃O₇ (YBCO), consisting of Y(Ba_1−xSr_1+x)(Cu_3−yM_y)O_7+δ-type compounds (22) with Ba and Sr interchanging and M=Cr, Co, Fe, Ga, Mo, etc. substituting for 5 to 100% of the Cu in the chain sites. For the YBCO type where data from many O stoichiometries are often available, p can be estimated by assigning a valence to M consistent with the high O activity (Mo = +6, Fe = +3, Ga = +3, etc.) and keeping the chain Cu charge at its already high value of +2.64 that is derived from the peak T_c occurring at p = 0.16 in YBa₂Cu₃O_6.98. These assumptions give p ∼ 0.3 for several compounds and still greater p values for others, even as their T_c values are constant or still increasing (Fig. 1). Even if a substantial amount of the excess charge goes onto the chain Cu, HPO compounds still differ significantly from the phase diagram as it applies to conventionally “overdoped” materials (23, 24), in that T_c shows no indication of rolling over up to the highest p values. X-ray measurements (25) and valence-bond sum calculations (26) that concur with the optimum p value for O₂-doped cuprates do, however, confirm that p does exceed 0.3 for HPO YSCO-Mo and related (Cu–Mo)-12s2 superconductors. In addition, the inception of superconductivity for some of these HPO compounds may begin below the standard p ∼ 0.06. Although there may be some disagreement on the exact placement of these materials on the diagram, it is apparent that the superconductivity in the HPO Y(Ba_1−xSr_1+x)(Cu_3−yM_y)O_7+δ compounds (2–8, 10–13, 22, 27, 28), SCO, and BCO does not conform to the dome of the conventional phase diagram and that the consistent behavior of these systems necessitates this second whose much broader and highly modified superconducting region challenges.

Fig. 1.

(A) Conventional phase diagram for YBCO with the addition of YSCO-Mo and other HPO compounds whose superconducting regions (9, 10, 13, 27, 70, 71) do not conform to the “dome” found for materials prepared by oxidation with O₂. (B) Half of the unit cell from the crystallographic structure (13) of YSCO-Mo, with labels as in SI Appendix, Fig. S1. The c axis is across the figure, through the Cu2–O1–Cu1 axis, with the CuO₂ plane adjacent to the Y and the Cu of the chains on the right side going vertically on and out of the page. One-fourth of the Cu1 are replaced by Mo. Cu1 and the substituting Mo are in the chains, Cu2 is in the CuO₂ planes, O1 or Oap is the apical O bridging Cu1/Mo with Cu2, O2 bridges between the Cu2 sites in the CuO2 planes (this is also O3 in orthorhombic YBCO because YSCO-Mo is tetragonal), O4 bridges the Cu1/Mo sites, and the XAFS results do not require the O5 or O6 sites of the YSCO-Mo crystal structure (SI Appendix, Fig. S1). Although the crystal structure places the O5 and O6 atoms of the Cu–Mo layer off of the Cu–Cu axes as in the crystal structure, the Cu–O and Mo–O distances determined by the XAFS locate the O4 atoms directly between the Mo–Mo and Cu–Cu pairs.

There are additional differences between the conventional and HPO materials. HPO compounds are tetragonal instead of orthorhombic. The projection of the heat capacity to a finite value at 0 K indicates that, although it is single phase, YSCO-Mo (13) and BCO (21) retain some Fermi liquid electrons to give coexisting superconducting and normal metallic electronic phases. The highly contracted Cu–Oap distances of the HPO compounds contradict the correlation (21) between higher T_c and increased distance from the apical O (Oap) to the Cu of the CuO₂ layer (29–32) or its opposite cation (33). This multitude of changes in composition and structure poses the questions of what are the effects of the separate modifications, how do they sum to stabilize the superconductivity to such an extent, and what is the microscopic mechanism underlying this second phase diagram? We address these questions via an X-ray absorption fine-structure (XAFS) study of YSCO-Mo at 52 K, using samples magnetically aligned along the c axis and in the aa plane. XAFS probes the local order of each element separately without the restrictions imposed by the space-group symmetry, so that it is not constrained to the average structure of the periodic fraction of the material. In addition, its structure factor, S(q, ω) (or S[q, t = 0]) (34–36), includes time and energy scales in the range of dynamical processes coupled to collective phenomena. XAFS therefore often provides key insights into the structure–property relationships of complex correlated materials.

We use the existing labels for YSCO-Mo (14) (Fig. 1A and SI Appendix, Fig. S1), T_c = 84 K (13) (SI Appendix, Fig. S2), derived from YBCO. Mo(IV–VI) in oxides is found in distorted octahedral geometries. These are incompatible with the square or highly elongated square pyramid or bipyramid displayed by Cu^2+δ as occurs in the YBCO/YSCO structure. The problem in YSCO-Mo and related materials is, therefore, how the Cu1 layer is arranged to minimize the stress between the different stable geometries of the Cu and substitutional element, M. The Cu1–M layer can easily take up the adventitious or superstoichiometric O if the substitutional element prefers more than four O neighbors at short distances, but only if the M–Cu pairs are accommodated in a way that minimizes the stress by maintaining their individual geometries. This includes conserving the square geometry of the Cu by limiting the number of Cu1–O4 pairs to two per Cu1 in addition to the two Cu–Oap, which constrains the relative positions of the Cu and M. The structure reported from neutron diffraction (13) with random, isomorphic substitution of the Cu by the Mo and the partially occupied, off-axis O sites in the Cu1–Mo plane may therefore only approximate the disorder in the atom positions, especially if the local symmetry is lower than the average tetragonal symmetry under which the structure was refined. Our XAFS results supplement these to provide a more precise depiction of the structure.

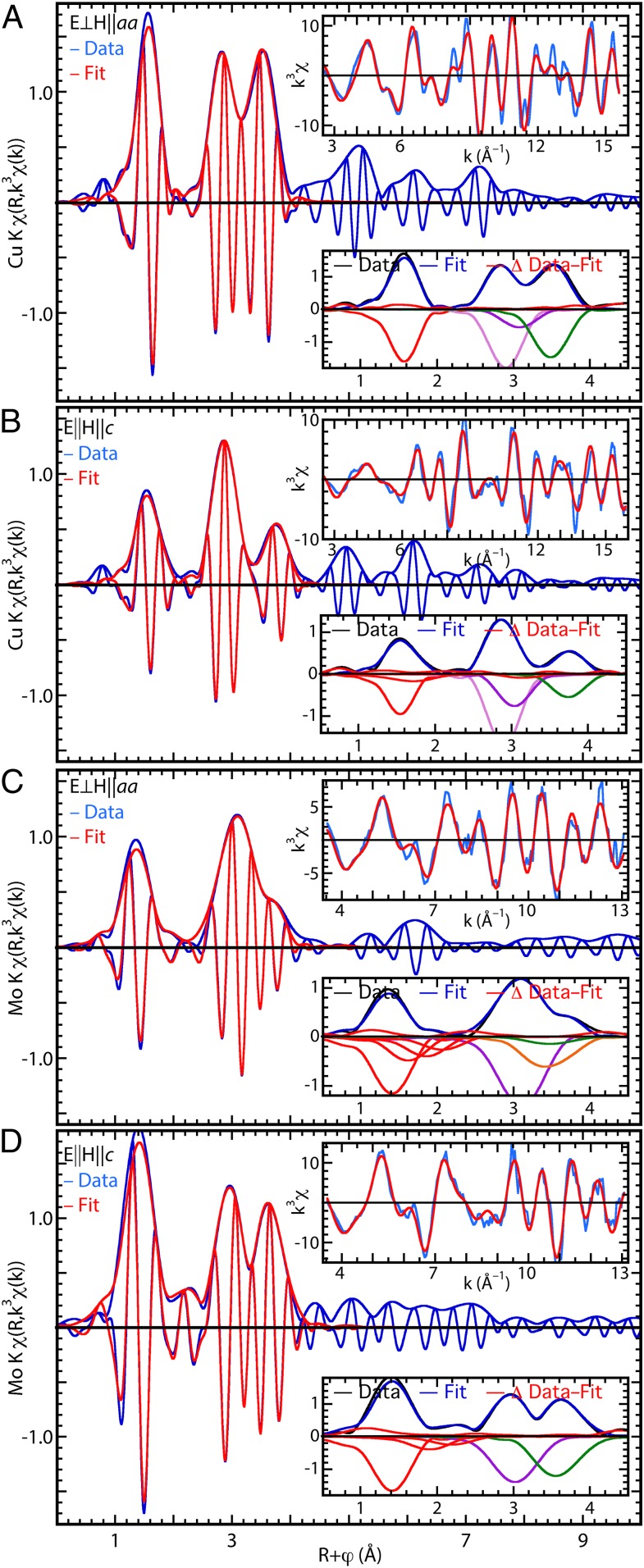
The information on the local structure around the Cu and Mo is obtained from the extended XAFS (EXAFS) spectra by observation of the spectra, comparison with related compounds, curve fits, and separation of the contributions of individual neighbors of particular interest. (A detailed discussion with additional figures is contained in SI Appendix.) The EXAFS spectra are presented as their Fourier transforms (FTs) that take χ(k) into χ(R) to give a modulus that approximates the Cu partial pair-distribution function. The caveats in this interpretation are that: the phase shifts cause a neighbor-element-specific reduction from the actual distance; the modulus only contains half of the total information, with the remainder in either the real or imaginary component; and the width of the modulus peak derives from the finite range of the data and is not only much wider than the actual distribution, but also wider than the inherent resolution of the spectrum (ΔR = π/2k_max), so that the contributions of various neighbors whose FTs overlap are actually easily separable. The Cu spectra of YSCO-Mo (Fig. 2 A and B) are very similar to those of YBCO (37–40), with the O nearest neighbors giving the features at R ∼ 1.6 Å, the Y and Sr at R ∼ 2.8 Å, and the Cu at R ∼ 3.5 Å. The lower numbers of O and Cu neighbors account for the lower amplitudes of the E||c spectrum. Excellent fits are obtained for both spectra by using only the crystallographic neighbors of the Cu. The distances calculated by the fits (Table 1) are within the standard EXAFS uncertainties of the crystallographic values. Uncorrelated thermal motion from weak bonding and resulting damping of the EXAFS signal accounts for the absence of contributions from the Cu1–Sr (41, 42) and Cu2–Cu2 pairs, which is typical of YBCO-type compounds (39, 40, 43, 44).

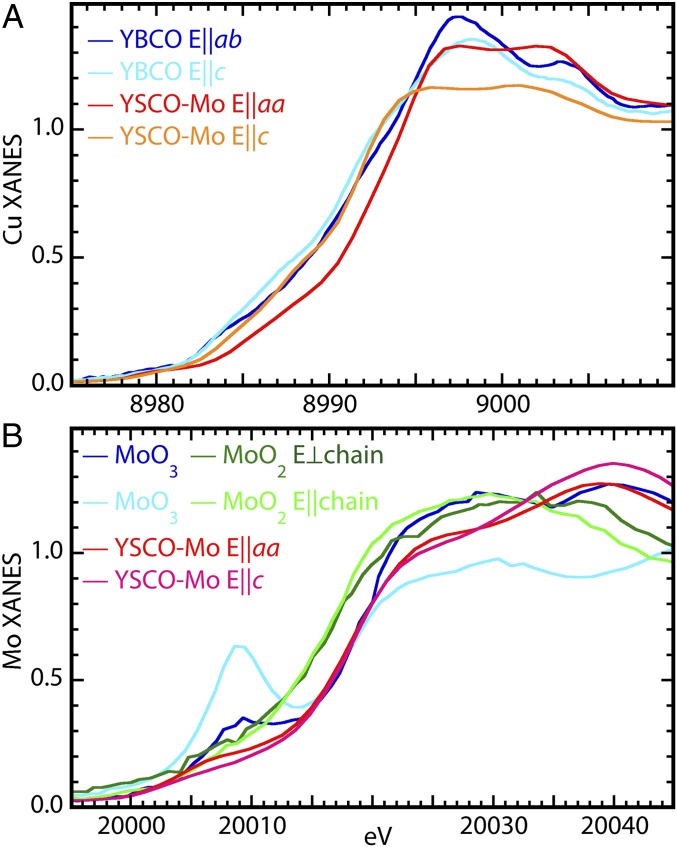
Fig. 2.

(A) Cu K-edge XANES of oriented YBCO and YSCO-Mo. (B) Mo K-edge XANES of MoO₃ parallel (light blue) and perpendicular (dark blue) to the very short, terminal Mo–O multiple bond, MoO₂ parallel and perpendicular to the directions of its Mo–Mo chains, and the YSCO-Mo along its two orientations.

Table 1.

Relevant interatomic distances

	Cu2–O2 Cu1–O5/6	Cu1–Cu1 Cu2–Cu2	Cu1–Oap	Cu2–Oap	Cu2–Cu2: E||c	Cu1–Cu2	Cu2–Y	Cu2–Sr	Cu1–Sr
xtal (14)	1.92	3.83	1.88	2.16	3.46	4.05	3.21	3.31	3.46
Cu EXAFS	1.91	3.81	1.88	2.14	3.45	4.04	3.19	3.35/3.31
Mo EXAFS	1.84/2.04/2.33/2.54	3.79 (Cu1)/3.95 (Mo)	1.88/2.39/2.69			4.05			3.48

Errors are 0.01 to 0.02 Å. The resolution limit for the Cu EXAFS is 0.11 Å and for the Mo EXAFS 0.12 Å. Because of the Cu/Mo and additional disorder, the referenced crystal structure found multiple Cu1–O positions labeled O5 and O6, none of which were along the Cu1–Cu1 vectors. In contrast, as stated in the description of the results of the curve fits, the EXAFS finds O positions very similar to YBa₂Cu₃O₇ for both the Cu and the Mo and, because there is apparently only a single O site, we labeled it O4. These differences are not unexpected; the diffraction analysis must describe the disorder under the constraints of the space-group symmetry derived from the average Cu and Mo positions that, as described in the text, are not equivalent. As discussed in the text and shown in Fig. 3A, the single Cu2–Oap distance listed here is the average position of its actual two-site distribution. The two-site Cu–Oap distribution was analyzed separately. The two Cu2–Sr distances are from the E||aa and E||c spectra. Cu1–Sr did not make an identifiable contribution to the EXAFS in both orientations. In the Cu EXAFS, the Cu1–Cu1 and Cu2–Cu2 contributions are combined; the Mo EXAFS will contain Mo–Cu1 and Mo–Mo. The analysis of the Mo E||aa EXAFS spectrum found four Mo–O4 distances separated by more than the resolution limit; the Mo E⊥||c found 3. The Mo–O pairs with Mo–O distances 2.33 and 2.39 Å Mo–O found in the analysis of the spectra from the two orientations most likely represent the same O neighbor that would be at an interstitial position off of the vectors through the Cu and Mo sites parallel to the crystallographic axes because it makes contributions to both orientations. The Mo–Mo, Mo–Cu2, and Cu1–Cu1/Cu2–Cu2 distances were obtained by using amplitudes and phases that are the sum of the two-, three-, and four-leg paths calculated by FEFF9 (69) from the crystal structure.

The Mo EXAFS for both orientations shows a similar pattern with three peaks (Fig. 2 C and D) that are the contributions of the O, Sr, and Cu/Mo. However, the substantial differences in the shapes of the χ(R) relative to the Cu beyond the difference from the lack of a Y neighbor demonstrate that the local structure around the Mo site is not identical to that of the Cu, as expected from the difference in their geometries in their oxides. The preference of the Mo for a distorted octahedral environment is found in that the Mo EXAFS requires O neighbors at more than one Mo–O distance for a complete fit (Table 1). In contrast to Cu1, the Mo–Sr contribution is large. The Mo–Cu pair distribution obtained from the EXAFS addresses the crucial issue of the Mo substitution mechanism. Of the four possibilities for the Cu or Mo neighbor, Cu or Mo with or without bridging O, curve fits showed that the Mo–M contribution was not only fit best, but also could be solely fit by the EXAFS from an Mo–O–Mo moiety. Mo–Mo and Mo–O–Cu were ruled out by being π out of phase, and the larger error with a Mo–Cu fit gave a likelihood of less than 0.01 (45) for that atom pair (SI Appendix, Fig. S4). This predominance of Mo neighbors indicates that the Mo is clustered so that the Cu1–Mo plane is separated into Mo- and Cu-enriched domains. Insofar as some Cu neighbors are required, it was found that up to one-fourth of the neighbors could be Cu without bridging O before the error in the fit became significantly worse (Fig. 2C). Consistent with the larger average size of Mo and the resulting strain from its substitution in the Cu site, the Mo–Mo distance is 3.95 Å, and the Mo–Cu is 3.79 Å, bracketing the Cu–Cu distance of 3.83 Å. In contrast to E||aa, the E||c EXAFS gives a local structure very similar to the Cu, with Mo–Oap and Mo–Cu2 distances the same as for Cu. The extra features in the spectrum are fit by O neighbors at 2.39 and 2.69, with low numbers of atoms indicating less than one atom per Mo. Together with the finding of an O at 2.33 to 2.39 Å for both orientations, implying an off-axis interstitial, these O atoms are most likely at the interfaces between the Mo and Cu domains.

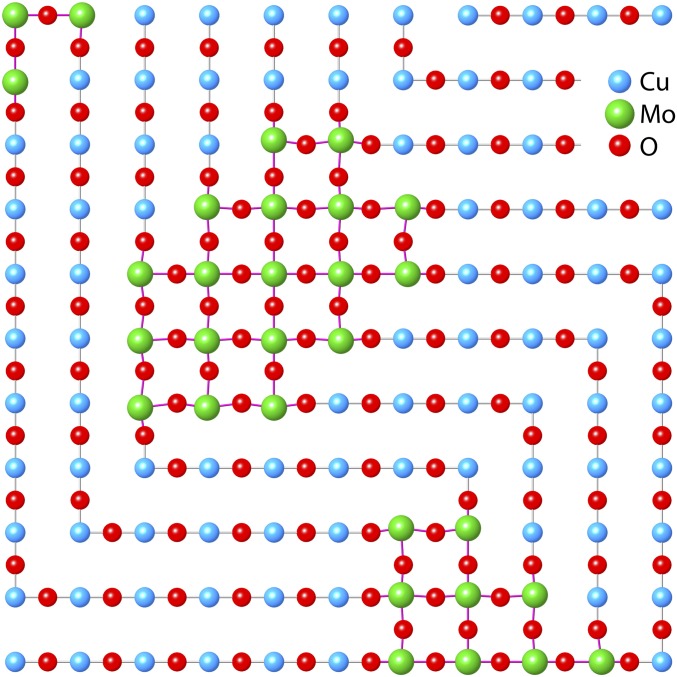
This analysis of the EXAFS therefore confirms that the YBCO-type crystal structure is conserved. The YBCO/YSCO structure is virtually identical for the Cu, with modifications around the Mo that result in its preferred distorted octahedral geometry, with the exception of the absence of O neighbors with Mo–O distances less than 1.8 Å that are typical of Mo(VI). This mechanism, whereby a different element substitutes for the Cu1, may apply to additional compounds; the Fe in YSr₂Cu_2.7Fe_0.3O_7+δ has also been stated to display its preferred geometry that differs from Cu (10). The EXAFS therefore indicates that the mechanism enabling this structure is clustering of the Mo, resulting in nanophase separation or nanoscale heterogeneity that divides the material into Mo- and Cu-type domains. The ordered Mo–O–Mo moieties are the internal or edge pairs of the cluster, with O bridges in four or three directions in the Cu1–Mo plane. The atoms constituting the disordered component are the Mo–Cu1 pairs at the edges of the clusters, many or most of which are not bridged to the Mo because the Cu already has its preferred O four neighbors at distances less than 2 Å, two each from the chain and Oap. Those that are bridged must have a wide range of distances, indicative of a disordered interface. The absence of a bridging O for the neighboring Cu ions and the presence of an interstitial O found in both orientations imply a rotation of a cohort of the O ions out of the aa plane. The limit on the relative numbers of Mo and Cu neighbors, the requirement of the domains being below the diffraction limit in size, and the accumulating stress as the domain size increases are all consistent with these clusters being three to four × four to five unit cells in size (Fig. 4). One fourth of the aa plane in the Cu1 layer would then be composed of these MoO₃ clusters, with the other three-quarters consisting of Cu–O chains isomorphic with the parent YSCO. The Mo domains limit Cu–O chain length, and the random orientations of these shorter chains would produce the average tetragonal symmetry. The greater number of O in the Mo domains may couple more strongly with the Sr, which would account for it being more correlated with the Mo than Cu1. At this size, a significant number of both types of atoms will also belong to the interfaces between the Cu and Mo domains.

Fig. 4.

Conceptual depiction of Cu1–Mo plane. As described in the text, the Mo is clustered into domains 12 to 20 unit cells (= 20 to 30 Mo atoms) in size, in which it can exert its preference for a distorted octahedral geometry, allowing the Cu1 to be in its preferred square geometry with its two Oaps. The disorder at the interfaces that results in some interstitial O out of this plane and the minimized contribution of the Mo–Cu pairs with bridging O caused by the strain from the average Mo–O distances being longer than the Cu–O ones is not fully shown. The alteration of the directions of the Cu–O chains at the Mo clusters result in the average tetragonal symmetry.

Fig. 3.

EXAFS [FT or χ(R) representation] of YSCO–Mo. (A) For Cu measured in the aa plane, the main graph shows the data and fit in real space, Upper Right Inset shows the same in k, and Lower Right Inset shows the FT moduli of the data, fits, and differences in their upper halves and the contributions of the individual neighbor shells, inverted for clarity, in their lower halves with red, O; violet, Y; purple, Sr; green, Cu; and orange, Mo. The relative numbers of atoms are reflected by the peak amplitudes, reduced by 1/R² and the Debye–Waller factor. (B) The same as A, but for Cu measured in the c direction. (C) The same as A, but for Mo measured in the aa plane. (D) The same as B for Mo in the c direction.

An additional finding is that, like other EXAFS studies of cuprates (46–51), the Cu2–Oap pair resides in a two-site distribution with Cu–O distances of 2.06 and 2.22 Å, so that the average location of the O is the same as the crystallographic one. The associated double-well potentials have been ascribed to tunneling polarons that are components of the dynamic structure. In contrast to a conventional polaron that transfers its excess charge and lattice distortion to a neighboring site by hopping, a tunneling polaron is a fixed set of atoms that oscillate between two conformations denoted by distinct geometries and charge distributions that in chemical terms would involve changes in the valence and resulting speciation of the affected atoms (34–36, 46). The signature for a tunneling polaron is that the two sites give individual signals in the EXAFS of only the Cu atom with that correlation for the Cu–O pair distribution, in this case Cu2 (SI Appendix, Fig. S5), but not in the signal from Cu1 atom or in diffraction. Although the location of the double-well potential in the Cu2–Oap bond is the same as for La₂CuO_4+δ and Tl-cuprates, in YBCO, it was associated with the Cu1–Oap bond. The 0.16-Å separation between the two sites in YSCO-Mo is quite close to these others, despite its unusually short Cu2–Oap distance. The quality of the fit indicates that all of the O atoms are located at one of these two positions. The Cu2–Oap double well therefore occurs for both Cu1 and Mo being the cation on its far side.

The Cu and Mo valences are probed by their X-ray absorption near-edge structure (XANES) that is interpreted by direct comparison with the spectra from related compounds and relevant standards (a more detailed discussion of the XANES is found in SI Appendix). The overall shape does not resemble those of YBCO, other cuprates (47–49), or Cu₂O, CuO, and NaCuO₂ (50). Since the local structures of the Cu are so similar, the origins of this difference must be a combination of modifications to the electronic structure and the dynamics that influence the oscillator strengths of forbidden transitions. The overall shift of the Cu absorption edge to higher energies relative to YBCO and the greater amplitude of certain spectral features (51, 52) (Fig. 4) show that the charge on the Cu atoms is significantly higher in YSCO-Mo. Similarly, the overlap in energy for the Mo XANES of YSCO-Mo with that of MoO₃ indicates that the Mo valence is (VI), as expected from the HPO synthesis. A second important characteristic centers on the 20,008-eV feature in MoO₃ that is assigned to the forbidden 1s → 4d transition at 20,008 eV and augmented by terminal, multiply bound, nonbridging O neighbors (53, 54). Consistent with the EXAFS, the absence of this spectral feature in the YSCO-Mo spectra demonstrates the corresponding absence of this type of O neighbor that is typical of Mo(VI).

Summarizing, the basic YBCO structure remains intact, in particular, the CuO₂ planes with their Y “glue” and the Cu1–O4–Oap₂ chains. Any impacts of the Mo substitution and higher O stoichiometry on the Cu local structure are therefore minimal. This is consistent with the clustering of the Mo that results in the excess O being located in the Mo-enriched domains, so that the local environments of most of the Cu atoms are unaffected. It is also consistent with overdoping with O₂, where the YBCO structure is retained at comparable stoichiometries. Although the substitution of the Cu1 with its +2.64 charge by Mo that is +6 takes up most of the charge from the additional O, the larger amount of O still significantly increases the charge on the Cu. This increased charge on Cu2 may also contribute to the overall contraction of the Cu1/Mo–Oap–Cu2 unit that in YSCO-Mo is intermediate in size between YBCO and the smaller YSCO. Apart from the Cu2–Oap contraction, the most notable difference relative to the structure of YBCO is that the Cu–Oap double well is shifted from its Cu1 location that is unique to YBCO, where the Cu–O bond length is 1.85 Å, to the Cu2–Oap pair with its 2.16-Å distance. This occurs for both Cu1 and Mo, both of which also display a single-site Cu–Oap distribution. This difference in the Cu–Oap distribution between Cu1 and Cu2 is one of the dynamical aspects of its structure expected for a tunneling polaron.

In attempting to explain the difference between overdoping with O₂ vs. HPO, since the overall structure is only minimally affected, the most likely change is that reaction with O₂ results in interstitial O atoms, whereas the much stronger conditions under HPO cause the observed clustering of the substitutional element and resulting nanoscale heterogeneity. Insofar as this does not alter the basic structure, the two factors that could enhance the superconductivity are therefore the dynamics (41) and modifications to the Fermi surface from the lowering of the Fermi level and the second nanophase. Changes in the dynamics are evident in the shift of the tunneling polaron from Cu1 to Cu2 and the large amplitude of the Mo–Sr pair relative to the complete damping of the Cu1–Sr. These two factors effect a division of the YBCO-type structure into two dynamical domains derived from these correlations. In YBCO, these are the CuO₂–Y–Ba–Oap and the Cu1–O4 layers, with the Cu2–Oap being the more highly correlated pair because it shows only a single position. In YSCO-Mo, the dynamically correlated domains are the CuO₂–Y–Sr, the Cu1–O4–Oap, and the Mo–O4–Sr–Oap domains. The heterogeneity would diminish the correlation lengths of the phonons to the interdomain spacing, making them intermediate between their normal size in a uniform crystal and local modes. These results for YSCO-Mo therefore point to a large role in exotic superconductivity for the dynamical properties and, in particular, dynamics outside of the harmonic regime. They also inform about the tunneling polarons that are coupled to the superconductivity (46, 55–61). The model that was used for the original theoretical analysis was the simplest one utilizing a symmetric cluster with a degenerate double well (34–36). This approximation may be valid in materials with a single CuO₂ layer or when the polaron is on the chain Cu of YBCO. However, in compounds with two or more layers where the polaron is on a CuO₂-type Cu, the square pyramidal geometry renders the two minima of the potential nondegenerate. The tunneling polaron forms within certain Cu–O bonds, but not for Mo–O. Nevertheless, it is sufficiently stable on or characteristic of Cu that the substitution of the Cu1 by Mo, or the Ba by Sr, does not eliminate it, but causes it to shift to the opposite Cu2–O pair. A final point is that since this shift occurs on all of the Cu2 ions, including the ones where the opposite ion is Mo, it is a collective rather than a local phenomenon. The significance of this observation is that the oscillatory exchange between the two conformations of the tunneling polarons would be facilitated by the cooperative action of a large number of atoms constituting a domain within a crystal where clustering of defects or substitutional atoms may already have caused the composition to differ from the bulk (62, 63). Collective behavior will then synchronize the oscillations over the domain.

Electronically, it is possible or even likely that the additional holes from the excess doping are the origin of the observed normal Fermi liquid carriers that are separate from the superconducting ones. It is possible that these reside in the Mo-enriched domains, so that this separation extends into real as well as momentum space. Insofar as the overall structure is conserved, the band structure in the Cu1-enriched domains will also be similar to that of the parent compound. If the Fermi level is lowered sufficiently, bands that are below it in the parent compound may intersect the Fermi surface (64). In addition, there is a second effect of the heterogeneity that must be considered; even if the band structure in the Cu1 domains is mostly similar to YBCO/YSCO, it will be modified within the Mo-enriched domains. The new Fermi level and second phase may cause the emergence of a second band, which has been shown to significantly enhance T_c in cuprates and other compounds (65, 66). If this does occur, then the pairing and condensation mechanism in the “overdoped” HPO cuprates may simply be an extension of the mechanism in the conventional one that does not necessarily require novel physics (23).

Materials and Methods

YSCO-Mo was the same sample as in the previous report (13) reannealed to give the same T_c, superconducting fraction, and other properties as before (SI Appendix, Fig. S2), although with a somewhat broader transition (13). Atom labels were the same as used previously (SI Appendix, Fig. S1). The material was ground with a mortar and pestle and suspended in epoxy, and the epoxy was allowed to set and cure in the bore of a 12-T magnet at Institut de minéralogie, de physique des matériaux et de cosmochimie. X-ray diffraction (XRD) analysis showed only the diffraction pattern of YSCO-Mo and the essentially complete cylindrical orientation of the YSCO-Mo along the c axis coincident with the applied field (SI Appendix, Figs. S6–S9). Its behavior was therefore identical with all other cuprates [except Sr_sCuO_3.3 (67)], giving E||c and E⊥c||aa (in the aa plane) spectra. XAFS measurements at 52 K were performed at the Stanford Synchrotron Radiation Lightsource on beamline 2-2 in the continuous-scanning mode. Transmission data were analyzed by standard methods, with one recent description in ref. 68. Curve fits of the EXAFS were performed with amplitudes and phases calculated by FEFF9 (69). The data ranges and other specific parameters are given in the text or figures. XRD data were obtained on powder diffractometers at IOP-CAS and the Commissariat à l’Énergie Atomique Saclay laboratory. More detailed descriptions of the materials and methods, the capabilities of XAFS vis-à-vis these results and their interpretations, the two-site Oap distribution, and the interpretation of the XANES are in SI Appendix.

Data Availability.

Data are available by request to S.D.C.