Abstract
The aim of this study was to analyse the front-crawl arm-pull kinetics and kinematics, comparing it before and after post-activation potentiation (PAP), and the associations between variables describing of the arm-pull kinetics. Twelve male competitive swimmers were randomly assigned to perform two different warm-ups in a crossover manner: (i) non-PAP (control condition); and (ii) PAP (experimental condition). PAP consisted of 2 × 5 arm-pulls with resistance bands by both upper-limbs. Eight minutes later, participants underwent a 25 m all-out trial in front-crawl arm-pull. Kinetics (i.e., peak thrust, mean thrust and thrust-time integral) and kinematics (i.e., speed and speed fluctuation) were collected by an in-house customised system composed of differential pressure sensors, speedo-meter and underwater camera. There was a significant and large improvement of the arm-pull kinetics after completing the warm-up with PAP sets (0.010 < P < 0.054, 0.50 < d < 0.74). There were non-significant and small effects of PAP on speed (P = 0.307, d = 0.18) and speed fluctuation (P = 0.498, d = 0.04). Correlation coefficients among kinetic variables were significant with large associations (0.51 < R < 0.90, 0.001 < P < 0.088). In conclusion, warm-ups including PAP conditioning sets elicit a large improvement in the thrust, but with small improvement in performance. Variables used to characterise thrust are strongly correlated and hence can be used interchangeably.
Subject terms: Behavioural methods, Behavioural methods, Biomedical engineering, Biomedical engineering
Introduction
Swimming is one of the most challenging locomotion techniques for humans. Human swimming is a multifactorial phenomenon, encompassing several determinants. Acceleration of human swimming is the net balance between drag force and thrust, taking into account the inertial term:
| 1 |
where a is the body’s acceleration, T the thrust, D the drag force and m the total mass. Equation 1 can be decomposed as:
| 2 |
where a is the acceleration, Th the thrust by the hands or upper-limbs, Th the thrust by the feet or lower-limbs, Df the friction drag, Dp the pressure drag, Dw the wave drag, BM the body mass and ma the added mass of water. Researchers have been conducting studies to understand the effect of drag force in human swimming by numerical simulations1, experimental testing2 and analytical procedures3. An interesting debate on how to measure human thrust is ongoing4,5. In comparison, the amount of research on the thrust production is far more limited. Nevertheless, researches making use of numerical simulations6,7, experimental testing8 and analytical procedures9 are available in the literature.
Most experimental testing protocols were conducted with robotic arms or legs mimicking the arm-pull or kicking, respectively8,10. These studies came in the wake of researches on propulsion in aquatic animals that also used bio-robots mimicking the motion, for instance, by fish11 or frogs12. Even though these studies provided valuable insights on the propulsive mechanisms in human swimming, the ecological validity of these findings are somehow limited. One can argue that despite their best attempt to mimic as much as possible the motion of human limbs swimming, this is not completely possible to be done based on these days’ technological advances. To tackle this concern, alternatively, it is possible to use tethered swimming13. In this evaluation technique, a human swimmer is invited to undergo an in-water testing session where he will swim fully or partially tethered by a string at waist level. The other end of the string is attached to a strain gauge, load cell, or any other sensor that can measure changes in the string tension. Although this technique assesses a human swimmer, some concerns have been raised on the water flow surrounding the body when tethered. When tethered, the stationary swim may affect the water flow around the swimmer’s body, and therefore interfering with propelling efficiency. Arguably, the transfer of kinetic energy to water is larger when tethered, leading to less ability to produce mechanical power to overcome the drag force. Altogether, these two techniques have advantages that cancel out the limitations of each other.
Ideally, there should exist a technique that would enable the assessment of humans in unrestricted swimming. The best option fulfilling these criteria is placing sensors on the propelling limbs of humans. A few studies have reported the use of differential pressure sensors14–16. Some of the procedures using differential pressure sensors are time-consuming, encompassing a heavy set-up, constraining the swimmer’s technique and a taxing data handling14. These challenges led to studies recruiting one to six swimmers14,17. Meanwhile, a straightforward and user-friendlier procedure is also available18. The use of this system or its components can facilitate the recruitment of a larger number of swimmers, providing a larger dataset with a better external validity. As far as our understanding goes, such report of human arm-pull thrust using differential pressure sensors during unrestricted swim in a large sample of swimmers cannot be found.
A concurrent interest is how to enhance the thrust production. Recent reports noted that the phenomenon of post-activation potentiation (PAP) can trigger a performance enhancement. PAP conditioning sets can be included as part of the warm-up routine before a race. After undergoing a PAP conditioning set, there is an increase in the muscle isometric twitch and low frequency tetanic19. I.e., the force exerted is increased due to its previous contraction during the PAP set. In the PAP set, the subject is requested to perform a high intensity resistance exercise via dynamic or isometric contraction. After sometime, known as latency period, the main exercise bout is performed20. Because there is a lag period between the main exercise bout (e.g. a sprint) and the conditioning set, PAP is most of the times incorporated in the warm-up routine. In human swimming, PAP may help to enhance the sprinting performance21–23; with mostly small effects sizes24. One study reported that PAP can induce an increase in the flutter kick thrust16. However, human kicking contributes to just 10–15% of overall swim speed25–27. Remaining 85–90% of the speed is due to arm-pull thrust. Thus, it remains to be investigated if a PAP conditioning set can enhance the arm-pull thrust and the swimming performance. If so, it might be possible to set a mechanistic relationship between PAP, thrust, swim kinematics and performance.
Assessing or monitoring the thrust in competitive swimming, it is a standard procedure to do the decomposition of the thrust-time curve. Likewise, this decomposition is a common practice in other settings where time-series of kinetic variables are collected, such as force-time or pressure-time. For instance, as the decomposition of the ground reaction force28 or the plantar pressure of the human gait29–31. In one of these studies, it was noted that the variables commonly used to characterise plantar pressures were highly inter-correlated (0.78 < R < 0.90)29. Hence, a smaller set of parameters may be more efficient for capturing the biomechanical behaviour of interest. I.e., plantar pressure parameters (peak, mean and impulse) can be reasonably compared across parameters, the authors argue. A systematic review concluded that reporting the impulse added limited value to the findings, as in the case when studying diabetic foot31. In the case of human swimming, there is not a conventional or standard procedure on the variables to be selected. Researchers reported on regular basis either the peak thrust, mean thrust or thrust-time integral. It is yet unclear to which extend these variables can be used, reported and interpreted interchangeably. If a strong association does exist, as in human gait on-land, the report of a smaller set of variables can be of interest for some researchers and practitioners. Thus, such findings can clarify this issue and help setting up a standard procedure on the variables to be reported when assessing human thrust.
The aim of this study was to: (1) analyse the front-crawl arm-pull kinetics and kinematics, comparing it before and after PAP, and (2) the associations between variables describing of the arm-pull kinetics. It was hypothesised that: (1) following PAP conditioning sets, the arm-pull kinetics as well as kinematics would improve and, as such, the performance; and (2) there would be a strong association among different kinetic variables.
Results
Figure 1 depicts the typical time-series of body’s speed and arm-pull thrust by one participant in both trials (PAP and non-PAP). There was a large improvement of the arm-pull kinetics after completing the warm-up with PAP sets (0.50 < d < 0.74) (Table 1). Worthwhile change between conditions was expected to be between 3.20% (in the case of peak thrust) and 5.54% (mean thrust). However, the percentage of individual change was much larger, ranging between 13.37% (peak thrust: P = 0.010, d = 0.74) and 18.90% (mean thrust: P = 0.054, d = 0.50). The bootstrapped 95CI of the peak thrust moved from 66.1–78.7N b and to 74.5–87.9N band. Likewise, the bootstrapped 95CI of the mean thrust moved from 23.8–32.4N to 26.6–36.7N, and of the thrust-time integral from 28.7–38.2N.s to 34.4–41.8N.s. Therefore, it was noted a meaningful improvement in arm-pull thrust after undergoing PAP warm-up.
Figure 1.
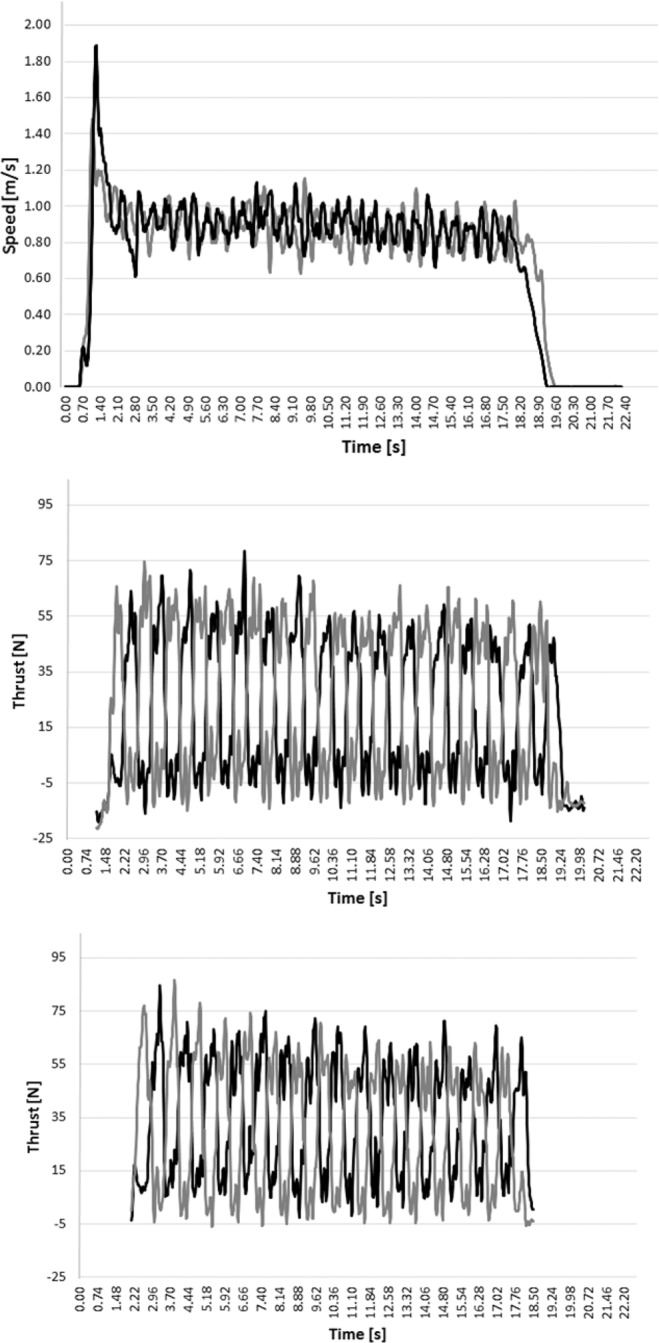
The typical time-series of one participant. Top panel: Black line depicts the body’s speed in PAP condition, whereas grey line non-PAP condition. Middle panel: Black line depicts left hand thrust and grey line right hand in non-PAP condition. Bottom panel: Black line depicts left hand thrust and grey line right hand in PAP condition.
Table 1.
The effect of post-activation potential (PAP) on arm-pull kinetics and kinematics.
| Non-PAP Mean±SD (95CI) |
PAP Mean±SD (95CI) |
Worthwhile change (% of non-PAP) |
Δ | P | |d| | |
|---|---|---|---|---|---|---|
| Peak thrust [N] | 72.3 ± 11.6 | 80.9 ± 11.9 | 2.32N | 13.37% | 0.010 | 0.74 |
| (66.1–78.7) | (74.5–87.9) | (3.20%) | ||||
| Mean Thrust [N] | 27.9 ± 7.7 | 31.9 ± 8.1 | 1.55N | 18.90% | 0.054 | 0.50 |
| (23.8–32.4) | (26.6–36.7) | (5.54%) | ||||
| Thrust-time integral [N.s] | 33.5 ± 8.6 | 38.3 ± 6.2 | 1.25N.s | 18.73% | 0.004 | 0.63 |
| (28.7–38.2) | (34.4–41.8) | (3.26%) | ||||
| Speed [m/s] | 0.84 ± 0.10 | 0.86 ± 0.09 | 0.02 m/s | 2.78% | 0.307 | 0.18 |
| (0.78–0.89) | (0.80–0.90) | (2.08%) | ||||
| Speed fluctuation [dimensionless] | 0.14 ± 0.02 | 0.14 ± 0.05 | 0.01 | 0.73% | 0.498 | 0.04 |
| (0.12–0.15) | (0.11–0.17) | (7.41%) |
SD – standard deviation, 95CI – bootstrapped 95% confidence interval, Δ – percentage of individual change, P – P-value, d – Cohen’s d.
There were non-significant and small effects of PAP on speed (P = 0.307, d = 0.18) and speed fluctuation (P = 0.498, d = 0.04) (Table 1). Worthwhile changes in speed and speed fluctuation were expected to be 2.08% and 7.41%, respectively. However, the percentage of mean individual change was just slightly higher in the case of speed (Δ = 2.78%, i.e. 0.70% above the expected worthwhile cut-off) and much smaller in the case of speed fluctuation (Δ = 0.73%, i.e. 6.68% away of the estimated 7.41%). Moreover, there is almost an overlap of the bootstrapped 95CI in both conditions in these two kinematic variables. As such, there is a trivial or small effect of PAP on speed and almost null on speed fluctuation.
All correlation coefficients between thrust variables were significant with a strong association, except the pairwise mean thrust vs. peak thrust in PAP that was non-significant but yet on the lower limit of a strong association (Table 2). Pooling the data (i.e. merging PAP and non-PAP datasets) the association between mean thrust and peak thrust was R = 0.69 (P < 0.001), between mean thrust and thrust-time integral R = 0.86 (P < 0.001), and between peak trust and trust-time integral R = 0.77 (P < 0.001). Hence, there is a significant and strong association among different kinetic variables, albeit the proportion of the variance is about 50–75% for pooled data.
Table 2.
Correlation matrix of the association among the kinetic variables during arm-pull. PAP - post-activation potential, P – P-value.
| Mean Thrust | Peak Thrust | Thrust-time integral | ||
|---|---|---|---|---|
| Non-PAP | Mean Thrust | 1.00 | ||
| Peak Thrust | 0.82 (P = 0.001) | 1.00 | ||
| Thrust-time integral | 0.90 (P < 0.001) | 0.76 (P = 0.004) | 1.00 | |
| PAP | Mean Thrust | 1.00 | ||
| Peak Thrust | 0.51 (P = 0.088) | 1.00 | ||
| Thrust-time integral | 0.81 (P = 0.001) | 0.74 (P = 0.006) | 1.00 | |
| Pooled data (Non-PAP + PAP) | Mean Thrust | 1.00 | ||
| Peak Thrust | 0.69 (P < 0.001) | 1.00 | ||
| Thrust-time integral | 0.86 (P < 0.001) | 0.77 (P < 0.001) | 1.00 |
Discussion
The aim of this study was to analyse the front-crawl arm-pull kinetics and kinematics by an experimental technique, comparing the PAP effect, and the associations between variables assessing the arm-pull thrust. The key findings were that after PAP sets, there is a large improvement in arm-pull thrust (about 13% to 19%) and a small improvement in performance (almost 3%). Variables commonly used to characterise thrust are strongly correlated (50–75% of variance).
Using differential pressure sensors on a triathlete, swimming at 0.8 m/s, thrust was noted as ranging between 20–40 N with each arm-pull14. In another study, selecting the same set-up, but at 0.90 m/s, authors reported peak force ranging between 35–50 N17. The peak force of an US Olympic champion, swimming at 1.66 m/s, was estimated to be 175 N by 3D video analysis and vector computation32. In another study, also on an US Olympic champion, but not reporting the swim speed, the peak thrust in the upsweep was 29.6 lb (i.e., 134N)33. Conversely, using a tethered technique, the mean thrust and peak thrust were 39N and 158N, respectively33. A coupled biomechanical–smoothed particle hydrodynamics fluid model estimated a peak force of 250–300 N at 1.45–1.47 m/s, on a highly-skilled Australian swimmer34. Therefore, if benchmarked with literature, and having as reference the competitive level of the swimmers recruited and the swim speed, our thrust values are within the expected range.
There was a large improvement of the arm-pull kinetics after completing the warm-up with PAP sets (0.004 < P < 0.054, 0.50 < d < 0.74). Worthwhile change was expected to range from 3.20% to 5.54%. The percentage of individual change was larger than this, being between 13.37% and 18.90%. In addition, the bootstrapped 95CI clearly shifted to the right, denoting an obvious increase in thrust production after PAP condition. A significant enhancement of kicking trust by 15% (0.40 < d < 0.66) was also reported in flutter kick after PAP conditioning sets consisting of unloaded countermovement jumps16. Hence, the relative improvement and standardised effect of PAP on arm-pull is similar to kicking.
Upper-limbs thrust (Th) can be modelled as35:
| 3 |
where ρ is water density, vR the upper limb’s velocity, Wth(r) the upper-limbs area (i.e. the width along the length of the upper-arm), CD(r) the upper-limb’s drag coefficient. One can argue that there are no significant changes in all these terms, except the vR. There is no change in ρ, Wth(r) and CD(r), unless if the swimmer makes significant changes in pitch angles7. Thus, the increase in Th must be related mostly to an increase in the limb’s velocity, which in turn, can be due to an acute enhancement of the neuromuscular mechanism.
PAP phenomenon is underpinned by a few mechanisms: (i) the phosphorylation of myosin regulatory light chains, causing the actin-myosin to be more sensitive to calcium released from the sarcoplasmic reticulum in follow-up muscle contractions and, therefore an increase in force tension36; (ii) increased synaptic excitation within the spinal cord, resulting in enhanced post-synaptic potentials, and hence increased force tension37; (iii) decreased pennation angle, increasing the mechanical advantage and force transmission to tendon-bone structures20; (iv) increased compliance by connective tissue and tendons20. Altogether, a meaningful improvement in arm-pull thrust was noted after the PAP conditioning sets.
There were non-significant and small effects of PAP on speed and almost null effects on speed fluctuation (0.307 < P < 0.498, 0.04 < d < 0.18). The percentage of individual change in speed was 2.78%, whereas the estimated worthwhile change was 2.08%. Hence, there was a small effect of PAP on swim speed. If a sprinter races the 100 m freestyle in 50 s, a 2.5–3.0% improvement in performance translates to a 0.98–1.25 s reduction in the final race time. Converting a d=0.18 to percentile gain, it represents a 7% improvement. I.e., everything else being equal, undergoing PAP can lead to moving up 7 places in a ranking featuring 100 contenders. For instance, if one is within the top-16 out of 100 contenders in a swimming event, undergoing PAP makes the sprinter jump to top-9, increasing the likelihood of going through the finals (top-8). The bootstrapped 95CI band from non-PAP to PAP shifted from 0.78–0.89 m/s to 0.80–0.90 m/s. Hence, there is 95% confidence that speed will increase by 0.01–0.02 m/s. Swimming the 100 m in 50 s, the average speed is 2.0 m/s. Increasing the average speed to 2.01 m/s and 2.02 m/s, the final race time will be 49.75 s and 49.50 s, respectively. Altogether, even though the small effect of PAP on speed, this should not be overlooked because it can have a meaningful impact on swimmer’s performance.
The thrust-time series can be decomposed into several variables to analyse and report the kinetics. Same procedure is carried out in time-series of on-land kinetic variables. Such as, ground reaction force28 and plantar pressure29–31. Due to the large amount of variables that it is possible to extract, one may wonder how redundant are they. I.e., if these variables can be interpreted interchangeably. All correlation coefficients were significant with a strong association, up to 81% of variance. For pooled data (PAP plus non-PAP sub-datasets) the variance ranged between 50 and 75%. The range of Pearson’s Correlation Coefficients match the values reported for plantar pressures (0.78 < R < 0.90)29. Another study also reported strong associations (R > 0.78) between peak pressure and pressure-time integrals30. A systematic review noted that the value of reporting pressure–time integral in addition to peak pressure data is limited in dynamic plantar pressure studies on diabetic foot31. Hence, for both human locomotion on-land and in-water, the report of the impulse (force-time or pressure-time integrals) is redundant to straightforward parameters such as peak and mean values. In some settings, such as when researchers or practitioners are facing time constrains or running the test on a large number of subjects, the report of a smaller set of variables (peak and mean values) can be very convenient. Also, the interpretation of findings across studies that report different variables is a possibility.
Two of the main findings were that after PAP conditioning sets thrust had a large improvement, whereas speed a small improvement. I.e., the proportion of improvement by performance (~2.5–3.0%) does not match the proportion of thrust enhancement (~13–19%). Thrust improved five times more than performance. Thus, it begs the question why there is a disproportional improvement in performance and thrust. In a study on the effect of PAP on flutter kick, thrust improved by 15% and performance ~10%16. It seems the efficiency of the transfer of thrust into speed is larger for kicking than for performing arm-pulls. Hence, one may wonder why is the efficiency smaller during arm-pulls. Equation 2 denotes the main swimming determinants. These encompass the thrust by lower-limbs and upper-limbs, the three drag components (friction drag, pressure drag and wave drag) and the mass to be carried out (body mass and added mass of water). As noted early on, both upper- and lower-limbs thrust increased. The added mass of water depends on the body anthropometrics, that remains unchanged38. As such, the disproportional improvement of speed with larger thrust might be explained by an increase of the drag force. Indeed swimming faster leads to larger drag force (D = k.v2). Friction drag (Df) can be estimated as3:
| 4 |
and,
| 5 |
| 6 |
| 7 |
where ρ is the density of the water, v the velocity, Awetted the wetted surface area, and CDf the friction drag coefficient, L the body length, BM the body mass, Re the Reynolds number, and υ the water kinematic viscosity. So, the increase in velocity leads to an increase in Df, as other terms will remain unchanged. Pressure drag (Dpr) is assumed as being related to bluff body separation, with viscous pressure resistance due to negligible boundary layer growth3:
| 8 |
where ρ is the density of the water, v the velocity, S the trunk transverse surface area, and CDpr the pressure drag coefficient. Again, most changes in Dpr are due to velocity and maybe CDpr, for instance, if there is any change in the limb’s 3D motor path underwater or body position that we did not monitored. Lastly, the wave drag (Dw):
| 9 |
where ρ is the density of the water, v the velocity, Awetted wetted surface area, and CDw the wave drag coefficient. CDw is strongly dependent on Froude number:
| 10 |
where v is the velocity, g the local gravitational acceleration and L the body length. Hence, the speed increase leads to larger Fr, CDw and Dw. Moreover, Dw accounts to 50% of total drag force39. Thus Dw might have played a key-role increasing the resistance to displacement and, therefore, ultimately in the smaller performance improvement.
The following limitations can be pointed out for this research: (1) the effect of different latency periods in the findings is not known; (2) the effect of PAP in other swim strokes (e.g., backstroke, breaststroke, butterfly stroke) is still unknown; (3) whether the PAP effect will be the same for distances longer than 25 m requires further investigation; (4) the system is only able to measure the normal component of the thrust vector, instead of the effective propulsive force (i.e. the propulsive force in the direction of body´s displacement). To report the effective propulsive force (i.e. the component of the thrust vector in the direction of displacement) one would need to run either a 3D kinematic analysis or, alternatively, to place IMUs on the hand/wrist. Having said that, this research is a crossover design and as such, the assumption is that in both conditions there is a not significant change in the hands’ underwater motor path and orientation. There are no reasons to believe that PAP would induce changes in the 3D motor path and hands’ orientation as compared to non-PAP.
In conclusion, after undergoing a warm-up that includes PAP sets for upper-body, there is a large improvement in arm-pull thrust and a small improvement in performance. Variables most commonly used to characterise thrust are strongly correlated and as such can be used interchangeably reporting the arm-pull thrust. PAP conditioning sets in the form of resistance band arm-pulls elicit large increases of thrust production that in turns, leads to a small enhancement of the performance.
Methods
Design
A randomised crossover research design was selected to compare the effects of a standard warm-up without a PAP set (non-PAP; control condition) and another with PAP sets (PAP; experimental condition) in the arm-pull kinetics, kinematics and performance. Participants attended three sessions. One familiarisation session with testing procedures and selection of the resistance band level. Then, one testing session of each condition. The first testing session took place 48 hours after familiarisation and second testing session one week after the first testing session. In each testing session, participants performed randomly one of the two conditions (non-PAP or PAP warm-ups) followed-up by a 25 m all-out trial in front-crawl arm-pull, with push-off start. Latency period between end of warm-up routine and in-water time trial was set at 8 minutes40.
Participants
Twelve skilful local male competitive swimmers were recruited (23.50 ± 3.35 years of age, 70.97 ± 7.91 kg of body mass, 1.76 ± 0.04 m tall, 8.08 ± 4.59 years of competitive experience). The inclusion criteria comprised: (1) males; (2) competitive swimmers; (3) racing at local, national or international competitions in the past. Exclusion criteria was as follows: (1) non-competitive swimmer (e.g. water polo players); (2) suffering from any injury or disease in the past six months; (3) unable to attend the three scheduled sessions of this study.
All procedures performed in studies involving human participants were in accordance with the ethical standards of the institutional and/or national research committee and with the 1964 Helsinki declaration and its later amendments or comparable ethical standards. The Institutional Review Board of the Nanyang Technological University approved the study. All participants had been briefed about their rights before signing a written informed consent form. In the case of under-age participants, parents and/or legal guardians were also briefed before signing a written informed consent.
Warm-up routines
Participants were randomly assigned to perform two different warm-ups in a crossover manner: (1) non-PAP (control condition) and (2) PAP (experimental condition). Latency period between each warm-up and carrying out in-water testing was set at 8 minutes16,22,41. For instance, it was compared the effects of PAP on swim start (time to 15 m) after ~15 s, 4, 8, 12 and 16 minutes in a group of international sprint swimmer. It was noted that best performance was delivered after a latency period of 8 minutes41. Warm-ups were designed based on past evidence and coaches’ experience16,42–44.
The total mileage for non-PAP warm-up was set at 1,400 m. The warm-up consisted of swimming 400 m in self-selected stroke and pace (i.e., any stroke and speed of choice), 200 m of front-crawl drills (25 m steady/25 m fast), 200 m of flutter kick drills using a kickboard (15 m fast/35 m steady), 4 × 100 m (2 front-crawls and 2 individual medleys with 10 s rest in between), 100 m (easy) and 2 × 50 m (dive followed by 15 m fast/35 m easy) of front-crawl drills.
In the PAP warm-up, participants were required to perform 700 m plus PAP sets to match the overall workload of non-PAP condition. I.e. warm-up routine was the same as in non-PAP condition, but performing half of its mileage. The warm-up consisted of swimming 200 m in self-selected stroke and pace, 100 m of front-crawl drills (25 m steady/25 m fast), 100 m of flutter kick drills using a kickboard (15 m fast/35 m steady), 2 × 100 m (1 front-crawl and 1 individual medley with 10 s rest in-between), 50 m (easy) and 50 m (dive followed by 15 m fast/35 m easy) of front-crawl drills. To induce PAP, in-water warm-up was followed-up by 5 minutes of rest before performing on-land 2 sets of 5 maximal repetitions of resistance band pull with 2 min of rest between sets by each upper-arm40,45. Resistance band level was chosen on individual basis during the familiarisation session (light-medium, medium or heavy; resistance range: 3.17–19.50 kg, 4.53–22.68 kg and 7.27–26.76 kg, respectively).
In-water testing
Participants performed the in-water testing 8 min after completing each warm-up. The in-water testing was a 25 m all-out bout, using arm-pull in front-crawl, while lower-limbs were held by a pull-buoy. Participants were instructed to minimise gliding after push-off from headwall.
Kinetics and kinematics were collected by an in-house customised system integrating and synchronising data from differential pressure sensors (Aquanex, Swimming Technologies, Florida, USA), speedo-meter (Swim speedo-meter, Swimsportec, Hildesheim, Germany) and underwater camera (Aquanex, Swimming Technology Research, Inc., USA). Differential pressure sensors were placed between 3rd and 4th proximal phalanges and metacarpals of each hand. It is assumed this place as being a good proxy of the application point of the thrust vector on the hand46,47. A larger number of sensors on each hand can lead to technique constraints because of the cabling surrounding the upper-limb. Also, increasing the number of sensors it might change the geometry and volume of the hand, having an impact on the ecological validity of the data. The sensors measure the change in pressure between inlet and outlet and then force is derived. In our case, it is measured the pressure of the water on the dorsal (low pressure field) and palmar (high pressure field) surfaces, being computed the difference between both. There is a diaphragm inside the sensor that flexes and is sensed as an electrical signal that is proportional to the difference in the two pressures. Accuracy of the system was reported elsewhere48. The speedo-meter was set on the headwall of the pool, about 0.2 m above water surface. The string of the speedo-meter was attached to a belt worn on the waist. Underwater camera was set-up 0.5 m deep on the headwall, providing an underwater view in the transverse plane.
A customised software (LabVIEW®, v.2017) was used to collect (f = 50 Hz), streaming and playback time-series data, as well as, video signal of each trial. Data was transferred from different components of the system (i.e., pressure sensors, speedo-meter and underwater camera) to software interface by a 14-bit resolution acquisition card (NI-6001, National Instruments, Austin, Texas, USA). Then, data was imported into a signal processing software (AcqKnowledge v. 3.9.1, Biopac Systems, Santa Barbara, USA). Fourteen arm-pulls by each upper-limb (overall: 28 arm-pulls) were analysed and mean values for selected kinetic and kinematic parameters were calculated for further analysis.
Multiplying the pressure by the area, the thrust is then calculated49. The kinetic variables analysed included the peak thrust (i.e., the maximal value, in N), mean thrust (in N) and thrust-time integral (in N.s) (Fig. 2). Speed (in m/s) and speed fluctuation (dimensionless) were selected as kinematic variables. Speed fluctuation can also be deemed as a proxy of energy cost. It was reported by analytical procedures and experimental testing that there is an inverse relationship, in full stroke swimming, between speed fluctuation and energy cost of transportation50.
Figure 2.
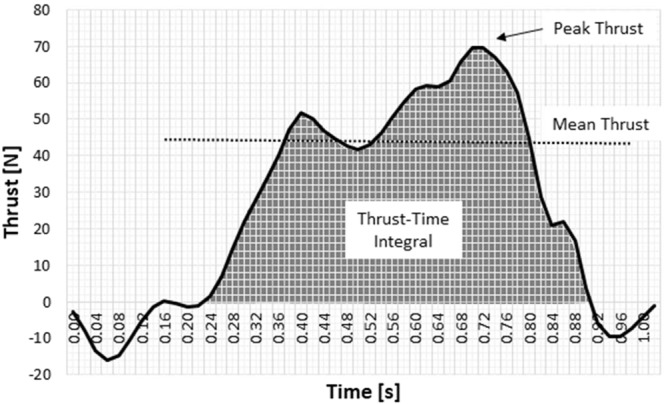
The typical thrust-time plot of an arm-pull in front-crawl and the selected kinetic variables.
Statistical analysis
Mean ± standard deviation (SD) and mean percentage of individual change are reported for all dependent variables. Uncertainty in each condition (i.e. independent variables) was computed by bootstrapping 95% confidence intervals (95CI) (1,000 samples).
Randomisation test was run to compare the differences between conditions (paired samples, 20 permutations, 1,000 repetitions, P ≤ 0.05). Cohen’s d was selected as standardised effect size of mean differences and deemed as: (i) |d | < 0.2 trivial; (ii) 0.2 < |d | ≤ 0.5 medium; (iii) |d | > 0.5 large.
Between-subjects worthwhile changes in control condition (non-PAP) were computed to examine the smallest meaningful improvement required when undergoing PAP. Worthwhile change was calculated by having d = 0.2 as the smallest standardized effect size in sports performance51. Then, worthwhile change was converted into smallest partial improvement to be expected having as reference the mean value of non-PAP condition (i.e., the smallest meaningful percentage of change expected to be meaningful from control to experimental conditions).
Pearson’s Correlation Coefficients among the three kinetic variables (peak thrust, mean thrust and thrust-time integral) were computed to assess the levels of association (P ≤ 0.05). Correlation effect sizes were deemed as: 0 < |R | ≤ 0.1 null; 0.1 < |R | ≤ 0.3 small; 0.3 < |R | ≤ 0.5 moderate; |R | > 0.5 strong. Statistical analyses were run on R.
Acknowledgements
This research was funded by NIE AcRF Grant (RI 6/17 TB), Portuguese Foundation for Science and Technology (UID04045/2020), and European Fund for Regional Development (FEDER)-COMPETE 2020 (POCI-01-0145-FEDER-006969).
Author contributions
Following authors have been involved in the design (T.B., D.L., G.B., D.M.), data collection and handling (T.B., J.W.Y.), and drafting the manuscript (T.B., J.W.Y., D.L., G.B., D.M.).
Data availability
The datasets generated and/or analysed during the current study are available from the corresponding author on reasonable request.
Competing interests
The authors declare no competing interests.
Footnotes
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
- 1.Bixler B, Pease D, Fairhurst F. The accuracy of computational fluid dynamics analysis of the passive drag of a male swimmer. Sports Biomech. 2007;6:81–98. doi: 10.1080/14763140601058581. [DOI] [PubMed] [Google Scholar]
- 2.Barbosa TM, et al. Characterization of speed fluctuation and drag force in young swimmers: A gender comparison. Hum. Mov. Sci. 2013;32:1214–1225. doi: 10.1016/j.humov.2012.07.009. [DOI] [PubMed] [Google Scholar]
- 3.Barbosa TM, et al. A comparison of experimental and analytical procedures to measure passive drag in human swimming. PloS One. 2015;10:e0130868. doi: 10.1371/journal.pone.0130868. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 4.Van Houwelingen J, et al. Effective propulsion in swimming: grasping the hydrodynamics of hand and arm movements. J. Appl. Biomech. 2017;33:87–100. doi: 10.1123/jab.2016-0064. [DOI] [PubMed] [Google Scholar]
- 5.Gatta G, Cortesi M, Zamparo P. The relationship between power generated by thrust and power to overcome drag in elite short distance swimmers. PloS One. 2016;9:e0162387. doi: 10.1371/journal.pone.0162387. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 6.Cohen RC, Cleary PW, Mason BR, Pease DL. The role of the hand during freestyle swimming. J. Biomech. Eng. 2015;37:111007. doi: 10.1115/1.4031586. [DOI] [PubMed] [Google Scholar]
- 7.Marinho DA, et al. Three-dimensional CFD analysis of the hand and forearm in swimming. J. Appl. Biomech. 2011;27:74–80. doi: 10.1123/jab.27.1.74. [DOI] [PubMed] [Google Scholar]
- 8.Nakashima M, Takahashi A. Clarification of unsteady fluid forces acting on limbs in swimming using an underwater robot arm. J. Fluid Sci. Tech. 2012;7:114–128. [Google Scholar]
- 9.Akis T, Orcan Y. Experimental and analytical investigation of the mechanics of crawl stroke swimming. Mech. Res. Commun. 2004;31:243–261. [Google Scholar]
- 10.Nakashima M, Kuwahara K. Realization and swimming performance of the breaststroke by a swimming humanoid robot. Robomech. J. 2016;3:10. [Google Scholar]
- 11.Tan, X. et al. An autonomous robotic fish for mobile sensing. 2006 IEEE/RSJ International Conference on Intelligent Robots and Systems. IEEE; 5424–5429 (2006).
- 12.Richards CT, Clemente CJ. A bio-robotic platform for integrating internal and external mechanics during muscle-powered swimming. Bioinspiration Biomimetics. 2012;7:016010. doi: 10.1088/1748-3182/7/1/016010. [DOI] [PubMed] [Google Scholar]
- 13.Morouço, P. G. et al. Relative contribution of arms and legs in 30 s fully tethered front crawl swimming. BioMed. Res. Int. 10.1155/2015/563206 (2015). [DOI] [PMC free article] [PubMed]
- 14.Takagi H, Wilson B. Calculating hydrodynamic force by using pressure differences in swimming. Biomech. Med. Swim. 1999;8:101–106. [Google Scholar]
- 15.Havriluk R. Seasonal variations in swimming force and training adaptation. J. Swim. Res. 2013;21:1–8. [Google Scholar]
- 16.Ng F, Yam JW, Lum D, Barbosa TM. Human thrust in aquatic environment: The effect of post-activation potentiation on flutter kick. J. Adv. Res. 2020;21:65–70. doi: 10.1016/j.jare.2019.10.001. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 17.Takagi H, Sanders R. Measurement of propulsion by the hand during competitive swimming. The Eng. Sport. 2002;4:631–637. [Google Scholar]
- 18.Havriluk, R. & Havriluk, M.A. U.S. Patent No. 5,258,927. Washington, DC: U.S. Patent and Trademark Office. (1993).
- 19.McGowan CJ, Pyne DB, Thompson KG, Rattray B. Warm-up strategies for sport and exercise: mechanisms and applications. Sports Med. 2015;45:1523–1546. doi: 10.1007/s40279-015-0376-x. [DOI] [PubMed] [Google Scholar]
- 20.Tillin NA, Bishop D. Factors modulating post-activation potentiation and its effect on performance of subsequent explosive activities. Sports Med. 2009;39:147–166. doi: 10.2165/00007256-200939020-00004. [DOI] [PubMed] [Google Scholar]
- 21.Sarramian VG, Turner AN, Greenhalgh AK. Effect of postactivation potentiation on fifty-meter freestyle in national swimmers. J. Strength Cond. Res. 2015;29:1003–1009. doi: 10.1519/JSC.0000000000000708. [DOI] [PubMed] [Google Scholar]
- 22.Cuenca-Fernández F, López-Contreras G, Arellano R. Effect on swimming start performance of two types of activation protocols: lunge and YoYo squat. J. Strength Cond. Res. 2015;29:647–655. doi: 10.1519/JSC.0000000000000696. [DOI] [PubMed] [Google Scholar]
- 23.Hancock AP, Sparks KE, Kullman EL. Postactivation potentiation enhances swim performance in collegiate swimmers. J. Strength Cond. Res. 2015;29:912–917. doi: 10.1519/JSC.0000000000000744. [DOI] [PubMed] [Google Scholar]
- 24.Seitz LB, Haff GG. Factors modulating post-activation potentiation of jump, sprint, throw, and upper-body ballistic performances: A systematic review with meta-analysis. Sports Med. 2016;46:231–240. doi: 10.1007/s40279-015-0415-7. [DOI] [PubMed] [Google Scholar]
- 25.Deschodt VJ, Arsac LM, Rouard AH. Relative contribution of arms and legs in humans to propulsion in 25-m sprint front-crawl swimming. Eur. J. Appl. Physiol. Occup. Physiol. 1999;80:192–199. doi: 10.1007/s004210050581. [DOI] [PubMed] [Google Scholar]
- 26.Gourgoulis V, et al. The effect of leg kick on sprint front crawl swimming. J. Sport Sci. 2014;32:278–289. doi: 10.1080/02640414.2013.823224. [DOI] [PubMed] [Google Scholar]
- 27.Bartolomeu RF, Costa MJ, Barbosa TM. Contribution of limbs’ actions to the four competitive swimming strokes: a nonlinear approach. J. Sport Sci. 2018;36:1836–1845. doi: 10.1080/02640414.2018.1423608. [DOI] [PubMed] [Google Scholar]
- 28.Davis BL, Cavanagh PR. Decomposition of superimposed ground reaction forces into left and right force profiles. J. Biomech. 1993;26:593–597. doi: 10.1016/0021-9290(93)90020-f. [DOI] [PubMed] [Google Scholar]
- 29.Keijsers NLW, Stolwijk NM, Pataky TC. Linear dependence of peak, mean, and pressure–time integral values in plantar pressure images. Gait Posture. 2010;31:140–142. doi: 10.1016/j.gaitpost.2009.08.248. [DOI] [PubMed] [Google Scholar]
- 30.Waaijman R, Bus SA. The interdependency of peak pressure and pressure–time integral in pressure studies on diabetic footwear: no need to report both parameters. Gait Posture. 2012;35:1–5. doi: 10.1016/j.gaitpost.2011.07.006. [DOI] [PubMed] [Google Scholar]
- 31.Bus SA, Waaijman R. The value of reporting pressure–time integral data in addition to peak pressure data in studies on the diabetic foot: a systematic review. Clin. Biomech. 2013;28:117–121. doi: 10.1016/j.clinbiomech.2012.12.002. [DOI] [PubMed] [Google Scholar]
- 32.Schleihauf, R. E. Propulsive techniques: front crawl stroke, butterfly, back stroke, and Breaststroke. Swimming Sci. 53-59 (1988).
- 33.Schleihauf, R. E. A hydrodynamic analysis of swimming propulsion. Swimming. 70-109 (1979).
- 34.Cohen RC, Cleary PW, Mason BR, Pease DL. The role of the hand during freestyle swimming. J. Biomech. Eng. 2015;137:111007. doi: 10.1115/1.4031586. [DOI] [PubMed] [Google Scholar]
- 35.Webb AP, et al. The effect of swimsuit resistance on freestyle swimming race time. Procedia Eng. 2014;72:709–714. [Google Scholar]
- 36.Vandenboom R, Grange RW, Houston ME. Myosin phosphorylation enhances rate of force development in fast-twitch skeletal muscle. Am. J. Physiol. 1995;268:C596–C603. doi: 10.1152/ajpcell.1995.268.3.C596. [DOI] [PubMed] [Google Scholar]
- 37.Rassier DE, Herzog W. Force enhancement following an active stretch in skeletal muscle. J. Electromyogr. Kinesiol. 2002;12:471–477. doi: 10.1016/s1050-6411(02)00041-x. [DOI] [PubMed] [Google Scholar]
- 38.Caspersen C, et al. Added mass in human swimmers: age and gender differences. J. Biomech. 2010;3:2369–2373. doi: 10.1016/j.jbiomech.2010.04.022. [DOI] [PubMed] [Google Scholar]
- 39.Vennell R, Pease D, Wilson B. Wave drag on human swimmers. J. Biomech. 2006;39:664–671. doi: 10.1016/j.jbiomech.2005.01.023. [DOI] [PubMed] [Google Scholar]
- 40.Lum D. Effects of various warm-up protocol on special judo fitness test performance. J. Strength Cond. Res. 2019;33:459–465. doi: 10.1519/JSC.0000000000001862. [DOI] [PubMed] [Google Scholar]
- 41.Kilduff LP, et al. Effect of postactivation potentiation on swimming starts in international sprint swimmers. J. Strength Cond. Res. 2011;5:2418–2423. doi: 10.1519/JSC.0b013e318201bf7a. [DOI] [PubMed] [Google Scholar]
- 42.Neiva HP, et al. The effects of different warm-up volumes on the 100-m swimming performance: a randomized crossover study. J. Strength Cond. Res. 2015;29:3026–3036. doi: 10.1519/JSC.0000000000001141. [DOI] [PubMed] [Google Scholar]
- 43.Neiva HP, et al. Warm-up for sprint swimming: Race-pace or aerobic stimulation? A randomized study. J. Strength Cond. Res. 2017;31:2423–2431. doi: 10.1519/JSC.0000000000001701. [DOI] [PubMed] [Google Scholar]
- 44.Neiva HP, et al. Warm-up and performance in competitive swimming. Sports Med. 2014;44:319–330. doi: 10.1007/s40279-013-0117-y. [DOI] [PubMed] [Google Scholar]
- 45.Baker D. Increases in bench throw power output when combined with heavier bench press plus accommodating chains resistance during complex training. J. Aust. Strength Cond. 2009;16:10–18. [Google Scholar]
- 46.Gourgoulis V, et al. Acute effect of front crawl sprint resisted swimming on the propulsive forces of the hand. J. Appl. Biomech. 2013;29:98–104. doi: 10.1123/jab.29.1.98. [DOI] [PubMed] [Google Scholar]
- 47.Sato, Y., & Hino, T. Estimation of thrust of swimmer’s hand using CFD. In Proceedings of Second International Symposium on Aqua Bio-mechanisms. pp. 81–86 (2003).
- 48.Havriluk R. Validation of a criterion measure for swimming technique. J. Swim. Res. 1988;4:11–16. [Google Scholar]
- 49.Takagi H, Sanders R. Measurement of propulsion by the hand during competitive swimming. The Eng. Sport. 2002;4:631–637. [Google Scholar]
- 50.Barbosa TM, et al. Energetics and biomechanics as determining factors of swimming performance: updating the state of the art. J. Sci. Med. Sports. 2010;13:262–269. doi: 10.1016/j.jsams.2009.01.003. [DOI] [PubMed] [Google Scholar]
- 51.Bucheit M. Chasing the 0.2. Int. J. Sports Physiol. Perf. 2016;11:417–418. doi: 10.1123/IJSPP.2016-0220. [DOI] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Data Availability Statement
The datasets generated and/or analysed during the current study are available from the corresponding author on reasonable request.


