Table 10. PP Matrix Result (as displayed in 3x4 form) and Geometric Analysis. Here we are able to compute the point source in the AP oblique. In addition, K-matrix (intrinsic matrix) components are shown including principal point, skew angle, and a simple average between alpha and beta. The plane is computed using the focal distance obtained from a quadratic solution and the normal of the detector plane. The normal of the computed detector plane relative to the normalized plane is also shown (Normal-to-Normal Angle). Note that the expected focal distance for O-arm2 is 1168 mm, whereas here we compute 1161.68 mm.

AP = Antero-Posterior; LAT = Lateral; VERT = Vertical; PP = Perspective Projection; Combined = Simple average of Alpha and Beta for focal length calculation; Alpha = alpha length from intrinsic matrix for focal length calculation; Beta = beta length from intrinsic matrix for focal length calculation

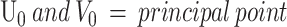
Theta = Angle used to determine skew angle (here presented in radians)

Distance from Point Source to Plane = Focal Length, which was calculated using Quadratic Solution

Normal-to-Normal Angle in degrees = detector plane normal compared to the normalized plane

PP Result
AP Matrix  (3x4)
0.706033	-1.57036	0.001052	217.2948
0.192262	0.05844	-1.70128	187.6501
0.001416	0.000382	4.55E-05	1
AP Oblique Point Source (AP, LAT, VERT):
-663.821	-160.061	29.78255
Combined	Alpha	Beta	Uo	Vo	Theta
1160.582	1158.306	1162.858	185.9213	100.8434	1.570177
Plane Calculation using Intrinsic Matrix from PP Reverse Cross Product (AP, LAT, VERT):
497.7189	0	0
494.5064	0	100
524.6757	-100	0
Focal Distance (Quadratic Solution):
1161.684
Normal-to-Normal Angle (degrees): 15.1883448297578