Cohesin and condensin extrude DNA loops in a cell cycle-dependent manner

Stefan Golfier; Thomas Quail; Hiroshi Kimura; Jan Brugués

doi:10.7554/eLife.53885

. 2020 May 12;9:e53885. doi: 10.7554/eLife.53885

Cohesin and condensin extrude DNA loops in a cell cycle-dependent manner

Stefan Golfier ^1,^2,^3,⁴, Thomas Quail ^1,^2,^3,⁴, Hiroshi Kimura ⁵, Jan Brugués ^1,^2,^3,^4,^✉

Editors: Job Dekker⁶, Kevin Struhl⁷

PMCID: PMC7316503 PMID: 32396063

Abstract

Loop extrusion by structural maintenance of chromosomes (SMC) complexes has been proposed as a mechanism to organize chromatin in interphase and metaphase. However, the requirements for chromatin organization in these cell cycle phases are different, and it is unknown whether loop extrusion dynamics and the complexes that extrude DNA also differ. Here, we used Xenopus egg extracts to reconstitute and image loop extrusion of single DNA molecules during the cell cycle. We show that loops form in both metaphase and interphase, but with distinct dynamic properties. Condensin extrudes DNA loops non-symmetrically in metaphase, whereas cohesin extrudes loops symmetrically in interphase. Our data show that loop extrusion is a general mechanism underlying DNA organization, with dynamic and structural properties that are biochemically regulated during the cell cycle.

Research organism: Xenopus

Introduction

Chromatin undergoes a dramatic reorganization during the cell cycle (Hirano and Mitchison, 1991; Rowley and Corces, 2018; Nagano et al., 2017). In interphase, chromatin is organized into compartments and topological-associating domains (TADs) that are cell-type specific (Bonev and Cavalli, 2016; Dekker and Mirny, 2016; Rao et al., 2014). TADs are composed of chromatin loops that have been hypothesized to regulate gene expression by spatially restricting contacts between genes and regulatory elements (Smith et al., 2016; Lupiáñez et al., 2015; Ren et al., 2017; Schoenfelder and Fraser, 2019). In metaphase, chromosomes undergo large-scale compaction, leading to the loss of specific boundaries and the shutdown of transcription, which is achieved by arranging chromatin into an array of condensed loops (Marsden and Laemmli, 1979; Earnshaw and Laemmli, 1983; Naumova et al., 2013; Goloborodko et al., 2016; Uhlmann, 2016; Kinoshita and Hirano, 2017). These different degrees of organization require the coordinated activity of protein complexes such as structural maintenance of chromosomes (SMCs) proteins (Nasmyth, 2001; Yatskevich et al., 2019; Fudenberg et al., 2016; Nuebler et al., 2018; Hirano et al., 1997; Bouwman and de Laat, 2015), but how these complexes organize chromatin dynamically during the cell cycle is still unknown. SMCs are thought to organize DNA by actively extruding DNA loops (Rao et al., 2014; Fudenberg et al., 2016; Alipour and Marko, 2012; Sanborn et al., 2015). Recent experimental studies have shown that yeast condensin extrudes DNA loops in a one-sided manner in vitro (Ganji et al., 2018). Although consistent with the loop-extrusion hypothesis, it is inconsistent with the requirement for two-sided loop extrusion predicted by theory (Banigan and Mirny, 2018; Banigan et al., 2019). One reason for this discrepancy could be that the properties of loop extrusion in cellular contexts differ from those in vitro and may be regulated during the cell cycle (Abramo et al., 2019; Losada et al., 1998). Notably, condensin complexes do not structure the genome during interphase (Abdennur, 2018), which raises intriguing questions about the molecular players that regulate DNA architecture in interphase. Recent in vitro work demonstrated that cohesin can extrude DNA loops symmetrically (Davidson et al., 2019; Kim et al., 2019), though this activity has not been directly visualized in cellular contexts (Rao et al., 2017; Schwarzer et al., 2017; Hansen et al., 2017). To bridge the gap between in vitro biochemical assays and physiological conditions, we used histone H3/H4-depleted Xenopus laevis egg extracts to reconstitute loop formation on single DNA molecules. These extracts can be cycled between metaphase and interphase and recapitulate many sub-cellular biological processes, such as the formation of mitotic chromatids and interphase nuclei (Hirano and Mitchison, 1991; Murray, 1991).

Results

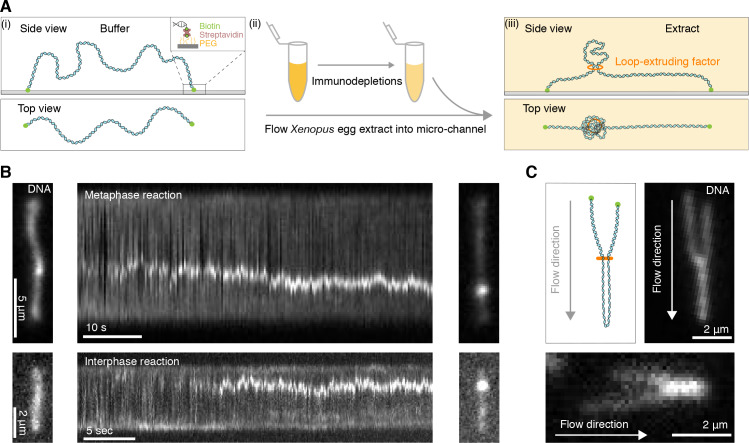
To visualize DNA loop formation in Xenopus laevis egg extracts, we attached lambda-phage DNA to a cover slide using biotin-streptavidin linkers (Ganji et al., 2016) in custom-built microfluidic chambers (Figure 1A). Addition of either metaphase-arrested or interphase Xenopus egg extracts into the chamber triggered the formation of small DNA enrichments, consistent with nucleosomal deposition (Yan et al., 2007; Gruszka et al., 2019), that rapidly reduced any slack in the DNA molecules (Figure 1—figure supplement 1A and Figure 1—videos 1–2). To increase the amount of available slack to allow for loop extrusion, we abolished nucleosomal assembly along the strand by depleting ~90–95% of soluble H3-H4 heterodimers in the extract (Zierhut et al., 2014; Figure 1—figure supplement 1B). This led to the formation of compacted DNA clusters that grew in size over time in both metaphase and interphase (Figure 1B and Videos 1–2; Figure 1—videos 3–6). To investigate whether these clusters exhibited a topology consistent with DNA loops, we hydrodynamically stretched the DNA strand by applying a flow in the perpendicular direction to the strand. This procedure revealed DNA clusters with a characteristic loop topology in both inter- and metaphase extracts (Figure 1C, Figure 1—figure supplement 1C and Figure 2—video 1; Figure 1—videos 7–9). In mock-depleted extracts, loops also formed but at a much lower frequency (Figure 1—figure supplement 1D and Figure 1—video 10) and seemed to compete with nucleosomes for available DNA slack. These results show that DNA loop extrusion can be reconstituted in Xenopus egg extracts in metaphase and interphase.

Figure 1. — (A) (i) Side and top view schematics of a single strand of λ-phage DNA attached to a functionalized cover slip via biotin-streptavidin linkers. (ii) *Xenopus* egg extract is flowed into the microfluidic chamber. (**iii**) Side and top view schematics visualizing how soluble active loop-extruding factors extrude loops in H3-H4-depleted extract. (B) Dynamics of the formation of DNA loops induced by H3-H4-depleted extract in metaphase (upper) and interphase (lower). Snapshot of a single molecule of λ-phage DNA visualized using Sytox Orange preceding treatment with H3-H4-depleted extract (left). Kymograph of DNA signal over time displaying a looping event upon addition of H3-H4-depleted extract (middle). Snapshot of steady-state DNA looping event after ~60 s (right). (C) Hydrodynamic flows reveal loop topology within DNA cluster. (i) Schematic of the loop topology revealed upon flow. (ii) Topology of extract-induced DNA loops in metaphase (upper) and interphase (lower) visualized using Sytox Orange revealed upon flow in the direction of the arrow.

Figure 1—figure supplement 1. — (A) (i) Side and top view schematics of a single strand of λ-phage DNA attached to a functionalized cover slip via biotin-streptavidin linkers. (ii) *Xenopus* egg extract is flowed into the microfluidic chamber. (**iii**) Side and top view schematics visualizing how soluble active loop-extruding factors extrude loops in H3-H4-depleted extract. (B) Dynamics of the formation of DNA loops induced by H3-H4-depleted extract in metaphase (upper) and interphase (lower). Snapshot of a single molecule of λ-phage DNA visualized using Sytox Orange preceding treatment with H3-H4-depleted extract (left). Kymograph of DNA signal over time displaying a looping event upon addition of H3-H4-depleted extract (middle). Snapshot of steady-state DNA looping event after ~60 s (right). (C) Hydrodynamic flows reveal loop topology within DNA cluster. (i) Schematic of the loop topology revealed upon flow. (ii) Topology of extract-induced DNA loops in metaphase (upper) and interphase (lower) visualized using Sytox Orange revealed upon flow in the direction of the arrow.

Video 1. Example of DNA loop formation in H3-H4-depleted egg extract arrested in metaphase visualized using Sytox Orange.

Download video file^{(78.3KB, mp4)}

Open in a new tab

The movie duration is 87 s and the scale bar is 5 μm.

Video 2. Example of DNA loop formation in H3-H4-depleted egg extract in interphase visualized using Sytox Orange.

Download video file^{(253.4KB, mp4)}

Open in a new tab

The movie duration is 80 s and the scale bar is 2 μm.

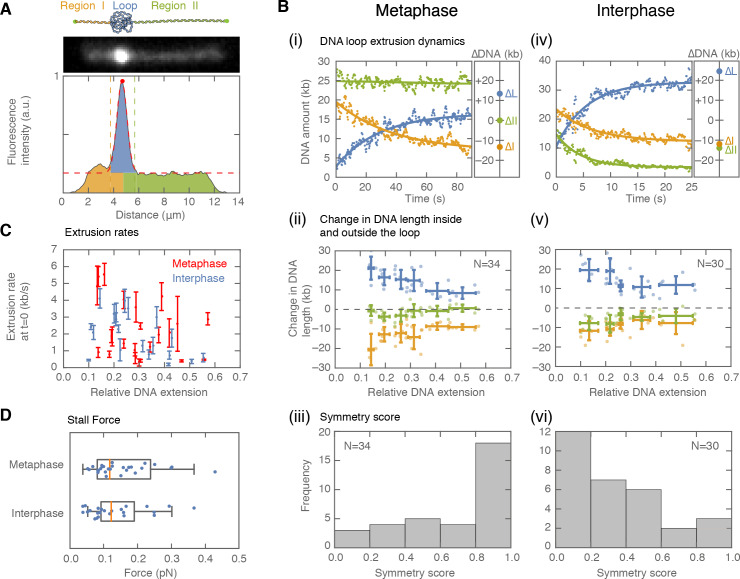
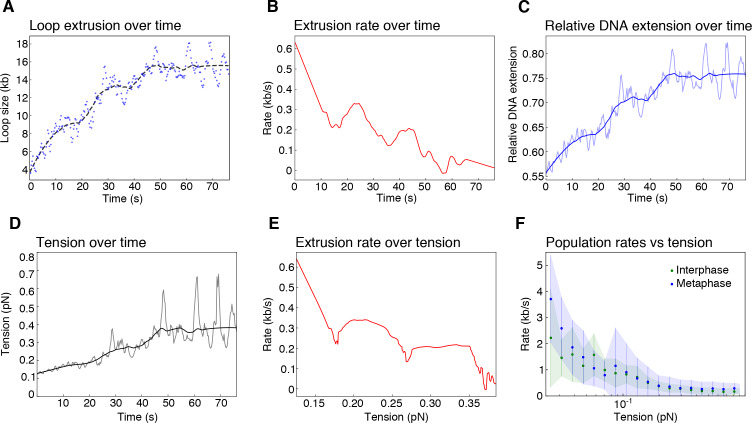
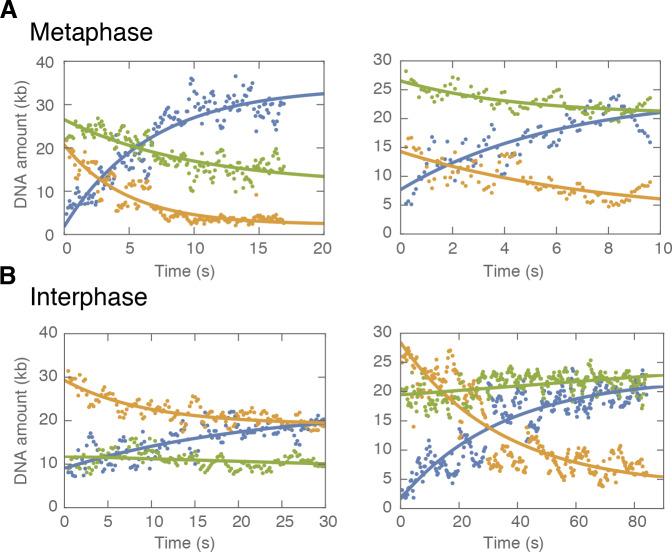
To characterize the dynamic properties of loop formation in Xenopus egg extracts, we quantified the DNA distribution inside the loop and to the left and right of the loop as a function of time (Figure 2). We computed the loop extrusion rate from the DNA amount that entered the loop over time, and could determine whether this DNA came from one or both of the non-looped regions. Briefly, we summed the fluorescence intensity of the DNA along the perpendicular direction to the DNA strand, and tracked the loop position defined by the local maximum of the DNA intensity. We then fitted a Gaussian function to the loop region and defined the loop boundaries as ±2 standard deviations away from the maximum value of the fit (Figure 2A). We obtained the amount of DNA inside the loop as the difference between the integrated intensity in the loop region minus the offset intensity from the Gaussian fit. Finally, the amount of DNA to the left and right of the loop corresponded to the integrated intensity of the DNA strands outside the loop region (see Supplementary Methods). This assay allows us to observe loop extrusion in extract, to quantify the partitioning of DNA between the looped and non-looped regions, and to examine the symmetry of the underlying DNA extrusion process.

Figure 2. — (A) Method to track DNA-loop dynamics through space and time. *Upper*: Schematic of the top view of a DNA-looping event segmented into three regions: region I (orange), region II (green), and the loop region (blue). *Middle*: Snapshot of DNA-looping event where DNA is labelled using Sytox Orange. *Bottom*: The integrated fluorescence intensity of the DNA generated by summing the intensity values along the perpendicular axis of the strand. The dashed red line represents a Gaussian fit to the data. Signal values above the fit’s offset define the looped region given in blue; signal values below this threshold correspond to the non-looped regions I and II, given in orange and in green. To convert the signal into DNA length, the integrated intensity of each region is divided by the total summed intensity of the DNA strand and multiplied by the total length of λ-phage DNA (48.5 kb). (B) Dynamics of DNA looping in H3-H4-depleted extract in metaphase and interphase. (**Bi,iv** left) DNA amount as a function of time computed for the looped region (blue) and non-looped regions I and II (green and orange). The dots represent experimental data and the solid lines represent exponential fits to the data. (**Bi,iv** right) The redistribution of DNA during the looping events shown in the left panel quantified as the change in DNA content in each region. (**Bii,v**) Change in the amount of DNA in the looped region and non-loop regions I and II (as in **Bi,iv** right) for the entire population of meta- and interphase looping events plotted as a function of the initial relative DNA extension of the corresponding molecules. Error bars correspond to standard deviations of data clustered by proximity. Points represent raw data. (**Biii, vi**) Analysis of loop extrusion symmetry shows predominantly non-symmetric extrusion (symmetry score ~1) in metaphase (**Biii**) and symmetric extrusion (symmetry score ~0) in interphase (**Bvi**). (C) Initial growth rates of DNA loop extrusion in metaphase (red) and interphase (blue) as a function of initial relative DNA extension. These rates were obtained from the slopes of the exponential fits to the loop data at time t = 0 for the subset of loop extrusion events that allowed for a fitting that converged within a tolerance (10⁻⁸relative change of the cost function), that corresponded to N = 21 out of 30 in interphase, and N = 24 out of 34 for metaphase. Error bars were obtained from error propagation of the uncertainties of the exponential fit parameters. (D) Box plots of the stall forces for DNA loop extrusion in metaphase and interphase obtained from the final relative extension of the DNA strand at the end of loop formation.

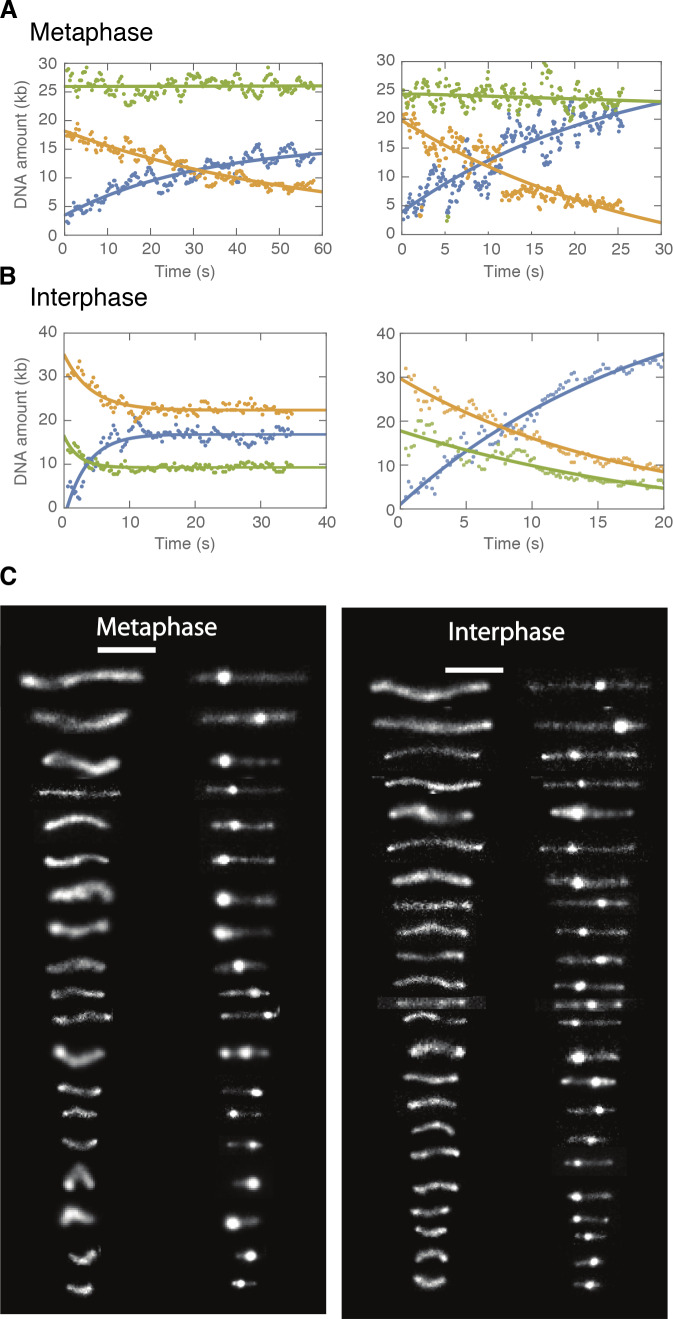
Figure 2—figure supplement 1. — (A) Method to track DNA-loop dynamics through space and time. *Upper*: Schematic of the top view of a DNA-looping event segmented into three regions: region I (orange), region II (green), and the loop region (blue). *Middle*: Snapshot of DNA-looping event where DNA is labelled using Sytox Orange. *Bottom*: The integrated fluorescence intensity of the DNA generated by summing the intensity values along the perpendicular axis of the strand. The dashed red line represents a Gaussian fit to the data. Signal values above the fit’s offset define the looped region given in blue; signal values below this threshold correspond to the non-looped regions I and II, given in orange and in green. To convert the signal into DNA length, the integrated intensity of each region is divided by the total summed intensity of the DNA strand and multiplied by the total length of λ-phage DNA (48.5 kb). (B) Dynamics of DNA looping in H3-H4-depleted extract in metaphase and interphase. (**Bi,iv** left) DNA amount as a function of time computed for the looped region (blue) and non-looped regions I and II (green and orange). The dots represent experimental data and the solid lines represent exponential fits to the data. (**Bi,iv** right) The redistribution of DNA during the looping events shown in the left panel quantified as the change in DNA content in each region. (**Bii,v**) Change in the amount of DNA in the looped region and non-loop regions I and II (as in **Bi,iv** right) for the entire population of meta- and interphase looping events plotted as a function of the initial relative DNA extension of the corresponding molecules. Error bars correspond to standard deviations of data clustered by proximity. Points represent raw data. (**Biii, vi**) Analysis of loop extrusion symmetry shows predominantly non-symmetric extrusion (symmetry score ~1) in metaphase (**Biii**) and symmetric extrusion (symmetry score ~0) in interphase (**Bvi**). (C) Initial growth rates of DNA loop extrusion in metaphase (red) and interphase (blue) as a function of initial relative DNA extension. These rates were obtained from the slopes of the exponential fits to the loop data at time t = 0 for the subset of loop extrusion events that allowed for a fitting that converged within a tolerance (10⁻⁸relative change of the cost function), that corresponded to N = 21 out of 30 in interphase, and N = 24 out of 34 for metaphase. Error bars were obtained from error propagation of the uncertainties of the exponential fit parameters. (D) Box plots of the stall forces for DNA loop extrusion in metaphase and interphase obtained from the final relative extension of the DNA strand at the end of loop formation.

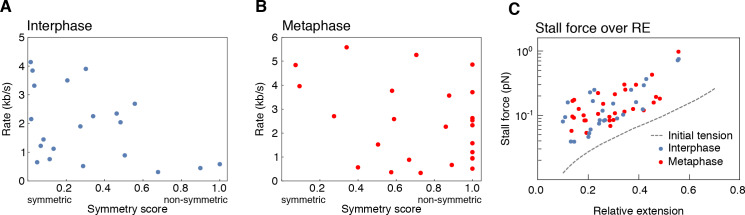
When applied to metaphase-arrested, H3-H4-depleted extract (n=7 extract days), this assay showed that DNA loops are initially extruded at 2.36 ± 0.35 kb/s (mean ± SEM) (Figure 2Bi, C; Figure 2—figure supplement 1A). However, loop growth rapidly slowed down as more DNA was pulled into the looped region (Figure 2—figure supplement 2A), suggesting that extrusion rates depend on DNA tension. To examine the relationship between loop extrusion and DNA tension, we used the worm-like chain model (Marko and Siggia, 1995) that relates the relative extension of the DNA outside the loop to the corresponding force on the DNA strand (see supplementary methods, Figure 2—figure supplement 2 and Figure 2—figure supplement 26). The relative extension outside the loop is a dynamic quantity defined as: RE(t)=L/(CL_λ-DNA_loop(t)), where L represents the end-to-end binding distance of the DNA molecule on the coverslip, CL_λ is the contour length of lambda-phage DNA, and DNA_loop is the amount of DNA in the looped region. These results show that the relationship between extrusion rates and DNA tension is generally conserved for all looping events (Figure 2—figure supplement 2F). Finally, loop extrusion stopped when the relative extension of DNA outside of the loop reached on average ~65%, corresponding to a stall force of 0.16 pN ± 0.01 pN (mean ± SEM), (Figure 2D). In rare cases, we observed individual looping events stalling at DNA extensions of up to ~85%, corresponding to forces up to ~1 pN (Figure 2—figure supplement 2C–5C).

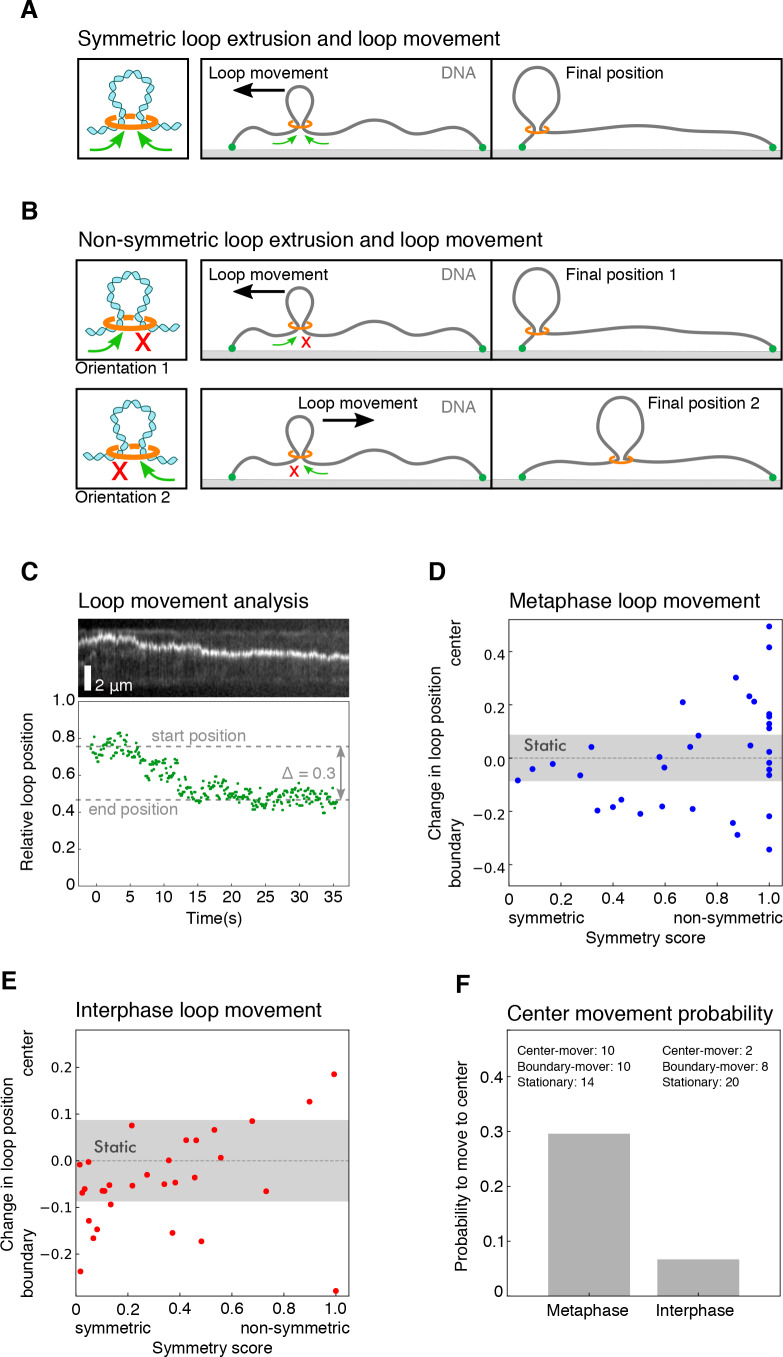
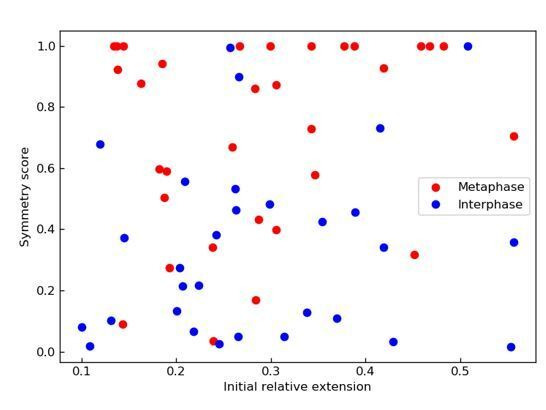
To characterize the extrusion symmetry in metaphase, we quantified the total decrease in DNA from the left and right regions of the loop between the onset of loop formation and the final steady-state size of the loop (Figure 2Bii). We used these quantities to define a symmetry score as the relative difference between the decrease of these two regions and the total amount of DNA extruded (supplementary methods). The majority of metaphase looping events had a symmetry score close to 1, which corresponds to one-sided (non-symmetric) loop extrusion (Figure 2Biii). However, a small population of ~20% of all metaphase looping events were two-sided (as defined by a symmetry score of less than 0.5, Figure 2—figure supplement 3A). To complement these symmetry score results, we tracked loop movement along the DNA strand (Figure 2—figure supplement 4). Consistent with one-sided loop extrusion, loops that were displaced during loop formation, moved with equal probability towards the boundaries or the center of the strand. This behavior is suggestive of non-symmetric DNA extruding factors landing in a random orientation on the DNA molecule. Taken together, our analysis demonstrates that DNA loop extrusion in metaphase is predominantly one-sided, with extrusion speeds and stall forces similar to those measured in vitro (Ganji et al., 2018; Strick et al., 2004; Kong et al., 2019).

Next, we used interphase H3-H4-depleted extract (n = 8 extract days) to investigate whether the dynamics of loop extrusion share similar properties throughout the cell cycle (Figure 2 Civ-vi). Loop extrusion in interphase displayed a similar distribution of extrusion rates, with a mean of 1.94 ± 0.26 kb/s, and average stall forces of 0.18 pN ±0.03 pN, with maximal forces of up to 0.82 pN (Figure 2C and D, Figure 2—figure supplement 2F). However, the distribution of symmetry scores of these looping events peaked towards zero, indicating that these loops are symmetrically extruded. Similar to metaphase looping, we observed that a sub-population of ~20% of all loops had the opposite symmetry (symmetry score larger than 0.5, Figure 2—figure supplement 3B). As predicted for symmetric extrusion, loops that started off-center on the strand displayed a strong bias to move towards the DNA boundary of the shorter DNA portion (Figure 2—figure supplement 4). Thus, we conclude that the mechanisms of DNA loop extrusion are different in interphase and metaphase.

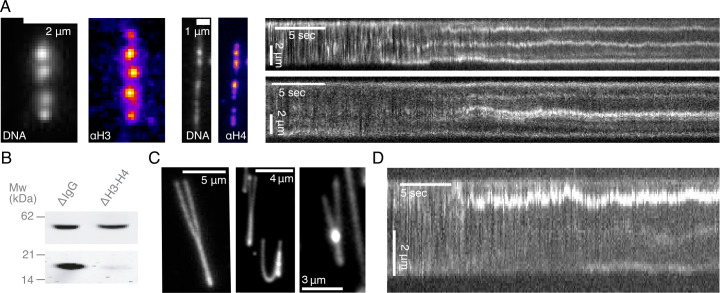
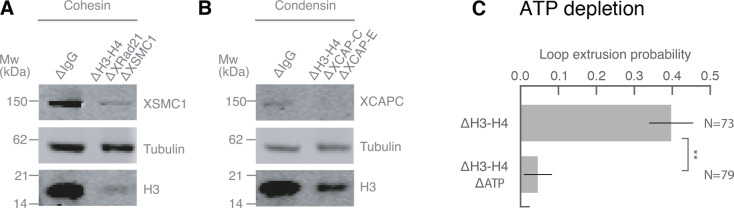
The different dynamic properties of DNA loop formation that we observe in interphase and metaphase suggest that different molecular activities may be responsible for loop formation during the cell cycle (Dekker and Mirny, 2016; Losada et al., 1998). The cell cycle-dependent activities of condensin and cohesin could account for the transition between symmetric and non-symmetric loop extrusion (Banigan et al., 2019; Abramo et al., 2019). To assess the role of cohesin and condensin during loop extrusion in interphase and metaphase, we selectively depleted these protein complexes in Xenopus egg extract. We used custom-made antibodies against XSMC1 and XRad21 for cohesin, and XCAP-C and XCAP-E (SMC2 and SMC4) for simultaneous depletion of condensin I and II (Figure 3—figure supplement 1). We then tested for loop extrusion activity in each depleted condition. We found that, in metaphase (n = 3 extract days), the occurrence of DNA loop extrusion was significantly reduced (p<0.01) upon depletion of condensin I and II but was unaffected by cohesin depletion (Figure 3A). In contrast, there was a significant decrease (p<0.01) in loop extrusion following cohesin depletion in interphase (n = 3 extract days), but was unaffected by condensin depletion (Figure 3A). We confirmed these depletions with immunostainings that showed colocalization of cohesin and condensin with the DNA loops observed in interphase and metaphase, respectively (Figure 3B). Additionally, we tested for ATPase activity of the loop extrusion factors by enzymatically depleting ATP using apyrase—which for technical reasons was limited to interphase in extract (see supplementary methods). When applied to interphase extract, apyrase-mediated ATP depletion resulted in a near-complete elimination of DNA looping activity , suggesting that cohesin actively extrudes loops in an ATP-dependent manner. Altogether, our results show that cohesin actively extrudes DNA loops symmetrically during interphase, whereas condensin extrudes DNA loops non-symmetrically in metaphase. This demonstrates that the molecular mechanisms of DNA loop extrusion are differentially regulated during the cell cycle.

Figure 3. — (A) DNA loop extrusion probability—the frequency at which looping occurs on a DNA strand with sufficient slack—in metaphase and interphase under different depletion conditions. In metaphase, co-depleting condensin I, condensin II, and H3-H4 (using anti-XCAP-C/E and anti-H4K12Ac) significantly (** represents p<0.01, Binomial test) reduced loop extrusion probability, whereas the same depletion condition in interphase had no effect on loop extrusion probability compared to the control H3-H4-depleted extract. However, co-depleting cohesin and H3-H4 (using anti-XRAD21/XSMC1 and anti-H4K12Ac) had no effect in metaphase, though significantly (p<0.01) decreased loop extrusion probability in interphase compared to H3-H4-depleted extract. (B) Snapshots of antibody stainings of representative loops in metaphase and interphase. (Top) In metaphase, Alexa488-labeled anti-XRad21 bound to cohesin does not localize to the DNA loop, whereas in interphase (right panels), the anti-XRad21 co-localizes to the loop. (Bottom) Alexa488-labeled anti-XCAP-C bound to condensin localizes to the DNA loop in metaphase, but does not localize to the loop in interphase.

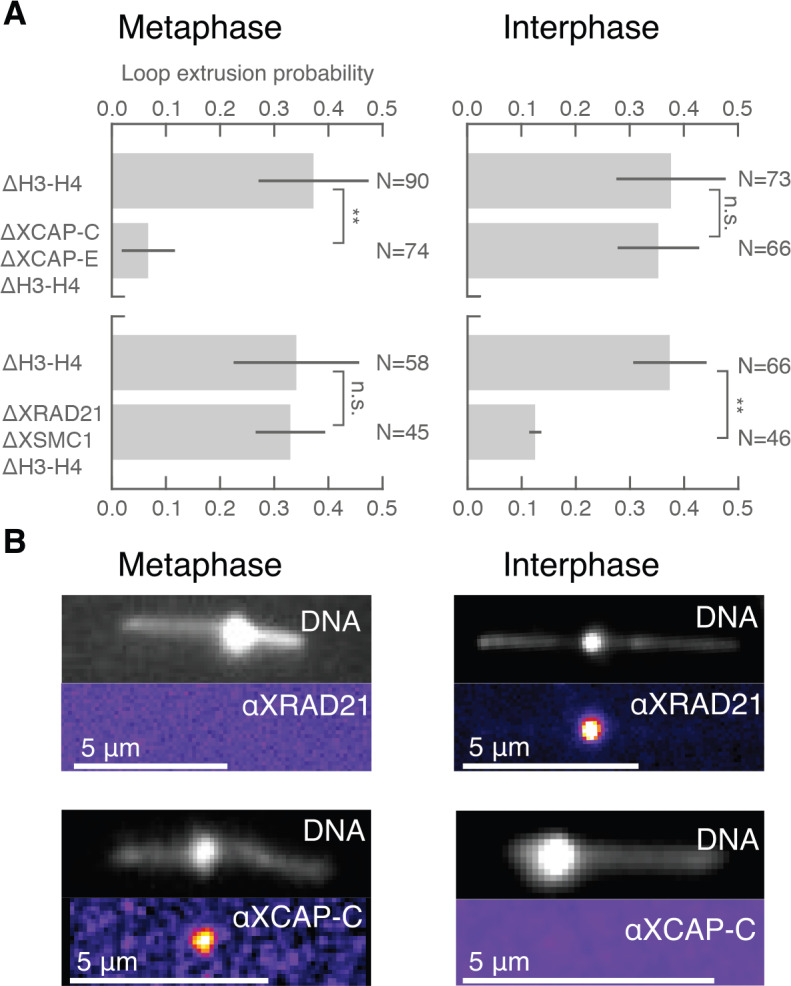
Figure 3—figure supplement 1. — (A) DNA loop extrusion probability—the frequency at which looping occurs on a DNA strand with sufficient slack—in metaphase and interphase under different depletion conditions. In metaphase, co-depleting condensin I, condensin II, and H3-H4 (using anti-XCAP-C/E and anti-H4K12Ac) significantly (** represents p<0.01, Binomial test) reduced loop extrusion probability, whereas the same depletion condition in interphase had no effect on loop extrusion probability compared to the control H3-H4-depleted extract. However, co-depleting cohesin and H3-H4 (using anti-XRAD21/XSMC1 and anti-H4K12Ac) had no effect in metaphase, though significantly (p<0.01) decreased loop extrusion probability in interphase compared to H3-H4-depleted extract. (B) Snapshots of antibody stainings of representative loops in metaphase and interphase. (Top) In metaphase, Alexa488-labeled anti-XRad21 bound to cohesin does not localize to the DNA loop, whereas in interphase (right panels), the anti-XRad21 co-localizes to the loop. (Bottom) Alexa488-labeled anti-XCAP-C bound to condensin localizes to the DNA loop in metaphase, but does not localize to the loop in interphase.

Discussion

Our findings provide the first direct evidence that loop extrusion is a general mechanism of DNA organization in a cellular context, and, furthermore, that it is differentially regulated during the cell cycle. This regulation is achieved by the distinct activities of cohesin (Rao et al., 2017; Schwarzer et al., 2017; Losada et al., 1998) and condensin (Kinoshita and Hirano, 2017; Hirano et al., 1997; Shintomi et al., 2017) during interphase and metaphase, and may control different levels of DNA organization during the cell cycle: from chromatin that is mostly decondensed and spatially organized into TAD structures during interphase to highly compacted chromosomes in metaphase (Abramo et al., 2019). Symmetric loop extrusion by cohesin in interphase may ensure the formation of specific TAD boundaries by bringing together distal CTCF sites (Sanborn et al., 2015; Tang et al., 2015). In metaphase, reorganization of loosely packed interphase chromatin into condensed chromosomes leads to the loss of TAD boundaries and the shutdown of transcription (Nagano et al., 2017; Naumova et al., 2013), which may be achieved by condensin activity (Goloborodko et al., 2016). However, many questions remain regarding how the cell cycle regulates condensin and cohesin activities. Previous studies have shown that condensin binds to chromatin in metaphase, but is largely undetected on chromatin in interphase (Hirano and Mitchison, 1994); whereas cohesin is bound to chromatin in interphase, but not as strongly in metaphase (Losada et al., 1998; Sumara et al., 2000). The CDK1-mediated phosphorylation of condensin HEAT subunits in metaphase may be the biochemical signal that triggers the association of condensin to chromatin (Hirano et al., 1997). In contrast, most cohesin is released from chromatin by a mechanism that involves the phosphorylation of cohesin’s SA subunit (Losada and Hirano, 2001; Hauf et al., 2005). Thus, the different affinities of condensin and cohesin for chromatin during the cell cycle could be a natural explanation for the different DNA loop extrusion activities that we see in our experiments.

Our demonstration of predominantly non-symmetric DNA loop extrusion during metaphase is consistent with recent in vitro data, but it is at odds with the theoretical requirements to fully compact chromosomes in metaphase (Banigan and Mirny, 2018; Banigan et al., 2019). However, these studies suggest that a small fraction of two-sided loop extruders—including extrusion events that reel in DNA at different rates from left and right—can facilitate higher levels of chromosome compaction. Our metaphase data suggest that loops with symmetry scores below 0.8 could be considered ‘slow’ two-sided extrusion events, as DNA is reeled into the loop from both sides, but at different rates. These events account for about 50% of the total population of metaphase looping events, which, according to theoretical predictions, could be sufficient to achieve 100-fold linear chromosome compaction (Banigan and Mirny, 2018). Thus, the mixed populations of loop extrusion symmetries we observe could play a crucial role for proper chromosome organization in metaphase. What is the origin of the small population of symmetric loop extrusion in metaphase? One possibility is that condensin I and condensin II compact DNA using different symmetries (Kong et al., 2019). In Xenopus egg extract, the relative abundances of condensin I and condensin II is roughly 5:1 (Ono et al., 2003), which would be consistent with the fraction of nearly symmetric loop extrusion events that we observe in metaphase (~20%). One limitation of our work, however, is that our antibodies simultaneously depleted those two complexes. As a consequence, we cannot rule out that the small population of symmetric loop extrusion may arise from residual cohesin activity in metaphase. In the future it will be interesting to investigate the origin of the different looping symmetries by using specific antibodies for condensin I and II. In addition, we observe a small sub-population (~20%) of non-symmetric loop extrusion events in interphase, suggesting a differential regulation of extrusion symmetries in both cell cycle phases.

Despite the differences in loop extrusion symmetries between interphase and metaphase, extrusion rates and stall forces seem to be conserved during the cell cycle. The mean extrusion rates we observe, however, are three to four times higher than those observed in previous in vitro studies (Ganji et al., 2018; Kim et al., 2019) for cohesin and condensin respectively. One possibility for this discrepancy could be that, in extract, several extruding factors participate in the extrusion of the same DNA loop in a cooperative manner. However, the average stall forces we estimate are about five to seven times lower than previous estimates in vitro (Ganji et al., 2018; Kim et al., 2019). We speculate that in cytoplasmic context of the H3-H4-depleted egg extract, many other DNA-binding factors—such as linker histone (Xiao et al., 2012)—may compete with the loop extrusion machinery for DNA slack. The large spread in the distribution of stall forces, with individual examples reaching values that compare to those reported in vitro, may suggest that secondary factors could cause the loop extrusion machinery to stop prematurely, and, consequently, we may underestimate the magnitude of the looping stall forces. We wonder, however, how condensin and cohesin share such similar extrusion rates, even though condensin predominantly extrudes non-symmetrically while cohesin extrudes loops symmetrically. The similar loop growth velocities would suggest that condensin reels in DNA from one side at twice the rate that cohesin reels in DNA from each of its two sides. This assumes, however, that cohesin functions by simultaneously extruding DNA from two sides. Alternatively, cohesin may be a one-sided motor that alternates its extrusion direction (Banigan et al., 2019)—though we did not observe this kind of switching within the temporal resolution of our measurements. We speculate that symmetric cohesin loop extrusion could originate from the dimerization of two identical non-symmetric motors, though recent in vitro work shows that this idea is controversial (Davidson et al., 2019; Kim et al., 2019). Interestingly, our results comparing extrusion velocities and corresponding symmetries in interphase show that, on average, symmetric loop extrusion rates are higher (roughly twice) compared to the non-symmetric events (Figure 2—figure supplement 5A). This difference in extrusion rates would be consistent with symmetric and non-symmetric loop extrusion mediated by a dimer and a monomer respectively. Our assay will allow for the dissection of the biochemical underpinnings of these processes, and more generally make it possible to reconstitute complex processes such as the formation of boundary elements and the interplay between transcription, replication, and loop extrusion in cellular contexts.

Materials and methods

Xenopus laevis egg extract preparation, immunodepletions, and ATP depletion

Cytostatic factor (CSF)-arrested Xenopus laevis (RRID:XEP_XIa_100) egg extract was prepared as described previously (Murray, 1991). In brief, unfertilized oocytes were dejellied and crushed by centrifugation, generating an extract that was arrested in meiosis II. We added protease inhibitors (LPC: leupeptin, pepstatin, chymostatin) and cytochalasin D (CyD) to a final concentration of 10 μg/ml each to the extract. In order to generate interphase extracts, CaCl₂ was added to a final concentration of 0.4 mM. To immunodeplete soluble H3-H4 heterodimers from the extract (Zierhut et al., 2014), we coupled 130 μg of a mouse monoclonal anti-H4K12Ac to 12.5 μl rProtein A Sepharose (GE Healthcare) slurry in antibody coupling buffer (10 mM K-HEPES pH = 8, 150 mM NaCl), rotating overnight at 4°C. After several washes with a wash buffer (10 mM HEPES pH = 7.7, 100 mM KCl, 150 mM Sucrose, 1 mM MgCl₂), we combined 50 μl fresh CSF extract with the beads and incubated the bead-extract mixture for 1.5 hr on ice, occasionally flicking the tubes in order to prevent the beads settling to the bottom. After recovering the extract from the beads, we immediately proceeded with the experiment. We generated mock-depleted extracts with the same protocol using 130 μg random mouse IgG antibodies (IgG from Mouse (polyclonal)-unconjugated, Jackson Immuno Research) in 50 μl of fresh CSF extract. To co-deplete H3-H4 and both condensin I and condensin II, we coupled 130 μg anti-H4K12Ac and 10 μg rabbit polyclonal antibodies of both anti-XCAP-C and anti-XCAP-E to 15 μl rProtein A Sepharose slurry and performed the same H3-H4 depletion method. To co-deplete H3-H4 and cohesin, we coupled 130 µg anti-H4K12Ac and 10 μg rabbit polyclonal anti-XRad21 and 10 μg anti-XSMC1 to 15 μl rProtein A Sepharose and performed the same H3-H4 depletion method. ATP was depleted by adding 0.03 U/μl apyrase (A6410; Sigma-Aldrich) to the extract reaction in the presence of 5 mM CaCl₂, followed by a 15 min incubation at room temperature. The ATP-depleted extract was then introduced into the DNA channels as described below.

Western blots

We prepared 1:25 dilutions of immunodepleted extract in 1X sample loading buffer (50 mM Tris-HCl, pH = 6.8, 2% SDS, 10% glycerol, 0.006% bromophenol blue, 100 mM DTT), ran a gel electrophoresis on a gradient gel, transferred to a nitrocellulose membrane with a semi-dry transfer approach, and performed primary antibody incubation with polyclonal rabbit antibodies anti-H3 (1:10000, ab1791, RRID:AB_302613), anti-XSMC1 (1:2500, MPI-CBG antibody facility), anti-XCAP-C (1:2000, MPI-CBG antibody facility) and monoclonal mouse antibodies to detect tubulin using anti-DM1a (1:10000, MPI-CBG antibody facility). We detected primary antibodies using LI-COR IRDye secondary antibodies and imaged the western blots using an Odyssey Infrared Imaging System. We analyzed the blots using FIJI.

Antibody production and labeling

We raised rabbit polyclonal antibodies for immunodepletion against peptides SDIVATPGPRFHTV and DLTKYPDANPNPND corresponding to antibodies that targeted cohesin’s XRAD21 and XSMC1 subunits. We also raised rabbit polyclonal antibodies against peptides AAKGLAEMQSVG and SKTKERRNRMEVDK corresponding to antibodies that targeted XCAP-C and XCAP-E for both condensin I and II for immunodepletion (Hirano et al., 1997). We added a cysteine residue on the peptide’s N-terminus for sulfhydryl coupling, and subsequent keyhole limpet hemocyanin conjugation and affinity purification was performed by MPI-CBG antibody facility. We labeled antibodies with fluorophores for localization using the small-scale on-resin labeling technique from Groen et al., 2014. Briefly, we prepared a 200 μl pipette tip to act as our resin bed. We then loaded 40 μl of rProtein A Sepharose (GE Healthcare) resin into the tip, washing three times with 10 mM K-HEPES (pH = 7.7), 150 mM NaCl. We labeled both the antibody targeting the cohesin subunit XRad21 and the antibody targeting condensin I and II’s subunit XCAP-C. We flowed 70 μg antibody 5 times consecutively through the packed resin bed in order to bind the antibody to the resin. The resin was then washed three times with 200 mM K-HEPES (pH = 7.7). We then added 0.5 μl 50 mM NHS-Ester-Alexa488 (Alexa Fluor NHS Ester, A20000, Thermo Fischer) to 25 μl 200 mM K-HEPES (pH = 7.7), and immediately added it to the resin, incubating the resin, antibody, and dye for 60 min at room temperature. To remove the unbound dye, the resin bed was washed 5 times with 10 mM K-HEPES (pH = 7.7), 150 mM NaCl. We eluted the labelled antibody with 5 × 15 μl of 200 mM acetic acid. We neutralized each eluate immediately with 5 μl of 1 M Tris-HCl, pH = 9, and cooled to 0°C on ice. The labelled antibody is stable for months kept at 4°C.

DNA functionalization

To biotinylate DNA purified from lambda-phage (λ-DNA) (Smith et al., 1996), we combined 10 μg of λ-DNA (NEB, N3011S) and 5 μl of a 10X polymerase buffer (50 mM Tris-HCl, pH = 7.2, 10 mM MgSO_4,100 μM DTT) to a total reaction volume of 50 μl. We then heated the mixture up to 65°C for 7 min to break apart the λ-DNA’s sticky ends. After heat treatment, we added 100x molar excess of biotinylated dATP, biotinylated dUTP, and dGTP, and dCTP. We then added one unit (~1 μl) of Klenow enzyme, mixed well, and incubated overnight at room temperature. We purified the biotinylated λ-DNA using ethanol precipitation and stored aliquotes at −20°C.

PEGylation of cover slips and DNA micro-channel preparation

We functionalized glass cover slips with mPEG and PEG-Biotin. We sonicated coverslips first in acetone for 15 min followed by 5 rinses with MilliQ water, and then another sonication step in 5 M KOH for 40 min. After rinsing the coverslips 3 times with water and then 3 times with methanol, we dried the coverslips with N₂. We silanized the coverslips combining 250 ml methanol, 12.5 ml acetic acid, and 2.5 ml (3-aminopropyl)-trimethoxysilane, incubating the coverslips in this mixture for 10 min at room temperature, sonicating for 1 min, and then incubating the coverslips for an additional 10 min. Next, we rinsed the coverslips once with methanol, once with water, and once again methanol, and dried with N_2. Then we mixed 100 mg mPEG and ~1.5 mg Biotin-PEG with 450 μl PEGylation buffer (0.1M Sodium Bicarbonate, pH = 8.5), and spun the reaction at 10.000 RPM for 1 min to remove air bubbles. We pipetted 25 μl of the PEG mixture onto a dried, silanized coverslip and put another coverslip on top, generating a coverslip sandwich. We incubated these sandwiches over night in distilled water-filled pipette tip-boxes in the dark. After incubation, we carefully disassembled the coverslips, rinsed with MilliQ water, and dried with N_2. To generate a channel for imaging, we first drilled holes through a cleaned cover slide—these holes acted as channel inlets and outlets. We placed custom-designed, laser-cut double-sided tape onto the coverslip, defining the channel geometry. We then placed a functionalized PEG-biotinylated coverslip on top of the double-sided tape, sealing the channel on either end with Valap. We filled the channel with ~10–15 μl of 0.1 mg/ml free streptavidin, incubating the channel with streptavidin for 1 min. To remove the free, unbound streptavidin, we flushed ~100 μl channel washing buffer (40 mM Tris-HCl, pH = 8.0, 20 mM NaCl, 0.4 mM EDTA) through the channel, using the drilled holes as channel inlets and outlets. We added 20 μl of 1:1000 biotinylated λ-DNA (~5 pM), incubating it for ~1 min and then washed the channel with 3 × 100 μl of channel washing buffer.

Imaging

For live imaging of looping events, we fluorescently stained immobilized DNA strands with 50–500 nM Sytox Orange (S11368, ThermoFisher), a DNA intercalating dye, in imaging buffer (50 mM Tris-HCl pH 7.7, 50 mM KCl, 2.5 mM MgCl₂, 2 mM ATP) similar to Ganji et al., 2018 or Xenopus Buffer (XB: 100 mM KCl, 1 mM MgCl₂, 0.1 mM CaCl₂, 2 mM ATP). We excited Sytox Orange-labelled DNA using a 561 nm laser, and imaged the strands using a Nikon Eclipse microscope stand with a Nikon 100x/NA 1.49 oil SR Apo TIRF and an Andor iXon3 EMCCD camera using a frame-rate of 100–300 ms. A highly inclined and laminated optical sheet (HILO) microscopy mode was established using a Nikon Ti-TIRF-E unit mounted onto the microscope stand to improve signal-to-noise ratio by excluding background fluorescence signal from unbound DNA dye in the buffer. To trigger the formation of DNA loops, we flowed about 2 ul of H3-H4-depleted extract into the channel (total channel volume ~10 ul) and let the extract diffuse further down the channel. We then imaged looping events at the moving front of the diffusing extract. A typical field of view contained 5–20 individual DNA molecules with typically between 2–7 strands having sufficient slack to support loop extrusion. Of these about 30% displayed looping events (Figure 3). As we could not control the concentration of loop extrusion factors, the majority of looping events displayed competition between two loops on the same strand. For this study we selected DNA strands that contained only a single looping event per strand.

Hydrodynamic stretching of loops

To visualize DNA loop topology which cannot be observed in the normal mode of data acquisition, we hydrodynamically stretched DNA strands that exhibited looping events using a flow-controlled syringe pump (Pro Sense B.V., NE-501), see also Figure 2—video 1; Figure 1—videos 7–9. The flow direction was set to be perpendicular to the strand orientation by a cross-shaped channel design. Depending on the width of the channel, we used flow rates between 100 μl/min and 500 μl/min to extend DNA loops. Specifically, we introduced H3-H4-depleted extract into the channel as described above and, upon loop formation, stretched DNA strands by flowing imaging buffer from the opposite side.

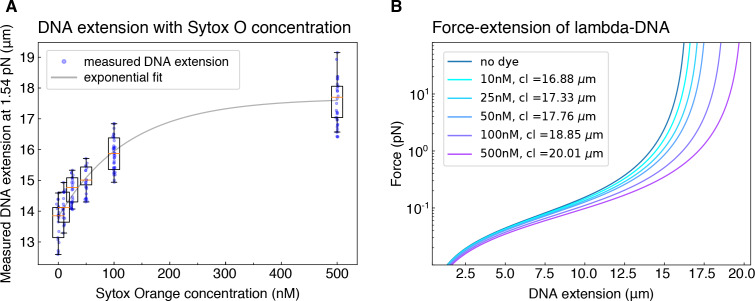
Correction of dye-induced DNA lengthening

As mentioned above, we used the DNA intercalating dye Sytox Orange at a range of concentrations to visualize our immobilized lambda DNA molecules. The intercalation of small dye molecules in between adjacent base pairs leads to a dye-concentration-dependent lengthening of the DNA molecules’ contour length (Ganji et al., 2016). As this effect influences downstream analysis, we sought to correct for the dye-induced lengthening of our DNA molecules by determining the effective contour length of the lambda DNA for each dye concentration used in this study. To this end, we hydrodynamically stretched immobilized DNA molecules in the absence of dye using a buffer flow perpendicular to the strand orientation. DNA molecules were visualized by previous covalently labelling of the DNA backbone with Cy5 fluorescent molecules (label IT nucleic acid labelling kit, Mirus) which does not compromise the DNA contour length. By measuring the extension of these DNA molecules at a certain flow rate, we calculated the corresponding force experienced by the DNA molecules using the worm-like chain model (Marko and Siggia, 1995). We then performed the same stretching experiment with DNA molecules exposed to various concentrations of Sytox Orange, keeping the flow rate (and thus the stretching force) constant (1.54 pN) (Figure 2—figure supplement 6A). The application of the worm-like chain model to the mean measured DNA extension values for a known force allowed us finally to obtain the contour length of lambda DNA at all examined dye concentrations (Figure 2—figure supplement 6B). All these calibrations were done in the same buffer that is present in the channels prior to introduction of the egg extract—which matches the pH and salt concentration of the extract. However, we want to point out, that in the extract, DNA molecules are exposed to a multitude of DNA binding proteins, which may further influence the properties of the DNA. However, for technical reasons, our calibrations of the effect of the dye on DNA length were limited to the buffer condition.

Loop extrusion analysis

DNA traces were analyzed using custom-written Python scripts motivated by Ganji et al., 2018, resulting in data files for further analysis that we added together with the source code in the supplement. We converted movies of fluorescent DNA molecules into one-dimensional intensity profiles by summing the intensity values along the direction perpendicular to the DNA strand in each frame. We removed the background signal using a median filter. From the summed intensity profile for each frame we built kymographs by concatenating all time points (Figure 2 and Figure 1—figure supplement 1). To yield the amount of DNA inside and outside the loop for each time point, we segmented the DNA intensity profiles into a loop region and two regions outside of the loop by first finding the maximum intensity value as the position of the loop and subsequent fitting of a Gaussian around that position. We defined the boundaries of the loop region and the regions outside of the loop by the positions + / - 2 standard deviations from the center of the Gaussian fit. Summing the intensity values of the regions outside of the loop and integrating the intensity under the Gaussian fit yielded the proportions of total signal intensity in each of the three regions for each time point. The difference between the integrated intensity below the loop and the offset from the Gaussian fit (corresponding to the intensity outside of the loop) was equally distributed to the regions outside of the loop as the signal from the incoming and outgoing DNA strands that are not part of the loop itself (Figure 2A).

We calculated the relative sizes of the three regions in kilo-base pairs (kb) for each time frame by multiplying the 48.5 kb total length of lambda DNA with the ratio of each summed intensity value and the total summed intensity of the strand for every time point. From these values we calculated the relative change of DNA in each region over time by subtracting the averaged ten last data points from the averaged ten first data points in each region. We used the resulting values a and b for the region left and right of the loop to assign a symmetry score for each looping event by calculating

s y m m e t r y s c o r e = \frac{M a x (a, b) - M i n (a, b)}{a + b}

This procedure orders the extrusion from region a and b such that the symmetry score is always positive and ranges from 0 to 1. Our symmetry score intends to quantify the amount of DNA extruded into the loop from the outer regions. A positive relative change from one side implies that no DNA from that side has been extruded into the loop—and indicates that DNA slipped from the loop to that region— and thus we set that change to 0 (if a > 0: a = 0; if b > 0: b = 0). The slipping of substantial amounts of DNA (>2 kb) was a rare event with three cases in metaphase (p=0.08) and 0 cases in interphase.

This procedure additionally allowed us to track the position of the loop for each time point during every loop extrusion event and study the movement of loops along the DNA strands in inter- and metaphase (Figure 2—figure supplement 4). To this end we quantified the change in relative position of the loop by subtracting the average loop positions of the last ten time point from the average loop positions of the first ten time points. (Figure 2—figure supplement 4C) This analysis was set up in such a way that, independent of a loop starting left or right of the center of the DNA, the change in loop position was always positive if the loop moved towards or crossed the center of the DNA molecule, and negative if the loop moved towards the closest DNA boundary. The absolute quantity of the change in loop position reflects the relative displacement of the loop along the DNA strand during the process of loop formation and it is referred to as static, if the displacement is below a threshold of 0.08. This analysis allowed us to display the relative displacements of loops as a function of the symmetry score of the corresponding looping in between meta- and interphase (Figure 2—figure supplement 4D&E) and compare the probability of the loop to move towards the center of the strand between both cell cycle phases (Figure 2—figure supplement 4F).

We extracted the initial loop extrusion rates from the first derivative at time point zero of a single exponential fit to the values of the loop growth over time (Figure 2B–C). The size of the loop at each time point further allowed us to continuously calculate the relative extension of the DNA molecule during the loop formation, by dividing the end-to-end distance of the DNA strand by the length of the regions outside of the loop. We estimated the tension on the DNA strand for each time point by applying the Worm Like Chain Model (WLC) of DNA (Marko and Siggia, 1995) to these relative extension values (Figure 2—figure supplement 2C & D). Since small fluctuations in the estimated relative extension of the DNA, as they occur via thermal agitation of the molecule, can lead to large fluctuations in the corresponding tension, we decided to reduce fluctuations by smoothing the initial loop data. To this end we applied a Savitzky-Golay filter with a 2^nd order polynomial and a window size of 63 points to the initial loop data, which significantly reduced fluctuations in the resulting relative DNA extension. The rate of loop extrusion was then extracted from a first order derivative of the smoothed curve and yielded similar initial rates as determined from the exponential fit to the raw loop data. We then applied the WLC model to the smoothed relative extension curve to obtain tension of the DNA molecule for each time point during the loop formation. This procedure allowed us to visualize the decrease in extrusion rate with the increasing tension on the DNA molecule (Figure 2—figure supplement 2E). To investigate the dependence of the rate of loop extrusion on the tension on the DNA strand for the entire population of inter- and metaphase looping events, we extracted the extrusion rates and corresponding tension values for each time point during every looping event from the exponential fits to the loop data. This allowed us to display the average decrease in extrusion rate (+ / - standard deviation) for the interphase and metaphase looping populations (Figure 2—figure supplement 2F). The stall force of each loop extrusion event (Figure 2D) was determined by taking the average steady state loop size of the last ten time points and converting the corresponding relative extension of the DNA molecule into one tension value per looping event using the WLC model. For the analysis of extrusion stall forces we only used DNA strands where the loop extrusion did not end (or was stalled) at the DNA end-binding sites (N = 52).

To quantify the effect of cohesin and condensin depletion, we determined the probability of loop extrusion by counting the number of observable loop extrusion events in all data taken for one condition and dividing it by the total number of DNA strands with sufficient slack (<0.6 relative extension) to support the formation of a loop for that condition.

Acknowledgements

We acknowledge L Mirny for initial discussions of this work, and AA Hyman, P Tomancak, M Loose, K Ishihara, I Patten, M Sarov, J Guck, F Stewart, F Jülicher, M Srinivasan, and K Nasmyth for discussions and revision of the manuscript. We thank M Elsner and V Murugesan for assistance in some of the experiments. We thank H Andreas for frog maintenance, the Light Microscopy Facility (LMF), and the Antibody Facility at MPI-CBG.

Funding Statement

The funders had no role in study design, data collection and interpretation, or the decision to submit the work for publication.

Contributor Information

Jan Brugués, Email: brugues@mpi-cbg.de.

Job Dekker, University of Massachusetts Medical School, United States.

Kevin Struhl, Harvard Medical School, United States.

Funding Information

This paper was supported by the following grants:

Human Frontier Science Program CDA00074/2014 to Jan Brugués.
European Molecular Biology Organization ALTF 1456-2015 to Thomas Quail.
Max-Planck-Gesellschaft ELBE fellowship to Stefan Golfier.
Japan Society for the Promotion of Science JP18H05527 to Hiroshi Kimura.
Japan Science and Technology Corporation JPMJCR16G1 to Hiroshi Kimura.

Additional information

Competing interests

No competing interests declared.

Author contributions

Conceptualization, Data curation, Software, Formal analysis, Investigation, Methodology, Writing - review and editing.

Investigation, Methodology, Writing - review and editing.

Resources, Methodology.

Conceptualization, Formal analysis, Supervision, Funding acquisition, Writing - original draft, Project administration, Writing - review and editing.

Ethics

Animal experimentation: Animal experimentation: All animals were handled according to the directive 2010/63/EU on the protection of animals used for scientific purposes, and the german animal welfare law under the license document number DD24-5131/367/9 from the Landesdirektion Sachsen (Dresden) - Section 24D.

Additional files

Source code 1. DNA-strand analysis.

elife-53885-code1.ipynb^{(1.8MB, ipynb)}

Source data 1. Interphase loops raw data.

elife-53885-data1.zip^{(77.1KB, zip)}

Source data 2. Metaphase loops raw data.

elife-53885-data2.zip^{(78.1KB, zip)}

Transparent reporting form

elife-53885-transrepform.docx^{(246.5KB, docx)}

Data availability

All data generated or analysed during this study are included in the manuscript.

References

Abdennur N. Condensin II inactivation in interphase does not affect chromatin folding or gene expression. bioRxiv. 2018 doi: 10.1101/437459. [DOI]
Abramo K, Valton A, Venev SV, Ozadam H. Chromosome folding intermediate at the condensin-to-cohesin transition during telophase. bioRxiv. 2019 doi: 10.1038/s41556-019-0406-2. [DOI] [PMC free article] [PubMed]
Alipour E, Marko JF. Self-organization of domain structures by DNA-loop-extruding enzymes. Nucleic Acids Research. 2012;40:11202–11212. doi: 10.1093/nar/gks925. [DOI] [PMC free article] [PubMed] [Google Scholar]
Banigan EJ, Berg A, Brandão HB, Marko JF. Chromosome organization by one-sided and two-sided loop extrusion. bioRxiv. 2019 doi: 10.7554/eLife.53558. [DOI] [PMC free article] [PubMed]
Banigan EJ, Mirny LA. Limits of chromosome compaction by loop-extruding motors. bioRxiv. 2018 doi: 10.1103/physrevx.9.031007. [DOI]
Bonev B, Cavalli G. Organization and function of the 3D genome. Nature Reviews Genetics. 2016;17:661–678. doi: 10.1038/nrg.2016.112. [DOI] [PubMed] [Google Scholar]
Bouwman BA, de Laat W. Getting the genome in shape: the formation of loops, domains and compartments. Genome Biology. 2015;16:1–9. doi: 10.1186/s13059-015-0730-1. [DOI] [PMC free article] [PubMed] [Google Scholar]
Davidson IF, Bauer B, Goetz D, Tang W, Wutz G, Peters JM. DNA loop extrusion by human cohesin. Science. 2019;366:1338–1345. doi: 10.1126/science.aaz3418. [DOI] [PubMed] [Google Scholar]
Dekker J, Mirny L. The 3D genome as moderator of chromosomal communication. Cell. 2016;164:1110–1121. doi: 10.1016/j.cell.2016.02.007. [DOI] [PMC free article] [PubMed] [Google Scholar]
Earnshaw WC, Laemmli UK. Architecture of metaphase chromosomes and chromosome scaffolds. The Journal of Cell Biology. 1983;96:84–93. doi: 10.1083/jcb.96.1.84. [DOI] [PMC free article] [PubMed] [Google Scholar]
Fudenberg G, Imakaev M, Lu C, Goloborodko A, Abdennur N, Mirny LA. Formation of chromosomal domains by loop extrusion. Cell Reports. 2016;15:2038–2049. doi: 10.1016/j.celrep.2016.04.085. [DOI] [PMC free article] [PubMed] [Google Scholar]
Ganji M, Kim SH, van der Torre J, Abbondanzieri E, Dekker C. Intercalation-Based Single-Molecule fluorescence assay to study DNA supercoil dynamics. Nano Letters. 2016;16:4699–4707. doi: 10.1021/acs.nanolett.6b02213. [DOI] [PubMed] [Google Scholar]
Ganji M, Shaltiel IA, Bisht S, Kim E, Kalichava A, Haering CH, Dekker C. Real-time imaging of DNA loop extrusion by condensin. Science. 2018;360:102–105. doi: 10.1126/science.aar7831. [DOI] [PMC free article] [PubMed] [Google Scholar]
Goloborodko A, Imakaev MV, Marko JF, Mirny L. Compaction and segregation of sister chromatids via active loop extrusion. eLife. 2016;5:e14864. doi: 10.7554/eLife.14864. [DOI] [PMC free article] [PubMed] [Google Scholar]
Groen AC, Ngyuen PA, Field CM, Ishihara K, Mitchison TJ. Glycogen-supplemented mitotic cytosol for analyzing Xenopus egg microtubule organization. Methods in Enzymology. 2014;540:417–433. doi: 10.1016/B978-0-12-397924-7.00023-6. [DOI] [PubMed] [Google Scholar]
Gruszka DT, Xie S, Kimura H, Yardimci H. Single-molecule imaging reveals control of parental histone recycling by free histones during DNA replication. bioRxiv. 2019 doi: 10.1101/789578. [DOI] [PMC free article] [PubMed]
Hansen AS, Pustova I, Cattoglio C, Tjian R, Darzacq X. CTCF and cohesin regulate chromatin loop stability with distinct dynamics. eLife. 2017;6:e25776. doi: 10.7554/eLife.25776. [DOI] [PMC free article] [PubMed] [Google Scholar]
Hauf S, Roitinger E, Koch B, Dittrich CM, Mechtler K, Peters JM. Dissociation of cohesin from chromosome arms and loss of arm cohesion during early mitosis depends on phosphorylation of SA2. PLOS Biology. 2005;3:e69. doi: 10.1371/journal.pbio.0030069. [DOI] [PMC free article] [PubMed] [Google Scholar]
Hirano T, Kobayashi R, Hirano M. Condensins, chromosome condensation protein complexes containing XCAP-C, XCAP-E and a Xenopus homolog of the Drosophila barren protein. Cell. 1997;89:511–521. doi: 10.1016/S0092-8674(00)80233-0. [DOI] [PubMed] [Google Scholar]
Hirano T, Mitchison TJ. Cell cycle control of higher-order chromatin assembly around naked DNA in vitro. The Journal of Cell Biology. 1991;115:1479–1489. doi: 10.1083/jcb.115.6.1479. [DOI] [PMC free article] [PubMed] [Google Scholar]
Hirano T, Mitchison TJ. A heterodimeric coiled-coil protein required for mitotic chromosome condensation in vitro. Cell. 1994;79:449–458. doi: 10.1016/0092-8674(94)90254-2. [DOI] [PubMed] [Google Scholar]
Kim Y, Shi Z, Zhang H, Finkelstein IJ, Yu H. Human cohesin compacts DNA by loop extrusion. Science. 2019;366:1345–1349. doi: 10.1126/science.aaz4475. [DOI] [PMC free article] [PubMed] [Google Scholar]
Kinoshita K, Hirano T. Dynamic organization of mitotic chromosomes. Current Opinion in Cell Biology. 2017;46:46–53. doi: 10.1016/j.ceb.2017.01.006. [DOI] [PubMed] [Google Scholar]
Kong M, Cutts E, Pan D, Beuron F, Kaliyappan T, Xue C, Morris E, Musacchio A, Vannini A. Human condensin I and II drive extensive ATP – dependent compaction of nucleosome – bound DNA. bioRxiv. 2019 doi: 10.1101/683540. [DOI] [PMC free article] [PubMed]
Losada A, Hirano M, Hirano T. Identification of Xenopus SMC protein complexes required for sister chromatid cohesion. Genes & Development. 1998;12:1986–1997. doi: 10.1101/gad.12.13.1986. [DOI] [PMC free article] [PubMed] [Google Scholar]
Losada A, Hirano T. Shaping the metaphase chromosome: coordination of cohesion and condensation. BioEssays. 2001;23:924–935. doi: 10.1002/bies.1133. [DOI] [PubMed] [Google Scholar]
Lupiáñez DG, Kraft K, Heinrich V, Krawitz P, Brancati F, Klopocki E, Horn D, Kayserili H, Opitz JM, Laxova R, Santos-Simarro F, Gilbert-Dussardier B, Wittler L, Borschiwer M, Haas SA, Osterwalder M, Franke M, Timmermann B, Hecht J, Spielmann M, Visel A, Mundlos S. Disruptions of topological chromatin domains cause pathogenic rewiring of gene-enhancer interactions. Cell. 2015;161:1012–1025. doi: 10.1016/j.cell.2015.04.004. [DOI] [PMC free article] [PubMed] [Google Scholar]
Marko JF, Siggia ED. Stretching DNA. Macromolecules. 1995;28:8759–8770. doi: 10.1021/ma00130a008. [DOI] [Google Scholar]
Marsden MP, Laemmli UK. Metaphase chromosome structure: evidence for a radial loop model. Cell. 1979;17:849–858. doi: 10.1016/0092-8674(79)90325-8. [DOI] [PubMed] [Google Scholar]
Murray AW. Methods in Cell Biology. Vol. 36. Elsevier; 1991. Cell cycle extracts; pp. 581–605. [DOI] [Google Scholar]
Nagano T, Lubling Y, Várnai C, Dudley C, Leung W, Baran Y, Mendelson Cohen N, Wingett S, Fraser P, Tanay A. Cell-cycle dynamics of chromosomal organization at single-cell resolution. Nature. 2017;547:61–67. doi: 10.1038/nature23001. [DOI] [PMC free article] [PubMed] [Google Scholar]
Nasmyth K. Disseminating the genome: joining, resolving, and separating sister chromatids during mitosis and meiosis. Annual Review of Genetics. 2001;35:673–745. doi: 10.1146/annurev.genet.35.102401.091334. [DOI] [PubMed] [Google Scholar]
Naumova N, Imakaev M, Fudenberg G, Zhan Y, Lajoie BR, Mirny LA, Dekker J. Organization of the mitotic chromosome. Science. 2013;342:948–953. doi: 10.1126/science.1236083. [DOI] [PMC free article] [PubMed] [Google Scholar]
Nuebler J, Fudenberg G, Imakaev M, Abdennur N, Mirny LA. Chromatin organization by an interplay of loop extrusion and compartmental segregation. PNAS. 2018;115:E6697–E6706. doi: 10.1073/pnas.1717730115. [DOI] [PMC free article] [PubMed] [Google Scholar]
Ono T, Losada A, Hirano M, Myers MP, Neuwald AF, Hirano T. Differential contributions of condensin I and condensin II to mitotic chromosome architecture in vertebrate cells. Cell. 2003;115:109–121. doi: 10.1016/S0092-8674(03)00724-4. [DOI] [PubMed] [Google Scholar]
Rao SS, Huntley MH, Durand NC, Stamenova EK, Bochkov ID, Robinson JT, Sanborn AL, Machol I, Omer AD, Lander ES, Aiden EL. A 3D map of the human genome at Kilobase resolution reveals principles of chromatin looping. Cell. 2014;159:1665–1680. doi: 10.1016/j.cell.2014.11.021. [DOI] [PMC free article] [PubMed] [Google Scholar]
Rao SSP, Huang SC, Glenn St Hilaire B, Engreitz JM, Perez EM, Kieffer-Kwon KR, Sanborn AL, Johnstone SE, Bascom GD, Bochkov ID, Huang X, Shamim MS, Shin J, Turner D, Ye Z, Omer AD, Robinson JT, Schlick T, Bernstein BE, Casellas R, Lander ES, Aiden EL. Cohesin loss eliminates all loop domains. Cell. 2017;171:305–320. doi: 10.1016/j.cell.2017.09.026. [DOI] [PMC free article] [PubMed] [Google Scholar]
Ren G, Jin W, Cui K, Rodrigez J, Hu G, Zhang Z, Larson DR, Zhao K. CTCF-Mediated Enhancer-Promoter interaction is a critical regulator of Cell-to-Cell variation of gene expression. Molecular Cell. 2017;67:1049–1058. doi: 10.1016/j.molcel.2017.08.026. [DOI] [PMC free article] [PubMed] [Google Scholar]
Rowley MJ, Corces VG. Organizational principles of 3D genome architecture. Nature Reviews Genetics. 2018;19:789–800. doi: 10.1038/s41576-018-0060-8. [DOI] [PMC free article] [PubMed] [Google Scholar]
Sanborn AL, Rao SS, Huang SC, Durand NC, Huntley MH, Jewett AI, Bochkov ID, Chinnappan D, Cutkosky A, Li J, Geeting KP, Gnirke A, Melnikov A, McKenna D, Stamenova EK, Lander ES, Aiden EL. Chromatin extrusion explains key features of loop and domain formation in wild-type and engineered genomes. PNAS. 2015;112:E6456–E6465. doi: 10.1073/pnas.1518552112. [DOI] [PMC free article] [PubMed] [Google Scholar]
Schoenfelder S, Fraser P. Long-range enhancer-promoter contacts in gene expression control. Nature Reviews Genetics. 2019;20:437–455. doi: 10.1038/s41576-019-0128-0. [DOI] [PubMed] [Google Scholar]
Schwarzer W, Abdennur N, Goloborodko A, Pekowska A, Fudenberg G, Loe-Mie Y, Fonseca NA, Huber W, Haering CH, Mirny L, Spitz F. Two independent modes of chromatin organization revealed by cohesin removal. Nature. 2017;551:51–56. doi: 10.1038/nature24281. [DOI] [PMC free article] [PubMed] [Google Scholar]
Shintomi K, Inoue F, Watanabe H, Ohsumi K, Ohsugi M, Hirano T. Mitotic chromosome assembly despite nucleosome depletion in Xenopus egg extracts. Science. 2017;356:1284–1287. doi: 10.1126/science.aam9702. [DOI] [PubMed] [Google Scholar]
Smith SB, Cui Y, Bustamante C. Overstretching B-DNA: the elastic response of individual double-stranded and single-stranded DNA molecules. Science. 1996;271:795–799. doi: 10.1126/science.271.5250.795. [DOI] [PubMed] [Google Scholar]
Smith EM, Lajoie BR, Jain G, Dekker J. Invariant TAD boundaries constrain Cell-Type-Specific looping interactions between promoters and distal elements around the CFTR locus. The American Journal of Human Genetics. 2016;98:185–201. doi: 10.1016/j.ajhg.2015.12.002. [DOI] [PMC free article] [PubMed] [Google Scholar]
Strick TR, Kawaguchi T, Hirano T. Real-Time detection of Single-Molecule DNA compaction by condensin I. Current Biology. 2004;14:874–880. doi: 10.1016/j.cub.2004.04.038. [DOI] [PubMed] [Google Scholar]
Sumara I, Vorlaufer E, Gieffers C, Peters BH, Peters JM. Characterization of vertebrate cohesin complexes and their regulation in prophase. The Journal of Cell Biology. 2000;151:749–762. doi: 10.1083/jcb.151.4.749. [DOI] [PMC free article] [PubMed] [Google Scholar]
Tang Z, Luo OJ, Li X, Zheng M, Zhu JJ, Szalaj P, Trzaskoma P, Magalska A, Wlodarczyk J, Ruszczycki B, Michalski P, Piecuch E, Wang P, Wang D, Tian SZ, Penrad-Mobayed M, Sachs LM, Ruan X, Wei CL, Liu ET, Wilczynski GM, Plewczynski D, Li G, Ruan Y. CTCF-Mediated human 3D genome architecture reveals chromatin topology for transcription. Cell. 2015;163:1611–1627. doi: 10.1016/j.cell.2015.11.024. [DOI] [PMC free article] [PubMed] [Google Scholar]
Uhlmann F. SMC complexes: from DNA to chromosomes. Nature Reviews Molecular Cell Biology. 2016;17:399–412. doi: 10.1038/nrm.2016.30. [DOI] [PubMed] [Google Scholar]
Xiao B, Freedman BS, Miller KE, Heald R, Marko JF. Histone H1 compacts DNA under force and during chromatin assembly. Molecular Biology of the Cell. 2012;23:4864–4871. doi: 10.1091/mbc.e12-07-0518. [DOI] [PMC free article] [PubMed] [Google Scholar]
Yan J, Maresca TJ, Skoko D, Adams CD, Xiao B, Christensen MO, Heald R, Marko JF. Micromanipulation studies of chromatin fibers in Xenopus egg extracts reveal ATP-dependent chromatin assembly dynamics. Molecular Biology of the Cell. 2007;18:464–474. doi: 10.1091/mbc.e06-09-0800. [DOI] [PMC free article] [PubMed] [Google Scholar]
Yatskevich S, Rhodes J, Nasmyth K. Organization of chromosomal DNA by SMC complexes. Annual Review of Genetics. 2019;53:445–482. doi: 10.1146/annurev-genet-112618-043633. [DOI] [PubMed] [Google Scholar]
Zierhut C, Jenness C, Kimura H, Funabiki H. Nucleosomal regulation of chromatin composition and nuclear assembly revealed by histone depletion. Nature Structural & Molecular Biology. 2014;21:617–625. doi: 10.1038/nsmb.2845. [DOI] [PMC free article] [PubMed] [Google Scholar]

eLife. doi: 10.7554/eLife.53885.sa1

Decision letter

Editor: Job Dekker¹

Reviewed by: Leonid A Mirny², Andrea Musacchio³, John F Marko⁴

In the interests of transparency, eLife publishes the most substantive revision requests and the accompanying author responses.

Acceptance summary:

This work is an important and timely contribution that addresses modalities of loop extrusion by SMC complexes. The present study goes beyond recent published work in this area, by having reconstituted both cohesin and condensin reactions in extracts and having demonstrated the cell cycle dependence of the activity of cohesin and condensin. Presented single-molecule experiments in mitotic and interphase extracts show different modes of loop extrusion, with condensins operating in the mitotic extract as one-sided extruders, and cohesin acting in interphase as a two-sided extruder. Although the process of loop extrusion by yeast condensin has been visualized by single-molecule experiments (Ganji et al., 2018), many questions remain, particularly the mode of loop extrusion by different SMCs complexes of higher eukaryotes. The study of Golfier et al. is the first to examine extrusion by both condensin and cohesin from the same higher organism, in cell extracts, and in a cell-cycle-stage dependent manner. The study also provides important quantitative estimates of the rates and stall forces of extrusion that can be important for future studies aiming to decipher the mechanism of extrusion.

Decision letter after peer review:

Thank you for submitting your article "Cohesin and condensin extrude loops in a cell-cycle dependent manner" for consideration by eLife. Your article has been reviewed by three peer reviewers, and the evaluation has been overseen by a Reviewing Editor and Kevin Struhl as the Senior Editor. The following individuals involved in review of your submission have agreed to reveal their identity: Leonid A Mirny (Reviewer #1); Andrea Musacchio (Reviewer #2); John F Marko (Reviewer #3).

The reviewers have discussed the reviews with one another and the Reviewing Editor has drafted this decision to help you prepare a revised submission.

Essential revisions:

1) Some caveats need to be included regarding the results. It is emphasized that the experiments put the looping experiments in a cellular context. Yes, extracts are used, but the first result of the paper is that H3-H4 dimers must be removed which makes these experiments rather more like in vitro experiments on naked DNA (e.g., by the Dekker and Peters labs) than experiments on chromatin. Further, it is emphasized that the experiments indicate that cohesin carries out symmetric extrusion which condensin is asymmetric, while Figure 2 indicates a mixed distribution, with peaks towards the symmetric and asymmetric cases for interphase/cohesin and metaphase/condensin. According to Figure 2 there is an appreciable subfraction of asymmetric cohesin traces and symmetric condensin traces in the data. The work of Banigan and Mirny puts a 90% compaction limit on pure-one-sided extrusion (with random orientation of extruders) but mixing even a small fraction of two-sided extruders will lead to appreciable further compaction by filling in gaps between the quiet sides of adjacent one-sided extruders.

2) No differentiation is made between condensin I and condensin II; the initial structure of metaphase chromosomes during mitotic prophase is established by condensin II, but Xenopus extracts contain condensin I in excess (estimates of Hirano's group are in the 5:1 range for condensin II:condensin I). Have experiments been done along the lines of those in Figure 2 to determine the nature of loop extrusion (symmetric vs. asymmetric) for depletions of condensin I and condensin II-specific components (e.g., XCAP-H and XCAP-H2)? It is therefore not clear whether the two species of condensin have the same loop-extrusion-symmetry properties and it could well be that the more symmetric cases in Figure 2Diii correspond to more symmetric activities of condensin II. For the extracts with excess condensin I it would seem wise to not draw conclusions about condensin II. I do not require new experiments with specific depletions of either condensin I or II (although if they can be added that would be interesting), but do ask for a more careful discussion of the possible roles of the two complexes.

3) There seems to be some inconsistency between the stall forces reported in the text (0.41 pN for condensin, and 0.23pN for cohesin) and those shown in Figure 2D (the mean ~0.1pN for both). I also wonder whether errors in determining the relative extension of DNA could have contributed to the estimate of the stall force. Perhaps authors can test whether estimated stall force for each trajectory depends on the initial and final slack.

4) Looking at Figure 2Biv and v I was a bit surprised that the sum of the length of three regions: DNA to the left from the loop, to the right from the loop, and in the loop don't appear to sum up to a constant. On Figure 2Biv the sum of the three at t=0 is 37Kb, at 30sec its about 43Kb… May be this is just a measurement error… The loop gained 25Kb, but the flanks lost less than 20Kb together. In fitting these curves, I wonder whether the assumption about the sum of the three to be constant was used (and whether using it would make a difference for the rates and the stall forces). Also, in Figure 2Bv, it looks like the loop is getting smaller for trajectories with larger relative extension, (initial, I assume) but the flanks are not getting smaller or larger. This plot is generally hard to understand. Authors may want to have a diagram to help the reader with Figure 2Bii, v and also explain what's shown: one point per trajectory or one point per time from a single (or multiple trajectories).

5) Figure 2C is very important as it provides the estimate of the speed of extrusion. It looks like this plot shows one point (rate at t=0) per trajectory. It may be interesting to see (i) whether rate vs. extension is a universal dependence; and (ii) the extent of variation around this dependence for individual trajectories. Perhaps authors can put not only t=0, but some smoothed velocity vs. extension for different time points on the same plot and/or their exp fits. In other words, if one trajectory starts with less extension and at a higher speed, while another starts at a greater extension and lower speed, will the speed of the first one gets down to the same level when it achieves the same extension as the initial one of the second trajectory. Using these data for all trajectories together (data for different t, not only t=0) may provide a more robust estimate of the rate of extrusion that the two exp fits (one to get rate(t=0) for each trajectory, and the other for rate(t=0) vs. extension).

I wonder whether more accuracy way of measuring the speed of extrusion (or reeling) could be achieved by using very low level of DNA labeling so that individual speckles of DNA can be vitalized and tracked in the flanking regions. This can, in principle, be precise enough to see pausing or switching events. May be this can be tried in future studies.

6) The difference in extrusion dynamics between interphase and mitotic extracts is one of the most exciting aspects of the manuscript. Since cohesin and condensins are labeled it would be great to look closer at the deviations from the textbook picture. So, I wonder whether authors can look at cohesin dynamics in the mitotic extract, and condensin dynamics in the interphase. Are cohesins completely unable to bind or to extrude in the mitotic exact? Can condensins bind/extrude in the interphase, though less efficiently? In other words, are these mere abundances of cohesin in the interphase and condensin in the metaphase, or is there a mechanistic control. Testing for such unconventional roles can be a very exciting twist of the story.

7) The major limit of the study is that there are no mechanistic details to explain the cell-cycle dependency of the reactions. Importantly, DNA loop extrusion by Cohesin had not been demonstrated until very recently, and it is clear that the authors reconstituted this reaction independently from the other two groups. I feel that at least some initial mechanistic detail on how the cell cycle regulates loop extrusion should be discussed.

[Editors' note: further revisions were suggested prior to acceptance, as described below.]

Thank you for re-submitting your article "Cohesin and condensin extrude loops in a cell-cycle dependent manner" for consideration by eLife. Your article has been reviewed by three peer reviewers, and the evaluation has been overseen by a Reviewing Editor and Kevin Struhl as the Senior Editor. The following individuals involved in review of your submission have agreed to reveal their identity: Leonid A Mirny (Reviewer #1); Andrea Musacchio (Reviewer #2); John F Marko (Reviewer #3).

The reviewers have discussed the reviews with one another and the Reviewing Editor has drafted this decision to help you prepare a revised submission.

We would like to draw your attention to changes in our revision policy that we have made in response to COVID-19 (https://elifesciences.org/articles/57162). Specifically, when editors judge that a submitted work as a whole belongs in eLife but that some conclusions require a modest amount of additional new data, as they do with your paper, we are asking that the manuscript be revised to either limit claims to those supported by data in hand, or to explicitly state that the relevant conclusions require additional supporting data.

Our expectation is that the authors will eventually carry out the additional experiments and report on how they affect the relevant conclusions either in a preprint on bioRxiv or medRxiv, or if appropriate, as a Research Advance in eLife, either of which would be linked to the original paper.

Essential revisions:

1) Reviewer #1 brought up these points:

I found the wording "symmetric" and "non-symmetric loops" a bit confusing, as loops are symmetric, and it is the process that generates them can be either symmetric or non-symmetric. The authors may want to replace "extrudes symmetric loops" with "extrudes loops symmetrically" (and similarly "extrudes loops non-symmetrically") in the Abstract and in the rest of the manuscript.

2) While presenting an equation for the Relative Extension (RE) is really helpful, some inconsistencies between RE presented on different plots remain an issue. Figure 2—figure supplement 5A and B largely disagree with all other plots presenting RE. According to Figure 2—figure supplement 5A, extrusion during interphase results in RE<0.5 for most of the cases, while extrusion during metaphase (Figure 2—figure supplement 5B) appears to be much more processive, resulting in RE>0.5 for most of the cases and at least 8 trajectories showing complete extrusion of all the slack in DNA into a loop (RE=1). Figure 2—figure supplement 5C, on the contrary, has all the points with RE<0.6; including 4 metaphase trajectories with RE<0.2, while only one such point is shown on Figure 2—figure supplement 5B. The number of trajectories (~20) on Figures 2—figure supplement 5A and B also disagree with the numbers of points shown in Figure 2—figure supplement 5C, and all inconsistent with the tallies of trajectories on Figure 2—figure supplement 4D. While Figure 2—figure supplement 5C agrees the main Figure 2, where most of RE <0.6 for both interphase and metaphase, they disagree with the text which states the typical maximum (stalling) RE is ~65% in the metaphase extract (consistent with Figure 2—figure supplement 5B, but not all other figures). Overall, if indeed RE is vastly different in mitotic and interphase trajectories – it's a very interesting result.

3) Since RE was used to infer the stall force, inconsistencies in RE may have propagated to those in the stall force estimates. In general, 0.16-0.18 pN forces reported here (with a smaller stall force for metaphase than for interphase, inconsistent with Figure 2—figure supplement 5C) is a factor of 10 lower than those found by for yeast condensin 1.2+-0.5pN and >0.8pN for human cohesin). It would be great if authors can comment on this. It appears that the quantification of force-extension (Figure 2—figure supplement 6) can make detecting of ~1pN forces extremely unlikely. According to Figure 2—figure supplement 6B, getting a force of about 1pN would require >96% extension. Works of Cees Dekker's lab use magnetic bead quantification (their Nano Letter 2016) where ~1pN are achieved at 80% extension. According to Figure 2—figure supplement 6B, 80% extension gives the force <0.1pN. This factor of 10 difference between force-extension quantification deserves authors attention. Furthermore, Figure 2—figure supplement 2F shows extrusion, albeit at a lower speed, above 0.1pN and up to 1pN force, raising further questions about the estimate of 0.16pN stall force.

4) At the end of the Discussion, the authors bring up a very interesting result (not mentioned in the Results section): "Interestingly, our results comparing interphase extrusion velocities with looping symmetries show that, on average, symmetric loops extrude with a higher rate (roughly twice) compared to the non-symmetric events (Figure 2—figure supplement 5)." Unfortunately, Figure 2—figure supplement 5 doesn't present symmetry vs. rate data. This is very interesting and important result that should be presented in the Results section, and further highlighted in the Discussion. Alongside with the missing symmetry vs. velocity, the authors may want to present symmetry as a function of the initial relative extension, as those slower and one-sided events might reflect partial stalling of the motor activity, and thus may be more abundant at higher extension.

5) The revision presents insightful new analysis of the movement of the loop relative to the border. While interesting, it may be hard for a reader to follow its logic without an illustration (and possibly also a mathematical formula in the Materials and methods). Such a figure can just explain why (i) two-sided extrusion initiated close to the border would make the loop move towards to the nearest border, while those initiated away from the border wouldn't; and (ii) why one-sided extrusion would move a loop to the border or toward the center with 50/50 chances.

6) Sentences comparing one-sided extrusion in metaphase with our recent theory paper are somewhat confusing. The manuscript reads "This (theory) work puts the lower limit on symmetric extrusion at 10%. In contrast to in vitro studies of condensin loop extrusion, we found a small sub-population of symmetric loop extrusion in metaphase (~20%) that is above the 10% theoretical limit." Our theory work states that purely one-sided extrusion would leave ~10% of DNA unextruded, setting a limit of ~10-fold compaction due to one-sided extrusion. Hence "10%" in our study does not refer to the fraction of one-sided or two-sided extruders that is required for compaction. However, we did consider mixtures of one-sided and two-sided extruders, demonstrating that a mixture of 40% one-sided and 60% two-sided extruders can achieve 100-fold linear compaction, while achieving 1000-fold compaction would require ~85% two-sided extruders in the mixture. Data presented in the revised manuscript indeed show that in the mitotic extract about 50% of condensins are one-sided (symmetry 0.8-1.0), while the remaining 50% are asymmetric, but two-sided events. As discussed in our PRX paper, even highly asymmetric extrusion can be consistent with "two-sided" extrusion if such asymmetric extruders stay on the chromatin long enough to close the gaps on their "slowly" extruding sides. Thus, the authors can state that this 50% asymmetric extrusion could be sufficient to achieve about 100-fold compaction. Further studies of extruders with different distributions of symmetries and speeds or residence times are underway in our group.

7) Reviewer #3 brings up these points:

The revision has addressed the comments and suggestions of the first round of review. I still think the paper is not as crystal clear as it should be about a key point, namely the use of H3/H4-depleted extracts (without which the effects reported in the paper could not be observed). It should be made very clear that these experiments are not on chromatinized DNA, as most people in the field know is rapidly formed when naked DNA is put into Xenopus extracts.

I think that the phrase "histone H3/H4-depleted" should be put in front of "Xenopus egg extracts" either in the Abstract or in the last paragraph of the Results.

Numbers of molecules are now included in the Results which is helpful. However, numbers of separate experiments are not listed (N=52 means 52 molecules in one flow cell or 13 molecules observed in 4 separate experiments (flow cells)). Perhaps this information is included in the revision and I apologize if I missed it. However, a clear statement of the number of separate experiments that were used in the study speaks to reproducibility of the results.

eLife. 2020 May 12;9:e53885. doi: 10.7554/eLife.53885.sa2

Author response

Essential revisions:

1) Some caveats need to be included regarding the results. It is emphasized that the experiments put the looping experiments in a cellular context. Yes, extracts are used, but the first result of the paper is that H3-H4 dimers must be removed which makes these experiments rather more like in vitro experiments on naked DNA (e.g., by the Dekker and Peters labs) than experiments on chromatin. Further, it is emphasized that the experiments indicate that cohesin carries out symmetric extrusion which condensin is asymmetric, while Figure 2 indicates a mixed distribution, with peaks towards the symmetric and asymmetric cases for interphase/cohesin and metaphase/condensin. According to Figure 2 there is an appreciable subfraction of asymmetric cohesin traces and symmetric condensin traces in the data. The work of Banigan and Mirny puts a 90% compaction limit on pure-one-sided extrusion (with random orientation of extruders) but mixing even a small fraction of two-sided extruders will lead to appreciable further compaction by filling in gaps between the quiet sides of adjacent one-sided extruders.

We thank the reviewers for raising these important points. One of the limitations of our study is that it lacks a comprehensive set of measurements on chromatinized DNA. Indeed we captured a number of looping examples in mock-depleted extracts, though these events were rare. Therefore, we decided to perform most of our measurements using naked DNA and nucleosome-depleted extract. We emphasized this limitation in the manuscript, and hypothesized that the tension generated by nucleosomal incorporation – in mock-depleted extracts – accounted for the low looping efficiency. In future work, our assay can tackle how nucleosomes influence looping activities driven by cohesin and condensin.

As the reviewers point out, Figure 2 displays symmetry scores that reveal differential modes of looping in a cell-cycle dependent manner. In interphase, the symmetry scores peak at “symmetric”, whereas in metaphase the symmetry scores peak at “non-symmetric”. We investigated whether the symmetric looping examples in metaphase (and, in contrast, non-symmetric looping examples in interphase) emerged as a consequence of noisy data, or whether they corresponded to genuine symmetric (or non-symmetric) loops. We now show some of those examples in Figure 2—figure supplement 3, and, indeed, these examples correspond to small sub-populations of symmetric and non-symmetric loops in metaphase and interphase, respectively. We also found that ~20% of all loops in metaphase are symmetric, which is above the 10% limit that Banigan and Mirny require in their paper to fully compact eukaryotic chromosomes. We have added this observation to the paper’s Results section, and comment on how our results relate to the Banigan and Mirny prediction in the Discussion.

2) No differentiation is made between condensin I and condensin II; the initial structure of metaphase chromosomes during mitotic prophase is established by condensin II, but Xenopus extracts contain condensin I in excess (estimates of Hirano's group are in the 5:1 range for condensin II:condensin I). Have experiments been done along the lines of those in Figure 2 to determine the nature of loop extrusion (symmetric vs. asymmetric) for depletions of condensin I and condensin II-specific components (e.g., XCAP-H and XCAP-H2)? It is therefore not clear whether the two species of condensin have the same loop-extrusion-symmetry properties and it could well be that the more symmetric cases in Figure 2Diii correspond to more symmetric activities of condensin II. For the extracts with excess condensin I it would seem wise to not draw conclusions about condensin II. I do not require new experiments with specific depletions of either condensin I or II (although if they can be added that would be interesting), but do ask for a more careful discussion of the possible roles of the two complexes.

We immunodepleted condensin using antibodies raised against SMC2 and SMC4, which are subunits shared by both condensin I and II. Thus, as the reviewers point out our study cannot distinguish the possible differential loop extrusion properties of condensin I and II. We have now added this point in the main text.

The Hirano lab estimated the relative abundance of condensin I : condensin II in Xenopus laevis egg extract as ~5:1. The Green lab recently showed that condensin I and condensin II compact DNA loops with different symmetries (Kong et al., 2019), which suggests that the symmetric sub-fraction that we observe in our metaphase looping data could originate from a differential looping mechanism of condensin II. To investigate this, we compared the symmetric looping event frequency (symmetry score below 0.5) to the whole population of metaphase looping events, showing that ~ 20% (7 out of 32) of metaphase looping events are symmetric. This number is surprisingly close to the estimated 1:5 relative abundance of condensin II vs. condensin I from the Hirano lab, suggesting a “possible” symmetric mechanism of loop extrusion by condensin II in Xenopus egg extract. We plan to perform Condensin I- and Condensin II-specific immunodepletions to understand these differences in symmetry, but these experiments are beyond the scope of this paper.

3) There seems to be some inconsistency between the stall forces reported in the text (0.41 pN for condensin, and 0.23pN for cohesin) and those shown in Figure 2D (the mean ~0.1pN for both). I also wonder whether errors in determining the relative extension of DNA could have contributed to the estimate of the stall force. Perhaps authors can test whether estimated stall force for each trajectory depends on the initial and final slack.

We thank the reviewers for pointing out this typographic error. Instead of 0.41 pN, it should have read 0.14 pN. We have now updated these force values with more data.

We computed the amount of DNA in a loop using relative fluorescence intensities from a region defined as “inside” the loop and two regions “outside” of the loop (Ganji et al., 2018; Ganji et al., 2016). Clearly, this method is vulnerable to DNA signal fluctuations – for example, via thermal fluctuations of the DNA molecule. To address these signal fluctuations, we used stall forces as a measure to describe looping events. Stall forces are more robust than other measurables, such as the initial extrusion rate, because segmentation errors in determining the loop amount decrease as the loop grows. Moreover, since the stall force is calculated when the loop amount reaches a plateau, the fluctuations around this plateau can be removed by fitting, smoothing, or taking a time average. All three methods provide a robust measure of the stall force and give consistent results. We have included these new analyses in Figure 2—figure supplement 2.

As the reviewers suggested, we tested whether the estimated stall forces for each trajectory depend on the initial slack. We found that the stall forces show a slight increase as a function of initial slack, which we now show in Figure 2—figure supplement 5. We reasoned that this slight increase comes from selecting loop extrusion events that have to overcome the initial strand tension. Thus, only loops that have higher stall forces than the initial tension can be measured, leading to a slight increase in the overall stall force as a function of decreasing initial slack. We also show the corresponding initial strand tension as computed from the worm-like-chain model for comparison in Figure 2—figure supplement 5.

4) Looking at Figure 2Biv and v I was a bit surprised that the sum of the length of three regions: DNA to the left from the loop, to the right from the loop, and in the loop don't appear to sum up to a constant. On Figure 2Biv the sum of the three at t=0 is 37Kb, at 30sec its about 43Kb… May be this is just a measurement error… The loop gained 25Kb, but the flanks lost less than 20Kb together. In fitting these curves, I wonder whether the assumption about the sum of the three to be constant was used (and whether using it would make a difference for the rates and the stall forces). Also, in Figure 2Bv, it looks like the loop is getting smaller for trajectories with larger relative extension, (initial, I assume) but the flanks are not getting smaller or larger.

This is an important point, as the total sum of DNA is conserved by definition. The inconsistency in Figure 2B was due to a plotting script error, and has been corrected for the manuscript’s current version. The corresponding stall forces and extrusion rates were not affected as the error occurred in the plotting script.

However, in our previous analysis, we overlooked the fact that the DNA intercalating dye (Sytox orange) lengthens the DNA’s contour length in a concentration-dependent manner. To control for this, we hydrodynamically stretched lambda DNA with a flow rate of known force, and exposed the DNA to buffer containing different Sytox Orange concentrations. By measuring the corresponding length distributions of the DNA molecules for all dye concentrations employed in this study, we acquired a calibration curve that allowed us to determine the contour length of the lambda DNA molecule for each dye concentration. Each looping data set has been re-analyzed with its respective dye-dependent contour length, which slightly affected downstream analysis of the stall forces. We have now added the calibration analysis in the Materials and methods section and showed the calibration curve in Figure 2—figure supplement 6.

This plot is generally hard to understand. Authors may want to have a diagram to help the reader with Figure 2Bii, v and also explain what's shown: one point per trajectory or one point per time from a single (or multiple trajectories).

We agree with the reviewers that the plot is hard to interpret. In Figure 2Bii, v, we sought to demonstrate the change in DNA distribution through the process of loop extrusion for the whole population of loop extrusion events in inter- and metaphase. Every loop extrusion event is represented by three values that display the total change in size of the loop region (blue) and the two regions outside of the loop (green and orange) between the initiation of a loop and the saturation in loop size. We decided to plot those values over the initial extension (the end-to-end distance over the DNA contour length) of the DNA strand to illustrate how those depend on the available slack in the strand. To facilitate the understanding of these plots, we have added small explanatory figures to Figure 2Bi and iv, demonstrating how we convert each individual loop extrusion curve to a triplet of values plotted for the whole population of looping events in Figure 2Bii and v.

5) Figure 2C is very important as it provides the estimate of the speed of extrusion. It looks like this plot shows one point (rate at t=0) per trajectory. It may be interesting to see (i) whether rate vs. extension is a universal dependence; and (ii) the extent of variation around this dependence for individual trajectories. Perhaps authors can put not only t=0, but some smoothed velocity vs. extension for different time points on the same plot and/or their exp fits. In other words, if one trajectory starts with less extension and at a higher speed, while another starts at a greater extension and lower speed, will the speed of the first one gets down to the same level when it achieves the same extension as the initial one of the second trajectory. Using these data for all trajectories together (data for different t, not only t=0) may provide a more robust estimate of the rate of extrusion that the two exp fits (one to get rate(t=0) for each trajectory, and the other for rate(t=0) vs. extension).

We thank the reviewer for this very important suggestion. We have analyzed the tension-dependent extrusion rates in Figure 2—figure supplement 2, including a single trajectory as well as population statistics for inter- and metaphase. Briefly, we analyzed the rate of loop extrusion over time from the smoothed loop data, which we used to further calculate the tension on the DNA molecule for each time point. This allowed us to plot the extrusion rate as a function of DNA tension, but also to demonstrate the effect of small fluctuations in the loop signal on the tension estimate. By fitting the loop data with an exponential function, together with the end-to-end distance of the corresponding DNA strand, we showed the general trend of the extrusion rate as a function of DNA tension for the entire inter- and metaphase population of looping events.

I wonder whether more accuracy way of measuring the speed of extrusion (or reeling) could be achieved by using very low level of DNA labeling so that individual speckles of DNA can be vitalized and tracked in the flanking regions. This can, in principle, be precise enough to see pausing or switching events. May be this can be tried in future studies.

We agree with the reviewer that speckles of DNA would be a complementary method to measure extrusion speed and symmetries. We previously developed a dCas9-EGFP labelling strategy that targeted two specific loci roughly 1/3 and 2/3 along the length of the lamda DNA genome. We consistently observed two GFP spots on the lambda phage genome. However, the low brightness of the probe made it very hard to track these foci on fluctuating DNA strands with enough slack to support loop extrusion. We turned to sparse labelling of the DNA backbone with Cy5 fluorophores, using a commercial kit (Mirus Label IT Nucleic Acid Labelling). This approach gave promising results, and will be optimized for future studies.

Thanks to the suggestion of the reviewer, we developed a complementary approach to identify different loop extrusion symmetries in inter- and metaphase independent of quantifying the DNA fluorescence signal, as shown in Figure 2—figure supplement 4. The method consists of simply tracking the movement of the loop along the DNA strand during its formation. If a non-symmetric loop-extruding motor lands with a random orientation off center of the DNA molecule, it has a 50% chance of reeling-in DNA either from either the short or the long end of the DNA molecule. Consequently, the loop will get pulled either to the center or the boundary of the DNA molecule with equal probabilities. A completely symmetric motor, which reels in DNA from both sides with equal rates, will always pull itself towards the shorter end of the DNA molecule, as the lower amount of total slack in this part will get used up more readily. Consequently, a loop that is formed off-center by a symmetric loop-extruding enzyme, will always move towards the boundary of the DNA molecule. As shown in Figure 2—figure supplement 4, we indeed observe a 50% chance that a loop will move towards the center of the DNA molecule in metaphase, and a much reduced probability to do so in interphase. This suggests a strongly one-sided (non-symmetric) loop extrusion process in metaphase and a two-sided (symmetric) extrusion process in interphase with a certain fraction of non-symmetric cases. We found that there is a strong correlation between asymmetry of loop extrusion and the movement of the DNA loop towards or across the center of the DNA molecule: All of those ‘center-movers’ exhibit strongly non-symmetric DNA redistribution during the formation of the loop. On the other hand, we did not observe a single looping event that exhibited symmetric DNA redistribution and translocated towards the center of the molecule. We have added a short paragraph describing this approach in the Results section.

6) The difference in extrusion dynamics between interphase and mitotic extracts is one of the most exciting aspects of the manuscript. Since cohesin and condensins are labeled it would be great to look closer at the deviations from the textbook picture. So, I wonder whether authors can look at cohesin dynamics in the mitotic extract, and condensin dynamics in the interphase. Are cohesins completely unable to bind or to extrude in the mitotic exact? Can condensins bind/extrude in the interphase, though less efficiently? In other words, are these mere abundances of cohesin in the interphase and condensin in the metaphase, or is there a mechanistic control. Testing for such unconventional roles can be a very exciting twist of the story.

Condensins and cohesins were shown to exhibit tightly regulated alternating chromatin association during the cell cycle (Hirano and Hirano, 1997; Hirano and Mitchison, 1994; Losada and Hirano, 1998). Consistent with this literature, our immunostainings suggest that there is very little DNA association of cohesin in metaphase and no condensin occupancy of DNA in interphase. Unfortunately, we do not have a method of live-imaging cohesin or condensin activity in either of the cell cycles, as all of our antibodies are function-blocking for loop extrusion. We are currently developing strategies to perform these suggested experiments, but these experiments go beyond the scope of the paper. We have now included a discussion of this point in the Discussion section.

7) The major limit of the study is that there are no mechanistic details to explain the cell-cycle dependency of the reactions. Importantly, DNA loop extrusion by Cohesin had not been demonstrated until very recently, and it is clear that the authors reconstituted this reaction independently from the other two groups. I feel that at least some initial mechanistic detail on how the cell cycle regulates loop extrusion should be discussed.

This is an important remark and we have now included a discussion of possible mechanisms of cell cycle regulation of loop extrusion in the Discussion. Generally, the chromosomal association and dissociation of cohesin and condensins shows a strong dependency on the cell cycle with alternating chromatin occupancies by either cohesin in interphase or condensins in metaphase (Losada, Hirano and Hirano, 1997; Abramo et al., 2019). In Xenopus, human and mice, about 95% of cohesins dissociate from chromatin upon mitotic entry and only a minor subfraction stays associated with chromatin and is thought to mediate sister chromatid cohesion. The timing of condensin association with chromatin contrasts sharply with cohesin. Condensins are undetectable on interphase chromatin, but bind specifically and abundantly on mitotic chromosomes (Hirano and Mitchison, 1994).

Many questions still remain regarding the mechanisms underlying the cell cycle regulation of cohesin and condensins. For condensins, several mechanisms such as DNA replication, nuclear envelope breakdown or a mutual dependency on cohesin chromatin occupancy can be ruled out (Losada, Hirano and Hirano, 1997). The Hirano group suggests that cdk phosphorylation of one of condensin’s heat subunits could trigger the affinity of condensins for chromatin, as they have observed the hyperphosphorylation of its non-SMC subunits. For cohesin, it has been proposed that the phosphorylation of one of its SA subunits could control its dissociation from chromatin in prophase (Losada and Hirano, 2001). In contrast, CDK1 phosphorylation of soluble cohesin complexes can decrease their ability to bind to chromatin in vitro, yet cdk1 activity was not sufficient to dissociate cohesin from chromatin (Losada et al., JCB 2000). On the other hand, the mitosis-specific dissociation of cohesin from chromatin in late prophase in Xenopus laevis egg extract was shown to be independent of cyclin B proteolysis and the anaphase promoting complex, suggesting a separase-independent pathway for the bulk of cohesin dissociation from chromatin (Sumara et al., 2000).

[Editors' note: further revisions were suggested prior to acceptance, as described below.]

Essential revisions:

1) Reviewer #1 brought up these points:

I found the wording "symmetric" and "non-symmetric loops" a bit confusing, as loops are symmetric, and it is the process that generates them can be either symmetric or non-symmetric. The authors may want to replace "extrudes symmetric loops" with "extrudes loops symmetrically" (and similarly "extrudes loops non-symmetrically") in the Abstract and in the rest of the manuscript.

We thank the reviewer for raising this important point. We have changed the wording in the main text as suggested.

2) While presenting an equation for the Relative Extension (RE) is really helpful, some inconsistencies between RE presented on different plots remain an issue. Figure 2—figure supplement 5A and B largely disagree with all other plots presenting RE. According to Figure 2—figure supplement 5A, extrusion during interphase results in RE<0.5 for most of the cases, while extrusion during metaphase (Figure 2—figure supplement 5B) appears to be much more processive, resulting in RE>0.5 for most of the cases and at least 8 trajectories showing complete extrusion of all the slack in DNA into a loop (RE=1). Figure 2—figure supplement 5C, on the contrary, has all the points with RE<0.6; including 4 metaphase trajectories with RE<0.2, while only one such point is shown on Figure 2—figure supplement 5B. The number of trajectories (~20) on Figures 2—figure supplement 5A and B also disagree with the numbers of points shown in Figure 2—figure supplement 5C, and all inconsistent with the tallies of trajectories on Figure 2—figure supplement 4D.

We thank the reviewer for pointing out this error in the caption and x-axis titles of Figure 2—figure supplement 5A and B. The two panels show the initial loop extrusion rate over the symmetry score of the corresponding looping events in inter- and metaphase. Figure 2—figure supplement 5C, in contrast, depicts the stall force of all looping events over the initial relative extension of the DNA molecules. We accidently mislabeled the x-axis in Figure 2—figure supplement 5A and B and propagated this error into the figure legend, albeit correctly referencing it in the main text.

We now corrected the axis labels and figure legends accordingly, indicating the symmetry score of 0 as symmetric and 1 as non-symmetric extrusion in Figure 2—figure supplement 5A and B. As no stable looping events were observed beyond relative extensions of 0.6, we adjusted the range of the x-axis in Figure 2—figure supplement 5C from 0 to 0.8, to make an obvious distinction to the other plots in this figure. We apologize for the confusion.

While Figure 2—figure supplement 5C agrees the main Figure 2, where most of RE <0.6 for both interphase and metaphase, they disagree with the text which states the typical maximum (stalling) RE is ~65% in the metaphase extract (consistent with Figure 2—figure supplement 5B, but not all other figures). Overall, if indeed RE is vastly different in mitotic and interphase trajectories – it's a very interesting result.

The parameter relative extension (RE) always refers to the initial relative extension of the DNA molecule before a loop is formed (i.e. in Figure 2Bii and iv, Figure 2C and Figure 2—figure supplement 5C), as indicated in the figure legends. It must not be confused with the final extension of the DNA molecule at the end of loop extrusion, which is used to calculate the stall force. This can maybe be better understood by looking at Figure 2—figure supplement 2C, showing how the relative extension of one λ DNA molecule increases from an initial value of 0.54 (10um end-to-end distance over 18.5um contour length) prior to loop formation, to a final 0.75 at the end of loop formation.

The relationship between the initial relative extension of the DNA molecule (in other words the distance between the attachment points over its contour length) and the corresponding initial tension on the molecule is shown as a grey dashed line in Figure 2—figure supplement 5C.

In our main figures, we use relative extension as a parameter to show how the size of DNA loops and the extrusion rates decay with increasing end-to-end distance of the molecules, as less slack DNA is available for the loop and higher initial tensions slow the reeling in of DNA (see Figure 2Bii and iv and Figure 2C).

3) Since RE was used to infer the stall force, inconsistencies in RE may have propagated to those in the stall force estimates.

We thank the reviewer for this important point, and assure that the inconsistencies in RE originated exclusively from the error in the x-axis label and caption in Figure 2—figure supplement 5A and B. Stall forces were calculated from the final relative extensions of the DNA molecules at the final steady state of loop extrusion, which usually resulted in final relative extensions around 0.65, corresponding to stall forces below 0.2pN. Only very few examples, as the one shown in Figure 2—figure supplement 2, stalled at higher relative DNA extensions around 0.75.

In general, 0.16-0.18 pN forces reported here (with a smaller stall force for metaphase than for interphase, inconsistent with Figure 2—figure supplement 5C) is a factor of 10 lower than those found by for yeast condensin 1.2+-0.5pN and >0.8pN for human cohesin). It would be great if authors can comment on this.

We now added a discussion of this discrepancy in the main text. In brief, one possibility for the origin of the differences to in vitro studies could be, that in extract other DNA-binding proteins, such as linker histone, could bind to the DNA, competing for DNA with the loop extrusion machinery and stalling the loop prematurely. Since we cannot control for these additional factors binding to DNA, we might consequently be underestimating the stall forces of the loop extrusion machinery.

It appears that the quantification of force-extension (Figure 2—figure supplement 6) can make detecting of ~1pN forces extremely unlikely. According to Figure 2—figure supplement 6B, getting a force of about 1pN would require >96% extension. Works of Cees Dekker's lab use magnetic bead quantification (their Nano Letter 2016) where ~1pN are achieved at 80% extension. According to Figure 2—figure supplement 6B, 80% extension gives the force <0.1pN. This factor of 10 difference between force-extension quantification deserves authors attention.

We thank the reviewer for their careful inspection of the manuscript and pointing out this discrepancy. Indeed, we use the same worm-like chain model (Marko and Siggia, 1995) as was employed in the Dekker lab for the aforementioned magnetic bead quantification. The discrepancy was simply caused by an error in the label of the logarithmic y-axis, which was shifted by one order of magnitude. This error most likely happened during the formatting of the fonts in the axis labels. We have corrected this error and apologize for the confusion.

Furthermore, Figure 2—figure supplement 2F shows extrusion, albeit at a lower speed, above 0.1pN and up to 1pN force, raising further questions about the estimate of 0.16pN stall force.

Figure 2—figure supplement 2F was created from the exponential fits to the loop data and relates the extracted extrusion rate to the tension on the DNA molecule, with a bin size of 0.055pN. The reviewer is correct in stating that most of the loops we observe stall at forces below forces of 0.2 pN as shown in Figure 2D. We did however observe three examples of loops that stalled at forces around 0.7pN, as indicated in Figure 2—figure supplement 5C. These constitute the data points in Figure 2—figure supplement 2F that reach up to the 0.8pN bin. The upper bound of 1pN force in this graph was misplaced and we removed it.

4) At the end of the Discussion, the authors bring up a very interesting result (not mentioned in the Results section): "Interestingly, our results comparing interphase extrusion velocities with looping symmetries show that, on average, symmetric loops extrude with a higher rate (roughly twice) compared to the non-symmetric events (Figure 2—figure supplement 5)." Unfortunately, Figure 2—figure supplement 5 doesn't present symmetry vs. rate data.

Please see our response to point 2. As stated there, the x-axis and captions of Figure 2—figure supplement 5A and B were labelled incorrectly and indeed show the extrusion rate over the symmetry score of the corresponding looping event. We have corrected the figure accordingly.

This is very interesting and important result that should be presented in the Results section, and further highlighted in the Discussion.

Indeed, we observe a doubling in extrusion rates when comparing symmetric to non-symmetric looping events in interphase, yet we are limited to very few data points for non-symmetric looping events in that cell cycle phase. Consequently, we bring up this result as an exciting initial observation that we will further investigate in future studies to provide a more comprehensive study of extrusion rates and symmetries.

Alongside with the missing symmetry vs. velocity, the authors may want to present symmetry as a function of the initial relative extension, as those slower and one-sided events might reflect partial stalling of the motor activity, and thus may be more abundant at higher extension.

Upon this interesting suggestion from the reviewer we have analyzed our data in that regard and find no dependency of the loop extrusion symmetry on the initial extension of the DNA molecules.

5) The revision presents insightful new analysis of the movement of the loop relative to the border. While interesting, it may be hard for a reader to follow its logic without an illustration (and possibly also a mathematical formula in the Materials and methods). Such a figure can just explain why (i) two-sided extrusion initiated close to the border would make the loop move towards to the nearest border, while those initiated away from the border wouldn't; and (ii) why one-sided extrusion would move a loop to the border or toward the center with 50/50 chances.

We thank the reviewer for his suggestion and have implemented explanatory figures Figure 2—figure supplement 4A and B which schematically explain the differential movements of loops based on the underlying extrusion symmetry.

6) Sentences comparing one-sided extrusion in metaphase with our recent theory paper are somewhat confusing. The manuscript reads "This (theory) work puts the lower limit on symmetric extrusion at 10%. In contrast to in vitro studies of condensin loop extrusion, we found a small sub-population of symmetric loop extrusion in metaphase (~20%) that is above the 10% theoretical limit." Our theory work states that purely one-sided extrusion would leave ~10% of DNA unextruded, setting a limit of ~10-fold compaction due to one-sided extrusion. Hence "10%" in our study does not refer to the fraction of one-sided or two-sided extruders that is required for compaction. However, we did consider mixtures of one-sided and two-sided extruders, demonstrating that a mixture of 40% one-sided and 60% two-sided extruders can achieve 100-fold linear compaction, while achieving 1000-fold compaction would require ~85% two-sided extruders in the mixture. Data presented in the revised manuscript indeed show that in the mitotic extract about 50% of condensins are one-sided (symmetry 0.8-1.0), while the remaining 50% are asymmetric, but two-sided events. As discussed in our PRX paper, even highly asymmetric extrusion can be consistent with "two-sided" extrusion if such asymmetric extruders stay on the chromatin long enough to close the gaps on their "slowly" extruding sides. Thus, the authors can state that this 50% asymmetric extrusion could be sufficient to achieve about 100-fold compaction. Further studies of extruders with different distributions of symmetries and speeds or residence times are underway in our group.

We thank the reviewer for bringing up this a very important point and apologize for the misleading statement in our previous manuscript. We have now updated our discussion of partially symmetric loop extrusion cases in metaphase, according to the suggestions of the reviewer.

7) Reviewer #3 brings up these points:

The revision has addressed the comments and suggestions of the first round of review. I still think the paper is not as crystal clear as it should be about a key point, namely the use of H3/H4-depleted extracts (without which the effects reported in the paper could not be observed). It should be made very clear that these experiments are not on chromatinized DNA, as most people in the field know is rapidly formed when naked DNA is put into Xenopus extracts.

I think that the phrase "histone H3/H4-depleted" should be put in front of "Xenopus egg extracts" either in the Abstract or in the last paragraph of the Results.

We thank the reviewer for this suggestion and have changed the manuscript accordingly.

Numbers of molecules are now included in the Results which is helpful. However, numbers of separate experiments are not listed (N=52 means 52 molecules in one flow cell or 13 molecules observed in 4 separate experiments (flow cells)). Perhaps this information is included in the revision and I apologize if I missed it. However, a clear statement of the number of separate experiments that were used in the study speaks to reproducibility of the results.

We have made sure that we state the number of days used to repeat the experiment in the main text and the corresponding figure legends.

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Supplementary Materials

Source code 1. DNA-strand analysis.

elife-53885-code1.ipynb^{(1.8MB, ipynb)}

Source data 1. Interphase loops raw data.

elife-53885-data1.zip^{(77.1KB, zip)}

Source data 2. Metaphase loops raw data.

elife-53885-data2.zip^{(78.1KB, zip)}

Transparent reporting form

elife-53885-transrepform.docx^{(246.5KB, docx)}

Data Availability Statement

All data generated or analysed during this study are included in the manuscript.

[bib1] Abdennur N. Condensin II inactivation in interphase does not affect chromatin folding or gene expression. bioRxiv. 2018 doi: 10.1101/437459. [DOI]

[bib2] Abramo K, Valton A, Venev SV, Ozadam H. Chromosome folding intermediate at the condensin-to-cohesin transition during telophase. bioRxiv. 2019 doi: 10.1038/s41556-019-0406-2. [DOI] [PMC free article] [PubMed]

[bib3] Alipour E, Marko JF. Self-organization of domain structures by DNA-loop-extruding enzymes. Nucleic Acids Research. 2012;40:11202–11212. doi: 10.1093/nar/gks925. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib4] Banigan EJ, Berg A, Brandão HB, Marko JF. Chromosome organization by one-sided and two-sided loop extrusion. bioRxiv. 2019 doi: 10.7554/eLife.53558. [DOI] [PMC free article] [PubMed]

[bib5] Banigan EJ, Mirny LA. Limits of chromosome compaction by loop-extruding motors. bioRxiv. 2018 doi: 10.1103/physrevx.9.031007. [DOI]

[bib6] Bonev B, Cavalli G. Organization and function of the 3D genome. Nature Reviews Genetics. 2016;17:661–678. doi: 10.1038/nrg.2016.112. [DOI] [PubMed] [Google Scholar]

[bib7] Bouwman BA, de Laat W. Getting the genome in shape: the formation of loops, domains and compartments. Genome Biology. 2015;16:1–9. doi: 10.1186/s13059-015-0730-1. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib8] Davidson IF, Bauer B, Goetz D, Tang W, Wutz G, Peters JM. DNA loop extrusion by human cohesin. Science. 2019;366:1338–1345. doi: 10.1126/science.aaz3418. [DOI] [PubMed] [Google Scholar]

[bib9] Dekker J, Mirny L. The 3D genome as moderator of chromosomal communication. Cell. 2016;164:1110–1121. doi: 10.1016/j.cell.2016.02.007. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib10] Earnshaw WC, Laemmli UK. Architecture of metaphase chromosomes and chromosome scaffolds. The Journal of Cell Biology. 1983;96:84–93. doi: 10.1083/jcb.96.1.84. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib11] Fudenberg G, Imakaev M, Lu C, Goloborodko A, Abdennur N, Mirny LA. Formation of chromosomal domains by loop extrusion. Cell Reports. 2016;15:2038–2049. doi: 10.1016/j.celrep.2016.04.085. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib12] Ganji M, Kim SH, van der Torre J, Abbondanzieri E, Dekker C. Intercalation-Based Single-Molecule fluorescence assay to study DNA supercoil dynamics. Nano Letters. 2016;16:4699–4707. doi: 10.1021/acs.nanolett.6b02213. [DOI] [PubMed] [Google Scholar]

[bib13] Ganji M, Shaltiel IA, Bisht S, Kim E, Kalichava A, Haering CH, Dekker C. Real-time imaging of DNA loop extrusion by condensin. Science. 2018;360:102–105. doi: 10.1126/science.aar7831. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib14] Goloborodko A, Imakaev MV, Marko JF, Mirny L. Compaction and segregation of sister chromatids via active loop extrusion. eLife. 2016;5:e14864. doi: 10.7554/eLife.14864. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib15] Groen AC, Ngyuen PA, Field CM, Ishihara K, Mitchison TJ. Glycogen-supplemented mitotic cytosol for analyzing Xenopus egg microtubule organization. Methods in Enzymology. 2014;540:417–433. doi: 10.1016/B978-0-12-397924-7.00023-6. [DOI] [PubMed] [Google Scholar]

[bib16] Gruszka DT, Xie S, Kimura H, Yardimci H. Single-molecule imaging reveals control of parental histone recycling by free histones during DNA replication. bioRxiv. 2019 doi: 10.1101/789578. [DOI] [PMC free article] [PubMed]

[bib17] Hansen AS, Pustova I, Cattoglio C, Tjian R, Darzacq X. CTCF and cohesin regulate chromatin loop stability with distinct dynamics. eLife. 2017;6:e25776. doi: 10.7554/eLife.25776. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib18] Hauf S, Roitinger E, Koch B, Dittrich CM, Mechtler K, Peters JM. Dissociation of cohesin from chromosome arms and loss of arm cohesion during early mitosis depends on phosphorylation of SA2. PLOS Biology. 2005;3:e69. doi: 10.1371/journal.pbio.0030069. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib19] Hirano T, Kobayashi R, Hirano M. Condensins, chromosome condensation protein complexes containing XCAP-C, XCAP-E and a Xenopus homolog of the Drosophila barren protein. Cell. 1997;89:511–521. doi: 10.1016/S0092-8674(00)80233-0. [DOI] [PubMed] [Google Scholar]

[bib20] Hirano T, Mitchison TJ. Cell cycle control of higher-order chromatin assembly around naked DNA in vitro. The Journal of Cell Biology. 1991;115:1479–1489. doi: 10.1083/jcb.115.6.1479. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib21] Hirano T, Mitchison TJ. A heterodimeric coiled-coil protein required for mitotic chromosome condensation in vitro. Cell. 1994;79:449–458. doi: 10.1016/0092-8674(94)90254-2. [DOI] [PubMed] [Google Scholar]

[bib22] Kim Y, Shi Z, Zhang H, Finkelstein IJ, Yu H. Human cohesin compacts DNA by loop extrusion. Science. 2019;366:1345–1349. doi: 10.1126/science.aaz4475. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib23] Kinoshita K, Hirano T. Dynamic organization of mitotic chromosomes. Current Opinion in Cell Biology. 2017;46:46–53. doi: 10.1016/j.ceb.2017.01.006. [DOI] [PubMed] [Google Scholar]

[bib24] Kong M, Cutts E, Pan D, Beuron F, Kaliyappan T, Xue C, Morris E, Musacchio A, Vannini A. Human condensin I and II drive extensive ATP – dependent compaction of nucleosome – bound DNA. bioRxiv. 2019 doi: 10.1101/683540. [DOI] [PMC free article] [PubMed]

[bib25] Losada A, Hirano M, Hirano T. Identification of Xenopus SMC protein complexes required for sister chromatid cohesion. Genes & Development. 1998;12:1986–1997. doi: 10.1101/gad.12.13.1986. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib26] Losada A, Hirano T. Shaping the metaphase chromosome: coordination of cohesion and condensation. BioEssays. 2001;23:924–935. doi: 10.1002/bies.1133. [DOI] [PubMed] [Google Scholar]

[bib27] Lupiáñez DG, Kraft K, Heinrich V, Krawitz P, Brancati F, Klopocki E, Horn D, Kayserili H, Opitz JM, Laxova R, Santos-Simarro F, Gilbert-Dussardier B, Wittler L, Borschiwer M, Haas SA, Osterwalder M, Franke M, Timmermann B, Hecht J, Spielmann M, Visel A, Mundlos S. Disruptions of topological chromatin domains cause pathogenic rewiring of gene-enhancer interactions. Cell. 2015;161:1012–1025. doi: 10.1016/j.cell.2015.04.004. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib28] Marko JF, Siggia ED. Stretching DNA. Macromolecules. 1995;28:8759–8770. doi: 10.1021/ma00130a008. [DOI] [Google Scholar]

[bib29] Marsden MP, Laemmli UK. Metaphase chromosome structure: evidence for a radial loop model. Cell. 1979;17:849–858. doi: 10.1016/0092-8674(79)90325-8. [DOI] [PubMed] [Google Scholar]

[bib30] Murray AW. Methods in Cell Biology. Vol. 36. Elsevier; 1991. Cell cycle extracts; pp. 581–605. [DOI] [Google Scholar]

[bib31] Nagano T, Lubling Y, Várnai C, Dudley C, Leung W, Baran Y, Mendelson Cohen N, Wingett S, Fraser P, Tanay A. Cell-cycle dynamics of chromosomal organization at single-cell resolution. Nature. 2017;547:61–67. doi: 10.1038/nature23001. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib32] Nasmyth K. Disseminating the genome: joining, resolving, and separating sister chromatids during mitosis and meiosis. Annual Review of Genetics. 2001;35:673–745. doi: 10.1146/annurev.genet.35.102401.091334. [DOI] [PubMed] [Google Scholar]

[bib33] Naumova N, Imakaev M, Fudenberg G, Zhan Y, Lajoie BR, Mirny LA, Dekker J. Organization of the mitotic chromosome. Science. 2013;342:948–953. doi: 10.1126/science.1236083. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib34] Nuebler J, Fudenberg G, Imakaev M, Abdennur N, Mirny LA. Chromatin organization by an interplay of loop extrusion and compartmental segregation. PNAS. 2018;115:E6697–E6706. doi: 10.1073/pnas.1717730115. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib35] Ono T, Losada A, Hirano M, Myers MP, Neuwald AF, Hirano T. Differential contributions of condensin I and condensin II to mitotic chromosome architecture in vertebrate cells. Cell. 2003;115:109–121. doi: 10.1016/S0092-8674(03)00724-4. [DOI] [PubMed] [Google Scholar]

[bib36] Rao SS, Huntley MH, Durand NC, Stamenova EK, Bochkov ID, Robinson JT, Sanborn AL, Machol I, Omer AD, Lander ES, Aiden EL. A 3D map of the human genome at Kilobase resolution reveals principles of chromatin looping. Cell. 2014;159:1665–1680. doi: 10.1016/j.cell.2014.11.021. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib37] Rao SSP, Huang SC, Glenn St Hilaire B, Engreitz JM, Perez EM, Kieffer-Kwon KR, Sanborn AL, Johnstone SE, Bascom GD, Bochkov ID, Huang X, Shamim MS, Shin J, Turner D, Ye Z, Omer AD, Robinson JT, Schlick T, Bernstein BE, Casellas R, Lander ES, Aiden EL. Cohesin loss eliminates all loop domains. Cell. 2017;171:305–320. doi: 10.1016/j.cell.2017.09.026. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib38] Ren G, Jin W, Cui K, Rodrigez J, Hu G, Zhang Z, Larson DR, Zhao K. CTCF-Mediated Enhancer-Promoter interaction is a critical regulator of Cell-to-Cell variation of gene expression. Molecular Cell. 2017;67:1049–1058. doi: 10.1016/j.molcel.2017.08.026. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib39] Rowley MJ, Corces VG. Organizational principles of 3D genome architecture. Nature Reviews Genetics. 2018;19:789–800. doi: 10.1038/s41576-018-0060-8. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib40] Sanborn AL, Rao SS, Huang SC, Durand NC, Huntley MH, Jewett AI, Bochkov ID, Chinnappan D, Cutkosky A, Li J, Geeting KP, Gnirke A, Melnikov A, McKenna D, Stamenova EK, Lander ES, Aiden EL. Chromatin extrusion explains key features of loop and domain formation in wild-type and engineered genomes. PNAS. 2015;112:E6456–E6465. doi: 10.1073/pnas.1518552112. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib41] Schoenfelder S, Fraser P. Long-range enhancer-promoter contacts in gene expression control. Nature Reviews Genetics. 2019;20:437–455. doi: 10.1038/s41576-019-0128-0. [DOI] [PubMed] [Google Scholar]

[bib42] Schwarzer W, Abdennur N, Goloborodko A, Pekowska A, Fudenberg G, Loe-Mie Y, Fonseca NA, Huber W, Haering CH, Mirny L, Spitz F. Two independent modes of chromatin organization revealed by cohesin removal. Nature. 2017;551:51–56. doi: 10.1038/nature24281. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib43] Shintomi K, Inoue F, Watanabe H, Ohsumi K, Ohsugi M, Hirano T. Mitotic chromosome assembly despite nucleosome depletion in Xenopus egg extracts. Science. 2017;356:1284–1287. doi: 10.1126/science.aam9702. [DOI] [PubMed] [Google Scholar]

[bib44] Smith SB, Cui Y, Bustamante C. Overstretching B-DNA: the elastic response of individual double-stranded and single-stranded DNA molecules. Science. 1996;271:795–799. doi: 10.1126/science.271.5250.795. [DOI] [PubMed] [Google Scholar]

[bib45] Smith EM, Lajoie BR, Jain G, Dekker J. Invariant TAD boundaries constrain Cell-Type-Specific looping interactions between promoters and distal elements around the CFTR locus. The American Journal of Human Genetics. 2016;98:185–201. doi: 10.1016/j.ajhg.2015.12.002. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib46] Strick TR, Kawaguchi T, Hirano T. Real-Time detection of Single-Molecule DNA compaction by condensin I. Current Biology. 2004;14:874–880. doi: 10.1016/j.cub.2004.04.038. [DOI] [PubMed] [Google Scholar]

[bib47] Sumara I, Vorlaufer E, Gieffers C, Peters BH, Peters JM. Characterization of vertebrate cohesin complexes and their regulation in prophase. The Journal of Cell Biology. 2000;151:749–762. doi: 10.1083/jcb.151.4.749. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib48] Tang Z, Luo OJ, Li X, Zheng M, Zhu JJ, Szalaj P, Trzaskoma P, Magalska A, Wlodarczyk J, Ruszczycki B, Michalski P, Piecuch E, Wang P, Wang D, Tian SZ, Penrad-Mobayed M, Sachs LM, Ruan X, Wei CL, Liu ET, Wilczynski GM, Plewczynski D, Li G, Ruan Y. CTCF-Mediated human 3D genome architecture reveals chromatin topology for transcription. Cell. 2015;163:1611–1627. doi: 10.1016/j.cell.2015.11.024. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib49] Uhlmann F. SMC complexes: from DNA to chromosomes. Nature Reviews Molecular Cell Biology. 2016;17:399–412. doi: 10.1038/nrm.2016.30. [DOI] [PubMed] [Google Scholar]

[bib50] Xiao B, Freedman BS, Miller KE, Heald R, Marko JF. Histone H1 compacts DNA under force and during chromatin assembly. Molecular Biology of the Cell. 2012;23:4864–4871. doi: 10.1091/mbc.e12-07-0518. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib51] Yan J, Maresca TJ, Skoko D, Adams CD, Xiao B, Christensen MO, Heald R, Marko JF. Micromanipulation studies of chromatin fibers in Xenopus egg extracts reveal ATP-dependent chromatin assembly dynamics. Molecular Biology of the Cell. 2007;18:464–474. doi: 10.1091/mbc.e06-09-0800. [DOI] [PMC free article] [PubMed] [Google Scholar]

[bib52] Yatskevich S, Rhodes J, Nasmyth K. Organization of chromosomal DNA by SMC complexes. Annual Review of Genetics. 2019;53:445–482. doi: 10.1146/annurev-genet-112618-043633. [DOI] [PubMed] [Google Scholar]

[bib53] Zierhut C, Jenness C, Kimura H, Funabiki H. Nucleosomal regulation of chromatin composition and nuclear assembly revealed by histone depletion. Nature Structural & Molecular Biology. 2014;21:617–625. doi: 10.1038/nsmb.2845. [DOI] [PMC free article] [PubMed] [Google Scholar]

PERMALINK

Cohesin and condensin extrude DNA loops in a cell cycle-dependent manner

Stefan Golfier

Thomas Quail

Hiroshi Kimura

Jan Brugués

Roles

Abstract

Introduction

Results

Figure 1. Single DNA molecule assay for direct visualization of DNA looping in Xenopus egg extracts.

Figure 1—figure supplement 1. Characterization of DNA compaction in Xenopus laevis egg extracts.

Figure 1—video 1. Addition of crude Xenopus egg extract to a single strand of λ-phage DNA, visualized using Sytox Orange, leads to the generation of multiple highly-enriched DNA clusters, suggestive of nucleosomal formation along the strand.

Figure 1—video 2. Addition of crude Xenopus egg extract to a single strand of λ-phage DNA, visualized using Sytox Orange, leads to the generation of multiple highly-enriched DNA clusters, suggestive of nucleosomal formation along the strand.

Figure 1—video 3. Example of DNA loop formation in H3-H4-depleted egg extract arrested in metaphase visualized using Sytox Orange.

Figure 1—video 4. Example of DNA loop formation in H3-H4-depleted egg extract in metaphase visualized using Sytox Orange.

Figure 1—video 5. Example of DNA loop formation in H3-H4-depleted egg extract in interphase visualized using Sytox Orange.

Figure 1—video 6. Example of DNA loop formation in H3-H4-depleted egg extract in interphase visualized using Sytox Orange.

Figure 1—video 7. Example of hydrodynamically stretched DNA loops in H3-H4-depleted extract arrested in metaphase visualized using Sytox Orange.

Figure 1—video 8. Example of a hydrodynamically stretched DNA loop in H3-H4-depleted interphase extract visualized using Sytox Orange.

Figure 1—video 9. Example of a hydrodynamically stretched DNA loop in H3-H4-depleted interphase extract visualized using Sytox Orange.

Figure 1—video 10. Example of DNA loop formation in non-depleted crude extract visualized using Sytox Orange.

Video 1. Example of DNA loop formation in H3-H4-depleted egg extract arrested in metaphase visualized using Sytox Orange.

Video 2. Example of DNA loop formation in H3-H4-depleted egg extract in interphase visualized using Sytox Orange.

Figure 2. Symmetry of DNA loop extrusion is cell cycle-dependent with similar extrusion rates and stalling forces.

Figure 2—figure supplement 1. DNA looping examples demonstrating non-symmetric loop extrusion in metaphase and symmetric loop extrusion in interphase.

Figure 2—figure supplement 2. Biophysical characterization of loop extrusion rate and DNA tension.

Figure 2—figure supplement 3. Examples of symmetric DNA loop extrusion in metaphase and non-symmetric loop extrusion in interphase.

Figure 2—figure supplement 4. Characterization of loop movement and symmetry.

Figure 2—figure supplement 5. Dependency of loop extrusion rate on extrusion symmetry and stall forces on initial relative DNA extension.

Figure 2—figure supplement 6. Correction for dye-induced DNA lengthening.

Figure 2—video 1. Example of hydrodynamically stretched DNA loops in H3-H4-depleted extract arrested in metaphase visualized using Sytox Orange.

Figure 3. Condensin extrudes DNA loops in metaphase and cohesin extrudes loops in interphase.

Figure 3—figure supplement 1. Co-immunodepletions of Xenopus egg extracts using antibodies targeting H3-H4, cohesin, and condensin I and II.

Discussion

Materials and methods

Xenopus laevis egg extract preparation, immunodepletions, and ATP depletion

Western blots

Antibody production and labeling

DNA functionalization

PEGylation of cover slips and DNA micro-channel preparation

Imaging

Hydrodynamic stretching of loops

Correction of dye-induced DNA lengthening

Loop extrusion analysis

Acknowledgements

Funding Statement

Contributor Information

Funding Information

Additional information

Competing interests

Author contributions

Ethics

Additional files

Data availability

References

Decision letter

Roles

Author response

Author response image 1.

Associated Data

Supplementary Materials

Data Availability Statement

ACTIONS

PERMALINK

RESOURCES

Similar articles

Cited by other articles

Links to NCBI Databases