Abstract
Objective: We compare population aging in Europe and Asia using a measure that is both consistent over time and appropriate for cross-country comparison. Method: Sanderson and Scherbov proposed to estimate the old-age threshold by the age at which the remaining life expectancy (RLE) equals 15 years. We propose an adjustment of this measure, taking into account cross-national differences in the exceptionality of reaching that age. Results: Our old-age threshold was lower than 65 years in 2012 in Central Asia, Southern Asia, Southeastern Asia, and many Eastern European countries. These populations also experienced a higher share of elderly compared with the RLE15 method. Our method revealed more geographical diversity in the shares of elderly. Both methods exhibited similar time trends for the old-age thresholds and the shares of elderly. Discussion: Our prospective and comparative measure reveals higher population aging estimates in most Asian and Eastern European countries and more diversity in aging.
Keywords: population aging, characteristics approach, adult survival, Europe, Asia
Introduction
In most countries, the numbers of elderly and their population shares have been increasing rapidly in recent decades, and these trends are expected to accelerate in the coming decades. Population aging will likely to be the most important social change of the 21st century (Lutz, Sanderson, & Scherbov, 2008a). Aging is occurring rather quickly in Europe and in Asia. The shares of elderly in the total population are the highest in European countries, whereas the absolute numbers of older people are the highest in Asian countries (United Nations [UN], 2016). However, these estimates on the alarming increase in the share of elderly are based on a fixed old-age threshold of 65 years.
An important drawback of the conventional measures of aging—such as the proportion of people aged 65 or 80 years and above, or the old-age dependency ratio (Lutz, Sanderson, & Scherbov, 2008b)—is that they do not take into account the large increases in life expectancy that have been observed in almost all parts of the world over the past five decades (Spijker, 2015). In many parts of the world, the elderly who are alive today are healthier and have less severe disabilities than their earlier counterparts (Christensen, Doblhammer, Rau, & Vaupel, 2009). The conventional measures do not account for such major improvements in health and life expectancy. Hence, there is a tendency to overestimate the impact of population aging when these indicators are used (Spijker & MacInnes, 2013).
Of the various alternative approaches to measure aging (Chu, 1997; d’Albis & Collard, 2013; Ryder, 1975; Kot & Kurkiewicz, 2004; Skirbekk, Loichinger, & Weber, 2012), the prospective age approach was a significant progress toward the measurement of population aging (Sanderson & Scherbov, 2005, 2007, 2008, 2010). In the original prospective age approach, the size of the elderly population (i.e., the people who are older than the old-age threshold) is estimated based not on chronological (and thus on retrospective) age but on a forward-looking approach that defines the old-age threshold based on a constant remaining life expectancy (RLE) of 15 years. The basic idea behind the prospective age approach is that, for a certain country, a 65-year-old person in 1970 is likely to be very different from a 65-year-old person in 2010, because of increases over time in life expectancy and because a person’s behavior is influenced more by a person’s expected remaining years of life than by a person’s previous years of life. Sanderson and Scherbov (2005, 2007, 2008, 2010, 2013, 2015a, 2015b, and 2015c) therefore argue—in line with the earlier argumentation by Ryder (1975)—that it is better to use an old-age threshold that is defined based on a fixed RLE instead of a fixed chronological age. That is, for a certain country, a person with 15 remaining years of life in 1970 is considered closely comparable to a person with 15 remaining years or life in 2010. By redefining aging based on a fixed RLE, the prospective age approach proposed a dynamic old-age threshold that changes over time by accommodating the improvements in the life expectancies of populations over time.
Later, the prospective age approach was further modified (Sanderson & Scherbov, 2013, 2015a, 2015b, 2015c; Scherbov & Sanderson, 2016) and generalized in the, so called, “characteristic approach.” Instead of working with a constant RLE of 15 years, other characteristics which have direct implications for aging like mortality rate, grip strength, chair rise speed, or equitable pension age (defined by life course ratio) can also be used to redefine aging. For example, if the average grip strength at age 70 years in the year 2000 was equal to that at age 60 years in the year 1950, the approach considered age 60 years in 1950 the same as age 70 years in 2000 (Sanderson et al., 2016). Within the generalized framework of the “characteristic approach,” the prospective age approach using RLE = 15 years remains its most popular application (Sanderson & Scherbov, 2013).
Although the prospective age approach and the more general characteristics approach was successful in obtaining a time horizon constant aging measure, it has limitations for comparisons across countries with varying mortality patterns. In a country with high mortality at young and adult ages, reaching the age at which the RLE is 15 years will be more exceptional than in a country with lower mortality. How exceptional it is to reach a particular old age in a country will determine the attitudes toward and status of the elderly (Angus & Reeve, 2006; Dowd & Bengtson, 1978; Giles & Reid, 2005). Someone who reaches the age at which the RLE is 15 years in a country where only a small percentage of the population reaches this age is likely to be considered older than a person who reaches this age in a country where this is quite common. This hampers the comparability of aging across countries.
In this article, we compare population aging in Europe and Asia using a measure that is both consistent over time and appropriate for cross-country comparison. More specifically, within the framework of the characteristic approach, we combine a country specific life course characteristic (adult survival ratio [ASR]) with a constant characteristic (RLE = 15 years) in benchmark country Japan. By doing so, we accommodate for differences in the chances of reaching the age at which RLE = 15 years across countries. Using a selected set of countries from Europe and Asia, we demonstrate how our measure is more useful for the cross-country comparison of aging in Europe and Asia as compared with the RLE15 method while maintaining comparability over time as well.
Data and Method
Data
For our analysis, we used life table and population data by age and sex for Asian and European countries, for different years (1972, 1992, 2012). We used two sources. For the Organisation for Economic Co-operation and Development (OECD) member countries in Europe and for Japan, we used the available data from the Human Mortality Database (2015). For the remaining countries, and the regions (see below), data from the World Population Prospects Revision 2015 (UN, 2015) prepared by the Population Division of the Department of Economic and Social Affairs of the UN are used.
Whereas the Human Mortality Database provides annual population and life table data by single year of age, the UN database provides data by 5-year age groups for 5-year time intervals. We used UN data for 1970-1975, 1990-1995, and 2010-2015 as an estimate for the year 1972, 1992, and 2012, respectively.
To obtain data by single year of age based on the UN data available by 5-year age groups, we applied linear interpolation to the population and life table data (lx, RLE; Shryock, Stockwell, & Edward, 1976). A sensitivity analysis in which we used a more advanced interpolation technique (e.g., TOPALS; de Beer, 2012) revealed the same results.
Method
In the original prospective age approach, the prospective old-age threshold (POAT) (=the age from which people can be considered older) is defined as the age at which the RLE is 15 years (Sanderson & Scherbov, 2007). The value of 15 was chosen because in Europe, in 1980, the RLE at age 65 years was indeed 15 years, and because the use of a RLE of 15 years was considered less sensitive to data issues than the use of other values such as the 10 years suggested by Ryder (1975; Sanderson & Scherbov, 2008).
However, the original prospective age approach cannot sufficiently account for cross-country differences in aging. That is, the chances of survival to the age at which RLE is 15 years may be considerably different between countries with different mortality experiences. Table 1 illustrates this for selected countries within our final analysis (see the section “Empirical Application”). In 2012, 81% of the Japanese population, 84% of the population of Norway, 72% of the population of Ukraine, and 70% of the population of India were still alive at the age at which RLE = 15 years (Table 1). As people living in Ukraine or India were less likely to reach the age at which RLE = 15 years than people living in Japan or Norway, these people constitute a more special group than in Japan and Norway, and therefore are more likely to be considered older.
Table 1.
Age at Which RLE is 15 years and Percentage of Survivors to the Age at Which RLE = 15 Years for Japan, the Netherlands and India, 2012.
| Country | Age at which RLE = 15 years | Percentage of survivors to the age at which RLE = 15 years (%) |
|---|---|---|
| Japan | 73 | 81 |
| Norway | 71 | 84 |
| The Netherlands | 71 | 82 |
| Ukraine | 65 | 72 |
| China | 67 | 81 |
| Azerbaijan | 66 | 77 |
| India | 64 | 70 |
Note. RLE = remaining life expectancy.
When examining aging trends across countries, it is essential to take into account differences between countries in the exceptionality of reaching a RLE of 15 years to avoid comparing groups that are less or more special.
Our new measure, which we call the comparative prospective old-age threshold (CPOAT), adapts the original POAT by taking into consideration the differentials of reaching a RLE of 15 years due to variations in adult survival between countries and over time. Our method can be regarded an extended application of the overarching characteristic approach (Sanderson & Scherbov, 2013, 2015a, 2015b; Scherbov & Sanderson, 2016), in that it also uses different characteristics to measure aging but does so in a way to enable optimal cross-county comparison.
Our approach takes into account changes over time and differences across countries in the ASR which is calculated as
The ASR for an age for a country is the ratio of the population surviving to age in country (lx,i) to the population surviving to age 15 years in country i in a life table population (l15,i). The values of and are obtained from the life tables of the respective countries.
We considered the ASR and not the complete survival chances. In considering the survival of adults after age 15 years, we have excluded infant and child mortality which are not that relevant for determining whether a person can be considered “older.” If survival at young ages is low but survival changes from age 15 years are quite high, then reaching a certain high age will very likely be considered not that exceptional. Also, if survival at young ages is high but adult survival changes are low (like in Russia), then reaching a certain high age is very likely to be considered exceptional.
We multiply the RLE at each age by the ASR, that is, by the probability that a person aged 15 years will survive to that age. As the benchmark country, we selected Japan in the year 1972. The reason is that for Japan in 1972 the age at which RLE = 15 years was 65 years (Human Mortality Database, 2015). The ASR up to age 65 years in Japan in 1972 was 82.9%. Multiplying the RLE and ASR yields 12.4 years. This can be interpreted as the number of years that someone in Japan aged 15 years in 1972 could expect to live after age 65 years taking into account the probability that the person will survive to age 65 years.
Our CPOAT for each country and each year is the age at which the value of ASR × RLE is closest to 12.4 years. Thus if in one country the value of ASR × RLE exceeds 12.4 years at age 65 years, the old-age threshold is higher than 65 years. This may be due to the fact that the ASR at age 65 years is higher than 82.9% (in that country it is more common to reach age 65 years than in Japan in 1972) or that RLE is higher than 15 years (people aged 65 years may expect to live longer than Japanese aged 65 years in 1972).
To calculate the share of elderly, we divided the population size equal and higher than the old-age threshold with the total population size.
Empirical Application
In our empirical application, we will show the results of our new comparative prospective aging measure in terms of both the old-age threshold in 1972, 1992, and 2012 and the share of elderly in 2012.
For a selection of countries, we will compare the results of our new method with the results using the prospective RLE15 method, and the traditional measure using chronological age 65 years as old-age threshold, in table format. For this purpose, we selected “typical” countries from the five Asian regions and four European regions that the UN distinguishes (UN, 2018). These were China (East Asia), Thailand (Southeast Asia), India (South Asia), Azerbaijan (West Asia), Uzbekistan (Central Asia), Norway (Northern Europe), the Netherlands (Western Europe), Spain (Southern Europe), and Ukraine (Eastern Europe). For these countries, similar results were obtained regarding the comparison of CPOAT and POAT as compared with the regions to which the countries belong. We also show the results for the totals in Europe and Asia, based on the aggregate life table and population figures from the UN. Furthermore, we distinguish within Europe between Eastern Europe and the rest of Europe (non-Eastern Europe) and within Asia between Eastern Asia and the rest of Asia (non-Eastern Asia), because of the different results we observed for these regions. For Eastern Europe and Eastern Asia, we used as well the aggregate life table and population figures from the UN. For the “rest of Europe” and the “rest of Asia,” however, we applied unweighted averages to our results for the different subregions that it consists of.
In addition, we will map the share of elderly using our new measure for all European and Asian countries in 2012, using QGIS 2.14.3 and the world map provided by the QGIS website (QGIS Development Team, 2016). To match our data with the map, we had to exclude the Channel Islands when mapping the results. In Supplemental Appendix Figure III, we will show similar maps for the RLE15 method and using age 65 years as old-age threshold.
Results
Table 2 shows the old-age threshold, that is, the age at which people can be considered “older,” for both our new method and the RLE15 method, for the different Asian and European countries, in 1972 and 2012.
Table 2.
Comparison of the CPOAT With the POAT, for Selected Countries in 1972 and 2012.
| Country | CPOAT |
POAT |
Difference (CPOAT – POAT) | |||||
|---|---|---|---|---|---|---|---|---|
| 1972 | 2012 | Change | 1972 | 2012 | Change | 1972 | 2012 | |
| Japan | 65.00 | 73.31 | 8.31 | 65.00 | 73.29 | 8.29 | 0.00 | 0.02 |
| China | 59.74 | 66.92 | 7.18 | 61.26 | 67.13 | 5.86 | −1.53 | −0.21 |
| Thailand | 59.85 | 67.76 | 7.91 | 63.80 | 70.01 | 6.21 | −3.95 | −2.25 |
| India | 56.22 | 63.05 | 6.82 | 58.28 | 64.90 | 6.62 | −2.06 | −1.86 |
| Azerbaijan | 62.95 | 64.93 | 1.98 | 64.96 | 65.81 | 0.85 | −2.00 | −0.88 |
| Uzbekistan | 62.79 | 63.51 | 0.73 | 65.26 | 65.59 | 0.33 | −2.47 | −2.08 |
| Norway | 66.40 | 71.22 | 4.82 | 67.30 | 70.97 | 3.68 | −0.89 | 0.25 |
| Netherlands | 65.78 | 70.90 | 5.12 | 67.03 | 70.73 | 3.70 | −1.25 | 0.16 |
| Spain | 65.55 | 72.13 | 6.58 | 66.39 | 71.96 | 5.57 | −0.84 | 0.17 |
| Ukraine | 64.16 | 62.86 | −1.30 | 66.39 | 65.02 | −1.38 | −2.24 | −2.16 |
| Asia | 58.72 | 65.93 | 7.21 | 60.77 | 67.27 | 6.50 | −2.05 | −1.34 |
| Europe | 64.09 | 69.01 | 4.92 | 65.46 | 70.65 | 5.19 | −1.37 | −1.64 |
Note. CPOAT = comparative prospective old-age threshold; POAT = prospective old-age threshold.
In Japan, in 1972, the age at which RLE = 15 years was 65 years. The POAT using the RLE15 method was therefore 65 years in Japan in 1972. The ASR at age 65 years in Japan in 1972 was 82.9%. Because we consider Japan as the benchmark country in our new CPOAT, the CPOAT for Japan in 1972 equals the POAT for Japan in 1972. In the other years for Japan and in the other countries, however, the CPOAT resembles the age at which ASR × RLE = 0.829 × 15 = 12.4 years.
For Japan, the age at which RLE = 15 years increased to 73 years in 2012. Because, in Japan, the ASR up to age 73 years in 2012 (0.832) largely resembles the ASR up to age 65 years in Japan in 1972, the CPOAT is similar to the POAT in Japan in 2012 as well.
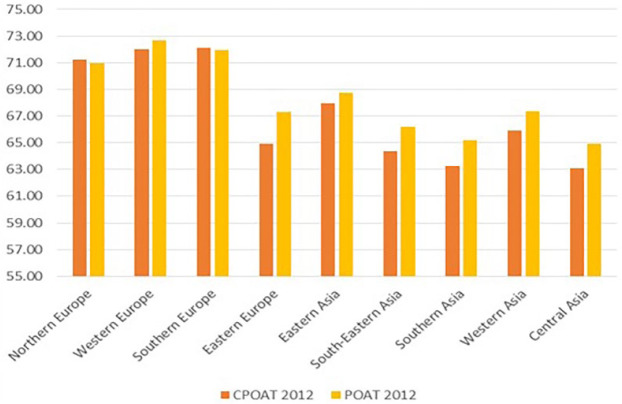
For the other countries, however, interesting differences exist between the POAT values and the CPOAT values. In general, the CPOAT values are smaller than the POAT values (see the negative values in the last two columns of Table 2). Differences are especially large for Thailand, India, Uzbekistan, and Ukraine. Figure 1 shows for 2012 that the lower CPOAT values compared with POAT values can be most clearly observed for Eastern Europe and the majority of Asian regions. Supplemental Appendix Figure 2, which shows the results for all individual European and Asian countries, illustrates this as well. The use of CPOAT over POAT therefore results in more diversity in old-age thresholds both within Europe and within Asia.
Figure 1.
Comparison of the CPOAT with the POAT, for Asian and European regions, 2012.
Note. CPOAT = comparative prospective old-age threshold; POAT = prospective old-age threshold.
All in all, in 2012, our CPOAT and POAT was highest among the selected countries, in Japan (73 years) and Spain (72 years), and lowest in Ukraine (63 years) and India (63 years). Our old-age threshold was higher than 65 years in 2012 in most countries, except in Southeastern Asia, Southern Asia, Central Asia, and many Eastern European countries. This in comparison to the POAT in 2012 which is 65 years or higher. For Europe as a whole, the CPOAT in 2012 was 3 years higher compared with Asia, 69 years and 66 years, respectively. Whereas Southern and Western Europe exhibited the highest average CPOAT (72 years), the lowest average CPOAT was observed for Southern Asia and Central Asia (63 years) (Tables 1 and A1 and Figure 1).
Table 2 also gives information about the change in the old-age thresholds from 1972 to 2012. In all selected countries, and using either our new measure or the RLE = 15 years measure, the old-age threshold has increased. Thus, in 2012 people are considered “old” when they are older as compared with 1972. One exception is for Ukraine, which shows a decline in the old-age threshold, as a result of the health crisis (Leon, 2011; McKee & Shkolnikov, 2001; Vallin & Meslé, 2004). For Central Asia, the old-age thresholds have been stable. According to our new measure, and for the selected countries, the increase in old-age thresholds has been strongest for Japan and Thailand (+8) and stronger for Asia (+7) as compared with Europe (+5). Interestingly, the changes over time we observed for the CPOAT measure are almost similar to the changes over time in the POAT measure. That is, a maximum difference of two is observed solely in Thailand and Western Asia.
Table 3 shows the share of elderly based on our new method for selected European and Asian countries in 2012. According to our method and among the countries considered, Ukraine has the highest share of elderly (17%), whereas Uzbekistan has the lowest share of elderly (5%). Europe has a share of elderly that is double from that of Asia, 13% and 6.5%, respectively.
Table 3.
Share of Elderly (%) With Our New Method, the RLE15 Method, and 65 as Old-Age Threshold, Selected European and Asian Countries Plus Average Asia and Europe, 2012.
| Country | Share of elderly 2012 | ||
|---|---|---|---|
| Our method | RLE15 method | Old-age threshold 65 years | |
| Japan | 13.86 | 13.86 | 23.70 |
| China | 7.27 | 7.27 | 8.65 |
| Thailand | 7.38 | 6.22 | 9.44 |
| India | 6.31 | 5.29 | 5.29 |
| Azerbaijan | 5.77 | 5.50 | 5.77 |
| Uzbekistan | 4.98 | 4.30 | 4.62 |
| Norway | 9.71 | 9.71 | 15.40 |
| Netherlands | 10.19 | 10.19 | 16.24 |
| Spain | 11.04 | 11.04 | 17.36 |
| Ukraine | 17.26 | 15.23 | 15.23 |
| Asia | 6.47 | 5.93 | 7.03 |
| Europe | 12.98 | 11.35 | 16.77 |
Note. RLE = remaining life expectancy.
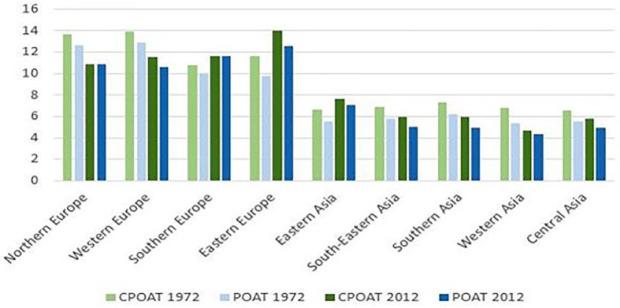
Table 3 also compares the share of elderly in 2012 obtained by our new method with the share of elderly using the RLE15 method, and the share of elderly using the traditional old-age threshold of 65 years. In addition, Figure 2 graphically shows the differences between the share of elderly in 1972 and 2012 for our new method and the RLE15 method. In line with our results on the old-age thresholds, we can see that the shares of elderly obtained for countries with low adult survival rates (most Asian countries, Ukraine) are slightly higher when our new method is used than when the RLE15 method is applied. The shares of elderly obtained for Southeastern Asia, Southern Asia, and Eastern Europe using the new method are also higher than the shares obtained using the traditional old-age threshold of 65 years. For Asia and Europe as a whole, the shares of elderly are as well slightly higher when using our method compared with the RLE15 method, but lower as compared with using age 65 years as old-age threshold. Examining the change in the share of elderly over time (Figure 2), we can observe both for our measure and the RLE15 method that the shares have been declining for most regions, except Southern Europe, Eastern Europe, and Eastern Asia, where the shares have been increasing.
Figure 2.
Share of elderly in 1972 and 2012 according to POAT and CPOAT.
Note. POAT = prospective old-age threshold; CPOAT = comparative prospective old-age threshold.
Figure 3 maps the shares of elderly for all European and Asian countries calculated using our method for the year 2012. Clear differences in the shares of elderly between and within the two continents exist. Within Asia, the United Arab Emirates and Qatar had the lowest shares of elderly (0.52% and 0.77%, respectively), whereas Japan and Georgia had the highest shares of elderly (13.86% and 13.48%, respectively). The resulting range (13.3) and variance (7.1) are substantially lower when 65 years is used as old-age threshold (22.9 and 15.8, respectively), and slightly higher (variance only) when the RLE15 method was used (13.3 and 6.9, respectively) (see Table 4 and Supplemental Appendix Figure III). Within Europe, Ireland and Iceland had the lowest share of elderly (7.37% and 7.50%, respectively) using our method for the year 2012, and Latvia and Lithuania had the highest shares (18.56% and 18.08%, respectively). The resulting range (11.2) and variance (7.5) are slightly higher than when 65 years is used as old-age threshold (10.8 and 6.5, respectively) but especially higher than when the RLE15 method was used (9.3 and 4.9, respectively). For all Asian and European countries combined, our CPOAT resulted in more diversity compared with the POAT and less diversity compared with the use of 65 years as old-age threshold.
Figure 3.

Share of elderly (%) in Europe and Asia using our new method, 2012.
Note. CPOAT = comparative prospective old-age threshold.
Table 4.
Comparison of the Range and Variation in the Share of Elderly for CPOAT, POAT, and 65 years as Old-Age Threshold, 2012.
| Maximum value | Country with maximum | Minimum value | Country with minimum | Range | Variance | |
|---|---|---|---|---|---|---|
| Asia | ||||||
| • CPOAT | 13.86 | Japan | 0.52 | United Arab Emirates | 13.34 | 7.08 |
| • POAT | 13.86 | Japan | 0.52 | United Arab Emirates | 13.34 | 6.87 |
| • 65 years | 23.70 | Japan | 0.85 | United Arab Emirates | 22.85 | 15.77 |
| Europe | ||||||
| • CPOAT | 18.56 | Latvia | 7.37 | Ireland | 11.19 | 7.53 |
| • POAT | 16.70 | Latvia | 7.37 | Ireland | 9.32 | 4.89 |
| • 65 years | 20.84 | Italy | 10.08 | Republic of Moldova | 10.76 | 6.50 |
| Asia and Europe | ||||||
| • CPOAT | 18.56 | Latvia | 0.52 | United Arab Emirates | 18.04 | 20.05 |
| • POAT | 16.70 | Latvia | 0.52 | United Arab Emirates | 16.18 | 18.11 |
| • 65 years | 23.70 | Japan | 0.85 | United Arab Emirates | 22.85 | 37.92 |
Note. CPOAT = comparative prospective old-age threshold; POAT = prospective old-age threshold.
Discussion
Summary of Results
We compared population aging in Europe and Asia using a measure that is both consistent over time and appropriate for cross-country comparison.
Our prospective and comparative old-age threshold (CPOAT) was lower than 65 years in 2012 in Central Asia, Southern Asia, Southeastern Asia, and many Eastern European countries. This in comparison to the POAT—as part of the RLE15 method—showed values of either 65 years and above. Consequently, the latter regions/countries experienced a higher share of elderly compared with both the use of 65 years as old-age threshold and the RLE15 method.
Our method resulted as well in more geographical diversity in the old-age threshold and the shares of elderly compared with the RLE15 method. Within Europe, our method also resulted in more diversity compared with the use of 65 years as old-age threshold.
Our CPOAT generally increased from 1972 to 2012 (Asia from 59 to 66; Europe from 64 to 69), in a similar manner as the POAT (Table A1). Changes over time in the shares of elderly were also roughly similar for the two methods and were almost absent for Asia and Europe as a whole (Table A2).
Explanation of the Observed Results
The lower CPOAT values compared with POAT values can be largely explained by differences in adult survival. Supplemental Appendix Figure 1 illustrates this for Thailand in 1972, for which we observed a CPOAT value of 60 that was 4 years lower than the POAT value of 64. Whereas, in 1972, RLE = 15 years occurs at age 64 years in Thailand (=POAT value) compared with age 65 years in Japan, the ASR64 for Thailand (0.658) is much lower than the ASR65 for Japan in 1972 (0.829). In other words, in Thailand which exhibits relatively high mortality at adult ages, many people do not survive to the age at which RLE = 15 years, or the traditional old-age threshold of 65 years. Controlling for these cross-national differences in the exceptionality of reaching RLE = 15 years, therefore, results in a lower old-age threshold as compared with the POAT for Thailand in 1972. By taking into account not only mortality in later life, as in the RLE method, but also differences between countries in survival rates across the life course, we clearly can account for differences in the exceptionality of reaching a certain old age.
Similarly, the other regions/countries for which we observe a lower CPOAT value compared with the POAT value (Eastern Europe, and the majority of Asian regions) exhibited relatively low adult survival, and consequently, chances of reaching a certain old age were lower. For Eastern Europe, the low adult survival can be largely linked to the health crisis that was experienced from 1975 onward as a result of communist regimes’ policies (Leon, 2011; McKee & Shkolnikov, 2001; Vallin & Meslé, 2004). For Asia, this can be linked to higher levels of adult mortality due to communicable diseases in the 1970s (Murray, Yang, & Qiao, 1992). Accounting for this low adult survival, and consequently, the exceptionality of reaching a certain old age as experienced in most Asian and Eastern European countries, as we do in our method, leads to a lower old-age threshold and, consequently, a higher share of elderly, as compared with the RLE15 method.
For these regions, our old-age threshold were lower and the shares of elderly were higher as compared with the traditional use of 65 years as the beginning of “old age” as well. Population aging, thus, seems to have been underestimated, so far, in most Asian and Eastern European countries.
Using the RLE15 method, not only the levels of population aging have been underestimated but also the differences between countries. That is, our method also resulted in more geographical diversity in the old-age threshold and the shares of elderly compared with the RLE15 method. Differences between methods proved especially strong within Europe when comparing Eastern European countries with the rest of Europe, and when comparing most Asian countries with non-Eastern European countries. In these comparisons, the RLE = 15 years clearly underestimates the differences (Supplemental Appendix Figure 2, Table 4) by not accommodating for the chance to reach RLE = 15 years. Within Europe, our method even resulted in more diversity in the shares of elderly compared with the use of 65 years as old-age threshold. This has important policy implications (see the section “Recommendations”).
Evaluation of Our Method
Within the general framework of the characteristics approach, we defined the prospective and comparative old-age threshold as the age at which the RLE multiplied by the ASR equals the value in a certain benchmark country in a given year.
The main argument for using the age at which RLE = 15 years and the ASR together is that (a) the RLE takes into account that older people are likely to be healthier if their life expectancy is higher (and, thus, that people are considered old at an older age) and (b) the ASR takes into account how common it is for people to reach a certain age (if many people survive to a certain age, that age is not considered old). One benefit of using CPOAT is that we only have to choose one benchmark country and the value for the RLE that we consider (RLE = 15 years in our case). The year and the value of the multiplication follow from that. Thus, if we choose Japan as benchmark country, the year in which RLE = 15 years at age 65 years is 1972. In that year, the ASR at age 65 years in Japan was 82.9. Thus our criterion for each country is the age at which ASR × RLE = 12.4 years. As both RLE and ASR decrease monotonously with age, for each country there is only one age at which ASR × RLE equals 12.4 years. This age is high in countries where survival in adult age is high (high value of ASR) and/or in countries in which health among elderly persons is good (high value of RLE).
Our new method is able to capture differences in the exceptionality of reaching “old” age between countries. However, an important feature of the RLE15 method is that it is also able to take into account life expectancy developments over time in a specific country (Sanderson & Scherbov, 2007). In fact, Sanderson and Scherbov (2007) showed, based on both analytical and empirical analyses, that the RLE15 method is rather insensitive to whether it is measured using period or cohort life tables. Our observation that the changes over time in old-age thresholds, and consequently shares of elderly, are almost similar over time for our new approach in comparison to the RLE15 method indicates that our measure is capable of accommodating for the improvements in mortality in different countries across time as well. Our new method, thus, not only maintains the feature of time-consistency of the RLE15 method but adds to it a way to better compare population aging among countries with diverse mortality patterns and improvements in ASRs.
In choosing our benchmark country, our starting point was that we want to examine changes in population aging over the last 40 years. As we wanted to compare our measure with (a) a constant threshold age of 65 years and (b) a threshold age based on the RLE = 15 years approach, we selected a country where the age at which RLE = 15 years was 65 years somewhere in the early 1970s. Another criterion was to select a country in which the value of ASR at that age was relatively high. The reason is that we wanted to select a country in which it was more common to reach that age compared with countries with a lower value of ASR. During the last 40 years, Japan has been a country with both high values of RLE and ASR. Another reason to select Japan as benchmark country was that in Japan, the ASR up to age 73 years in 2012 largely resembles the ASR up to age 65 years in 1972. As a result, the CPOAT is similar to the POAT in Japan in both 1972 and 2012.
We compared our new measure with the RLE15 method because the RLE15 method is the most popular application of the “characteristic approach” (Sanderson & Scherbov, 2013). Another meaningful comparison would be with an alternative character or indicator of aging proposed by Sanderson and Scherbov (2013): the life course ratio. The life course ratio refers to the ratio of person years lived above an equitable pension age x and beyond to the number of person years lived from the onset of working ages (15 or 20 years) onward. It is measured by dividing Tx with T15 or T20 as obtained from the life table. Additional analysis in which we performed such a comparison (using Tx / T15; Appendix B) revealed similar conclusions as when comparing our new method with the RLE15 method: Our method resulted in a higher share of elderly in 2012 in most Asian and Eastern European countries, and in more geographic diversity in the shares of elderly. This is not a surprising outcome, given that—similar to the RLE15 method—the life course ratio method only accounts for differences over time, but not for cross-national differences. Our new measure can better aid policy making as compared with the RLE15 method. Using the RLE15 method, aging will be diminished not only by improving the health of elderly (resulting in an increase in RLE15) but also by higher premature mortality. When less people reach the age at which RLE = 15 years, this will result in less older people and, consequently, less aging. Increasing premature mortality can, however, not be a goal of policy makers, of course. Using the CPOAT measure, aging will be diminished both by improving the health of elderly (increase in RLE) and by better survival to the age at which RLE = 15 years (increase in ASR). Which will be more in line of health policy.
Recommendations
We observed that the old-age threshold, calculated by means of our time-consistent and country-comparative measures, is lower than previously estimated in countries with low ASRs, for example, most Asian and Eastern European countries. As a result, the old-age dependency ratio is larger in these countries than was estimated using age 65 years as the old-age threshold or the RLE15 method. This observation has important implications for policy makers. These countries not only perform worse on health measures, but they also appear to have larger shares of elderly people than previously estimated, which warrants attention.
Our measure advances the previous methods in that it takes into account not only improvements over time in life expectancy at older ages but also differences between countries in the commonality of surviving until a particular RLE threshold. However, in addition to life expectancy and survival, also morbidity, productivity, skills, cognition, and labor force participation could influence what can be considered old across countries. We therefore recommend future research on aging measures to consider more distinct human capital features and differences therein between countries (Day & Dowrick, 2004; Engelhardt, Buber, Skirbekk, & Prskawetz, 2010; Skirbekk, 2004; Skirbekk et al., 2012).
Moreover, given the differences in life expectancy between various subgroups, such as sex, class, and regions (Crimmins et al., 2011; Jasilionis & Shkolnikov, 2016; Luy & Minagawa, 2014; Rieker & Bird, 2005), and the differences in human capital in general between different subgroups (Balachandran & James, 2019; Becker, 1985; Blau & Kahn, 2000; Bloom, Canning, & Malaney, 2000; Goujon & Samir, 2008; Prskawetz et al., 2006; Skirbekk, 2004), it might be worthwhile for these and future measures of aging to provide group-specific estimates. We believe that our measure also has implications for other social science disciplines like economics, sociology, and political science, and thus can be applied in these disciplines. For example, our measure can possibly substitute the current traditional measures of aging in many cross-country comparisons of macroeconomic models that study savings, expenditure, health care reforms, and fiscal burdens due to aging.
Supplemental Material
Supplemental material, Appendix-Figure-2-_a_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-2-_b_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-I-_a_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-I-_b_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-I-_c_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-III-_a_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-III-_b_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Appendix A
Table A1.
Comparison of the CPOAT With the POAT, for Selected Countries and the Different Regions, Asia and Europe, 1972, 1992, 2012.
| Country | 1972 | 1992 | 2012 | ||||||
|---|---|---|---|---|---|---|---|---|---|
| CPOAT | POAT | CPOAT – POAT | CPOAT | POAT | CPOAT – POAT | CPOAT | POAT | CPOAT – POAT | |
| Japan | 65.00 | 65.00 | 0.00 | 70.56 | 71.21 | −0.64 | 73.31 | 73.29 | 0.02 |
| China | 59.74 | 61.26 | −1.53 | 63.51 | 64.51 | −1.01 | 66.92 | 67.13 | −0.21 |
| Thailand | 59.85 | 63.80 | −3.95 | 65.39 | 67.34 | −1.95 | 67.76 | 70.01 | −2.25 |
| India | 56.22 | 58.28 | −2.06 | 59.28 | 61.39 | −2.11 | 63.05 | 64.90 | −1.86 |
| Azerbaijan | 62.95 | 64.96 | −2.00 | 63.48 | 65.39 | −1.92 | 64.93 | 65.81 | −0.88 |
| Uzbekistan | 62.79 | 65.26 | −2.47 | 62.77 | 65.49 | −2.72 | 63.51 | 65.59 | −2.08 |
| Norway | 66.40 | 67.30 | −0.89 | 68.47 | 69.24 | −0.77 | 71.22 | 70.97 | 0.25 |
| Netherlands | 65.78 | 67.03 | −1.25 | 68.40 | 69.21 | −0.81 | 70.90 | 70.73 | 0.16 |
| Spain | 65.55 | 66.39 | −0.84 | 69.33 | 70.22 | −0.90 | 72.13 | 71.96 | 0.17 |
| Ukraine | 64.16 | 66.39 | −2.24 | 62.40 | 64.51 | −2.10 | 62.86 | 65.02 | −2.16 |
| Asia | 58.72 | 60.77 | −2.05 | 63.35 | 64.18 | −0.82 | 65.93 | 67.27 | −1.34 |
| Eastern Asia | 61.47 | 62.57 | −1.10 | 65.10 | 65.59 | −0.50 | 67.99 | 68.75 | −0.76 |
| Southeastern Asia | 58.00 | 60.38 | −2.38 | 62.55 | 63.83 | −1.28 | 64.35 | 66.19 | −1.83 |
| Southern Asia | 56.66 | 58.78 | −2.12 | 59.78 | 61.53 | −1.75 | 63.24 | 65.19 | −1.95 |
| Western Asia | 59.93 | 62.68 | −2.75 | 63.03 | 65.32 | −2.29 | 65.94 | 67.35 | −1.41 |
| Central Asia | 62.57 | 65.47 | −2.89 | 62.43 | 65.31 | −2.88 | 63.11 | 64.91 | −1.80 |
| Non-Eastern Asia | 59.29 | 61.83 | −2.54 | 61.95 | 64.00 | −2.05 | 64.16 | 65.91 | −1.75 |
| Europe | 64.09 | 65.46 | −1.37 | 65.36 | 67.48 | −2.12 | 69.01 | 70.65 | −1.64 |
| Northern Europe | 65.00 | 66.04 | −1.03 | 67.37 | 68.42 | −1.05 | 71.22 | 70.97 | 0.25 |
| Western Europe | 65.00 | 65.79 | −0.79 | 68.53 | 69.35 | −0.83 | 72.02 | 72.69 | −0.67 |
| Southern Europe | 64.69 | 65.89 | −1.20 | 68.57 | 69.21 | −0.65 | 72.13 | 71.96 | 0.17 |
| Eastern Europe | 63.22 | 64.87 | −1.65 | 61.82 | 64.52 | −2.69 | 64.93 | 67.33 | −2.40 |
| Non-Eastern Europe | 64.90 | 65.90 | −1.01 | 68.15 | 69.00 | −0.84 | 71.79 | 71.87 | −0.08 |
Note. CPOAT = comparative prospective old-age threshold; POAT = prospective old-age threshold.
Table A2.
Comparison of the Share of Elderly Calculated With the New Measure, the RLE = 15 Years Measure, and Using 65 Years as Old-Age Threshold, for Selected Countries and the Different Regions, Asia and Europe, 1972, 1992, 2012.
| Country | With our method | With RLE15 method | With 65 years as old-age threshold | With our method | With RLE15 method | With 65 years as old-age threshold | With our method | With RLE15 method | With 65 years as old-age threshold |
|---|---|---|---|---|---|---|---|---|---|
| 1972 | 1992 | 2012 | |||||||
| Japan | 7.70 | 7.70 | 7.70 | 8.33 | 8.33 | 14.10 | 13.86 | 13.86 | 23.70 |
| China | 6.75 | 6.22 | 4.11 | 6.79 | 6.16 | 6.16 | 7.27 | 7.27 | 8.65 |
| Thailand | 5.44 | 3.95 | 3.58 | 5.40 | 4.49 | 5.40 | 7.38 | 6.22 | 9.44 |
| India | 7.98 | 6.84 | 3.49 | 7.04 | 5.95 | 4.03 | 6.31 | 5.29 | 5.29 |
| Azerbaijan | 6.02 | 5.11 | 5.11 | 6.71 | 5.39 | 5.39 | 5.77 | 5.50 | 5.77 |
| Uzbekistan | 6.57 | 5.68 | 5.68 | 5.36 | 4.10 | 4.49 | 4.98 | 4.30 | 4.62 |
| Norway | 12.74 | 11.80 | 13.67 | 12.39 | 12.39 | 15.77 | 9.71 | 9.71 | 15.40 |
| Netherlands | 9.91 | 9.16 | 10.66 | 10.91 | 10.11 | 13.31 | 10.19 | 10.19 | 16.24 |
| Spain | 9.50 | 9.50 | 10.25 | 11.09 | 10.11 | 15.03 | 11.04 | 11.04 | 17.36 |
| Ukraine | 11.58 | 9.72 | 10.56 | 16.31 | 13.46 | 13.46 | 17.26 | 15.23 | 15.23 |
| Asia | 7.05 | 6.45 | 4.01 | 6.42 | 5.86 | 5.30 | 6.47 | 5.93 | 7.03 |
| Eastern Asia | 6.63 | 5.54 | 4.44 | 6.87 | 6.34 | 6.87 | 7.69 | 7.03 | 9.66 |
| Southeastern Asia | 6.85 | 5.82 | 3.68 | 5.86 | 4.89 | 4.41 | 5.98 | 5.05 | 5.45 |
| Southern Asia | 7.31 | 6.18 | 3.45 | 6.38 | 5.45 | 4.06 | 6.00 | 4.97 | 4.97 |
| Western Asia | 6.80 | 5.38 | 4.44 | 5.08 | 4.60 | 4.60 | 4.70 | 4.35 | 5.05 |
| Central Asia | 6.53 | 5.53 | 5.53 | 6.56 | 5.13 | 5.13 | 5.77 | 4.95 | 4.95 |
| Non-Eastern Asia | 6.87 | 5.73 | 4.27 | 5.97 | 5.02 | 4.55 | 5.61 | 4.83 | 5.11 |
| Europe | 12.50 | 11.51 | 11.51 | 13.65 | 11.70 | 13.65 | 12.98 | 11.35 | 16.77 |
| Northern Europe | 13.63 | 12.65 | 13.63 | 13.40 | 12.49 | 15.21 | 10.88 | 10.88 | 16.51 |
| Western Europe | 13.92 | 12.92 | 13.92 | 12.02 | 11.07 | 14.86 | 11.53 | 10.58 | 18.37 |
| Southern Europe | 10.80 | 9.99 | 10.80 | 11.99 | 10.98 | 14.99 | 11.63 | 11.63 | 18.21 |
| Eastern Europe | 11.66 | 9.74 | 9.74 | 15.04 | 12.14 | 12.14 | 14.01 | 12.54 | 14.01 |
| Non-Eastern Europe | 12.78 | 11.85 | 12.78 | 12.47 | 11.51 | 15.02 | 10.88 | 10.88 | 16.51 |
Note. RLE = remaining life expectancy.
Appendix B
Comparison of Our New Measure With the Life Course Ratio Measure
Table B1.
Comparison of the CPOAT With the Old-Age Threshold Obtained With the LCROAT, 2012.
| Country | CPOAT | LCROAT | Difference (CPOAT – LCROAT) |
|---|---|---|---|
| Japan | 73.31 | 72.03 | 1.28 |
| China | 66.92 | 70.11 | −3.19 |
| Thailand | 67.76 | 70.72 | −2.96 |
| India | 63.05 | 70.01 | −6.96 |
| Azerbaijan | 64.93 | 66.21 | −1.28 |
| Uzbekistan | 63.51 | 65.42 | −1.91 |
| Norway | 71.22 | 69.72 | 1.5 |
| Netherlands | 70.90 | 75.71 | −4.81 |
| Spain | 72.13 | 71.26 | 0.87 |
| Ukraine | 62.86 | 68.07 | −5.21 |
| Asia | 65.93 | 70.51 | −4.58 |
| Eastern Asia | 67.99 | 70.35 | −2.36 |
| Southeastern Asia | 64.35 | 69.49 | −5.14 |
| Southern Asia | 63.24 | 70.50 | −7.26 |
| Western Asia | 65.94 | 69.19 | −3.25 |
| Central Asia | 63.11 | 65.53 | −2.42 |
| Non-Eastern Asia | 64.16 | 68.68 | −4.52 |
| Europe | 69.01 | 69.26 | −0.25 |
| Northern Europe | 71.22 | 70.14 | 1.08 |
| Western Europe | 72.02 | 71.97 | 0.05 |
| Southern Europe | 72.13 | 70.84 | 1.29 |
| Eastern Europe | 64.93 | 66.34 | −1.41 |
| Non-Eastern Europe | 71.79 | 69.82 | 1.97 |
Note. CPOAT = comparative prospective old-age threshold; LCROAT = life course ratio old-age threshold.
Table B2.
Comparison of the Share of Elderly Calculated With Our New Measure and Using LCR as Old-Age Threshold for Selected Countries and the Different Regions, Asia and Europe, 2012.
| Country | With our method | With LCR as old-age threshold |
|---|---|---|
| Japan | 13.86 | 16.12 |
| China | 7.27 | 5.52 |
| Thailand | 7.38 | 6.22 |
| India | 6.31 | 3.24 |
| Azerbaijan | 5.77 | 5.50 |
| Uzbekistan | 4.98 | 4.62 |
| Norway | 9.71 | 11.22 |
| Netherlands | 10.19 | 10.19 |
| Spain | 11.04 | 12.68 |
| Ukraine | 17.26 | 17.26 |
| Asia | 6.47 | 4.95 |
| Eastern Asia | 7.69 | 5.84 |
| Southeastern Asia | 5.98 | 4.76 |
| Southern Asia | 6.00 | 2.74 |
| Western Asia | 4.70 | 3.65 |
| Central Asia | 5.77 | 4.95 |
| Non-Eastern Asia | 5.61 | 4.03 |
| Europe | 12.98 | 11.35 |
| Northern Europe | 10.88 | 11.67 |
| Western Europe | 11.53 | 12.48 |
| Southern Europe | 11.63 | 13.45 |
| Eastern Europe | 14.01 | 13.28 |
| Non-Eastern Europe | 10.88 | 12.72 |
Note. LCR = life course ratio.
Table B3.
Comparison of the Range and Variation in the Share of Elderly for CPOAT and LCR as Old-Age Threshold, 2012.
| Maximum value | Country with maximum | Minimum value | Country with minimum | Range | Variance | |
|---|---|---|---|---|---|---|
| Asia | ||||||
| • CPOAT | 13.86 | Japan | 0.52 | United Arab Emirates | 13.34 | 7.08 |
| • LCR | 13.48 | Georgia | 0.40 | United Arab Emirates | 13.08 | 5.27 |
| Europe | ||||||
| • CPOAT | 18.56 | Latvia | 7.37 | Ireland | 11.19 | 7.53 |
| • LCR | 17.18 | Lithuania | 7.13 | Netherlands | 10.05 | 5.93 |
| Asia and Europe | ||||||
| • CPOAT | 18.56 | Latvia | 0.52 | United Arab Emirates | 18.04 | 20.05 |
| • LCR | 17.18 | Lithuania | 0.40 | United Arab Emirates | 16.78 | 18.56 |
Note. CPOAT = comparative prospective old-age threshold; LCR = life course ratio.
Footnotes
Author Contribution: All authors have contributed to the conception and design of the research and read and approved the final version of the manuscript. A.B. performed all analyses, with help from J.d.B. and F.J. A.B., J.d.B., and F.J. interpreted the results. A.B. and F.J. wrote the manuscript. J.d.B., J.K.S., and L.v.W. critically revised the manuscript.
Declaration of Conflicting Interests: The authors declared no potential conflicts of interest with respect to the research, authorship, and/or publication of this article.
Funding: The authors disclosed receipt of the following financial support for the research, authorship, and/or publication of this article: The first author would like to acknowledge funding from the Ubbo Emmius fund (alumnus and friends of the University of Groningen) and the Indian European research networking grant—aging and well-being in a globalizing world (NWO 465-11-009), funded by NWO-ESRC-ICSSR.
Supplemental Material: Supplemental material for this article is available online.
ORCID iD: Arun Balachandran  https://orcid.org/0000-0001-6856-7140
https://orcid.org/0000-0001-6856-7140
References
- Angus J., Reeve P. (2006). Ageism: A threat to “aging well” in the 21st century. Journal of Applied Gerontology, 25, 137-152. [Google Scholar]
- Balachandran A., James K. S. (2019). A multi-dimensional measure of population ageing accounting for Quantum and Quality in life years: An application of selected countries in Europe and Asia. SSM-Population Health, 7, 100330. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Becker G. S. (1985). Human capital, effort, and the sexual division of labor. Journal of Labor Economics, 3(1), S33-S58. [Google Scholar]
- Blau F. D., Kahn L. M. (2000). Gender differences in pay. Journal of Economic Perspectives, 14(4), 75-100. [Google Scholar]
- Bloom D. E., Canning D., Malaney P. N. (2000). Population dynamics and economic growth in Asia. Population and Development Review, 26, 257-290. [Google Scholar]
- Christensen K., Doblhammer G., Rau R., Vaupel J. W. (2009). Ageing populations: The challenges ahead. The Lancet, 374, 1196-1208. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Chu C. Y. (1997). Age-distribution dynamics and aging indexes. Demography, 34, 551-563. [PubMed] [Google Scholar]
- Crimmins E. M., Kim J. K., Solé-Auró A. (2011). Gender differences in health: Results from SHARE, ELSA and HRS. European Journal of Public Health, 21, 81-91. [DOI] [PMC free article] [PubMed] [Google Scholar]
- d’Albis H., Collard F. (2013). Age groups and the measure of population aging. Demographic Research, 29, 617-640. [Google Scholar]
- Day C., Dowrick S. (2004). Ageing economics: Human capital, productivity and fertility. Agenda, 11, 3-20. [Google Scholar]
- de Beer J. A. A. (2012). Smoothing and projecting age-specific probabilities of death by TOPALS. Demographic Research, 27, 543-592. [Google Scholar]
- Dowd J. J., Bengtson V. L. (1978). Aging in minority populations: An examination of the double jeopardy hypothesis. Journal of Gerontology, 33, 427-436. [DOI] [PubMed] [Google Scholar]
- Engelhardt H., Buber I., Skirbekk V., Prskawetz A. (2010). Social involvement, behavioural risks and cognitive functioning among older people. Ageing & Society, 30, 779-809. [Google Scholar]
- Giles H., Reid S. A. (2005). Ageism across the lifespan: Towards a self-categorization model of ageing. Journal of Social Issues, 61, 389-404. [Google Scholar]
- Goujon A., Samir K. C. (2008). The past and future of human capital in South-East Asia: From 1970 to 2030. Asian Population Studies, 4, 31-56. [Google Scholar]
- Human Mortality Database. (2015). University of California, Berkeley, and Max Planck Institute for Demographic Research. Available from www.mortality.org
- Jasilionis D., Shkolnikov V. M. (2016). Longevity and education: A demographic perspective. Gerontology, 62, 253-262. [DOI] [PubMed] [Google Scholar]
- Kot S., Kurkiewicz J. (2004). The new measures of the population aging. Studia Demograficzne, 146(2), 17-29. [Google Scholar]
- Lee R., Goldstein J. R. (2003). Rescaling the life cycle: Longevity and proportionality. Population and Development Review, 29, 183-207. [Google Scholar]
- Leon D. A. (2011). Trends in European life expectancy: A salutary view. International Journal of Epidemiology, 40, 271-277. [DOI] [PubMed] [Google Scholar]
- Lutz W., Sanderson W. C., Scherbov S. (2008. a). The coming acceleration of global population ageing. Nature, 451, 716-719. [DOI] [PubMed] [Google Scholar]
- Lutz W., Sanderson W. C., Scherbov S. (2008. b). Global and regional population ageing: How certain are we of its dimensions? Journal of Population Ageing, 1, 75-97. [Google Scholar]
- Luy M., Minagawa Y. (2014). Gender gaps—Life expectancy and proportion of life in poor health. Health Reports, 25(12), 12-19. [PubMed] [Google Scholar]
- McKee M., Shkolnikov V. (2001). Understanding the toll of premature death among men in Eastern Europe. British Medical Journal, 323, 1051-1055. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Murray C. J., Yang G., Qiao X. (1992). Adult mortality: Levels, patterns and causes. In Feachem R. G. (Ed.), The health of adults in the developing world (pp. 23-111). New York, NY: Oxford University Press. [Google Scholar]
- Prskawetz A., Mahlberg B., Skirbekk V., Freund I., Winkler-Dworak M., Lindh T., . . . Andersson F. (2006). The impact of population ageing on innovation and productivity growth in Europe. In Prskawetz A., Lindh T. (Eds.). Vienna: Vienna Institute of Demography. [Google Scholar]
- QGIS Development Team. (2016). QGIS Geographic Information System. Open Source Geospatial Foundation. Available from http://qgis.osgeo.org
- Rieker P. P., Bird C. E. (2005). Rethinking gender differences in health: Why we need to integrate social and biological perspectives. The Journals of Gerontology, Series B: Psychological Sciences & Social Sciences, 60B(II), 40-47. [DOI] [PubMed] [Google Scholar]
- Ryder N. B. (1975). Notes on stationary populations. Population Index, 41, 3-28. [Google Scholar]
- Sanderson W. C., Scherbov S. (2005). Average remaining lifetimes can increase as human populations age. Nature, 435, 811-813. [DOI] [PubMed] [Google Scholar]
- Sanderson W. C., Scherbov S. (2007). A new perspective on population aging. Demographic Research, 16(2), 27-58. [Google Scholar]
- Sanderson W. C., Scherbov S. (2008). Rethinking age and aging. Population Bulletin, 63(4), 1-16. [Google Scholar]
- Sanderson W. C., Scherbov S. (2010). Demography. Remeasuring aging. Science, 329, 1287-1288. [DOI] [PubMed] [Google Scholar]
- Sanderson W. C., Scherbov S. (2013). The characteristics approach to the measurement of population aging. Population and Development Review, 39, 673-685. [Google Scholar]
- Sanderson W. C., Scherbov S. (2015. a). Are we overly dependent on conventional dependency ratios? Population and Development Review, 41, 687-708. [Google Scholar]
- Sanderson W. C., Scherbov S. (2015. b). An easily understood and intergenerationally equitable normal pension age. In Marin B. (Ed.), The future of welfare in a global Europe (pp. 193-220). Farnham, UK: Ashgate. [Google Scholar]
- Sanderson W. C., Scherbov S. (2015. c). Faster increases in human life expectancy could lead to slower population aging. PLoS ONE, 10(4), e0121922. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Sanderson W. C., Scherbov S., Weber D., Bordone V. (2016). Combined measures of upper and lower body strength and subgroup differences in subsequent survival among the older population of England. Journal of aging and health, 28(7), 1178-1193. [DOI] [PubMed] [Google Scholar]
- Scherbov S., Sanderson W. C. (2016). New approaches to the conceptualization and measurement of age and aging. Journal of Aging and Health, 28, 1159-1177. [DOI] [PubMed] [Google Scholar]
- Shryock H. S. S., Stockwell J. S., Edward G. (1976). Methods and materials of demography (Shryock H. S., Siegel J. S., and associates, Eds.). New York, NY: Academic Press. [Google Scholar]
- Skirbekk V. (2004). Age and individual productivity: A literature survey. In Skirbekk V. (Ed.), Vienna yearbook of population research (pp. 133-153). Vienna. [Google Scholar]
- Skirbekk V., Loichinger E., Weber D. (2012). Variation in cognitive functioning as a refined approach to comparing aging across countries. Proceedings of the National Academy of Sciences of the United States of America, 109, 770-774. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Spijker J. (2015, April). Alternative indicators of population ageing: An inventory (Working Papers No. 4/2015). Vienna, Austria: Vienna Institute of Demography. [Google Scholar]
- Spijker J., MacInnes J. (2013). Population ageing: The timebomb that isn’t? British Medical Journal, 347, Article f6598. [DOI] [PubMed] [Google Scholar]
- United Nations. (2015). World population prospects: The 2015 revision 2015. New York, NY: Department of Economic and Social Affairs, United Nations. [Google Scholar]
- United Nations. (2016). Concise report on strengthening demographic evidence base for the post-2015 development agenda. New York, NY: Department of Economic and Social Affairs, United Nations. [Google Scholar]
- United Nations. (2018). Methodology: Standard country or area codes for statistical use (M49). Statistics Division, United Nations. Retrieved from https://unstats.un.org/unsd/methodology/m49/
- Vallin J., Meslé F. (2004). Convergences and divergences in mortality: A new approach of health transition. Demographic Research, Special Collection 2(2), 11-44. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Supplemental material, Appendix-Figure-2-_a_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-2-_b_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-I-_a_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-I-_b_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-I-_c_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-III-_a_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health
Supplemental material, Appendix-Figure-III-_b_ for Comparison of Population Aging in Europe and Asia Using a Time-Consistent and Comparative Aging Measure by Arun Balachandran, Joop de Beer, K. S. James, Leo van Wissen and Fanny Janssen in Journal of Aging and Health