Mammalian Skull Dimensions and the Golden Ratio (Φ)

Abstract

The Golden Ratio (Phi, or Φ = 1.618…) is a potentially unifying quantity of structure and function in nature, as best observed in phyllotactic patterns in plants. For centuries, Φ has been identified in human anatomy, and in recent decades, Φ has been identified in human physiology as well. The anatomy and evolution of the human skull have been the focus of intense study. Evolving over millenia, the human skull embodies an elegant harmonization of structure and function. The authors explored the dimensions of the neurocranium by focusing on the midline calvarial perimeter between the nasion and inion (nasioiniac arc) and its partition by bregma into 2 sub-arcs. The authors studied 100 human skulls and 70 skulls of 6 other mammalian species and calculated 2 ratios: 1) the nasioiniac arc divided by the parieto-occipital arc (between bregma and inion), and 2) the parieto-occipital arc divided by the frontal arc (between nasion and bregma). The authors report that in humans these 2 ratios coincide (1.64 ± 0.04 and 1.57 ± 0.10) and approximate Φ. In the other 6 mammalian species, these 2 ratios were not only different, but also unique to each species. The difference between the ratios showed a trend toward convergence on Φ correlating with species complexity. The partition of the nasioiniac arc by bregma into 2 unequal arcs is a situation analogous to that of the geometrical division of a line into Φ. The authors hypothesize that the Golden Ratio (Φ) principle, documented in other biological systems, may be present in the architecture and evolution of the human skull.

The Golden Ratio, represented by the Greek upper-case letter phi (Φ), belongs to the set of irrational numbers and expands in a decimal form as 1.618033…. Irrational numbers cannot be expressed as either whole numbers or fractions, do not terminate (i.e., are “infinite”), and do not have any repeating numerical sequence in their decimal expansion. Over centuries, several investigators have demonstrated the presence of Φ in a wide variety of mathematical, biological, and natural systems.^1–4 Geometrically and historically, Φ traditionally has been derived by dividing a line into 2 unequal segments such that the ratio of the length of the entire line to that of the longer segment is identical to the ratio of the length of the longer segment to that of the shorter segment (Fig. 1). In mathematical terms, this means that division of a line measuring 1.0 (1 unit) into the Golden Ratio is accomplished when the shorter segment is approximately 0.382 (or 38.2%) of the entire line and the longer segment is approximately 0.618 (or 61.8%) so that 1.0/0.618 = 0.618/0.382 = 1.618.

FIGURE 1.

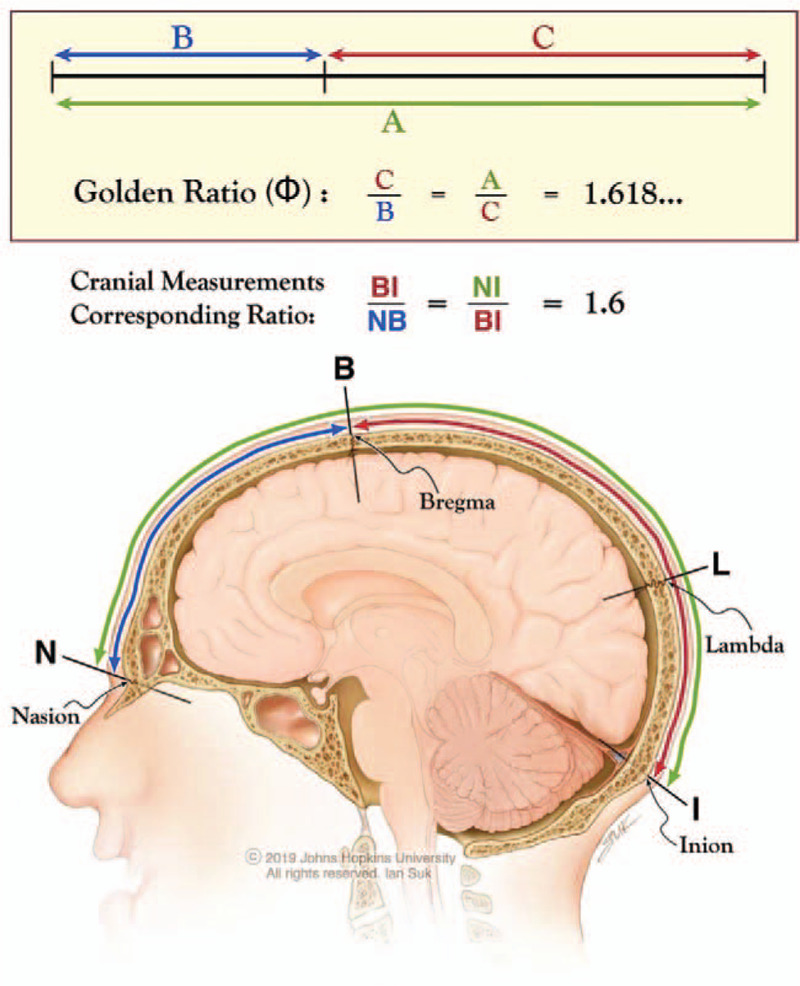
Golden Ratio (Φ) in the partition of a line and also of the nasioiniac arc on the human skull. Division of a line into 2 segments such that the ratio of the line (A) to the longer segment (C) is identical to the ratio of the longer to the shorter segment (B). This ratio is 1.618…, known as the Golden Ratio or Φ. In an analogous situation in human skulls, division of the nasioiniac arc (from nasion to inion, NI) by bregma into a shorter frontal arc (from nasion to bregma, NB) and longer parieto-occipital arc (from bregma to inion BI), creates a geometrical relationship in which the ratio of the nasioiniac arc over the bregma-inion arc (NI/BI) coincides with the ratio of the bregma-inion arc over the nasion-bregma arc (BI/NB), both 1.6. The subdivision of the nasioiniac arc by bregma into 2 unequal arcs emulates the geometrical division of a line into the Golden Ratio.

The Golden Ratio characterizes a unique relationship in the study of integers, a branch of mathematics called Number Theory. Numerically, Φ can be calculated as a ratio within the Fibonacci series as the series approaches infinity. The Fibonacci series is a well-known, infinite series in which the next term in the series is the sum of the prior 2 terms. The Fibonacci series starts as 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55… Initially, the ratios of consecutive terms in the Fibonacci series (F_n / F_n-1) fluctuate between 1 and 2, but rapidly converge on the value Φ as the series grows: 1/1 = 1, 2/1 = 2, 3/2 = 1.5, 5/3 = 1.667, 8/5 = 1.6, 13/8 = 1.625, 21/34 = 1.615, 34/21 = 1.619, 55/34 = 1.618, …

The division of the 40th Fibonacci term (102,334,155) by the 39th (63,245,986) matches Φ to 15 decimal places. For convenience, authors and mathematicians typically truncate Φ to 1.618. Interestingly, the reciprocal of Φ (1/Φ or 1/1.61803…) is 1 integer less than Φ (0.61803…) and has the same decimal extension as Φ, and the square of Φ (Φ² or 1.61803…²) is 1 integer more than Φ (2.61803…) and also has the same decimal extension. Φ is the only number that has these 2 properties. By convention, the reciprocal of Φ is represented by the Greek lower-case letter phi (ϕ), although sometimes this convention is reversed.⁴

Since its inception, Φ has been found in a variety of mathematical systems and has been used in architectural designs and artistic masterpieces.^1–3 Beyond its mathematical and aesthetic appeal, Φ has been recognized as a potentially unifying quantity in physics, biology, and human physiology. Studies in the physical and biological sciences have revealed the presence of Φ in atomic physics,^5,6 quantum phase transitions,⁷ nucleotide frequencies in the human genome,⁸ cell shape, growth, and arrangements,⁹ and in phyllotactic patterns in plants.^10,11 In the clinical sciences, Φ has been found to underlie cardiac anatomy and physiology,^12–18 gait mechanics,^19,20 and the aesthetic dimensions of the face.^4,21–26 It has been postulated that Φ emerges repeatedly in the analysis of such diverse natural systems because it somehow reflects the optimization of structure and function, an essential principle in nature.

The shape and evolution of the human skull have attracted intense study in several disciplines.²⁷ The vertebrate skull has 3 major functions: food intake, respiratory flow, and protection of the brain and 4 of the 5 senses (sight, hearing, olfaction, and taste).²⁸ Evolution of the skull has been a design challenge in which several iterations have eventually harmonized its structure and functions. The neurocranium is the portion of the skull that supports and protects the brain and the 4 sensory organs, and it has a dome called the calvarium. The viscerocranium is the other component of the skull, consisting of the facial and jaw bones. The human calvarium, in particular, has been of interest in anthropology and the neurosciences because of its intimate relation to the brain. Evolutionarily, the shape of the calvarium has been determined in humans primarily by an expanding and increasingly complex brain, since increasing biological and social complexities have resulted in a disproportionally large cerebrum in comparison to the brainstem and cerebellum.^29–31 Increasing physiological and behavioral complexity in higher mammals has been reflected in frontal lobes that are proportionally larger than those of lower species. In humans and the great apes, the cerebrum is characterized by relatively large frontal lobes, which regulate voluntary movements, higher cognitive functions, motor aspects of vocalization, and complex emotions generated by the limbic system. The relatively large frontal lobes have resulted in expansion of the frontal region of the calvarium.²⁷

The “nasioiniac arc” is the midline, curvilinear path on the calvarium between the nasion (at the boundary between the nasal and frontal bones, the frontonasal suture) and the inion (the external occipital protuberance). It corresponds to important underlying neural structures in humans and other animals. In the lower mammalian order Rodentia (e.g., rats and mice), the nasioiniac arc overlies the large olfactory bulbs, the primitive cerebrum, and the cerebellum. By contrast, expansion of the neocortex in humans has resulted in the nasioiniac arc overlying only the cerebrum within the supratentorial compartment. In 1900, Taylor and Haughton showed that cranial sutures and craniometric points reliably demarcate anatomical boundaries of brain structures.³² In humans, the 2 distinct demarcation points along the nasioiniac arc are bregma and lambda. Bregma is the junction of the coronal and sagittal sutures, and lambda is the junction of the lambdoid and sagittal sutures. Bregma subdivides the nasioiniac arc into a “frontal arc” (the surface distance between the nasion and bregma) and a “parieto-occipital arc” (the surface distance between bregma and the inion). Although bregma does not represent the exact anatomic demarcation between the frontal and parietal lobes in humans (the central sulcus or Rolandic fissure), the frontal arc can be used as a correlate for the underlying frontal lobe and the parieto-occipital arc similarly can be used as a correlate for the underlying parietal and occipital lobes.

We studied the dimensions of the calvarium in several mammals by focusing on the nasioiniac arc, assuming that increasing complexity of the brain and expanding size of the cerebrum might be reflected externally in this measurement. Specifically, we studied the ratios between the nasion-bregma (NB), the bregma-inion (BI), and the nasioiniac arcs (NI) in 100 adult human skulls and 70 skulls of 6 other mammalian species. We report that in humans the ratio of the nasioiniac arc over the parieto-occipital arc as well as that of the parieto-occipital arc over the frontal arc coincide at 1.6, which is surprisingly close to the Φ value of 1.618. In addition, we report that humans have the smallest difference between the 2 measured cranial ratios of the 7 species surveyed, which may be an indicator of evolutionary sophistication. The subdivision of the nasioiniac arc by bregma into 2 unequal arcs is analogous to the geometrical division of a line into the Golden Ratio. We hypothesize that the Golden Ratio principle, which has been documented in other biological and natural systems, may be present in the architecture and evolution of the human skull as well.

METHODS

Anthropometric cranial measurements were obtained in 100 normal adults (age range 18–97 years, mean age 52 years; male 47%) from a retrospective review of 3-dimensional (3D) computerized axial tomography (CAT) scans acquired for clinical purposes. These CAT scans have been repeatedly confirmed as having sub-millimetric accuracy in their representation of the imaged skulls without distortion of the anatomy. These images are routinely used for stereotactic neurosurgical procedures in which sub-millimetric accuracy is paramount. Therefore, measuring calvarial arcs on these images is essentially equivalent to measuring actual skulls. The Johns Hopkins Medicine Institutional Review Board approved completion of this study without patient informed consent because all radiographic data had been acquired previously for clinical purposes and was de-identified for this study. The 100 normal specimens were selected after excluding adults with a prior history or radiographic evidence of intracranial masses or conditions potentially affecting skull size (hydrocephalus, craniosynostosis, skeletal or growth abnormalities) were excluded. Indications for radiographic evaluation included trauma (36%), altered mental status or loss of consciousness (33%), headache (18%), change in neurologic exam (18%), and seizures (6%). Regardless of the indications, head CAT scans revealing abnormal findings (cerebral infarct, subdural hemorrhage, etc) or calvarial fractures that could alter contour measurements were excluded. Imaging criteria for the human skulls included high resolution (axial slice thickness ≤1 mm), 3D-reconstructed head CAT scans. Radiographic images generated on UltraVisual Advanced Visualization software were rotated to view lateral skull projections (right or left). Selected images allowed visualization of anatomic landmarks along the mid-sagittal plane and acquisition of cranial measurements.

Using standard terminology,³³ the nasion was defined as the midline point where the 2 nasal bones and the frontal bone intersect along the frontonasal suture. In humans, it is located at the depression immediately above the bridge of the nose and below the glabella. Bregma was defined as the midline point where the coronal and sagittal sutures intersect. The inion was defined as the midline point at the base of the external occipital protuberance. The curvilinear measurements taken along the surface of the skull between the points defined above are referred to as “arcs.”³³ Curvilinear measurements were obtained using UltraVisual Advanced Visualization software and Synedra View Personal 3. Ratios of cranial measurements were computed for all study subjects and analyzed using descriptive statistics. The distance between the nasion and inion is labeled the NI (nasion-inion or nasioiniac arc), between the nasion and bregma the NB (nasion-bregma arc), and between bregma and the inion the BI (bregma-inion arc).

Similar cranial measurements were performed in 5 adult higher mammalian species (3 from the order Carnivora and 2 from the order Primates) and 1 lower mammalian species (from the order Lagomorpha) by direct examination of skulls from the collection at The Smithsonian Institution National Museum of Natural History in Washington, D.C., which granted us access to their collection of adult mammalian specimens for calvarial measurements. The 6 species surveyed were: tigers (Panthera tigris), lions (Panthera leo), Blue monkeys (Cercopithecus mitis), Rhesus monkeys (Macaca mulatta), domestic dogs (Canis lupus familiaris), and the Eastern cottontail rabbit (Sylvilagus floridanus). Smithsonian male and female specimens included skulls collected over the past 200 years from national and international locations. We excluded animals with horns because these structures altered the cranial contours. Specimen availability determined gender distribution within each species studied (Blue monkey: 54% male; Rhesus monkey: 20% male). However, incomplete gender and age information for several specimens precluded statistical analysis of these demographic data. Adequate visualization and/or palpation of the fronto-nasal, coronal, and lambdoid sutures and external occipital protuberance determined selection of appropriate specimens. We included species for which we had at least 10 specimens. Consultation of references describing the craniofacial skeleton of different monkeys aided confirmation of skull surface landmarks in primate specimens.^34,35 Regarding Rhesus monkeys; we identified the inion as the external occipital protuberance below a natural prominence inferior to the lambdoid suture. In agreement with adult human skulls, this landmark for the inion overlies the midline confluence of venous sinuses. In the lower mammals, lambda roughly demarcates the boundary between the cerebrum and the cerebellum and therefore the posterior aspect of the nasioiniac arc overlies the cerebellar surface and not the surface of the cerebrum. Cranial measurements on all mammalian skull specimens were performed using 2 surgical clamps bounding either silk sutures or tape measure. Ratios of skull dimensions, similar to those described for anthropometric cranial measurements, were computed and analyzed using descriptive statistics.

RESULTS

Among the 7 species surveyed, only humans had NI/BI and BI/NB ratios that coincided and thus conformed to the Golden Ratio parameters. In humans, the ratio of the nasioiniac arc over the parieto-occipital arc (NI/BI = 1.64 ± 0.04) and the ratio of the parieto-occipital arc over the frontal arc (BI/NB = 1.57 ± 0.10) are essentially identical and closely approximate Φ (1.618) within 1 standard deviation. This finding suggests that bregma, a special point along the nasioiniac arc, divides this arc into 2 unequal segments such that the ratio of the length of the entire arc to that of the longer segment matches the ratio of the arc of the longer segment to that of the shorter segment. By contrast, similar measurements of lambda (another craniometric point on the nasioiniac line that does not correlate with any important brain landmarks in humans) results in a partition of the nasioiniac arc into 0.77/0.23 (3.3) and 1.0/0.77 (1.3) ratios.

In the 5 other higher mammalian species in which the nasioiniac arc spans the contour of the cerebrum, mean NI/BI ratios were higher and mean BI/NI ratios were lower than the corresponding proportions in humans (Table 1). In the Easter cottontail rabbit, a lower mammalian species in which the posterior aspect of the cerebrum is demarcated by lambda and the posterior segment of the nasioiniac arc (from lambda to inion) overlies the cerebellum, the NI/BI and BI/NB ratios were also higher and lower, respectively than the corresponding proportions in humans (Table 1).

TABLE 1.

Craniometric Ratios

Genus Species	Common Name	n	NI/BI (Mean ± SD)	BI/NB (Mean ± SD)
Homo sapiens	Human	100	1.64 ± 0.04	1.57 ± 0.10
Panthera leo	Lion	11	1.74 ± 0.07	1.36 ± 0.14
Panthera tigris	Tiger	13	1.77 ± 0.09	1.32 ± 0.17
Macaca mulatta	Rhesus monkey	10	1.86 ± 0.12	1.18 ± 0.15
Canis familiaris	Domestic dog	11	1.91 ± 0.10	1.12 ± 0.13
Cercopithecus mitis	Blue monkey	13	1.95 ± 0.11	1.06 ± 0.12
Sylvilagus floridanus	Eastern cottontail rabbit	12	2.25 ± 0.10	0.81 ± 0.07

BI, bregma-inion arc; n, number in sample; NB, nasion-bregma arc; NI, nasion-inion arc; SD, standard deviation.

Most importantly, we found that the difference between the NI/BI and BI/NB ratios became smaller proceeding from the less complex mammal in this study, that is, the Eastern cottontail rabbit, which has a ratio difference of 1.44, through the 2 monkeys and dogs, which have intermediate ratio differences of 0.89–0.68, to the tigers and lions, which have ratio differences of 0.45 and 0.38, respectively. Humans lie at the end of this spectrum with a ratio difference of 0.07 (Fig. 2). Thus, in this limited sampling of mammalian skulls, we observed a progression toward the Golden Ratio in the partition of the nasioiniac arc by bregma.

FIGURE 2.

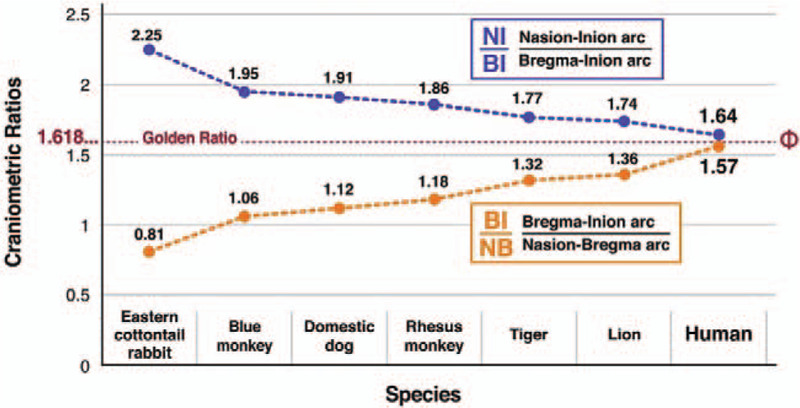
Craniometric ratios of the 7 sampled mammalian species, including humans. The NI/BI and BI/NB ratios of the 7 mammalian species sampled were different in all cases except humans. Subtraction of the lower BI/NB ratio from higher NI/BI ratio reveals a convergence toward the Golden Ratio (in which the result of the subtraction is 0) which regresses from the less complex mammal, i.e. the Eastern cottontail rabbit, which has a ratio difference of 1.44, through 2 monkeys and dogs, which have intermediate ratios of 0.89–0.68, to tigers and lions, which have ratio differences of 0.45 and 0.38, respectively. Humans lie at the end of this spectrum with a ratio difference of 0.07. Thus, in this limited sampling of mammalian skulls, we observed a progression toward the Golden Ratio in the partition of the nasioiniac arc by bregma.

DISCUSSION

The history of Φ is colorful and fascinating.^2,4 Pythagoras of Samos (ca. 570—ca. 495 B.C.E.) was a philosopher, mathematician, and numerologist who inspired a large number of followers. One of his most prominent disciples was Hippasus of Metapontum, an ancient city in southern Italy. Hippasus was a Pythagorean who lived in the 5th century B.C.E. and is credited by Livio as probably having discovered irrational numbers in general (which cannot be expressed as either a whole number or a fraction) and specifically the Golden Ratio. If Hippasus was not the actual discoverer of Φ, it is likely that another Pythagorean disciple or disciples did. It is claimed by some art historians that the Greek sculptor Phidias (ca. 490—ca. 430 B.C.E.), remembered for his lost sculptures of “Zeus” at Olympia and “Athena” in the Parthenon, used the Golden Ratio to proportion his statues.

The first written description and illustration of how to obtain the Golden Ratio geometrically came from Euclid of Alexandria (ca. 325 B.C.E. - ?). ^2,4 Euclid, known as the “Father of Geometry,” published around 300 B.C.E. his book Stoicheia (“Elements”), in which he provided the following description:

“A straight line is said to have been cut in extreme and mean ratio when, as the whole line is to the greater segment, so is the greater to the less.” (Book VI, Definition 3)³⁶

Eighteen centuries after Euclid, in 1509, Fra Luca Bartolomeo de Pacioli (ca. 1447–1517), a Franciscan friar and mathematician born in the Tuscan town of Sansepolcro, dedicated an entire book to the Golden ratio and titled it De Divina Proportione (“The Divine Proportion”). Leonardo daVinci (1452–1519), a friend and mathematics pupil of Pacioli, illustrated De Divina Proportione. Da Vinci used the Golden Ratio in paintings during his career, starting as early as 1472 to 1475 when he painted Annunciation. Painters and sculptors throughout history have used the Golden Ratio to enhance the beauty of their works.⁴

Almost 9 decades after Pacioli's book, in 1597, Michael Mästlin (1550–1631), a German astronomer and mathematician best known as Johannes Kepler's (1571–1630) mentor, calculated the reciprocal of the Golden Ratio (1/Φ) and included this number in a letter to Kepler in 1597 as “about 0.6180340” (actual expansion is 0.61803398…).⁴ According to Livio, the term “Golden Section” was introduced in 1935 by Martin Ohm (1792–1872), A German mathematician who was the younger brother of the physicist Georg Ohm, in the second edition of Die Reine Elementar-Mathematik (“The Pure Elementary Mathematics”).²

An interesting method for calculating Φ involves its derivation from the Fibonacci series.^2,4 Leonardo Fibonacci (ca. 1170—ca. 1250) was an Italian mathematician born in Pisa who in 1202 published the Liber Abaci (“Book of the Abacus”). In Chapter XII of this book, Fibonacci presented a problem concerning breeding pairs of rabbits, which gave rise to the now-famous Fibonacci series. The Fibonacci series is a well-known infinite series in which the next number in the series is the sum of the prior two numbers, which is represented mathematically as F_n = F_n-1 + F_n-2 (0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55…). In a letter written in 1608, Kepler described that the ratio of consecutive Fibonacci numbers converges on Φ; the division of the 17th Fibonacci number (987) by the 16th (610) number equals 1.618033 (rounded). Finally, Mark Barr (1871–1950), an American mathematician, engineer, and inventor, is credited with labelling the Golden ratio as Φ, because it is the first Greek letter in Phidias’ name, the Greek sculptor reported by some art historians to have used Φ in creating his statues. Alternatively, other authors state that it is based phonetically on the first 2 letters of “Fibonacci.”

In a wide variety and increasing number of biological systems, Φ has emerged as an underpinning of optimized structure or function. The reason for this remains unclear, but this ratio somehow relates to structural or functional efficiency. The Golden Ratio has been studied most thoroughly in phyllotaxy, the arrangement of leaves along a branch or branches along a trunk in plants. In soft plants and trees, the spiral arrangement of branches or leaves maximizes the exposure of each leaf to sunlight and water. These spiral arrangements—which can also be identified in the scales of pinecones, the seeds of sunflowers, the hexagonal scales on pineapples, and the arrangements of petals on roses—follow mathematical principles based on the ratios of terms in the Fibonacci series and on angles determined by Φ. For instance, Bravais and Bravais in 1837³⁷ and Church in 1904³⁸ described that new leaves emerge from a stem in a spiral pattern in which the angles of the lines emerging from the stem along the axes of the leaves form an angle of 137.5 degrees, representing the “Golden Angle.” When the 360 degrees of either a circle or a complete spiral are divided by Φ, the resulting angles are 222.5 and 137.5 degrees. The angle of 137.5° is known as the Golden Angle.² Several other spiral structures in nature, such as the shell of the marine mollusk nautilus, the horns of rams, the tusks of elephants, and the shape of spiral galaxies (such as the Milky Way), exhibit the structure of a “logarithmic” or “equiangular” spiral. The logarithmic spiral has the unique property that it does not alter its shape as it grows and that the distance between its coils grows proportionally as the spiral expands. It is also called the equiangular spiral because any line drawn from the center or pole of the spiral to any point of the spiral generates the same angle. ² The logarithmic spiral is a Φ-derived structure that can be constructed using “nested” Golden Rectangles or Golden Triangles.⁴

In recent years several investigators have identified the Φ in human cardiac physiology and anatomy, gait mechanics, and the structure of erythrocytes. In 2011, researchers at the Heart Center in Umea University, Sweden, reported that the vertical and transverse dimensions of normal left ventricles, and left ventricular inlet length and width (mitral annulus dimensions) conform to Φ. They showed that in pathological conditions these ratios depart from Φ.¹² They also showed that the anatomical angle between the right ventricular outflow tract axis and the continuation of the inflow tract axis is 138° (similar to the Golden Angle, 137.5°) and that this angle increases in the presence of right heart dilated cardiomyopathy, and mitral regurgitation.¹² Similar Φ ratios have been reported for the dimensions of the left ventricle to the aortic root.¹⁵ In 2013, researchers at Trakya University in Erdine, Turkey, reported that the diastolic to systolic time interval ratio is 1.611.¹⁴ In 2011, researchers at the Imperial College in London, United Kingdom, reported that the branching structure of the coronary arterial tree follows the Fibonacci sequence of 2, 3, 5, 8, and 13 branches from base to apex of the heart.¹⁶ In 2019, researchers at the National and Kapodistrian University of Athens, Greece, described in a study of 31,622 individuals that deviation from Φ in systolic and diastolic pressure ratios was associated with a higher mortality regardless of other risk factors.³⁹ In a different field, in 2016 researchers at the Sapienza University in Rome studying anthropometric gait and motion, concluded that humans probably evolved body and lower extremity dimensions that allowed for gait harmonics based on Φ.^19,20 In 2018 researchers at the University of Memphis in Tennessee proposed that Φ correlates with the optimal toroidal shape and CO₂-carrying capacity of erythrocytes.⁹

The nasioiniac arc is of particular interest because it spans not only the calvarium but almost the entire skull. The nasioiniac line has changed primarily as a result of changes in the underlying neuraxis. It is, therefore, a simple curvilinear measurement that incorporates and mirrors the complexity of the neurocranium. It demarcates the anterior and posterior boundaries of the cerebrum in higher mammals and of the entire cranial neuraxis in lower mammals. The frontal arc (from the nasion to bregma) is also of interest because in humans this dimension has expanded as the frontal lobes have grown, providing humans with a characteristic forehead (or sinciput), which is absent in other mammals and even in other hominins. In lower mammals such as the Eastern Cottontail rabbit and the rat, the nasioiniac arc spans from the olfactory bulbs anteriorly to the cerebellum posteriorly, which in turn overlies the cervicomedullary junction. In higher mammals, the cerebrum has expanded to overlie the diencephalon, cerebellum, and brainstem. The frontal lobes, in particular, occupy a large proportion of the cerebrum in primates.⁴⁰ Although it was originally thought that humans had disproportionally large frontal lobes as compared to other primates, recent research suggests that the human frontal cortex is proportionally similar to that of the great apes (gorillas, orangutans, bonobos, and chimpanzees).⁴¹ Similarly, the frontal arc has increased with increasing neural complexity and expansion of the frontal lobes.

As in other biological system in which Φ has been identified, it is unclear why it emerges in the human skull and not in those of lower mammals. It is thought that Φ may emerge when 2 interrelated structures or functions co-evolve such that expansion of one occurs at the expense of the other. A compromise, therefore, must be reached for structural and functional stability. In linear structures, such as lines, spirals, and circles this is accomplished when the shorter segment or smaller component is approximately 0.382 (or 38.2% of the entire structure) and the longer segment or component is approximately 0.618 (or 61.8%) so that 1.0/0.618 = 0.618/0.382 = 1.618. In nature, perfect symmetry rarely emerges as a solution to optimal structure and function, and the alternative 0.618:0.382 compromise may be often ideal.

In the 6 other species studied, the NI/BI ratios were larger than BI/NB ratios, which is a mathematical consequence of this geometrical arrangement. Interestingly, subtraction of the smaller from the higher ratio resulted in a spectrum in which humans are at 1 end and the simplest mammal sampled, the Eastern Cottontail rabbit, at the other. We do not propose, however, that this necessarily represents an index for structural complexity in animals in general or even in mammals in particular. For instance, the brain-to-body mass ratio was developed in an attempt to quantify neural or evolutionary sophistication or complexity. Although useful in general terms, the brain-to-body mass ratios have been fraught with inconsistencies and are therefore not universally applicable. For instance, the masked shrew (Sorex cinereus) and the water shrew (Sorex palustris) have among the highest known brain-to-body mass ratios (2.5% and 2.3%, respectively), which are 1.2 to 1.3 times that of humans (1.4 kg/75 kg = 1.9%).⁴² The encephalization quotient (EQ) was developed as a more sophisticated version of the brain-to-body mass index and consists of a ratio between observed and predicted brain mass for a mammal of size predicted by nonlinear regression in turn based on allometric parameters. The EQ is limited in its use since it on can be applied only within specific taxa and has yielded paradoxical data.^27,43

In summary, we report that in humans the ratio of the nasioiniac arc over the parieto-occipital arc, as well as that of the parieto-occipital arc over the frontal arc, essentially coincide and that this ratio is 1.6, which is surprisingly close to the Φ, 1.618. In addition, we report that humans have the lowest nasion-inion arc to bregma -inion arc cranial ratio of the 7 mammalian species surveyed, which may be an indicator of evolutionary sophistication. The subdivision of the nasioiniac arc by bregma into 2 unequal arcs is a situation analogous to that of the geometrical division of a line into the Golden Ratio. We hypothesize that the Golden Ratio principle, which has been documented in other biological and natural systems, may be present in the architecture and evolution of the human skull as well.