Skip to main content
PMC home page
Journal of International Society of Preventive & Community Dentistry logoLink to Journal of International Society of Preventive & Community Dentistry
. 2020 Apr 2;10(2):134–141. doi: 10.4103/jispcd.JISPCD_406_19

How Fluoride Protects Dental Enamel from Demineralization

James Patrick Simmer 1,✉, Nina C Hardy 1, Afriti F Chinoy 1, John D Bartlett 2, Jan C-C Hu 1
PMCID: PMC7339990  PMID: 32670900

ABSTRACT

Introduction:

How fluoride (F–) protects dental enamel from caries is here conveyed to dental health-care providers by making simplifying approximations that accurately convey the essential principles, without obscuring them in a myriad of qualifications.

Materials and Methods:

We approximate that dental enamel is composed of calcium hydroxyapatite (HAP), a sparingly soluble ionic solid with the chemical formula Ca10(PO4)6(OH)2.

Results:

The electrostatic forces binding ionic solids together are described by Coulomb’s law, which shows that attractions between opposite charges increase greatly as their separation decreases. Relatively large phosphate ions (PO43–) dominate the structure of HAP, which approximates a hexagonal close-packed structure. The smaller Ca2+ and OH– ions fit into the small spaces (interstices) between phosphates, slightly expanding the close-packed structure. F– ions are smaller than OH– ions, so substituting F– for OH– allows packing the same number of ions into a smaller volume, increasing their forces of attraction. Dental decay results from tipping the solubility equilibrium Ca10(PO4)6(OH)2 (s) ⇔ 10Ca2+ (aq) + 6PO42– (aq) + 2OH– (aq) toward dissolution. HAP dissolves when the product of its ion concentrations, [Ca2+]10×[PO43–]6×[OH–]2, falls below the solubility product constant (Ksp) for HAP.

Conclusion:

Because of its more compact crystal structure, the Ksp for fluorapatite (FAP) is lower than the Ksp for HAP, so its ion product, [Ca2+]10×[PO43–]6×[F–]2, must fall further before demineralization can occur. Lowering the pH of the fluid surrounding enamel greatly reduces [PO43–] (lowering the ion products of HAP and FAP equally), but [OH–] falls much more rapidly than [F–], so FAP better resists acid attack.

KEYWORDS: Caries, hydroxyapatite, solubility product constant, tooth

INTRODUCTION

Dental caries is highly prevalent worldwide and remains a major public health concern.[1,2] Dental enamel consists largely of the mineral calcium hydroxyapatite (HAP), which is described by the empirical formula Ca10(PO4)6(OH)2. Resistance to dental caries is imparted by the substitution of some hydroxyl (OH–) ions with fluoride (F–) ions, creating areas of fluorohydroxyapatite, or Ca10(PO4)6(OH)(2–x)Fx, mostly on the surface enamel.[3]

Community water fluoridation is the upward adjustment of the natural fluoride level in a community’s water supply to prevent dental caries. It is a population-based method of primary prevention that uses community water systems to deliver low-dose fluoride that is meant to be ingested over frequent intervals.[4] Water fluoridation is effective in reducing community caries prevalence,[5] even in communities with relatively low caries experience.[6] On the other hand, F– has toxic properties that cause developmental enamel malformations known as dental fluorosis, which increase in communities with fluoridated water supplies.[7,8] The U.S. Public Health Service currently recommends an optimal fluoride concentration of 0.7mg/L.[9] Some communities have groundwater with a naturally high concentration of fluoride, so fluorosis of the skeleton and the dentition becomes a greater concern than caries.[10,11] The World Health Organization recommends reducing the [F–] when it exceeds 1.5mg/L.[12] Given that water fluoridation comes with a fluorosis trade-off, discussion of the pros and cons of water fluoridation will continue. It is important that dental health providers should be part of that discussion and should know how fluoride protects against dental caries. This knowledge is also important for dental care providers to prevent and treat dental decay clinically.

Our purpose in this review was to provide dental health-care providers with a clear understanding of the structure of calcium HAP, how it demineralizes, and how substituting OH– with F– reduces susceptibility to demineralization.

COULOMB’S LAW

Ionic solids such as HAP hold together by electrostatic interactions. The forces of attraction between oppositely charged ions in ionic solids are described by Coulomb’s law [Figure 1]. The strength of attraction is determined by the magnitudes of the charges, their distance apart, and by the dielectric constant of the surrounding medium. The ion charges (q) are multiplied in the numerator, while the denominator has the distance of charge separation squared (r2) multiplied by the dielectric constant (D). From this equation, one can readily appreciate the outsized effect of ion separation on the force of attraction. Therefore, the more tightly packed the ions in a crystal, the more difficult it is to pull apart through dissolution.

Figure 1.

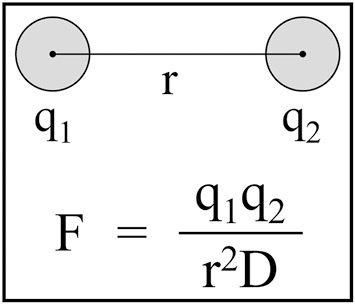
Figure 1

Open in a new tab

Coulomb’s law. Coulomb’s law quantifies the amount of electrostatic force (F) between charged bodies at rest, which are the forces that hold together ionic solids, such as the nonstoichiometric calcium hydroxyapatite in teeth, which deviates from perfect hydroxyapatite by the presence of vacancies and substitutions. The D or dielectric constant in the denominator shows how the forces holding together an ionic solid are diminished by the nature of the surrounding medium. The r2 shows how the force of attraction between two opposite charges diminishes by the square of their separation. The strength of an electrostatic force (F) holding together ions in a crystal is directly proportional to the product of the charges (q1 × q2) and inversely proportional to the square of their separation (r2). F = electrostatic force, q1 and q2 = values for the charges, r = the distance between charges, D = the dielectric constant (= 1 for vacuum and 80 for water)

Many different ionic solids can form from calcium and phosphate ions, but the least soluble are calcium HAP and calcium fluorapatite (FAP) due to the close packing of ions in their structures.[13] HAP and FAP are closely related crystals, having essentially the same structure except for the substitution of OH– in HAP with F– in FAP. Their highly compact structures enable strong forces of attraction between the oppositely charged ions, which contribute to their low solubility in aqueous solutions and suitability as a material for enamel. Amelogenin (Amelx) (which encodes the most abundant matrix protein during enamel formation) knockout mice produce only a thin layer of octacalcium phosphate (OCP) on the surface of dentin.[14] OCP is a more soluble mineral than HAP and also forms on dentin in Mmp20 knockout mice,[15] suggesting that enamel proteins actively promote the formation of HAP over other mineral phases.

The dielectric constant in the denominator of Coulomb’s law indicates that medium surrounding an ionic solid can have a great influence on the forces of attraction binding ionic solids together. The dielectric constant for water is 80 and for a vacuum is 1. Because the forces of attraction of an ionic solid in water are only 1/80th as strong as they would be in a vacuum, Coulomb’s law indicates that ionic solids will tend to dissolve in water. The dielectric constant of water is high because of its polarity, which arises from the electronegativity difference between oxygen and hydrogen. This causes unequal sharing of electrons and creates a dipole moment with a partial negative charge on oxygen and a partial positive charge on hydrogen. The polar water molecules form electrostatic interactions with the ions on the surface of dental enamel, causing a certain rate of ions leaving the crystal surface and going into solution. Unless there is an equal or greater rate of ions depositing on the enamel surface, there will be net demineralization. Fortunately, saliva generally contains concentrations of Ca2+, PO43–, and OH– that offset the loss of ions into the media with an equal or higher rate of ion deposition.[16,17]

THE STRUCTURE OF CALCIUM HYDROXYAPATITE [Ca2+]10[PO43–]6[OH–]2

Using some minor simplifying approximations so that the essential features of the HAP structure are not obscured, we consider the HAP structure as a packing together of rigid spheres of discrete sizes.[18] The calcium HAP structure is dominated by phosphate anions (PO43–) that are significantly larger than the other ions (Ca2+ and OH–) in the crystal. Phosphate ions contain a central phosphorus atom, with four oxygen atoms positioned at the apices of a tetrahedron. We conceptualize the HAP structure as phosphate spheres arranged as layered sheets in a hexagonal close-packed (HCP) arrangement [Figure 2]. The calcium and hydroxyl ions are tucked into the spaces (interstices) between the phosphates. This simplification turns out to be a good approximation, but in the actual HAP structure the small ions, particularly the hydroxyls, are too large to fit in the interstices without expanding slightly the nearly close-packed arrangement of phosphates.

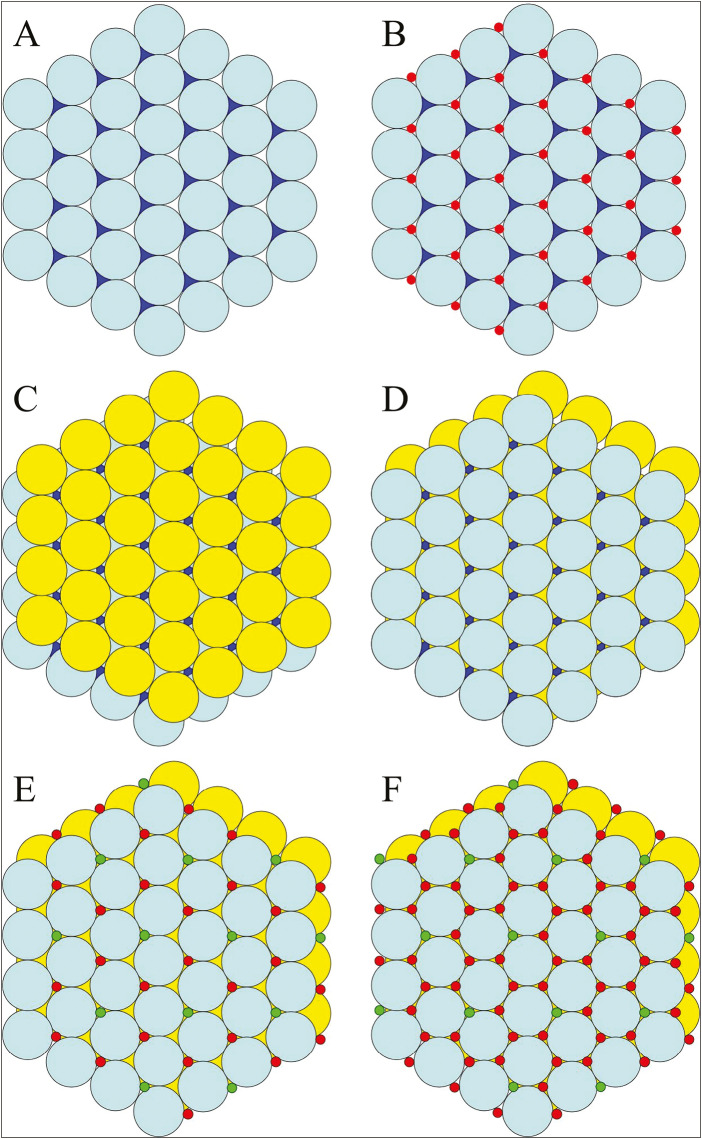
Figure 2.

Figure 2

Open in a new tab

Building calcium hydroxyapatite. (A) Phosphate ions (PO43–) can be imagined as single layer (cyan layer, “A”) of billiard balls confined by a hexagonal rack. In the hexagonal close-packed layer of phosphate spheres, there are twice as many interstices as spheres. Half of the spaces are leftward pointing (white); half are rightward pointing (blue). (B) All of the leftward-pointing interstices are filled with smaller spheres (red), representing Ca2+ ions (designated Ca2). (C) A second layer of billiard balls (yellow, “B”) will not stack directly on top of the A layer, but rests on the depressions over the leftward-pointing interstices filled with Ca2 ions. (D) The third layer of phosphate ions (cyan) stacks directly on top of the original A layer. Because we have not added the hydroxyl ions or most of the Ca2+ ions, it is clear that the rightward-pointing interstices of the original A layer are still visible and that the ions occupying these spaces will form a column extending up the entire height of the crystal. (E) The column interstices are occupied by Ca2+ (designated Ca1) and OH– ions in a ratio of 2:1. The column interstices are filled so that each particular column is filled either with OH– or Ca2+ ions, and not a mixture, so there are columns of Ca1 and OH– ions running from top to bottom throughout the crystal. (F) All of the interstices of the nearly close-packed hexagonally arranged PO43– ions are filled in hydroxyapatite. Ca2 ions occupy the leftward-pointing interstices of the A layers and the rightward-pointing interstices of the B layers, which are capped by phosphate ions above and below

An HCP arrangement represents the densest possible packing of the phosphate “spheres” [Figure 2A]. Within a single layer of spheres, each phosphate has six phosphate nearest neighbors. There are two spaces between adjacent spheres. Looking down on the HCP layer, these spaces appear as leftward or rightward-pointing triangles. There are twice as many interstitial sites as there are spheres (one left-pointing and one right-pointing), which provide the exact number of spaces needed for the calcium and hydroxyl ions. In HAP, the second layer of phosphate spheres rests in the depressions above the leftward-pointing triangles [Figure 2C]. The third phosphate layer sits on the rightward-pointing triangles of the second layer, and directly over the phosphates in the first layer [Figure 2D]. The layers of phosphate spheres are thus arranged in an A–B–A–B… sequence. The phosphates in an A layer are vertically aligned with phosphates in the other A layers. The phosphates in the B layers are vertically aligned with phosphates in the other B layers but are displaced with respect to the phosphates in the A layers. It can be seen from the stacked phosphate spheres that there are empty channels or columns running up and down the lattice. The columns pass through the rightward-pointing triangles of the A layers and the leftward-pointing triangles of the B layers. These channels are filled with ions: one-third of the column interstices are filled with hydroxyl ions, while two-thirds are filled with calcium ions (Ca2+) [Figure 2E]. The hydroxyl columns are also aligned in sheets. Only 40+ of the calcium ions are in columns (designated Ca1). The leftward-pointing triangular spaces of the A layers and the rightward-pointing triangular spaces of the B layers are occupied by non-columnar calcium ions (designated Ca2), and are capped with phosphates from the layers above and below [Figure 2B and C]. The calcium and hydroxyl ions in the interstices between the hexagonally packed phosphates force the phosphate ions apart, so they are no longer close-packed. Hydroxyl ions are larger than the calcium ions and expand the crystal structure to a greater degree. Fluoride ions are smaller than hydroxyl ions, so substituting F– for OH– allows the phosphates to achieve closer packing (reducing unit cell dimensions),[19] which increases both the forces of attraction between oppositely charged ions and the stability of the crystal in aqueous solutions.

PRECIPITATION OF IONIC SOLIDS FROM SOLUTIONIN VIVO

Ionic solids such as HAP and enamel form by the precipitation of ions from solution [Figure 3]. As ions go into the solid state, electrostatic interactions with water are replaced by electrostatic interactions with ions in the crystal. Precipitation occurs in two stages: nucleation and crystal growth. Nucleation is the initial formation of tiny crystals, which can be unpredictably slow unless facilitated by a nucleator (a surface to form on). Enamel mineral ribbons initiate on the surface of freshly mineralized dentin[20] as amorphous calcium phosphate,[21] which transforms into calcium HAP crystals. In enamel formation, crystal growth is divided into two stages: crystal elongation (appositional growth) and crystal maturation. Crystal elongation occurs first and involves the lengthening of the crystals along a particular axis, called the c-axis, in close proximity to the secretory surfaces of the ameloblast distal membrane.[22] These long, thin crystallites are surrounded by matrix proteins and will continue to grow longer until the entire thickness of the enamel layer is achieved. Crystal maturation is the deposition of ions on the sides of the crystals, which increases their width and thickness.[23] This occurs as matrix proteins are removed so that the soft protein-rich enamel becomes hard and virtually protein free. Enamel formation occurs in a highly controlled extracellular environment that favors mineralization.[24] Following tooth eruption, control over the milieu surrounding enamel shifts to saliva, which is normally supersaturated with respect to HAP[17] and contains proteins that favor the maintenance of a healthy oral microbiota.[25] Saliva, however, cannot always maintain an anticaries environment. One factor is that dietary sucrose metabolized into lactic acid by Streptococcus mutans may exceed the neutralizing capacity of salivary bicarbonate, lowering plaque pH, which in turn drops [PO43–] and [OH–] below levels needed to sustain mineralization and remineralization.[26,27]

Figure 3.

Figure 3

Open in a new tab

Interaction of hydroxyapatite with ions in solution. Hydroxyapatite (HAP) demineralization and remineralization reactions are reversible and depend on the concentrations of ions in the solution surrounding the crystal. The negatively charged ions in solution that maintain the ion product (Qsp) above or equal to the solubility product constant (Ksp) of hydroxyapatite are reduced in concentration by a drop in pH. When the pH of the solution in contact with HAP falls from 7 to 5, the [OH–] falls from 10–7 to 10–9 M (is reduced by 10–2), reducing the Qsp by (10–2)2 or 10–4. The [PO43–] falls even further, from an original concentration of N, to 1.6 × 10–4 × N, reducing the Qsp by (1.6 × 10–4)6 or 1.7 × 10–23. The total fall in Qsp from the [OH–] and [PO43–] would be 1.7 × 10–27. If this reduced the Qsp below the Ksp for HAP (5.5 × 10–118), then HAP would start to demineralize. A similar drop in pH for fluorapatite barely affects [F–], so the fall in the Qsp of fluorapatite would be that caused by the drop in [PO43–], or by 1.7 × 10–23. If this reduced the Qsp below the lower Ksp for FAP (5.0 × 10–123), then the FAP would start to demineralize

SOLUBILITY PRODUCTS (KSP) AND IN PRODUCTS (QSP) AND DEMINERALIZATION

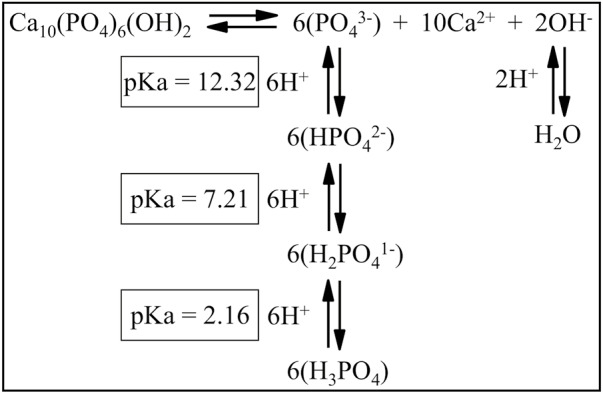
Ionic solids are continuously reacting with the surrounding media. At equilibrium, an ionic solid (s) in water will have an equal number of ions leaving the crystal surface and going into aqueous (aq) solution as are adsorbing onto the crystal. For HAP, the solubility equilibrium expression is as follows:

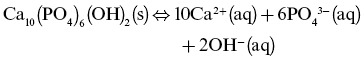
graphic file with name JISPCD-10-134-g004.jpg

This type of chemical equilibrium is described by a solubility product constant (Ksp):

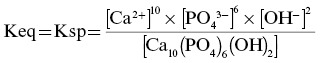
graphic file with name JISPCD-10-134-g005.jpg

Equilibrium constants generally take the form of the mathematical product of the concentrations of the reaction products over the mathematical product of the concentrations of the reactants, each raised to the power corresponding to its coefficient in the chemical equilibrium expression. As HAP is a sparingly soluble solid, its concentration (in the denominator) is given a value of 1, simplifying the expression to:

graphic file with name JISPCD-10-134-g006.jpg

The ion product (Qsp) is represented by the same formula as Ksp but with reactant and product concentrations from any specific point in the reaction’s progress, not just at equilibrium. The Ksp of an ionic solid is equal to the ion product (Qsp) of the concentrations of the ions that make up the solid (raised to the appropriate power) when the ionic solid is at equilibrium with its surroundings. A crystal with a low Ksp is less soluble than a crystal with a high Ksp. The Ksp for a particular crystal structure is approximated to be a constant that is a characteristic of that crystal. Different crystal structures have different Ksp values. The lower the Ksp of a crystal, the fewer constituent ions must be in the surrounding solution to prevent the crystal from dissolving. When the ion product (Qsp) of a solution equals the solubility product constant (Ksp) of a crystal, there will be no net gain or loss of ions from the crystal. Thus, Qsp equals Ksp when a crystal (solid phase) is at equilibrium with the ions in solution (aqueous phase). Such a solution is minimally saturated with respect to the mineral. When the Qsp of an ionic solution surrounding a crystal is less than the Ksp, the crystal will dissolve. When the Qsp is greater than the Ksp, the crystal grows. Raising the concentration of any ionic component of a particular mineral increases its Qsp, but increasing the concentration of the most abundant ion in the crystal (Ca2+ in the case of HAP) has the greatest effect on the degree of saturation of the surrounding fluid. A solution is called “supersaturated” with respect to a mineral within it when its Qsp is greater than the Ksp of the mineral.

Ksp is a useful measure of the solubility of a crystal. Recall that a crystal with a low Ksp is less soluble than a crystal with a high Ksp. The Ksp for calcium HAP is about 5.5 × 10–118 M,[13] a very low number. Calcium HAP has a low Ksp because of its near close-packed structure, which minimizes the r in the r2 term in Coulomb’s law. The Ksp for calcium FAP is about 5.0 × 10–123 M, which is even lower than the Qsp of HAP.[13] This reflects the even tighter packing of phosphates and the slightly reduced volume of the FAP unit cell relative to HAP, which both contain the same number of charges.

DEPENDENCE OF ION PRODUCT ON pH

The stability of enamel mineral is dependent up on the Qsp of the media surrounding it. Holding total calcium and phosphate concentrations constant, acidity in the media reduces the stability of enamel mineral by reducing the Qsp of the surrounding fluid. Acidity refers to the hydrogen ion [H+] or proton concentration of the liquid media. Hydrogen ions associate with anions (negatively charged ions) in solution, lowering the concentrations of the unprotonated forms (such as PO43– and OH–) that are in equilibrium with the mineral, thereby reducing the Qsp of HAP.

The pH of saliva normally varies.[28] An average pH of 6.9 ± 0.6 was observed in the unstimulated saliva of 50 healthy individuals.[29] The pH in the oral cavity falls due to the conversion of sucrose into organic acids, such as lactate. A common misconception is that a “critical pH” exists below which enamel will demineralize.[30,31,32] This critical pH concept conveys the useful idea that if the pH falls far enough, enamel will start to demineralize, but it does not demineralize when the pH reaches a certain point. It demineralizes when the Qsp falls below the Ksp of the mineral, which depends on salivary gland excretions of calcium, phosphate, and bicarbonate, as well as fluoride levels in the enamel. Dental health-care providers should be aware that a loss of salivary gland function, for instance following irradiation cancer treatments, results in very high caries rates.[33,34] The pH at which fluoridated enamel will start to demineralize is lower than the pH at which non-fluoridated HAP starts to demineralize. The pH at which tooth mineral will demineralize does not have a single, universal value. A given amount of acid introduced into the oral cavity will not even cause the pH to fall predictably, due to variations in the amount of bicarbonate in the saliva, which neutralizes acid.

Pure water has an H2O concentration ~55.5 M. A very small fraction of the water molecules dissociate into ions: H2O ⇔ H+ + OH–. The equilibrium constant (Keq) for this reaction is [H+] × [OH–]/[H2O]. Since the concentration of H2O is essentially unchanged by the dissociation of water, the expression simplifies to Keq = Kw = [H+] × [OH–] and is found to equal 10–14 M2. In pure water, the [H+] and [OH–] are both equal to about 10–7 M. If the pH of the aqueous solution drops from 7 to 5, the [H+] increases to 10–5 M and [OH–] falls to 10–9 M, or to 1+ of its previous concentration.

To understand how [F–] is affected by a drop in pH, we turn to the Henderson–Hasselbalch (HH) equation: pH = pKa + log([A–]/[HA]), which defines pKa as the pH at which a weak acid is half dissociated (when [A–] = [HA] and [A–]/[HA] = 1). Because the log of 1 = 0, pH = pKa when [A–] = [HA]. For the weak acid hydrogen fluoride (HF ⇔ H+ + F–), the pKa is 3.2. Let us start, like we did for OH–, with a pH of 7 and an F– concentration of 10–7 M. Plugging into the HH equation, 7.0 = 3.2 + log([10–7]/[HF]), we determine that [HF] = 1.58 × 10–11, so 99.8+ of the fluorine is in the F– (fluoride) form. When we drop the pH to 5.0, the HH equation shows that [F–] falls to 9.84 × 10–8, or to 98.4+ of its concentration at pH 7, a drop of less than 2+:

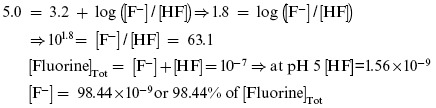
graphic file with name JISPCD-10-134-g007.jpg

The ion product (Qsp) for HAP is [Ca2+]10×[PO43–]6×[OH–]2. When the pH falls from 7 to 5, the [OH–] falls to 1+ (1/100 or 10–2) of its original value, and the Qsp of HAP falls by (10–2)2 to 10–4 (1/10,000) of its previous level. The ion product (Qsp) for FAP is [Ca2+]10×[PO43–]6×[F–]2. When the pH falls from 7 to 5, [F–] falls to 98.4+ and the Qsp of FAP falls by (0.984)2 or to approximately 97+ of its previous level, a trivial reduction. Phosphate concentration [PO43–] also falls with a drop in pH from 7 to 5, but this affects the ion products of HAP and FAP equally.

CONCLUSION

We presented a model for calcium HAP[18] that facilitates understanding its hexagonal near close-packing arrangement of PO43– ions, which is only slightly expanded by the presence of Ca2+ and OH– ions in the interstices between phosphates. We discussed how the substitution of F– for OH– allows the PO43– ions to achieve closer packing and applied Coulomb’s law to indicate that reducing the distance between oppositely charged ions greatly increases their forces of attraction. Having the same number of charges in a smaller volume gives FAP a Ksp that is lower than that of HAP. Although the substitution of F– for OH– on the enamel surface is not likely to approach 100+, the ion product of the oral fluid must fall thousands of fold further than that of HAP before fluoridated enamel will start to demineralize. We also discussed how a drop in pH from 7 to 5 lowers the [OH–] 100-fold and the Qsp of HAP in the oral fluid 10,000-fold, whereas the same fall in pH lowers the [F–] by less than 2+. Fluoride improves the intrinsic stability of the mineral structure (lowering its Ksp) and prevents its Qsp from falling as rapidly when pH drops. The benefits are particularly helpful after a drop in pH from 7 to 5, where the ion products of both HAP and fluoridated HAP fall by 1.7 × 10–23 fold due to the drop in [PO43–] alone.

FINANCIAL SUPPORT AND SPONSORSHIP

This study was supported by the National Institute of Dental and Craniofacial Research (NIDCR)/the National Institutes of Health (NIH) grants DE-015846 (to JH), DE-27675 (to JPS), and DE-028297 (to JDB).

CONFLICTS OF INTEREST

There are no conflicts of interest.

AUTHOR CONTRIBUTIONS

All authors (JPS, NCH, AFC, JDB, and JC-CH) contributed to literature search and analysis, manuscript and figure preparation, manuscript editing, and review. In addition, JPS and JCCH initially outlined the manuscript, which was revised by JDB before manuscript preparation.

ETHICAL POLICY AND INSTITUTIONAL REVIEW BOARD STATEMENT

No clinical data was presented in this review, so no Ethical policy and Institutional Review board statement is needed or appropriate.

PATIENT DECLARATION OF CONSENT

No patients were recruited for this review, so no patient declarations of consent are needed or appropriate.

DATA AVAILABILITY STATEMENT

No data was collected or presented in this review, so no data can be made available.

ACKNOWLEDGEMENTS

All authors (JPS, NCH, AFC, JDB, and JC-CH) contributed to literature search and analysis, manuscript and figure preparation, manuscript editing, and review. In addition, JPS and JCCH initially outlined the manuscript, which was revised by JDB before manuscript preparation.

REFERENCES

  • 1.Kassebaum NJ, Smith AGC, Bernabé E, Fleming TD, Reynolds AE, Vos T, et al. GBD 2015 Oral Health Collaborators. Global, regional, and national prevalence, incidence, and disability-adjusted life years for oral conditions for 195 countries, 1990-2015: A systematic analysis for the global burden of diseases, injuries, and risk factors. J Dent Res. 2017;96:380–7. doi: 10.1177/0022034517693566. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 2.Collaborators GDaIIaP. Global, regional, and national incidence, prevalence, and years lived with disability for 354 diseases and injuries for 195 countries and territories, 1990–2017: A systematic analysis for the Global Burden of Disease Study 2017. Lancet. 2018;392:1789–858. doi: 10.1016/S0140-6736(18)32279-7. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 3.Scholz KJ, Federlin M, Hiller KA, Ebensberger H, Ferstl G, Buchalla W. EDX-analysis of fluoride precipitation on human enamel. Sci Rep. 2019;9:13442. doi: 10.1038/s41598-019-49742-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 4.Neenan EM, Easley MW, Ruiz M. Water fluoridation. In: Harris NO, Garcia-Godoy F, editors. Primary Preventive Dentistry. 6th ed. Upper Saddle River, NJ: Pearson Education; 2004. pp. 181–240. [Google Scholar]
  • 5.Iheozor-Ejiofor Z, Worthington HV, Walsh T, O’Malley L, Clarkson JE, Macey R, et al. Water fluoridation for the prevention of dental caries. Cochrane Database Syst Rev. 2015;18:CD010856. doi: 10.1002/14651858.CD010856.pub2. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 6.Cruz MGBD, Narvai PC. Caries and fluoridated water in two Brazilian municipalities with low prevalence of the disease. Rev Saude Publica. 2018;52:28. doi: 10.11606/S1518-8787.2018052016330. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 7.Whelton H, Crowley E, O’Mullane D, Donaldson M, Kelleher V, Cronin M. Dental caries and enamel fluorosis among the fluoridated and non-fluoridated populations in the republic of Ireland in 2002. Community Dent Health. 2004;21:37–44. [PubMed] [Google Scholar]
  • 8.Macey R, Tickle M, MacKay L, McGrady M, Pretty IA. A comparison of dental fluorosis in adult populations with and without lifetime exposure to water fluoridation. Community Dent Oral Epidemiol. 2018;46:608–14. doi: 10.1111/cdoe.12411. [DOI] [PubMed] [Google Scholar]
  • 9.Fluoridation FPoCW. U.S. Public Health Service Recommendation for fluoride concentration in drinking water for the prevention of dental caries. Public Health Rep. 2015;130:318–31. doi: 10.1177/003335491513000408. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 10.Unde MP, Patil RU, Dastoor PP. The untold story of fluoridation: Revisiting the changing perspectives. Indian J Occup Environ Med. 2018;22:121–7. doi: 10.4103/ijoem.IJOEM_124_18. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 11.Yadav KK, Kumar V, Gupta N, Kumar S, Rezania S, Singh N. Human health risk assessment: Study of a population exposed to fluoride through groundwater of Agra City, India. Regul Toxicol Pharmacol. 2019;106:68–80. doi: 10.1016/j.yrtph.2019.04.013. [DOI] [PubMed] [Google Scholar]
  • 12.World Health Organization. Guidelines for Drinking-Water Quality Fourth Edition Incorporating First Addendum, 4th ed + 1st Add. Geneva, Switzerland: World Health Organization; 2017. [PubMed] [Google Scholar]
  • 13.Johnsson MS, Nancollas GH. The role of brushite and octacalcium phosphate in apatite formation. Crit Rev Oral Biol Med. 1992;3:61–82. doi: 10.1177/10454411920030010601. [DOI] [PubMed] [Google Scholar]
  • 14.Hu Y, Smith CE, Cai Z, Donnelly LA, Yang J, Hu JC, et al. Enamel ribbons, surface nodules, and octacalcium phosphate in C57Bl/6 Amelx -/- mice and Amelx +/- lyonization. Mol Genet Genomic Med. 2016;4:641–61. doi: 10.1002/mgg3.252. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 15.Yamazaki H, Tran B, Beniash E, Kwak SY, Margolis HC. Proteolysis by MMP20 prevents aberrant mineralization in secretory enamel. J Dent Res. 2019;98:468–75. doi: 10.1177/0022034518823537. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 16.Rathje W. Oversaturation of saliva with hydroxyapatite. J Dent Res. 1956;35:245–8. doi: 10.1177/00220345560350021301. [DOI] [PubMed] [Google Scholar]
  • 17.Hay DI. Salivary factors in caries models. Adv Dent Res. 1995;9:239–43. doi: 10.1177/08959374950090030801. [DOI] [PubMed] [Google Scholar]
  • 18.Elliott JC. The problems of the composition and structure of the mineral components of the hard tissues. Clin Orthop. 1973;93:313–45. doi: 10.1097/00003086-197306000-00037. [DOI] [PubMed] [Google Scholar]
  • 19.Bhatnagar VM. Unit cell dimensions of synthetic apatites. Experientia. 1968;24:765. doi: 10.1007/BF02144852. [DOI] [PubMed] [Google Scholar]
  • 20.Smith CE, Hu Y, Hu JC, Simmer JP. Ultrastructure of early amelogenesis in wild-type, Amelx-/-, and Enam-/- mice: Enamel ribbon initiation on dentin mineral and ribbon orientation by ameloblasts. Mol Genet Genomic Med. 2016;4:662–83. doi: 10.1002/mgg3.253. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 21.Beniash E, Metzler RA, Lam RS, Gilbert PU. Transient amorphous calcium phosphate in forming enamel. J Struct Biol. 2009;166:133–43. doi: 10.1016/j.jsb.2009.02.001. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 22.Simmer JP, Richardson AS, Hu YY, Smith CE, Ching-Chun Hu J. A post-classical theory of enamel biomineralization… and why we need one. Int J Oral Sci. 2012;4:129–34. doi: 10.1038/ijos.2012.59. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 23.Smith CE. Cellular and chemical events during enamel maturation. Crit Rev Oral Biol Med. 1998;9:128–61. doi: 10.1177/10454411980090020101. [DOI] [PubMed] [Google Scholar]
  • 24.Simmer JP, Fincham AG. Molecular mechanisms of dental enamel formation. Crit Rev Oral Biol Med. 1995;6:84–108. doi: 10.1177/10454411950060020701. [DOI] [PubMed] [Google Scholar]
  • 25.Lynge Pedersen AM, Belstrøm D. The role of natural salivary defences in maintaining a healthy oral microbiota. J Dent. 2019;80:S3–12. doi: 10.1016/j.jdent.2018.08.010. [DOI] [PubMed] [Google Scholar]
  • 26.Loesche WJ. Role of Streptococcus mutans in human dental decay. Microbiol Rev. 1986;50:353–80. doi: 10.1128/mr.50.4.353-380.1986. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 27.Gupta M. Sugar substitutes: Mechanism, availability, current use and safety concerns-an update. Open Access Maced J Med Sci. 2018;6:1888–94. doi: 10.3889/oamjms.2018.336. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 28.Humphrey SP, Williamson RT. A review of saliva: Normal composition, flow, and function. J Prosthet Dent. 2001;85:162–9. doi: 10.1067/mpr.2001.113778. [DOI] [PubMed] [Google Scholar]
  • 29.Patel RM, Varma S, Suragimath G, Zope S. Estimation and comparison of salivary calcium, phosphorous, alkaline phosphatase and pH levels in periodontal health and disease: A cross-sectional biochemical study. J Clin Diagn Res. 2016;10:ZC58–61. doi: 10.7860/JCDR/2016/20973.8182. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 30.Clapp O, Morgan MZ, Fairchild RM. The top five selling UK energy drinks: Implications for dental and general health. Br Dent J. 2019;226:493–7. doi: 10.1038/s41415-019-0114-0. [DOI] [PubMed] [Google Scholar]
  • 31.Dawes C. What is the critical pH and why does a tooth dissolve in acid? J Can Dent Assoc. 2003;69:722–4. [PubMed] [Google Scholar]
  • 32.Buzalaf MAR, Pessan JP, Honório HM, Ten Cate JM. Mechanisms of action of fluoride for caries control. Monogr Oral Sci. 2011;22:97–114. doi: 10.1159/000325151. [DOI] [PubMed] [Google Scholar]
  • 33.Sroussi HY, Epstein JB, Bensadoun RJ, Saunders DP, Lalla RV, Migliorati CA, et al. Common oral complications of head and neck cancer radiation therapy: Mucositis, infections, saliva change, fibrosis, sensory dysfunctions, dental caries, periodontal disease, and osteoradionecrosis. Cancer Med. 2017;6:2918–31. doi: 10.1002/cam4.1221. [DOI] [PMC free article] [PubMed] [Google Scholar]
  • 34.Palmier NR, Ribeiro ACP, Fonsêca JM, Salvajoli JV, Vargas PA, Lopes MA, et al. Radiation-related caries assessment through the international caries detection and assessment system and the post-radiation dental index. Oral Surg Oral Med Oral Pathol Oral Radiol. 2017;124:542–7. doi: 10.1016/j.oooo.2017.08.019. [DOI] [PubMed] [Google Scholar]

Associated Data

This section collects any data citations, data availability statements, or supplementary materials included in this article.

Data Availability Statement

No data was collected or presented in this review, so no data can be made available.

Articles from Journal of International Society of Preventive & Community Dentistry are provided here courtesy of Wolters Kluwer -- Medknow Publications

RESOURCES