E487K-Induced Disorder in Functionally Relevant Dynamics of Mitochondrial Aldehyde Dehydrogenase 2

Abstract

Mitochondrial aldehyde dehydrogenase 2 (ALDH2), which is a homotetramer assembled by two equivalent dimers, is an important enzyme that metabolizes ethanol-derived acetaldehyde to acetate in a coenzyme-dependent manner. The highly reactive acetaldehyde exhibits a toxic effect, indicating that the proper functioning of ALDH2 is essential to counteract aldehyde-associated diseases. It is known that the catalytic activity of ALDH2 is drastically impaired by a frequently observed mutation, E487K, in a dominant fashion. However, the molecular basis of the inactivation mechanism is elusive because of the complex nature of the dynamic behavior. Here, we performed microsecond-timescale molecular dynamics simulations of the proteins complexed with coenzymes. The E487K mutation elevated the conformational heterogeneity of the dimer interfaces, which are relatively distal from the substituted residue. Dynamic network analyses showed that Glu487 and the dimer interface were dynamically communicated, and the dynamic community further spanned throughout all of the subunits in the wild-type; however, this network was completely rearranged by the E487K mutation. The perturbation of the dynamic properties led to alterations of the global conformational motions and destabilization of the coenzyme binding required for receiving a proton from the catalytic nucleophile. The insights into the dynamic behavior of the dominant negative mutant in this work will provide clues to restore its function.

Significance

The E487K mutation in mitochondrial aldehyde dehydrogenase 2 has been implicated in perturbing the dynamic property in its extensive region, inducing drastic impairments of the coenzyme-dependent catalytic activity in a dominant fashion. However, the dynamics studies are limited so far, making the inactivation mechanism obscure. Our study aims to clarify how the single amino acid substitution significantly impairs the function using molecular dynamics simulations. The dynamics network analyses revealed that the dynamic community containing Glu487 spanned throughout the overall molecule to maintain the proper conformational motion in the wild-type, and the mutation completely rearranged it. The perturbation of the dynamic network resulted in diversification of the global conformation and impairment of the stable binding of the coenzyme essential for the catalysis.

Introduction

Mitochondrial aldehyde dehydrogenase 2 (ALDH2), which acts as a homotetramer formed by a pair of equivalent dimers (Fig. S1 A), is known to be the most important enzyme to metabolize ethanol-derived acetaldehyde to acetate in an NAD(P)⁺-dependent manner (1, 2, 3). Because the highly reactive acetaldehyde is known to exhibit a toxic effect on biological functions through the formation of its adducts with biological macromolecules, including lipids, proteins, and DNA (4, 5, 6), proper functioning of ALDH2 is essential to play a protective role against various aldehyde-associated diseases, such as malignant tumors and neurological diseases (5,7, 8, 9).

It is widely known that an ALDH2 gene allele, associated with the substitution of the glutamate at the position 487 by the lysine (E487K), generates a variant that is most commonly found in the East Asian population (∼40%) (10). This single amino acid substitution drastically impairs the activity of ALDH2 by increasing the K_M-value for NAD⁺ by ∼200-fold and decreasing the k_cat-value by a factor of 10 (11). Furthermore, the negative effect is exhibited in a dominant fashion, as the E487K substitution in even one half of the subunits decreases the catalytic activity to less than 20% (12, 13, 14). Therefore, a precise understanding of the deactivation mechanism would be clinically significant to counteract aldehyde-associated diseases.

An individual subunit of ALDH2 is synthesized as a 517-residue precursor protein containing a 17-residue mitochondrial targeting sequence that is subsequently cleaved to generate a 500-residue mature protein (this residue number is used hereafter). A previous crystallographic study revealed that the individual subunit of ALDH2 is composed of a coenzyme binding domain, catalytic domain, and oligomerization domain (Fig. S1 B) (3,15). The dimer is stabilized by the formation of contacts between the equivalent αG-helices (residues 247–260) and parallel β-sheet formation between the β18- and β19-strands (Fig. S1, A and B). Glu487 substituted in the variant is located on the β19-strand and forms salt bridges with Arg264 from the same subunit and Arg475 from the opposing subunit in the dimer (Fig. S1, B and C). The binding site of the coenzyme, NAD⁺, is located between the αG-helix and the spatially adjacent αF-helix. Whereas the adenosine ring portion establishes a contact between the residues arising from the two α-helices at the protein surface, the nicotinamide ring portion is inserted toward the catalytic center, Cys302 (Figs. S1, A and B and S2, A and B).

The complexed NAD⁺ adopts at least two distinct conformations because of the flexible nature of the nicotinamide ring portion in wild-type (WT) ALDH2 (Fig. S2 B; (3,15,16)). One is an extended conformation, termed as a “hydride transfer” conformation (HTC), which is essential for reduction by an enzyme-substrate adduct during a reaction process (16). In this structure, the conformational configuration of the nicotinamide ring is stabilized by hydrogen bonding interactions between the carbonyl oxygen of Leu269 and the amino group in the ring and those between the side chain of Glu399and the nicotinamide ribose oxygens (Fig. S2 C). The second one is a contracted conformation, termed as the “hydrolysis” conformation (HC), in which the nicotinamide ring is shifted from the position of HTC toward the bulk solvent (Fig. S2, B and C). This structural configuration is identical to complexed NADH (15), implying its irrelevance in NAD⁺-mediated catalytic process. The flexible property of the complexed NAD⁺ has been explored by solution NMR technique and fluorescence microscopy (16). Intriguingly, transferred NOESY experiments implied the existence of an additional conformation arising because of an NOE peak between the ribose H_N2 and H_N3′ protons in the nicotinamide ring (Fig. S2, A and B), which could not be accounted for when compared with the available crystal structures.

Crystallographic study of the E487K variant in the apo form (Protein Data Bank, PDB: 1zum) indicates that the dynamic property in its extensive region is likely to be perturbed by the amino acid substitution because the interpretable electron densities are missing, not only in the region spatially adjacent to Lys487 but also in the relatively distal αG-helices (Fig. S3; (2)). This conformational perturbation is likely to also affect the bound state of NAD⁺, as shown by the crystal structure of the E487K variant, in which only HC is observed (2). These experimental observations substantially emphasized the need for structural investigation focusing on their dynamic nature in solution. In this regard, a molecular dynamics (MD) study on the WT ALDH2 and E487K variant in the absence of a coenzyme was recently reported (17). The results reveal that the E487K mutation induces several structural perturbations, including the elevation of the solvent-accessible surface area and loss of residual interactions involved in the catalytic center Cys302. Nevertheless, it is still unclear how their dynamics changes are associated with the experimental observations, such as the loss of electron density at the NAD⁺ binding region, conformational selectivity of the bound NAD⁺, and dominant effect of the E487K mutation on the catalytic activity.

In this study, we carried out MD simulations of WT ALDH2 and its E487K variant complexed with NAD⁺ in HTC or HC on a microsecond order to investigate the impact of the mutation on functionally relevant dynamics, including the intra- and intermolecular dynamic correlations and NAD⁺ binding. The E487K mutation significantly deviated the local conformations of the αG-helices, which is consistent with previous crystallographic observations. Dynamical network analyses (18) demonstrated that Glu487 and the αG-helices were dynamically connected, and the inter-residue communications spanned the overall molecule. The E487K mutation exhaustively rearranged them to diversify the overall motion, accompanied with alteration in the relative positions of the subunits. The perturbations in the dynamic property impaired the NAD⁺ binding in HTC, but not in HC. Further, an additional conformation implicated only in the NMR experiments was found as an intermediate state between the crystallographically observed conformations (HTC and HC) and the more loosely bound state. These insights into the dynamic nature of ALDH2 would provide clues to restore the proper function of its dominant negative mutant.

Methods

MD simulation

The initial coordinates of ALDH2 complexed with NAD⁺ in HTC and HC were derived from PDB: 1o00 (15). The N-termini of all the protein models were capped with acetyl groups, and Mg²⁺ ions were removed. The distance between Na⁺ ions and the coordinating atoms was restrained because the previous crystallographic study pointed that the coordination was essential for NAD⁺ recognition. To clearly extract the effect of the amino acid substitution without any artifacts, the E487K mutation was introduced into the structure of WT ALDH2 using the Structure Preparation module in the Molecular Operating Environment (MOE, Chemical Computing Group, Montreal, Canada), version 2016.08. Addition of the hydrogen atoms and generation of the topology files were carried out by using a pdb2gmx module in the GROMACS package 2016.5 (19). The electrostatic potential for NAD⁺ was calculated at the RHF/6–31G^∗ level using the General Atomic and Molecular Electronic Structure System (GAMESS) program (20), after which the atomic partial charges were assigned by the restrained electrostatic potential (RESP) approach (21). The partial charges of NAD⁺ were found to be similar to those published in the Amber database (Fig. S4; (22,23)). Other parameters for NAD⁺ were determined by the general Amber force field (GAFF) using the antechamber module of AMBER Tools (24,25). All MD simulations were carried out in periodic boundary conditions by using GROMACS 2016.5 (19) on high-performance computing infrastructure equipped with NVIDIA GeForce GTX 1080 GPGPUs. The Amber ff99SB-ILDN force field was used for proteins and ions (26), and TIP3P was used for water molecules (27). Approximately 48,000 water molecules were placed around the complex model with an encompassing distance of 10 Å to form a 132 × 124 × 108 Å³ periodic box. Counterions were added to neutralize the system. Electrostatic interactions were calculated using the particle mesh Ewald (PME) method (28) with a cutoff radius of 10 Å, and a nonbonded cutoff of 10 Å was used for Van der Waals interactions. The P-LINCS algorithm was used to constrain all bond lengths (29). Flat-bottom potentials with force constants of 10 kJ/mol/Ų were applied to restrain the distances between Na⁺ ions and their coordinating atoms during the simulation. After energy minimization of the fully solvated systems, the resulting systems were equilibrated for 100 ps using a constant number of molecules, volume, and temperature condition (NVT) and run for 100 ps, employing a constant number of molecules, pressure, and temperature condition (NPT), with the heavy atoms of the protein and coenzyme held in fixed positions. The temperature was maintained at 298 K with velocity rescaling with a stochastic term (30), and the pressure was maintained at 1 bar with the help of Parrinello-Rahman pressure coupling (31), with temperature and pressure time constants set to 0.1 and 2 ps, respectively. Next, 1-μs production runs with three different initial velocities for four species—WT in HTC, E487K in HTC, WT in HC, and E487K in HC—were carried out under the NPT condition without positional restraints, resulting in generations of 12 trajectories. The MD trajectories were analyzed by the GROMACS package (19), PyMOL (DeLano Scientific, LLC, South San Francisco, CA), VMD (32) and High Throughput Molecular Dynamics (HTMD) environment 1.14.0 (33). The trajectories of the dimer and monomer were generated by concatenating those of the equivalent dimers and all the subunits, respectively, and aligning them with overall Cα atoms. The monomer trajectories were used for calculations of the root mean-square fluctuation (RMSF), distances between the nitrogen atom of NHⁱ and the oxygen atom of CO^{i + 4} at the αG-helices, and conformational analyses of the complexed NAD⁺. The dimer trajectories were used for generation of principle component analysis (PCA)-derived protein motion, analyses of dynamic cross correlation between each pair of Cα atoms, and dynamical network analyses. The tetramer trajectories were used to calculate the radius of gyration, dynamical network analysis, and massively parallel computation of absolute biding free energy with well-equilibrated states (MP-CAFEE). Because the protein behaviors in HTC- and HC-initiated simulations were nearly identical, the data obtained from HTC are mainly shown in this article.

Dynamical network analyses

Dynamical network analyses, which identify communities formed by the residues densely interconnecting each other during the simulations, were carried out by a previously described procedure (18,34). Each amino acid was assigned as a node centered on a Cα atom, and edges between nodes were defined as relationships that showed that the two residues were within 4.5 Å for at least 75% of the whole trajectory. The weights of the edges were derived from pairwise correlation data calculated by the program Carma (35), followed by definition of the dynamical communities, in which the residues within a same community are strongly interconnected, based on the Girvan-Newman algorithm (36).

PCA

The dimer and monomer trajectories were used for PCAs of the dimer and the complexed NAD⁺ conformations, respectively. Constructions and diagonalizations of the mass-weighted covariance matrices were carried out by using a covar module in the GROMACS package 2016.5 after the main chains in the dimer/monomer were structurally aligned. The projections onto the first and second principal components (PC) were carried out by using an anaeig module in the GROMACS package. Protein motions in the dimer were analyzed by extracting the second principal mode calculated from PCAs. The free energy surface (in kcal/mol) of the complexed NAD⁺ in WT were calculated using the conformational populations on the PCA plane, using the following equation: ΔG = RTln(P) − RTln(P_min), where R is gas constant, T is temperature, P is the population at the indicated area, and P_min is the minimal population among them. The population was obtained by counting the number of snapshots assigned in a rectangular area with widths of 2 for a PC1 axis and 1 for PC2 axis.

MP-CAFEE

MP-CAFEE is a double annihilation method to calculate the protein-compound binding free energy (37). In this method, a sufficient number of intermediate states (λ) between the bound (λ = 0) and unbound (λ = 1) states are defined, and multiple MD simulations are performed at each λ point. After the free energy difference between neighboring λ points is calculated by the Bennett acceptance ratio method (BAR) (38), each free energy difference is accumulated into total free energy differences upon λ = 0 → 1. Previously, we have shown that MP-CAFEE successfully ranks the binding affinity of compounds toward several target proteins (39,40) and quantifies the mutation-induced drug sensitivity changes in several medical fields (41,42). MP-CAFEE was carried out by using the previously determined simulation parameters (39), in which Coulomb and van der Waals interactions between an NAD⁺ molecule in the tetramer and other molecules were decoupled at 11 and 21 λ points, respectively. For each λ, six independent 2-ns simulations were performed with different initial velocities. The calculation errors were estimated as SD of ΔG_bind across the six independent simulations.

Results

E487K-mutation-induced collapse of the dynamic connectivity between Glu487 and the αG-helix

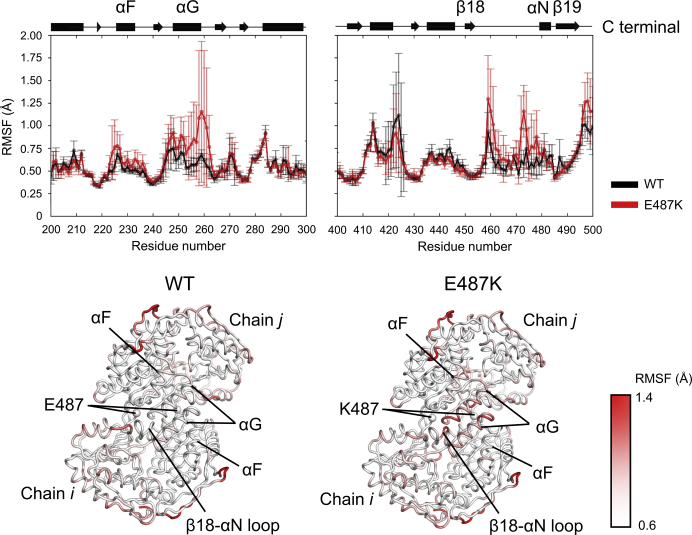
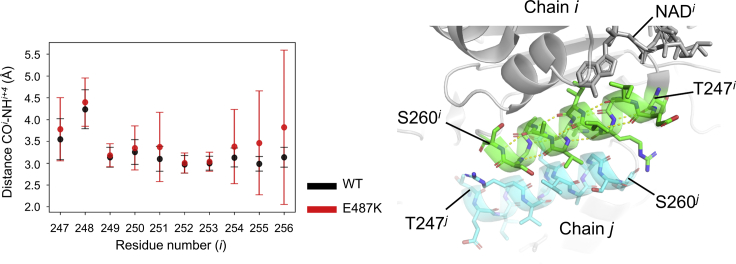
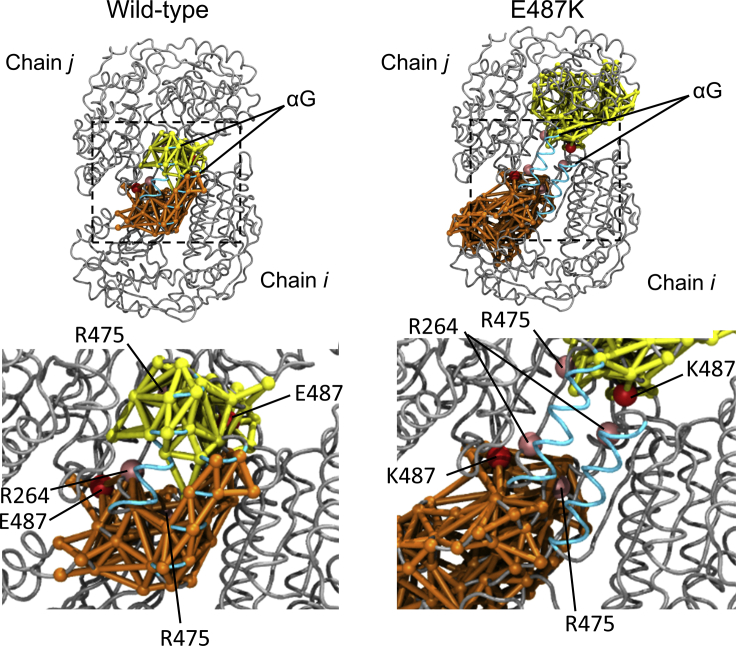
To investigate the dynamics behaviors of WT and its E487K variant, we performed 1-μs MD simulations of their complexes with NAD⁺ in HTC or HC state. The RMSF analyses demonstrated that the E487K mutation significantly elevated the flexibilities in the long loop connecting the β18-strand and the αN-helix, which is spatially adjacent to Lys487 (Figs. 1 and S5). Furthermore, RMSF-values of the αG- and αF-helices in the variant, which are relatively distal (∼20 Å) from the substituted residue, were higher than those in WT (Figs. 1 and S5). Among them, the αG-helices are involved in both the recognition of NAD⁺ and the intermolecular contact to form an equivalent dimer. To confirm the conformational stability of the helix, we assessed the distances characterizing the formation of an α-helix (i.e., the distance between the backbone oxygen atoms of COⁱ and the nitrogen atoms of NH^{i + 4} in the αG-helix). In this analysis, the pairs of Gln254-Gly258, Val255-Ser259, and Ala256-Ser260 were significantly deviated in the variant, whereas those in WT were stable (Fig. 2), implying that the E487K mutation destabilized the local structure of the helix. This observation raised the question as to why the single amino acid substitution affects the conformational state of the distal helix. This distal effect would imply the existence of the dynamics connectivity between Glu487 and the αG-helix. To clarify this point, we performed the dynamical network analysis, which identifies communities formed by the residues densely interconnecting each other during the simulations (18), for dimer trajectories in WT and its E487K variant. The analysis for WT demonstrated that the networks were partitioned into 19 tightly interconnected subnetworks called community networks (Fig. S6). The αG-helices belonged to the community containing Glu487 (Glu487 community), indicating that they were dynamically connected (Fig. 3, left). On the contrary, in the E487K variant, the corresponding community (Lys487 community) was rearranged so as to spread toward the opposite direction; consequently, the direct communication between Lys487 and the αG-helices was no longer available (Fig. 3, right).

Figure 1.

Impact of E487K mutation on residual fluctuations. The RMSF-values of the Cα atoms were calculated by using the monomer trajectories. The values for wild-type (WT) ALDH2 and the E487K variant complexed with NAD⁺ in HTC are indicated by black and red lines, respectively (upper panel). A diagram representing the secondary structure elements in WT (PDB: 1o00) is shown at the top, in which α-helices and β-strands are indicated by rectangles and arrows, respectively. The error bars in the upper panels indicate the SDs derived from the 12 trajectories of the monomer (= four subunits × three independent simulations). The RMSF-values are mapped onto the MD-derived mean structures of the dimers by a gradual color change, from white (0.6 Å) to red (1.4 Å). The individual subunits are denoted as “Chain i” and “Chain j.” The RMSF-values were nearly identical with those of the proteins complexed with HC (Fig. S5). Therefore, data obtained from the trajectories in HTC are shown hereafter in this article, unless otherwise stated. To see this figure in color, go online.

Figure 2.

Conformational heterogeneity in backbone structure of the αG-helix. The distances between the backbone oxygen atom of COⁱ and the nitrogen atom of NH^{i + 4} in WT and E487K are colored by black and red, respectively (left). The error bars indicate the SDs for all of the subunits. The close-up view of the αG-helices in WT ALDH2 (PDB: 1o00) is shown in the right panel. The helices are colored green and cyan. The residues forming the helices are represented by stick models, and the salt bridges formed between them are shown by yellow dashed lines. To see this figure in color, go online.

Figure 3.

Distribution of Glu487 community (left) and Lys487 community (right) in the dimers. The communities of each subunit are individually colored as orange or yellow and are superposed onto the initial model of the simulations. The individual subunits in the dimer are denoted in Fig. 1 as well. Cα atoms of Glu/Lys487 (red) and their interacting partners in WT, Arg264 and Arg475 (pink), are indicated by sphere models. The αG-helices are colored cyan. The lower panels show close-up views of the regions indicated by the dashed rectangles in the upper panels. To see this figure in color, go online.

Alteration in the global conformational dynamics induced by collapse of the interconnected Glu487 communities

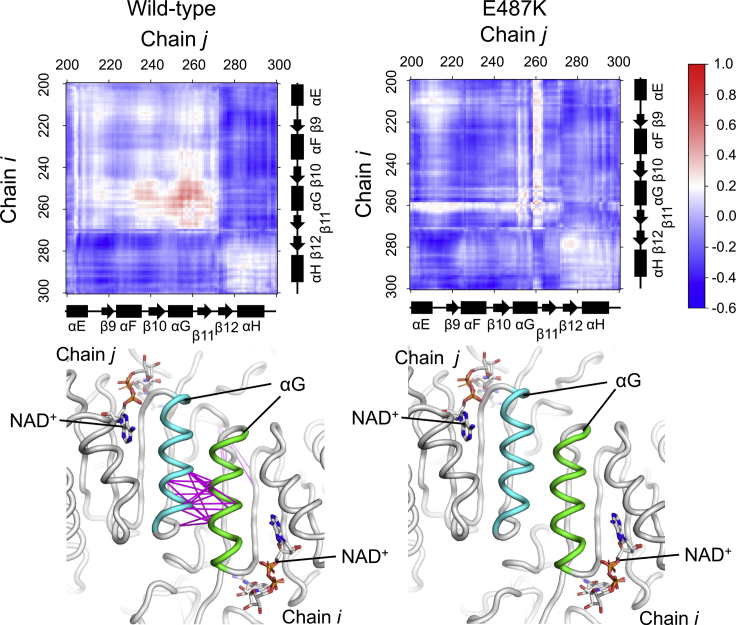
To further investigate the effect of the E487K mutation on the dynamic motion associated with the multimer formation, dynamic cross correlation analyses were performed for the dimers. The equivalent αG-helices in the dimer of WT exhibited concerted motion during the simulations, as shown in the dynamic cross correlation plot (Figs. 4 and S7, left). This characteristic dynamic behavior was associated with Glu487 because the Glu487 communities in each subunit were interconnected at the terminal ends of the helices (Fig. 3, left). The Glu487 communities in the dimer were further interconnected with those present in the opposing dimer and consequently spanned all of the subunits in the homotetramer (Fig. 5). These observations indicated that the Glu487 community contributed toward maintenance of a global conformational motion. In the variant, the dynamic correlation at the dimer interface was eliminated because of rearrangement of the networks (Fig. 4), suggesting that the E487K mutation induced the global conformational deviation.

Figure 4.

Dynamic cross correlation between equivalent subunits in the dimer. In the upper panels, the coefficient values (Cij) of WT (left) and the E487K variant (right) are shown by a gradual color change from blue (−0.6) to red (1.0) for the regions close to dimer interfaces, which are indicated by black rectangles on the overall plots shown in Fig. S7. The residue numbers of the individual subunits (Chain i and Chain j) are labeled at the left and top. The secondary structure elements, in which α-helices and β-strands are indicated by rectangles and arrows, respectively, are schematically represented at the right and bottom with respect to those in WT ALDH2. The correlations having Cij larger than 0.5 are shown in the lower panels, depicted as magenta lines on the average backbone structure of the dimers during the simulations. The αG-helices in the individual subunits are colored green and cyan. NAD⁺ is indicated by stick models. To see this figure in color, go online.

Figure 5.

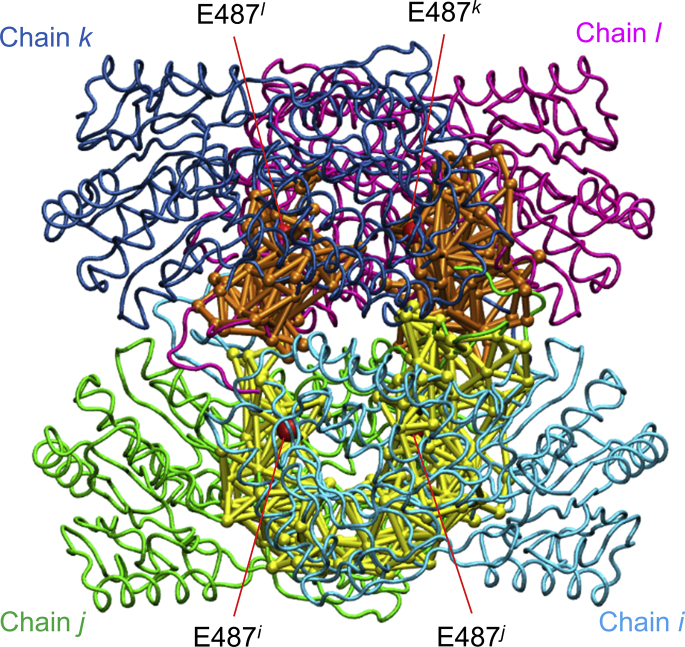
Distribution of Glu487 community in the homotetramer. Individual subunits in the tetramer are denoted as “Chain i,” “Chain j,” “Chain k,” and “Chain l” and colored green, cyan, magenta, and blue, respectively. The Glu487 community in each equivalent dimer is individually colored as orange or yellow. Cα atoms of Glu487 are shown as red spherical models. To see this figure in color, go online.

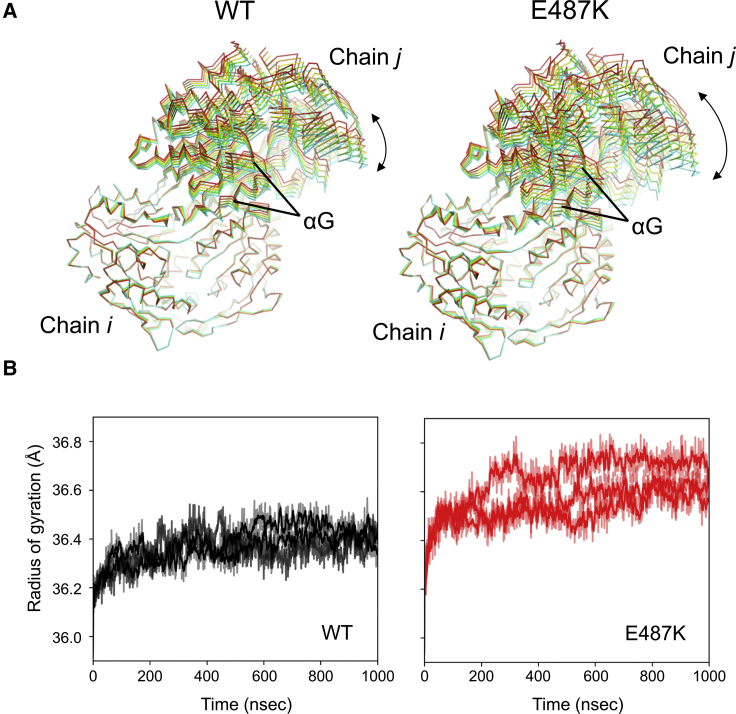
PCAs of the overall structure by using the dimer trajectories showed that the emerging structures in the trajectories of the variant were more broadly spread on the PCA plane than WT (Fig. S8). Motion of the PCA-derived protein along the second eigenvector showed that the E487K mutation led to a conformational diversification by changing the relative positions of the subunits through hinge motion (Fig. 6 A). On the other hand, the conformational preferences seem to be similar between WT and the E487K mutant in solution because their mean backbone structures of the conformations emerging during the simulations were well superimposed with the global root mean-square deviation (RMSD) value of 0.61 Å (Fig. S9 A). This is consistent with the static crystal structures in which the conformational difference in the relative position was also observed (15,43) but the extent was less obvious than that observed in the simulated structures (Fig. S9 B). The conformational fluctuation in the dimer may have further affected a global conformational motion in the homotetramer. This was supported by destabilization of the radius of gyration in the variant (Fig. 6 B).

Figure 6.

Global conformational fluctuation induced by E487K mutation. (A) Dynamic properties of the second principal mode in the dimer calculated using principle component analyses (PCAs). The individual subunits are denoted in Fig. 1 as well. Each structure is colored red, orange, yellow, green, or cyan. (B) Time-dependent transitions of the radius of gyration of the tetramers during the simulations. The individual transition curves calculated from three independent simulations are shown. Black and red lines indicate WT and the E487K mutant, respectively. To see this figure in color, go online.

Impairment of coenzyme binding accompanied with E487K-induced perturbation of the dynamic property

The regions covered by the Glu487 community are involved in NAD⁺ binding. Especially, Leu269, which forms a hydrogen bonding interaction with the amino group in the nicotinamide ring (Fig. S2 C), is a member of this community (Fig. S10). These observations prompted us to investigate the bound state of NAD⁺.

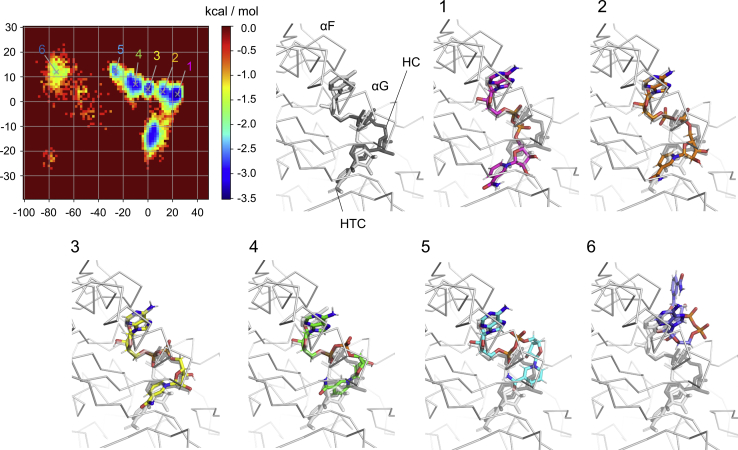
The PCA-based free energy surfaces of the NAD⁺ conformations emerging in the simulation of WT revealed that HTC was the most stable state (1 in Fig. 7), and at least five states existed during the course from the initial HTC toward the unstable states (2–6 in Fig. 7). Assessment of the representative conformations with the lowest free energy in each state showed that destabilization of HTC was initiated by the dissociation of the nicotinamide ring (2 in Fig. 7), to move toward HC (3 in Fig. 7) and the more loosely bound states (4 and 5 in Fig. 7), followed by the destabilization of the adenine ring binding (6 in Fig. 7). Intriguingly, the NMR-observed additional conformations, whose atomic distance between H_N2 and H_N3′ got closer within less than 5 Å (Figs. S2, A and B; (16)), were found to have relatively stable states (4 and 5 in Figs. 7 and S11). A representative conformation shows that the nicotinamide ring was slightly shifted from the position in HC toward the bulk solvent, which further rotated to place H_N2 closer to H_N3′.

Figure 7.

Conformational states of the complexed NAD⁺ emerging in the MD simulations of WT ALDH2. PCA for the NAD⁺ conformations was carried out using WT trajectories obtained from HTC- and HC-initiated simulations. The free energy surface (in kcal/mol) was calculated from the conformational populations in each region on the PCA plane. The plots are gradually colored according to the free energy values as indicated by the color bar. The representative conformations labeled in the PCA plots are shown by stick models, which are superimposed onto a backbone trace of the crystal structure of WT ALDH2 (PDB: 1o00) and the complexed HTC (gray) and HC (black). The colors of the carbon atoms of the simulated NAD⁺ conformations are identical to those of the labels in the PCA plots. The representative conformations are those close to HTC (1), with a slight shifting of the nicotinamide ring toward the bulk solvent (2), close to HC (3), with <5-Å distance between H_N2 and H_N3′ (4 and 5), and with a dissociation of the nicotinamide ring from the protein surface and shifting of the adenosine ring toward the bulk solvent (6). To see this figure in color, go online.

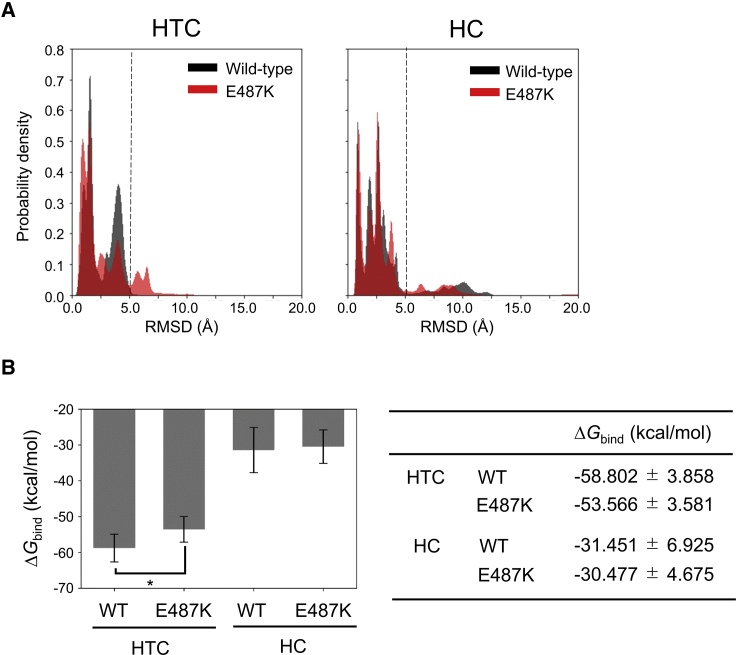
Distribution of the RMSD-values for NAD⁺ in HTC-initiated trajectories demonstrated that significant conformational displacements (larger than 5 Å) occurred only in the variant (Fig. 8 A, left), whereas no significant difference was observed in those in HC-initiated trajectories (Fig. 8 A, right). These observations indicate that the mutation impairs the bound state in HTC. To quantitatively explore the effect of the mutation on the bound states of NAD⁺, their binding free energies (ΔG_bind) were calculated using MP-CAFEE (37), which is an MD-based alchemical free energy perturbation method to successfully rank the binding affinities of small molecules in a relative manner (39, 40, 41, 42). The calculated binding free energy of HTC in the variant (ΔG_bind = −58.802 ± 3.858 kcal/mol) was significantly lower than that of WT (ΔG_bind = −53.566 ± 3.581 kcal/mol), whereas those of HC and WT were nearly identical (Fig. 8 B), supporting the mutation-induced destabilization effect unique to HTC.

Figure 8.

Binding stability of the complexed NAD⁺ in WT and the E487K variant during MD simulations. (A) Histogram-based distribution of RMSD-values for complexed NAD⁺ in WT (black) and E487K (red). The RMSD-values were calculated from the initial structure of the coenzymes. The distributions derived from HTC- and HC-initiated simulations are shown in the left and right panels, respectively. The value of 5 Å is indicated by a dashed line. The distribution of the RMSD-values from HTC for the HTC-initiated trajectories demonstrated that the conformations with the values more than 5 Å were uniquely present only in the variant, whereas those for HC-initiated trajectories showed no significant difference. (B) Binding free energy of HTC and HC calculated by MP-CAFEE. The average values are indicated by black boxes, and the error bars indicate SDs across six independent simulations (left panel). ΔG_bind in WT was significantly lower than that in the E487K variant for HTC, whereas those for HC were nearly identical. The calculated values are shown in the right panel. ^∗p < 0.005 (t-test). To see this figure in color, go online.

Discussion

Although it has been implicated that the drastic inactivation induced by the E487K mutation in ALDH2 is attributed to impairment of NAD⁺ binding and reduced protein stability, it is unclear why such a single amino acid substitution potently changes the overall property from the point of view of focusing on the dynamic behavior. The recent MD study of ALDH2 in the absence of a coenzyme with 100-ns duration successfully captured the mutation-induced perturbation in the protein dynamics (17). Considering the transition curve for the radius of gyration, which reached an equilibrium phase at around 400 ns (Fig. 6 B), in our system modeled from the NAD⁺-bound form, a 100-ns MD simulation is presumed to be insufficient for investigating the relationship between the perturbation in the dynamics and the bound state of NAD⁺. Indeed, significant differences in the conformational distribution of the coenzyme between WT and E487K variant were not observed within 100 ns, whereas the mutation-induced loss-of-dynamics correlation in the αG-helices were observed (Fig. S12, A and B). In the trajectory with the duration of 400 ns, the difference in the conformational distribution was clearly identified, as well as in the analysis using 1000 ns trajectories, albeit less pronounced, indicating that at least a 400-ns trajectory would be required for leading to our conclusion (Fig. S12 C).

Our study comprehensively showed that the extensive impact of the E487K mutation was attributed to the collapse of the Glu487 community connecting Glu487 to the αG-helices. The perturbations in the dynamics are less likely to affect the conformational preference but significantly deviate the conformational distributions (Figs. 6 A, S8, and S9). This agreed well with the observation that the conformational changes observed in the static crystal structures were relatively small compared with the simulated structures. Because the Glu487 community in the individual subunits are mutually interconnected, the impact of the substitution, even in one subunit, would readily spread toward other subunits, consequently perturbing the overall dynamics property to potently impair the activity of the mutated ALDH2 in a dominant fashion. The perturbations were accompanied with diversification of the local structures in the αG-helices (Figs. 1 and 2), which would cause a loss of their interpretable electron density in the crystal structure of E487K (Fig. S3; (43)).

Conformational analyses of the complexed NAD⁺ and ΔG_bind calculation clearly showed that the E487K mutation impaired the stability of the catalytically essential state, HTC (Fig. 8). This HTC-unique effect agreed well with a previous observation, in which only the HC state was available on the crystal structure of E487K complexed with NAD⁺ (PDB: 2onm) (2). In WT, Leu269, which stabilizes the conformational configuration of the nicotinamide ring in HTC (Fig. S2 C), belongs to the Glu487 community, indicating that the conformational change of Leu269 through the mutation-induced loss of coupling with residue 487 would hinder the stable binding of HTC, thus impairing catalytic activity. These results indicate that regulation of the Glu487 community to stabilize the HTC state would enable restoration of the proper function of the dominant negative mutant.

Our simulation study also showed the existence of the NAD⁺ conformation, observed only in NMR experiments (16), as a relatively stable state (4 and 5 in Fig. 7), supporting the fact that our calculations reproduce the behavior of ALDH2 in solution. Because the nicotinamide ring and the neighboring ribose were shifted from their position in the HC state toward the bulk solvent, the conformation was assigned as an intermediate state between crystallographically observed conformations and the loosely bound state (6 in Fig. 7). The increase in mobility in the nicotinamide moiety would hinder the crystallographic observation.

This MD-based observation guides an experimental strategy to further understand the structural connectivity in ALDH2 and its involvement in the function. Amino acid substitution of a residue, which is part of the Glu487 community, may impair the catalytic activity by perturbing the connectivity. The side chain of Leu269, which contributes to stabilization of NAD⁺ conformation in HTC, occupies the hydrophobic pocket formed by Thr247, Leu262, and Leu267 in the crystal structure of WT (Fig. S13). The substitution by a residue with a smaller side chain would destabilize the NAD⁺ binding and is presumed to impair the activity in a dominant fashion by propagation of the effect through the dynamics connectivity spanning the subunits. Because such a mutation is presumed to impair the hydride transfer, the kinetic analysis to identify the rate-limiting step during the catalysis would be also useful to validate these insights. Experimental validations of information obtained from the dynamical network analysis will help to understand the molecular basis of ALDH2 function and further clarify the target site of the small molecule to restore function.

Author Contributions

S.M., M.A., and Y.O. designed the study. S.M., M.A., Y.I., F.O., and Y.O. performed the calculations and analyzed the data. M.A. and Y.O. conceived the idea for the project and wrote the work with S.M. All the authors reviewed the results and approved the final version of the manuscript.

Editor: Alan Grossfield.