Differential impact of exogenous and endogenous attention on the contrast sensitivity function across eccentricity

Abstract

Both exogenous and endogenous covert spatial attention enhance contrast sensitivity, a fundamental measure of visual function that depends substantially on the spatial frequency and eccentricity of a stimulus. Whether and how each type of attention systematically improves contrast sensitivity across spatial frequency and eccentricity are fundamental to our understanding of visual perception. Previous studies have assessed the effects of spatial attention at individual spatial frequencies and, separately, at different eccentricities, but this is the first study to do so parametrically with the same task and observers. Using an orientation discrimination task, we investigated the effect of attention on contrast sensitivity over a wide range of spatial frequencies and eccentricities. Targets were presented alone or among distractors to assess signal enhancement and distractor suppression mechanisms of spatial attention. At each eccentricity, we found that exogenous attention preferentially enhanced spatial frequencies higher than the peak frequency in the baseline condition. In contrast, endogenous attention similarly enhanced a broad range of lower and higher spatial frequencies. The presence or absence of distractors did not alter the pattern of enhancement by each type of attention. Our findings reveal how the two types of covert spatial attention differentially shape how we perceive basic visual dimensions across the visual field.

Introduction

Contrast sensitivity, the ability to discriminate visual patterns from a uniform background, is a fundamental measure of visual function that depends substantially on the pattern's spatial frequency (SF) and eccentricity (Campbell & Robson, 1968; De Valois, Morgan, Snodderly, 1974; Hilz & Cavonius, 1974; Kelly, 1977; Owsley, 2003; Robson, 1966; Robson & Graham, 1981; Rovamo Virsu, & Näsänen, 1978; Virsu & Rovamo, 1979). Covert spatial attention (henceforth attention)—the prioritization of discrete spatial locations in the absence of eye movements—enhances contrast sensitivity for a wide range of spatial frequencies and at several eccentricities (Barbot, Landy, & Carrasco, 2011, Barbot, Landy, & Carrasco, 2012; Cameron, Tai, & Carrasco, 2002; Carrasco, Penpeci-Talgar, & Eckstein, 2000; Carrasco & McElree, 2001; Dosher & Lu, 2000a; Dosher & Lu, 2000b; Fernández et al., 2019; Foley & Schwarz, 1998; Herrmann, Montaser-Kouhsari, Carrasco, & Heeger, 2010; Huang & Dobkins, 2005; Lee et al., 1997; Lee, Itti, Koch, & Braun, 1999; Ling & Carrasco, 2006a; Ling & Carrasco, 2006b; Liu, Pestilli, & Carrasco, 2005; Lu, Lesmes, & Dosher, 2002; Lu & Dosher, 1998; Lu & Dosher, 2000; Morgan, Ward, & Castet, et al.,1998; Morrone, Denti, & Spinelli, 2002; Morrone, Denti, & Spinelli, 2004; Pestilli, Viera, & Carrasco, 2007; Pestilli & Carrasco, 2005; Smith, Wolfgang, & Sinclair, 2004; Solomon, 2004; Solomon, Lavie, & Morgan, 1997; for reviews see, Carrasco, 2006, Carrasco, 2011; Carrasco, 2014). However, very few studies have directly compared the effects of exogenous (involuntary) and endogenous (voluntary) attention on contrast sensitivity (Barbot et al., 2012; Herrmann et al., 2010; Ling & Carrasco, 2006b; Lu & Dosher, 2000), and no single study has jointly manipulated the SF and eccentricity of their stimuli. Given that the SF and eccentricity of a stimulus have profound effects on contrast sensitivity, attention may operate differentially across each dimension. Indeed, the effects of attention on spatial resolution—an important limiting factor for visual performance—are related to modulations of contrast sensitivity that vary across eccentricity (for reviews see, Anton-Erxleben & Carrasco, 2013; Carrasco & Barbot, 2014; Carrasco & Yeshurun, 2009). However, direct measures of attentional effects on contrast sensitivity across eccentricity are lacking. Thus, whether and how exogenous and endogenous attention alter contrast sensitivity across SF and eccentricity remain open questions. We systematically address these questions here. We measured the effects of exogenous and endogenous attention using the same task and observers to provide fundamental knowledge about how attention shapes the perception of basic visual dimensions across the visual field.

It is well established that contrast sensitivity varies systematically with the SF and eccentricity of a stimulus. The contrast sensitivity function (CSF) characterizes an individual's ability to reliably discriminate different levels of SF at a given eccentricity. At the fovea, contrast sensitivity is maximal for SFs between two and six cycles per degree (cpd) and declines sharply for lower and higher frequencies, resulting in the typical bandpass shape of the CSF (Campbell & Robson, 1968; Kelly, 1977; Owsley, 2003; Robson, 1966). At farther eccentricities, the CSF remains bandpass, but overall sensitivity declines and peak sensitivity shifts to lower SFs (e.g., Hilz & Cavonius, 1974; Rovamo et al., 1978; Virsu & Rovamo, 1979). These features of the CSF are attributed to spatial characteristics of the cone mosaic, retinal, and striate cells, as well as the temporal characteristics of visual stimuli (for reviews see, DeValois & DeValois, 1990; Graham, 1989; Kelly, 1977) and oculomotor processes that generate small fixational eye movements (Casile, Victor, & Rucci, 2019). Overall, the CSF encapsulates the sensitivity of quasi-independent “channels,” each tuned to individual SFs with a bandwidth of roughly one to two octaves (Blakemore & Campbell, 1969; Graham, 1989). Each channel acts as a filtering mechanism that analyzes the visual scene into its SF components and primarily reflects the aggregation of optical and neural factors that contribute to the perception of SFs (DeValois & DeValois, 1990; Graham, 1989).

Attention has been widely demonstrated to increase contrast sensitivity across the CSF (Cameron et al., 2002; Carrasco et al., 2000) and separately at several eccentricities (Barbot et al., 2011; Barbot et al., 2012; Carrasco, 2006; Carrasco & McElree, 2001; Dosher & Lu, 2000a, 2000b; Fernández, Li, & Carrasco, 2019; Foley & Schwarz, 1998; Herrmann et al., 2010; Huang & Dobkins, 2005; Lee, Koch, & Braun, 1997; Lee, Koch, & Braun, 1999; Ling & Carrasco, 2006a; Ling & Carrasco, 2006b; Liu et al., 2005; Lu et al., 2002; Lu & Dosher, 1998; Lu & Dosher, 2000; Morgan et al., 1998; Morrone et al., 2002, 2004; Pestilli et al., 2007; Pestilli & Carrasco, 2005; Smith et al., 2004; Solomon, 2004; Solomon et al., 1997). On the perceptual level, such attentional modulation is governed by two mechanisms: signal enhancement and external noise reduction. The signal enhancement mechanism strengthens the neural representation of an attended stimulus and yields its largest effects when stimulus displays are devoid of external noise sources (Bashinski & Bacharach, 1980; Cameron et al., 2002; Carrasco et al., 2000, 2002; Ling & Carrasco, 2006b; Lu & Dosher, 1998, 2000; Luck et al., 1996; Smith et al., 2004). External noise reduction encapsulates two nonmutually exclusive operations: noise exclusion and distractor suppression. According to noise exclusion, attention operates via a perceptual template that filters out distracting visual input that overlap with the target stimulus (Dosher & Lu, 2000a, 2000b; Lu et al., 2002; Lu & Dosher, 1998, 2000, 2005). Distractor suppression posits that attention diminishes the impact of distractors outside the attended location and exhibits more pronounced effects as the number of distractors increases (Baldassi & Burr, 2000; Cameron et al., 2004; Eckstein & Whiting, 1996; Foley & Schwarz, 1998; Morgan et al., 1998; Palmer, 1994; Solomon et al., 1997; Verghese, 2001). Both forms of external noise reduction yield their largest effects when displays contain sources of external noise, and in this study we assessed the distractor suppression mechanism in particular.

Both signal enhancement and distractor suppression mechanisms underlie the effects of two distinct types of attention, exogenous and endogenous (e.g., Ling & Carrasco, 2006b; Lu & Dosher, 2000). Exogenous attention operates at short timescales (100–120 ms) and is engaged by salient, peripheral cues that automatically and transiently orient attention to the cued location. Endogenous attention operates on longer time-scales (≥ 300 ms), is deployed voluntarily, and can be sustained at a location specified by a symbolic cue (for reviews see, Carrasco, 2006; Carrasco, 2011; Carrasco, 2014; Carrasco & Barbot, 2014). Both types of attention are associated with partially overlapping cortical networks (e.g., Beck & Kastner, 2009; Corbetta & Shulman, 2002; Dugué, Merriam, Heeger, & Carrasco, 2018) and often have similar effects on visual perception, but can differ (Barbot et al., 2012; Hein, Rolke, & Ulrich, 2006; Jigo & Carrasco, 2018; Sharp et al., 2018; Yeshurun, Montagna, Carrasco, 2008; Yeshurun & Levy, 2003).

Here, we investigate the effects of both exogenous and endogenous attention on the CSF at several eccentricities using an orientation discrimination task. We use the orientation dimension because it has been well characterized both psychophysically and neurophysiologically, and there is an established link between findings obtained with these two approaches (DeValois & DeValois, 1990; Graham, 1989; Regan & Beverley, 1985; Ringach, Hawken, & Shapley, 1997). Orientation discrimination is used to assess the effect of attention on stimulus contrast because performance on this task is monotonically contingent on contrast (Nachmias, 1967; Pestilli, Ling, & Carrasco, 2009; Skottun, Bradley, Sclar, Ohzawa, & Freeman, 1987), and because fMRI response increases monotonically with stimulus contrast (Boynton, Demb, Glover, & Heeger, 1999). Furthermore, the nonlinear (i.e., saturating) contrast response of neural populations has been linked to psychophysical performance and to the effects of attention in this orientation discrimination task (Pestilli et al., 2009). Importantly, both types of attention, eccentricity, SF, and contrast were independently manipulated while keeping observers, task, and stimuli constant. In addition, because adding distractors to a stimulus display may amplify the effects of attention, targets were displayed alone or among distractors in separate experiments to assess whether signal enhancement and distractor suppression mechanisms distinctly modulate the CSF across the visual field.

To briefly preview our results, we found that exogenous attention preferentially enhanced SFs higher than the peak frequency in the baseline condition. In contrast, endogenous attention similarly enhanced a broad range of lower and higher SFs than the peak frequency in the baseline condition. These distinct patterns of attentional benefits occurred at each eccentricity regardless of whether the target appeared alone or among distractors. Overall, we provide evidence that exogenous and endogenous attention differentially shape fundamental visual ability.

Experiment 1

Experiment 1 was designed to examine the effects of exogenous and endogenous attention on contrast sensitivity to sinusoidal gratings of six possible SFs presented at four possible eccentricities within the fovea, parafovea, perifovea and periphery. Gratings were presented alone, and location uncertainty was minimized via response cues. This experimental design enabled us to assess the signal enhancement mechanism of attention while systematically investigating the effects of attention on contrast sensitivity across SF and eccentricity.

Methods

Participants

Ten observers participated in Experiment 1 (aged 21–35 years, five female). All observers provided written informed consent under the protocol approved by the University Committee on Activities involving Human Subjects at New York University. All experimental procedures were in agreement with the Declaration of Helsinki. Observers had normal or corrected-to-normal vision, and all, except author M.J., were naïve to the purpose of the experiment. Six observers were compensated at a rate of $10/hr, and four observers volunteered. To assess the presence of outlier data, we computed the overall cueing effect (grand-average cueing effect across SF and eccentricity) for each observer. The difference between an observer's overall cueing effect and the median across the group indexed their similarity to the group. Cueing effects that were ≥ ±3 median absolute deviations (Leys, Ley, Klein, Bernard, & Licata, 2013) from the group were considered outliers. One observer's exogenous cueing effects deviated significantly from the group and, given the repeated measures design of our study, his data were removed from both exogenous and endogenous attention conditions. Thus the results reported here are based on nine participants.

Sample size estimation

We estimated an a priori sample size using data from previous studies that measured the effects of exogenous (Cameron et al., 2002; Carrasco et al., 2000) and endogenous attention (Ling & Carrasco, 2006b) on contrast sensitivity using similar experimental designs. To accomplish this, we used a bootstrap approach (McConnell & Vera-Hernández, 2015). Two to twelve observers were randomly resampled, with replacement, from each study's dataset and an analysis of variance (ANOVA) was conducted on the resampled data. For Carrasco et al., 2000 and Cameron et al., 2002, two-way (cue × SF) repeated measures ANOVAs were performed. For Ling and Carrasco, 2006, a one-way (cue) repeated measures ANOVA was performed. This process was repeated 10,000 times and separate p value distributions were constructed for the main effect(s) and, when applicable, the interaction. Power was computed as the proportion of significant (p < 0.05) effects for each p value distribution and power greater than 0.8 was considered sufficient (Cohen, 1988). Assuming we would observe cueing effects of a similar magnitude (on average, a 15% improvement in contrast sensitivity due to attention for each study), across all three studies we found that a sample size of nine was the largest needed to yield sufficient power for the main effect of cue.

Apparatus

Stimuli were generated on an Apple iMac using MGL (http://justingardner.net/mgl), a set of OpenGL libraries running in MATLAB (Mathworks, Natick, MA, USA), and displayed on a cathode-ray tube (CRT) monitor (1280 × 960; 100 Hz). The monitor was gamma-corrected using a Konica Minolta LS-100 (Konica Minolta, Tokyo, Japan). Observers viewed the display binocularly with their heads stabilized by a chin-and-head rest positioned 79 cm away from the monitor. Eye position was monitored monocularly at 500 Hz using an Eyelink 1000 (SR Research, Ottawa, Ontario, Canada).

Stimuli

Target stimuli were sinusoidal gratings with a center SF of 0.5, 1, 2, 4, 8, or 11 cpd. Gratings were windowed by a two-dimensional raised cosine function that was 4° wide (full-width-at-half-maximum: 2°) and centered on the peak luminance (i.e., white stripe) for each grating. As a result, each SF was displayed with a minimum of two cycles within the window, which ensured near asymptotic spatial summation for each SF (Banks, Sekuler, & Anderson, 1991; Estevez & Cavonius, 1976; Hoekstra, Van der Goot, Van den Brink, & Bilsen, 1974; Howell & Hess, 1978) and a distribution of power in the SF domain that was narrowly centered on the nominal SFs (Kelly, 1977). In sum, the spatial characteristics of our grating stimuli enabled frequency-specific measures of contrast sensitivity. Each grating was randomly presented in either the left or right hemifield along the horizontal meridian at 0°, 3°, 6°, or 12° eccentricity (seven total possible locations). Gratings were tilted ±45° and displayed at a minimum of five levels of Michelson contrast (henceforth contrast), which were determined separately for each combination of eccentricity and SF for each observer (see “Procedure”).

Two types of spatial cues were used: pairs of white dots (56 cd/m²; diameter, 1°) displayed 3.75° above and below the horizontal meridian, or a white “N” and integers (56 cd/m²) ranging from 0 to 3 (0.5° × 0.5°) along with white line(s) (0.5° × 0.25°) displayed 2° above the horizontal meridian. The lines were displaced horizontally from the center of the alphanumeric characters by 0.35°. The white integers and lines served as precues to manipulate endogenous attention. The white dots served as both precues to manipulate exogenous attention and response cues that equated location uncertainty across cueing conditions. Response cues signaled observers to respond (see “Procedure”). Observers fixated a dim and gray (17 cd/m²) central “X” subtending 0.35° visual angle, and all stimuli were presented on a medium gray background (26 cd/m²) throughout the experiment.

Behavioral tasks

Orientation discrimination task

We measured contrast sensitivity using a two-alternative forced-choice (2AFC) orientation discrimination task (Figure 1). Observers were required to discriminate between orthogonal (±45°) orientations at several values of contrast. In addition to the reasons outlined in the Introduction, our decision to use orientation discrimination rather than yes-no detection is motivated by the following considerations. First, when orientations differ by more than 20°, observers are equally accurate at discriminating between them as they are detecting each against a uniform background (Thomas & Gille, 1979). Thus a large orientation difference between stimuli equates discrimination and detection performance. Similar conclusions have been drawn from experiments that presented near-threshold stimuli and required observers to discriminate between and detect SFs (Furchner, Thomas, & Campbell, 1977; Nachmias & Weber, 1975; Watson & Robson, 1981) and motion directions (Watson et al., 1980). Second, measurements of contrast sensitivity using detection, motion direction discrimination, and orientation discrimination tasks in the same observer resulted in equivalent CSFs (Virsu & Rovamo, 1979). Importantly, the measures of contrast sensitivity obtained from each task exhibited the typical variations with SF and eccentricity. Third, 2AFC tasks are relatively unbiased compared to yes-no tasks in which threshold estimates may be contaminated by the observer's decisional criterion (Green & Swets, 1973; Yeshurun, Carrasco, et al., 2008).

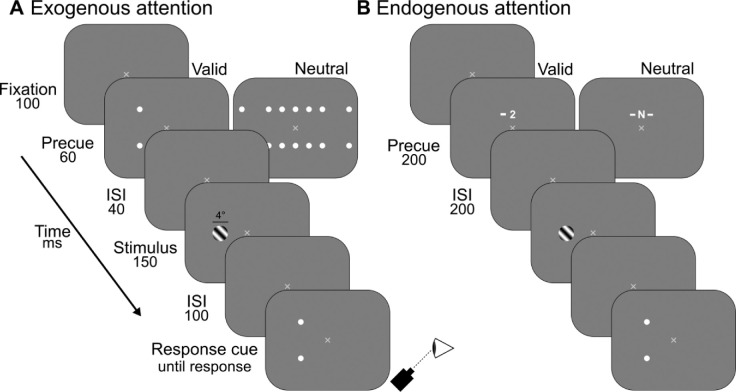
Figure 1.

Experiment 1 trial sequence. (A) Schematic for exogenous attention condition. Observers discriminated clockwise (+45°) versus counterclockwise (−45°) tilts of a sinusoidal grating presented with one of six spatial frequencies (0.5–11 cpd) at four possible eccentricities (0°, 3°, 6°, 12°). Grating contrast was manipulated via the method of constant stimuli. Precues directed attention to a specific location (Valid) or distributed attention across the visual field (Neutral). Observers were seated 79 cm away from the display, and their eyes were tracked to ensure stable fixation. The horizontal black line was not displayed during the experiment. (B) Schematic for endogenous attention condition. Duplicate timing information was omitted from B.

Exogenous attention condition

On trial onset, observers fixated a central “X” for 100 ms after which a precue was presented for 60 ms (Figure 1A). On half the trials a Neutral precue was displayed consisting of seven pairs of white dots, each centered on a possible target location. For each dot pair, one dot was above and the other below the potential target location (see “Stimuli”). This cue informed observers of the temporal onset of the target, but provided no prior information about its location. The Neutral condition served as the baseline. On the other half of trials, a 100% Valid precue was displayed consisting of a single pair of white dots centered on the upcoming target location. Following a 40 ms interstimulus interval (ISI), a single oriented grating was displayed at one of seven possible locations for 150 ms. After a 100 ms ISI, a response cue was presented consisting of a single pair of white dots centered on the target location. The response cue equated location uncertainty between Neutral and Valid cue conditions, and indicated that observers should respond. Observers were instructed to use the left or right arrow keys on a keyboard to report whether the grating was tilted to the left or right of vertical, respectively. We instructed observers to be as accurate as possible, without time stress, and auditory feedback was provided for incorrect responses. At the end of each block, observers were shown their overall accuracy (percent correct) as visual feedback.

Endogenous attention condition

To facilitate direct comparisons between the effects of exogenous and endogenous attention, the task design was identical to that described above with the following exceptions regarding cue parameters. Such changes were necessary to appropriately manipulate endogenous attention (Figure 1B). Following the initial fixation period, a precue was presented for 200 ms, followed by a 200 ms ISI. On half the trials, a Neutral precue was displayed consisting of a white letter “N” along with white lines on both sides. This cue indicated the temporal onset of the target but gave no information about its location. On the other half of trials, a 100% Valid precue was displayed consisting of a white integer (0–3) and a single line to the right or left of the number. Similar to previous studies (Barbot & Carrasco, 2017; Jigo & Carrasco, 2018; Yeshurun, Montagna, et al., 2008), the line indicated which hemifield the target would appear and the number indicated the target's eccentricity: 0 represented the fovea and 1 through 3 indicated eccentric locations 3°, 6°, and 12° respectively. For foveal targets, no lines were presented with the Valid precue. The foveal location was tested twice as often to equate the number of trials with that of the eccentric locations.

Procedure

Preliminary estimate of contrast threshold

Given that contrast sensitivity varies considerably across SF and eccentricity (e.g., Robson, 1966; Virsu & Rovamo, 1979), observers completed a preliminary session in which an initial estimate of contrast threshold, the contrast required to achieve 70% discrimination accuracy, was obtained for each combination of SF and eccentricity (24 thresholds total). Measures of contrast sensitivity were then defined as the reciprocal of contrast thresholds. The accuracy level (70%) was arbitrarily chosen to define preliminary contrast thresholds as it falls within the dynamic range of observers’ psychometric functions. Before the experimental sessions, each observer completed 1440 trials (60 trials/threshold) of the Neutral condition over 10 blocks. The contrast on each trial was adjusted by a weighted one-down/one-up staircase that initially displayed gratings at 15% contrast. After each incorrect response, grating contrast increased (step-up) by 0.24 log₁₀ units, and after each correct response, contrast decreased (step-down) by 0.1 log₁₀ units. This step-size ratio (step-up:step-down = 2.4) ensured that the staircase converged at ∼70% accuracy (García-Pérez, 1998). Preliminary threshold estimates were computed as the average contrast (in log₁₀ units) across the last five trials of the staircase run. For half of the observers, preliminary estimates were obtained using the Neutral condition for exogenous attention, and for the other half they were obtained using the Neutral condition for endogenous attention.

Method of constant stimuli

We differentiate between contrast levels and contrast values. Whereas contrast levels denote an experimental parameter in our task design, contrast values denote the precise amount of grating contrast (e.g., 10% contrast). Within each session, performance was always assessed at only five contrast levels for each combination of SF and eccentricity, but contrast values could differ across conditions and sessions. Contrast values were determined as follows. To get an accurate measure of the upper asymptote in performance (Prins, 2012), gratings were presented at 100% contrast. The remaining four contrast values for a given experimental session were ±0.075 and ±0.225 log₁₀ units from the preliminary threshold estimate. For preliminary estimates that were within 0.225 log₁₀ units of 100% contrast (i.e., ≥ 60% contrast), the four contrast values were 0.15 to 0.6 log₁₀ units smaller than the preliminary estimate (equally spaced in steps of 0.15 log₁₀ units). For some observers, the initial contrast values tested did not completely span the dynamic range of their psychometric functions. In these cases, contrast values were adjusted by the experimenter and further experimental trials were performed in separate experimental sessions. Across the group and across experimental sessions, observers were tested at a total of five to 13 contrast values for each combination of SF and eccentricity conditions. Each observer completed 40 ± 3 trials per contrast value.

Experimental block structure & task order

Both exogenous and endogenous attention tasks adhered to the same block structure. Each block contained 160 trials. Overall, observers completed 12,000±1329 trials total across 5 ± 0.55 experimental sessions for each type of attention. Within each block, SF (six levels), eccentricity (four levels), hemifield (two levels), orientation (two levels), contrast (five levels), and cue (two levels) were randomly interleaved. As a result, the probability of encountering any combination of these conditions was uniform across all trials. Thus observers had no foreknowledge of the SF, contrast, and orientation of the target on a given trial. The order of exogenous and endogenous attention tasks was counterbalanced across observers.

Eye tracking

Observers were instructed to maintain fixation until response cue onset. If a blink or eye movement >1° occurred, the trial was immediately aborted, and a tone was played, reminding observers to maintain fixation. These aborted trials were rerun at the end of the block.

Data analysis

Model

To characterize each observer's psychometric functions, CSF, and pattern of attentional modulation across SF, we used the following model (Figure 2) that consisted of the three components described below. Each component is motivated by well-known characteristics of contrast sensitivity. First, the monotonic increase in discrimination performance with stimulus contrast was modeled by a Naka-Rushton function (e.g., Herrmann et al., 2010; Huang & Dobkins, 2005; Ling & Carrasco, 2006b; Pestilli et al., 2009). Second, the conventional bandpass shape of the CSF was modeled by a double-exponential function (Movshon & Kiorpes, 1988; Wang, Wang, Huang, Zhou, & Tzvetanov, 2017) and generated the values of contrast threshold used by the Naka-Rushton function. By constraining contrast thresholds to a functional form of the CSF, we greatly reduced the number of parameters required to explain each observers’ dataset while adhering to known characteristics of the human visual system. Third, we tested two distinct hypotheses of the pattern of attentional modulation across SF: one in which modulation is selective for a narrow range of frequencies (Gaussian) and another in which modulation is equivalent for a broad range of frequencies (Plateau). These hypotheses are motivated by studies that exhibit attentional modulations that automatically enhance a range of high spatial frequencies (Carrasco et al., 2006) or flexibly adjust sensitivity based on task demands (Barbot & Carrasco, 2017) and uniformly modulate sensitivity to SF (Lu & Dosher, 2004).

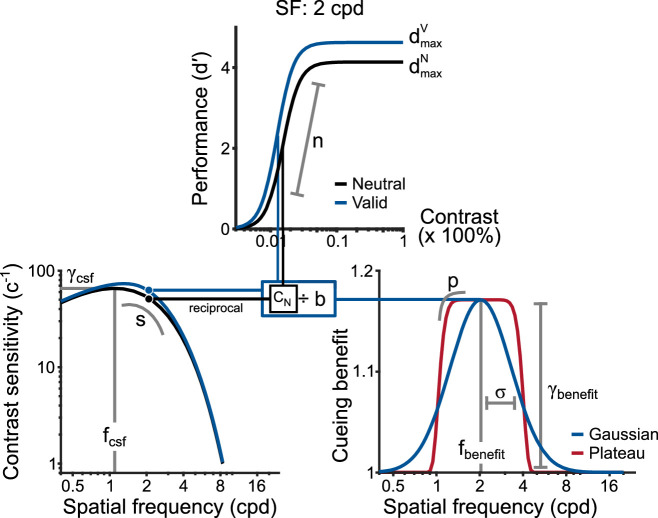
Figure 2.

Graphical depiction of analysis model for Experiment 1. (Top) Neutral and Valid psychometric functions, shown here for a 2 cpd grating at a single eccentricity, were modeled as Naka-Rushton functions whose slope was controlled by n and fixed between cues and SFs. The upper asymptote for each cueing condition was defined by $d_{max}^N$and $d_{max}^V$, respectively. Contrast threshold, c—the level of contrast required to reach half-maximum performance—in the Neutral condition (vertical black line) was determined by a model of the contrast sensitivity function. (Bottom-left) Contrast sensitivity functions for individual eccentricities were modeled as double-exponential functions. f_csf defined the SF where sensitivity was highest, γ_csf defined peak sensitivity, and s controlled the slope of the function about the peak. The reciprocal of sensitivity values served as contrast threshold for the Neutral condition. Valid contrast thresholds (vertical blue line) were determined by scaling Neutral thresholds via an attention modulation function. (Bottom-right) Two candidate models were compared: Gaussian (blue) and Plateau (red). Each was generated from a raised Gaussian function in which f_benefit defined its center, γ_benefitdefined its amplitude, σ controlled its width, and p determined its shape (Gaussian, p = 2; Plateau, p > 2). Attention modulation functions were defined across spatial frequency (on a log-axis). The scalar, b, for a given frequency determined the magnitude of cueing benefits. In this example, the Gaussian model was used to modulate the Neutral CSF, which yielded the Valid CSF, and determined the leftward shift of the psychometric function for the Valid condition.

Psychometric function. Performance at each contrast value was defined in terms of d′: z(hit rate) − z(false alarm rate). As in previous studies (e.g., Herrmann et al., 2010; Zhang, Morrone, & Alais, 2019), hits were (arbitrarily) defined as counter-clockwise (CCW) responses to CCW tilts (−45°) and false alarms as CCW responses to a clockwise tilt (+45°). To avoid infinite values when computing d′, we followed the conservative log-linear rule in which 0.5 was added to the number of hits, misses, correct rejections, and false alarms before computing d′ (Brown & White, 2005; Hautus, 1995). Responses were collapsed across hemifields before computing d′. We also evaluated performance as proportion correct; our results were not impacted by the performance measure used. Results using d′ are reported. A Naka-Rushton function characterized performance across contrast values, c, for each SF, f.

$$d_f^A\left(c\right)=d_{max}^A\frac{c^n}{c^n+{\left[c_{50}^A\left(f\right)\right]}^n}$$ (1)

The superscript A denotes the attentional cueing condition, which could either be N for Neutral or V for Valid. d_max defined the upper asymptote and n controlled the function's slope. c₅₀defined the contrast value at which half-maximum performance was reached and served as the measure of contrast threshold. c₅₀ in the Neutral condition varied as a function of SF as defined by a functional form of the CSF described below.

Neutral CSF. Contrast thresholds for all SFs in the Neutral condition were governed by a double-exponential function (adapted from Movshon & Kiorpes, 1988; Wang et al., 2017) of the form:

$$c_{50}^N\left(f\right)={\left({mf}^{\frac{f_{csf}}{s}}{e}^{-\frac{f}{s}}\right)}^{-1}$$ (2)

where f_csf defines the peak SF of the CSF (i.e., the SF where contrast sensitivity is highest) and s controls the slope of the function about its peak. The peak amplitude of the CSF (γ_csf) is defined by the following:

$${\gamma }_{csf}=m{f_{csf}}^{\frac{f_{csf}}{s}}{e}^{-\frac{f_{csf}}{s}}$$

Attention modulation. Attentional benefits on contrast threshold were instantiated by scaling Neutral contrast threshold:

$$c_{50}^V\left(f\right)=\frac{c_{50}^N\left(f\right)}{b\left(f\right)}$$

The magnitude of attentional benefits, b, across SF was modeled by a raised Gaussian function of the form:

$$b\left(f\right)={\gamma }_{benefit}{e}^{-{\left(\frac{f-f_{benefit}}{\sigma }\right)}^p}+\delta$$ (3)

where γ_benefit defines the maximum attentional benefit, f_benefitdefines the SF at which the maximum benefit occurs, σ controls the spread of the function, p controls its shape, and δ controls the baseline which was fixed at 1. When p = 2 the function is equivalent to a Gaussian, and when p > 2 the function remains constant about the peak, resembling a plateau.

Model specification and fitting procedure

The model was fit to each observer's performance across contrast values, SFs, and eccentricities. At a minimum, the model needed to capture the pattern of performance across 5 contrast values × 6 SFs × 4 eccentricities × 2 cues (240 conditions total).

The model fit to the data had 77 free parameters. All three parameters of the Neutral CSF (f_csf, s, m; Equation 2) were free to vary across the four eccentricities (12 parameters). In addition, three parameters for the pattern of attentional modulation (γ_benefit, f_benefit, σ; Equation 3) were allowed to vary across eccentricity whereas p was fixed (13 parameters). Lastly, previous reports have demonstrated that the slope of the psychometric function does not vary across SFs when measured at a single eccentricity (Mayer & Tyler, 1986; Wallis, Baker, Meese, & Georgeson, 2013). Thus, within each eccentricity, the parameter n (Equation 1) was fixed across SFs resulting in four total slope parameters. The upper asymptote of the psychometric functions (d_max; Equation 1) was allowed to vary across SFs, eccentricities, and cues (48 parameters), which allowed us to assess whether the upper asymptote fluctuated with SF and eccentricity, and, importantly, allowed us to test for attentional response gain modulations (Barbot et al., 2011; Barbot et al., 2012; Herrmann et al., 2010; Huang & Dobkins, 2005; Ling & Carrasco, 2006b; Morrone et al., 2002; Morrone et al., 2004; Pestilli et al., 2009).

The MATLAB fmincon function was used to search for the parameters that minimized the sum of squared error weighted by number of trials for each contrast value. The optimization procedure was repeated thirty times using the MATLAB MultiStart function with randomized initial parameters each time. The best fit across iterations was selected.

Model comparisons

We compared two models of attentional modulation defined by Equation 3: Gaussian and Plateau. For the Gaussian model, p was fixed at 2; for the Plateau model, p was allowed to vary between 5 and 10. For the Plateau model, the value of p was fixed across eccentricity within each observer but was allowed to vary across observers. We compared models using the Akaike Information Criterion (AIC), computed with the assumption of a normal error distribution, as it is asymptotically equivalent to model comparison via cross-validation (Burnham & Anderson, 2002):

$$AIC=oln\left(\frac{RSS}{o}\right)+2k$$

where o is the number of observations, RSS is the residual sum of squares, and k is the number of free parameters. To report the AIC index, we computed the difference in AIC values between Plateau and Gaussian models for each observer and then averaged AIC indexes across observers. Positive values reflect the Gaussian model outperforming the Plateau.

Statistical analyses

Repeated measures ANOVAs were used to assess the effects of SF, eccentricity, and attentional cueing conditions. In all cases in which Mauchly's test of sphericity indicated a violation of the sphericity assumption, lower-bound estimate corrections were used (Geisser & Greenhouse, 1958). Effect sizes are reported in terms of generalized eta-squared (${\eta }_G^2$; Bakeman, 2005; Lakens, 2013; Olejnik & Algina, 2003).

Results

Observers performed an orientation discrimination task in which exogenous (Figure 1A) or endogenous attention (Figure 1B) were distributed across eccentricity (Neutral) or directed to a specific location (Valid). Stimulus displays contained no added external noise. Performance (d′) was measured at six SFs, four eccentricities, and, on average, six contrast values per condition across observers, which enabled measures of the CSF across eccentricity. Observers’ Neutral and Valid performance across contrast values were characterized for each SF and eccentricity using the model depicted in Figure 2. Psychometric functions were modeled by Naka-Rushton functions (Figure 2, top) whose thresholds (i.e., contrast level at which performance reached half-maximum) in the Neutral condition were determined by a double-exponential function across SF (Figure 2, bottom-left). Neutral thresholds were then scaled by an attention modulation function (Figure 2, bottom-right) to determine Valid contrast thresholds. Each parameter of the model was iteratively adjusted until the residual sum-of-squares error between observers’ performance and the corresponding Naka-Rushton functions, weighted by the number of trials at each contrast value, was minimized.

This modeling approach was motivated by well-known characteristics of contrast sensitivity and reported attentional modulations on SF. In particular, we modeled the monotonic increase in discrimination performance with increasing stimulus contrast using Naka-Rushton functions (e.g., Herrmann et al., 2010; Huang & Dobkins, 2005; Ling & Carrasco, 2006b; Pestilli et al., 2009). Furthermore, for each eccentricity, we constrained the variation in contrast thresholds across SF by a functional form of the CSF that adheres to its conventional bandpass shape (Movshon & Kiorpes, 1988; Wang et al., 2017). In doing so, we greatly improved the parsimony of our modeling approach while conforming to known variations of contrast sensitivity across SF (Campbell & Robson, 1968; De Valois et al., 1974; Hilz & Cavonius, 1974; Kelly, 1977; Owsley, 2003; Robson, 1966; Robson & Graham, 1981; Rovamo et al., 1978; Virsu & Rovamo, 1979). Lastly, we assessed two distinct hypotheses of the pattern of multiplicative attentional modulation across SF: one in which modulation is selective for a narrow range of frequencies (Gaussian) and another in which modulation is equivalent for a broad range of frequencies (Plateau). These hypotheses instantiate two attentional mechanisms that have been reported previously: one that selectively and preferentially enhances a range of high SFs (Carrasco et al., 2006) and another that flexibly adjusts sensitivity to SF based on task demands (Barbot & Carrasco, 2017) and uniformly modulates sensitivity to SF (Lu & Dosher, 2004). In sum, this modeling approach facilitated model comparisons between explicit hypotheses of attentional modulation and allowed us to explain observers’ performance while adhering to known characteristics of the human visual system.

Covert spatial attention improved contrast sensitivity in the absence of external noise

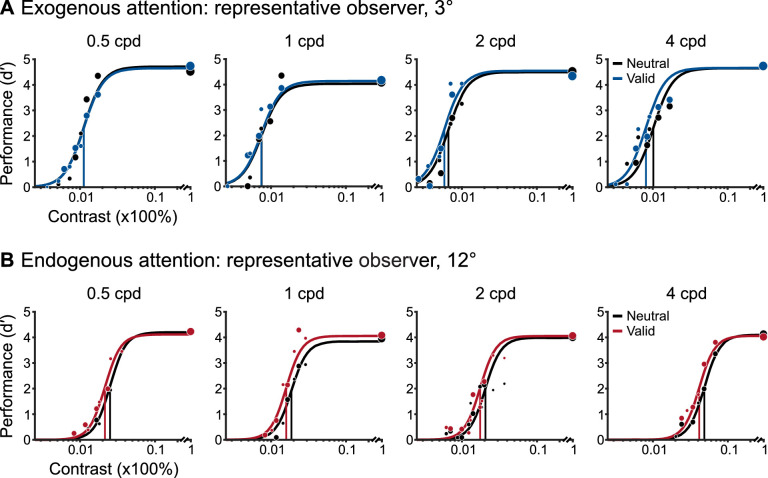
Figure 3 depicts a subset of psychometric functions for representative observers in the exogenous (Figure 3A) and endogenous attention (Figure 3B) conditions. For these representative observers, Neutral contrast thresholds were similar for both types of attention and varied with SF: thresholds decreased to a minimum at 1 cpd and increased thereafter. Critically, attention reduced thresholds (i.e., increased sensitivity) across SF. The largest change due to exogenous attention occurred for 2 and 4 cpd. For endogenous attention, thresholds were similarly reduced for a broader range of SFs between 0.5 and 4 cpd. Thus, for these representative observers, both types of covert spatial attention improved contrast sensitivity in the absence of external noise. Estimates of the upper asymptote (d_max) were not consistently different between Neutral and Valid conditions across SF in either exogenous or endogenous attention conditions.

Figure 3.

Subset of psychometric functions for representative observers’ performance on targets presented at (A) 3° eccentricity in the exogenous attention condition and (B) 12° eccentricity in the endogenous attention condition. The size of individual dots represents the number of trials performed at a given contrast value. Vertical lines depict contrast thresholds.

Neutral contrast sensitivity declined and shifted to lower SFs with eccentricity

On the group-level, contrast sensitivity for the Neutral condition behaved similarly for both exogenous (Figure 4A) and endogenous attention (Figure 4B) conditions. Contrast sensitivity was bandpass across SF, peaking at intermediate SFs and decreasing for lower and higher values. At farther eccentricities, overall contrast sensitivity declined. A two-way repeated measures ANOVA was conducted to assess the effects of SF and eccentricity (independent variables) on contrast sensitivity (dependent variable) in the Neutral condition. Note, when statistical results are reported for each type of attention, “exo” will refer to exogenous and “endo” to endogenous attention. There were significant main effects of SF (exo: F[0.22, 1.74] = 320, p < 10⁻⁷_, ${\eta }_G^2$ = 0.98; endo: F[0.22, 1.74] = 261, p < 10⁻⁶_, ${\eta }_G^2$ = 0.97) and eccentricity (exo: F[0.13, 1.04] = 189, p < 10⁻⁶_, ${\eta }_G^2$ = 0.96; endo: F[0.13, 1.04] = 226, p < 10⁻⁶_, ${\eta }_G^2$ = 0.97), and their interaction (exo: F[0.65, 5.22] = 55.8, p < 10⁻⁴_, ${\eta }_G^2$ = 0.87; endo: F[0.65, 5.22] = 40.5, p < 10⁻⁴_, ${\eta }_G^2$ = 0.84).

Figure 4.

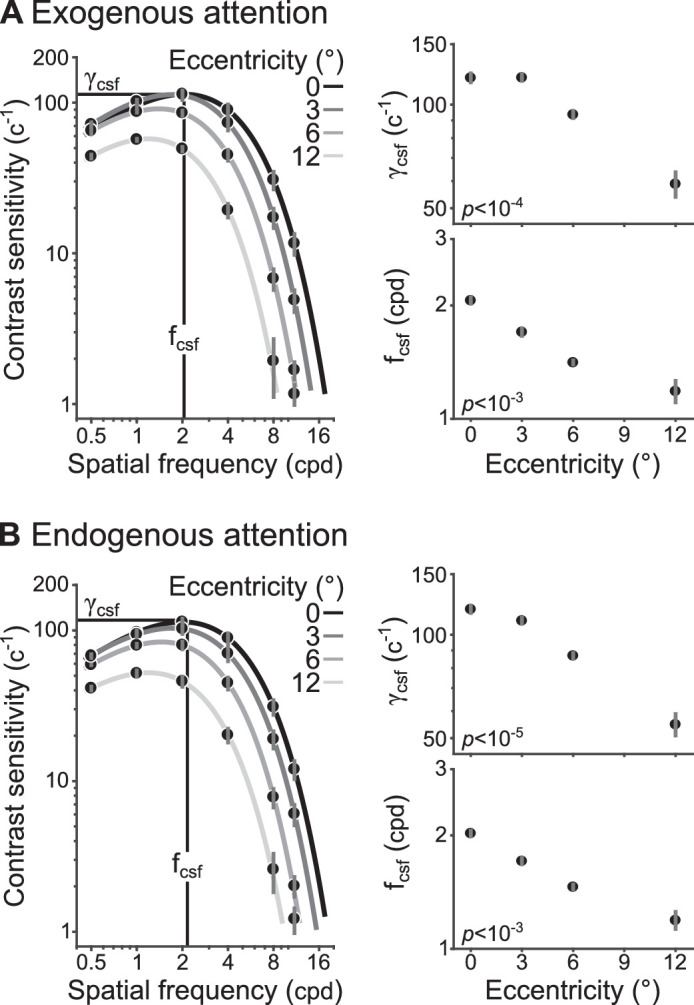
Neutral contrast sensitivity functions for (A) exogenous and (B) endogenous attention conditions. Parameters f_csf (vertical black line) and γ_csf (horizontal black line) are shown for foveal (0°) targets. Estimates for γ_csf and f_csf at each eccentricity are shown in the right column. The p values were determined from one-way ANOVAs assessing the effect of eccentricity on each parameter. Dots show group-average and error bars represent ±1 SEM.

The interaction effect reflected the shift in peak SF with eccentricity. Group-level estimates of the peak SF (f_csf) and peak amplitude (γ_csf) showed that as amplitude decreased at farther eccentricities, peak SF shifted to lower SFs. To assess this observation, one-way repeated measures ANOVAs were conducted to evaluate the effect of eccentricity on f_csf and γ_csf separately. There were significant main effects of eccentricity on f_csf (exo: F[1,8] = 32.2, p < 10⁻³_, ${\eta }_G^2$ = 0.80; endo: F[1, 8] = 34.5, p < 10⁻³_, ${\eta }_G^2$ = 0.81) and γ_csf (exo: F[1, 8] = 73.6, p < 10⁻⁴_, ${\eta }_G^2$ = 0.90; endo: F[1, 8] = 101, p < 10⁻⁵_, ${\eta }_G^2$ = 0.93).

Contrast sensitivity functions in the Neutral condition did not differ between exogenous and endogenous attention conditions across eccentricity. Two-way repeated measures ANOVAs were conducted to assess the effect of attention type (exogenous, endogenous) and eccentricity on f_csf and γ_csf separately. There were main effects of eccentricity on f_csf (F[0.43, 3.43] = 41.7, p < 10⁻³_, ${\eta }_G^2$ = 0.84) and γ_csf (F[0.43,3.43] = 109, p < 10⁻⁵_, ${\eta }_G^2$ = 0.93). Neither the main effects of attention type (f_csf : F[1, 8] < 1; γ_csf: F[1, 8] = 2.42, p > 0.1) nor the interaction effects (f_csf : F[0.43, 3.43] < 1; γ_csf: F[0.43, 3.43] = 1.88, p > 0.2) were significant. Therefore, despite cue location and temporal differences in the manipulation of each type of attention, Neutral contrast sensitivity was equivalent.

We additionally evaluated the impact of SF and eccentricity on d_max in the Neutral condition. d_max was largely determined by performance at 100% contrast, which fell to chance for high SFs, particularly at far eccentricities. A two-way repeated measures ANOVA assessed the effects of SF and eccentricity on d_max in the Neutral condition. There were significant main effects of SF (exo: F[0.22, 1.74] = 33.0, p < 0.001_, ${\eta }_G^2$ = 0.80; endo: F[0.22, 1.74] = 57.4, p < 10⁻⁴_, ${\eta }_G^2$ = 0.88) and eccentricity (exo: F[0.13, 1.04] = 40.8, p < 0.001_, ${\eta }_G^2$ = 0.84; endo: F[0.13, 1.04] = 83.3, p < 10⁻⁴_, ${\eta }_G^2$ = 0.91), and their interaction (exo: F[0.65, 5.22] = 13.8, p < 0.01_, ${\eta }_G^2$ = 0.63; endo: F[0.65, 5.22] = 24.7, p < 0.001_, ${\eta }_G^2$ = 0.76). Thus SF and eccentricity similarly affected Neutral contrast sensitivity and d_max.

Exogenous and endogenous attention improved contrast sensitivity differently

Both exogenous and endogenous attention improved contrast sensitivity across SF and eccentricity. A three-way repeated measures ANOVA was conducted to assess the effects of cue (Neutral, Valid), SF, and eccentricity on contrast sensitivity. There was a significant main effect of cue (exo: F[1, 8] = 34.8, p < 0.001_, ${\eta }_G^2$ = 0.81; endo: F[1, 8] = 41.7, p < 0.001_, ${\eta }_G^2$ = 0.84). The cue × SF (exo: F[0.11, 0.85] = 2.97, p > 0.1; endo: F[0.11, 0.85] = 4.28, p > 0.05), cue x eccentricity (exo: F[0.064, 0.51] = 1.01, p > 0.3; endo: F[3, 24]<1), and three-way interactions (exo: F[0.32, 2.55] = 3.02, p > 0.1; endo: F[0.32, 2.55] = 1.00, p > 0.3) were not significant. All main effects of SF and eccentricity, and their interaction were identical to the Neutral condition described above.

Neither type of attention altered d_max. A three-way repeated measures ANOVA was conducted to assess the effects of cue, SF, and eccentricity on d_max. There was no significant main effect of cue (exo: F[1, 8] < 1; endo: F[1, 8] < 1). The cue × SF (exo: F[5, 40] < 1; endo: F[0.11, 0.85) = 2.67, p > 0.1), cue × eccentricity (exo: F[0.064, 0.51] = 4.12, p > 0.05; endo: F[3, 24]<1), and three-way interactions were not significant (exo: F[15, 120] < 1; endo: F[15, 120] < 1). All main effects of SF and eccentricity, and their interaction, were identical to the Neutral condition described above. Thus exogenous and endogenous attention improved contrast sensitivity across SF and eccentricity without affecting the upper asymptote of the psychometric function.

We compared two models of attentional modulation that could have generated the improvements in contrast sensitivity: Gaussian and Plateau (Figure 2, bottom-right). Whereas the Gaussian instantiated an attentional mechanism that is SF-selective, the Plateau reflected a nonselective attentional mechanism that enhances a broad range of SFs. Akaike Information Criterion (AIC) was used for model comparison (Figure 5). AIC values for the Gaussian model were subtracted from the Plateau model and averaged across observers. Whereas positive values reflect the Gaussian outperforming the Plateau, negative values reflect the Plateau outperforming the Gaussian. We found that the Gaussian outperformed the Plateau model for exogenous attention (AIC_Plateau − AIC_Gaussian = 1.91 ± 0.87). In contrast, for endogenous attention, the Plateau outperformed the Gaussian model (AIC_Plateau − AIC_Gaussian = −1.69 ± 0.87).

Figure 5.

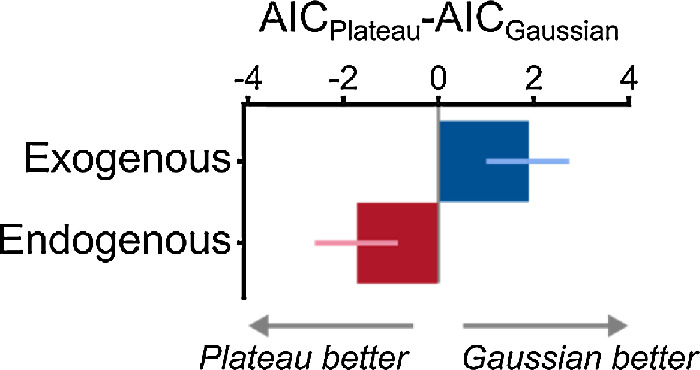
Model comparisons using ΔAIC for Experiment 1. Bars represent group-average ΔAIC, and error bars represent ±1 within-subject SEM (Morey, 2008).

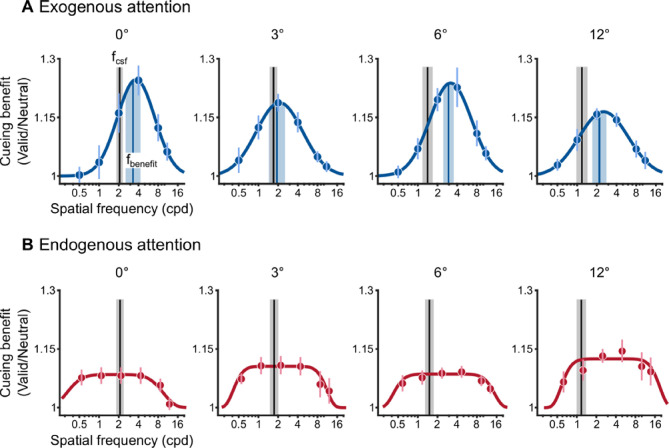
Figure 6 depicts the group-average cueing benefits for exogenous (Figure 6A) and endogenous attention (Figure 6B), each fit with their respective models. Cueing benefits by exogenous attention were selective and peaked at a given frequency (f_benefit). Overall, exogenous attention benefits peaked at SFs higher than f_csf (i.e., the peak SF in the Neutral condition) at each eccentricity (median shift across eccentricity = 0.54 octaves above f_csf). A two-way repeated measures ANOVA was conducted to assess the effect of peak type (f_csf, f_benefit) and eccentricity on peak SF. There was a main effect of peak type (F[1, 8] = 7.02, p = 0.029_, ${\eta }_G^2$ = 0.47), but neither the main effect of eccentricity (F[0.43, 3.43] = 2.18, p > 0.1) nor the peak × eccentricity interaction were significant (F[3, 24] < 1). These results indicate that exogenous attention preferentially enhanced higher SFs at each eccentricity.

Figure 6.

Attention modulation functions across eccentricity. (A) Exogenous attention modulation modeled as Gaussian functions, which were fit to the group-average cueing benefit. Black vertical lines depict group-average peak SF in the Neutral condition (f_csf). Blue vertical lines depict average SF of peak cueing benefit (f_benefit) across observers and were not determined by the fit to the group-average cueing benefit (blue curves). (B) Endogenous attention modulation modeled as Plateau functions, which were fit to the group-average cueing benefit (red curves). Dots show group-average, error bars and shaded regions depict ±1 within-subject SEM (Morey, 2008).

In contrast, endogenous attention similarly improved a broad range of lower and higher spatial frequencies on either side of f_csf. Across the group, improvements by endogenous attention were centered on 3.14 ± 0.33 cpd with equivalent improvements that spread (indexed by σ) to 1.21 ± 0.054 octaves on either side. The center and spread were equivalent across eccentricity, as supported by a one-way repeated measures ANOVA with eccentricity as a factor. The main effect of eccentricity was not significant for either the center (F[3, 24] < 1) or the spread (F[3, 24] < 1) parameters. Thus improvements by endogenous attention spanned a similar range of SFs at each eccentricity despite f_csf falling to lower frequencies in the periphery. As a result, the Plateau functions become centered at SFs higher than f_csf, particularly at 6° and 12°. This, however, is a consequence of our experimental design. We would have likely observed shifts in the center parameter had we extended the range of lower SFs that were tested (i.e., below 0.5 cpd). Nonetheless, the apparent shift in the Plateau functions for endogenous attention should not be confused with the preferential enhancement of higher SFs by exogenous attention. Whereas the effects of endogenous attention improved a broad range of lower and higher SFs to a similar degree, exogenous attention improved higher SFs more prominently than lower SFs.

Experiment 2

In this experiment, we assessed whether and how the presence of distractors would modulate the patterns of attentional modulation that were observed when targets were displayed in isolation. Furthermore, because previous studies have reported that attentional effects become more pronounced when distractors are added to a display (Cameron et al., 2004; Carrasco & McElree, 2001; Carrasco & Yeshurun, 1998; Eckstein & Whiting, 1996; Foley & Schwarz, 1998; Palmer, 1994; Verghese, 2001), this experiment could potentially reveal attentional effects that were not elicited with the sparse displays in Experiment 1. Here four gratings were presented simultaneously, and the task-relevant grating was indicated at the end of each trial by the presentation of a response cue. The task-relevant grating was displayed at one of two eccentricities (2° and 6°), and all gratings had one of eight possible SFs. Furthermore, given that attention effects in Experiment 1 were confined to the dynamic range of the psychometric function, gratings were presented at a single contrast level corresponding to the midpoint of the psychometric function.

Methods

Participants

Ten observers participated in Experiment 2 (aged 18–33 years, seven female). Four observers also participated in Experiment 1. All observers provided written informed consent under the protocol approved by the University Committee on Activities involving Human Subjects at New York University. All experimental procedures were in agreement with the Declaration of Helsinki. Each observer had normal or corrected-to-normal vision, and all, except author M.J., were naïve to the purpose of the experiment. Four observers were compensated at a rate of $10/hr and the others volunteered. This sample size was based on the sample size estimation conducted for Experiment 1.

Apparatus, stimuli, and tasks

Apparatus, stimuli, and tasks were identical to those used in Experiment 1 with the following exceptions: First, SFs were more finely sampled in this experiment to get a more detailed profile of attentional modulation across frequencies; gratings could have one of nine SFs: 0.5, 1, 1.41, 2, 2.83, 4, 8, 11.31, and 16 cpd. Second, to adequately display the highest SFs, observers were seated 115 cm from the monitor. Third, gratings were displayed at 2° and 6° visual angle. These two eccentricities were chosen to prevent any spatial overlap among gratings when displayed. Fourth, four gratings were presented on each trial, one at each eccentricity in each hemifield. Fifth, each SF at each eccentricity was presented at a fixed contrast level within a block. This manipulation allowed us to account for the detrimental impact of distractors at each eccentricity on performance (see “Procedure”). Sixth, Neutral and Valid endogenous cues were displayed for 300 ms followed by a 100 ms ISI. The duration of endogenous cues was extended to give observers more time to perceive and interpret the alphanumeric characters. The ISI was shortened to match the stimulus-onset-asynchrony of Experiment 1 (400 ms).

Procedure

Preliminary estimate of contrast threshold

Preliminary contrast thresholds were obtained for each combination of SF and eccentricity conditions. Before the experimental sessions, each observer completed 1152 to 1440 trials of the Neutral condition over eight to 10 blocks. For half the observers, threshold estimates were obtained from performance in the Neutral exogenous attention condition (Figure 7A), whereas for the other half they were obtained from performance in the Neutral endogenous attention condition (Figure 7B). During each trial, four gratings were displayed. The contrast of each grating was independently updated by two interleaved three-down/one-up staircases (García-Pérez, 1998); one staircase was initialized at 2.5% contrast and the other at 5% contrast. For each staircase, incorrect responses increased (step-up) contrast by 0.32 log₁₀ units, whereas three consecutive correct responses decreased (step-down) contrast by 0.44 log₁₀ units. This step-size ratio (step-up:step-down = 0.73) ensured that each staircase converged at ∼75% accuracy (García-Pérez, 1998). The preliminary threshold estimates were calculated as the average contrast (in log₁₀ units) across the last eight reversals of each staircase. Critically, because distractors were present when thresholds were determined, this procedure adjusted for their detrimental impact on performance. The staircase protocol was adjusted to target the midpoint for accuracy in a 2AFC task, which lies within the dynamic range of the underlying psychometric function.

Figure 7.

Experiment 2 trial sequence. (A) Schematic for exogenous attention condition. Observers discriminated clockwise (+45°) versus counterclockwise (−45°) tilts of a target sinusoidal grating presented among distractors. Gratings were presented with one of nine spatial frequencies (0.5–16 cpd) at two possible eccentricities (2°, 6°). Grating contrast was fixed within a block for each SF and eccentricity. Precues directed attention to a specific location (Valid) or distributed attention across the visual field (Neutral). Observers were seated 114 cm away from the display, and their eyes were tracked to ensure stable fixation. The horizontal black line was not displayed during the experiment. (B) Schematic for endogenous attention condition. Duplicate timing information was omitted from B.

Performance-based updates of contrast threshold

During the experimental sessions, gratings were presented at the same contrast value for Valid and Neutral conditions. Contrast values were updated based on accuracy on the Neutral condition after every 20 trials for each combination of SF and eccentricity conditions (i.e., after five blocks). For each condition in which accuracy (defined in percent correct) deviated from 75%, contrast (c), in log₁₀ units, was adjusted as follows:

$$\Delta c=\left(75-p_{Neutral}\right)\times 0.0125$$

where p_Neutralcorresponds to accuracy on the Neutral condition. Thus, for every 1% deviation, threshold was adjusted by 0.0125 log₁₀ units. This adjustment rate was based on the slope of the contrast response functions from Experiment 1. Final contrast thresholds were computed as the geometric mean of the unique contrast values displayed throughout the experiment.

Experimental block structure & task order

Both exogenous and endogenous attention conditions adhered to the same block structure. Each block contained 144 trials, and observers completed 4320 trials total (120 trials/SF × eccentricity × cue). Within each block, SF (nine levels), eccentricity (two levels), hemifield (two levels), orientation (two levels), and cue (two levels) were randomly interleaved. The order of exogenous and endogenous attention tasks was counterbalanced across observers.

Data analysis

Task performance

As in Experiment 1, task performance was defined in terms of d′. Performance was averaged across hemifields and repeated measures ANOVAs were used to assess the effects of cue, SF, and eccentricity. We also evaluated performance as proportion correct; our results were not impacted by the performance measure used. Results using d′ are reported below. Cueing benefits were defined as the performance difference between Valid and Neutral conditions.

Performance for 16 cpd at 6° visual angle was at floor for eight of 10 observers across both attention conditions. That is, a majority of observers were at 50% accuracy with 100% contrast 16 cpd gratings. Thus, to avoid the influence of floor effects on our statistical analyses, results reported below reflect performance on all (0.5–11 cpd) but the 16 cpd at each eccentricity.

Model specification and comparisons

In a similar manner to Experiment 1, we compared the Gaussian and Plateau models of attentional modulation generated from Equation 3. Whereas the Gaussian model instantiated an attentional mechanism that is selective for a narrow range of SFs, the Plateau instantiated an attentional mechanism that similarly enhances a broad range of SFs. For this experiment, the baseline parameter, δ, was fixed at 0 and p was fixed at 10 for the Plateau model to match the estimates from Experiment 1. Each model was fit to the cueing benefits at each eccentricity. In total, six parameters ([γ_benefit, f_benefit, σ] × 2 eccentricities) were used to fit the 16 conditions (8 SFs × 2 eccentricities). Model comparisons were conducted in an identical manner as Experiment 1.

Statistical analyses

The results of repeated measures ANOVAs are reported as in Experiment 1.

Results

Observers performed an orientation discrimination task in which exogenous (Figure 7A) or endogenous attention (Figure 7B) was distributed across eccentricity (Neutral) or directed to a specific location (Valid). Visual displays contained a stimulus at each of four possible locations (i.e., one target and three distractors). Performance (d′) was measured for eight SFs and two eccentricities with grating contrast fixed to equate performance across SFs and eccentricities.

Neutral performance was equated across SF and eccentricity

CSFs for exogenous (Figure 8A, top) and endogenous attention (Figure 8B, top) were bandpass across SF—peaking at intermediate SFs and decreasing for lower and higher values—and declined between 2° and 6° of eccentricity. The effects of SF and eccentricity on contrast sensitivity were assessed with a two-way repeated measures ANOVA and supported our observations. There were significant main effects of SF (exo: F[0.47, 4.20] = 148, p < 10⁻⁶_, ${\eta }_G^2$ = 0.94; endo: F[0.47, 4.20] = 126, p < 10⁻⁵_, ${\eta }_G^2$ = 0.93) and eccentricity (exo: F[0.067, 0.60] = 17.9, p < 0.01_, ${\eta }_G^2$ = 0.67; endo: F[0.067, 0.60] = 110, p < 10⁻⁵_, ${\eta }_G^2$ = 0.92), and their interaction (exo: F[0.47, 4.20] = 9.91, p < 0.01_, ${\eta }_G^2$ = 0.52; endo: F[0.47, 4.20] = 13.8, p < 0.01_, ${\eta }_G^2$ = 0.61).

Figure 8.

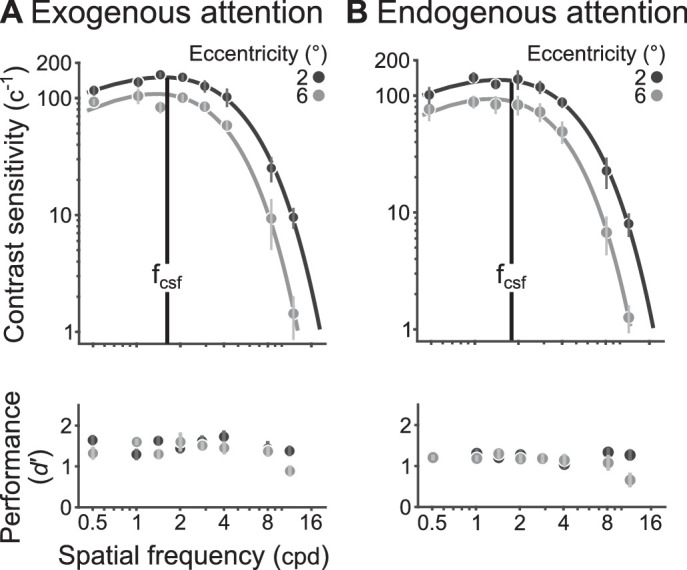
Neutral contrast sensitivity functions (top) and performance (bottom) for (A) exogenous and (B) endogenous attention conditions. f_csf (vertical black line) is shown for 2° targets. Dots show group-average, and error bars represent ±1 within-subject SEM (Morey, 2008).

The interaction effect primarily reflected a sharper decline in sensitivity for high than low SFs and not a shift in peak SF to lower SFs with eccentricity. We characterized the peak SF (f_csf) by fitting a double-exponential function to the CSFs. A repeated measures one-way ANOVA assessed the effect of eccentricity on f_csf and supported this observation. The main effect was nonsignificant for either type of attention (exo: F[1, 9] < 1; endo: F[1, 9] < 1).

Critically, the associated contrast thresholds (i.e., reciprocal of contrast sensitivity) equated Neutral performance across SF and eccentricity for exogenous (Figure 8A, bottom) and endogenous attention (Figure 8B, bottom). The effects of SF and eccentricity on performance were assessed with a two-way repeated measures ANOVA, and their results supported our observations. The main effects of SF (exo: F[0.47, 4.2] = 1.70, p > 0.2; endo: F[0.47, 4.2] = 1.78, p > 0.2) or eccentricity (exo: F[1, 9] = 1.07, p > 0.3; endo: F[1, 9] = 1.38, p > 0.2), and their interaction (exo: F[0.47, 4.2] = 1.28, p > 0.2; endo: F[0.47, 4.2] = 1.91, p > 0.2) were not significant. Thus the staircase procedure successfully equated Neutral performance for targets among distractors.

Exogenous and endogenous attention improved contrast sensitivity differently

Both exogenous and endogenous attention improved performance across SF and eccentricity. A three-way repeated measures ANOVA assessed the effects of cue (Neutral, Valid), SF and eccentricity on performance and supported this observation; there was a significant main effect of cue (exo: F[1, 9] = 6.93, p < 0.05_, ${\eta }_G^2$ = 0.44; endo: F[1, 9] = 5.17, p < 0.05_, ${\eta }_G^2$ = 0.36). The cue × SF (exo: F[0.23, 2.03] = 1.59, p > 0.2; endo: F[7, 63] < 1), cue × eccentricity (exo: F[1, 9] < 1; endo: F[1, 9] < 1), and three-way interactions (exo: F[7, 63] < 1; endo: F[0.23, 2.03] = 1.90, p > 0.2) were not significant. Therefore both exogenous and endogenous attention improved contrast sensitivity for the target across SFs and eccentricity.

We compared the Gaussian and Plateau models of attentional modulation using AIC values (Figure 9). Consistent with Experiment 1, we found that whereas the Gaussian model outperformed the Plateau model for exogenous attention (AIC_Plateau −AIC_Gaussian = 1.72 ± 0.67), the reverse was the case for endogenous attention (i.e., the Plateau outperformed the Gaussian; AIC_Plateau −AIC_Gaussian = −2.13 ± 0.67).

Figure 9.

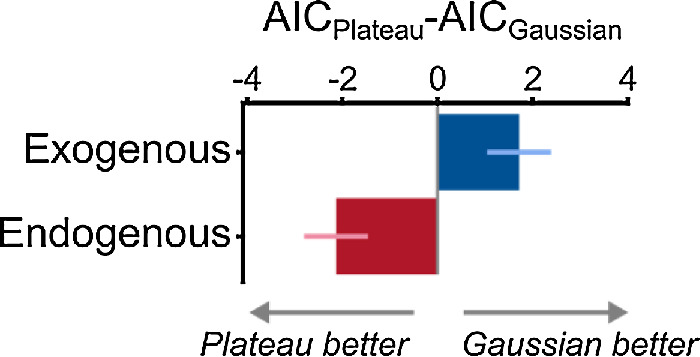
Model comparisons using ΔAIC for Experiment 2. Bars represent group-average ΔAIC, and error bars represent ±1 within-subject SEM (Morey, 2008).

Figure 10 depicts the group-average cueing benefits for exogenous (Figure 10A) and endogenous attention (Figure 10B), each fit with their respective models. Cueing benefits by exogenous attention were selective and peaked at a given frequency (f_benefit). At 2° of eccentricity, f_benefit was relatively near f_csf (median shift = 0.4 octaves above f_csf). However, at 6° the shift was more pronounced with cueing benefits peaking at much higher SFs than f_csf (median shift = 1.9 octaves above f_csf). A two-way repeated-measures ANOVA assessed the effect of peak type (f_csf, f_benefit) and eccentricity on peak SF. There was a main effect of peak type (F[1, 9] = 27.9, p < 0.001_, ${\eta }_G^2$ = 0.76). Neither the main effect of eccentricity (F[1, 9] = 1.92, p > 0.1) nor the interaction (F[1, 9] = 2.34, p > 0.1) were significant. Thus, at each eccentricity, exogenous attention preferentially enhanced SFs higher than the peak SF in the Neutral condition.

Figure 10.

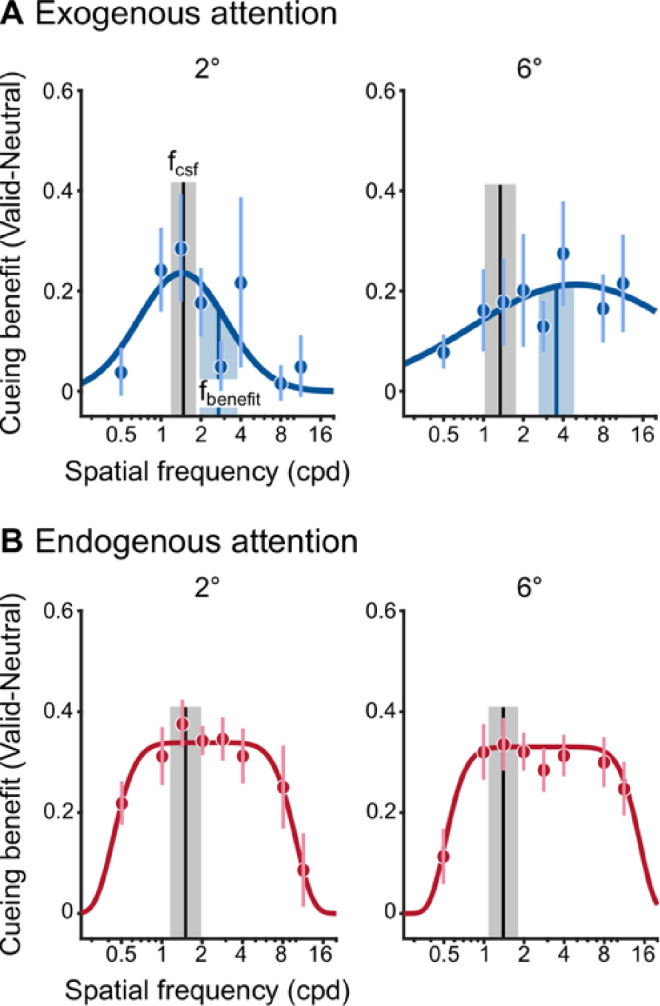
Attention modulation functions across eccentricity. (A) Exogenous attention modulation modeled as Gaussian functions, which were fit to the group-average cueing benefit. Black vertical lines depict group-average peak SF in the Neutral condition (f_csf). Blue vertical lines depict the average SF of peak cueing benefit (f_benefit) across observers, and were not determined by the fit to the group-average cueing benefit (blue curves). (B) Endogenous attention modulation modeled as Plateau functions, which were fit to the group-average cueing benefit (red curves). Dots show group-average, error bars and shaded regions depict ±1 within-subject SEM (Morey, 2008).

In contrast, endogenous attention similarly improved a broad range of lower and higher spatial frequencies on either side of f_csf. Across the group, improvements by endogenous attention were centered on 2.65 ± 0.50 cpd, with equivalent improvements that spread (indexed by σ) to 1.22 ± 0.067 octaves on either side. The center and spread were equivalent across eccentricity. A one-way repeated-measures ANOVA assessed the effect of eccentricity on the center and spread parameters, separately. The main effect of eccentricity was not significant for either the center (F[1, 9] = 1.22, p > 0.2) or the spread (F[1, 9] < 1) parameters. Thus improvements by endogenous attention spanned a similar range of SFs at each eccentricity. Similar to Experiment 1, the Plateau functions are centered at SFs higher than f_csf. However, because the effects of endogenous attention exhibit equivalent benefits for a wide range of SFs, its pattern is unlike the selective and preferential enhancement of higher SFs exhibited by exogenous attention.

Discussion

Exogenous and endogenous attention distinctively alter the CSF across eccentricity

By jointly manipulating the SF and eccentricity of grating stimuli while separately directing exogenous or endogenous attention in the same task and observers, we characterized the distinctive effects of covert attention across the CSF and eccentricity. We found that the effects of exogenous and endogenous attention differed relative to the peak SF in the Neutral condition. Whereas exogenous attention preferentially enhanced higher SFs, endogenous attention similarly enhanced a broad range of lower and higher SFs. These distinct effects of exogenous and endogenous attention across SF persisted across eccentricity regardless of whether the target appeared by itself or among distractors. Specifically, as the peak SF shifted to lower frequencies in the periphery, exogenous attention consistently exhibited the largest improvements for higher SFs. In contrast, the same range of lower and higher SFs were similarly enhanced by endogenous attention. Therefore exogenous and endogenous attention differentially modulate contrast sensitivity across SF and eccentricity, thereby distinctively shaping our perception across the visual field.

These novel findings advance our understanding of the effects of exogenous and endogenous attention on a fundamental measure of visual function, contrast sensitivity. Although many previous studies have demonstrated that both exogenous attention (Barbot et al., 2011; Barbot et al., 2012; Cameron et al., 2002; Carrasco et al., 2000; Carrasco & McElree, 2001; Fernández et al., 2019; Foley & Schwarz, 1998; Herrmann et al., 2010; Ling & Carrasco, 2006b; Liu et al., 2005; Morgan et al., 1998; Pestilli et al., 2007; Pestilli & Carrasco, 2005; Smith et al., 2004; Solomon, Lavie, & Morgan, 2004) and endogenous attention (Barbot et al., 2012; Dosher & Lu, 2000a, 2000b; Herrmann et al., 2010; Huang & Dobkins, 2005; Lee et al., 1997, 1999; Ling & Carrasco, 2006a, 2006b; Lu et al., 2002; Lu & Dosher, 1998, 2000, 2004; Morrone et al., 2002, 2004) improve contrast sensitivity, we characterized their effects across SF and eccentricity to reveal that each operates differently relative to the constraints of the visual system at each eccentricity.

Exogenous attention selectively enhanced higher SFs, in spite of contrast sensitivity peaking at lower SFs with increasing eccentricity (e.g., Hilz & Cavonius, 1974; Rovamo et al., 1978; Virsu & Rovamo, 1979). Such a pattern of enhancement suggests an attentional mechanism that serves to improve perception specifically for fine spatial patterns when confronted with brief, salient events in the periphery. Exogenous attentional effects that are selective for high SFs have been demonstrated previously for texture-defined stimuli (Barbot et al., 2011, 2012; Carrasco et al., 2006). The present findings reveal that such effects generalize to contrast sensitivity for luminance-modulated stimuli. In contrast, the effects of endogenous attention suggest a nonselective attentional mechanism across SF that serves to improve the perception of spatial patterns indiscriminately. Whereas a previous study has demonstrated that endogenous attention uniformly excludes external noise across SF (Lu & Dosher, 2004), our current findings provide complementary evidence that endogenous attention similarly enhances contrast sensitivity for a broad range of SFs even in the absence of external noise. By systematically characterizing the effects of exogenous and endogenous attention across SF and eccentricity, we provide converging evidence of two attentional mechanisms with distinct modes of operation across the visual field.

The current findings also provide possible explanations for discrepant findings in the literature regarding the effects of exogenous attention on contrast sensitivity across SF. A recent study (Fernández et al., 2019) used psychophysical reverse correlation to assess how exogenous attention altered sensitivity to orientation and SF to improve task performance. The authors found that behavioral improvements were not associated with enhanced sensitivity to SFs, which would seemingly conflict with the enhancements observed here. However, the authors discuss that the lack of SF enhancement could be attributed, in part, to the narrow range of SFs (1–2.25 cpd) that were assessed in the study. It is likely that the peak SF in the Neutral condition, and consequently the maximum cueing benefit, occurred outside the range of SFs measured. Similarly, an earlier study (Megna, Rocchi, & Baldassi, 2012) reported that the preferential enhancement for higher SFs by exogenous attention occurred for near (3°) but not far (9°) eccentricities. Their results seemingly conflict with the consistent pattern of selective high-SF enhancement we observed at each eccentricity. However, it is likely that the discrepancy is due to the range of low SFs (0.2–1 cpd) that were assessed. We found that the effects of exogenous attention were largest for SFs higher than the Neutral peak SF, which reached a minimum of 1 cpd at our farthest eccentricity (12°). Thus the relatively low SFs they measured likely precluded consistent measures of the largest attention effects, which presumably occurred outside the assessed range. In sum, by characterizing the effects of attention across a wide range of SFs and eccentricities, we provide data that likely reconcile the findings of previous research and constrain the design of future studies, as well as models of visual attention.

Exogenous and endogenous attention improve contrast sensitivity with and without distractors

The current findings demonstrate that the attentional improvements of the CSF across eccentricity were similar when targets were presented alone or among distractors. Specifically, in Experiment 1 we targeted the signal enhancement mechanism by displaying individual gratings without masks or distractors (Cameron et al., 2002; Carrasco et al., 2000, 2002; Ling & Carrasco, 2006b) and provide converging evidence that exogenous (Cameron et al., 2002; Carrasco et al., 2000) and endogenous attention (Ling & Carrasco, 2006b) improve contrast sensitivity in zero-noise displays. Moreover, we demonstrate that signal enhancement across SF and eccentricity differ between each type of attention. In Experiment 2, targets were displayed among distractors to additionally engage the distractor suppression mechanism of attention (Baldassi & Burr, 2000; Cameron et al., 2004; Dosher & Lu, 2000a; Lu & Dosher, 2000; Morgan, Ward, & Castet, 1998; Solomon et al., 1997). Previous studies have reported that the effects of attention are more pronounced when the number of distractors are increased (Cameron et al., 2004; Carrasco & McElree, 2001; Carrasco & Yeshurun, 1998, 1998; Eckstein & Whiting, 1996; Foley & Schwarz, 1998; Giordano, McElree, & Carrasco, 2009; Palmer, 1994; Verghese, 2001). Therefore Experiment 2 could have amplified patterns of attentional effects that were too weak to be observed in Experiment 1. Nevertheless, the same pattern of preferential high-SF enhancement by exogenous attention and broad enhancement of lower and higher SFs by endogenous attention were observed in both experiments. Therefore our findings reveal that both exogenous and endogenous attention operate similarly when directed to either isolated targets or targets among distracting visual input.

Previous studies (Dosher & Lu, 2000a; Lu et al., 2002; Lu & Dosher, 1998; Lu & Dosher, 2000) have reported that endogenous attention operates only when sources of external noise are present. A possible explanation for this discrepancy is that their short (150 ms) SOA could have prevented observers from fully deploying endogenous attention. This proposal has been offered previously (Ling & Carrasco, 2006b). For most observers, at least 300 ms are required to observe the effects of endogenous spatial attention (e.g., Cheal & Lyon, 1991; Liu, Stevens, & Carrasco, 2007; Müller & Rabbitt, 1989). In our study we provided observers 400 ms to voluntarily orient their attention, which allowed us to observe the effects of attention in noiseless displays.

It is unlikely that the effects of attention in our study are due to statistical uncertainty reduction (Pelli, 1985). Statistical uncertainty models assert that valid cues improve performance simply by reducing the number of locations to be monitored from all possible target locations to one (Eckstein, 1998; Foley & Schwarz, 1998). However, location uncertainty in both Neutral and Valid conditions was equated by presenting post-stimulus response cues in each condition. Earlier studies have also shown that attentional improvements exceed those predicted signal detection models of uncertainty (Carrasco et al., 2000; Morgan et al., 1998) and that exogenous attention improves contrast sensitivity to the same extent in different conditions of spatial uncertainty (Cameron et al., 2004). Moreover, similar improvements by exogenous attention have been reported even when observers could perfectly localize the target stimulus (Carrasco et al., 2000). In sum, these studies demonstrate that uncertainty reduction is not a primary mechanism underlying attentional benefits.

Visual perception is modulated inflexibly by exogenous attention and flexibly by endogenous attention

The specific enhancement of high SFs by exogenous attention differs from the nonspecific enhancement of low and high SFs by endogenous attention, and reflects their respective inflexibility and flexibility in modulating visual perception. Whereas exogenous attention exerts its effects automatically in response to brief and salient cues, the effects of endogenous attention adapt to the demands of the task (Giordano et al., 2009). The perceptual consequences of exogenous attention's automaticity have been demonstrated in texture segmentation tasks, which are constrained by spatial resolution (for reviews see, Anton-Erxleben & Carrasco, 2013; Carrasco & Barbot, 2014; Carrasco & Yeshurun, 2009). In these tasks, directing exogenous attention leads to an automatic increase in spatial resolution that improves performance for targets in the periphery where resolution is low but impairs performance near the fovea where resolution is already too high for the target (Carrasco et al., 2006; Jigo & Carrasco, 2018; Talgar & Carrasco, 2002; Yeshurun, Montagna, et al., 2008; Yeshurun & Carrasco, 1998; Yeshurun & Carrasco, 2000). These impairments caused by valid exogenous cues are unique to texture segmentation tasks, which are characterized by a central performance drop, and have been attributed to an automatic and specific enhancement of contrast sensitivity to high SFs (Carrasco et al., 2006). Thus our current findings contribute direct evidence for a specific enhancement of contrast sensitivity to high SFs at each eccentricity. Moreover, our findings provide converging evidence for an inflexible exogenous attentional mechanism.

Texture segmentation tasks also provide a unique perspective into the flexibility of endogenous attention. When observers direct endogenous attention in these tasks, performance is improved at all eccentricities (Barbot & Carrasco, 2017; Jigo & Carrasco, 2018; Yeshurun, Montagna, et al., 2008). This has been attributed to endogenous attention increasing spatial resolution in the periphery by enhancing contrast sensitivity to high SFs, and decreasing resolution near the fovea by suppressing contrast sensitivity to high SFs (Barbot & Carrasco, 2017). Such control of the sensitivity to high SFs is owed to the flexibility of endogenous attention, which adjusts contrast sensitivity according to task demands. In our current study, observers were not incentivized to suppress sensitivity to any range of SFs, which likely led to the broad enhancements across SF that were observed. However, previous studies have demonstrated that endogenous attention indeed suppresses distracting SFs that are detrimental to the task (Lu & Dosher, 2004). Thus our findings provide complementary evidence of an endogenous attentional mechanism that can enhance a broad range of SFs when required by the task.

The automaticity of exogenous attention and flexibility of endogenous attention have also been demonstrated for the temporal aspects of processing. On the one hand, exogenous attention exhibits a tradeoff between spatial and temporal resolution at the attended location; exogenous attention improves spatial resolution and impairs temporal resolution (Hein et al., 2006; Yeshurun & Levy, 2003; but see, Chica & Christie, 2009). An attentional mechanism that favors parvocellular over magnocellular neurons has been proposed to explain this perceptual tradeoff (Yeshurun, 2004; Yeshurun & Hein, 2011; Yeshurun & Levy, 2003; Yeshurun & Marom, 2008; Yeshurun & Sabo, 2012). According to this explanation, exogenous attention selectively enhances parvocellular neurons, which exhibit better spatial resolution but worse temporal resolution than magnocellular neurons. On the other hand, endogenous attention can flexibly improve or impair temporal resolution based on task demands (Hein et al., 2006; Sharp et al., 2018). Therefore the distinct effects of both types of covert attention are not restricted to spatial aspects of visual perception.

Attentional modulations were restricted to contrast gain by task design

Attentional improvements of contrast sensitivity by both types of covert attention were restricted to the dynamic range of observers’ psychometric functions, by design. Specifically, we targeted contrast gain modulation, which leads to a leftward shift of the psychometric function and produces the largest benefits at the midpoint of the function's dynamic range (Herrmann et al., 2010; Ling & Carrasco, 2006b; Pestilli et al., 2007; Pestilli et al., 2009). Although attentional modulation can also manifest as response gain, wherein performance is scaled multiplicatively to yield the largest benefits at the upper asymptote (Herrmann et al., 2010; Ling & Carrasco, 2006b; Morrone et al., 2002, 2004; Pestilli et al., 2009), or a mix between contrast and response gain (Huang & Dobkins, 2005; Ling & Carrasco, 2006b; Pestilli et al., 2009), our task design ensured that attention effects would manifest as contrast gain. A prominent computational model of covert attention (Reynolds & Heeger, 2009) and previous psychophysical experiments (Herrmann et al., 2010) have demonstrated that stimulus size and the size of the attended area (i.e., attention field size indexed by spatial uncertainty) determine whether contrast or response gain would occur. However, when both these variables are not explicitly manipulated, the effects of endogenous attention manifest as contrast gain and the effects of exogenous attention can manifest as a mix between contrast and response gain (Ling & Carrasco, 2006b; Pestilli et al., 2009). Thus one would expect that we would observe a change in the upper asymptote for the exogenous attention condition. However, despite equating stimulus size across both types of attention and not manipulating spatial uncertainty, our task differed from previous studies as we used a coarse discrimination (±45°) task to assess performance. With this task, performance at high contrasts left little room to test for response gain (Cameron et al., 2002). We opted for a coarse discrimination task as it yields similar measures of performance as a detection task (Furchner et al., 1977; Nachmias & Weber, 1975; Thomas & Gille, 1979; Watson, Thompson, Murphy, & Nachmias, 1980; Watson & Robson, 1981) and maximizes the number of SFs and eccentricities that could be measured. Had we used a fine discrimination task (e.g., ±4°) to avoid ceiling effects, performance would have been too low for high SFs, particularly at far eccentricities, for us to obtain reliable estimates of contrast sensitivity. Therefore our task was designed to yield contrast gain modulations to measure the effects of attention on the CSF across eccentricity.

Possible contributions of feature-based attention to task performance

Could feature-based attention (FBA)—the prioritization of stimulus features (e.g., orientation and spatial frequency) irrespective of their spatial locations—have contributed to the observed effects of endogenous and exogenous spatial attention? Both the task design and the pattern of results indicate this was not the case. Our task design minimized the contributions of feature-based attention. First, observers were only allowed 100 ms to deploy exogenous attention and 400 ms to deploy endogenous attention. These durations precluded the deployment of FBA, which requires at least 500 ms to be reliably deployed (Liu et al., 2007). Second, observers had no prior information regarding the stimulus features they would encounter on a given trial as the orientation and SF of the grating stimuli were randomly interleaved throughout the experiment. Thus an observer would need to attend a minimum of six SFs and two orientations on a given trial. When more than one feature is attended, the effects of FBA decline sharply; the benefit brought about by attending to one feature is halved when two features are attended (Liu et al., 2013; Liu & Jigo, 2017). As a result, any attentional benefits due to FBA would have been negligible.

The pattern of our results provides evidence that the effects of FBA were indeed minimal in our study. First, whereas the effects of FBA manifest as response gain regardless of location uncertainty (Herrmann et al., 2012), we observed contrast gain modulations in our study. Second, FBA effects spread across the visual field (Liu & Mance, 2011; Serences & Boynton, 2007; White, Rolfs, & Carrasco, 2015; White & Carrasco, 2011) and are often independent from spatial attention (Hayden & Gallant, 2009; Treue & Trujillo, 1999; White et al., 2015). As a result, the effects of FBA would have been constant across the visual field and identical among exogenous attention, endogenous attention, and their respective neutral conditions. In sum, the contributions of FBA to our results, were negligible, if any.

Conclusion

The present study systematically characterized and compared the effects of exogenous and endogenous attention on contrast sensitivity across several SFs and eccentricities using the same task, stimuli, and observers. We demonstrated that both types of attention enhanced contrast sensitivity across SF and eccentricity, in the presence or absence of distractors. Importantly, this study revealed that exogenous and endogenous attention exhibit distinct patterns of benefits. Whereas exogenous attention preferentially enhanced higher SFs than the peak SF at each eccentricity, endogenous attention similarly improved contrast sensitivity over a broad range of low and high SFs. Overall, our results highlight how exogenous and endogenous attention distinctively shape visual perception across the visual field.