Abstract
Taking action in response to anthropometrics is important to respirator fit. We aimed to investigate the associations between the filtering facepiece respirator (FFR) fit and the head-face dimensions among Chinese people. We used data from 85 volunteers. We focused on fit factors and 8 head-facial dimensions of subjects. The fit factors from 4 respirator models with different protection levels and shapes were measured by a PortaCount fit tester. Each subject tested four respirator models, for a total of four quantitative fit tests per subject. Passing rate (PR) of each model was determined at fit factor level no less than 100. The data of 85 subjects aged 22–51 yr old were analyzed using χ2 test, one-way ANOVA test, t-test and non-conditional logistic regression model. The PRs for the 4 models were 52.9%, 61.2%, 40.0% and 63.5%, which were significantly different. We found the positive effect of morphological facial length and negative effect of bitragion-submandibular arc on fit factors. This confirms it is necessary to conduct fit test before using a respirator. PRs varied among 4 models regardless of their protection level and shape. Anthropometric dimension of the user, which had effects on FFR fit, should be considered when designing respirator.
Keywords: Fit factor, Anthropometrics, Head-face dimension, Filtering facepiece respirator, Fit test
Introduction
The filtering facepiece respirator (FFR) has become increasingly important in the workplace and daily life. It’s the last defense preventing particles from entering the human body. The respirator fit plays an important role in protection1). The respirator fit test is necessary to ensure that respirators can provide their expected level of protection. The occupational safety and health administration (OSHA) requires that a fit test should be conducted annually with additional fit tests if the users’ physical conditions change such as facial scarring or weight gain2). Changes or differences in head-face dimension could cause changes in FFR fit. Therefore, the design and research of respirators should also be focused on improving its fit to achieve the optimal protection. Exploring the influencing factors of fit is one of the important contents in this field.
Factors that may affect the FFR fit are the facial anthropometric dimensions and the gender of the user, and the shape of the FFR. Facial anthropometric dimensions, such as bizygomatic breadth, menton-subnasale length, biocular breadth and bitragion-subnasale arc, were associated with the FFR fit in both Korean males and females. In addition to the above dimension indicators, the FFR fit was also affected by biectoorbitale breadth, subnasale-nasion length and lip width in Korean females3). Previous studies have suggested that there may be a link between the shape and fit of respirators. Ciotti C et al. showed that the passing rates (PRs) of flat-fold, duckbill and hard-shell respirators decreased progressively for healthcare workers in France4). The users’ facial anthropometric dimensions seem to have a greater effect on the fit of cup-shaped respirators. In the Chinese population, the face width, bigonial breadth and face length of the subjects that passed the fit test were significantly larger than those of the subjects that failed the fit test when they used cup-shaped respirators. But there were no significant differences of facial dimensions between the subjects that passed and the subjects that failed the fit test for folding respirators5).
The influence of gender on FFR fit has always been controversial. Kim et al. found that males achieved better respirator fit than females regardless of respirator brands tested in Korea3). On the other hand, respirator fit is not specific to gender characteristics. Fit factors did not differ significantly by gender despite significant differences in the facial dimensions in the general South African population6). Oestenstad et al. conducted a quantitative fit test in white people and concluded that there were no significant differences in respirator fits between males and females7).
Although some studies have revealed the influencing factors of FFR fit in different populations, the factors related to FFR fit in Chinese people are still unclear. In addition, the current design of FFRs mostly refers to the head-face dimensions of European and American people. FFR fit in Chinese still needs to be discussed. Therefore, the aim of the present study is to determine what are the related factors to FFR fit, especially the head and face dimension, in order to provide data to support the evaluation and design of FFRs.
Subjects and Methods
Subjects
Eighty-five test subjects (31 males and 54 females) participated in the study. Each was tested with four respirators for one visit in the lab. This study was approved and funded by the Natural Science Foundation of Beijing Municipality (Item No.7144233). The test in this study was approved by Medical Ethics Committee of National Institute for Occupational Health and Poison Control, Chinese Center for Disease Control and Prevention. All subjects had signed informed consents. Subjects were graduate students (the average age was 27 ± 4.4 yr old.) who were willing to participate in the experiment in Chinese Center for Disease Control and Prevention (CDC). All of them were trained on how to wear the respirators correctly. Exclusionary criteria included a history of uncontrolled chronic asthma, pneumonia, and high blood pressure.
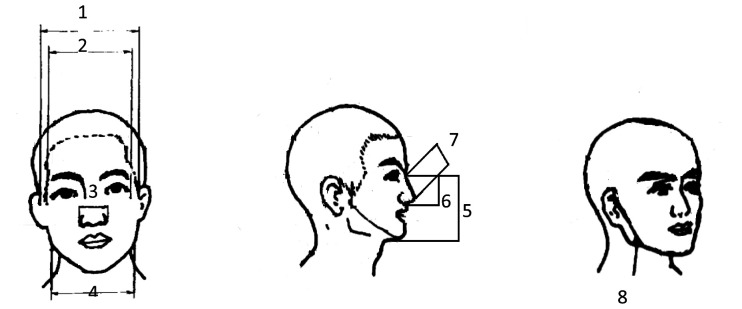
Eight anthropometric measurements (Fig. 1) were collected with bending angle gauge, right angle square and tape.
Fig. 1.
Anthropometric measurements. 1. bitragion breadth; 2. bizygomatic breadth; 3. nose breadth; 4. bigonial breadth; 5. morphological facial length; 6. nose height; 7. nose length; 8. bitragion-submandibular arc.
Respirators
The four models tested were three N95 FFRs and one FFP3 FFR. The PRs of the four FFRs of the same imported brand were evaluated. The respirators models were available in one-size-only. Four models of FFRs were numbered as model 1 (N95, cup, no exhalation valve), model 2 (N95, cup, with exhalation valve), model 3 (N95, fold, no exhalation valve) and model 4 (FFP3, fold, with exhalation valve), respectively. Model 1–3 were certified by National Institute for Occupational Safety and Health (NIOSH) and model 4 was certified by European Union (EU).
Fit test method
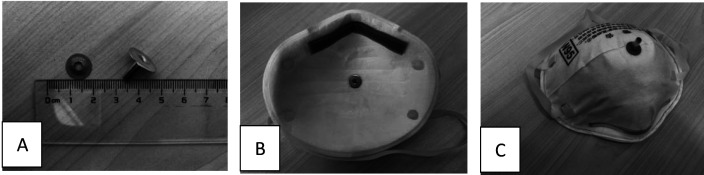
The fit test was performed inside a general laboratory. Each respirator was probed for measuring the concentration of aerosol particles inside the facepiece; a separate tube sampled the ambient air. Probing kit (model: 8025; TSI, Inc.; Shoreview, MN, USA) was used to probe the respirators and fix the air pipes (Fig. 2). This probe placement sampled the air inside the respirator to approximate the actual particle concentration that the wearer would breathe.
Fig. 2.
Fit test probe. A. probe inlet with affixed sampling tube (with a ruler); B. interior view of the nose cup showing probe inlet; C. side view of respirator showing probe inserted through a cup-shaped respirator.
Subjects were given training on each respirator model’s donning and adjustment procedures using the user instructions provided by the manufacturer. Subjects were then asked to don the respirator and perform a user seal check in accordance with the user instructions specific to the model they tested. If the subject failed the user seal check, they were asked to re-adjust the respirator on their face and adjust strap tension as necessary. Once these adjustments were made, they were asked to perform another user seal check. This process was repeated until the subject passed the user seal check. There were five minutes for the subjects to adapt, then the test would begin.
Quantitative fit testing was performed with the PortaCount® Plus Respirator Fit Tester (model: 8038; TSI, Inc.; Shoreview, MN, USA). The PortaCount® uses condensation nuclei counting (CNC) technology to determine a quantitative estimate of respirator fit. To measure the concentration of aerosol particles inside the respirator, flexible sampling tube was connected from the “sample” inlet on the PortaCount® to the outlet of the respirator sampling probe. Another tube was connected from the “ambient” inlet on the PortaCount® to collect an air sample from the test chamber.
During an individual fit test, subject conducted eight fit test exercises: normal breathing, deep breathing, turning head side to side, moving head up and down, talking (reciting the “rainbow passage”), grimacing, bending over (bending at the waist as if to touch the toes), and normal breathing. For each individual test exercise (with the exception of “grimace”), an individual exercise fit factor (FFe) is calculated as the ratio of the ambient particle concentration to the in-mask particle concentration; an FFe is not calculated for “grimace”. The overall fit factor (FFo) (the harmonic average of the individual exercise FFe, excluding the grimace exercise) was calculated by the PortaCount® at the end of the test. For each FFR, a FFo ≥ 100 is the passing criterion for the fit test.
The study design set out to obtain a total of 340 FFo data points (85 subjects × 4 respirator models × 1 donning/model/visit × 1 visits = 340 FFo data points). One donning of each of the four different models was to be performed on each one laboratory visit; 340 data points were collected.
Data analysis
The passing rate (PR) is defined as the percentage of subjects that a FFR model is capable of achieving acceptable fit as defined by specific passing criteria. Geometric mean (GM) FFo and geometric standard deviation (GSD) were calculated for the subjects. χ2 test was used to compare the PR; one-way ANOVA and t-test was used to compare the GM FFo between different shapes or different models; partial correlation analysis was used to analyze the correlation between facial dimension and GM FFo; non-conditional logistic regression (backward (Wald)) method, the probability value of factor entry into and out of the equation were <0.05 and >0.1, was used to explore the factors to FFR fit. A significance level of 0.05 was selected to test the null hypothesis. SPSS version 16.0 (IBM Corporation, Armonk, NY, USA) was used for all calculations and analyses.
Results
Three hundred forty fit tests were performed. The overall fit factors (FFos) of 155 fit tests were no less than 100. All samples had a PR of 45.59% (155/340). PRs for the FFRs are summarized in Table 1. The PRs of the four models were 52.9%, 61.2%, 40% and 63.5% respectively, which followed the general trend: model 4> model 2> model 1> model 3. There was a significant difference of PRs between the four models of respirators (χ2=12.288, p<0.05). The number of the subjects who could pass the fit-tests with all the four models was 17 (20%). The difference of PRs in FFRs of different shapes was not significant, as shown in Table 2 (p>0.05). The significant difference of PRs between genders could be observed only in model 3 (t=3.151, p<0.05). For all the 340 fit-tests, gender difference of PR was not significant (p>0.05).
Table 1. Passing rate (PR) for different respirator models (N=85, males:31; females: 54).
| Respirator | Subjects | PR | ||
|---|---|---|---|---|
| Total | Male | Female | ||
| Model 1 | 85 | 52.9% (45/85) | 54.8% (17/31) | 51.9% (28/54) |
| Model 2 | 85 | 61.2% (52/85) | 61.3% (19/31) | 61.1% (33/54) |
| Model 3 | 85 | 40.0% (34/85) | 54.8% (17/31) | 31.5% (17/54) |
| Model 4 | 85 | 63.5% (54/85) | 61.3% (19/31) | 64.8% (35/54) |
Table 2. Passing rate (PR) for different respirator shapes (N=85, males: 31; females: 54).
| Respirator | Subjects | PR | ||
|---|---|---|---|---|
| Total | Male | Female | ||
| Cup | 170 person time | 57.1% (97/170) | 58.1% (36/62) | 56.5% (61/108) |
| Fold | 170 person time | 51.8% (88/170) | 58.1% (36/62) | 48.1% (52/108) |
Geometric mean FFo results for the subjects are shown in Table 3 by respirator models and respirator shapes. One-way ANOVA on means indicated that there was a significant difference of the Geometric mean FFo among the four models (F=4.546, p<0.05). T-test on means indicated that the Geometric mean FFo were not significantly different between the cup-shaped and the folding respirators (p>0.05).
Table 3. Geometric mean (GM) overall fit factors (FFo) by respirator models and shapes.
| Model | Form | GM FFo | GSD FFo | Min FFo | Max FFo |
|---|---|---|---|---|---|
| 1 | Cup | 112.5 | 58.4 | 13 | 200 |
| 2 | 121.5 | 57.0 | 9.1 | 200 | |
| 3 | Fold | 92.2 | 62.6 | 7.2 | 200 |
| 4 | 121.0 | 58.7 | 10 | 200 |
GSD: geometric standard deviation.
The facial dimensions comparison between the “passed” and “failed” subjects are listed in Table 4 by respirator models. T-test on means showed that there were significant differences of morphological facial length, nose height and nose length between “passed” and “failed” subjects by model 3 (t=−4.525, −2.738 and −2.725, respectively, p<0.05). Other dimensions between the “passed” and “failed” subjects by each model were not significantly different (p>0.05).
Table 4. Dimensions of test-passed and test-failed subjects by respirator models.
| Dimension | Model 1 | Model 2 | Model 3 | Model 4 | ||||
|---|---|---|---|---|---|---|---|---|
| Pass | Fail | Pass | Fail | Pass | Fail | Pass | Fail | |
| Morphological facial length | 113.8 ± 6.3 | 112.1 ± 8.5 | 113.3 ± 6.4 | 112.3 ± 8.2 | 116.7 ± 5.7 | 110.2 ± 6.9 | 112.9 ± 6.6 | 112.6 ± 8.3 |
| Bizygomatic breadth | 112.0 ± 9.4 | 108.2 ± 9.7 | 111.2 ± 9.1 | 108.9 ± 10.9 | 112.2 ± 9.3 | 108.8 ± 9.8 | 110.4 ± 9.1 | 109.8 ± 10.8 |
| Bitragion breadth | 140.9 ± 7.9 | 138.3 ± 7.7 | 140.1 ± 7.8 | 139.3 ± 8.0 | 141.1 ± 8.0 | 138.7 ± 7.5 | 138.8 ± 7.4 | 141.1 ± 8.1 |
| Bigonial breadth | 108.0 ± 10.1 | 105.5 ± 8.1 | 106.8 ± 8.7 | 106.6 ± 9.8 | 107.7 ± 10.7 | 106.0 ± 7.8 | 106.0 ± 8.7 | 107.8 ± 9.7 |
| Nose height | 46.2 ± 4.0 | 46.3 ± 4.0 | 46.9 ± 3.9 | 45.5 ± 3.9 | 47.6 ± 3.9 | 45.3 ± 3.7 | 46.2 ± 3.7 | 46.3 ± 4.4 |
| Nose length | 39.7 ± 4.5 | 39.6 ± 3.7 | 40.2 ± 4.3 | 39.0 ± 3.8 | 41.2 ± 4.9 | 38.6 ± 3.1 | 39.4 ± 3.6 | 40.0 ± 5.0 |
| Nose breadth | 37.4 ± 3.4 | 36.6 ± 2.8 | 37.0 ± 3.2 | 37.0 ± 3.0 | 37.5 ± 3.6 | 36.6 ± 2.7 | 36.5 ± 3.0 | 37.8 ± 3.2 |
| Bitragion-submandibular arc | 282.0 ± 21.5 | 279.3 ± 9.7 | 278.9 ± 20.5 | 283.0 ± 20.1 | 284.1 ± 21.6 | 278.0 ± 19.3 | 278.7 ± 20.0 | 283.6 ± 20.7 |
The partial regression coefficients of the facial dimensions and the fit factors are shown in Table 5 by respirator models. According to the results of partial correlation analysis, there were positive correlations between the morphological facial length and fit factors by model 1 (p<0.05) and model 3 (p<0.05) respectively. Also, there was positive correlation between the nose height and fit factors by model 2 (p<0.05). Lastly, there was negative correlation between the nose breadth and fit factors by model 4 (p<0.05).
Table 5. Partial correlation analysis of fit factors and facial dimensions.
| Dimension | Model 1 | Model 2 | Model 3 | Model 4 | ||||
|---|---|---|---|---|---|---|---|---|
| Partial regression coefficient | p | Partial regression coefficient | p | Partial regression coefficient | p | Partial regression coefficient | p | |
| Morphological facial length | 0.215 | 0.049 | 0.127 | 0.249 | 0.338 | 0.002 | 0.112 | 0.309 |
| Bizygomatic breadth | 0.210 | 0.055 | 0.087 | 0.430 | 0.045 | 0.685 | −0.021 | 0.849 |
| Bitragion breadth | 0.208 | 0.058 | 0.082 | 0.459 | −0.012 | 0.914 | −0.150 | 0.173 |
| Bigonial breadth | 0.072 | 0.515 | −0.026 | 0.814 | 0.006 | 0.954 | −0.126 | 0.253 |
| Nose height | 0.062 | 0.577 | 0.248 | 0.023 | 0.050 | 0.653 | 0.087 | 0.429 |
| Nose length | 0.067 | 0.543 | 0.183 | 0.096 | 0.089 | 0.419 | 0.007 | 0.947 |
| Nose breadth | 0.212 | 0.053 | 0.004 | 0.968 | 0.015 | 0.890 | −0.223 | 0.041 |
| Bitragion-submandibular arc | 0.001 | 0.991 | −0.130 | 0.239 | 0.000 | 0.996 | −0.143 | 0.194 |
The fit test result and its related factors are demonstrated in Table 6. The dependent variable was a two-level, categorical variable (subjects who had measured fit factors equal to or greater than 100 and fit factors less than 100) and the independent variables were facial dimensions and gender of the user, and model and shape of the respirator. At the beginning, gender, model, shape and facial dimensions were all in the equation. Finally, non-conditional logistic regression (backward (Wald)) indicated that morphological facial length and bitragion-submandibular arc were the factors that influenced the fit test results.
Table 6. Logistic regression equation of factors to fit test result.
| Factor | β | p | OR | 95%CI |
|---|---|---|---|---|
| Morphological facial length | 0.062 | 0.002 | 1.064 | 1.022–1.107 |
| Bitragion-submandibular arc | −0.018 | 0.012 | 0.982 | 0.969–0.996 |
OR: odds ratio.
Discussion
Fit tests can help to choose a correct respirator which fits the wearer. It is necessary to perform a fit test of the FFR before using it1). However, there are a significant number of workers who continue to use respirators that have not been selected through a fit test8). Therefore, in order to adapt to more people, respirators should be designed to match users’ major characteristics as much as possible. Facial dimensions are the important factors for respirator design. Full consideration of the wearer’s facial characteristics can help to improve the fit.
As illustrated in Table 1, in the same population, there was a significant difference of the PRs among the different models. The respirators suitable for Chinese people can be screened out through fit test. Lee et al. suggested specifying a fit test PR of at least 90% of randomly selected wearers as a criterion for a certified respirator9). According to PR standard of 90%, the four models in the present study do not fit Chinese very well.
In the present study, we did observe significant differences of PR between genders in model 3: PR for males was higher than that for females. In Koreans, the respirators were more suitable for males than females in fitting performance10). McMahon et al.’s study also showed that first-choice respirator provided a successful fit of 95.1% for men and 85.4% for women11). It seems that the fit of respirators was better in men than in women. However, for all the 340 tests in the present study, the gender difference of PR disappeared. This finding corresponded well with the results of Spies et al.’s study6) that there was no significant difference in fit test results between men and women in South Africans. About the gender difference of PR in fit test, the existing studies have come to different conclusions. Since the respirators we use do not need to be distinguished between males and females, we can infer that the effect of gender on facial characteristics does not play a significant role in respirator fit.
According to Tables 2 and 3, the effect of FFR shape on overall fit factors and PR was not significant. Niezgoda et al. found that no significant differences were noted in fit factors, but more individuals passed fit testing wearing flat-fold respirators than cup-shaped respirators12). On the other hand, Manganyi et al.’s research showed that respirator shapes were significant predictors of overall fit, but this factor explained only a small percentage of fit outcomes (OR=0.56)13). Ciotti et al. claimed that flat-fold (PR=57.5%) and duckbill (PR=18.3%) respirator masks seem to be better adapted for healthcare workers than hard-shell (PR=3.3%) respirator masks4). From the perspective of respirator design, the shape of the respirator should not be a factor to fit. More experiments are needed to verify the effect of shape on fit. If the effect of shape on fit is not significant, cheaper and more comfortable respirator shapes should be preferred.
Facial characteristics, including morphological facial length and width, have been identified as important factors in correctly fitting a respirator11). As shown in Table 4, morphological facial length, nose height and nose length were different between the “passed” and “failed” fit-test groups by model 3. To cope with the confounding effect of gender, partial correlation analysis was used to clear the correlation between the fit factors and facial dimension. The results showed morphological facial length, nose height and nose breadth correlated to fit factor respectively in Table 5. Cheng et al.’s research demonstrated that positive effects of morphological facial length on mean log-transformed fit factor with cup-shaped respirators in Chinese people5). Thus, morphological facial length seemed to be a dimension index related to fit. In order to avoid confounding bias and explore the factors to fit, non-conditional logistic regression analysis had been done and showed that morphological face length and bitragion-submandibular arc were the relevant factors to overall fit factor in Table 6. As morphological face length increased, respirator fit also increased. Oestenstad et al.’s research also demonstrated that morphological facial length was the key dimension related to respirator fit14). As the bitragion-submandibular arc decreased, the fit of the respirator increased. Han and Choi. concluded that in Koreans, bitragion-submandibular arc should be considered as an important dimension when designing the respirator15).
In view of the relationship between head-face dimensions and fit, a respirator fit test panel with facial size distribution representative of intended users is essential to the evaluation of respirator fit for new models of respirators. In China, some panels have been developed. For example, two new respirator fit test panels were developed with the same techniques used to create the NIOSH panels from 3,000 Chinese subjects by Chen et al16). After that, Yang et al. used the additional number label (ANL) method for youths born in central China, which not only provided a new methodology in quantifying the characteristics of facial anthropometric dimensions for any ethnic/racial group, but also extended the scope of PCA panel studies to higher dimensions17). A large-scale anthropometric survey based on the fit test is urgently needed in China to establish a database of respiratory apparatus evaluation and design.
NIOSH conducted benchmark testing of 101 respirator models on the market from 2008 to 2009, and developed key test parameters and pass/fail criteria options for a respirator fit capability test for half-mask air-purifying particulate respirators18). The NIOSH study found that 30 percent of the models tested did not have good fitting characteristics, i.e., passing rate was less than 50%. NIOSH is currently developing a standard to establish a performance requirement, called respirator fit capability, to assess respirator face-sealing characteristics. Further studies using the methodology by Zhuang et al.18) are needed to test many respirator models in China with Chinese test subjects. Similar standard may then be developed for China.
The method described in this paper was performed as a research which incorporated quantitative fit testing and facial dimensions measurement. The fit test can help to estimate new models of respirators and the measurement of facial dimensions can supply data to design new models of respirators. Further studies are needed to verify whether the results in this study would be in agreement with those of facial anthropometric survey in wider models. If some dimensions had been chosen as the related factors to fit, the next step is to find a balance point of each dimension to make the fit factor reach the maximum value.
References
- 1.U.S. Centers for Disease Control and Prevention (1996) Laboratory performance evaluation of N95 filtering facepiece respirators, 1996. https://www.cdc.gov/mmwr/preview/mmwrhtml/00055954.htm. Accessed August 29, 2019.
- 2)U.S. Occupational safety and health administration (1998)Respiratory Protection, 29 CFR 1910.134: Final Rule. Fed Regist 63: 1152–300. [PubMed] [Google Scholar]
- 3.Kim H, Han DH, Roh YM, Kim K, Park YG. (2003) Facial anthropometric dimensions of Koreans and their associations with fit of quarter-mask respirators. Ind Health 41, 8–18. [DOI] [PubMed] [Google Scholar]
- 4.Ciotti C, Pellissier G, Rabaud C, Lucet JC, Abiteboul D,, Bouvet E, GERES (2012) Effectiveness of respirator masks for healthcare workers, in France. Med Mal Infect 42, 264–9. [DOI] [PubMed] [Google Scholar]
- 5.Cheng WJ, Yu YY, Yu D, Ping J, Chen WH. (2012) [The relationship between fit characteristics of two kinds of self-inhalation air-purifying dust respirators and head-face dimensions of subjects]. Zhonghua Lao Dong Wei Sheng Zhi Ye Bing Za Zhi 30, 352–5 (in Chinese). [PubMed] [Google Scholar]
- 6.Spies A, Wilson KS, Ferrie R. (2011) Respirator fit of a medium mask on a group of South Africans: a cross-sectional study. Environ Health 10, 17. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 7.Oestenstad RK, Elliott LJ, Beasley TM. (2007) The effect of gender and respirator brand on the association of respirator fit with facial dimensions. J Occup Environ Hyg 4, 923–30. [DOI] [PubMed] [Google Scholar]
- 8.Lofgren DJ. (2012) A must for NIOSH: certify fit performance of the half mask particulate respirator. J Occup Environ Hyg 9, D191–5. [DOI] [PubMed] [Google Scholar]
- 9.Lee K, Slavcev A, Nicas M. (2004) Respiratory protection against mycobacterium tuberculosis: quantitative fit test outcomes for five type N95 filtering-facepiece respirators. J Occup Environ Hyg 1, 22–8. [DOI] [PubMed] [Google Scholar]
- 10.Han DH. (2000) Fit factors for quarter masks and facial size categories. Ann Occup Hyg 44, 227–34. [PubMed] [Google Scholar]
- 11.McMahon E, Wada K, Dufresne A. (2008) Implementing fit testing for N95 filtering facepiece respirators: practical information from a large cohort of hospital workers. Am J Infect Control 36, 298–300. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 12.Niezgoda G, Kim JH, Roberge RJ, Benson SM. (2013) Flat fold and cup-shaped N95 filtering facepiece respirator face seal area and pressure determinations: a stereophotogrammetry study. J Occup Environ Hyg 10, 419–24. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13.Manganyi J, Wilson KS, Rees D. (2017) Quantitative respirator fit, face sizes, and determinants of fit in South African diagnostic laboratory respirator users. Ann Work Expo Health 61, 1154–62. [DOI] [PubMed] [Google Scholar]
- 14.Oestenstad RK, Perkins LL. (1992) An assessment of critical anthropometric dimensions for predicting the fit of a half-mask respirator. Am Ind Hyg Assoc J 53, 639–44. [DOI] [PubMed] [Google Scholar]
- 15.Han DH, Choi KL. (2003) Facial dimensions and predictors of fit for half-mask respirators in Koreans. AIHA J (Fairfax, Va) 64, 815–22. [DOI] [PubMed] [Google Scholar]
- 16.Chen W, Zhuang Z, Benson S, Du L, Yu D, Landsittel D, Wang L, Viscusi D, Shaffer RE. (2009) New respirator fit test panels representing the current Chinese civilian workers. Ann Occup Hyg 53, 297–305. [DOI] [PubMed] [Google Scholar]
- 17.Yang L, Wei R, Shen H. (2017) The fractal characteristic of facial anthropometric data for developing PCA fit test panels for youth born in central China. J Occup Environ Hyg 14, 9–16. [DOI] [PubMed] [Google Scholar]
- 18.Zhuang Z, Bergman M, Lei Z, Niezgoda G, Shaffer R. (2017) Recommended test methods and pass/fail criteria for a respirator fit capability test of half-mask air-purifying respirators. J Occup Environ Hyg 14, 473–81. [DOI] [PMC free article] [PubMed] [Google Scholar]