Abstract
Photopolymerization-based 3D printing has emerged as a promising technique to fabricate 3D structures. However, during the printing process, polymerized materials such as hydrogels often become highly light-scattering, thus perturbing incident light distribution and thereby deteriorating the final print resolution. To overcome this scattering-induced resolution deterioration, we developed a novel method termed flashing photopolymerization (FPP). Our FPP approach is informed by the fundamental kinetics of photopolymerization reactions, where light exposure is delivered in millisecond-scale ‘flashes’, as opposed to continuous light exposure. During the period of flash exposure, the prepolymer material negligibly scatters light. The material then polymerizes and opacifies in absence of light, therefore the exposure pattern is not perturbed by scattering. Compared to the conventional use of a continuous wave (CW) light source, the FPP fabrication resolution is improved. FPP also shows little dependency on the exposure, thus minimizing trial-and-error type optimization. Using FPP, we demonstrate its use in generating high-fidelity 3D printed constructs.
Keywords: 3D printing, flashing photopolymerization, chemical kinetics, light scattering
1. Introduction
Photopolymerization-based 3D printing techniques [1–5] are powerful tools in 3D freeform structure fabrication–they are able to fabricate micro- and nano- scale complex geometries that would otherwise be challenging to achieve with traditional fabrication methods, such as machining or molding. Among the various types of photopolymerization-based 3D printing techniques, light-projection-based 3D printing methods, such as continuous liquid interface production (CLIP),[1] projection micro-stereolithography (PμSL),[2] and dynamic optical projection stereolithography (DOPsL) [6] employ a digital light processing (DLP) technique to project arbitrary patterns onto a prepolymer solution, achieving both a fine resolution and fast fabrication speed.[7] Photopolymerization-based 3D printing has found numerous promising applications in consumer products as well as biomedical engineering such as implantation,[8] imaging,[9] tissue culture,[10,11] drug delivery,[12] and so on.[13–16] However, despite these successful demonstrations of 3D polymeric structure fabrication, this technique faces significant challenges to fabricate functional devices with micron-sized features when using materials that are not optimized for fabrication. For example, water-containing hydrogel scaffolds for biomedical applications often demand a complex 3D architecture with micron-scale features in order to capture the dynamic interactions between the cells and microenvironment, yet most hydrogel materials can hardly be fabricated at a very fine resolution.
To achieve a high resolution in photopolymerization-based 3D printing, the proper light exposure dose must be determined. Insufficient exposure doses cannot photopolymerize the material, while excessive exposure doses can lead to polymerization beyond the desired regions. Generally, the proper exposure dose window is very narrow and needs to be identified for each desired structure and prepolymer material; usually done through manual trial-and-error. This optimization process is time-consuming and costly, and often the resultant fabrication resolution achieved is suboptimal compared to both the desired designed dimensions and the printer’s optical resolution.
This resolution deterioration is mainly caused by three factors, the first of which is light scattering. Optically-clear media allow for sharp patterns of high-fidelity, but the same patterns would be inevitably blurred or have suboptimal features in an optically-scattering media. The second factor is optical depth of focus—depending on the printing media, light can penetrate and polymerize up to a certain depth from the initial plane of incidence. If this cure depth is greater than the optical depth of field, then the out-of-focus plane may experience unwanted polymerization. According to ray optics theory, an imaging system with a lens of numerical aperture of 0.05 and a resolution requirement of 5 μm will have a depth of focus of 100 μm. The cure depth is determined by the absorption of the material, typically ranging from 100 μm to a few millimeters. [17,18] By doping the media with light absorbers, the cure depth can be significantly reduced, minimizing resolution deterioration. The third is molecular diffusion, related to free-radical generation and propagation. Although free radicals are only generated within the light-illuminated region, free radicals and propagating chains can diffuse out of the light-illuminated areas and thus cause unwanted polymerization. According to Fick’s laws of diffusion, the diffusion length can be estimated as , where D is the diffusivity and t is the free-radical lifetime,[19] where the diffusion coefficient of common free radicals are reported to be around 1 × 10−5 cm−2/s in both polar and nonpolar solvents, [20,21] and free radical lifetimes has been reported to be at the scale of 10 milliseconds. [22] Thus, the free radical diffusion length is at the scale of a few microns, but by doping free radical quenchers, the diffusion length can be reduced. [6] These three factors may all be negligible in fabricating a macro-scale device, yet they have substantial influence in microstructure fabrication or biological structure fabrication, where required feature sizes are on the order of microns as well. Among these three factors, light scattering represents a significant challenge, and can be difficult to mitigate since it is a material-dependent property.
Depending on the prepolymer’s formulation and homogeneity, it may be optically clear prior to the start of fabrication, yet light scattering can increase as the material begins to polymerize. Some polymers, such as poly(methyl methacrylate) (PMMA), are as transparent as glass, thus barely suffer from the light scattering problem. Some others, notably hydrogels such as poly(ethylene glycol) diacrylate (PEGDA) hydrogel and di(ethylene glycol) dimethacrylate (DEGDMA) hydrogel, are initially a transparent liquid before polymerization, but once polymerized, they become translucent like agarose, thus can be scattering.
Ideally, light exposure should be avoided as scattering increases, however current light-projection-based 3D printing techniques employ a continuous wave (CW) light source, such as a mercury lamp, laser, or light emitting diode (LED), to photopolymerize the prepolymer solution. With such CW sources, the light exposure, polymerization propagation, and increased scattering (opacification) all begin to overlap during the printing process, compounding the inevitability of scattering-induced photopolymerization of undesired regions, thus resulting in low print fidelity.
To address these challenges, we take advantage of how free-radical photopolymerization is a multi-step process, where light exposure conditions only affect free radical generation, [23,24] while the propagation of polymerizing chains can continue to take place even in dark conditions (i.e. after light illumination). Scherzer et. al. used real-time Fourier-transform infrared (FTIR) spectroscopy to investigate the photopolymerization process of tripropylene glycol diacrylate (TPGDA) and found that chain propagation continues to proceed a few seconds after a short (~ 100 ms) and intense light exposure dose, eventually reaching a conversion rate similar to that when using a CW exposure.[25] Only a small fraction of the monomers in solution was consumed during the exposure period, while the majority was consumed during the dark period thereafter.
Here, we report a light-projection-based 3D printing system that uses flashing exposures for photopolymerization, henceforth referred to as flashing photopolymerization (FPP). With FPP, we chronologically separate three key events: light exposure, polymerization, and opacification. First, we apply a flashing exposure to generate a large amount of free radicals in the desired pattern; this is the light exposure step. Second, after light exposure has ceased, the prepolymer solution undergoes polymerization and opacification in the dark. In this way, the prepolymer is only exposed to light while it is negligibly scattering, thus minimizing scattering-induced resolution deterioration.
In this report, we first present the setup of the FPP 3D printer as well as examples of FPP-printed constructs. Next, we conducted resolution comparisons between CW and FPP and show how polymerization can increase scattering. Lastly, we model and simulate the photopolymerization process to explain the mechanism of FPP.
2. High fidelity 3D printing by flashing photopolymerization
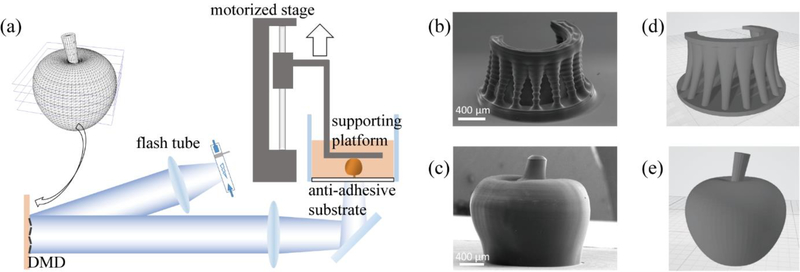
The schematic of the FPP 3D printer is shown in Figure 1(a). The system uses a xenon flash tube as the light source, which is connected to an electronically-triggered controller unit. Using an optical lens setup, a digital micro-mirror device (DMD) projects the photomask image onto and through a transparent anti-adhesion substrate made of polydimethylsiloxane (PDMS, Sylgard 184, Dow Corning) coated on a glass vat containing the prepolymer solution. A motorized stage is used to control the motion of the sample-supporting platform. Finally, a computer with custom software controls and synchronizes these mechanisms.
Figure 1. Flashing photopolymerization 3D printing.
(a) Schematic of the FPP 3D printing system. (b) SEM image of a micro “altar” printed by FPP with 100 μm layer thickness. Scale bar = 400 μm. (c) SEM image of a micro “apple” printed by FPP with 20 μm layer thickness. Scale bar = 400 μm. (d) and (e) are the original 3D models of (b) and (c), respectively.
The 3D printing process is as follows: a digitally-designed 3D model is sliced into 2D cross-sectional images. The supporting platform is lowered to maintain a very narrow spacing (typically between 10 to 100 microns) between the supporting platform and the anti-adhesion substrate before printing. During printing, the xenon flash tube is triggered to flash at a specified energy; the resulting first layer photopolymerizes and attaches to the supporting platform. The motorized stage then raises the supporting platform by one layer thickness of typically 10–100 microns so that unpolymerized material can refill the subsequent vacant space between the anti-adhesion substrate and the previously-polymerized layer(s). A new 2D image slice can be loaded onto the DMD, and the flash tube flashes to solidify this new layer. By repeating these steps, a 3D object can be printed in a layer-by-layer manner.
In general, there is a tradeoff between print speed and quality, usually mediated by layer thickness. Printing with larger layer thicknesses allows for faster print times, albeit at the expense of more inter-layer artifacts and a generally coarser quality, while printing with smaller layer thicknesses will produce better fabrication quality over a longer period of time. We demonstrate this with the 3D-printing of two representative structures using 100% PEGDA (Mn = 575 Da) and 4% (w/v) Irgacure 784, depicted in Figure 1. Figure 1(b) shows the scanning electron microscopy (SEM) image of an altar-like structure printed with a 100-μm-layer thickness, and Figure 1(c) shows the SEM image of an apple-like structure printed with 20-μm-layer thickness, which has a much smoother surface compared to the altar.
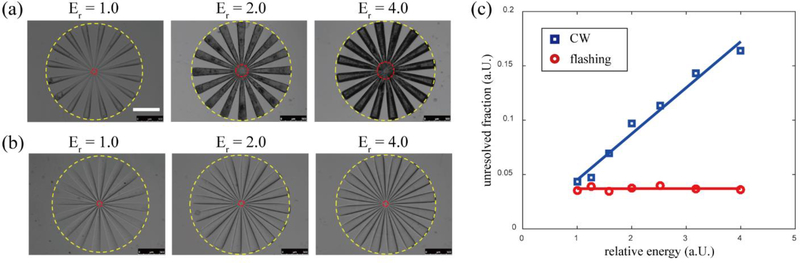
To compare the resolution differences between the FPP and CW printing modes, we designed a photomask with sharp, fine lines culminating in a spoke-like pattern. The photomask was printed on the same instrument using a UV-LED for CW and a xenon flash tube for FPP modes and with an aqueous hydrogel prepolymer solution containing 50% (v/v) PEGDA mixed with 4% (w/v) lithium phenyl-2,4,6-trimethylbenzoylphosphinate (LAP) as the photoinitiator. To simplify testing and analysis, we limited the printed structure to a single 250-μm thick layer, where different exposure doses were used to polymerize the structure. For the continuous UV-LED, we used a series of different energy outputs but kept the illumination time constant, whereas for the flash tube we used a series of different total energies but delivered in a single flash exposure for each. We evaluated resolution in this case by determining the unresolved fraction, that is, the ratio between the unresolved diameter and the outer diameter of the spoke-pattern. Here, a smaller unresolved fraction would mean a better resolution.
To calibrate the exposure dose, we used a series of different LED output powers and a series of different flash energies to polymerize a volume of prepolymer solution. At low exposure doses, the material is unable to polymerize, but as we increased the energy, at a certain value the spoke-pattern was able to polymerize – we defined this value as the minimum unit exposure dose. Note that the unit exposure dose represents different energies in the CW vs. FPP modes as they are significantly different in both duration time and electromagnetic spectrum. From this, we defined a relative exposure dose Er the ratio between the actual exposure energy and the unit exposure dose. We used Er = {1, 1.26, 1.59, 2, 2.52, 3.18, 4} across both the CW and FPP modes to polymerize our spoke pattern structure and assess the unresolved fractions for each mode.
Figures 2(a) and 3(b) show bright-field microscopy images of the resultant structures for the CW exposure and FPP exposure modes, respectively. We noted two trends: 1) that the peripheral parts of the spoke pattern are often well-resolved due to their large relative spacing, while the centers are difficult to resolve due to their small relative spacing; and 2) that a higher total exposure dose leads to a larger unresolved area. The outer diameter of the spoke is 1.9 mm, and the unresolved diameter is less than 0.4 mm for all samples. The relation between the exposure energy and unresolved fraction is plotted in Figure 2(c). As can be seen from the plot, the FPP exposure mode always has a better resolution than that of the continuous mode. For the CW mode, it is clear that the unresolved fraction is strongly-dependent on the exposure dose, while for the FPP exposure mode, the unresolved fraction is insensitive to the exposure energy. When Er = 1, the unresolved region resulting from FPP is 82% as large as that of when using CW. When Er = 4, the unresolved region resulting from FPP is 23% as large as that of when using CW. These results show that using FPP can achieve better capability in resolving fine structures than using CW, and by using a flashing light source, the tolerance window of exposure dose is significantly broadened while simultaneously increasing fabrication resolution, thus significantly simplifying the optimization process.
Figure 2. Resolution test for the CW exposure mode and FPP exposure mode.
(a) Patterns printed with CW exposure at different relative energies. (b). Patterns printed with FPP exposure at different relative energies. (c) Relation between the relative energy and unresolved fraction. Yellow and red circles in (a) and (b) indicate the outer diameter and the unresolved diameter, respectively. Scale bar = 500 μm.
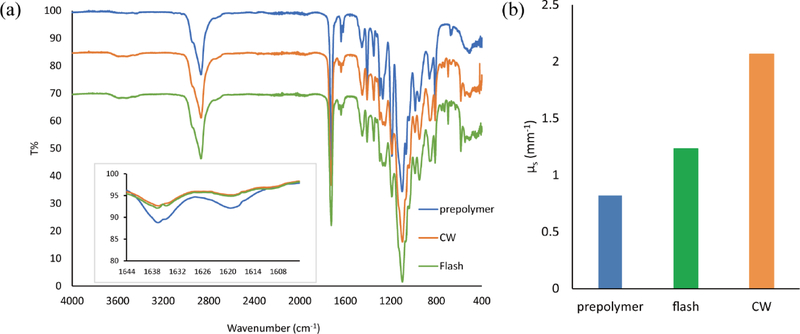
Figure. 3. Scattering changes during polymerization.
(a) FTIR spectrum of pure PEGDA (Mn = 575), dehydrated PEGDA slab polymerized with FPP exposure, and dehydrated PEGDA slab polymerized by CW exposure. Inset: zoom-in view at around 1630 cm−1. (b) Scattering coefficient of PEGDA prepolymer solution, PEGDA slab polymerized with FPP exposure, and PEGDA slab polymerized by CW exposure at 365 nm.
3. Theory and mechanism
3.1. Material scattering
Scattering is a significant factor in resolution deterioration, and there are three main factors that determine optical scattering in polymers. The first factor is the size of the molecules themselves – in a homogeneous polymer system, Rayleigh scattering dominates, where the intensity is proportional to the molecular weight of the polymer.[23] The second factor is the degree of crystallinity, where some polymers can form micron-size crystallites which induce strong Mie scattering.[26] The third factor is phase separation. Typically, a polymer has porous microstructures if it is polymerized from a monomer in solution because local solubility decreases as the polymer chain length increases. As polymerization continues, system homogeneity decreases as a result of phase separation, making it highly scattering.[27] In photopolymerization-based 3D printing, all three of these effects may occur and compound – light exposure induces molecular weight increase and thus Rayleigh scattering also increases. As the liquid-state prepolymer starts to solidify, crystallites also begin forming, thus causing further light scattering. In cases where the prepolymer contains solvents (e.g. hydrogels), the scattering phenomenon can be even stronger due to the resultant phase separation. Altogether, opacification of the material leads to nonspecific exposure and polymerization in undesired areas, resulting in deteriorated fabrication resolution.
In order to demonstrate that the material opacifies as it polymerizes, we fabricated slab samples with 50% PEGDA and 4% LAP via both CW and FPP methods and measured their monomer conversion rates and scattering coefficients at 365 nm. A FTIR spectroscope was used to measure the infrared (IR) transmittance of the unpolymerized PEGDA, the slabs polymerized via CW, and the slabs polymerized via FPP, the results of which are shown in Figure 3(a). As compared to unpolymerized PEGDA, the alkene groups were consumed after exposure as shown in the reduction of absorption peak of C=C bond around 1630 cm−1.[28,29] The conversion rate of PEGDA polymer after CW and FPP exposure was calculated to be 27.5% and 22.2%, respectively.
Separately, a UV-Vis-NIR spectroscope with integrating sphere was used to measure the scattering property of the same three sample types. The scattering coefficients were calculated with the Inverse Adding-Doubling (IAD) algorithm, which is widely used in calculating the scattering coefficient and absorption coefficient of thick biological tissue. [30,31] The scattering coefficients at 365 nm were calculated to be 0.82 mm−1, 1.23 mm−1, and 2.07 mm−1 for the prepolymer sample, slab polymerized via FPP, and slab polymerized via CW exposure, respectively (Figure 3(b)). This shows that as the PEGDA prepolymer undergoes photopolymerization either by CW or FPP exposure, its scattering coefficient increases. A high-speed camera was used to record this opacification in real-time to further validate the opacification phenomenon, shown in Figure S4.
3.2. Photopolymerization kinetics
Using FPP we can chronologically separate the light exposure event from downstream polymerization and opacification effects, the mechanism of which can be explained by photopolymerization kinetics. The free-radical photopolymerization process can be divided into three stages: 1) photoinitiation, where upon exposure to light, a photoinitiator molecule is homolytically-cleaved into two free radicals. These react with monomers and then become active propagating chains. 2) The second stage is propagation, where the initial chains continue to react with monomers and grow longer. 3) The third is termination, where an active chain stops propagating after combining with a free radical or another propagating chain.[32]
The initiation rate is proportional to the photoinitiator quantum yield Φ and photon absorption quantity per unit volume per unit time Nabs. The reaction rate of chain propagation rp is proportional to reactive functional group concentration [M], propagating chain concentration [P*], and chain propagation rate coefficient kp. The reaction rate of chain termination is proportional to the square of propagating chain concentration [P*] and chain termination rate coefficient kt. The initiation rate, propagation rate and termination rate are given by Equations (1)–(3). [23]
| (1) |
| (2) |
| (3) |
The change of reactive functional group concentration and propagating chain concentration are given by Equations (4), (5).
| (4) |
| (5) |
As the prepolymer solution becomes more viscous, both the propagation kinetic constant and termination kinetic constant decrease during polymerization reaction. According to the well-established diffusion-controlled free-radical polymerization model,[28,33–37] the propagation rate coefficient kp and the termination rate coefficient kt can be determined by Equations (6) and (7), [28,37]
| (6) |
| (7) |
where kp0 is the propagation rate coefficient without diffusion control; kp,D characterizes the diffusion-controlled part of the propagation reaction; kt,sD is the segmental-diffusion-controlled termination rate coefficient; kt,TD is the translational-diffusion-controlled termination rate coefficient; kt,RD is the reaction-diffusion-controlled termination rate coefficient. Additional equations and constants to model the photopolymerization process are available in the Experimental Section.
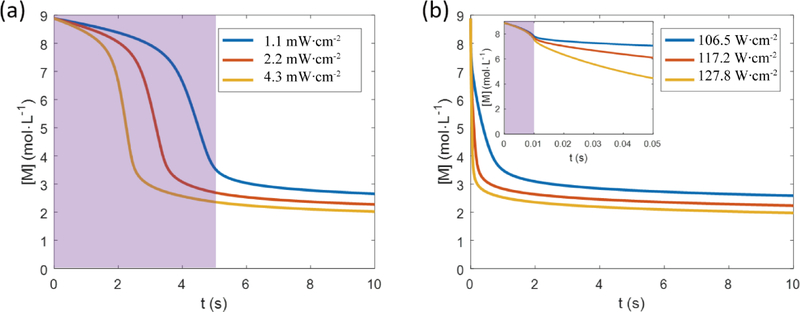
Here we use the model described above to numerically solve the photopolymerization kinetics problem in two scenarios. For both scenarios, the material system is 100% PEGDA with 4% (w/v) Irgacure 784 photoinitiator. Sample thickness is 100 microns. The photoinitiator has an absorbance A = 1.6 at 365 nm at 1 cm thickness at 0.1% concentration, thus A = 0.64 at 100 micron thickness at 4% concentration. We assume that the quantum efficiency of the photoinitiator is 1. In the first scenario, there is a low-intensity CW exposure lasting for 5 seconds. We simulated the photopolymerization kinetics under the light illumination intensity at 1.1 Mw·cm−2, 2.2 mW·cm−2, or 4.3 mW·cm−2. In the second scenario, there is a flashing exposure, which lasts for 10 milliseconds. We simulated the average flashing intensity at 106.5 W·cm−2, 117.2 W·cm−2, or 127.8 W·cm−2. (See Table S1 for the relation between illumination intensity and free radical generation rate).
The simulation results are shown in Figure 4(a) and (b), respectively. The shaded area in Figure 4 indicates the light exposure period. The material properties and kinetic parameters of PEGDA have been studied previously [28] and are summarized in the Experimental Section.
FIGURE 4. PEGDA photopolymerization simulation of the CW mode and the FPP mode.
(a) Unconverted functional group concentration versus time. The system is subject to a 5-second light exposure under the light illumination intensity at 1.1 mW·cm−2, 2.2 mW·cm−2, or 4.3 mW·cm−2. (b) Unconverted functional group concentration versus time. The system is subject to a 10-millisecond light exposure at 106.5 W·cm−2, 117.2 W·cm−2, or 127.8 W·cm−2. The inset of (b) is a zoom-in view in time scale. The shaded area indicates the light exposure period.
From Figure 4, we can see that both scenarios reach a similar final conversion rate, showing that even a short exposure is sufficient to photopolymerize the monomer. The difference is that in the CW exposure scenario, polymerization and opacification begins and continues to occur while the light is still on, and the aforementioned scattering effects will deflect light outside of the desired areas. In the FPP scenario, the material can ‘safely’ polymerize and opacify in darkness, with no light to scatter into undesired areas.
4. Conclusion
We have successfully developed a flashing photopolymerization (FPP) method for photopolymerization-based 3D printing. By using a brief flash exposure instead of a continuous exposure, the material remains optically clear during the exposure period, thus minimizing light scattering and resulting in finer fabrication resolution. Both theoretical analysis and experimental demonstration have revealed the different scattering effects associated with CW vs. FPP exposure modes. By chronologically separating the light exposure event from the polymerization and opacification events, one can significantly improve the fidelity of 3D-printed structures. This is particularly significant for microscale 3D printing where scattering effects can have significant impacts on the feature sizes necessary for microstructure formation, such as hydrogel 3D printing for bioengineering.
5. Experimental Section
Materials
PEGDA (Mn = 575 Da) was purchased from Sigma-Aldrich. Irgacure 784 was purchased from Ciba Specialty Chemicals, now a subsidiary of BASF. LAP was synthesized in-house following previously-published methods.[38] The xenon flash tube was purchased from Xenon Flash Tubes.
Simulation of photopolymerization
The propagation and termination kinetic of the system is related to the degree of conversion, as shown in Equations (8)–(12), [28,37]
| (8) |
| (9) |
| (10) |
| (11) |
| (12) |
where X is the degree of conversion; [M] is the unconverted functional group (C=C double bond) concentration, [M]0 is the initial unconverted functional group concentration; c is the relative viscosity coefficient; kp,D0 is the diffusion-controlled propagation rate coefficient at zero conversion; kt,TD0 and kt,SD0 are the translational-diffusion-controlled termination rate coefficient and the segmental-diffusion-controlled termination rate coefficient at zero conversion; CRD is the reaction-diffusion proportion parameter.
The material properties and kinetic parameters of PEGDA (Mn = 250) determined by Wu, et. al, are listed in Table 1. [28] Matlab was used to perform the numerical simulation.
Table 1.
Material properties and kinetic parameters of PEGDA
| [M0] | 8.88 mol·L−1 | c | 34.149 |
| kp0 | 1860 L·mol−1·s−1 | kp,D0 | 8.994 × 1011 L·mol−1·s−1 |
| kt,SD | 4.39 × 106 L·mol−1·s−1 | kt,TD0 | 1.002 × 107 L·mol−1·s−1 |
| CRD | 1.0146 |
Infrared spectrum measurement
IR spectrum measurements were performed on a Perkin Elmer Spectrum Two FTIR spectroscope. Polymerized samples were dried to eliminate the influence of the spectrum of water; first by snap-freeze in liquid nitrogen, then dried by lyophilization (Labconco Freezone, lyophilize at −55 °C for 3 days).
Scattering coefficient measurement
Three samples were prepared for the measurement in a UV-Vis-NIR spectroscope (Perkin Elmer, Lambda 1050). The prepolymer solution (50% PEGDA, 4% LAP) is loaded in a 1 mm wide glass container. The FPP sample is polymerized by a single flash (20 J) into a 1 mm slab. The CW sample is polymerized by UV-LED (0.4 mW cm−2, 10 s) into a 1 mm slab.
By using the integrating sphere, the diffusive reflectance Rd, total reflectance Rt, diffusive transmittance Td, and total transmittance Tt at 365 nm wavelength are measured. Then we used Inverse Adding-Doubling (IAD) algorithm to calculate the scattering coefficient of the samples. The executable program of IAD algorithm was acquired from https://omlc.org/software/iad/index.html, copyright 2017 Scott Prahl.
Supplementary Material
ACKNOWLEDGMENT
This work was supported in part by National Institutes of Health (R21AR074763, R33HD090662, and R01EB021857) and National Science Foundation (NSF) (CMMI-1644967). Part of the work is performed at San Diego Nanotechnology Infrastructure (SDNI) of UCSD, a member of the National Nanotechnology Coordinated Infrastructure (NNCI), which is supported by NSF (Grant ECCS-1542148). This material is based upon work supported by the National Science Foundation Graduate Research Fellowship Program under Grant No. DGE-1650112 to JS.
Footnotes
The authors declare no conflict of interest.
Publisher's Disclaimer: This is a PDF file of an unedited manuscript that has been accepted for publication. As a service to our customers we are providing this early version of the manuscript. The manuscript will undergo copyediting, typesetting, and review of the resulting proof before it is published in its final citable form. Please note that during the production process errors may be discovered which could affect the content, and all legal disclaimers that apply to the journal pertain.
REFERENCES
- [1].Tumbleston JR, Shirvanyants D, Ermoshkin N, Janusziewicz R, Johnson AR, Kelly D, Chen K, Pinschmidt R, Rolland JP, Ermoshkin A, Samulski ET, DeSimone JM, Continuous liquid interface production of 3D objects, Science. 347 (2015) 1349–1352. doi: 10.1126/science.aaa2397. [DOI] [PubMed] [Google Scholar]
- [2].Sun C, Fang N, Wu DM, Zhang X, Projection micro-stereolithography using digital micro-mirror dynamic mask, Sensors and Actuators A: Physical. 121 (2005) 113–120. doi: 10.1016/j.sna.2004.12.011. [DOI] [Google Scholar]
- [3].Gauvin R, Chen Y-C, Lee JW, Soman P, Zorlutuna P, Nichol JW, Bae H, Chen S, Khademhosseini A, Microfabrication of complex porous tissue engineering scaffolds using 3D projection stereolithography, Biomaterials. 33 (2012) 3824–3834. doi: 10.1016/j.biomaterials.2012.01.048. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [4].Han L-H, Easley JA, Ellison CJ, Chen S, Fluorinated Colloidal Emulsion of Photochangeable Rheological Behavior as a Sacrificial Agent to Fabricate Organic, Three-Dimensional Microstructures, Langmuir. 26 (2010) 6108–6110. doi: 10.1021/la100014k. [DOI] [PubMed] [Google Scholar]
- [5].Soman P, Tobe BTD, Lee JW, Winquist AAM, Singec I, Vecchio KS, Snyder EY, Chen S, Three-dimensional scaffolding to investigate neuronal derivatives of human embryonic stem cells, Biomedical Microdevices. 14 (2012) 829–838. doi: 10.1007/s10544-012-9662-7. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [6].Zhang AP, Qu X, Soman P, Hribar KC, Lee JW, Chen S, He S, Rapid Fabrication of Complex 3D Extracellular Microenvironments by Dynamic Optical Projection Stereolithography, Advanced Materials. 24 (2012) 4266–4270. doi: 10.1002/adma.201202024. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [7].Zhu W, Ma X, Gou M, Mei D, Zhang K, Chen S, 3D printing of functional biomaterials for tissue engineering, Current Opinion in Biotechnology. 40 (2016) 103–112. [DOI] [PubMed] [Google Scholar]
- [8].Zhu W, Tringale KR, Woller SA, You S, Johnson S, Shen H, Schimelman J, Whitney M, Steinauer J, Xu W, Rapid continuous 3D printing of customizable peripheral nerve guidance conduits, Materials Today. (2018). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [9].Berry DB, You S, Warner J, Frank LR, Chen S, Ward SR, A 3D tissue-printing approach for validation of diffusion tensor imaging in skeletal muscle, Tissue Engineering Part A. 23 (2017) 980–988. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [10].Zhu W, Qu X, Zhu J, Ma X, Patel S, Liu J, Wang P, Lai CSE, Gou M, Xu Y, Zhang K, Chen S, Direct 3D bioprinting of prevascularized tissue constructs with complex microarchitecture, Biomaterials. 124 (2017) 106–115. doi: 10.1016/j.biomaterials.2017.01.042. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [11].Qu X, Zhu W, Huang S, Li Y-S, Chien S, Zhang K, Chen S, Relative impact of uniaxial alignment vs. form-induced stress on differentiation of human adipose derived stem cells, Biomaterials. 34 (2013) 9812–9818. doi: 10.1016/j.biomaterials.2013.09.009. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [12].Xing J-F, Zheng M-L, Duan X-M, Two-photon polymerization microfabrication of hydrogels: an advanced 3D printing technology for tissue engineering and drug delivery, Chemical Society Reviews. 44 (2015) 5031–5039. doi: 10.1039/C5CS00278H. [DOI] [PubMed] [Google Scholar]
- [13].Soman P, Fozdar DY, Lee JW, Phadke A, Varghese S, Chen S, A three-dimensional polymer scaffolding material exhibiting a zero Poisson’s ratio, Soft Matter. 8 (2012) 4946. doi: 10.1039/c2sm07354d. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [14].Chia HN, Wu BM, Recent advances in 3D printing of biomaterials, Journal of Biological Engineering. 9 (2015) 4. doi: 10.1186/s13036-015-0001-4. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [15].Brown TE, Anseth KS, Spatiotemporal hydrogel biomaterials for regenerative medicine, Chemical Society Reviews. 46 (2017) 6532–6552. doi: 10.1039/C7CS00445A. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [16].Soman P, Lee JW, Phadke A, Varghese S, Chen S, Spatial tuning of negative and positive Poisson’s ratio in a multi-layer scaffold, Acta Biomaterialia. 8 (2012) 2587–2594. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [17].del Campo A, Greiner C, SU-8: a photoresist for high-aspect-ratio and 3D submicron lithography, Journal of Micromechanics and Microengineering. 17 (2007) R81–R95. doi: 10.1088/0960-1317/17/6/R01. [DOI] [Google Scholar]
- [18].You S, Zhu W, Wang P, Chen S, Projection Printing of Ultrathin Structures with Nanoscale Thickness Control, ACS Applied Materials & Interfaces. 11 (2019) 16059–16064. doi: 10.1021/acsami.9b02728. [DOI] [PubMed] [Google Scholar]
- [19].Bird RB, Stewart WE, Lightfoot EN, Transport phenomena, John Wiley & Sons, 2007. [Google Scholar]
- [20].Terazima M, Nogami Y, Tominaga T, Diffusion of a radical from an initiator of a free radical polymerization: a radical from AIBN, Chemical Physics Letters. 332 (2000) 503–507. doi: 10.1016/S0009-2614(00)01298-7. [DOI] [Google Scholar]
- [21].Donkers RL, Leaist DG, Diffusion of Free Radicals in Solution. TEMPO, Diphenylpicrylhydrazyl, and Nitrosodisulfonate, The Journal of Physical Chemistry B. 101 (1997) 304–308. doi: 10.1021/jp961957k. [DOI] [Google Scholar]
- [22].Reed W, Guterman L, Tundo P, Fendler JH, Polymerized surfactant vesicles: kinetics and mechanism of photopolymerization, Journal of the American Chemical Society. 106 (1984) 1897–1907. doi: 10.1021/ja00319a001. [DOI] [Google Scholar]
- [23].Carraher CE Jr, Introduction to polymer chemistry, CRC press, 2017. [Google Scholar]
- [24].Cowie JMG, Arrighi V, Polymers: chemistry and physics of modern materials, CRC press, 2007. [Google Scholar]
- [25].Scherzer T, Decker U, Real-time FTIR–ATR spectroscopy to study the kinetics of ultrafast photopolymerization reactions induced by monochromatic UV light, Vibrational Spectroscopy. 19 (1999) 385–398. [Google Scholar]
- [26].Auger J-C, Barrera RG, Stout B, Scattering efficiency of clusters composed by aggregated spheres, Journal of Quantitative Spectroscopy and Radiative Transfer. 79–80 (2003) 521–531. doi: 10.1016/S0022-4073(02)00305-9. [DOI] [Google Scholar]
- [27].Seeboth A, Lotzsch D, Potechius E, Phase transitions and phase separations in aqueous polyether systems, Colloid Polym Sci. 279 (2001) 696–704. doi: 10.1007/s003960000474. [DOI] [Google Scholar]
- [28].Wu J, Zhao Z, Hamel CM, Mu X, Kuang X, Guo Z, Qi HJ, Evolution of material properties during free radical photopolymerization, Journal of the Mechanics and Physics of Solids. 112 (2018) 25–49. doi: 10.1016/j.jmps.2017.11.018. [DOI] [Google Scholar]
- [29].Hsueh Y-H, Liaw W-C, Kuo J-M, Deng C-S, Wu C-H, Hydrogel Film-Immobilized Lactobacillus brevis RK03 for γ-Aminobutyric Acid Production, International Journal of Molecular Sciences. 18 (2017) 2324. doi: 10.3390/ijms18n2324. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [30].Mesradi M, Genoux A, Cuplov V, Abi Haidar D, Jan S, Buvat I, Pain F, Experimental and analytical comparative study of optical coefficient of fresh and frozen rat tissues, Journal of Biomedical Optics. 18 (2013) 117010. doi: 10.1117/1.JBO.18.11.117010. [DOI] [PubMed] [Google Scholar]
- [31].Prahl SA, van Gemert MJC, Welch AJ, Determining the optical properties of turbid media by using the adding–doubling method, Applied Optics. 32 (1993) 559. doi: 10.1364/AO.32.000559. [DOI] [PubMed] [Google Scholar]
- [32].Bowman CN, Kloxin CJ, Toward an enhanced understanding and implementation of photopolymerization reactions, AIChE Journal. 54 (2008) 2775–2795. doi: 10.1002/aic.11678. [DOI] [Google Scholar]
- [33].Goodner MD, Lee HR, Bowman CN, Method for determining the kinetic parameters in diffusion-controlled free-radical homopolymerizations, Industrial & Engineering Chemistry Research. 36 (1997) 1247–1252. [Google Scholar]
- [34].Goodner MD, Bowman CN, Modeling primary radical termination and its effects on autoacceleration in photopolymerization kinetics, Macromolecules. 32 (1999) 6552–6559. [Google Scholar]
- [35].Gleeson MR, Sheridan JT, Nonlocal photopolymerization kinetics including multiple termination mechanisms and dark reactions Part I Modeling, Journal of the Optical Society of America B. 26 (2009) 1736. doi: 10.1364/JOSAB.26.001736. [DOI] [Google Scholar]
- [36].Goodner MD, Bowman CN, Development of a comprehensive free radical photopolymerization model incorporating heat and mass transfer effects in thick films, Chemical Engineering Science. 57 (2002) 887–900. doi: 10.1016/S0009-2509(01)00287-1. [DOI] [Google Scholar]
- [37].Buback M, Free-radical polymerization up to high conversion. A general kinetic treatment, Die Makromolekulare Chemie. 191 (1990) 1575–1587. doi: 10.1002/macp.1990.021910710. [DOI] [Google Scholar]
- [38].Fairbanks BD, Schwartz MP, Bowman CN, Anseth KS, Photoinitiated polymerization of PEG-diacrylate with lithium phenyl-2,4,6-trimethylbenzoylphosphinate: polymerization rate and cytocompatibility, Biomaterials. 30 (2009) 6702–6707. doi: 10.1016/j.biomaterials.2009.08.055. [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.