Kelp beds as coastal protection: wave attenuation of Ecklonia radiata in a shallow coastal bay

Abstract

Background and Aims

Coastal protection from erosion and flooding is a significant ecosystem service provided by vegetated marine systems. Kelp beds are a dominant habitat-forming species on temperate reefs worldwide. While they are valued as hotspots of biodiversity, there is a paucity of information that supports their use in nature-based coastal defence. This includes the effectiveness of kelp beds in attenuating waves approaching the shore and how this influences sediment transport.

Methods

Wave loggers were deployed at paired kelp bed and control (urchin barren) treatments at four sites in Port Phillip Bay, Australia. The significant wave height offshore (exposed side) to onshore (sheltered side) of the treatment were compared to determine wave attenuation.

Key Results

At three sites, the wave attenuation of kelp beds was significantly less than that of the control. This result was consistent across the environmental conditions recorded in this study. At the fourth site, on average there was no significant difference in wave transmission between kelp and control. However, wave attenuation at kelp beds was 10 % greater than the control during periods of northerly winds. We highlight the importance of disentangling the effects of the reef substratum and kelp when evaluating the efficacy of kelp at providing coastal protection.

Conclusions

We have highlighted a significant gap in the research on ecosystem services provided by kelp beds. A greater understanding is needed on which kelp species are able to provide coastal protection, and under what conditions. Such future research is essential for providing managers and policy makers with actionable information on sustainable and cost-effective solutions for coastal defence when faced with a changing climate.

INTRODUCTION

Kelp is a dominant habitat-forming organism in temperate coastal reef systems worldwide (Steneck et al., 2002). Kelp beds provide a number of ecosystem services such as the provision of habitat that supports high biodiversity (Teagle et al., 2017), productive fisheries (Bertocci et al., 2015), nutrient cycling (Bennett et al., 2016) and recreation (Menzel et al., 2013), and thus have high ecological and socio-economic value. Recently, there has been increasing interest in whether coastal habitats, including kelp beds (Duarte et al., 2013; Ferrario et al., 2014), can provide another highly valued ecosystem service – coastal protection. Climate change and coastal urbanization are increasing the risk of erosion and flooding along coastlines globally (Kittinger and Ayers, 2010; Hinkel et al., 2014). Future climate change is predicted to intensify the drivers of coastal hazards through increases in sea level, greater wave height and more extreme storm events (Young et al., 2011; IPCC, 2014). As urbanization along our coastlines continues to grow, a greater number of people and infrastructure are threatened by these hazards. Identifying the appropriate solutions for protection from contemporary and future hazards is one of the greatest challenges facing coastal communities today (Morris et al., 2018).

Armouring the coast with ‘hard’ engineered structures, such as seawalls and breakwaters, is currently the most common solution for defence; however, these structures are becoming less environmentally and economically sustainable. Financial costs of building and maintaining these structures under future climate change scenarios are significant (Hinkel et al., 2014). Equally, substantial ecological impacts are caused through the replacement of natural habitats with artificial structures, and the introduction of novel substrata for colonization, which are often hotspots for invasive species (Bulleri and Chapman, 2010). In response, there is increasing research investigating the value of natural ecosystems, such as biogenic reefs, dunes, beaches and vegetation, to provide protection against erosion and waves, with the benefit that these systems can adapt to changes in climate, self-repair after major storm events and provide co-benefits in terms of other ecosystem services (e.g. biodiversity provision, productive fisheries or bioremediation; Gittman et al., 2014; Morris et al., 2018).

There are still a number of barriers to the investment in what is often called ‘natural and nature-based infrastructure’ (Sutton-Grier et al., 2018), not least of which are quantitative data that support which habitats are effective at providing coastal defence, the types of environments in which they work best and the habitat characteristics that promote long-term protection (Bouma et al., 2014; Morris et al., 2018). Further, this research is more advanced for some habitats (e.g. coral reefs and saltmarsh; Shepard et al., 2011; Ferrario et al., 2014) than others (e.g. kelp beds; Smale et al., 2013; Narayan et al., 2016; Morris et al., 2018), where there are too few studies across different locations to make informed decisions about the role these habitats play in coastal defence. This review presents the current state of knowledge on the ability of kelp beds to provide coastal protection, beginning briefly with an overview of the coastal processes that are important for this discussion. We extend the information on the effect of kelp on waves by presenting field data comparing significant wave height on subtidal reefs with and without kelp across multiple sites under different wave conditions.

Coastal processes in nearshore systems

Waves.

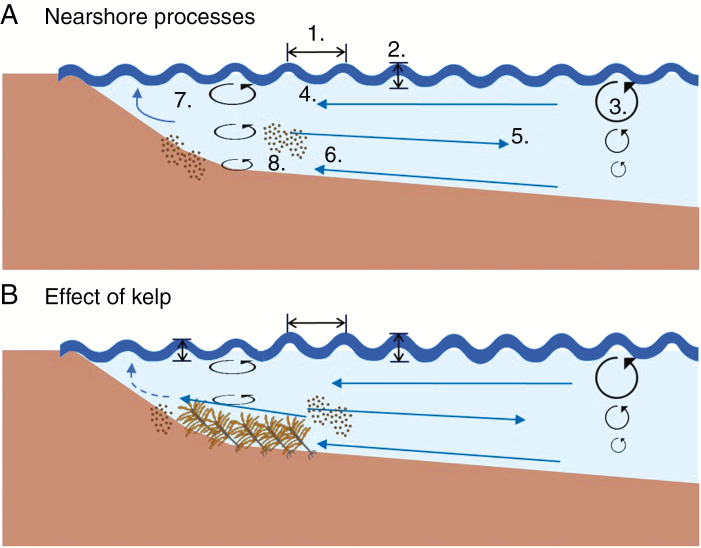
Waves are the primary force responsible for coastal erosion and sediment transport, and thus modification of the coastline (Holman, 1995). Waves have crests (the peak of the wave) and troughs (the lowest part of the wave) and are often characterized by the wave height (the difference in height between the crest and trough), wavelength (the distance between two crests or troughs) and period (the time between successive wave crests or troughs) (Fig. 1A). The wave height, length and period increase with greater wind speed, duration and fetch (distance over water that the wind blows). It should be acknowledged that waves can be driven by mechanisms other than wind, such as earthquakes and tides; however, ordinary wind or ‘gravity’ waves are most common, and are characterized by periods of 0.25–30 s (Davidson-Arnott, 2010). As the wave crest approaches, water particles within the wave move upwards and forwards, and downwards and backwards during the trough, creating orbital motion (Fig. 1A). In deep water (when the depth is greater than half the wavelength), the orbit is circular and decreases exponentially with depth, such that the effect of the seafloor on surface waves is negligible (Koch et al., 2006). In intermediate and shallow water (when the depth is less than half the wavelength) however, the waves drive orbital motion at the bed. The seafloor affects the shape of the orbit and it becomes elliptical, resulting in a change in orbital motion and surface wave form (Fig. 1A). The wave height is reduced in shallow water as energy is lost through bottom friction and increased wave breaking (Koch et al., 2006). In addition, the orbital motion at the bed creates shear stress and sediment transport (Van Rijn, 1993).

Fig. 1.

(A) Waves are characterized by wavelength, period (1) and wave height (2), and orbital motion is created as waves propagate (3). Stokes drift (4) and boundary layer streaming at the bed (6) promote onshore currents, while a net offshore current is generated through the undertow (5) and longshore currents by oblique waves (7). Waves and currents drive sediment motion and transport (8). (B) Kelp beds may reduce the longshore current and wave energy, in addition to creating onshore currents, which can promote the movement of sediment onshore.

Currents.

Vertical gradients in oscillatory velocity drive mean currents in the direction of wave propagation, such as Stokes drift at the water surface (Kenyon, 1969) and boundary layer streaming at the bed (Kranenburg et al., 2012). Wave breaking also leads to an increase in mean water level towards the shoreline, termed wave set-up. The onshore flow of water with waves must reach a dynamic equilibrium with water returning offshore. Between the wave trough and sea bed, an undertow develops where mean flow is directed offshore (Christensen et al., 2002; Fig. 1A). In addition to cross-shore currents, waves that approach at an angle to the shore produce longshore currents (Davidson-Arnott, 2010). The strength of the currents is influenced by the size of wave set-up, but can also be affected by local winds and tides. Offshore coastal winds reduce the surface onshore flow of swell waves, and thus reduce the strength of the undertow, whereas the opposite is seen when onshore winds enhance set-up at the shoreline (Davidson-Arnott, 2010). In areas where orbital motion from waves is weak, tides can also cause significant longshore currents and thus can play an important role in sediment transport (Davidson-Arnott, 2010).

Sediment response.

Wave breaking and oscillatory motion cause sediment suspension, the transport of which then depends on an interaction of wave and current patterns. On energetic coasts, fine sediments (e.g. silt and clay) are usually kept in suspension and are either deposited in deeper water offshore, or in sheltered estuaries and bays, which is often promoted by the presence of vegetation, such as mangroves or seagrass (Ward et al., 1984). Coarser particles of sand and gravel though are often exchanged cross- and alongshore, which can result in short- or long-term changes in the shore profile (Pruszak et al., 2011). During storms, large waves erode sediment from the beach and dunes, and it is transported by offshore currents (Lu et al., 2015). During periods of calm wave conditions, the oscillatory motion is not strong enough to cause sediments to be suspended high in the water column, and flow through the undertow offshore is weak, which results in the promotion of onshore sediment movement (Lu et al., 2015). For longshore movement, net sediment transport depends on the magnitude and frequency of waves from all directions, and thus is a function of wave climate and local shore orientation (Miller et al., 2011), and can lead to localized erosion and accretion patterns. Natural shorelines are characterized by periods of progradation and erosion as a result of the processes described, which can be influenced by the presence of coastal features, such as headlands and subtidal reefs (George et al., 2015). Sediment can also be accumulated artificially using man-made coastal protection structures (e.g. groynes or breakwaters). However, interference of coastal processes through the placement of artificial structures is often the cause of human-induced problems with locally enhanced erosion (e.g. Fletcher et al., 1997; Ranasinghe and Turner, 2006).

The response of kelp to the hydrodynamic environment

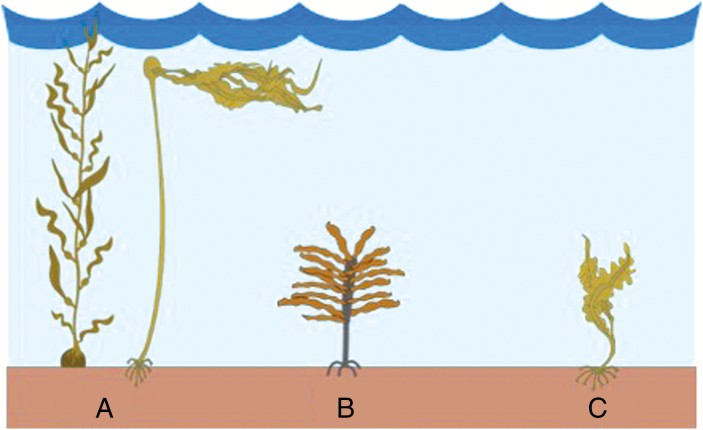
Kelps are large, canopy-forming brown algae in the order Laminariales (Dayton, 1985). Kelp species can be grouped into three broad guilds based on their morphology: floating canopy (large species with fronds at or near the surface, e.g. Macrocystis and Nereocystis); stipitate (erect understorey where fronds are supported by a stipe above the understorey, e.g. Ecklonia) and prostrate canopy (fronds lie on or immediately above the sub-stratum, e.g. some Laminaria spp.) (Dayton, 1985; Fig. 2). Within a species, morphology can vary widely according to environmental conditions, such as light availability, nutrient concentrations, temperature, wave conditions and biotic factors (Wernberg et al., 2003; Shibneva and Skriptsova, 2015). Kelps are found in sheltered and wave-swept environments; their flexibility allows them to reconfigure and reorientate under flow and still achieve the large sizes that would not normally be expected under high energy conditions (Denny and Cowen, 1997). Thallus streamlining through narrower, thicker and flatter fronds occurs in kelps in wave-exposed environments (Koehl et al., 2008), in addition to a thicker stipe and greater holdfast biomass (Fowler-Walker et al., 2006). Some kelps also display allometric growth, such that taller individuals have less blade material for their size than do shorter individuals (Gaylord and Denny, 1997). Morphology can also vary seasonally, with a reduction in biomass coincident with times of the year that experience the greatest wave energy (de Bettignies et al., 2013, 2015). These adaptations have been found to be plastic traits, with changes occurring rapidly when individuals are exposed to a new environment (Fowler-Walker et al., 2006; Koehl et al., 2008). Streamlining reduces drag forces on the algae, whereas greater tenacity is a strategy to resist drag forces, and prevent breakage and/or dislodgement (Starko and Martone, 2016). Across different kelp species, a continuum of drag avoiders to drag tolerators has evolved, which may contribute to the maintenance of morphological diversity within this group (Starko and Martone, 2016). Just as kelp responds to water motion, kelp beds also modify water motion, altering the environment and available resources for organisms living within the kelp bed (Rosman et al., 2010).

Fig. 2.

Kelp guilds, (A) floating canopy, e.g. Macrocystis and Nereocystis; (B) stipitate, e.g. Ecklonia; and (C) prostrate canopy, e.g. some Laminaria species. Symbols are courtesy of the Integration and Application Network, University of Maryland Center for Environmental Science (ian.umces.edu/symbols/).

The effect of kelp on coastal processes in nearshore systems

Aquatic macrophytes have a significant effect on the structure of mean currents and surface waves through exerting drag in the water column (Okubo et al., 2001; Koch et al., 2006), which in turn can modify sediment transport processes (López and García, 1998). The capacity of coastal vegetation to provide protection against erosion and flooding is therefore dependent on their ability to exert drag. Thus, kelp-induced drag could create wave attenuation and potentially reduce sediment transport.

Vegetation characteristics such as height, density and individual stiffness, as well as hydrodynamic properties, such as wave height and period, wave–current interactions and water depth, have been identified as determinants for energy dissipation (Maza et al., 2015). In the following sections, we use these parameters as a framework to outline canopy-scale effects of kelp on current and wave attenuation and discuss possible implications for sediment transport and erosion mitigation.

Sensitivity of current attenuation potential to vegetation characteristics

The presence of coastal vegetation, such as kelp, (1) significantly reduces current velocity and (2) can generate a strong shoreward mean current at the canopy–water interface as a result of vertical gradients in oscillatory velocity (Abdolahpour et al., 2017). A reduction in current velocity occurs through two mechanisms: (1) a change in velocity profile shape in line with the vertical distribution of drag; and (2) a decrease in depth-averaged currents due to lateral flow deviation around the kelp bed (Rosman et al., 2010). This leads to an increase in current velocities at the edge of the bed, but a decrease within the bed (Jackson and Winant, 1983; Gaylord et al., 2007). Kelp has a particularly large effect on the alongshore (as opposed to cross-shore) current (Gaylord et al., 2007; Rosman et al., 2007; Fig. 1B), with a 3-fold reduction in current speeds and water residence times of up to a week observed within large kelp beds (e.g. 7 km long, 1 km wide Macrocystis bed; Jackson and Winant, 1983). In contrast, kelps have less effect on cross-shore currents (Jackson and Winant, 1983; Rosman et al., 2007). This translates into cross-shore flows being more important than longshore flows for transport of material via fluid exchange into the kelp bed (Jackson, 1997), especially for large, wide (kilometre-scale) kelp beds (Gaylord et al., 2007).

The distance that the longshore velocity profile takes to adjust to the vertical drag profile is affected by kelp density and stipe diameter (Rosman et al., 2010). The denser the canopy, the greater the bulk drag coefficient and current attenuation (Rosman et al., 2010). The effect of the kelp canopy can vary widely among species. For instance, Eualaria fistulosa, which has one large blade throughout the water column, attenuated currents less than Nereocystis luetkeana that has numerous blades at or near the surface (Hondolero and Edwards, 2017). This difference in current attenuation between the two species is restricted to the upper few metres of the water column where there is a prominent difference in kelp morphology. Even within the one species or canopy, however, the tidal cycle (Rosman et al., 2010) and season (Gaylord et al., 2007) can impact the biomass of the surface canopy. In addition, drag is impacted by a kelp’s flexibility. In comparison with early studies that used rigid cylinders to describe the interaction of vegetation with currents and waves (e.g. Kobayashi et al., 1993), the predicted rates of energy loss by flexible organisms are 2–20 times lower than they would be for rigid organisms (Gaylord et al., 2003). Flow development lengths (i.e. the distance until the velocity profile no longer changes) are greater for a reduction in current due to lateral flow deviation around the bed than due to a change in velocity profile shape (by an order of magnitude) (Rosman et al., 2010), thus larger kelp beds (>100 m) are predicted to have a greater effect on current attenuation (e.g. Gaylord et al., 2007).

Sensitivity of current attenuation potential to flow conditions

As currents become stronger, current attenuation by vegetation tends to decline (Lacy and Wyllie-Echeverria, 2011). This is due to the posture of flexible individuals, which bend and become more streamlined under increasing velocities (Luhar and Nepf, 2011). When individuals are pushed over in the flow, drag is exerted over less of the water column. This is also the case when the depth of water is increased. Thus, current attenuation is expected to be lower where hydrodynamic forcing by the current exceeds restoring forces (i.e. through vegetation buoyancy and stiffness) and/or where the water depth is greater than the canopy height (Luhar and Nepf, 2011).

Sensitivity of wave attenuation potential to vegetation characteristics

In the presence of waves, inertial forces act on kelp when an individual becomes fully extended, in addition to drag in both the onshore and offshore directions as the waves cycle over time (Denny and Cowen, 1997; Gaylord et al. 2008). These forces have the potential to alter the properties of waves as they propagate through the bed, resulting in the removal of wave energy (Rosman et al., 2013; Fig. 1B). Despite generalizations that coastal vegetation has a significant wave-damping effect, few studies have extended the effect of drag on currents within kelp beds to surface waves (Rosman et al., 2013); where available, data have provided variable results among studies.

Similar to current attenuation (discussed above), the importance of vegetation density on wave attenuation has been previously highlighted (e.g. Shepard et al., 2011). The stipitate kelp, Laminaria hyperborea, attenuated waves (Mork, 1996), and this wave damping increased with bed density (Dubi and Tørum, 1994). The leaf area index has been used for saltmarshes and seagrasses (Paul et al., 2012; Maza et al., 2015), which is defined as the total one-sided leaf area per unit ground surface area (Watson, 1947). A greater leaf area index is positively correlated with wave attenuation, in addition to plant stiffness, which differs among species (Paul et al., 2012; Maza et al., 2015). Plants with greater flexibility need a greater leaf area index to achieve the same wave attenuation as stiffer plants (Paul et al., 2012). Kelps have traditionally been viewed to ‘go with the flow’ by moving passively with the wave cycles to minimize drag. In particular, large floating canopy species have previously been thought to have negligible effects on surface waves (Denny and Cowen, 1997; Gaylord et al., 2003). This may explain field measurements of a Macrocystis bed (13 m deep, 350 m wide), where there was no difference in wave attenuation between the kelp bed and control sites for waves of periods 3–20 s (Elwany et al., 1995), and similar results were found in a later study (Rosman et al., 2007).

Sensitivity of wave attenuation potential to wave conditions

Wave period and water depth are two key parameters that have been shown to affect wave attenuation. Greater wave attenuation has been observed to occur for shorter (2–6 s) rather than longer period waves (7–20 s) (Dubi and Tørum, 1996; Lowe et al., 2007; Maza et al., 2015). Furthermore, as depth increases, wave attenuation decreases, such that attenuation is negligible at 10 m for L. hyperborea (2 m tall individuals; Dubi and Tørum, 1996). This can be explained by the plant submergence ratio, where a shallower water depth implies that a higher fraction of the water column is affected by the vegetation, and is therefore also dependent on vegetation height (i.e. taller vegetation in shallower water depths creates greater wave attenuation; Maza et al., 2015).

A factor that is often not accounted for in laboratory studies is the interaction between currents and waves that would occur in natural systems. Alongshore currents can mitigate the effect of Stokes drift on floating-canopy kelp, reducing the inertial load and, thus, the extent of wave damping (Gaylord et al., 2003). Similarly, for saltmarshes, currents following and opposing wave direction decreased and increased wave attenuation, respectively, although this has not been tested for kelp (Maza et al., 2015).

Sediment transport

Any effect of kelp on currents and waves could in turn influence sediment transport and, thus, patterns of erosion and sedimentation (Fig. 1B). However, few studies have investigated this directly, or made the link between changes in hydrodynamics caused by kelp beds and subsequent impacts on shoreline profile (e.g. Elwany and Flick, 1996; but see Price et al., 1968; Lovas and Torum, 2001). Limited evidence suggests that where a kelp bed provides wave attenuation, this could build beach profiles by promoting the onshore transport of sediment (Price et al., 1968). This could be due to the onshore current created by submerged vegetation, which has the potential to carry sediment and support onshore sediment transport (Abdolahpour et al., 2017). However, in other cases, the effect of kelp on beach profiles was only relevant in periods of successive storms, where the presence of a kelp bed reduced dune damage by decreasing the time taken for dune re-equilibrium and erosion to end (Lovas and Torum, 2001).

Coastal protection by kelp beds: a summary

These variable impacts of vegetation on coastal processes create challenges for understanding the conditions under which natural habitats provide coastal protection. For example, floating-canopy kelp species are of a large enough size that they rarely become fully extended under oscillatory motion, and therefore have the ability to ‘go with the flow’, resulting in a potential negligible effect on wave attenuation (Friedland and Denny, 1995). In contrast, some of the stipitate kelps withstand wave energy through increasing strength, rather than flexibility (Koehl, 1984), and these species may be capable of significant wave attenuation under certain environmental conditions (Dubi and Tørum, 1996). There are, however, a limited number of studies that have investigated the coastal protection provided by kelp beds, with only a few species examined.

Here, we extend the current information on wave attenuation by kelp through presenting field data under different wave conditions for a stipitate species that has not been investigated previously, Ecklonia radiata. We focus on wave attenuation as this is the primary response elicited by offshore biogenic reefs that can contribute to coastal hazard reduction (Morris et al., 2018). It has been noted that in order to obtain a more mechanistic approach to wave attenuation, a greater number of field studies are needed to understand how wave damping changes under different conditions (Kobayashi et al., 1993; Pinsky et al., 2013). Once we have a greater understanding of how kelp influences hydrodynamic parameters, the link between the changes in these processes and coastal erosion then needs to be better understood.

The study was conducted in Port Phillip Bay, Victoria, Australia, which is characterized by short-period, wind-driven waves. Based on the previous work outlined above, it was predicted that under these wave conditions E. radiata could attenuate waves; however, this would depend on the density and submergence of the canopy across different sites.

MATERIALS AND METHODS

Characteristics of Ecklonia radiata

Ecklonia radiata is a dominant habitat-forming macroalga on temperate and subtropical reefs in Australia (Connell and Irving, 2008). It is a small, stipitate kelp, reaching a maximum length of 2 m, although this varies considerably along its distribution (e.g. 0.7 – 1.35 m in Wernberg et al., 2003). Ecklonia radiata is commonly studied in the shallow subtidal (1–15 m), but can be found up to 40–50 m deep (Marzinelli et al., 2015). The morphology of E. radiata differs at sheltered vs. wave-exposed sites (Fowler-Walker et al., 2006). Individuals at wave-exposed areas typically have a thicker stipe and thallus, but a smaller surface area, than individuals in sheltered environments (Fowler-Walker et al., 2006).

Experimental set-up

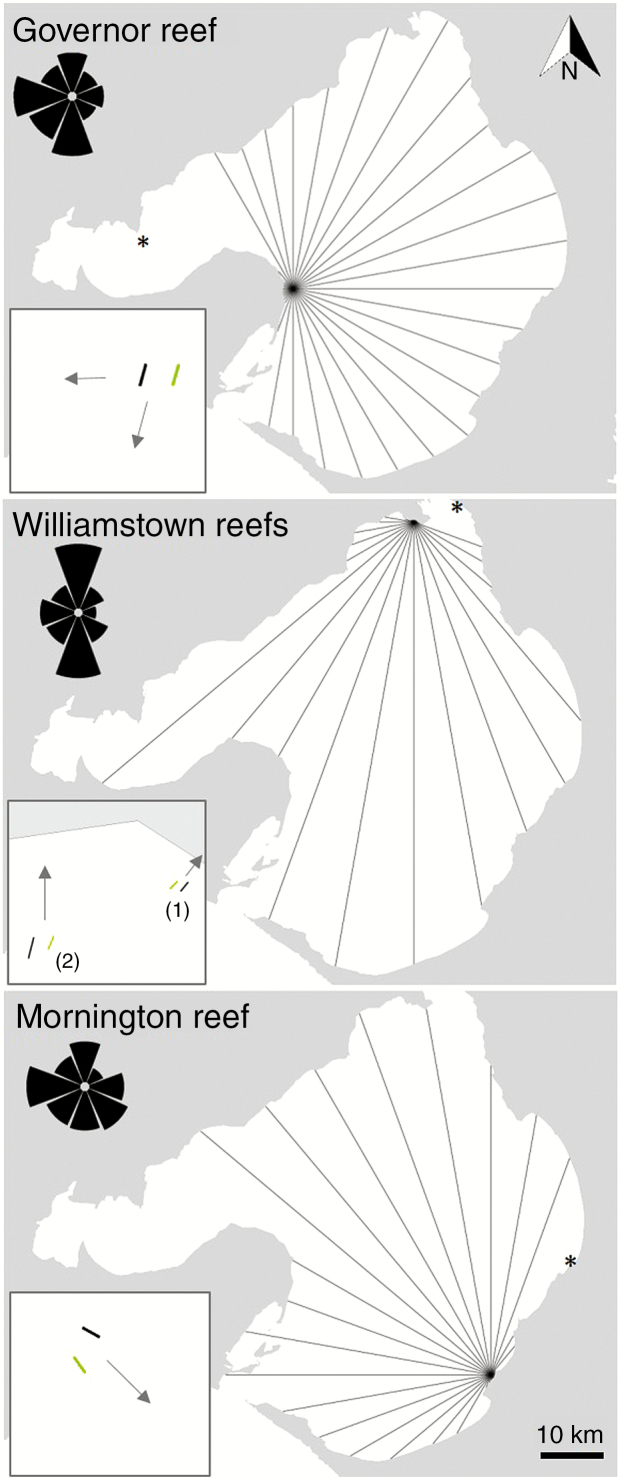
Four reefs were used in Port Phillip Bay: Governor Reef (–38.1473, 144.7332); Williamstown Reefs (–37.8692, 144.8940; two reefs) and Mornington Reef (–38.213794, 145.034339) (Fig. 3). These reefs were chosen as they have areas of urchin barrens (areas of reef where kelp has been deforested through urchin overgrazing; Filbee-Dexter and Scheibling, 2014) directly adjacent to intact E. radiata (hereafter ‘kelp’) beds. Wave loggers (RBR^®solo D wave; hereafter ‘RBR’) were deployed for approx. 2 weeks at each location between December 2017 and April 2018; deployment and retrieval of RBRs by divers was weather dependent. At each reef, six RBRs were deployed at a control (urchin barren) and kelp treatment; one each placed offshore, onshore and mid-reef (hereafter ‘midshore’). The RBRs were attached with cable ties (approx. 0.05 m above the bed) to star pickets that were hammered into the seabed. The RBRs were programmed (speed = 1 Hz; duration = 1024) to collect hourly wave data (significant wave height, H_s, in metres and associated period, T, in s). During retrieval of the RBRs, the benthic community along the transect between the onshore and offshore RBR at each treatment was surveyed. Photo-quadrats (1 m²) were taken by divers at approx. 5 m intervals, and the lamina length of each kelp (when present) was recorded, along with kelp density.

Fig. 3.

Study sites and fetch vectors for Governor Reef, Williamstown reefs and Mornington reef, Port Phillip Bay, Australia. The inset shows the transects for kelp (green) and control (black) relative to the shore (denoted with an arrow). The wind roses show the wind direction summary over the past 5 years (refer to Supplementary data Fig. S1 for details). *Indicates the closest weather observation station.

Data processing and analyses

To characterize the conditions at each site, wind roses were produced for the 5 year period from 2014 to 2018 (1 January 2014 to 3 January 2019) and compared with the conditions during RBR deployment. The data for the wind roses (wind speed in m s^–1 and wind direction in degrees) were obtained from the Australian Government’s Bureau of Meteorology (bom.gov.au) and used the weather stations closest to the sites (St Kilda, Frankston and Point Wilson for Williamstown, Mornington and Governor Reef, respectively; Fig. 3). Half hourly wind data points were used for the 5 year summary, whereas hourly observations were used for the RBR deployment summaries to match the sampling frequency of the RBRs. Wind fetch distances were calculated for each site using fetchR (Seers, 2018).

The pressure values recorded by the RBRs were corrected for atmospheric pressure by subtracting the air pressure recorded at the closest weather stations to each site (those that recorded atmospheric pressure were Geelong, Melbourne and Cerberus for Governor Reef, Williamstown and Mornington, respectively). Water densities were calculated using the Thermodynamic Equation of Seawater-2010 (TEOS-10; IOC et al., 2010), using water temperatures and salinities obtained from World Sea Temperatures (www.seatemperature.org). The corrected pressure data were then converted to water depth using this calculated water density [eqn (1)].

$${\displaystyle \begin{array}{l}{\displaystyle d=P/{\rho }_{\mathrm{w}}g}\end{array}}$$ (1)

where d is the water depth, P is the pressure, ρ_w is the density of water, and g is the acceleration due to gravity.

The water levels were linearly detrended to remove low-frequency signal, which provided an average water depth for each burst (of 1024 samples h^–1, as above) and a zero-average input for fast-Fourier transform. A pressure response factor, K_p, was determined for each frequency bin of the fast-Fourier transform [eqn (2); Kamphius, 2010].

$${\displaystyle \begin{array}{l}{\displaystyle K_p=\frac{\cosh \left(k(d+z)\right)}{\cosh \left(kd\right)}}\end{array}}$$

Where k is the wave number, d is the water depth and z is the logger level from the surface. The wave energy density spectrum was then corrected for depth by dividing it by the pressure response factor squared. The output wave energy density spectrum was divided into sea (1–10 s period) and swell (10–20 s period) components for separate analysis (USACE, 1984). Significant wave heights, H_s, were determined from the wave spectrum [eqn (3); Moeller et al., 1996].

$${\displaystyle \begin{array}{l}{\displaystyle H_s=4\sqrt{E_{total}/\left({\rho }_{w}g\right)}}\end{array}}$$

where E_total is the total energy defined as the integral of the wave energy density spectrum. The wave period corresponding to the significant wave height, T_1/3, was approximated as 1.2 T_m0.1, where T_m0.1 is the zero-crossing period [eqn (4); Goda, 2010].

$${\displaystyle \begin{array}{l}{\displaystyle T_{m01}=\sqrt{m_0/m_2}}\end{array}}$$ (4)

where m₀ and m₂ are the zeroth and second moments of the wave energy density spectrum, respectively. Linear wave theory was used to calculate deep water wave characteristics based on the offshore RBR. With the assumption that wave period did not change as the wave approached the shore, deep water wavelength, celerity and group velocity were calculated from the wave period. Wave celerity at the other RBRs within each treatment at a site was then estimated based on Hunt (1979). This was used to calculate the shoaling coefficient [eqn (5); Haynes, 2018].

$${\displaystyle \begin{array}{l}{\displaystyle K_{\mathrm{s}}=\sqrt{C_{g0}/C_{gn}}}\end{array}}$$

where C_g0 is the deep water wave group celerity, and C_gn is the wave group celerity at the midshore or onshore RBR. Predicted wave heights were generated to account for shoaling [eqn (6)].

$${\displaystyle \begin{array}{l}{\displaystyle H_{\mathrm{s}\mathrm{\_}\mathrm{p}\mathrm{r}\mathrm{e}\mathrm{d}}=H_{\mathrm{i}}{K}_{\mathrm{s}}}\end{array}}$$ (6)

where H_{s_pred} is the predicted wave height and H_i is the incident wave height. A wave transmission coefficient, K_t, was defined as the ratio of measured to predicted wave height, accounting for phenomena other than shoaling affecting wave height [eqn (7); Haynes, 2018].

$${\displaystyle \begin{array}{l}{\displaystyle K_{\mathrm{t}}=H_{\mathrm{s}}/H_{\mathrm{s}\mathrm{\_}\mathrm{p}\mathrm{r}\mathrm{e}\mathrm{d}}}\end{array}}$$ (7)

where H_s is the recorded wave height at the midshore or onshore RBR. All processing was done in MATLAB (MathWorks, 1996) and resulted in hourly data for water depth, significant wave height, wave period and the wave transmission coefficient. For the Mornington and Williamstown reefs, the wave-exposed and sheltered side of each reef was relatively simple to identify, as the reefs were parallel to shore. Governor Reef, however, was at an angle to the shore, with a less obvious sheltered and exposed side. For this reason, the wave transmission coefficients for the kelp treatment and control at each reef were also calculated for the upwind (exposed) and downwind (sheltered) sides determined for each record based on wind direction and reef orientation (Wiberg et al., 2018).

All data were filtered according to offshore significant wave height, and only those records >0.05 m were used. This is similar to the minimum wave heights used for other studies (e.g. 0.03 m in Taube, 2010). This filtering removed the swell components of the waves (i.e. all waves classed as swell were <0.05 m), thus the analyses presented are on sea (wind) waves only. Wave attenuation from the offshore to midshore, offshore to onshore, upwind to midshore and upwind to downwind RBRs were compared between controls and kelp beds using a two-way analysis of variance (ANOVA; ‘car’ package in R; Fox and Weisberg, 2018; type III sum of squares), with K_t as the response variable and treatment and site as fixed factors. A post-hoc test (Tukey HSD) was used to determine differences between groups when there was a significant effect. In addition, wave spectra were calculated (as per Moeller et al., 1996) and examined for differences across the treatments. The relative importance of significant wave height, wave period, water depth, longshore and cross-shore wind vectors on the magnitude of difference in K_t between the kelp and control treatments at each site for the off- (upwind for Governor Reef) to onshore (downwind for Governor Reef) data was tested using partial leverage plots in JMP^®. Vegetation characteristics (density and lamina length) were used qualitatively to help explain any differences in wave attenuation between the sites; however, formal analyses were not done as there was only one kelp bed, and thus no variability in these parameters, at each site.

RESULTS

Site conditions

The density of the kelp beds ranged from 6 to 14 individuals per m², with an average lamina length of 17.98–26.72 cm (Table 1). Control areas at each site had either no (Governor Reef and Mornington) or low (Williamstown reefs) densities of kelp (Table 1). The largest fetch is from the east, south and west for Governor Reef (approx. 32 km), Williamstown reefs (approx. 42 km) and Mornington reef (approx. 32 km), respectively (Fig. 3). For Williamstown and Mornington, the southerly and westerly winds, respectively, are also frequent and have a high wind speed (Fig. 3; Supplementary data Fig. S1). At Governor Reef, easterly winds were the least frequent over the last 5 years. This site would, however, also be affected by winds from the north and south, although it is protected from the prevailing westerly winds (Fig. 3; Supplementary data Fig. S1). In general, the wind observations during the RBR deployment period were representative of the wind conditions at each site over the last 5 years, with the exception of more frequent than average southerly winds at Governor Reef and Williamstown reefs. There was a variety of wind directions and speeds recorded over the study period (Supplementary data Fig. S1). The average water depth at each site is listed in Table 1.

Table 1.

Summary of measurements at the sampling sites and treatments

	Governor		Williamstown 1		Williamstown 2		Mornington
	Kelp	Control	Kelp	Control	Kelp	Control	Kelp	Control
Significant wave height (m; mean/max)	0.31/0.88	0.33/0.78	0.39/1.03	0.36/0.91	0.77/3.81	0.88/5.63	0.69/2.51	0.79/2.84
Average water depth (m; across three RBRs)	3.05	2.47	3.00	2.42	5.32	5.20	3.50	3.72
Average wave period (s)	3.1	3.1	3.5	3.5	3.0	2.9	3.5	3.4
Fraction of time offshore side of reef is upwind	0.30		0.54		0.70		0.71
Fraction of time sig. wave height >0.05 m	0.57		0.68		0.70		0.57
Average density of E. radiata (ind m–2)	6	0	10	1	9	1	14	0
Average surface area of E. radiata (m2)	1.80	0	2.63	0.15	2.13	0.18	2.84	0
Average lamina length of E. radiata (cm)	26.72	NA	22.25	14.43	20.90	16.83	17.98	NA
Average Kt (range)	0.92 (0.69–1.36)	0.87 (0.67–1.37)	0.98 (0.77–1.06)	0.75 (0.55–1.19)	0.81 (0.32–1.01)	0.65 (0.19–1.26)	1.07 (0.94–1.25)	0.83 (0.52–1.27)

Wave attenuation of E. radiata

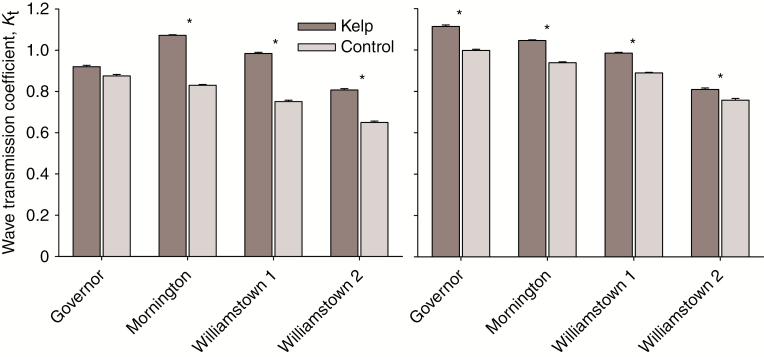
Average significant wave heights of 0.31–0.88 m were recorded at the sites during the study period, with maximum heights of 0.78–5.63 m (Table 1; Supplementary data Fig. S2). There was a significant difference in wave attenuation from the off- to onshore RBRs between the kelp and control treatments for Mornington and both Williamstown sites (Fig. 4A; Supplementary data Table S1). Contrary to expectations, wave attenuation was greater for the control compared with the kelp treatment (Fig. 4A).

Fig. 4.

Mean ± s.e. wave transmission coefficient (K_t) for kelp beds (dark grey) in comparison with a barren control (light grey) between (A) an offshore and onshore RBR (upwind and downwind for Governor Reef); and (B) an offshore and midshore RBR (upwind and midshore for Governor Reef) at four sites in Port Phillip Bay, Australia. Values below 1 indicate a reduction in wave height, whereas values above 1 indicate an increase in wave height.

As expected, this pattern did not change for Mornington and the Williamstown sites when the data were analysed based on wind direction (Supplementary data Table S1), as the reefs at these sites are parallel to shore. For Governor Reef, however, the reef is at an angle to shore and although the southern side was angled closer to the shoreline, winds from this direction could still generate waves that approached the reef from its southern side. When comparing off- with onshore for Governor Reef, wave attenuation was significantly greater where the kelp bed was present (Supplementary data Table S1). In contrast, there was no significant difference between the treatments at Governor Reef comparing wave attenuation from upwind to downwind (Fig. 4A; Supplementary data Table S1).

Wave attenuation between the RBRs placed offshore (for Mornington and Williamstown reefs) or upwind (Governor Reef) and midshore was significantly different between treatments for all sites (Fig. 4B; Supplementary data Table S1). Mornington and the Williamstown sites showed the same pattern as the off- to onshore RBRs, with lower wave transmission at the control compared with kelp treatments. This same pattern was observed at Governor Reef; however, this was in contrast to no treatment effect when the entire kelp bed was considered (i.e. up- to downwind RBRs) for this site (Fig. 4B). Wave spectra for a randomly chosen sub-set of time points for each site support these results (Supplementary data Fig. S3).

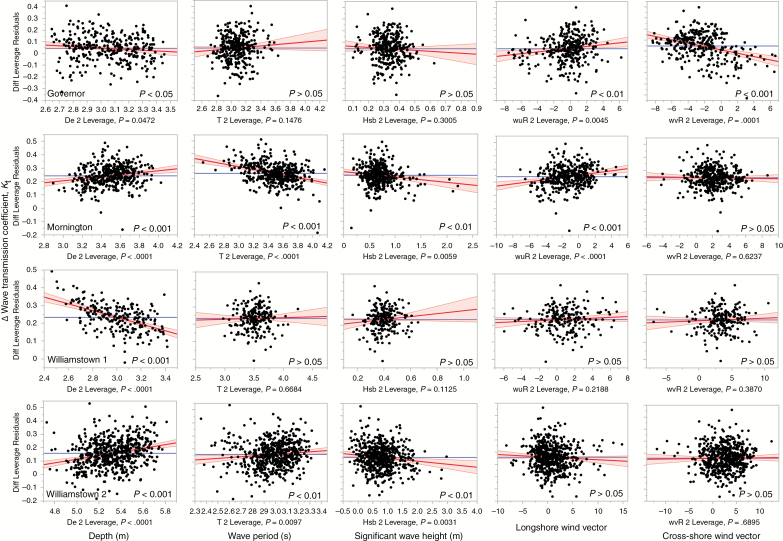
At the Mornington and Williamstown sites (Fig. 5), a lower wave transmission at control compared with kelp treatments was consistent across all environmental conditions (i.e. the difference between control and kelp treatments was positive for most data points; Fig. 5). However, at Governor Reef, wave attenuation at the kelp bed became greater than the control during northerly winds (i.e. when the cross-shore wind vector was positive, which indicated an onshore wind direction) (Fig. 5). Depth, wave period (excluding Williamstown 1), wave height (excluding Williamstown 1) and the longshore wind vector (Mornington only) had a significant effect on the magnitude of difference between control and kelp at Mornington and the Williamstown sites; however, the direction of the effect was inconsistent among sites (Fig. 5).

Fig. 5.

Difference in wave transmission (ΔK_t) between kelp and control treatments as a function of depth, wave period, significant wave height, longshore and cross-shore wind vectors at four sites: Governor Reef; Mornington; and Williamstown 1 and Williamstown 2. Negative values for ΔK_t indicates greater wave damping at kelp beds compared with control, whereas positive values indicate smaller K_t values at the control than at the kelp bed. The wind vector values were standardized to the reef position. Positive values for the longshore wind vector indicate a wind direction blowing to the right when looking at the beach from offshore. Positive values for the cross-shore wind vector indicate onshore winds.

DISCUSSION

What is the effect of E. radiata on wave attenuation?

There was significantly lower wave transmission at urchin barrens compared with kelp beds. Thus, contrary to predictions, on average we did not find evidence that the presence of kelp beds contributed to shoreline protection. This result was consistent under the different environmental conditions measured during the study for the Mornington and Williamstown sites. For Governor Reef, there was a trend for lower wave transmission at kelp beds than the control during northerly wind conditions. Wave attenuation at the kelp bed was 10 % greater than the control during northerly winds, compared with 8 % less than the control during southerly winds.

A negligible effect of E. radiata on wave attenuation conforms with previous research on Macrocystis pyrifera in California (Elwany et al., 1995; Rosman et al., 2007). This supports arguments that the flexibility of kelp allows individuals to move passively with the wave cycles, which minimizes drag, and thus the efficacy of kelp at attenuating waves (Denny and Cowen, 1997; Gaylord et al., 2003). However, this is in contrast to a laboratory study on L. hyperborea that, based on theoretical and experimental results, estimated 50 % wave attenuation over a 76 m kelp bed in 4 m water depth (Dubi and Tørum, 1996). This wave attenuation became negligable in 10 m water depth, at which point L. hyperborea occupied only 20 % of the water column (i.e. individuals were 2 m tall; Dubi and Tørum, 1996). This relationship between vegetation height and water depth has been commonly identified in previous studies (e.g. Dubi and Tørum, 1996; Allen and Webb, 2011; Maza et al., 2015). The effect of vegetation on wave attenuation has been shown to be greatest at shallower water depths relative to the height of the vegetation, when the vegetation occupies a greater proportion of the water column. Here, on average, E. radiata individuals occupied <10 % of the water column (Table 1), which could be one reason why wave attenuation was not observed in this study. The average lamina length of E. radiata measured in Port Phillip Bay was lower than that recorded in other areas of its Australasian range (22 cm in comparison with 49–89 cm in Wernberg et al., 2003). Thus, similar or more detailed measurements over a wider range of reefs across the range of E. radiata morphologies and environments would be useful in testing the generality of our results. One aspect not accounted for in wave flume studies of kelp, however, is the potential interaction between the effect of the reef sub-stratum the kelp inhabits and the kelp itself.

Unlike other coastal vegetation (e.g. seagrass, mangroves or saltmarsh), kelp colonizes rocky reef. This rocky substratum is often rugose and creates a shallowing of water, which alone can be expected to provide wave attenuation. For instance, the reef substratum that has been used to create oyster and coral reefs can provide wave attenuation and protection against erosion (Arnouil, 2008; Allen and Webb, 2011). The interest, however, is in what happens to the wave attenuation of the reef structure when it becomes more and more colonized by complex, habitat-forming organisms (Morris et al., 2019). Here, we controlled for the effect of the reef by comparing the kelp bed with a nearby denuded reef (urchin barren). Thus, any conclusions are based on the assumption that the reef bathymetry along the kelp transect is similar to the control transect, such that any differences in wave transmission can be attributed to kelp and not bathymetry-induced differences (e.g. Elwany et al., 1995). We were able to qualitatively assess this assumption using bathymetric data for the bay (Allemand et al., 2017), which showed similar rugosity between treatments at each site at a 5 m scale (Supplementary data Fig. S4). Despite this, it is a pervasive challenge that controls act as imperfect baselines in research such as this. The greater wave attenuation observed at control sites could have been due to smaller scale topographical differences in the reef area between control and kelp treatments. Further, two identical values of average rugosity at any scale could interact quite differently with an incident wave field through processes such as shoaling, reflection, diffraction and refraction (Reeve et al., 2012) as the topography is unlikely to match exactly. With adequate data, numerical models can be used to separate the effect of topography and kelp (Elwany et al., 1995). Nevertheless, our research supports the importance of having controls, in particular when evaluating reef-colonizing organisms. In previous field research where it was concluded that there was a significant damping effect of kelp without the use of controls, it is possible that this was due to the effect of the reef and not the kelp (Mork, 1996).

While three of the four sites had consistently no effect of kelp, at Governor Reef during northerly wind conditions the wave transmission at the kelp bed was lower than at the control. The primary effect of wind is its influence on wave conditions (Wiberg et al., 2018). Previous research has shown that flexible vegetation may have a greater effect on wave attenuation under smaller wave heights, as increasing wave height can cause the drag of flexible vegetation to reduce due to the higher velocities and bending forces associated with larger waves (Bradley and Houser, 2009). During the study period, winds from the northerly direction were less frequent, and also of a lower wind speed than southerly winds (Supplementary data Fig. S1). Despite this, average wave height was similar during the two different wind directions (0.34 m and 0.33 m for northerly and southerly winds, respectively) and, in contrast to previous studies, there was no effect of wave height on the magnitude of difference between kelp and control at Governor Reef (Fig. 5). Other explanations for a lower wave transmission at the kelp bed during northerly winds (e.g. wave–current interactions) need further investigation. However, despite the mechanism being unclear, if the presence of a kelp bed consistently results in a decrease in wave height during northerly wind conditions, this could be beneficial for coastal protection at the shoreline to the south of the reef. Governor Reef presented a different type of kelp bed from the other sites in the study, as it was an offshore patch reef as opposed to a fringing reef parallel to the shore. Previous studies have shown that oyster reefs of this type attenuate waves differently from fringing reefs (Wiberg et al., 2018). Patch reefs perpendicular, rather than parallel, to the shore are less likely to be used for shoreline protection. It is useful, however, to quantify the influence of orientation and distance from shore on wave attenuation to inform the design of reefs for nature-based coastal defence. This could be addressed more specifically in future studies.

How can the information be applied to the management of coastal ecosystems and hazards?

In Port Phillip Bay, there has been a major decline in kelp beds due to overgrazing by sea urchins (Carnell and Keough, 2019). A similar decline in kelp is also occurring in many other locations globally (Krumhansl et al., 2016). This has prompted restoration efforts to reduce urchin numbers (Tracey et al., 2015) and actively restore kelp beds (Carney et al., 2005; Campbell et al., 2014). Although kelp beds provide irreplaceable habitat for a large number of marine species (Dayton, 1985), there is increasing effort to quantify and economically evaluate (Barbier et al., 2011) other ecosystem services of socio-economic value to make a case for their restoration. Simultaneously, there is a growing interest in using natural or restored systems to provide a sustainable coastal defence solution (Temmerman et al., 2013). To support the integration of nature-based coastal defence into shoreline protection schemes, we need to know what habitats provide coastal defence, and under what conditions (Morris et al., 2018). Based on the sites and conditions tested in this study, we did not find strong evidence of wave attenuation by E. radiata. These results join a limited body of research globally that has reported variable or negligible effects of kelp on wave attenuation (e.g. Elwany et al., 1995; Rosman et al., 2007). This is in contrast to the effects of: (1) other coastal vegetation (i.e. saltmarsh, mangroves and seagrass); and (2) other reef-colonizing organisms (i.e. shellfish and corals), which are considered effective at providing coastal defence (e.g. Shepard et al., 2011; Ferrario et al., 2014). This study has highlighted the importance of disentangling the effects of the reef substratum and the colonizing organism on wave attenuation. A useful next step, therefore, would be to use numerical modelling to more effectively isolate the effect of kelp, although this would require more research to parameterize kelp drag. An alternative would be to bring the experiment into the lab and test the damping performance of E. radiata under various wave conditions using a more controlled environment in a wave flume, or field observations of wave transmission on reefs before and after the loss or recovery of kelp. This would provide a better understanding of why kelp increased wave transmission in this study. Other factors to consider, which we know very little about, would be potential indirect effects of kelp such as maintaining or stabilizing a rocky reef structure.

CONCLUSIONS

As climate change drives an increased risk of coastal hazards to a proliferating coastal population, there is a growing body of evidence to support the use of natural and nature-based infrastructure as sustainable and cost-effective coastal defence tools (e.g. Shepard et al., 2011; Ferrario et al., 2014; Narayan et al., 2016; Reguero et al., 2018). For kelp beds globally, however, there is a paucity of data to support their recommendation as a natural coastal protection infrastructure. Here, we concluded that kelp causing wave attenuation is not a universal truth. Kelp includes a diversity of species and morphologies, thus there is a need to understand what species are able to provide coastal protection, and under what conditions. This requires the collection of more field data for multiple species across different environments, which could be supported by numerical and physical modelling. Kelp is one of the dominant habitat-forming organisms of temperate reefs worldwide, making it a good candidate for nature-based coastal protection. Kelp beds are, however, also in decline in many parts of the world (Krumhansl et al., 2016), and a greater understanding of the suite of ecosystem services being lost underpins effective management of these ecosystems. Such future efforts will be key in delivering actionable information for managers and policy makers.

SUPPLEMENTARY DATA

Supplementary data are available online at https://academic.oup.com/aob and consist of the following. Table S1: analyses of variance and pairwise tests assessing the effects of treatment and site on wave attenuation. Figure S1: distribution of wind speed and direction (a) for the period January 2014–2019 and (b) for the period of RBR deployments. Figure S2: wave and wind time series for (a) Governor Reef; (b) Williamstown 1; (c) Williamstown 2; and (d) Mornington. Figure S3: wave spectra examples for (a) Governor Reef; (b) Williamstown 1; (c) Williamstown 2; and (d) Mornington where a kelp bed is present or absent. Figure S4: heat maps of rugosity at four sites in Port Phillip Bay.

FUNDING

This work was supported by the National Centre for Coasts and Climate, funded through The Earth Systems and Climate Change Hub by the Australian Government’s National Environmental Science Program.