Pathways for nicotinic receptor desensitization

Agonist actions at transition states can explain the observation that different pathways are taken for acetylcholine receptor desensitization and recovery.

Abstract

Nicotinic acetylcholine receptors (AChRs) are ligand-gated ion channels that generate transient currents by binding agonists and switching rapidly between closed- and open-channel conformations. Upon sustained exposure to ACh, the cell response diminishes slowly because of desensitization, a process that shuts the channel even with agonists still bound. In liganded receptors, the main desensitization pathway is from the open-channel conformation, but after agonists dissociate the main recovery pathway is to the closed-channel conformation. In this Viewpoint, I discuss two mechanisms that can explain the selection of different pathways, a question that has puzzled the community for 60 yr. The first is based on a discrete-state model (the “prism”), in which closed, open, and desensitized conformational states interconnect directly. This model predicts that 5% of unliganded AChRs are desensitized. Different pathways are taken with versus without agonists because ligands have different energy properties (φ values) at the transition states of the desensitization and recovery reactions. The second is a potential energy surface model (the “monkey saddle”), in which the states connect indirectly at a shared transition state region. Different pathways are taken because agonists shift the position of the gating transition state relative to the point where gating and desensitization conformational trajectories intersect. Understanding desensitization pathways appears to be a problem of kinetics rather than of thermodynamics. Other aspects of the two mechanisms are considered, as are experiments that may someday distinguish them.

Introduction

Nicotinic acetylcholine receptors (AChRs) are allosteric proteins that mediate cell signaling in the nervous and immune systems. Exposure of muscle cells to a high concentration of acetylcholine (ACh) produces a membrane current that rises rapidly and then declines slowly, a characteristic response that is determined by the receptors switching between three global conformations called closed, open, and desensitized (C, O, and D). C, the predominant shape of unliganded AChRs, binds agonists weakly and has a closed ion channel. O is transient, binds agonists strongly, and has an open channel. D is sluggish and also binds agonists strongly but has a closed channel. After agonists bind to C, the current rises rapidly because the increase in binding energy enables transitions to O, then declines slowly as receptors gradually change to D.

A longstanding question in the field has been with regard to the connections between D and C versus O. Experiments show that after binding agonists, AChRs desensitize mainly from the open-channel conformation, but that after agonists dissociate, desensitized receptors recover without generating any current (Katz and Thesleff, 1957; Sakmann et al., 1980; Cachelin and Colquhoun, 1989; Dilger and Liu, 1992; Franke et al., 1993; Auerbach and Akk, 1998). What determines that the primary desensitization pathway is O→D in liganded receptors but D→C in unliganded receptors?

Below, I consider two nonexclusive mechanisms that can account for this behavior, a discrete-state “prism” scheme (Fig. 1) and a “monkey-saddle” scheme that invokes a malleable potential energy surface (Fig. 2). In both, different pathways for desensitization (with agonists) and recovery (without agonists) are taken because of agonist effects at the transition states for conformational change.

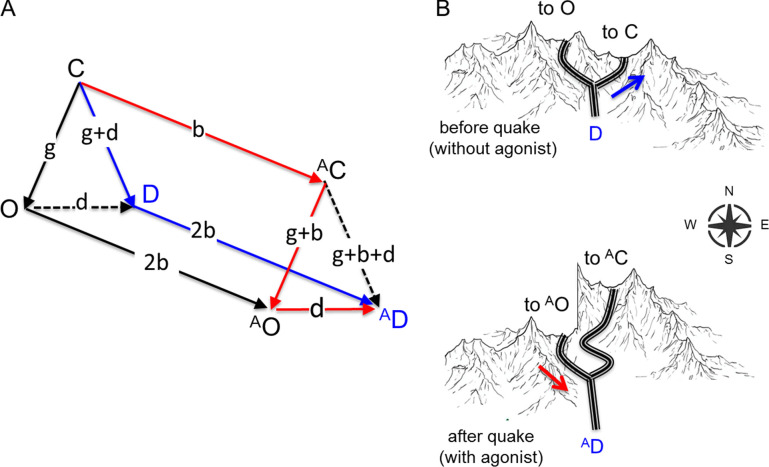
Figure 1.

Prism: pathway selection by φ. (A) Vertices, stable C, O, and D conformations; superscript A, agonist; lowercase letters, free energy changes (direction of arrow); dashed line, unused pathway. Independent energy changes are g, unliganded gating; b, agonist binding; d, desensitization. Binding energy is the same to D and O (twice that to C); agonists influence a reaction only by a binding energy change; other energies set by microscopic reversibility. In adult mammalian AChRs g = +8.3, d = −6.5 kcal/mol, so (g + d) = +1.8 kcal/mol (5% of unliganded receptors are D). Red, main path with a bound agonist: ^AD is connected to ^AO because here φ ∼ 1; blue, main recovery path without agonists; D is connected to C because here φ ∼ 0. (B) Landscape analogy. Top: Before the earthquake (the agonist), a hiker in the valley of D takes the easier, east trail to C (blue arrow). Bottom: An earthquake collapses only the west side of the range, to lower ^AD (and ^AO) valleys and west pass. The trail from ^AD to ^AO is the same as before the quake, but the unaltered east trail to ^AC is now disfavored. Not shown. The quake also levels the once-high pass between ^AC and ^AO (behind the central peak). After reaching ^AO, the hiker can cross readily back and forth along this “gating” trail, eventually to revisit ^AD by the easier west trail from ^AO (red arrow).

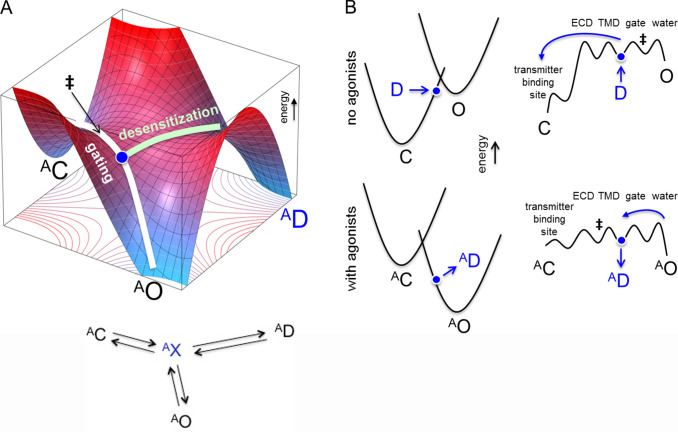
Figure 2.

Monkey saddle: pathway selection by a soft transition state. (A) Top: Three-well potential energy surface (PES). The conformational trajectories for gating (white) and desensitization (green) intersect at a short-lived intermediate state (blue dot). The bifurcation is on the ^AO-side of the gating committor (‡), so in experiments, ^AD appears to be connected to ^AO. Not depicted: Without agonists, ‡ moves closer to O to put the bifurcation on the C-side, so D appears to be connected to C. Bottom: Discrete-state model of the monkey-saddle landscape (^AX, the intermediate configuration at the bifurcation). Top modified from https://en.wikipedia.org/wiki/Monkey_saddle. (B) Cross sections through the gating PES. Left: C and O energy wells as parabolas (the D well is out of the plane). Top: Without agonists, the bifurcation to D is on the wall of the C parabola. Bottom: Agonists lower the relative free energy of O and move the bifurcation onto the wall of the O parabola. Right: Gating transition state region as a rugged landscape. Short-lived intermediates (wells) reflect fractional rearrangements of the global gating isomerization (ECD, extracellular domain; TMD, transmembrane domain). The position of ‡ relative to the bifurcation is malleable and depends on the tilt of the “roof line” that connects the barrier tops (Zhou et al., 2005). Without agonists, D appears to connect to C (top), but with agonists, ^AD appears to connect to ^AO (bottom).

Definitions

Gating refers to the global, closed-open isomerization of the receptor (C↔O) that switches low↔high both the neurotransmitter binding-site affinity and the channel conductance. Desensitization refers to two conformational changes. In C↔D, the affinity switches low↔high but the conductance is unchanged. In O↔D, the conductance switches high↔low but the affinity stays approximately the same.

Agonists are tiny compared with the receptor (∼150 D versus ∼300 kD). A small molecule at a neurotransmitter binding site does not alter the essential conformational status of the protein. However, an agonist can alter the rate and equilibrium constants of an intrinsic protein conformational change by providing newfound binding energy. Like a hornet on a racehorse, an agonist at a binding site increases the probability of a built-in behavior. To emphasize that an agonist is a structural perturbation that promotes preexisting conformational changes but does not create new ones, I denote it as a superscript A.

Here, “state” has two meanings. It can refer to a stable, global protein conformation such as C, O, or D. In this use, ^AC and C represent the same conformational state. In a discrete-state model, the capital letters are states and whatever happens to link these are inside the arrows. In this use, ^AC and C represent different model states.

The time constant of the sag (decline) in a macroscopic current depends on both (a) the microscopic desensitization entry and recovery rate constants and (b) the time-dependent probability of being in and leaving a conformational state that connects to D. The following two examples distinguish macroscopic and microscopic “desensitization.”

Desensitization of liganded receptors mainly proceeds from O, so the time constant and plateau of the sag depend on binding and gating equilibrium constants that influence the probability of being in the O conformation (P_O). First, consider perturbation that decreases P_O by slowing the agonist association rate constant (for instance, a binding site mutation or a low-efficacy agonist). Consequently, the sag will be prolonged even though the microscopic desensitization rate constants (O↔D and C↔D) are unchanged. Second, consider a mutation (for instance, at the pore gate) that increases the depth of the O energy well (and the lifetime of the O conformation) without altering the heights of either flanking energy barrier. This would happen if the mutation has a low ϕ value in C↔O and a high ϕ value in O↔D (the definition of ϕ is given below). Consequently, the sag will be prolonged without altering microscopic desensitization.

Below, I make two simplifications. First, skeletal-muscle AChRs have two neurotransmitter binding sites that in adults are independent and nearly identical (Akk and Auerbach, 1996; Nayak and Auerbach, 2017). I consider schemes for receptors with only one functional binding site. These can be readily extended to apply to two-site receptors, but at the expense of adding unnecessary complexity. Second, these AChRs have multiple D conformational states with lifetimes ranging from milliseconds to minutes (Paradiso and Brehm, 1998; Elenes and Auerbach, 2002). I consider pathways with regard to only the most stable, longest-lived D state. The connections between the various D states are not known, so extending this simple version to include all is not straightforward.

Prism

In the prism scheme, C, O, and D conformational states interconnect directly (Franke et al., 1993; Elenes et al., 2006). Agonists bind to (and dissociate from) all three conformations, so the full reaction scheme has the shape of a prism, with the two triangular endcaps representing model states without and with a bound ligand (Fig. 1 A). Certainly, there are short-lived intermediate configurations within each of the transitions in the prism (within each connecting arrow), but these are not relevant at the moment because I first consider free energy differences between the stable structures, and these are not influenced by sojourns in intermediates.

The free energy change in a chemical reaction (ΔG, product minus reactant) is related to the equilibrium constant (K_eq) by ΔG = −RTlnK_eq, where T is the absolute temperature and R is the gas constant (∼2 × 10⁻³ kcal · °K⁻¹ · mol⁻¹). For instance, in adult-type mouse AChRs at 23°C (RT = 0.59), the equilibrium constant for the gating conformational change with two bound ACh molecules is ∼25, which means that diliganded O is more stable than diliganded C by −1.9 kcal/mol. In contrast, the gating equilibrium constant of adult-type AChRs without any bound agonists is ∼7.4 × 10⁻⁷ (Nayak et al., 2012), which means that unliganded O is less stable than unliganded C by +8.3 kcal/mol. The whopping −10.2 kcal/mol increase in stability of the system with versus without ACh is generated by a binding energy increase for two bound neurotransmitter molecules that takes place within the channel-opening transition. At each mouse adult AChR neurotransmitter binding site, the equilibrium dissociation constant for ACh is ∼5,700-fold smaller in O versus C, which means ACh binds more strongly to O by approximately −5.1 kcal/mol. Hence, when a diliganded receptor begins (spontaneously!) to switch from C to O, approximately −10.2 kcal/mol of favorable ACh binding energy is generated to increase the opening rate constant (see below), P_O, and the cell response.

Equilibrium constants (free energy differences) for all of the transitions in the front plane of the prism (the binding-gating cycle) have been measured independently at individual human AChR binding sites for several different agonists (Nayak and Auerbach, 2017). These experiments show that the sum of the energy changes around this cycle is 0. Hence, microscopic reversibility is satisfied, and the system is in thermal equilibrium. All transitions, with and without a bound agonist, happen spontaneously and are motivated only by temperature.

Allosteric theory dictates that agonists increase P_O because they bind more strongly to O versus C, or that the equilibrium dissociation constant (K_d) must be smaller in O than in C. However, it was surprising to learn from experiments that that in the AChRs and for many small agonists, the decrease in K_d follows a rule: K_dO is approximately equal to K_dC² (Jadey and Auerbach, 2012). That is, binding free energy approximately doubles upon receptor activation (^AC→^AO), regardless of the agonist’s affinity or efficacy. For example, the binding energy ratio (logK_dO/logK_dC) is ∼2 for both the full agonist ACh (log 30 nM/log 170 µM) and the partial agonist choline (log 27 µM/log 6.8 mM; Nayak and Auerbach, 2017). As described elsewhere (Nayak et al., 2019), the binding energy ratio is related to the efficiency with which chemical energy from the affinity increase is converted into mechanical energy for the gating conformational change, with a value of 2 indicating an efficiency of 50%. The binding energy ratio for larger agonists related to the frog toxin epibatidine is ∼1.7 (K_dO is ∼K_dC^1.7), indicating that these ligands have a lower efficiency of ∼40%.

In Fig. 1 A, the lowercase letters associated with each transition are shorthand for the free energy differences between model states in the direction of the arrow, for unliganded gating (g), low-affinity binding to C (b), and desensitization (d). Note that Fig. 1 A pertains to ACh-like agonists because the energy of high-affinity binding to O is assigned the value 2b.

There are three features of the scheme to consider further. First, agonist binding energy (proportional to log affinity) is assumed to be approximately the same to D and to O. This has not been proven definitively, but there is some experimental support. Binding assays of mammalian AChRs estimate that K_dD is 24 nM for ACh and 200 nM for carbamylcholine (Weiland and Taylor, 1979; Sine and Taylor, 1982), whereas electrophysiology measurements of mammalian AChRs estimate that K_dO at adult binding sites is 16–34 nM for ACh and 192 nM for carbamylcholine (Grosman and Auerbach, 2001; Nayak and Auerbach, 2017). It appears that agonist binding energies to D and O sites are similar, if not identical. In the prism scheme, the neurotransmitter binding sites are binary with regard to binding energy, being either weak (b, to C) or strong (2b, to D and O).

Second, there are only three independent energy changes: g, b, and d. The others are constrained either by microscopic reversibility (the sum of energy changes around each cycle is 0) or the fixed binding-energy ratio. For example, in unliganded receptors, the C↔O energy change is g and the O↔D energy change is d, so microscopic reversibility demands that the C↔D energy change is (g + d). Likewise, in the front plane of the scheme, the unliganded gating energy change is g and the energy change in binding to C is b, so because binding energy doubles, the net ^AC↔^AO energy change must be (g + 2b − b).

A third feature of the prism scheme is that agonists influence the equilibrium constant of a conformational change (gating or desensitization) only by virtue of a change in binding energy. This is a corollary of the premise that agonists only perturb built-in conformational changes and do not induce new ones. For example, because O and D conformations have the same affinity, the energy change upon desensitization with a bound agonist (^AO↔^AD) is the same as without (O↔D), namely d. Likewise, the energy change in liganded ^AC↔^AD differs from that of unliganded C↔D only by the binding-energy change, b, that is the same as in ^AC↔^AO. The idea that agonists only add binding energy to an intrinsic energy profile is supported by a large number of experiments showing that the energetic consequences to binding and gating from the agonist and from side chain substitutions (away from a neurotransmitter binding site) are independent (Jadey et al., 2011; Gupta et al., 2017). That agonists do not affect substantially intrinsic desensitization is supported by the observation that desensitization and recovery from desensitization appear to be similar (but perhaps not identical) in receptors activated by agonists versus by mutations that increase the constitutive P_O (Purohit and Auerbach, 2009).

These features of the prism model allow the actions of unliganded receptors to be calculated from the three independent energy changes that have been measured experimentally in adult-type mouse AChRs: unliganded gating (g = +8.3 kcal/mol; Nayak et al., 2012), agonist binding to C (b = −5.1 kcal/mol for ACh; Jadey et al., 2011), and desensitization from ^AO (d = −6.5 kcal/mol; Elenes and Auerbach, 2002). These correspond, respectively, to 7.4 × 10⁻⁷ for the unliganded gating equilibrium constant, 170 µM for K_dC, and because the C↔D energy change is g + d or +1.8 kcal/mol, 0.047 for the equilibrium constant for unliganded desensitization from C. The unliganded C, O, and D occupancy probabilities can be calculated from these equilibrium constants, (1 − K_eq⁻¹)⁻¹. Without any bound agonists, P_C is ∼0.95, P_D is ∼0.05, and P_O is ∼10⁻⁶. At resting mammalian neuromuscular synapses, on average, 95% of receptors are C, 5% are D, and approximately one in a million is O.

We can also estimate the rate constants for unliganded transitions from C. The O→C rate constant is 12,000 s⁻¹ (Grosman, 2003), so from the corresponding equilibrium constant, C→O is 0.009 s⁻¹. The D→C rate constant is ∼3.3 s⁻¹ (Elenes et al., 2006), so from the corresponding equilibrium constant, C→D is ∼0.14 s⁻¹. On average, an unliganded C adult-type AChR spontaneously opens once every ∼110 s (for ∼80 µs) or desensitizes once every ∼7 s (for ∼300 ms).

Prism: pathway selection by φ

Red lines in Fig. 1 A mark the main physiological conformational-change pathway in the presence of agonists: C↔^AC↔^AO↔^AD (the arrows are binding, gating, and desensitization). With a bound agonist, ^AC→^AD is effectively barred (dashed line; Auerbach and Akk, 1998). A reaction rate constant is a function of the height of the energy barrier that separates reactant from product, with larger barriers meaning slower rate constants. When a strong agonist is bound, ^AO is occupied with a much higher probability than ^AC. Hence, the proclivity for entering ^AD from ^AO rather than ^AC indicates that with a strong agonist at the binding site, the ^AC→^AD barrier is large compared with the ^AO→^AD barrier.

This difference in barrier heights can happen if agonists reduce the ^AC→^AO barrier but not the ^AC→^AD barrier. Although the neurotransmitter binding sites undergo the same low-to-high affinity change in these conformational transitions, the stabilizing effect of the agonist on the separating barrier could be much greater in gating versus desensitization. The other possibility, that the intrinsic C→D barrier is so large that it remains high even with full stabilization from the affinity change, is unlikely. Two bound ACh molecules reduce the gating barrier by ∼10.2 kcal/mol, so the intrinsic barrier would have to be extraordinarily high and support an unacceptably slow unliganded recovery rate (Elenes et al., 2006).

Blue lines in Fig. 1 A mark the recovery pathway when agonist application is terminated: ^AD↔D↔C. After the ligand dissociates, receptors almost always recover to C directly without passing through O. This indicates that with an empty binding site (no bound agonists) the D→C barrier is small compared with the one in D→O.

Agonists increase an equilibrium constant by providing newfound, favorable binding energy to the product state, for example to ^AO more than to ^AC. To understand how the C→D path might be preferred without, but not with, a bound agonist, let us consider how a ligand influences the free energy of a transition state (‡).

The extent to which an agonist reduces the free energy of ‡ depends on its structure (energy) in the binding site at that point in the reaction. If, at the gating ‡, the agonist is in its low-affinity (C-like) configuration, it will not add favorable binding energy, and the barrier will not be lowered. If, however, at ‡ the ligand resembles its high-affinity (O-like) configuration, favorable binding energy will be added, and the barrier will be reduced to the same extent as the energy of the final, ^AO conformation. At ‡, the ligand and its binding-site site can exist in a conformation that is intermediate between those of ^AC and ^AO. For any binding reaction, the degree to which an agonist lowers the separating barrier is given by the parameter φ that ranges from 0 (structure at ‡ is the same as the starting state) to 1 (structure at ‡ is the same as the ending state).

Gating φ values have been measured for agonists and side chain mutations in different regions of the AChR (Grosman et al., 2000; Purohit et al., 2013). Agonists and residues at the binding sites have φ ∼ 0.95, indicating that at the gating ‡ these elements have almost the same structure (energy) as in ^AO. Indeed, a high gating φ value is the reason that agonists increase both P_O and the opening rate constant. If agonists had a gating φ value of 0, they would increase P_O to the same extent, but only by making openings longer lived rather than by increasing their frequency. The high agonist gating φ value is the reason that vesicular neurotransmitter release results in a synaptic impulse rather than a slow, sustained depolarization.

Different agonist φ values in ^AC↔^AD versus ^AC↔^AO can explain the desensitization pathways, as follows. Agonists have about the same (high) affinity for the two product states (D and O) and so increase favorably ^AO and ^AD energies compared with ^AC by about the same amount. The observation that ^AC→^AD is barred indicates the presence of a high barrier that, unlike with gating, is not much affected by the ligand, which is to say has a low agonist φ value. The different effects of agonists on the ^AC→^AO versus ^AC→^AD energy barriers is explained by the ligand having a product, O-like structure at the gating ‡ (a high φ) versus a reactant, C-like structure at the desensitization ‡ (a low φ). Starting from ^AC, the pathway to ^AO is leveled by the high-φ agonist and therefore easier to cross than the unleveled, low-φ pathway to ^AD. This would account for the observation that liganded receptors usually desensitize from the O conformation rather than from the C conformation (Fig. 1 B, bottom).

Regarding recovery, consider a D receptor that has just lost its agonist (Fig. 1 B, top). It, too, is faced with alternative routes, to C versus to O. The energy barrier between C and D is high but the same as in the presence of (low-φ) agonists. However, the pathway to O is even higher. Unliganded recovery through O is rare because this ‡ is not stabilized by the agonist that binds with equal affinity to the two end states of this conformational change, ^AO and ^AD. Without agonists, the barrier between D and C is easier to cross than the one between D and O, so unliganded receptors recover from desensitization directly into C without passing through O. Thus, different pathways will be taken if the agonist φ ∼ 1 in gating (C↔O) gating and φ ∼ 0 in desensitization (C↔D).

φ has a physical meaning. The isomerization of a large protein is a complex process that contains many local, microscopic rearrangements. Rather than being a point of intersection between two parabolas, in such complex reactions, the separation between reactant and product (‡) is called the committor, which is the position in the energy landscape from which there is an equal probability of reaching rapidly either absorbing end state (Bolhuis et al., 2002). A perturbation (for instance, an agonist) can change an equilibrium constant by changing the forward rate constant, the backward rate constant, or both. φ represents the degree of change in the forward versus the backward rate constant and ranges between 1 (only the forward rate constant changes) to 0 (only the backward rate constant changes). φ has been interpreted to reflect the position in the overall isomerization relative to ‡ at which the perturbed structural element undergoes an energy change generated by a local rearrangement (Grosman et al., 2000; Auerbach, 2005; Zhou et al., 2005; Gupta et al., 2017). Accordingly, high φ values for agonists and transmitter binding site residues suggest that the rearrangement that generates extra favorable binding energy occurs relatively early in the ^AC→^AO transition. Likewise, a low φ value for agonists in the desensitization process suggests that this affinity-changing rearrangement occurs relatively late in the ^AC→^AD transition.

A spatial map of gating φ values for AChR mutations indicates that the ^AC→^AO conformational change occurs as a coarse-grained cascade of rearrangements that propagates through the protein in four stages (Auerbach, 2005; Purohit et al., 2013; Gupta et al., 2017). The channel-opening rearrangement starts at the agonist/neurotransmitter binding sites (φ = 0.95), proceeds to the extracellular domain (φ = 0.79), then to the intracellular domain (φ = 0.58), and then to the equatorial M2 gate (φ = 0.33). The final step in the opening process is wetting of the gate region to initiate ion conduction (φ = 0.06).

Desensitization, too, likely involves global conformational changes because it entails a change in the affinity at the binding sites, the conductance, and the stability of the gate. Moreover, structures show that the arrangement of the transmembrane-domain helices is different in D than in C conformational states (Morales-Perez et al., 2016; Rahman et al., 2020). Mutations of a proline in the αM1 transmembrane segment increase the ^AO→^AD rate constant (Purohit et al., 2015), indicating a high φ value and suggesting that this residue undergoes its rearrangement relatively early in this reaction.

If experiments were to show that this proline also as a high φ value in the other desensitization reaction, ^AC↔^AD, the low φ value for the agonist would raise the interesting possibility that the spatial sequence of energy change in this isomerization is in the opposite directions as in gating. However, at this time, there is no experimental evidence for this. Furthermore, a mutation in εM2 (also in the transmembrane domain) has been shown to slow unliganded recovery (Elenes et al., 2006), indicating a φ value <1 in unliganded C↔D.

Monkey saddle

Another mechanism that accounts for the selection of different desensitization pathways with versus without agonists is shown as a three-well, monkey-saddle energy landscape (potential energy surface), with the wells separated by a shared energy barrier (Fig. 2 A, top). Although in this mechanism the shared barrier pertains to conformations with and without agonists, Fig. 2 A depicts only one of the two endcaps (with agonists) of this emergent prism. A simple discrete-state version of this landscape (the liganded endcap) is shown in Fig. 2 A, bottom, and more complex discrete-state variants are presented elsewhere (Auerbach, 2005; Zhou et al., 2005; Gupta et al., 2017). As described below, the key element of the saddle mechanism is malleability in the positions of ‡ that is easier to visualize as a potential energy surface than as a discrete-state model.

In the monkey saddle scheme, the global gating and desensitization conformational change pathways intersect at a shared barrier region. Fig. 2 A shows only the liganded conformational states, with the three energy wells (stable conformational states) representing ^AC, ^AO, and ^AD. Not shown is the unliganded version of the landscape (the unliganded endcap) that is connected to the companion endcap by agonist binding to each conformational state. However, unlike the discrete-state prism, in the saddle, the stable conformational states are not linked to each other directly but rather via a brief intermediate configuration in the barrier. This short-lived species forms and disappears transiently in gating (on the submicrosecond time scale) and comprises a point of bifurcation, or fork, between gating (white line) and desensitization (green line) conformational trajectories.

Some features of desensitization make this mechanism attractive a priori. For instance, desensitization happens on a much slower timescale than gating (seconds versus milliseconds). A slow process can arise from a large energy barrier, but the saddle mechanism offers the possibility that instead the slowness of desensitization arises from a low probability of taking and rejoining the fork (small transmission coefficients), as shown previously by simulation (Auerbach, 2005).

Another appealing feature of the monkey saddle is that it immediately accounts for the hybrid nature of the D conformation phenotype, namely having a shut channel (like C) but a high affinity for agonists (like O). As mentioned above, in the opening process, the neurotransmitter binding sites change from low to high affinity early, and the gate region of the pore changes from shut to open late. Hence, all of the intermediate configurations, including at the bifurcation point, have a hybrid character, namely a C-like conductance and an O-like agonist binding energy. The monkey-saddle mechanism predicts a priori some features of desensitized states, namely high affinity, shut channel, and slow kinetics.

Fig. 2 B shows cross sections of the monkey saddle cut along the gating trajectory. In a simple version (left), the C and O conformational states are represented as parabolic energy wells with a point intersection at the gating ‡. The bifurcation to D (out of the plane) is shown as a blue circle located on a parabola wall, ∼60% of the way through the gating reaction. Although experiments do not clearly pinpoint a structural correlate of this fork, mutations of the abovementioned αM1 proline influence both gating and desensitization, so this residue is a candidate. The φ value of this proline is 0.6, suggesting that its gating rearrangement occurs ∼60% of the way through the C→O process.

The top panel in Fig. 2 B represents the landscape for an unliganded receptor. C to O is uphill energetically and, hence, the gating equilibrium constant is small (<<1). The bifurcation point is on the C parabola, so the pathway for recovery from desensitization without agonists will appear in experiments to be D→C. The bottom panel shows the landscape with agonists at the binding sites. Now, because of the favorable agonist binding energy to ^AO, the reaction is downhill, and hence the gating equilibrium constant is >1. By virtue of making O more stable, agonists shift the parabola intersection toward C. The bifurcation, still positioned 60% of the way through the reaction, it is now located on the wall of the ^AO parabola, so the pathway for entry into desensitization with agonists will appear in experiments to be ^AO→^AD. A shift in the position of ‡ caused by a change in the gating equilibrium constant has been shown to occur in AChRs (Grosman, 2003).

Somewhat more realistic representations of gating trajectory cross sections are shown in Fig. 2 B (right). Here, brief intermediate configurations along the gating path are represented explicitly as a series of shallow energy wells separated by small energy barriers. Each energy barrier reflects a local rearrangement, and each energy well represents an intermediate structural configuration, with the sequence and structural associations inferred from gating φ values (Gupta et al., 2017).

The committor is the position in a landscape from which the system will evolve rapidly to become either end state with equal probability. Hence, in a rugged energy landscape, the position that separates reactant and product, ‡, can be anywhere along the landscape rather than at the intersection of parabolas. In Fig. 2 B, the fork is again located at φ = 0.60, at an intermediate conformation in which the neurotransmitter binding sites, extracellular domain, and transmembrane helix arrangements have already switched to their O-like structure but the gate is still shut and dry, as in C. Accordingly, when in the channel-opening process the system reaches this point, the next microscopic transition can lead either to the opening and wetting of the equatorial gate to become ^AO or to further rearrangements of the transmembrane domain to (eventually) become ^AD.

The top and bottom panels of Fig. 2 B (right) depict the rugged landscape without (top) and with (bottom) agonists. In the absence of agonists, ‡ is located near the end of the opening process, close to O. Hence, when an unliganded receptor recovers from D to enter the rugged gating landscape at φ = 0.6, it will almost always first reach and be absorbed by C without visiting O.

Experiments show that the main effect of an agonist is to lower the barrier out of the first gating intermediate conformation that represents the extracellular domain rearrangement and occurs after the binding site has switched to the high-affinity conformation (Gupta et al., 2017). Consequently, with a bound agonist, ‡ is positioned closer to ^AC (Fig. 2 B, bottom), and the bifurcation is on the ^AO-side of the landscape. With a bound agonist, the receptor recovers from ^AD to enter the rugged gating landscape at φ = 0.6, hence almost always first reaching ^AO without visiting ^AC. In the monkey-saddle mechanism, a shift in the position of ‡ caused by newfound agonist binding energy explains the different desensitization pathways, namely that liganded receptors enter ^AD from ^AO but unliganded receptors recover from D to C.

Experiments

A full complement of atomic-resolution structures of C, O, and D conformations, with and without bound agonists, as well as those of short-lived intermediate configurations that connect the stable conformational states, might provide clues for the mechanism for different desensitization pathways. Finding that the agonist has a high-affinity structure at the gating transition state but a low-affinity structure at the desensitization transition state would support the prism mechanism. Someday, all of the salient structures will be determined, put into sequence, and assigned Boltzmann energies, but perhaps not anytime soon.

Until then, other experiments might shed some light on the alternate desensitization pathways. To calculate unliganded desensitization parameters, I relied on an old result indicating that K_dD and K_dO are similar. These experiments should be updated with new K_dD estimates for more agonists and at different binding sites. It is also possible to use mutated AChRs and single-channel electrophysiology to measure unliganded desensitization parameters directly (and, hence, estimate K_dD indirectly). Finally, kinetic analyses of single-channel currents might illuminate whether gate mutations slow the macroscopic current sag simply by making the O energy well deeper or whether they change the microscopic rates of desensitization.

To explain the pathway pattern using the prism scheme, agonists need to have a high φ value in ^AC↔^AO gating (confirmed by experiment) and a low φ value in ^AC↔^AD desensitization (untested). It is difficult, however, to make measurements of φ in ^AC↔^AD, as there is no change in conductance in this reaction. While it is certainly possible to estimate effects of mutations on the backward, D→C recovery rate constant (by paired-pulse experiments), effects on the forward, C→D rate constant (or equilibrium constant), too, must be measured to estimate a φ value. Corresponding measurements in ^AO↔^AD may be informative, but this is a different reaction and also difficult to probe, because most side chain substitutions do not influence the equilibrium constant substantially. Finally, the existence of multiple D states, with unknown interconnections, obfuscates the estimation of all desensitization rate and equilibrium constants.

Examination of weak, high-φ agonists may also provide a test of the prism hypothesis. Weak (low-efficacy) agonists have smaller ^AO versus ^AC energy difference compared with strong agonists and so yield similarly smaller reductions in the height of the gating transition state. Whereas with strong agonists, the rate of entry into ^AD is correlated linearly with P_O (Auerbach and Akk, 1998), perhaps with an extraordinarily weak agonist the gating and desensitization barriers would become nearly equivalent, so that ^AD could also be reached from ^AC. Accordingly, the net rate of entry into ^AD should not be a function of P_O but rather of the probability of being liganded.

It may also be possible to test the monkey-saddle mechanism experimentally. In the parabola description of the potential energy surface, agonists change the position of ‡ by lowering the energy of the O well. In a rugged landscape, a mutation changes the position of ‡ by changing the energy of a microscopic well (that represents an intermediate-state conformation). Hence, it may be possible to engineer the position of ‡ by combining mutations in different regions of the protein (different microscopic wells) that have known, independent energetic consequences (Gupta and Auerbach, 2011; Gupta et al., 2017). If receptors were constructed that pass through O during recovery, the presence (and position) of a bifurcation in the rugged gating landscape would be revealed. These experiments are challenging not only because it is difficult to use mutations to position ‡ without influencing the gating equilibrium constant, but also because it is necessary to identify recovery openings arising from D versus from C.

The key to solving the mechanism for different desensitization pathways is to consider agonist effects on transition states. Understanding desensitization appears as a kinetic rather than thermodynamic problem, with corresponding structures that pertain to unstable intermediate states rather than to stable conformations. The prism scheme is conventional and needs only one new experimental result to be confirmed, that agonists have a low φ in C↔D (a C-like structure at this ‡). The monkey-saddle scheme is more adventurous because it posits that gating and desensitization conformational trajectories intersect, but is elegant insofar as it predicts a priori properties of desensitized receptors (high affinity, shut channel, slow kinetics) and has components that are already established (agonists increase P_O, the gating transition state is malleable). Most of the experiments suggested above are not easy, but someday a combination of structural, functional, and computational investigations will reveal unambiguously the mechanism for pathway selection in nicotinic receptor desensitization.