Abstract
Background: Upper extremity length and circumference abnormalities are present in a number of conditions in the pediatric population. In most cases, upper limb hypoplasia and hypertrophy are diagnosed when one limb appears substantially different from the other during physical examination. However, occasionally when this discrepancy exists, it can be difficult to determine which limb is the abnormal one. The purpose of this study was to establish normal values for upper extremity length, circumference, and rate of growth in children aged 0 to 17 years. Methods: In all, 377 participants had 4 measurements taken of each upper extremity: upper arm length, upper arm circumference, forearm length, and forearm circumference. Statistical analysis was performed to identify differences and rates of growth. Results: Mean values for arm and forearm length and circumference for each age, 0 to 17 years, were established. The determination of a child’s expected arm length is dependent on his or her height, age, and sex, while the calculation of a child’s expected forearm length depends on his or her weight, age, and sex. Male and female arms and forearms have similar growth rates of lengths and circumferences. No significant differences were found between right and left extremities for each of the 4 measurements taken. Conclusions: Contralateral limbs can be used for comparison of length and circumference of the arm and forearm in cases of unilateral upper extremity abnormality. The establishment of normal values for upper extremity length, circumference, and growth rate will be a useful diagnostic tool for upper extremity hypoplasia and hypertrophy.
Keywords: upper extremity, normal ranges, pediatrics, circumference, growth rate
Introduction
Upper extremity length and girth discrepancies characterize a number of clinical disorders in the pediatric population. For example, patients with brachial plexus birth palsy may expect the affected arm and forearm to be approximately 90% to 95% the length and girth of the contralateral limb.1,2 Furthermore, upper limb asymmetries are used in the diagnosis of certain conditions such as Proteus syndrome3 and lymphedema.4,5 The use of a healthy, unaffected limb as a control for the evaluation of an affected limb is common both clinically and during research investigations.5,6 However, the validity of upper limb discrepancy studies and the associated diagnostic criteria depends on the critical assumption that arm and forearm lengths and girths are the same, bilaterally, in the pediatric population.
Several studies have previously attempted to establish normative values for arm and forearm length and girth. Hensinger provided mean arm and forearm lengths for children aged 2 to 18, whereas Fryar et al and Neu et al established normal values for arm and forearm circumference, respectively.7-9 However, each of these studies provides normative values that are based on data recorded unilaterally. Therefore, the validity of these established values again depends on the assumption that arm and forearm lengths and girths are the same, bilaterally, in the pediatric population, although to our knowledge no data exist to support this assumption.
The purpose of this study was to determine whether the length and circumference of children’s upper extremities are the same, bilaterally, at each age. A secondary goal of this study was to develop a novel method for the estimation of a child’s expected arm and forearm length and circumference on the basis of his or her age, height, weight, and sex.
Materials and Methods
Pediatric orthopedic patients or siblings of patients between the ages of 0 and 17 years were recruited for this study following institutional review board approval. Subjects were prospectively recruited prior to being evaluated in the clinic and were excluded if they had a history of an upper extremity injury or surgery, brachial plexus birth palsy, or neurodevelopmental disorder. Prior to taking measurements, demographic information including age, sex, hand dominance, height, and weight was recorded for each participant.
Four measurements were performed on each upper extremity using a standard tape measure: the distance from the tip of the acromion to the elbow flexion crease (arm length), the distance from the elbow flexion crease to the wrist flexion crease (forearm length), the arm circumference measured at 5 or 10 cm proximal to the elbow flexion crease depending on the size of the participant (aiming to measure around the thickest portion of the arm), and the forearm circumference measured at 5 or 10 cm distal to the elbow flexion crease depending on the size of the patient (aiming to measure around the thickest portion of the forearm). These 4 measurements were first taken on the right upper extremity and then repeated on the left, providing a total of 8 measurements recorded for each subject. All of the measurements were performed by the same evaluator (T.E.) to ensure consistency.
Statistical Analysis
Mean values for arm and forearm length and circumference, on both the right and left upper extremities, were determined for each age between 0 and 17 years. Multivariable linear regression analysis was performed to generate a predictive model for both right and left arm and forearm length and circumference values. Eight predictive equations were generated on the basis of height, weight, sex, and age. Covariates with a P value greater than .05 were removed from the final model.
To compare average values for arm and forearm length and circumference between the right and left upper extremities, 4 multivariate correlation analyses were performed for each of the measurements: arm length, arm circumference, forearm length, and forearm circumference. Data were deemed significant for P < .05.
Results
Measurements from 377 participants were included in the study. In this study, 56% of the participants were women and 44% were men; 80% of the participants were right-handed. While the sample comprised participants aged 0 to 17 years, the data were not distributed equally, with 10% of the participants being 12 years old and less than 1% of participants being 17 years old. (Table 1)
Table 1.
Participant Demographics.
| Demographics | No. of subjects tested, No. (%) |
|---|---|
| Age group (average age), y | |
| 0 (0.6) | 8 (2.1) |
| 1 (1.6) | 33 (8.8) |
| 2 (2.4) | 22 (5.8) |
| 3 (3.5) | 21 (5.6) |
| 4 (4.5) | 17 (4.5) |
| 5 (5.4) | 23 (6.1) |
| 6 (6.5) | 24 (6.4) |
| 7 (7.4) | 23 (6.1) |
| 8 (8.5) | 25 (6.6) |
| 9 (9.4) | 26 (6.9) |
| 10 (10.5) | 30 (8.0) |
| 11 (11.6) | 21 (5.6) |
| 12 (12.5) | 36 (9.5) |
| 13 (13.5) | 21 (5.6) |
| 14 (14.5) | 20 (5.3) |
| 15 (15.4) | 17 (4.5) |
| 16 (16.4) | 7 (1.9) |
| 17 (17.4) | 3 (0.8) |
| Total | 377 (100.0) |
| Sex | |
| Male | 165 (43.8) |
| Female | 212 (56.2) |
| Total | 377 (100.0) |
| Hand dominance | |
| Right | 302 (80.1) |
| Left | 38 (10.1) |
| Ambidextrous | 10 (2.7) |
| Undetermined | 27 (7.2) |
| Total | 377 (100.0) |
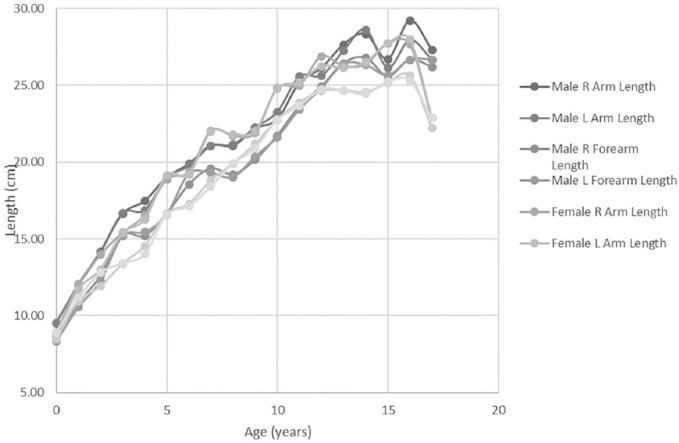
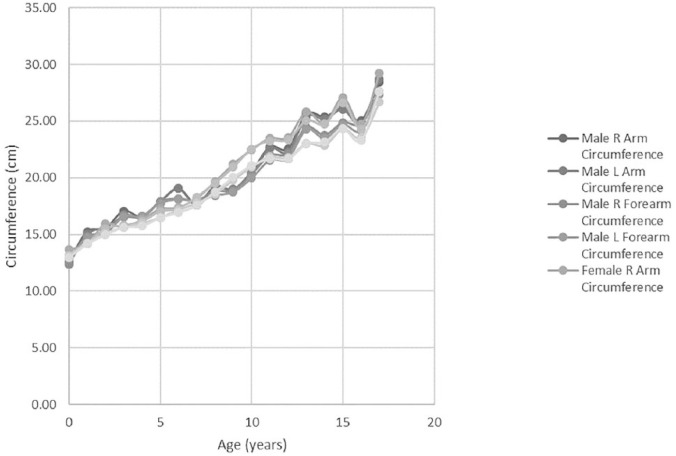
Average values for arm and forearm length (Figure 1) and circumference (Figure 2) are summarized by age in Table 2. All 4 multivariate correlation analyses generated pairwise comparisons with P < .0001, indicating a significant correlation between each of the 4 measurements taken from the right and left upper limbs (Table 3). Thus, there is no significant difference between the right and left upper extremities for arm length, arm circumference, forearm length, and forearm circumference.
Figure 1.
Relationship between arm and forearm length versus age.
Note. R = right; L = left.
Figure 2.
Relationship between arm and forearm circumference versus age.
Note. R = right; L = left.
Table 2.
Average Arm and Forearm Length and Circumference.
| Age, y | No. of subjects tested | Average R arm length (SD) | Average L arm length (SD) | Average R arm circumference (SD) | Average L arm circumference (SD) | Average R forearm length (SD) | Average L forearm length (SD) | Average R forearm circumference (SD) | Average L forearm circumference (SD) |
|---|---|---|---|---|---|---|---|---|---|
| 0 | 8 | 9.21 (0.57) | 9.13 (0.54) | 13.10 (1.10) | 12.78 (1.06) | 8.57 (0.71) | 8.61 (0.74) | 12.74 (0.72) | 12.90 (0.60) |
| 1 | 33 | 12.04 (0.46) | 11.85 (0.46) | 14.74 (0.73) | 14.61 (0.74) | 10.86 (0.37) | 10.85 (0.37) | 14.55 (0.47) | 14.44 (0.51) |
| 2 | 22 | 14.11 (0.72) | 13.80 (0.76) | 15.64 (0.66) | 15.59 (0.67) | 12.41 (0.56) | 12.41 (0.58) | 15.04 (0.58) | 15.05 (0.45) |
| 3 | 21 | 15.88 (1.09) | 15.86 (0.99) | 16.19 (1.22) | 16.07 (1.13) | 14.09 (1.31) | 14.11 (1.28) | 16.04 (1.06) | 15.99 (0.92) |
| 4 | 17 | 17.03 (0.70) | 16.58 (0.76) | 16.45 (0.63) | 16.38 (0.57) | 14.89 (0.47) | 14.77 (0.49) | 16.21 (0.40) | 16.21 (0.48) |
| 5 | 23 | 18.93 (0.88) | 19.09 (0.77) | 17.45 (0.83) | 17.28 (0.73) | 16.65 (0.64) | 16.59 (0.59) | 16.90 (0.62) | 16.87 (0.60) |
| 6 | 24 | 19.47 (0.82) | 19.31 (0.80) | 17.74 (1.03) | 17.63 (1.04) | 17.54 (0.66) | 17.58 (0.71) | 17.36 (0.70) | 17.18 (0.73) |
| 7 | 23 | 21.63 (0.85) | 21.58 (0.86) | 17.99 (0.84) | 17.99 (0.81) | 19.15 (0.50) | 18.80 (0.61) | 17.85 (0.59) | 17.72 (0.61) |
| 8 | 25 | 21.60 (0.86) | 21.50 (0.81) | 19.44 (0.98) | 19.33 (0.94) | 19.67 (0.53) | 19.53 (0.60) | 18.66 (0.64) | 18.57 (0.66) |
| 9 | 26 | 22.04 (0.78) | 22.14 (0.74) | 20.20 (1.34) | 20.03 (1.29) | 20.71 (0.57) | 20.67 (0.56) | 19.37 (0.82) | 19.44 (0.91) |
| 10 | 30 | 24.07 (0.94) | 24.23 (0.82) | 21.76 (1.41) | 21.74 (1.41) | 22.39 (0.69) | 22.34 (0.67) | 20.72 (0.92) | 20.67 (0.95) |
| 11 | 21 | 25.07 (0.89) | 25.37 (0.81) | 23.13 (1.29) | 22.95 (1.17) | 23.75 (0.81) | 23.56 (0.82) | 21.91 (1.04) | 21.62 (1.03) |
| 12 | 36 | 26.59 (0.94) | 26.01 (0.87) | 23.18 (1.31) | 22.92 (1.36) | 24.75 (0.95) | 24.77 (0.88) | 21.92 (0.99) | 21.68 (1.04) |
| 13 | 21 | 26.99 (0.84) | 26.72 (0.92) | 25.73 (1.53) | 25.23 (1.39) | 25.60 (0.58) | 25.57 (0.59) | 23.77 (1.07) | 23.66 (0.97) |
| 14 | 20 | 27.16 (0.89) | 27.35 (0.87) | 25.03 (1.67) | 24.91 (1.58) | 25.45 (0.82) | 25.19 (0.85) | 23.23 (0.99) | 23.29 (0.96) |
| 15 | 17 | 27.19 (1.33) | 26.89 (1.37) | 26.60 (2.50) | 26.30 (2.33) | 25.38 (1.36) | 25.46 (1.29) | 24.62 (1.58) | 24.52 (1.62) |
| 16 | 7 | 27.89 (0.67) | 27.99 (0.58) | 24.77 (0.70) | 24.36 (0.61) | 25.76 (0.64) | 25.49 (0.53) | 23.72 (0.54) | 23.40 (0.55) |
| 17 | 3 | 25.82 (1.04) | 25.40 (1.00) | 28.68 (0.83) | 28.36 (1.21) | 24.87 (1.00) | 25.40 (1.00) | 27.31 (1.15) | 27.41 (1.25) |
Note. Measurements reported in centimeters. R = right; L = left.
Table 3.
Correlation Between Left and Right Upper Extremity Measurements.
| Variable | By variable | Correlation | N | Lower 95% | Upper 95% | P value |
|---|---|---|---|---|---|---|
| L arm length, cm | R arm length, cm | 0.9815 | 377 | 0.9773 | 0.9848 | <.0001* |
| L arm circumference, cm | R arm circumference, cm | 0.9864 | 377 | 0.9833 | 0.9889 | <.0001* |
| L forearm length, cm | R forearm length, cm | 0.9842 | 377 | 0.9807 | 0.9871 | <.0001* |
| L forearm circumference, cm | R forearm circumference, cm | 0.9842 | 377 | 0.9807 | 0.9871 | <.0001* |
Note. R= right; L = left.
statistically significant.
Variables including age, height (cm), weight (kg), and sex were analyzed via multivariable linear regression analysis to generate equations to determine expected upper extremity length and circumference in healthy individuals. The parameters used to produce each equation are listed in Table 4, and a summary of the 8 equations with statistically significant parameters are listed in Table 5.
Table 4.
Factors Associated With Upper Extremity Length and Circumference for Predictive Equations.
| R arm length | L arm length | R arm circumference | L arm circumference | R forearm length | L forearm length | R forearm circumference | L forearm circumference | |
|---|---|---|---|---|---|---|---|---|
| Intercept (P) | 0.58 (.4118) | 0.49 (.4735) | 12.11 (<.0001*) | 12.10 (<.0001*) | 0.07 (.911) | 0.50 (.4018) | 11.86 (<.0001*) | 11.90 (<.0001*) |
| Height, cm (P) | 0.14 (<.0001*) | 0.14 (<.0001*) | — | — | 0.12 (<.0001*) | 0.12 (<.0001*) | 0.01 (.0882) | 0.01 (.1317) |
| Weight, kg (P) | — | — | 0.05 (<.0001*) | 0.05 (<.0001*) | 0.01 (<.0001*) | 0.01 (<.0001*) | 0.03 (<.0001*) | 0.03 (<.0001*) |
| Sex (F) (P) | 0.41 (.0286*) | 0.41 (.0252*) | 0.52 (.0030*) | 0.50 (.0041*) | 0.15 (.3329) | 0.14 (.3224) | 0.13 (.2623) | — |
| Age, y (P) | 0.28 (<.0001*) | 0.28 (<.0001*) | −0.07 (.0410*) | −0.04 (.2242) | 0.25 (<.0001*) | 0.27 (<.0001*) | 0.07 (.0902) | 0.05 (.1629) |
Note. R= right; L = left.
statistically significant.
Table 5.
Equations to Determine Upper Extremity Length and Circumference Based on Patient Height, Weight, Age, and Sex.
| Measurement | Equation | R 2 |
|---|---|---|
| R arm length | Y = 0.14 (height) + 0.28 (age) + 0.41 (sex) | 0.90 |
| L arm length | Y = 0.14 (height) + 0.28 (age) + 0.41 (sex) | 0.90 |
| R arm circumference | Y = 12.11 + 0.05 (weight) + 0.52 (sex) – 0.07 (age) | 0.88 |
| L arm circumference | Y = 12.10 + 0.05 (weight) + 0.50 (sex) | 0.88 |
| R forearm length | Y = 0.12 (height) + 0.01 (weight) + 0.25 (age) | 0.93 |
| L forearm length | Y = 0.12 (height) + 0.01 (weight) + 0.27 (age) | 0.94 |
| R forearm circumference | Y = 11.86 + 0.03 (weight) | 0.90 |
| L forearm circumference | Y = 11.90 + 0.03 (weight) | 0.90 |
Note. Weight is expressed in kilograms; height, in centimeters; age, in years. R = right; L = left.
Height (P < .0001), sex (P = .0286), and age (P < .0001) were used to calculate the expected right arm length. These 3 variables (height [P < .0001], sex [P = .0252], and age [P < .0001]) were also used to calculate the expected left arm length. The intercept (P < .0001), weight (P < .0001), sex (P = .0030), and age (P = .0410) were used to predict right arm circumference. The intercept (P < .0001), weight (P < .0001), and sex (P = .0041) were used to calculate the expected left arm circumference. Height (P < .0001), weight (P < .0001), and age (P < .0001) were used to calculate the expected right forearm length. These 3 variables (height [P < .0001], weight [P < .0001], and age [P < .0001]) were also used to calculate an individual’s expected left forearm length. Only the intercept (P < .0001) and weight (P < .0001) are significant in the calculation of the right forearm circumference. This holds true for the calculation of the expected left forearm circumference as well (intercept [P < .0001] and weight [P < .0001]), demonstrating that weight alone is an accurate indicator of forearm circumference.
Arm Length Predictive Equation
Based on the data collected, a child’s expected arm length was determined from his or her height, age, and sex: right arm length = 0.14 (height) + 0.28 (age) + 0.41 (sex), R2 = 0.90; left arm length = 0.14 (height) + 0.28 (age) + 0.41 (sex), R2 = 0.90. The equations for both the right and left arm are equivalent, and thus, arm length in centimeters can be determined by the following equation:
with height in centimeters, age in years, and a binary code for sex (male = 0, female = 1)
Arm Circumference Predictive Equation
The expected arm circumference of a child was determined based on his or her weight, sex, and age: right arm circumference = 12.11 + 0.05 (weight) + 0.52 (sex) – 0.07 (age), R2 = 0.88; left arm circumference = 12.10 + 0.05 (weight) + 0.50 (sex), R2 = 0.88. The slight difference between the equations for right and left arm circumference is likely not of clinical significance as no significant difference between the left and right arm circumferences was found. However, the equation for right arm circumference is a slightly better predictor (R2 = 0.884) than the equation for left arm circumference (R2 = 0.880), and therefore, the expected bilateral pediatric arm circumference in centimeters can be determined by the following equation:
with weight in kilograms, age in years, and a binary code for sex (male = 0, female = 1)
Forearm Length Predictive Equation
In contrast to arm length, forearm length was determined from a child’s height, weight, and age and is independent of his or her sex: right forearm length = 0.12 (height) + 0.01 (weight) + 0.25 (age), R2 = 0.93; left forearm length = 0.12 (height) + 0.01 (weight) + 0.27 (age), R2 = 0.94. Again, there is a slight difference between the equations for right and left forearm lengths. The present study found no difference between right and left forearm lengths; thus, this small difference does not appear to be of any clinical significance. The equation for the left forearm (R2 = 0.94) is a slightly better predictor of forearm length than the equation for the right forearm (R2 = 0.93). Thus, the expected bilateral pediatric forearm length in centimeters can be determined by the following equation:
with height in centimeters, weight in kilograms, and age in years.
Forearm Circumference
Based on the data collected, forearm circumference is independent of height, sex, and age and was determined solely by a child’s weight: right forearm circumference = 11.86 + 0.03 (weight), R2 = 0.90; left forearm circumference = 11.90 + 0.03 (weight), R2 = 0.90. The slight difference between the equations for right and left forearm circumferences is not clinically significant as, again, the present study found no difference between the circumferences of the right and left forearms. The equation for the left forearm (R2 = 0.900) was a slightly better predictor of forearm circumference than the equation for the right forearm (R2 = 0.899). Therefore, it was determined that a child’s expected bilateral forearm circumference in centimeters can be determined by the following equation:
with weight in kilograms.
Discussion
No significant differences exist between the right and left upper extremities in any of the measurements taken during this study (arm length, arm circumference, forearm length, forearm circumference). This suggests that arm and forearm lengths and circumferences are the same bilaterally in the normal pediatric population. Thus, observed differences in the length and girth of upper extremities in conditions such as brachial plexus birth palsy and lymphedema are, in fact, pathologic.1,2 These findings indicate that the use of upper limb asymmetries in the diagnosis of certain conditions also appears to be valid as both limbs should be of the same size in the healthy population.3-5
Previous studies that established normal values for pediatric arm and forearm length based on measurements from a single upper limb can be applied bilaterally as the present study found no difference between the right and left upper extremities in healthy children. The normal values for arm length established in the current study differ slightly from those reported by Hensinger7 and Fryar et al.8 This is likely due to differences in the technique that arm length was measured. Both Hensinger7 and Fryar et al8 defined arm length as the distance between the acromion process and the tip of the olecranon process, whereas the present study defines arm length as the distance between the tip of the acromion process and the elbow flexion crease. Therefore, these 2 definitions of arm length are not equivalent, and care must be taken to use the same measurement method as that used to establish the normal values when making comparisons in clinical settings. In contrast, the values for forearm length established in the present study agree with those previously reported in the literature, despite differences in the definition of forearm length. Hensinger and Neu et al defined forearm length as the distance between the tip of the olecranon process and the styloid process of the ulna, whereas the present study defines this length as the distance between the elbow flexion crease and the wrist flexion crease.7,9 As both definitions of forearm length appear to be equivalent, either method of measurement can be used clinically for the assessment of forearm length.
Normal values for pediatric arm circumference have been established several times in the literature8,10-12; however, there are no data regarding normal forearm circumference to our knowledge. The values for arm circumference established in the present study are in agreement with those provided previously in the literature.8,10-12 Similar to arm and forearm length measurements, established values for arm circumference rely on data taken unilaterally; however, unilateral measurements can be applied bilaterally as the present study found no difference between left and right arm circumferences in the pediatric population. Furthermore, the current study provides the first normal values for forearm circumference in the pediatric population. Neu et al9 established average values for forearm cross-sectional area in children using unilateral peripheral quantitative computed tomography. Unfortunately, cross-sectional area measurements of the forearm are impractical for clinical purposes as they either require advanced imaging or are calculated on the basis of complicated equations based on forearm circumference and shape. In contrast, the normal values established for forearm circumference in the current study can be used to quickly and easily examine forearm girth clinically.
The equations for the estimation of arm and forearm length and circumference provide more personalized estimates for normal limb size than the normative values previously reported in the literature. For children with a limb length discrepancy, the healthy, unaffected limb can serve as a control for the evaluation of the affected limb; however, in situations where a child presents with bilateral upper extremity hypoplasia or hyperplasia, diagnosis tends to rely on the established values for arm and forearm length and arm circumference.3-5,13-15 Previously established average values have been based solely on a child’s age and sex.7-10,16 The data presented in the current study indicate that the calculation of a child’s expected arm and forearm length and circumference relies on more than just age and sex. The determination of a child’s expected arm length was found to be dependent on height, age, and sex. On the contrary, the calculation of a child’s expected arm circumference depends on weight, age, and sex. The determination of a child’s expected forearm length depends on height, weight, and age, but is independent of his or her sex. The current study provides the first normal values for forearm circumference in the pediatric population. It was determined that a child’s expected forearm circumference can be determined solely from his or her weight. It was also found that a child’s expected arm and forearm length depends on more than just his or her age and sex; therefore, when dealing with bilateral upper limb hypoplasia or hypertrophy, the equations provided by the current study can be used to give a more accurate estimate of a child’s expected arm and forearm length and circumference than a comparison with the normal values currently reported in the literature.
The equations for the determination of arm and forearm length and circumference presented here are limited in that they can only be used to determine a child’s expected upper extremity size at his or her current age based on current physical parameters. To predict the adult length of a child’s arm and forearm, Paley et al17 provided upper extremity multipliers that can be used in combination with a patient’s age and current arm or forearm length. This method can be used to accurately predict the extent of a child’s limb length discrepancy in adulthood.18,19 Currently, there is no method for predicting the adulthood circumference of a child’s upper extremity.
Arm and forearm length and circumference are the same bilaterally in the pediatric population, and contralateral limbs can be used for comparison of length and circumference of the arm and forearm in cases of unilateral upper extremity abnormality. Moreover, the present study provides several equations for the determination of a child’s expected arm and forearm length and circumference that are not solely dependent on age and sex like the previously established normative values. These equations can be used to determine a child’s expected arm and forearm length and circumference in situations where the use of a healthy, unaffected limb as a control for the evaluation of the affected limb is not possible.
Footnotes
Ethical Approval: All procedures performed in studies involving animals were in accordance with the ethical standards of the institution or practice at which the studies were conducted.
Statement of Human and Animal Rights: All procedures followed were in accordance with the ethical standards of the responsible committee on human experimentation (institutional and national) and with the Helsinki Declaration of 1975, as revised in 2008 (5).
Statement of Informed Consent: Informed consent was obtained from all patients for being included in the study. Additional informed consent was obtained from all individual participants for whom identifying information is included in this article.
Declaration of Conflicting Interests: The author(s) declared no potential conflicts of interest with respect to the research, authorship, and/or publication of this article.
Funding: The author(s) received no financial support for the research, authorship, and/or publication of this article.
References
- 1. Bae DS, Ferretti M, Waters PM. Upper extremity size differences in brachial plexus birth palsy. Hand. 2008;3(4):297-303. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 2. McDaid PJ, Kozin SH, Thoder JJ, et al. Upper extremity limb-length discrepancy in brachial plexus palsy. J Pediatr Ortho. 2002;22(3):364-366. [PubMed] [Google Scholar]
- 3. Cohen MM. Proteus syndrome: an update. Am J Med Genet C Semin Med Gentr. 2005;137C(1):38-52. [DOI] [PubMed] [Google Scholar]
- 4. Armer JM, Stewart BR. A comparison of four diagnostic criteria for lymphedema in a post-breast cancer population. Lymphat Res Biol. 2005;3(4):208-217. [DOI] [PubMed] [Google Scholar]
- 5. Phillips JJ, Gordon SJ. Conservative management of lymphoedema in children: a systematic review. J Pediatr Rehabil Med. 2014;7(4):361-372. [DOI] [PubMed] [Google Scholar]
- 6. Jones HH, Priest JD, Hayes WC, et al. Humeral hypertrophy in response to exercise. J Bone Joint Surg Am. 1977;59(2):204-208. [PubMed] [Google Scholar]
- 7. Hensinger RN. Standards in Pediatric Orthopedics: Tables, Charts, and Graphs Illustrating Growth. Philadelphia, PA: Lippincott Williams & Wilkins; 1986. [Google Scholar]
- 8. Fryar CD, Gu Q, Ogden CL. Anthropometric reference data for children and adults: United States, 2007-2010. Vital Health Stat 11. 2012:1-48. [PubMed] [Google Scholar]
- 9. Neu CM, Rauch F, Rittweger J, et al. Influence of puberty on muscle development at the forearm. Am J Physiol Endocrinol Metab. 2002;283(1):E103-E107. [DOI] [PubMed] [Google Scholar]
- 10. Dewey KG, de Onis M, Cohen RJ, et al. Implementation of the WHO multicentre growth reference study in the United States. Food Nutr Bull. 2004;25:S84-S89. [DOI] [PubMed] [Google Scholar]
- 11. Ozturk A, Budak N, Cicek B, et al. Cross-sectional reference values for mid-upper arm circumference, triceps skinfold thickness and arm fat area of Turkish children and adolescents. Int J Food Sci Nutr. 2008;60(4):267-281. [DOI] [PubMed] [Google Scholar]
- 12. Tong F, Fu T. Upper arm circumference development in Chinese children and adolescents: a pooled analysis. J Physiol Anthropol. 2015;34(1):24. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 13. Loeys BL, Dietz HC, Braverman AC, et al. The revised Ghent nosology for the Marfan syndrome. J Med Genet. 2010;47(7):476-485. [DOI] [PubMed] [Google Scholar]
- 14. Ramirez F, Dietz HC. Marfan syndrome: from molecular pathogenesis to clinical treatment. Curr Opin Genet Dev. 2007;17(3):252-258. [DOI] [PubMed] [Google Scholar]
- 15. Stout Gergich NL, Pfalzer LA, McGarvey C, et al. Preoperative assessment enables the early diagnosis and successful treatment of lymphedema. Cancer. 2008;112(12):2809-2819. [DOI] [PubMed] [Google Scholar]
- 16. Maresh MM. Linear growth of long bones of extremities from infancy through adolescence: continuing studies. AMA Am J Dis Child. 1955;89(6):725-742. [PubMed] [Google Scholar]
- 17. Paley D, Gelman A, Shualy MB, et al. Multiplier method for limb-length prediction in the upper extremity. J Hand Surg Am. 2008;33(3):385-391. [DOI] [PubMed] [Google Scholar]
- 18. Aguilar JA, Paley D, Paley J, et al. Clinical validation of the multiplier method for predicting limb length at maturity, part I. J Pediatr Ortho. 2005. a;25(2):186-191. [DOI] [PubMed] [Google Scholar]
- 19. Aguilar JA, Paley D, Paley J, et al. Clinical validation of the multiplier method for predicting limb length discrepancy and outcome of epiphysiodesis, part II. J Pediatr Ortho. 2005. b;25(2):192-196. [DOI] [PubMed] [Google Scholar]