Abstract
In quantum dot superlattices, wherein quantum dots are periodically arranged, electronic states between adjacent quantum dots are coupled by quantum resonance, which arises from the short-range electronic coupling of wave functions, and thus the formation of minibands is expected. Quantum dot superlattices have the potential to be key materials for new optoelectronic devices, such as highly efficient solar cells and photodetectors. Herein, we report the fabrication of CdTe quantum dot superlattices via the layer-by-layer assembly of positively charged polyelectrolytes and negatively charged CdTe quantum dots. We can thus control the dimension of the quantum resonance by independently changing the distances between quantum dots in the stacking (out-of-plane) and in-plane directions. Furthermore, we experimentally verify the miniband formation by measuring the excitation energy dependence of the photoluminescence spectra and detection energy dependence of the photoluminescence excitation spectra.
Subject terms: Nanophotonics and plasmonics, Quantum dots
Designing quantum dot superlattices remains a challenge. Here, the authors present CdTe quantum dot superlattices via the layer-by-layer assembly and verify the miniband formation by measuring the excitation energy the dependence of the photoluminescence spectra and the detection energy dependence of the excitation spectra.
Introduction
Semiconductor quantum dots (QDs) have high photoluminescence (PL) quantum yields (QYs) at room temperature, and their absorption and PL energies can be tuned by changing their sizes1. Therefore, they have potential applications in displays2,3, bioimaging4,5, and optoelectronic devices such as photodetectors6 and solar cells7,8. Novel optical properties based on the interaction between QDs are realised when they are sufficiently close to each other1,9–12. In particular, only when the surface-to-surface distance between QDs becomes shorter than ~2 nm, the quantum resonance, which arises from the short-range electronic coupling of wave functions, occurs between adjacent QDs11,12. When such quantum resonance appears in QD superlattices (QDSLs) in which colloidal QDs are regularly arranged, the formation of coupled electronic states (minibands) is expected. These minibands greatly improve charge transport properties; therefore, the fabrication of new optoelectronic devices utilising inter-QD interactions, such as field-effect transistors and highly efficient solar cells, have attracted considerable attention in recent years13–19.
Murray et al. were the first to report the fabrication of QDSLs, and they produced CdSe QDSLs by tailoring the composition of the dispersing medium to slowly destabilise the colloidal QD dispersion as the solvent evaporated1. Following this work, researchers have reported various methods for fabricating QDSLs such as drop-casting colloidal QD solution onto quartz substrates20, ligand exchange21, and cluster-assisted assembly22. These articles mainly discussed the fabrication process of the QDSLs.
The formation of minibands in QDSLs requires quantum resonance between adjacent QDs. However, to synthesise colloidal QDs using the hot injection method, long-chain molecules such as trioctylphosphine oxide (TOPO) or oleic acid (OA) have been usually used as ligands23,24, while the long ligands prevent short-range quantum resonance25. To solve this problem, the long ligands are usually exchanged with short-chain ligands such as ethanedithiol (EDT) or propanedithiol (PDT)17. Recently, exchange with exciton delocalised ligands that lower the QDSL potential barrier has been proposed26–29. However, the ligand exchange process degrades the PL properties of QDs30; thus, the quantum resonance must be realised between adjacent QDs in QDSLs without such ligand exchange.
One promising method is the use of water-soluble QDs because short-chain ligands such as thioglycolic acid (TGA), 3-mercaptopropionic acid (MPA), and N-acetyl-l-cysteine (NAC) are often used to synthesise water-soluble QDs11,31–36. These ligands are 0.4–0.6 nm long and are shorter than the TOPO and OA used to synthesise oil-soluble QDs. Thus, water-soluble QDs offer the advantage of reducing the distance between QDs37. In fact, we previously found the quantum resonance in the three-dimensional (3D) QDSLs composed of NAC-capped CdTe QDs deposited by the layer-by-layer (LBL) method11. The LBL assembly process is a simple and useful method for preparing highly homogeneous multilayer structures of water-soluble QDs11,38–40. This method is advantageous because the interlayer space between homogeneous QD monolayers can be precisely controlled within a single nanometre accuracy using a spacer layer composed of oppositely charged polyelectrolytes deposited during the LBL assembly11,40. The quantum resonance has been clearly observed between the CdTe QD layers in the stacking direction without ligand exchange because the QDs were synthesised using a short NAC ligand from the beginning, which enables the sufficient shortening of the distance between QDs. Therefore, the intrinsic optical properties can be investigated based on the quantum resonance between adjacent QDs without the ligand exchange. The formation of miniband in CdSe13–15, InAs16, and PbSe17,18 QD solids has been discussed mainly from the viewpoint of transport properties. In contrast, the formation of minibands derived from the quantum resonance between QDs by our approach can be discussed from the viewpoint of optical properties such as absorption and PL properties.
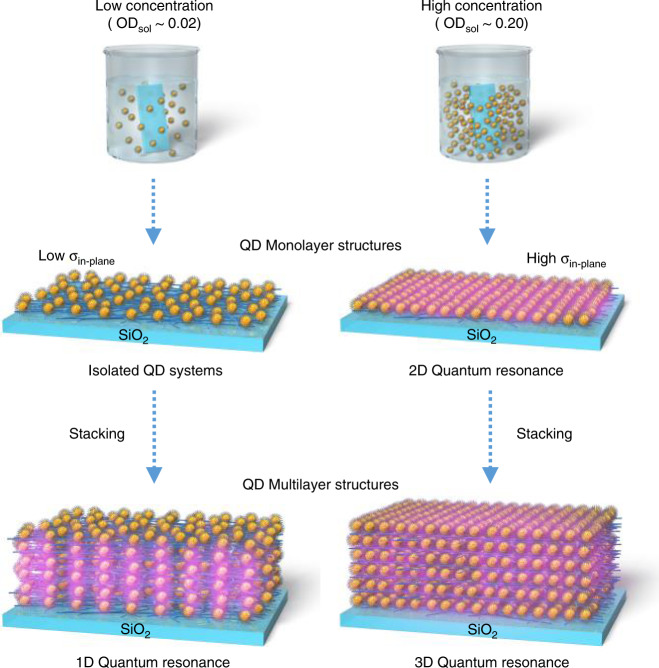
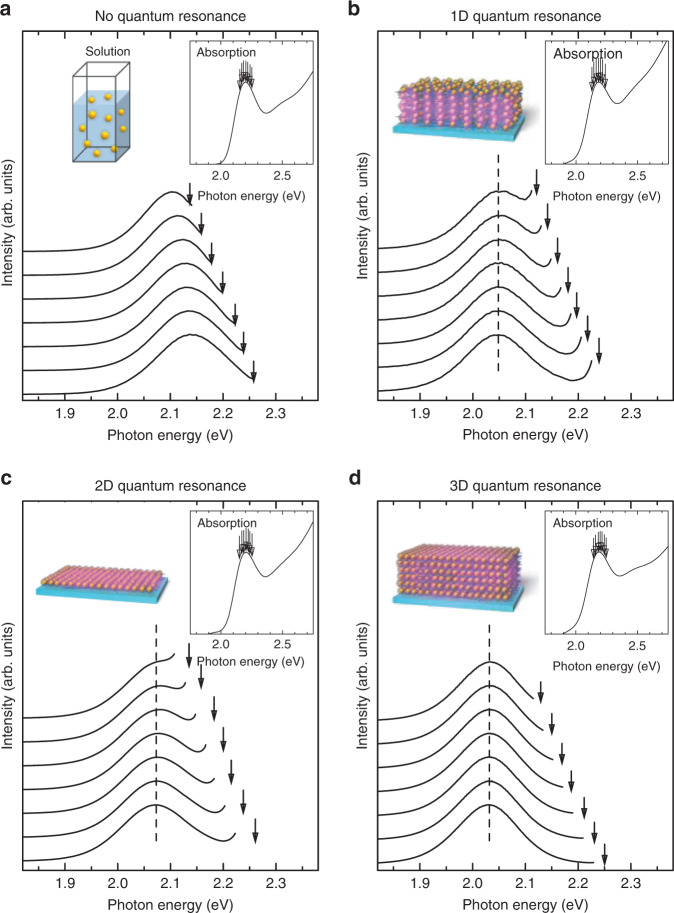
In the CdTe QDSLs deposited via the LBL method, the inter-QD surfaces become shorter than 1 nm in the in-plane direction11; thus, the quantum resonance is considered to occur not only in the stacking direction but also in the in-plane direction. In other words, the 3D quantum resonance appears in the 3D QDSLs composed of CdTe QDs. As mentioned above, during the LBL assembly, the QD interlayer distance can be controlled by the thickness of the spacer layer. If this thickness is 2 nm or more, only the in-plane (i.e. two-dimensional (2D)) quantum resonance occurs, as in the QD monolayer structure (Fig. 1). Furthermore, if the in-plane QD distance is increased, that is, if the in-plane QD density (σin-plane) becomes low, the quantum resonance in the in-plane direction is suppressed. When the QD multilayer structures are fabricated under these conditions, the quantum resonance may be realised only in the stacking direction, that is, one-dimensional (1D) quantum resonance (Fig. 1), which is connected to the motivation of this study. In this paper, we report the successful control of the quantum resonance dimensions. In addition, we evaluate the formation of minibands in the QDSLs, in which the 1D, 2D, and 3D quantum resonance takes place, by measuring both the excitation energy dependence of the PL spectra and detection energy dependence of the PL excitation (PLE) spectra.
Fig. 1. Schematics of quantum dot (QD) layer structures in which the 1D, 2D, and 3D quantum resonances occur.
We controlled the in-plane QD density (σin-plane) by changing the concentration of the QD solution and hence the optical density of the QD solution (ODsol) using a layer-by-layer assembly method. The left and right sides of the figure represent QD layer structures with low and high σin-plane, respectively. If σin-plane is low, the quantum resonance in the in-plane direction is suppressed (isolated QD systems). When QD multilayer structures are fabricated under the conditions of low σin-plane, the quantum resonance occurs only in the stacking direction, that is, the 1D quantum resonance is realised. In the QD monolayer structure with high σin-plane, only the in-plane 2D quantum resonance occurs. In the QD multilayer structures with high σin-plane, the quantum resonance appears not only in the stacking direction but also in the in-plane direction. In other words, the 3D quantum resonance occurs in the 3D QD superlattices. The coupled electronic states are represented by translucent purple colour.
Results
2D quantum resonance in QD monolayers
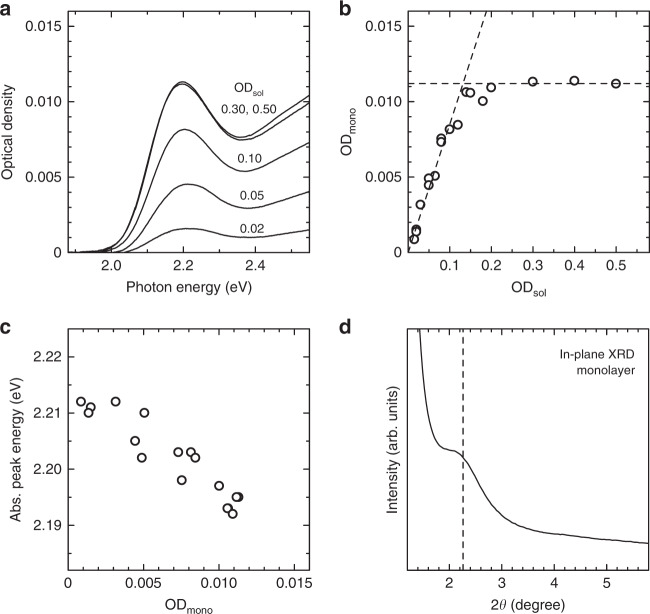
We used the NAC-capped CdTe QDs with a diameter d = 3.4 ± 0.3 nm. The typical transmission electron microscopy (TEM) image and size histogram are shown in Supplementary Fig. 1. Figure 2a shows the absorption spectra of various CdTe QD monolayers prepared using colloidal QD solutions with different concentrations. The absorption spectra were obtained by correcting the baseline in the measured extinction spectra. The details of the analysis of the absorption spectra are described in Supplementary Information (Supplementary Figs. 2 and 3). We define ODsol as the optical density (OD) of the first absorption peak in the colloidal solution, measured using a cell with an optical path length of 10 mm. We plotted the OD of the absorption peak of the monolayers (ODmono) against ODsol, as shown in Fig. 2b. In the low-concentration region (i.e. ODsol < 0.15), ODmono increases linearly. This finding indicates that σin-plane of the monolayers can be controlled by changing the concentration of the colloidal solution. In addition, in the high-concentration region (ODsol > 0.15), ODmono is saturated, which indicates that the QDs are closely packed in the monolayer without any space for accepting another QD.
Fig. 2. Absorption properties and X-ray diffraction (XRD) structural analyses of quantum dot monolayers.
a Absorption spectra of CdTe quantum dot monolayers fabricated from colloidal quantum dot solutions with different concentrations. b Optical densities (ODs) of the absorption peaks of the monolayers (ODmono) versus the OD of the absorption peak of the colloidal quantum dot solution (ODsol). One of the dashed lines is proportional to ODsol, which shows that the ODmono is proportional to ODsol. The other dashed line shows the value at which the ODmono is saturated. c Dependence of the absorption peak energy of the quantum dot monolayers on ODmono. d In-plane XRD pattern of the CdTe quantum dot monolayer with high in-plane quantum dot density (σin-plane), which exhibits 2D quantum resonance. The dashed line shows the peak value.
We focused on the absorption peak energy of the monolayers. Figure 2c shows the dependence of the absorption peak energy of the monolayers on ODmono. When ODmono is 0.005 or less, the absorption peak energy of the monolayer is nearly constant and coincides with the absorption peak energy of the colloidal solution in which the QDs are randomly dispersed. This observation indicates that QDs in the monolayers with low σin-plane do not couple with each other, and the absorption properties of isolated individual QDs are observed. On the other hand, when ODmono is 0.005 or more, the absorption peak shifts to a lower energy as σin-plane increases. This low-energy shift can be attributed to the in-plane quantum resonance between adjacent QDs.
We recorded the in-plane X-ray diffraction (XRD) patterns of the closely packed monolayer to confirm the periodicity in the in-plane direction as shown in Fig. 2d. The diffraction peak appears at 2θ angle of 2.3°, which means that the QD monolayer has an in-plane spatial period of ~3.9 nm, which is almost similar to the QD diameter. Therefore, the XRD results indicate that the QDs are closely packed and periodically arrayed in the plane; therefore, we successfully fabricated the 2D QDSLs. Because the average diameter of the CdTe QDs is 3.4 nm, the surface-to-surface distance between QDs is estimated to be ~0.5 nm. As discussed in ref. 11, the quantum resonance arises from the coupling of the wave functions between the adjacent QDs, which is a short-range interaction, and its strength weakens exponentially with the increase in surface-to-surface distance between QDs. When the QD interlayer distance is larger than 2 nm, the absorption peak hardly shifts. Therefore, that the quantum resonance can occur only when the QD distance is ~2 nm or less, and the distance of 0.5 nm is sufficiently short to realise miniband formation.
The in-plane quantum resonance can be concluded to cause the low-energy shift in the absorption peak energy of the 2D QDSL (ODsol > 0.005) relative to the absorption peak energy of the QD monolayer in which the QDs are randomly dispersed in the plane (ODsol < 0.005), as shown in Fig. 2c. The absorption energy decreased by 19 meV, which can be considered as the coupling energy caused by the in-plane quantum resonance. We define this energy shift as ΔEin-plane (=19 meV), as schematically shown in Supplementary Fig. 4.
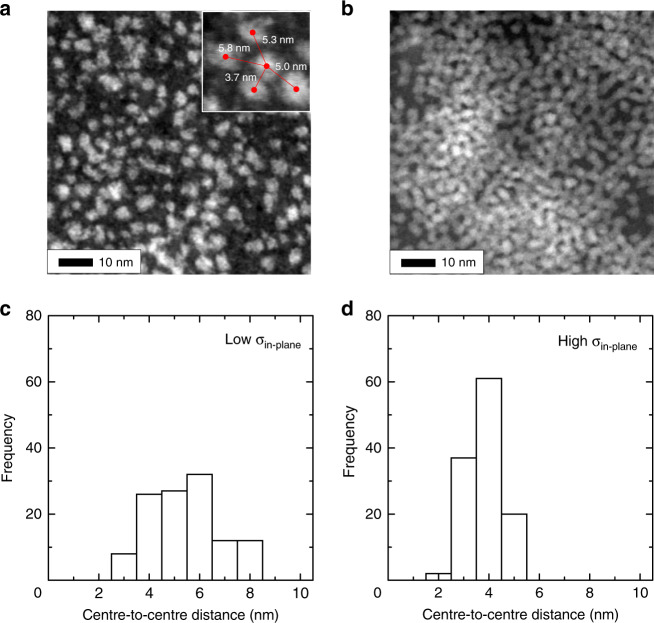
The quantum resonance between adjacent QDs greatly depends on the distance between the QDs. To control the in-plane quantum resonance, the distance between QDs in the QD monolayer must be carefully controlled within a single nanometre accuracy. We determined the average surface-to-surface distance between QDs by examining scanning transmission electron microscopy (STEM) images of the monolayers. Figure 3a, b show the STEM images of the monolayers fabricated under the conditions without (ODsol =0.02) and with (ODsol = 0.15).the occurrence of in-plane quantum resonance, respectively. In the images, we directly measured 120 centre-to-centre distances between QDs as shown in the inset in Fig. 3a; the corresponding histograms of the centre-to-centre distances are shown in Fig. 3c, d. From the histograms, the average centre-to-centre distances are estimated to be 5.6 nm with ODsol = 0.02 and 3.9 nm with ODsol = 0.15. The average diameter of the CdTe QDs is 3.4 nm; therefore, the average surface-to-surface distances can be estimated to be 2.2 nm with ODsol = 0.02 and 0.5 nm with ODsol = 0.15. In addition, the variance of the average surface-to-surface distances is larger with ODsol = 0.02 than with ODsol = 0.15, implying that QDs are dispersed not periodically but quite randomly in the former compared to the latter. The results in Figs. 2 and 3 clearly demonstrate that by merely changing σin-plane, we can effectively control the fabrication of either the isolated QD systems without any quantum resonance or the 2D QDSLs with in-plane quantum resonance.
Fig. 3. Scanning transmission electron microscopy images of quantum dot monolayers.
a, b Scanning transmission electron microscopy images of quantum dot monolayers with low a and high b in-plane quantum dot density (σin-plane), which were fabricated with ODsol = 0.02 and 0.15, respectively. The inset in the figure is the enlarged image. The red dots point to the centre of the quantum dots, and the red lines connect the points. The numbers shown in white indicate the length of the red lines, which are centre-to-centre distance between quantum dots. c, d Histograms of the centre-to-centre distances between quantum dots in the quantum dot monolayers with low c and high d σin-plane.
1D and 3D quantum resonance in QD multilayers
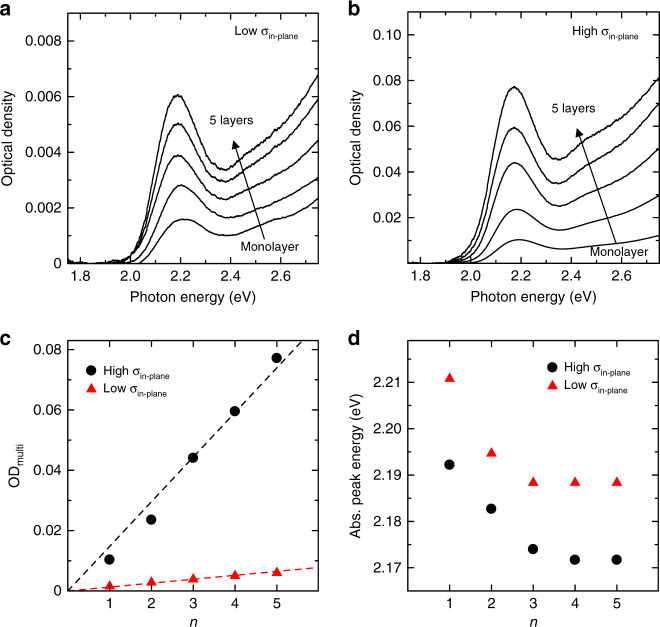
Next, we discuss the absorption properties of the multilayer CdTe QD structures (hereafter referred to as ‘QD multilayers’ for simplicity) with low σin-plane in which the in-plane quantum resonance does not occur and the QD multilayers with high σin-plane in which the in-plane quantum resonance occurs. We prepared the QD multilayers with low and high σin-plane by using the colloidal solutions of ODsol = 0.02 and 0.20, respectively. Figure 4a, b show the corresponding absorption spectra of the QD multilayers prepared with these two different optical densities. Figure 4c shows the OD of the absorption peak in the QD multilayer (ODmulti) as a function of the number of layers (n). ODmulti linearly increases with the number of layers, which suggests that QDs can be stacked at almost the same density in each layer. In addition, the difference in the increasing slope indicates that the σin-plane values are different in the QD multilayers that are fabricated under the conditions of the different ODsol values. Figure 4d shows the absorption peak energy of the QD multilayers as a function of n. Notably, the absorption peak energy decreases as n increases in the QD multilayers with high σin-plane. Also, in the QD multilayers with low σin-plane, the absorption peak shifts to the lower energy with an increase in n.
Fig. 4. Absorption properties of quantum dot multilayers.
a, b Absorption spectra of the CdTe quantum dot multilayers with a low and b high in-plane quantum dot density (σin-plane). c Optical density of the absorption peak of the multilayers (ODmulti) versus the number of layers (n). The dashed lines are proportional to n, which show that the ODmulti are proportional to n. d Absorption peak energy of the multilayers versus n.
We discuss the amount of absorption energy shift. We define ΔEstacking as the difference in the absorption peak energies between the QD monolayer with n = 1 and QD multilayer with n = 5 in which the absorption energy stops shifting. In the samples with low and high σin-plane, the ΔEstacking values are 22 and 20 meV, respectively, which are almost the same. In the QD multilayers with low σin-plane, although the shift of the absorption peak energy stops at n = 5, the peak energy does not approach the same number of layers in the QD multilayers with high σin-plane. This result indicates that the red shift in the QD multilayers prepared with low σin-plane is not due to increase in σin-plane. The QD multilayers were prepared by the LBL assembly of the cationic polymer and CdTe QDs. It is considered that the presence of the polymer layer makes it possible to fabricate the QD multilayers with low σin-plane without increasing σin-plane even at n = 3–5. These results strongly support that the quantum resonance occurs in the stacking direction not only in the QD multilayers with high σin-plane but also in the QD multilayers with low σin-plane. Therefore, 1D quantum resonance occurs in the stacking direction in QD multilayers prepared with low σin-plane.
We also measured out-of-plane XRD patterns to confirm the periodic structural ordering in the stacking direction. Figure 5 shows the out-of-plane XRD patterns of the CdTe QD multilayers (30 layers) prepared under with high and low σin-plane. Notably, the diffraction peak is observed at almost the same 2θ angle of 2.5° in the both samples prepared under these two conditions. These results indicate that both of the CdTe QD multilayers exhibit the same structural regularity with a period of ~3.5 nm in the stacking direction. The coincidence of the diffraction angles suggests that they are aligned with the same spatial period in the stacking direction regardless of σin-plane. In other words, QDs are regularly arranged in the stacking direction even in QD multilayers with low σin-plane. Actually, as shown in Fig. 4d, the 1D quantum resonance occurs in the stacking direction even in the QD multilayers with low σin-plane. In the CdTe QD multilayers prepared with high σin-plane, the quantum resonance occurs in both the in-plane and stacking directions, that is, the 3D quantum resonance. In the CdTe QD monolayer prepared with high σin-plane, the 2D quantum resonance occurs only in the in-plane direction. Therefore, we can control the dimension of the quantum resonance by changing σin-plane and the number of layers in the LBL assembly.
Fig. 5. X-ray diffraction structural analysis of quantum dot multilayers.
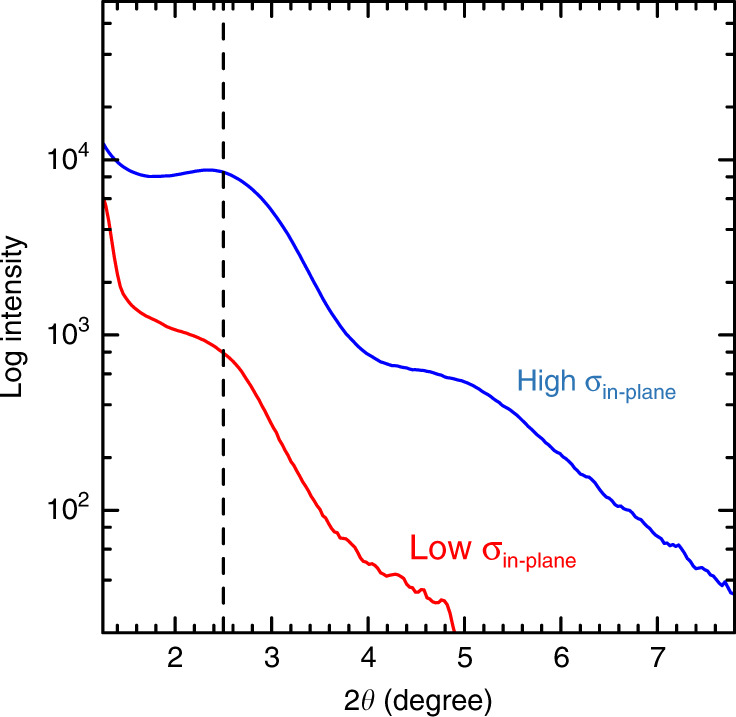
Out-of-plane X-ray diffraction patterns of CdTe quantum dot 30 layers with low (red) and high (blue) in-plane quantum dot density (σin-plane). The dashed lines show the peak value of the X-ray diffraction.
Supplementary Fig. 4 schematically shows the energy shift of the absorption peak due to the quantum resonance. It was revealed that ΔEin-plane, which is the energy shift due to the in-plane quantum resonance, is 19 meV as discussed in Fig. 2c. In addition, as discussed Fig. 4d, the energy shift ΔEin-plane owing to the quantum resonance in the stacking direction is 22 and 20 meV in the QD multilayers prepared with low and high σin-plane, respectively. The difference between the absorption energies of isolated QD sample and 3D QDSL is 39 meV, which corresponds to the sum of the resonant coupling energies due to the quantum resonance in both the stacking and in-plane direction.
The shift of the absorption peak in the QDSL in which 1D quantum resonance occurs was more than that of the QDSL in which 2D quantum resonance occurs. The reason for this is that the in-plane QD distance of 3.9 nm is longer than the interlayer distance of 3.5 nm due to the electrostatic repulsion between the ligand of NAC. In addition, since there is no medium between the in-plane QDs and the medium between the QDs in the stacking direction is poly(diallyldimethylammonium chloride) (PDDA), the presence of PDDA may make the quantum resonance between the QDs in the stacking direction stronger than in the in-plane direction.
Dimensional effect of quantum resonance in QDSLs
To evaluate the formation of the minibands in the QDSLs, we measured the excitation energy dependence of the PL spectra and detection energy dependence of the PLE spectra in the QDSL fabricated by the LBL method. For comparison, we performed the same measurements for the QD solution. The QDs are randomly dispersed in the solution, and sufficiently large distances between QDs cause no interactions between them. Figure 6a shows the excitation energy dependence of the PL spectra of the CdTe QD solution. Evidently, the PL peak shifts towards the lower energies as the excitation energy decreases, which corresponds to typical size-selective spectroscopy results41–43. The optical spectra of semiconductor QDs are usually inhomogeneously broadened because of the QD size distribution. When the excitation energy is low, relatively large QDs are selectively excited, and the PL peak energy decreases, and vice versa. The current experimental results observed in the QD solution originated from such selective excitation of a specific size of QDs that resonated with a specific excitation energy. In contrast, the experimental results for the QDSLs were completely different from those of the solution. Figure 6b–d show the excitation energy dependence of the PL spectra of the QDSLs in which the 1D, 2D, and 3D quantum resonance occurs, respectively. We found that the PL peak did not shift in any of the three QDSLs even when the excitation energy was changed; the size selectivity was not observed in the QDSLs.
Fig. 6. Excitation energy dependence of the photoluminescence spectra.
a–d Excitation energy dependence of the photoluminescence spectra of CdTe quantum dot solution in which quantum dots are randomly dispersed a, and CdTe quantum dot superlattices exhibiting 1D b, 2D c, and 3D d quantum resonances. The solid lines show photoluminescence spectra with different excitation energies. The excitation energy increases from top to bottom. The insets show the absorption spectra of each sample. The down arrows indicate the excitation energies in the photoluminescence measurements. The dashed lines show the peak value of the photoluminescence spectra.
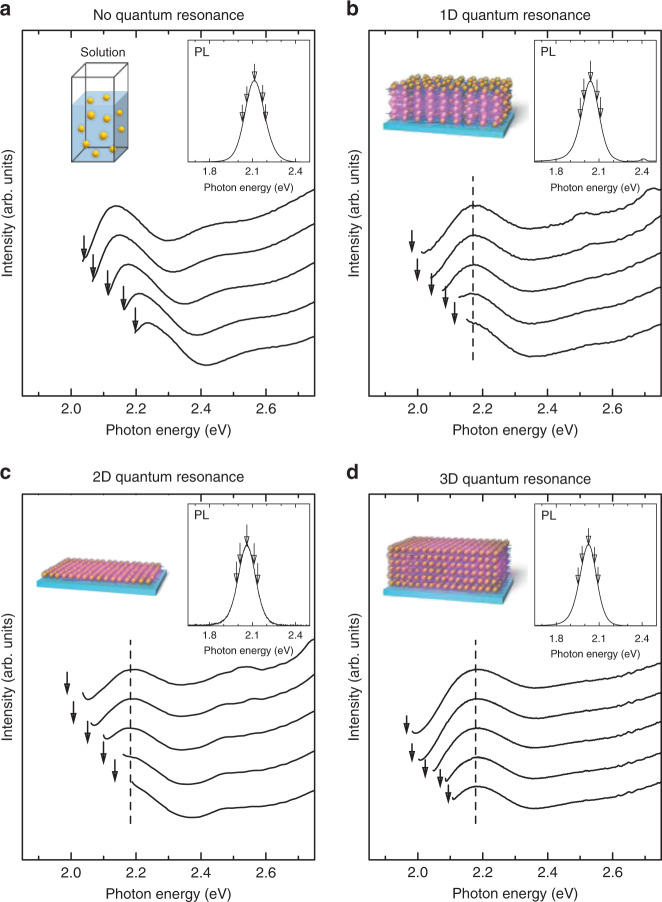
We also measured the detection energy dependence of the PLE spectra in the QD solution and three QDSLs, as shown in Fig. 7. The inset represents the PL spectrum, and the down arrows indicate the detection energies. Similar to the PL spectra behaviour, the PLE peak energy increases as the detection energy increases in the case of the QD solution. This is again a typical result of the size-selective spectroscopy41–43. Figure 7b–d show the detection energy dependence of the PLE spectra in the three QDSLs in which the 1D, 2D, and 3D quantum resonance appears, respectively. We found that the PLE peak energy did not shift even when the detection energy changed in all the QDSLs.
Fig. 7. Detection energy dependence of the photoluminescence (PL) excitation spectra.
a–d Detection energy dependence of the PL excitation spectra of the CdTe quantum dot solution a and CdTe quantum dot superlattices in which the 1D b, 2D c, and 3D d quantum resonances occur. The solid lines show PL excitation spectra with different detection energies. The detection energy increases from top to bottom. The insets show the PL spectra of each sample. The down arrows indicate the detection energies in the PL excitation measurements. The dashed lines show the peak value of the PL excitation spectra.
Thus far, Kagan and Crooker et al. have investigated the optical properties of CdSe and CdSe/ZnS QD solids and interpreted their results by considering the energy transfer (ET) mechanism44–47. In these QD solids, the shift of the absorption peak was not observed compared with solution samples, and thus it is considered that quantum resonance between QDs does not occur in these systems. Miyazaki et al. also discussed exciton hopping based on ET that occurs in QD arrays by comparing the optical properties of CdSe/ZnS QD arrays and solution samples20,43. No shift of absorption peak was observed in the CdSe/ZnS QD arrays as well20. That is, the quantum resonance between QDs did not occur, and only long-range ET between QDs occurred in the CdSe/ZnS QD arrays because the distance between the QDs is large in the CdSe/ZnS QDSLs owing to the presence of long ligands of octadecylamine. Furthermore, they reported experimental results on the excitation energy dependence of PL spectra in the QDSLs of octadecylamine-capped CdSe/ZnS QDs43. Although the shifts of the PL energy in the CdSe/ZnS QDSLs with respect to changes in the excitation energy were slightly smaller than that in solution samples, the size selectivity was still clearly observed even in the QDSLs.
To confirm this observation, we prepared OA-capped CdTe QDs and their QDSLs possessing a longer inter-QD distance (Supplementary Fig. 5a) and investigated the excitation energy dependence of the PL spectra. From Supplementary Fig. 5b, c, it can be seen that the PL peak shifts to the higher energy side as the excitation energy increases not only in the solution sample but also in QDSL, that is, the size selectivity is clearly observed. As shown in Supplementary Fig. 5d, in OA-capped CdTe QDSL, the shift of the PLE peak is also clearly observed when the detection energy is changed. In contrast, in the present NAC-capped CdTe QDSLs, the quantum resonance clearly occurs as discussed above, and the size selectivity is lost, reflecting the formation of the coupled states between QDs.
Thus far, in one-dimensional systems such as quantum wires and 1D QDSLs of InAs, it has been reported theoretically and experimentally that the radiative recombination lifetime depends on temperature and is proportional to T0.5 48–51. We measured the temperature dependence of the PL-decay profiles to confirm that a one-dimensional miniband was formed in the QD multilayer prepared with the condition of low σin-plane. Supplementary Fig. 6a shows the PL-decay profiles measured at 80, 180, and 250 K. The solid curves are the results of fittings performed using three exponential functions. The temperature dependence of the average decay time is shown in Supplementary Fig. 6b. In the temperature range from 80 to 250 K, the PL intensity is almost constant, and that non-radiative recombination process contributes minimally. Thus, the observed PL decay time reflects the radiative recombination lifetime. For the film samples in which CdTe QDs are randomly dispersed, the PL decay time is independent of temperature52, while for the QD multilayer prepared with the condition of low σin-plane, the decay time depends on the temperature. Furthermore, in the temperature region above 80 K, the decay is proportional to T0.5, which clearly demonstrates the formation of a one-dimensional miniband. In addition, we measured the temperature dependence of the PL decay times in the QDSLs in which 2D and 3D quantum resonances occur (Supplementary Fig. 6c, d) as well as 1D (Supplementary Fig. 6b). As shown in Supplementary Fig. 6c, d. PL decay times in CdTe QDSLs in which the 1D and 2D quantum resonances occur are proportional to T0.5 and T1.0, respectively. In ref. 49, Akiyama et al. theoretically and experimentally demonstrated that the PL decay times of quantum wires and quantum wells, in which 1D and 2D electronic states are formed, are proportional to T0.5 and T1.0, respectively. Therefore, the present temperature dependence of PL decay times strongly demonstrate the formation of the 1D and 2D minibands in the CdTe QDSLs. Furthermore, the PL decay time in the 3D CdTe QDSLs is approximately proportional to T1.5, demonstrating 3D miniband formation. We believe that these experimental results further demonstrate the quantum resonance-based miniband formation in the CdTe QDSLs.
The PL QYs for 1D, 2D, and 3D structures are 27%, 26%, and 22%, respectively, and the PL decay times are 2.7, 3.6, and 4.4 ns, respectively. The PL QY decreases slightly, and the PL decay time increases slightly as the dimensionality increases. These results suggest that excitons can be more widely delocalised owing to the stronger quantum resonance with the higher dimensionality. It is expected that the radiation mechanism in QDSLs will be further clarified by examining the transient absorption and its temperature dependence in detail.
Discussion
We fabricated the CdTe QDSLs by LBL method and successfully controlled their dimensions of quantum resonance. The experimental results of the excitation energy dependence of the PL spectra and detection energy dependence of the PLE spectra clearly demonstrate the formation of coupled electronic states (minibands) in the CdTe QDSLs. This is the first report to show that the dimension of the quantum resonance can be controlled and that the resulting minibands are clearly formed. The fabrication of the CdTe QDSLs by the LBL method proposed in this study can be applied to other water-soluble semiconductor QDs and metal nanoparticles, as well as oxide, dielectric, and magnetic nanoparticles. Furthermore, the ability to combine different types of semiconductor QDs or combine semiconductor QDs with other nanoparticles will expand the possibilities of new material design including more complex heteronanostructures. QDSLs have been theoretically shown to have a higher multiple-exciton generation efficiency than isolated QDs53, and the efficiency changes depending on the dimension of QDSLs54. In this study, we were able to clarify the formation of the minibands based on the picture of the 1D, 2D, and 3D quantum resonance in the CdTe QDSLs. Further elucidation of novel optical properties such as the multiple-exciton generation and hot electron dynamics in QDSLs is desired.
Methods
We prepared NAC-capped CdTe QDs using a previously reported procedure34. First, we added 250 mg of tellurium powder (purity 99.99%; purchased from Kojundo Chemical) and 312.5 mg of sodium borohydride (purity 98%; purchased from Kanto Chemical) to a vial and then added 6.0 mL of deionized (DI) water before sealing the vial. We then cooled the vial in an ice bath and allowed it to react for ~8 h. In the solution, the chemical reaction generates NaHTe as a tellurium ion source and Na2B4O7 as a precipitate. Next, we dissolved NAC (purity 98%; purchased from Kishida Chemical) and Cd(ClO4)2·6H2O (purity 99%; purchased from FUJIFILM Wako Pure Chemical) in 100 mL of DI water. We adjusted the solution to pH 7.0 via the stepwise addition of 0.2 mM NaOH (purchased from Kishida Chemical), and then, we added the prepared NaHTe solution. Finally, we added dilute HCl (purchased from Kishida Chemical) and adjusted the pH to 5.0 to complete the CdTe QD precursor. The molar ratio in the precursor was Cd:Te:NAC = 1.0:0.3:1.2, and the concentration was [Cd2+] = 20 mM. We synthesised CdTe QDs by heating 10 mL of the precursor solution in an autoclave at 200 °C for 20 min and then cooling it in an ice bath.
We fabricated CdTe QDSLs via LBL assembly. First, we immersed a quartz substrate in a piranha solution (98% H2SO4:30% H2O2 = 7:3, v/v) heated at 150 °C to perform a hydrophilic treatment and to negatively charge the substrate surface. Next, we alternately immersed the substrate in a PDDA solution (20 wt% in H2O; purchased from Sigma-Aldrich), which is a cationic polymer, and a polyacrylic acid (PAA) solution ((~25%); purchased from FUJIFILM Wako Pure Chemical), which is an anionic polymer. In this process, we washed the substrate with DI water before immersing the substrate in a different solution. After we fabricated the PDDA/(PAA/PDDA)2 layers on the substrate, we immersed the substrate in a NAC-capped CdTe QD solution. Because NAC has negative chargeability in water, the QD monolayer can be fabricated on the substrate by utilising electrostatic interactions. In addition, we fabricated a QD multilayer by alternatively immersing the substrate in the PDDA solution and CdTe QD solution.
For the STEM and TEM measurements, we fabricated CdTe QD monolayers with low and high σin-plane on a Cu grid supported by an elastic carbon film (ELS-C10 Cu100P grid; purchased from OkenShoji). First, we hydrophilized the surface of the grid using a plasma treatment. Next, we prepared QD monolayers by drop-casting the PDDA, PAA, and QD solutions onto the grid in the same order as described previously. During this process, we washed the grid with DI water before the different solutions were drop-cast.
OA-capped CdTe was synthesised by hot-injection methods55. In a glovebox, a Te solution was prepared by dissolving 0.0512 g of Te powder (0.40 mmol) in 0.414 g of tri-n-butylphosphine (TBP) and subsequent dilution with 19.94 mL of 1-octadecene (ODE). A Cd source mixture was prepared by dissolving 0.0128 g of CdO (0.10 mmol), 0.1138 mL of OA (0.36 mmol), and 4.91 mL of ODE at 295 °C in a three-neck flask to obtain a clear solution. At this temperature, 2.535 mL of Te (0.05 mmol) solution was quickly injected into the Cd source solution. After 1 min from the injection, reaction vessel was transferred into ice bath to stop the reaction. For the purification, 4 mL of as-prepared CdTe QD solution and a mixture of hexane and methanol (1/2, v/v) were vigorously shook in a 15 mL centrifuge tube and then centrifuged at 10,000 rpm for 5 min. After the ODE phase was extracted, same procedure was repeated once and then the CdTe QD in the ODE phase was precipitated with 40 mL of acetone. The OA-capped CdTe QD solution was obtained by centrifugation (10,000 rpm for 10 min), decantation and re-dispersion into 4 mL of toluene. All synthesis and purification processes were carried out under nitrogen flow condition.
We recorded the extinction spectra using a JASCO V-650 spectrometer with a spectral resolution of 0.2 nm. The absorption spectra specific to CdTe QD layers were obtained by correcting the baseline in the measured extinction spectra. The details of the analysis of the absorption spectra is described in Supplementary Information. We measured the PL and PLE spectra using a JASCO FP-8300 spectrofluorometer with a spectral resolution of 0.5 nm. The PL QYs were measured using a JASCO FP-8300 spectrofluorometer equipped with an integrating sphere. For the measurements of PL-decay profiles, a laser-diode (405 nm, PicoQuant LDH-P-C-405) with a pulse duration of 50 ps and a repetition of 125 kHz was used as the excitation light. The PL-decay profiles were recorded using a Hamamatsu C5094 imaging spectrograph and C4334 streak scope. We recorded XRD patterns with a Rigaku SmartLab using Cu Kα radiation (λ = 0.154 nm). We performed STEM measurements by using a Thermo Fisher Scientific Talos at an accelerating voltage of 200 kV, and we obtained TEM images using a JEOL JEM-2100F/SP operated at 200 kV.
Supplementary information
Acknowledgements
The authors acknowledge Thermo Fisher Co. for the STEM measurements. K.H.-D. acknowledges financial support from JST (PRESTO), Toyota Mobility Foundation, and Grant-in-Aid for Scientific Research on Innovative Areas, Grant No. 18H05407. D.K. acknowledges financial support from JSPS KAKENHI, Grant Nos. 24560015 and 20H02549.
Author contributions
T.L. and K.O. designed the experiment, prepared CdTe QDs and QDSLs, acquired and analysed absorption, PL, and PLE spectra. K.E. acquired TEM images, prepared OA-capped CdTe QDSLs, and analysed PL and PLE spectra. D.I. and T.K. supported the STEM measurements. K.H.-D., Y.-J.P. and D.K. provided conceptual advice. All authors contributed to the interpretation of the results and preparation of the manuscript.
Data availability
The data that support the findings of this study are available from the corresponding author upon reasonable request.
Competing interests
The authors declare no competing interests.
Footnotes
Peer review information Nature Communications thanks Chih-Jen Shih and the other, anonymous reviewer for their contribution to the peer review of this work. Peer reviewer reports are available.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Contributor Information
Yong-Jin Pu, Email: yongjin.pu@riken.jp.
DaeGwi Kim, Email: tegi@a-phys.eng.osaka-cu.ac.jp.
Supplementary information
Supplementary information is available for this paper at 10.1038/s41467-020-19337-0.
References
- 1.Murray CB, Kagan CR, Bawendi MG. Synthesis and characterization of monodisperse nanocrystals and close-packed nanocrystal assemblies. Annu. Rev. Mater. Sci. 2000;30:545–610. doi: 10.1146/annurev.matsci.30.1.545. [DOI] [Google Scholar]
- 2.Jang BE, et al. White-light-emitting diodes with quantum dot color converters for display backlights. Adv. Mater. 2010;22:3076–3080. doi: 10.1002/adma.201000525. [DOI] [PubMed] [Google Scholar]
- 3.Erden T, Demir HV. Colloidal nanocrystals for quality lighting and displays: milestones and recent developments. Nanophotonics. 2016;5:74–95. doi: 10.1515/nanoph-2016-0009. [DOI] [Google Scholar]
- 4.Resch-Genger U, Grabolle M, Cavaliere-Jaricot S, Nitschke R, Nann T. Quantum dots versus organic dyes as fluorescent labels. Nat. Methods. 2008;5:763–775. doi: 10.1038/nmeth.1248. [DOI] [PubMed] [Google Scholar]
- 5.Zhou J, Yang Y, Zhang C. Toward biocompatible semiconductor quantum dots: From biosynthesis and bioconjugation to biomedical application. Chem. Rev. 2015;115:11669–11717. doi: 10.1021/acs.chemrev.5b00049. [DOI] [PubMed] [Google Scholar]
- 6.Carey GH, et al. Colloidal quantum dot solar cells. Chem. Rev. 2015;115:12732–12763. doi: 10.1021/acs.chemrev.5b00063. [DOI] [PubMed] [Google Scholar]
- 7.Konstantatos G, et al. Ultrasensitive solution-cast quantum dot photodetectors. Nature. 2006;442:180–183. doi: 10.1038/nature04855. [DOI] [PubMed] [Google Scholar]
- 8.Talapin DV, Lee J-S, Kovalenko MV, Shevchenko EV. Prospects of colloidal nanocrystals for electronic and optoelectronic applications. Chem. Rev. 2010;110:389–458. doi: 10.1021/cr900137k. [DOI] [PubMed] [Google Scholar]
- 9.Hanrath T. Colloidal nanocrystal quantum dot assemblies as artificial solids. J. Vac. Sci. Technol. A. 2012;30:030802. doi: 10.1116/1.4705402. [DOI] [Google Scholar]
- 10.Kholmicheva N, Moroz P, Eckard H, Jensen G, Zamkov M. Energy transfer in quantum dot solids. ACS Energy Lett. 2017;2:154–160. doi: 10.1021/acsenergylett.6b00569. [DOI] [Google Scholar]
- 11.Kim D, et al. Evidence of quantum resonance in periodically-ordered three-dimensional superlattice of CdTe quantum dots. Nano Lett. 2015;15:4343–4347. doi: 10.1021/acs.nanolett.5b00335. [DOI] [PubMed] [Google Scholar]
- 12.Kagan CR, Murray CB. Charge transport in strongly coupled quantum dot solids. Nat. Nanotechnol. 2015;10:1013–1026. doi: 10.1038/nnano.2015.247. [DOI] [PubMed] [Google Scholar]
- 13.Kovalenko MV, Scheele M, Talapin DV. Colloidal nanocrystals with molecular metal chalcogenide surface ligands. Science. 2009;324:1417. doi: 10.1126/science.1170524. [DOI] [PubMed] [Google Scholar]
- 14.Lee J-S, Kovalenko MV, Huang J, Chung DS, Talapin DV. Band-like transport, high electron mobility and high photoconductivity in all-inorganic nanocrystal arrays. Nat. Nanotechnol. 2011;6:348–352. doi: 10.1038/nnano.2011.46. [DOI] [PubMed] [Google Scholar]
- 15.Choi J-H, et al. Bandlike transport in strongly coupled and doped quantum dot solids: a route to high-performance thin-film electronics. Nano Lett. 2012;12:2631–2638. doi: 10.1021/nl301104z. [DOI] [PubMed] [Google Scholar]
- 16.Jang J, Liu W, Son JS, Talapin DV. Temperature-dependent hall and field-effect mobility in strongly coupled all-inorganic nanocrystal arrays. Nano Lett. 2014;14:653–662. doi: 10.1021/nl403889u. [DOI] [PubMed] [Google Scholar]
- 17.Liu Y, et al. Dependence of carrier mobility on nanocrystal size and ligand length in PbSe nanocrystal solids. Nano Lett. 2010;10:1960–1969. doi: 10.1021/nl101284k. [DOI] [PubMed] [Google Scholar]
- 18.Talgorn E, et al. Unity quantum yield of photogenerated charges and band-like transport in quantum-dot solids. Nat. Nanotechnol. 2011;6:733–739. doi: 10.1038/nnano.2011.159. [DOI] [PubMed] [Google Scholar]
- 19.Kagan CR, Lifshitz E, Sargent EH, Talapin DV. Building devices from colloidal quantum dots. Science. 2016;353:aac5523. doi: 10.1126/science.aac5523. [DOI] [PubMed] [Google Scholar]
- 20.Miyazaki J, Kinoshita S. Temperature-dependent exciton hopping in an array of inhomogeneously broadened quantum dots. J. Phys. Soc. Jpn. 2012;81:074708. doi: 10.1143/JPSJ.81.074708. [DOI] [Google Scholar]
- 21.Dong A, Chen J, Vora PM, Kikkawa JM, Murray CB. Binary nanocrystal superlattice membranes self-assembled at the liquid-air Interface. Nature. 2010;466:474–477. doi: 10.1038/nature09188. [DOI] [PubMed] [Google Scholar]
- 22.Zhang X, et al. Self-assembly of one-dimensional nanocrystal superlattice chains mediated by molecular clusters. J. Am. Chem. Soc. 2016;138:3290–3293. doi: 10.1021/jacs.6b00055. [DOI] [PubMed] [Google Scholar]
- 23.Murray CB, Norris DJ, Bawendi MG. Synthesis and characterization of nearly monodisperse CdE (E = S, Se, Te) semiconductor nanocrystallites. J. Am. Chem. Soc. 1993;115:8706–8715. doi: 10.1021/ja00072a025. [DOI] [Google Scholar]
- 24.Reiss P, Carrière M, Lincheneau C, Vaure L, Tamang S. Synthesis of semiconductor nanocrystals, focusing on nontoxic and earth-abundant materials. Chem. Rev. 2016;116:10731–10819. doi: 10.1021/acs.chemrev.6b00116. [DOI] [PubMed] [Google Scholar]
- 25.Yoon SJ, Guo Z, Dos Santos Claro PC, Shevchenko EV, Huang L. Direct imaging of long-range exciton transport in quantum dot superlattices by ultrafast microscopy. ACS Nano. 2016;10:7208–7215. doi: 10.1021/acsnano.6b03700. [DOI] [PubMed] [Google Scholar]
- 26.Frederick MT, Weiss EA. Relaxation of exciton confinement in CdSe quantum dots by modification with a conjugated dithiocarbamate ligand. ACS Nano. 2010;4:3195–3200. doi: 10.1021/nn1007435. [DOI] [PubMed] [Google Scholar]
- 27.Frederick MT, Amin VA, Swenson NK, Ho AY, Weiss EA. Control of exciton confinement in quantum dot−organic complexes through energetic alignment of interfacial orbitals. Nano Lett. 2013;13:287–292. doi: 10.1021/nl304098e. [DOI] [PubMed] [Google Scholar]
- 28.Frederick MT, Amin VA, Weiss EA. Optical properties of strongly coupled quantum dot−ligand systems. J. Phys. Chem. Lett. 2013;4:634–640. doi: 10.1021/jz301905n. [DOI] [PubMed] [Google Scholar]
- 29.Azzaro MS, Dodin A, Zhang DY, Willard AP, Roberts ST. Exciton-delocalizing ligands can speed up energy migration in nanocrystal solids. Nano Lett. 2018;18:3259–3270. doi: 10.1021/acs.nanolett.8b01079. [DOI] [PubMed] [Google Scholar]
- 30.Reiss P, Blueuse J, Pron A. Highly luminescent CdSe/ZnSe core/shell nanocrystals of low size dispersion. Nano Lett. 2002;2:781–784. doi: 10.1021/nl025596y. [DOI] [Google Scholar]
- 31.Zhang H, et al. Hydrothermal synthesis for high-quality CdTe nanocrystals. Adv. Mater. 2003;15:1712–1715. doi: 10.1002/adma.200305653. [DOI] [Google Scholar]
- 32.Guo J, Yang W, Wang C. Systematic study of the photoluminescence dependence of thiol-capped CdTe nanocrystals on the reaction conditions. J. Phys. Chem. B. 2005;109:17467–17473. doi: 10.1021/jp044770z. [DOI] [PubMed] [Google Scholar]
- 33.Rogach AL, et al. Aqueous synthesis of thiol-capped CdTe nanocrystals: State-of-the-art. J. Phys. Chem. C. 2007;111:14628–14637. doi: 10.1021/jp072463y. [DOI] [Google Scholar]
- 34.Bu H-B, et al. Hydrothermal synthesis of thiol-capped CdTe nanoparticles and their optical properties. Phys. Chem. Chem. Phys. 2013;15:2903–2911. doi: 10.1039/c2cp43299d. [DOI] [PubMed] [Google Scholar]
- 35.Watanabe T, et al. Synthesis of Type-I CdTe core and Type-II CdTe/CdS core/Shell quantum dots by a hydrothermal method and their optical properties. Bull. Chem. Soc. Jpn. 2017;90:52–58. doi: 10.1246/bcsj.20160299. [DOI] [Google Scholar]
- 36.Lee Y-S, et al. Hydrothermal synthesis of NAC-capped II–VI semiconductor ZnSe quantum dots in acidic condition. Chem. Lett. 2016;45:878–880. doi: 10.1246/cl.160431. [DOI] [Google Scholar]
- 37.Jing L, et al. Aqueous based semiconductor nanocrystals. Chem. Rev. 2016;116:10623–10730. doi: 10.1021/acs.chemrev.6b00041. [DOI] [PubMed] [Google Scholar]
- 38.Decher G. Fuzzy nanoassemblies: toward layered polymeric multicomposites. Science. 1997;277:1232–1237. doi: 10.1126/science.277.5330.1232. [DOI] [Google Scholar]
- 39.Shavel A, Gaponik N, Eychmüller A. The assembling of semiconductor nanocrystals. Eur. J. Inorg. Chem. 2005;2005:3613–3623. doi: 10.1002/ejic.200500322. [DOI] [Google Scholar]
- 40.Kim D, Okahara S, Nakayama M, Shim Y. Experimental verification of Förster energy transfer between semiconductor quantum dots. Phys. Rev. B. 2008;78:153301. doi: 10.1103/PhysRevB.78.153301. [DOI] [Google Scholar]
- 41.Rodrigues PAM, Tamulaitis G, Yu PY, Risbud SH. Size selective photoluminescence excitation spectroscopy in CdSe nanocrystals. Solid State Commun. 1995;94:583. doi: 10.1016/0038-1098(95)00135-2. [DOI] [Google Scholar]
- 42.Norris DJ, Efros AL, Rosen M, Bawendi MG. Size dependence of exciton fine structure in CdSe quantum dots. Phys. Rev. B. 1996;53:16347. doi: 10.1103/PhysRevB.53.16347. [DOI] [PubMed] [Google Scholar]
- 43.Miyazaki J, Kinoshita S. Site-selective spectroscopic study on the dynamics of exciton hopping in an array of inhomogeneously broadened quantum dots. Phys. Rev. B. 2012;86:035303. doi: 10.1103/PhysRevB.86.035303. [DOI] [Google Scholar]
- 44.Kagan CR, Murray CB, Bawendi MG. Long-range resonance transfer of electronic excitations in close-packed CdSe quantum-dot solids. Phys. Rev. B. 1996;54:8633. doi: 10.1103/PhysRevB.54.8633. [DOI] [PubMed] [Google Scholar]
- 45.Kagan CR, Murray CB, Nirmal M, Bawendi MG. Electronic energy transfer in CdSe quantum dot solids. Phys. Rev. B. 1996;76:1517. doi: 10.1103/PhysRevLett.76.1517. [DOI] [PubMed] [Google Scholar]
- 46.Crooker SA, Hollingsworth JA, Tretiak S, Klimov VI. Spectrally resolved dynamics of energy transfer in quantum-dot assemblies: towards engineered energy frows in artificial materials. Phys. Rev. Lett. 2002;89:186802. doi: 10.1103/PhysRevLett.89.186802. [DOI] [PubMed] [Google Scholar]
- 47.Achermann M, Petruska MA, Crooker SA, Klimov VI. Picosecond energy transfer in quantum dot Langmuir–Blodgett nanoassemblies. J. Phys. Chem. B. 2003;107:13782–13787. doi: 10.1021/jp036497r. [DOI] [Google Scholar]
- 48.Citrin DS. Long intrinsic radiative lifetimes of excitons in quantum wires. Phys. Rev. Lett. 1992;69:3393. doi: 10.1103/PhysRevLett.69.3393. [DOI] [PubMed] [Google Scholar]
- 49.Akiyama H, et al. Thermalization effect on radiative decay of excitons in quantum wires. Phys. Rev. Lett. 1994;50:8930. doi: 10.1103/PhysRevLett.72.924. [DOI] [PubMed] [Google Scholar]
- 50.Takahashi A, Ueda T, Bessho Y, Harada Y, Kita T. One-dimensional miniband formation in closely stacked InAs/GaAs quantum dots. Phys. Rev. B. 2013;87:235323. doi: 10.1103/PhysRevB.87.235323. [DOI] [Google Scholar]
- 51.Kaizu T, Tajiri Y, Kita T. Wide-wavelength-range control of photoluminescence polarization in closely stacked InAs/GaAs quantum dots. J. Appl. Phys. 2019;125:234304. doi: 10.1063/1.5096411. [DOI] [Google Scholar]
- 52.Watanabe T, Takahashi K, Shimura K, Kim D. Influence of carrier localization at the core/shell interface on the temperature dependence of the Stokes shift and the photoluminescence decay time in CdTe/CdS type-II quantum dots. Phys. Rev. B. 2017;96:035305. doi: 10.1103/PhysRevB.96.035305. [DOI] [Google Scholar]
- 53.Chang I-Y, Kim D, Hyeon-Deuk K. Control of multiple exciton generation and electron−phonon coupling by interior nanospace in hyperstructured quantum dot superlattice. ACS Appl. Mater. Interfaces. 2017;9:32080–32088. doi: 10.1021/acsami.7b08137. [DOI] [PubMed] [Google Scholar]
- 54.Chang I-Y, Kim D, Hyeon-Deuk K. Correlated roles of temperature and dimensionality for multiple exciton generation and electronic structures in quantum dot superlattices. J. Phys. Chem. C. 2019;123:2549–2556. doi: 10.1021/acs.jpcc.8b10565. [DOI] [Google Scholar]
- 55.Yu WW, Wang A, Peng X. Formation and stability of size-, shape-, and structure-controlled CdTe nanocrystals: ligand effects on monomers and nanocrystals. Chem. Mater. 2003;15:4300–4308. doi: 10.1021/cm034729t. [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
The data that support the findings of this study are available from the corresponding author upon reasonable request.