Probing excited state ¹Hα chemical shifts in intrinsically disordered proteins with a triple resonance-based CEST experiment: Application to a disorder-to-order switch

Abstract

Over 40 % of eukaryotic proteomes and 15 % of bacterial proteomes are predicted to be intrinsically disordered based on their amino acid sequence. Intrinsically disordered proteins (IDPs) exist as heterogeneous ensembles of interconverting conformations and pose a challenge to the structure-function paradigm by apparently functioning without possessing stable structural elements. IDPs play a prominent role in biological processes involving extensive intermolecular interaction networks and their inherently dynamic nature facilitates their promiscuous interaction with multiple structurally diverse partner molecules. NMR spectroscopy has made pivotal contributions to our understanding of IDPs because of its unique ability to characterize heterogeneity at atomic resolution. NMR methods such as Chemical Exchange Saturation Transfer (CEST) and relaxation dispersion have enabled the detection of ‘invisible’ excited states in biomolecules which are transiently and sparsely populated, yet central for function. Here, we develop a ¹Hα CEST pulse sequence which overcomes the resonance overlap problem in the ¹Hα-¹³Cα plane of IDPs by taking advantage of the superior resolution in the ¹H-¹⁵N correlation spectrum. In this sequence, magnetization is transferred after ¹H CEST using a triple resonance coherence transfer pathway from ¹Hα (i) to ¹HN(i+1) during which the ¹⁵N(t₁) and ¹HN(t₂) are frequency labelled. This approach is integrated with spin state-selective CEST for eliminating spurious dips in CEST profiles resulting from dipolar cross-relaxation. We apply this sequence to determine the excited state ¹Hα chemical shifts of the intrinsically disordered DNA binding domain (CytR^N) of the bacterial cytidine repressor (CytR), which transiently acquires a functional globally folded conformation because of thermal fluctuations. The structure of the excited state, calculated using ¹Hα chemical shifts in conjunction with other excited state NMR restraints, is a three-helix bundle incorporating a helix-turn-helix motif that is vital for binding DNA.

1. Introduction

Intrinsically disordered proteins and protein regions (IDPs) are common in all living organisms and constitute over 40 % of the proteomes of eukaryotes (1). Despite lacking a stable three-dimensional structure, IDPs perform their function in a variety of different ways. Some IDPs fold in the presence of a binding partner (2–5) or upon post-translational modifications such as phosphorylation (6). Other IDPs participate in pathways where a unique structure is not required for function (5) and IDPs can remain disordered in their functional ligand-bound state (7). The amino acid sequences of IDPs are much simpler than those of their folded counterparts, being depleted in large hydrophobic aliphatic and aromatic amino acids like Leu, Ile, Val and Trp that promote order formation. Instead, IDP sequences are enriched in small polar and charged amino acids that include Ser, Gly, Arg and Glu which increase the tendency for disorder in polypeptide chains (8, 9). As a consequence, IDPs exist as structurally heterogeneous ensembles of interconverting conformations. The structural malleability of IDPs and their ability to switch rapidly between conformations enables them to interact with multiple structurally unrelated binding partners (10, 11) and IDPs frequently serve as scaffolds in biological processes such as signal transduction cascades (12, 13) and transcriptional regulation (14, 15). The conformational dynamics of IDPs is central for their function and characterizing the structures populated in the IDP ensemble is a vital step in understanding how IDPs function.

Nuclear magnetic resonance (NMR) spectroscopy is a powerful tool for probing the dynamics of biomolecules such as proteins, nucleic acids and glycans (16–18). The site-specificity afforded by NMR helps in constructing a coherent picture of conformational dynamics across the entire molecule of interest. A number of experiments such as Chemical Exchange and Dark-state Exchange Saturation Transfer (CEST (19–21) and DEST(22)), Carr-Purcell-Meiboom-Gill (CPMG) (23–25), R_1ρ Relaxation Dispersion (RD) (26, 27) and Paramagnetic Relaxation Enhancement (PRE) (28, 29) have recently been developed that can detect and structurally characterize transiently (τ_E > 25 μs) and sparsely (p_E > 0.5 %) populated biomolecular conformations (referred to as excited states (E) here). The application of these methods has revealed the importance of rare conformational transitions in protein folding (30–32), Hsp70 chaperone binding (33–35), light sensing by the LOV domain (36), enzyme catalysis by tyrosine phosphatases (37) and dihydrofolate reductase (38, 39), post-translational maturation of superoxide dismutase (40), DNA replication fidelity (41, 42) and transcription termination by the fluoride riboswitch (43), as well as in aberrant protein oligomerization (44, 45) and aggregation (46). However, these methods have found limited applicability in the domain of IDPs (47–50), primarily because IDPs are believed to have flat free energy landscapes devoid of kinetically separated excited state conformations (3, 49, 51).

We recently showed that the intrinsically disordered N-terminal DNA binding domain of the cytidine repressor (CytR), CytR^N (CytR(1–66)), is functionally regulated by a conformationally excited state (52). CytR belongs to the LacR family of bacterial transcriptional repressors and regulates the transcription of genes involved in ribonucleotide uptake and degradation (53–56). CytR^N has a high charge-to-hydrophobicity ratio as well as a large number of Ala and Lys residues, which cause it to be intrinsically disordered both in the free form and in full-length CytR (52). In the DNA-bound state, CytR^N folds into a stable three-helix bundle which interacts with DNA through a helix-turn-helix (HTH) motif formed from the first two helices (H1 and H2) (57). While the conformation of CytR^N in the DNA-bound state is very similar to that of its family members such as FruR^N and LacR^N (58, 59), the latter molecules are folded even in the absence of DNA (60–62). We used multinuclear ¹⁵N, ¹H^N, ¹³C’ and ¹³Cα CEST experiments to show that the disordered ensemble of CytR^N exists in equilibrium with a globally folded conformation that shares the same three-helix bundle topology found in the CytR^N-DNA complex (52). We obtained alpha carbon and carbonyl carbon chemical shifts of the excited state using previously reported triple resonance-based CEST experiments that were critical in defining the backbone fold of the CytR^N excited state (63, 64). However, ¹Hα excited state chemical shifts could not be obtained because the existing aliphatic ¹H-CEST pulse sequence relies on quantifying residue-specific peak intensities in the 2D ¹H-¹³C correlation spectrum (65), where there is severe overlap of resonances because of the disordered nature of native CytR^N (Figure 1A). The CPMG relaxation dispersion-based method for measuring ¹Hα excited state chemical shifts also suffers from the same overlap problem (66). This is a significant impediment in characterizing millisecond timescale IDP dynamics because ¹Hα chemical shifts are sensitive reporters of protein secondary structure (67, 68).

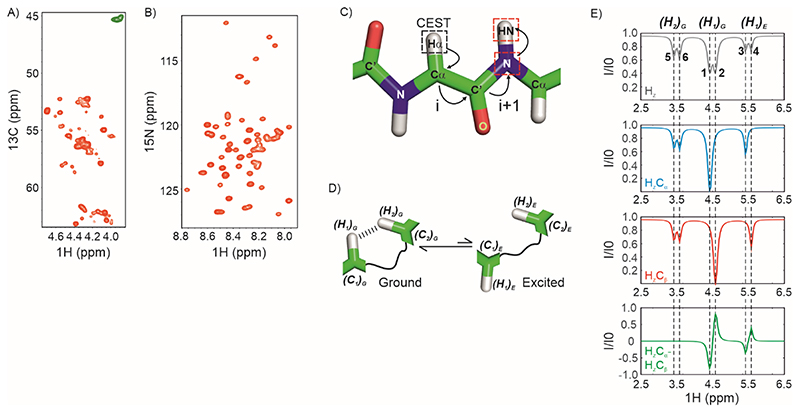
Figure 1. Strategy for acquiring ¹Hα CEST data on IDPs.

A) The ¹Hα region of a constant-time ¹H-¹³C HSQC spectrum of U-¹³C,¹⁵N labelled CytR^N. Green resonances arise from Gly which do not have a scalar coupled sidechain ¹³Cβ. B) ¹H-¹⁵N HSQC spectrum of CytR^N. C) The coherence transfer pathway in the ¹Hα CEST experiment reported here. CEST is carried out on ¹Hα (black dashed square) and the magnetization is then transferred from ¹Hα(i) through ¹³Cα(i) and ¹³CO(i) to ¹⁵N (i+1) and then to ¹HN(i+1) for detection. Red dashed squares indicate nuclei that are frequency labelled in the experiment. D) Cartoon representation of conformational exchange between ground and excited states of a protein where the alpha proton H₁ comes close (< 5 Å) to H₂ in the ground state. E) The spin-state-selective approach for acquiring proton CEST without interference from dipolar cross-relaxation. (Top) ¹H CEST profile of an alpha proton resonating at 4.5 ppm (major dip), exchanging with a second conformation where the chemical shift of the ¹Hα is 5.5 ppm (minor dip). The ¹Hα is proximal to a second ¹Hα which results in an NOE dip at 3.5 ppm. There is no ¹³C-decoupling during the CEST period; therefore doublets separated by ¹J_HαCα are seen for each dip. (Middle) Spin-state-selective CEST data selecting for H_zC_α (blue) and H_zC_β (red). (Bottom) CEST profile of H_zC_α-H_zC_β =2H_zC_z, where the NOE dip cancels out (green).

Herein, we report a ¹Hα CEST pulse sequence (HACACONNH-HA-CEST) that combines spin-state-selective ¹Hα CEST acquisition with triple resonance coherence transfer that can be used for determining excited state ¹Hα chemical shifts in folded as well as disordered proteins. We validate this experiment on the L24A variant of the FF domain, in which exchange between the folded state and an on-pathway folding intermediate has been extensively characterized in literature (31). We then demonstrate the applicability of this sequence for IDPs by using it to measure ¹Hα chemical shifts of 37 residues in natively disordered CytR^N. The measurements reinforce the conclusion that disordered CytR^N undergoes a disorder-to-order transition to an excited state structure composed of three helices and containing a helix-turn-helix motif.

2. Results and discussion

2.1. Designing the triple resonance ¹Hα CEST pulse sequence

The Chemical Exchange Saturation Transfer (CEST) experiment consists of the application of a weak radiofrequency (RF) field of amplitude B₁ at a specific chemical shift offset of the nucleus of interest (¹Hα in our case) for an exchange duration T_ex (69). Longitudinal magnetization (19) or two-spin-order (34) is present at the beginning of the exchange period and the density operator of interest at the end of T_ex is quantified through peak intensities in suitable 2D correlation spectra. The RF field is swept across the offset range for the nucleus under study and an array of 2D spectra, one for each offset value, is generated in the CEST experiment. The per-residue CEST profile is a plot of the normalized intensity (I/I₀) of the corresponding peak in the 2D spectrum as a function of the offset at which RF irradiation occurs. For a protein in exchange between a major ground state and a minor excited state, the presence of exchange in CEST profiles is visualized as a second minor dip in intensity besides the major saturation dip that occurs at the chemical shift of the nucleus in the excited state.

The chemical exchange saturation transfer (CEST) pulse sequence that is currently used for obtaining excited state aliphatic proton chemical shifts in proteins relies on quantifying peak intensities in a ¹H-¹³C correlation spectrum (65). Figure 1A shows the ¹Hα-¹³Cα region of the HSQC spectrum of the intrinsically disordered DNA binding domain of the Escherichia coli cytidine repressor (CytR^N). There is a lot of resonance overlap in this spectrum, which makes it impossible to reliably extract intensities for many of the ¹Hα-¹³Cα correlations. In addition, it can be challenging to obtain the intensities of ¹Hα-¹³Cα correlations because the ¹Hα resonances in IDPs cluster near the water resonance. In contrast, resonances are much better resolved in the ¹H-¹⁵N HSQC spectrum of CytR^N (Figure 1B), making this 2D plane an attractive candidate for detecting intensity modulations from CEST.

In order to take advantage of the superior resolution in the ¹H-¹⁵N 2D plane, our approach for determining excited state ¹Hα chemical shifts involves carrying out CEST on ¹Hα at the beginning of the pulse sequence and then transferring the magnetization from ¹Hα of the i^th residue to the ¹HN of the i+1 residue through ¹³Cα(i), ¹³CO(i) and ¹⁵N(i+1) using one-bond scalar couplings (Figure 1C). The ¹H CEST profile recorded by directly ‘saturating’ the ¹H resonances as described earlier will often contain additional dips due to ¹H-¹H dipolar interactions (NOEs) with other proximal ¹H spins (70, 71). As these additional dips can mask the presence of a minor state dip and can also be accidentally assigned to a minor state resonance it is imperative to record ¹H CEST profiles that do not contain these spurious dips. Two approaches that exploit the presence of the attached X-nuclei (¹³C in this case) have been devised to eliminate the NOE induced spurious dips. The first involves carrying X-spin CEST experiments with weak ¹H CW decoupling to indirectly detect the minor state ¹H resonance positions (70), while the second involves carrying out (X-) spin-state-selective ¹H CEST experiments (71). Here we have adopted the spin-state-selective approach to eliminate spurious NOE dips from ¹Hα CEST profiles.

Consider a ¹Hα nucleus (H₁) exchanging between two environments corresponding to the ground (G) and excited states of a protein (E) (Figure 1D). In addition, H₁ is dipolar coupled to a proton H₂ which is in close spatial proximity (< ~5 Å) to H₁ in the ground state. H₁ and H₂ are in-turn scalar coupled to their respective covalently bonded carbons C₁ and C₂. The top panel of Figure 1E (grey) shows the conventional ¹Hα CEST profile for this system quantifying the intensity of H_1Z in the ground state at the end of T_ex. The major dip from the ground state and the minor dip from the excited state are centred at the chemical shifts of (H₁)_G (4.5 ppm) and (H₁)_E (5.6 ppm) respectively. Each dip is split by the one-bond scalar coupling of H₁ to C₁ (¹J_C1H1) because there is no ¹³C decoupling during T_ex; i.e., the major dip splits into dips 1 and 2, while the minor dip splits into dips 3 and 4. The dip arising due to H₂ centred at 3.5 ppm is also split into dips 5 and 6 due to the scalar coupling between H₂ and C₂. Separating the CEST profiles based on the spin-state (α or β) of (C₁)_G (blue (α) and red (β) in Figure 1E) results in profiles that contain a single dip arising due to H₁ rather than a doublet (dips 2 and 4 for the α spin-state and dips 1 and 3 for the β spin-state). Dips arising due to H₂ remain doublets as they arise due to the ¹J_C2H2 coupling (i.e. the spin-state of C₂) rather than the ¹J_C1H1 coupling. As the dips arising due to H₁ are displaced by ¹J_C1H1 between the two spin-state-selective CEST profiles, subtracting the two profiles will result in antiphase features at the major and minor state H₁ shifts while dips 5 and 6 arising due to H₂ are subtracted out (Figure 1E, green profile). Following the approach detailed in Yuwen et al. (65), this subtraction is done ‘in-line’ in the HACACONNH-HA-CEST pulse sequence by selecting the 2H_ZC_Z operator at the end of T_ex since 2H_ZC_Z = H_zC^α – H_ZC^β. This improves the sensitivity of the overall experiment by a factor of $\sqrt{2}$ compared to acquiring separate CEST profiles for ${(H_{1z}{C}_1^{\alpha })}_G$ and ${(H_{1z}{C}_1^{\beta })}_G$ and subtracting them post-acquisition.

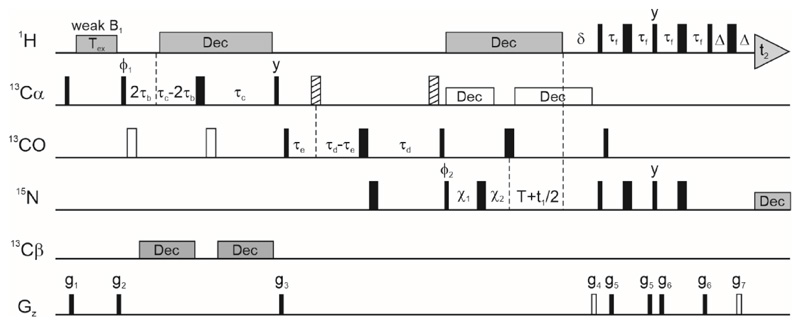
Figure 2 shows the HACACONNH-HA-CEST pulse sequence, in which CEST on ¹Hα is carried out at the beginning of the pulse train and ¹Hα magnetization is aligned along the z-axis at the beginning of the CEST element. The 2H_zC_z operator is then selected at the end of T_ex and magnetization is transferred to the (i+1)^th amide proton using a standard coherence pathway (63, 72–74) taking advantage of scalar couplings ¹J_CαHα, ¹J_CαCO, ¹J_CON and ¹J_NH in succession. The amide nitrogen and amide proton of the (i+1)^th residue are frequency labelled in the indirect and direct dimensions respectively to extract CEST profiles of the i^{th 1}Hα nucleus using the high resolution ¹H-¹⁵N 2D plane. The weak B₁ field (usually 5-50 Hz) is swept through the range of ¹Hα chemical shift offsets (typically 2.5 – 6 ppm) and one 2D plane is collected for each offset. The spacing between offsets is approximately the same as the amplitude of the weak RF field (in Hz), though it has been shown recently that significantly larger spacings can be employed without impairing the robustness of the exchange parameters extracted from the CEST data (75). A reference plane is collected for each CEST dataset using a modified version of the pulse sequence lacking the exchange duration (T_ex = 0), in which an INEPT pulse train replaces the CEST module for transferring H_z to 2H_zC_z. Peak intensities in this reference plane (I₀) are used to generate the normalized CEST profile.

Figure 2. The pulse sequence for acquiring HACACONNH-based ¹Hα CEST data.

The ¹H carrier is centred on the water resonance except during the CEST period and the ¹⁵N carrier at ~ 118 ppm, while the ¹³C carrier is placed at 58 ppm till gradient g3 and then jumped to 176 ppm for the rest of the pulse sequence. All pulses are applied along the x-axis unless specified otherwise. Narrow and wide rectangles represent 90° and 180° pulses respectively. Open rectangles correspond to rectangular pulses applied 118 ppm off-resonance while the hashed rectangles are rectangular pulses applied -118 ppm off-resonance. ¹H and ¹⁵N pulses are applied at the highest power while ¹³C 90° and 180° pulses are applied at RF field amplitudes of $\xi /\sqrt{15}$ and $\xi /\sqrt{3}$ Hz respectively, where ξ is the frequency difference in Hz between the ¹³Cα and ¹³CO spectral bands (90). ¹H decoupling is implemented via a ~6 kHz WALTZ-16 (91) composite pulse decoupling pattern. Off-resonance ¹³Cα decoupling is carried out with a cosine modulated SEDUCE-1 pattern (92, 93) with a pulse width of 315 μs (at 600 MHz B₀ field strength), and ¹⁵N decoupling during acquisition is carried out with a ~ 1 kHz WALTZ-16 pattern. WURST-2 adiabatic decoupling (94) is used for ¹³Cβ decoupling in the 16.5-41.5 ppm and 68-72 ppm bands. The phase cycling used in the sequence is: ϕ₁ = (-y,y), ϕ₂ = (x,x,-x,-x) and receiver = (x,-x,-x,x). The delays used in the experiment are: τ_b = 1.8 ms, τ_c = 4.4 ms, τ_d = 12.4 ms, τ_e = 5 ms, τ_f = 2.3 ms, T = 12.4 ms, δ = 5.5 ms and Δ = 0.5 ms. The amplitudes and durations of the various gradients in (G/cm, ms) are: g₁: (12000, 0.5), g₂: (-8343, 0.5), g₃: (10000, 0.8), g₄: (30000, 1.25), g₅: (4000, 0.3), g₆: (2000, 0.4), g₇: (30400, 0.125). The two gradients g₄ and g₇ represented as open rectangles are required for coherence selection. Delays χ₁ and χ₂ are set to the larger of the two values (T-t₁/2, 0) and (0, t₁/2-T) respectively, and this allows ¹⁵N chemical shift evolution in t₁ to occur in a semi-constant manner (72, 95). Quadrature detection in the F1 dimension is implemented using enhanced sensitivity gradient coherence selection (96–98).

Protein CEST profiles on low-γ ¹³C and ¹⁵N nuclei are generally acquired by co-adding scans where the starting magnetization is along the +z and -z directions. This ensures that magnetization decays exponentially to 0 rather than to its equilibrium value of M₀, enabling an accurate ‘in situ’ measurement of R₁ and eliminating the dependence of the CEST profile on equilibrium magnetization, thereby reducing one fitting variable. It has been previously shown that such an approach is not optimal for ¹H CEST and results in smaller dips than if magnetization decays to M₀ (76). Therefore, ¹Hα magnetization is always aligned along the +z axis at the start of T_ex in the current pulse sequence (Figure 2); residue-specific ¹Hα longitudinal relaxation rate constants (R_1H) are separately estimated by varying T_ex using an altered version of the pulse sequence in which an INEPT module is inserted after T_ex to transfer magnetization from H_z to 2H_zC_z, and no B₁ field is applied during T_ex in this experiment.

2.2. Testing the sequence on a system without measurable millisecond timescale exchange: ubiquitin:

We first tested the HACACONNH-HA-CEST pulse sequence on the folded protein (Figure 3A) ubiquitin that lacks measurable conformational exchange in the millisecond timescale. The signal-to-noise (S/N) of a reference 2D ¹H-¹⁵N plane from the HACACONNH-HA-CEST experiment for ubiquitin (averaged over 50 isolated peaks) is 1.6 ± 0.5 – fold higher than a constant-time 2D ¹³C-¹H correlation spectrum (averaged over 31 isolated peaks separated by at least 0.4 ppm from water). This is probably because magnetization evolves on the faster relaxing ¹³Cα nucleus during the 29.4 ms constant time period in the ¹³C-¹H HSQC, compared to evolution for 24.8 ms on the slower relaxing ¹⁵N nucleus in the semi-constant period of the HACACONNH-HA-CEST sequence. In addition, the HACACONNH-HA-CEST sequence incorporates a gradient sensitivity enhancement module that improves sensitivity by a factor of $\sqrt{2}.$ The sensitivity of the ¹Hα-¹³Cα 2D correlation map can be further increased by decoupling ¹³Cβ nuclei with adiabatic decoupling schemes and using a non-constant-time evolution element, as well as by including the sensitivity enhancement scheme for the ¹H-¹³C AX spin pair (77). Nevertheless, it is apparent that the sensitivity disadvantage in using the HACACONNH-HA-CEST experiment is not significant especially for intrinsically disordered proteins that have smaller transverse relaxation rates compared to ordered proteins of the same mass.

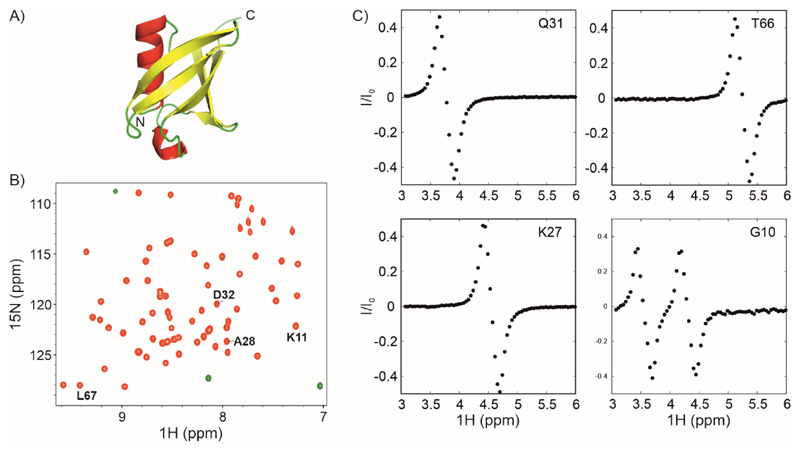
Figure 3. ¹Hα CEST on U-¹³C,¹⁵N labelled ubiquitin.

A) Three-dimensional structure of ubiquitin showing helices, sheets and loops in red, yellow and green respectively (PDB ID: 1UBQ (99)). B) ¹H-¹⁵N HSQC spectrum of ubiquitin. C) ¹Hα CEST profiles of selected residues in ubiquitin that were obtained by analyzing the i+1 correlations indicated in the HSQC spectrum in panel B. ¹Hα CEST data were acquired using the pulse sequence in Figure 2. The ¹Hα CEST profile for Gly10 shows two dips arising from each of the two diastereotopic ¹Hα protons of Gly10.

Figure 3B shows the ¹H-¹⁵N HSQC spectrum of ubiquitin and Figure 3C shows the ¹Hα CEST profiles obtained using the HACACONNH-HA-CEST pulse sequence by quantifying the intensity ¹H-¹⁵N correlations of selected residues as a function of chemical shift offset. The ¹Hα CEST profiles of K27, Q31 and T66 appear like the difference in two Lorentzians separated by ¹J_CαHα, analogous to the spectrum of the antiphase operator 2H_zC_z. We refer to this as the ‘major dip’ in correspondence with the in-phase major dip in ¹⁵N or ¹³C CEST profiles. While the maximum of the major dip occurs at the chemical shift offset of the TROSY component of the ¹Hα-¹³Cα doublet, the minimum occurs at the chemical shift offset of the anti-TROSY component. The downfield component has negative intensity and the upfield component has positive intensity, unlike in Figure 1E, because the reference and CEST planes are phased opposite to each other in this sequence, with 2H_zN_z detected in the reference plane and -2H_zN_z in the CEST planes. Notably, there are no NOEs observable in these three ¹Hα CEST profiles, confirming that the effect of dipolar cross-relaxation has been suppressed in this pulse sequence.

The ¹Hα CEST profile of G10 (Figure 3C, bottom right) shows the presence of two major dips in intensity at the chemical shifts of the two diastereotopic ¹Hα1 and ¹Hα2 of G10. Saturation transfer carried out on both ¹Hα atoms transfer to the same ¹HN of K11 and both major dips are observed in the same CEST profile. Therefore, this pulse sequence cannot be used to obtain excited state ¹Hα chemical shifts of Gly residues (78).

2.3. Validating the sequence on the L24A FF domain to characterize N-I exchange

Next, we tested the HACACONNH-HA-CEST pulse sequence on the L24A variant of the FF domain, which is known to exchange between its folded native state (N) and a partially folded on-pathway folding intermediate (I) that has a lifetime of 1.8 ms and a population of 5.4 % (303 K) (Figure 4A, (31, 79)). Since the exchange rate constant between these two states is ~ 580 s^-1, N↔I exchange can be conveniently studied with CEST. In addition, there is substantial conformational rearrangement in going from N, which is a four-helix bundle, to I, in which the third helix is longer than in N and the fourth helix is disordered (Figure 4A). A number of residues in H3 and H4 are therefore expected to show large ¹Hα chemical shift changes, furnishing a convenient testing system for the HACACONNH-HA-CEST pulse sequence.

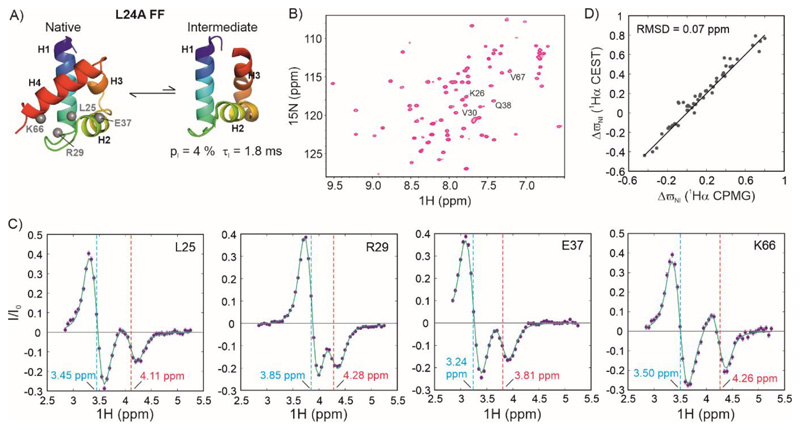
Figure 4. Characterizing exchange in the folded L24A FF domain using ¹Hα CEST.

A) Cartoon representation of the native (31) and intermediate states of L24A FF (PDB ID:2L9V (31)) in exchange on the millisecond timescale. B) ¹H-¹⁵N HSQC spectrum of L24A FF. Resonances whose ¹Hα CEST profiles are quantified in panel C are labelled in the spectrum. C) ¹Hα CEST profiles of selected resonances of L24A FF. Cyan and red dashed lines indicate the chemical shifts of the native and intermediate states respectively. D) Correlation between the ¹Hα chemical shifts of L24A FF intermediate states acquired using ¹Hα CPMG (y-axis, (31)) and ¹Hα CEST (x-axis). The solid line depicts the y=x function.

Figure 4B shows the 2D ¹H-¹⁵N correlation spectrum of L24A FF and Figure 4C shows ¹Hα CEST profiles of four residues L25, R29, E37 and K66 with large (> 0.4 ppm) chemical shift changes between N and I. In addition to the major dip in each profile, there is a minor dip consisting of two Lorentzians of opposite signs separated by ¹J_CαHα,E (the J-coupling in the excited state) that arises due to conformational exchange between N and I. Since there is partial overlap between the TROSY and anti-TROSY components of the major and minor dips, all four extrema cannot be visualized separately in these CEST profiles.

In order to extract exchange parameters from the ¹Hα CEST profiles, we fit profiles of 22 residues with |Δϖ_IN| > 0.35 ppm acquired at 3 B₁ field strengths each (15 Hz, 33 Hz and 54 Hz) globally to a two-state model of conformational exchange using the Bloch-McConnell equations (80). The fitting routine results in parameters of k_ex,IN = 582 ± 6 s^-1 and p_I = 4.24 ± 0.04 %. While k_ex,IN matches very well with the previously reported value (580 s^-1), p_I is significantly lower (5.4 %). This is likely because of the impact of cross-relaxation on the CEST profiles (71). It is therefore preferable to measure k_ex and p_E from ¹⁵N CEST experiments and use ¹Hα CEST data solely for determining excited state ¹Hα chemical shifts.

As the next step, we carried out residue-specific fits of ¹Hα CEST profiles of 50 residues to extract chemical shift differences between the native and intermediate states. During this step, k_ex,IN and p_I were fixed to the value obtained from the global analysis of ¹Hα CEST data (see Materials and Methods). Figure 4D shows a comparison of these Δϖ_IN values with those determined using CPMG relaxation dispersion methods (31). There is very good agreement between the two measurements, with an RMSD over 50 residues of 0.07 ppm, confirming the robustness of the HACACONNH-HA-CEST pulse sequence.

2.4. Measuring ¹Hα chemical shifts of the excited state of intrinsically disordered CytR^N

Having established the reliability of the HACACONNH-HA-CEST pulse sequence for measuring excited state ¹Hα chemical shifts, we applied the methodology to the disorder-to-order transition in CytR^N (Figure 5A). The native state of CytR^N is intrinsically disordered, as seen from both sequence-based metrics such as the amino acid composition, charge and hydropathy, as well as from circular dichroism and NMR spectroscopy. We recently showed (52, 81) that CytR^N transiently populates a higher free energy state in which it adopts a globally folded three-helix bundle structure that resembles the conformation of the DNA-bound protein. We also showed that the folded conformation is responsible for binding DNA and that there is no measurable reactive flux directly from the native disordered state to DNA-bound CytR^N (52).

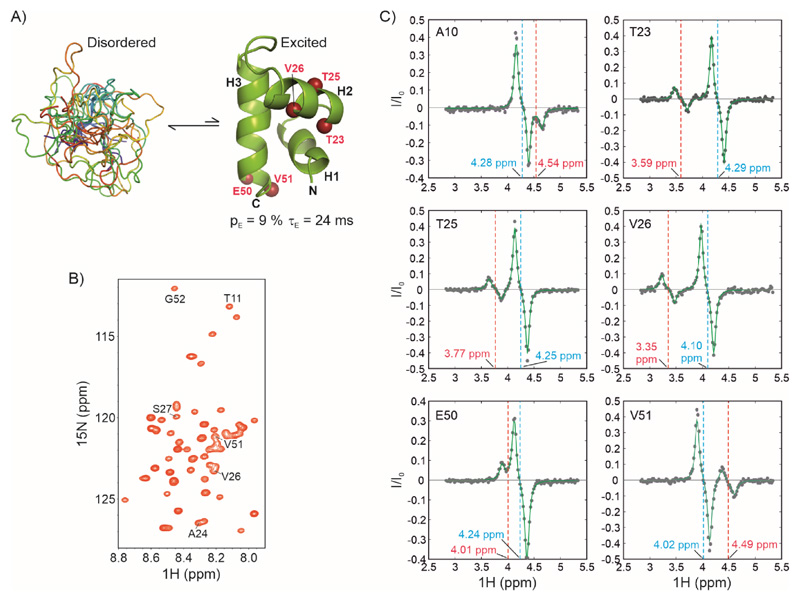
Figure 5. Characterizing exchange in the IDP CytR^N using ¹Hα CEST.

A) CytR^N exchanges between a disordered ensemble and a three-helix bundle folded state (52). B) ¹H-¹⁵N HSQC spectrum of disordered CytR^N showing limited spectral dispersion characteristic of an IDP. Resonances for which ¹Hα CEST profiles are quantified in panel C are labelled on the spectrum (except A10, which is not a part of the fragment included in the structure calculation). C) ¹Hα CEST profiles of selected residues of CytR^N. The chemical shift positions of the ground (disordered) and excited (folded) states are indicated by cyan and red dashed lines respectively.

The structure of the globally folded excited state of CytR^N was calculated using backbone ¹HN, ¹⁵N, ¹³Cα and ¹³C’ chemical shifts derived from CEST experiments. ¹³Cα and ¹³C’ chemical shifts were determined using previously published triple resonance schemes (63, 64). In order to obtain ¹Hα chemical shifts in the folded state of CytR^N, we acquired HACACONNH-HA-CEST data at two B₁ fields (13 Hz and 36 Hz, see Materials and Methods) on U-¹³C,¹⁵N labelled CytR^N at 288 K. The folded conformation of CytR^N in exchange with the intrinsically disordered form can be clearly discerned in ¹Hα CEST profiles as a minor dip in I/I₀, resulting in four extrema (two from the major dip and two from the minor dip) in CEST profiles of T23, T25, V26 and V51 (Figure 5C). ¹Hα chemical shifts for 37 non-overlapped residues (Table S1) were extracted using residue-wise fits of ¹Hα CEST profiles to the two-state Bloch-McConnell equations. Due to overlap in the ¹³C-¹H plane, minor state ¹Hα chemical shifts from only 18 sites are independently quantifiable using the ¹H-¹³C based experiment illustrating the efficacy of the HACACONNH-HA-CEST experiment described here. The ¹Hα chemical shift differences between folded and disordered CytR^N are substantial and vary between -0.79 and 0.58 ppm. The large changes occurring in these shifts as a result of the helix-coil transition in CytR^N underscores the importance of the ¹Hα nucleus in determining the structures of locally or globally folded conformations transiently sampled by IDPs.

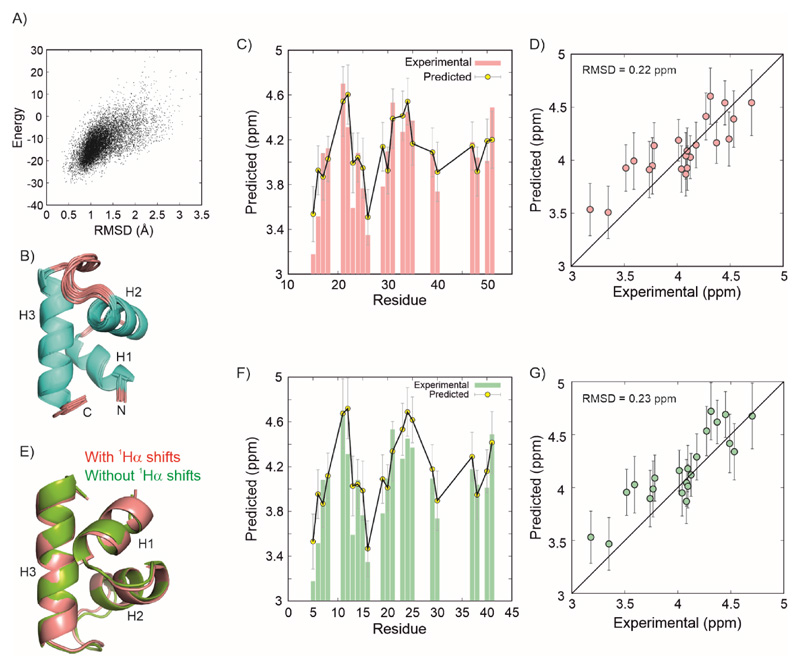
Finally, we assembled 23 ¹Hα shifts belonging to the structured region of the CytR^N excited state (CytR^N(11–53)) along with 33 ¹HN, 34 ¹⁵N, 25 ¹³Cα and 26 ¹³C’ shifts, as well as 31 ¹H-¹⁵N RDCs from alignment in C₈E₅/octanol (PEG) and 34 from a 6 % stretched polyacrylamide gel (PAG) to calculate the structure of the excited state using CS-Rosetta (82, 83), as described earlier (52). The structure calculation converges smoothly with a well-defined funnel (Figure 6A) in the energy vs RMSD (to the lowest energy structure) plot and Figure 6B shows the ensemble of 10 lowest energy structures. The structures closely resemble each other with a pairwise all-atom RMSD of 0.97 Å (Cα RMSD = 0.43 Å) and confirm that the excited state adopts a three-helix bundle conformation. Residue-specific ¹Hα chemical shifts predicted using Sparta+ (84) for the lowest energy structure match very well with the experimental values (Figure 6C) and the overall RMSD between the predicted and observed chemical shifts is 0.22 ppm (over 22 residues for which predictions are available (Figure 6C, 6D)), well within the prediction accuracy of Sparta+ (0.25 ppm for ¹Hα) (84). The structures calculated in the absence and presence of ¹Hα chemical shifts (Figure 6E) agree well with each other (RMSD = 0.6 Å over 41 Cα atoms) and have the same helix boundaries for H1 (M12-A19), H2 (T23-M31) and H3 (Q38-V51). The only difference between the two structures is in the small orientations of the H1-H2 and H2-H3 loops. Indeed, the ¹Hα chemical shifts predicted by Sparta+ from the CS-Rosetta structure calculated without input ¹Hα chemical shifts agree well with the experimental chemical shifts (RMSD = 0.23 ppm over 22 residues, Figure 6F,6G), providing an independent validation of the proposed excited state structure of CytR^N. The ¹Hα chemical shifts therefore reinforce the conclusion that CytR^N undergoes a disorder-to-order switch from its native disordered form to a three-helix bundle conformation compatible with DNA binding. The ¹Hα measurements also emphasize the fact that the heterogeneity in the structural ensemble of an IDP can include globally folded conformations with stable secondary and tertiary structure.

Figure 6. Structure of the CytR^N excited state calculated chemical shifts and RDCs.

A) Composite chemical shift and Rosetta energy score of structures plotted against the RMSD of each structure to the lowest energy structure. B) Overlay of the cartoon representations of the 10 structures with the lowest energy. Comparison between the experimental (red bars) and predicted ¹Hα chemical shifts (yellow circles) for the CytR^N excited state shown as a function of residues (C) and as a correlation plot (D). Predictions were done using Sparta+ on the lowest energy structure. Error bars are uncertainties in residue-specific chemical shift predictions from Sparta+. E) Overlay of the CytR^N excited state calculated without (green) and with (red) ¹Hα chemical shifts. F,G) A comparison of the same experimental data as in panels (C) and (D) with predictions made using Sparta+ (84) on the lowest energy structure calculated without using input ¹Hα chemical shifts (52).

3. Conclusions

Intrinsically disordered proteins are structurally malleable polymers in which dynamics is central for function. Here, we report the HACACONNH-HA-CEST NMR pulse sequence tailored towards characterizing millisecond dynamics in IDPs that facilitates the measurement of ¹Hα chemical shifts in IDP excited states. In this sequence, we combine spin state-selective ¹Hα CEST with triple resonance coherence transfer to the neighbouring ¹HN in order to take advantage the superior resolution in the ¹H-¹⁵N 2D available for IDPs. This sequence adds to the rapidly growing toolbox of NMR methods for elucidating the structure of transiently and sparsely populated protein conformations (excited states) and pinpointing their importance for function and malfunction.

In contrast to the CPMG-based approach for determining excited state ¹Hα chemical shifts (66), a specific isotope labelling scheme is not required for measuring HACACONNH-HA-CEST data and the sequence can applied on the same U-¹³C,¹⁵N labelled samples used for acquiring ¹³Cα, ¹³Cβ and ¹³CO CEST. This makes the CEST-based strategy very attractive as it allows us to obtain excited state chemical shifts for all protein backbone nuclei of folded and disordered proteins from a single sample. While CPMG experiments generally require lesser time than CEST, CEST data acquisition can be speeded up using multifrequency irradiation schemes such as DANTE-CEST (85). In addition, the CEST approach automatically provides the sign of the chemical shift difference between the ground and excited states unlike CPMG measurements, where additional experiments must be acquired in order to extract sign information.

4. Materials and methods

4.1. Overexpression and purification of proteins

All proteins were expressed by transforming plasmids containing the gene of interest in BL21(DE3) cells. Ubiquitin was cloned into multiple cloning site of a pET-15b vector while CytR^N and L24A FF were cloned into the pET-29b+ vector. Protein expression was carried out in 500 mL 2xM9 minimal media (86) containing the appropriate selection antibiotic. The media was supplemented with 1g/L ¹⁵N labelled ammonium chloride and 3g/L U-¹³C-labelled glucose as the sole nitrogen and carbon sources respectively. Protein expression was induced by adding 1 mM IPTG to the media when the absorbance at 600 nm (OD) reached 0.8-1 for all proteins. Induction was done at 18-20 °C for all proteins typically for 18 hours. Cells were harvested by centrifugation at 7500 rpm for 20 minutes and lysed by sonication in lysis buffer. The purification protocol followed for each protein is described briefly below.

4.1.1. Ubiquitin

Cells were lysed in 50 mM Tris HCl buffer pH 7.6 containing 1 mM EDTA and 0.05% Triton-X100 by sonication followed by incubation at room temperature with 1 mM phenyl methyl sulfonyl fluoride (PMSF) and 7 mg lysozyme per gram of the cell pellet. The lysate was clarified by centrifugation at 15000 rpm for 1 hr and glacial acetic acid was added dropwise to the clear supernatant while stirring until the solution turned milky white. The lysate was stirred for 30 minutes in room temperature and precipitated bacterial proteins were pelleted down by centrifugation for 30 minutes at 15000 rpm. The supernatant was dialyzed twice against 50 mM sodium acetate pH 4.5 at 4 °C. Dialyzed fractions were applied onto a SP-Sepharose cation exchange column with the dialysis buffer for column equilibration and wash, and the same buffer with 0.5 M NaCl (Buffer B) for linear gradient elution. Ubiquitin eluted at ~50 % Buffer B concentration. Elution fractions containing ubiquitin were purified further using gel filtration chromatography on a 120 mL Superdex75 column from which ubiquitin eluted between 82-90 mL. NMR data was acquired in Tris -HCl pH 7.6, 1 mM EDTA, 25 mM NaCl and 10 % D₂O.

4.1.2. CytR^N

Cells were lysed as described for ubiquitin above and the supernatant after cell lysis was made up to 60 % of the saturation value of ammonium sulphate to precipitate bacterial proteins. The precipitated proteins were removed by centrifugation and the supernatant was dialyzed into 50 mM sodium phosphate buffer containing 30 mM NaCl and 1 mM EDTA (lysis and equilibration buffer) before being applied to a SP-Sepharose cation exchange column. The equilibration buffer containing 1 M NaCl was used for elution (Buffer B). CytR^N eluted at ~60 % Buffer B concentration. Fractions containing CytR^N were pooled and further purified by gel filtration chromatography on a 120 mL Superdex 75 size exclusion column equilibrated in 150 mM ammonium acetate, from which CytR^N eluted at ~70 mL. Pure fractions were checked on SDS-PAGE, pooled and lyophilized. NMR data were acquired in buffer containing 20 mM sodium phosphate buffer (pH 7.0) and 10 % D₂O.

4.1.3. L24A FF domain

Cells expressing L24A FF were lysed as described for ubiquitin above. Purification was done using cation exchange chromatography on an SP-Sepharose column as mentioned previously for ubiquitin. The lysis buffer was 50 mM sodium phosphate buffer (pH 5.7) containing 1 mM EDTA, and the same buffer for used for column equilibration. Elution was done with the equilibration buffer containing 500 mM NaCl (Buffer B). L24A FF eluted at ~60 % Buffer B concentration; fractions containing protein were further purified on a 120 mL Superdex 75 column, from which L24A FF eluted at a volume of ~83 mL. NMR samples were prepared in 50 mM sodium acetate buffer pH 5.7, 50 mM NaCl, 1 mM EDTA and 10 % D₂O.

4.2. NMR spectroscopy: data acquisition and processing

All NMR experiments were carried out on an Agilent DD2 14.1 T (600 MHz ¹H Larmor frequency) spectrometer equipped with a room-temperature (L24A FF) or cryogenically cooled (ubiquitin and CytR^N) triple resonance single-axis gradient probe. Measurements on ubiquitin, L24A FF and CytR^N were done at 298 K, 303 K and 288 K respectively. All NMR datasets were processed with nmrPipe (87) and visualized with nmrDraw (87) and NMRFAM-Sparky (88).

4.3. ¹Hα CEST

All ¹Hα CEST data were acquired in an interleaved pseudo-3D manner with the pulse sequence shown in Figure 2. Each dataset contained a reference plane measured as described in Section 2.1 for obtaining normalizing CEST profiles. Peak intensities (I) in each plane of the CEST dataset were extracted by global lineshape analysis using FuDA and plotted as a ratio with the intensity in the reference plane (I₀) against the chemical shift offset for that particular plane to generate the CEST profile. Uncertainties in the I/I₀ values for each CEST profile were calculated using the scatter in the CEST baselines.

In order to extract Δϖ_GE values, ¹Hα CEST profiles were fit globally or residue-wise to the two-state Bloch-McConnell equations as described earlier using the software package ChemEx (https://github.com/gbouvignies/ChemEx), which numerically integrates the Bloch-McConnell equations (80). The radiofrequency field used in each ¹Hα CEST experiment was calibrated using the method of Guennuegues et al (89). ¹Hα R_1H values were measured using a modified version of the HACACONNH-HA-CEST pulse sequence as described in the Section 2.1. Nine delays (including two additional repeat points for error analysis) ranging from 1 to 350 ms were used and intensities were fit to a single exponential decay equation as a function of the delay to extract R_1H. ¹Hα R_1H values were then input into the fitting routine for determining Δϖ_GE and were not allowed to vary during the fit. It is not essential to measure R_1H in order to extract Δϖ_GE; fixing R_1H to a reasonable value (2 s^-1) results in comparable fit quality and very similar Δϖ_GE for CytR^N (maximum deviation of 18 ppb and average deviation of 2.4 ppb over 37 residues).

4.3.1. Ubiquitin

¹Hα CEST profiles were acquired at a B₁ field of 28 Hz over a chemical shift range of 3.1 to 6.1 ppm (50 planes) for an exchange duration (T_ex) of 250 ms.

4.3.2. L24A FF

¹Hα CEST profiles were acquired at B1 fields of 15 Hz (15 Hz spacing, 98 planes), 33 Hz (30 Hz spacing, 50 planes) and 54 Hz (50 Hz spacing, 34 planes) over a chemical shift range of 2.85 to 5.25 ppm (15 Hz and 33 Hz) or 2.71 to 5.38 ppm (54 Hz) for an exchange duration (T_ex) of 400 ms. Three B₁ field ¹Hα CEST profiles for 22 residues (T13, A17, K18, F21, L25, R29, S35, W36, E37, A39, M40, K41, I43, I44, Y49, A51, L55, S56, K58, K59, N63 and K66) with |Δϖ_IN| > 0.35 ppm were globally fit to the two-state Bloch-McConnell equations to extract k_ex,IN (582 ± 6 s^-1) and p_I (4.24 ± 0.04 %). The ground state in-phase ¹Hα transverse relaxation rate constant for the operator H_± (R_2,N) was allowed to vary, while ΔR₂ = R_2,I – R_2,N was fixed to 0 to enforce the condition that the excited and ground states have the same transverse relaxation rate constants. Here, R_2,I is the in-phase transverse relaxation rate constant for the excited state I. Antiphase transverse relaxation rate constants (2Hα_±Cα_z) of ground and excited state ¹Hα were calculated as R_i(2H_±C_z) = R_2,i – R_1,i, while the relaxation rate constant of longitudinal two-spin order 2H_ZC_Z was calculated as R_i(2H_zC_z) = R_1C,i – R_1H,i. The longitudinal and transverse cross-correlated relaxation rate constants η_z and η_xy are also varied during the fit within the constraints η_z,G = η_z,E and η_xy,G = η_xy,G. η_z accounts for any small deviation of the ¹Hα CEST profile baseline from 0. ¹J_CαHα was fixed for both the ground and excited states to 143.5 Hz. For residues with small Δϖ_IN, the ¹Hα CEST profile acquired with a B₁ field of 33 Hz was fit residue-wise by fixing the p_I and k_ex,IN to the values obtained from the global fit in order to extract Δϖ_IN.

4.3.3. CytR^N

¹Hα CEST profiles were acquired at B₁ fields of 13 Hz (12 Hz spacing, 127 planes) and 36 Hz (30 Hz spacing, 52 planes) over a chemical shift range of 2.83 to 5.33 ppm for an exchange duration (T_ex) of 500 ms. Global fitting to the two-state Bloch-McConnell equations was done as described above for L24A FF using 2 B₁ field ¹Hα CEST profiles of A10, V21, T23, A24, T25, V26, T40, E50, V51, Y53 and P55, all of which have |Δϖ_DE| > 0.2 ppm. In this case, ΔR₂ was allowed to float as it resulted in a significant improvement in the quality of the fit compared to the case of ΔR₂ = 0. Exchange parameters of k_ex,DE = 38 ± 2 s^-1 and p_E = 4.5 ± 0.1% were obtained from these global fits. For residues with small |Δϖ_DE|, p_E and k_ex,DE were fixed to the values determined from the global fits and the 13 and 36 Hz ¹Hα CEST profiles were fit together at the residue level to extract Δϖ_DE.

4.4. CS-Rosetta structure calculations

The structure of the CytR^N excited state was calculated using 119 chemical shifts (including 23 ¹Hα shifts) and 65 ¹H-¹⁵N residual dipolar couplings (determined in (52)) as described in detail in (52).