Minute oscillation stretching: A novel modality for reducing musculo‐tendinous stiffness and maintaining muscle strength

Abstract

A novel stretching modality was developed to provide repetitive small length changes to the plantar flexors undergoing passive stretch defined as "minute oscillation stretching" (MOS). This study investigated the effects of MOS on neuromuscular activity during force production, the rate of torque development (RTD), and the elastic properties of the plantar flexors and Achilles tendon. Ten healthy males participated in this study. The neuromuscular activity of the triceps surae and tibialis anterior muscles during maximal voluntary plantar flexion torque [MVT], RTD of plantar flexion, Achilles tendon stiffness, and muscle stiffness were measured before and after two types of interventions for a total of 5 minutes: static stretching (SS) and MOS at 15 Hz and without intervention (control). Achilles tendon stiffness was calculated from the tendon elongation measuring by ultrasonography. Muscle stiffness was determined for the medial gastrocnemius [MG] using shear wave elastography. The MVT, mean electromyographic amplitudes [mEMG] of MG and lateral gastrocnemius [LG], and RTD were significantly decreased following SS (MVT: −7.2 ± 7.9%; mEMG of MG: −8.7 ± 10.2%; mEMG of LG: −12.4 ± 10.5%; RTD: −6.6 ± 6.8%), but not after MOS. Achilles tendon stiffness significantly decreased after SS (−13.4 ± 12.3%) and MOS (−9.7 ± 11.5%), with no significant differences between them. Muscle stiffness significantly decreased in SS and MOS, with relative changes being significantly greater for MOS (−7.9 ± 8.3%) than SS (−2.3 ± 2.9%) interventions. All variables remained unchanged in the controls. In conclusion, MOS changed muscle‐tendon compliance without loss of muscle function.

1. INTRODUCTION

Modalities to improve flexibility (eg, joint range of motion, joint stiffness) include static stretching (SS), which stretches a muscle holding the joint at a fixed angle, and dynamic stretching (DS), which repeats dynamic muscle length changes (stretch‐shortening). ¹ Although not conclusive in the literature, several studies have demonstrated superior increases in flexibility with SS versus DS. ² , ³ , ⁴ One of the factors implicated in improving flexibility after SS is increased extensibility of the muscle‐tendon unit (MTU) that works as a series elastic component across its joints. ¹ Previous studies have reported that SS decreases musculo‐tendinous stiffness, which is considered to be a mechanism of flexibility improvement by SS. ⁵ , ⁶ , ⁷ , ⁸ However, SS has been shown to temporarily decrease muscle functions such as maximal muscle strength and explosive power exertion (often observed in sports, including the rate of torque development [RTD] or jump performance). ³ , ⁴ , ⁹ , ¹⁰ , ¹¹ , ¹² Therefore, it is desirable to perform stretching that reduces musculo‐tendinous stiffness and does not decrease muscle function.

In a previous study, we developed a novel stretching technique, "minute oscillation stretching" (MOS), which comprises advantages of both SS and DS approaches. We have reported that the MOS modality improves flexibility as well as SS without decreasing maximal muscle strength, unlike SS. ¹² MOS is a modality of conditioning the MTU that provides repetitive small longitudinal length changes (stretch‐shortening) to the plantar flexors by ankle joint movement undergoing passive stretch (dorsiflexion of approximately 5°, which is likely passive DS in the form of oscillation) (Figure 1).

Figure 1.

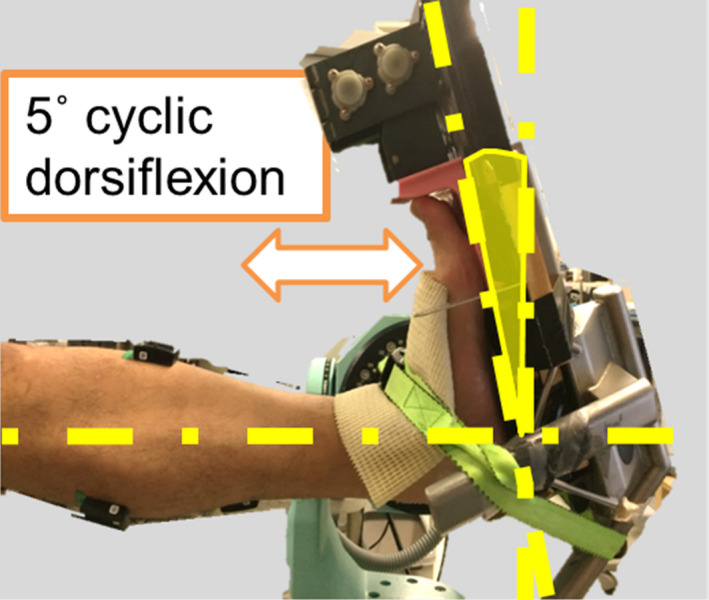
A picture showing the implementation of minute oscillation stretching

The increase in the extensibility of the MTU by SS is known to be contributed by both increasing muscle belly elongation and tendinous tissue elongation after SS. ⁷ , ⁸ , ¹² , ¹³ Moreover, the tendinous tissue stiffness, often assessed by the linear slope of the load‐elongation relationship of the tendinous tissue during isometric force exertion, ⁵ , ⁶ , ¹⁴ is affected by tendinous tissue extensibility and is decreased after long‐duration SS (>3 minutes). ⁵ , ⁶ , ¹⁵ A previous study has also shown that MOS increases gastrocnemius muscle elongation during passive dorsiflexion; however, it does not increase Achilles tendon elongation, ¹² which differs from the findings for SS. However, this study only evaluated part of the load‐elongation relationship of the tendinous tissue, while the change in tendinous tissue stiffness represented by the slope of the linear region under higher tension has not been verified yet. Furthermore, tendinous tissue hysteresis, which is an indicator of viscosity and a parameter correlating with stiffness, ¹⁶ , ¹⁷ also decreases after SS. ⁶ Thus, tendinous tissue stiffness and hysteresis decrease after SS, and similar findings are expected after MOS as well.

Muscle function is known to be attenuated after SS of over 60 seconds per muscle group, ³ , ⁹ , ¹¹ which may be attributed to the reduction in neuromuscular activity during force production in the stretching target muscle group. ¹¹ , ¹⁸ , ¹⁹ MOS does not decrease muscle strength, which is unlike SS ¹² ; thus, it may be presumed that neuromuscular activity during force production remains unchanged after MOS. The calculated RTD derived from the torque‐duration curve during explosive force exertion is decreased after SS; that is, muscle force exertion becomes slower. ¹⁰ , ²⁰ , ²¹ , ²² RTD is mostly influenced by the degree of maximal isometric strength ²⁰ ; therefore, it is speculated that the MOS does not reduce the RTD, but this idea has not yet been tested.

This study investigated the effects of MOS on neuromuscular activity during force production, the RTD, and the elastic properties of the ankle plantar flexors and Achilles tendon. We hypothesized that MOS to plantar flexors would reduce musculo‐tendinous stiffness and tendinous tissue hysteresis of the triceps surae, while not decreasing neuromuscular activity during force production and RTD.

2. MATERIALS AND METHODS

2.1. Participants

Ten healthy male volunteers (age, 25 ± 3 years; body height, 1.73 ± 0.04 m; body weight, 67.9 ± 12.4 kg; all mean ± standard deviation [SD]) with no history of orthopedic diseases (eg, injury to the muscle, tendon, joint capsule or ligament, peripheral neuropathy) in the lower limbs participated in this study. All subjects provided written consent to participate in this study after they were informed of the purpose of this study, the procedures, and the benefits and risks associated with it. This study was approved by the Ethics Committee on Research Involving Human Subjects of the affiliated institution (number: 2015‐171) and was performed in accordance with the Declaration of Helsinki.

2.2. Study design

A total of three conditions for MTU of the major plantar flexors were set: two stretching modalities (SS and MOS) and the control condition (no stretching). Before and after each intervention, we measured maximal voluntary plantar flexion torque and the neuromuscular activity of the triceps surae and tibialis anterior (TA), in addition to the RTD of the isometric plantar flexion, Achilles tendon stiffness and hysteresis, ankle joint stiffness, and muscle stiffness. Each condition was performed at intervals of seven days or more in random order.

2.3. Stretching protocol

An isokinetic dynamometer (CON‐TREX, CMV‐AG, Switzerland) equipped with a footplate dynamic stretching device (Kenko‐yusuri JM‐25, TOPRUN, Japan) was used for both SS and MOS conditions. The subjects maintained fully extended positions of the knee joint in the sitting position and positioned the forefoot on the pedal of the dynamic stretching device. Similar to previous studies, time allocated for SS and MOS was 5 minutes each in total (five sets of 1‐minute and at 20‐second intervals). ⁷ , ¹⁸ The stretching intensity for both SS and MOS was set as the maximal acceptable dorsiflexion angle without severe pain sensation. ⁷ , ¹⁸ Subjective pain was assessed by the visual analog scale, with a score of 1‐5 (VAS; 1: no pain at all; 5: intolerable pain; 0.5 increments). SS and MOS were performed with scores 4 (a subtle pain sensation). The dorsiflexion angle was fixed across all five sets of the stretching intervention (angle‐matched). The initial ankle joint angle during the interval was set at a plantar flexion of 30°. For the MOS condition, the dynamic stretching device was used for plantar flexion and dorsiflexion of the ankle joint by approximately 5° (Figure 1), which was repeated at a frequency of 15 Hz for 1 minute. ¹² For the control condition, the subjects were instructed to rest in a sitting position on the dynamometer for 6 minutes, which was the same time allocated to the SS and MOS interventions, and to avoid stretching the plantar flexors.

2.4. Maximal voluntary isometric plantar flexion torque

The maximal voluntary isometric plantar flexion torque was measured using the isometric dynamometer (VTF‐002, VINE, Japan) in the sitting position. For measurements, the knee joint was fully extended, and the ankle joint angle was fixed to the footplate of the dynamometer at 0° (anatomical position). The subjects performed the warm‐up that produced a force at 80% of maximal effort twice, and then, maximal voluntary plantar flexion torque exertions were performed twice with a 1‐minute rest between trials. The peak torque of each measurement was analyzed, and a third measurement was obtained when the second measured value differed by 5% or more from the first; when three measurements were performed, two close measurement values were adopted. The maximal voluntary isometric plantar flexion torque was defined as the mean value of the two measurements. The signal obtained from the dynamometer was amplified by the supplied amplifier (DPM‐711B, Kyowa, Japan), converted into a digital code at 1 kHz via an A/D converter (PowerLab/16SP, ADInstruments, Australia), and recorded using analysis software (LabChart 7, ADInstruments, Australia) on a personal computer (FMV Lifebook, Fujitsu, Japan).

The neuromuscular activity of the triceps surae and the TA during maximal voluntary isometric plantar flexion torque measurement was recorded using surface electromyography. The electromyogram was recorded using the Delsys EMG data acquisition system (Trigno, Delsys, USA). Active surface electrodes (inter‐electrode distance of 10 mm, recording diameter of 1 mm × 5 mm [bar shape], Delsys, USA) were attached to the skin underlying the muscle bellies of the medial gastrocnemius (MG), lateral gastrocnemius (LG), soleus (SOL), and TA. Electrodes of gastrocnemii and TA were placed approximately 30% of the lower leg length from their proximal insertion. The SOL electrode was placed approximately 30% of the lower leg length from the distal insertion. These electrode attachment positions were adjusted to be over the muscle bellies using ultrasonography (Prosoundα‐7, Hitachi Medical, Japan). The skin was shaved, lightly abraded, and cleaned with alcohol before the electrodes were applied. Similar to the data obtained by the dynamometer, these electromyogram signals were converted into a digital code at 1 kHz via the A/D converter and recorded using the analysis software. After full‐wave rectification and data smoothing of these signals on the software (bandpass filter, passband at 25‐450 Hz), the mean electromyographic amplitude (mEMG) per second including the peak torque was recorded for each measurement. For the TA, which is the antagonistic muscle in plantar flexion, based on the mEMG value of maximal voluntary isometric dorsiflexion torque, the mEMG value at the maximal isometric plantar flexion torque level was expressed as a percentage and was defined as the co‐contraction level. ²³ For the neuromuscular activity of each muscle, we used the mean value of the two measurements.

The RTD of the isometric plantar flexion was repeated five times. The subjects were instructed to perform torque development "as fast and powerfully as possible." ²⁰ , ²⁴ The obtained torque signal was smoothed with a cutoff frequency of 15 Hz using a fourth‐order zero‐lag digital Butterworth filter. ²⁴ The maximum value of time derivative of the torque waveform was defined as RTD and analyzed for each trial. The RTD was acquired from a point of time at 0.134 ± 0.046 seconds after the onset of torque development. The mean value of three trials, excluding trials with maximum and minimal values, was calculated.

2.5. Achilles tendon stiffness and hysteresis

Achilles tendon stiffness and hysteresis were measured using ultrasonography (Prosoundα‐7, Hitachi Medical, Japan). The subjects increased torque development from the relaxed state to the maximal isometric plantar flexion torque level gradually in approximately 5 seconds. After maintaining the maximal torque level for 2 seconds, torque development decreased to the relaxed state gradually over approximately 5 seconds. ⁶ , ²⁵ During this ramp torque development trial, the proximo‐displacement of the distal end of the muscle belly of the MG was recorded using ultrasonic apparatus measurements acquired at 32 Hz. ⁵ , ¹³ , ²⁵ The linear ultrasonic probe (frequency 7.5 MHz, scan width 60 mm; UST‐5712, Hitachi Medical, Japan, with a water bag, MP‐2468, Hitachi Medical, Japan) was firmly fixed on the skin directly over the distal end of the muscle belly of the MG using a double‐sided tape and custom‐made device, consisting of a series of Velcro straps affixed to a Styrofoam template. During the trial, real‐time torque waveforms were displayed on the monitor for visual feedback. The trial was conducted twice with a 1‐minute rest between trials. The trial with the higher peak value of torque development was analyzed for Achilles tendon stiffness and hysteresis.

To obtain changes in the ankle joint angle during the trial, the foot region and lower leg were recorded (Exilim, Casio, Japan) at 30 Hz from the medial malleolus side. Reflecting markers were applied to the four landmarks: the distal end of the first metatarsal bone, the calcaneus, the medial malleolus, and the tibia (located at the middle point of the line between the center of the knee joint and the medial malleolus). Two‐dimensional coordinates of the above‐indicated markers were obtained from the recorded video using analysis software (FrameDIAS5, DKH, Japan). The ankle joint angle was defined as the angle formed by the following two vectors: from the calcaneus to the first metatarsal bone (plantar) and from the medial malleolus to the tibia. A synchronizer system (PH‐100, DKH, Japan) was used to synchronize all equipment.

2.6. Ultrasonographic analysis

Video analysis software (ImageJ, National Institute of Health, USA) was used for the analysis of the video obtained from the ultrasonic apparatus. The displacement of the distal end of the muscle belly of the MG (defined as Achilles tendon elongation; Figure 2) during the ramp torque development trial was measured with force at every 10% of peak torque from the relaxed state to the peak torque during the increasing torque phase (ascending phase) and from the peak torque to the relaxed state during the decreasing torque phase (descending phase) (Figure 3). ⁵ , ²⁵ The ankle plantar flexion torque at the ramp torque development trial was divided by the triceps surae moment arm (estimated from a previous study ²⁶ ) to calculate the Achilles tendon force. ²⁵ Achilles tendon stiffness and hysteresis were determined from the tendon force‐tendon elongation relationship. Achilles tendon stiffness was defined as the slope of the tendon force‐elongation relationship from the 50% to 100% peak force transition in the ascending phase (Figure 3A). ⁶ , ²⁵ During ascending and descending phases, the tendon force‐elongation curves produced a loop. The tendon hysteresis was calculated using the equation: 100 × (Wa ‐ Wd)/Wa (where Wa was an integrated value under the ascending phase [area], and Wd was an integrated value under the descending phase [area]) (Figure 3B). ⁶ , ¹⁷ , ²⁷ The plantar flexion rotation that inevitably occurred during an active torque development increased the proximal displacement of the distal end of the MG muscle belly. To correct this, the measured change in ankle angle during the plantar flexion contraction and the joint rotation–induced displacement of the distal end of the MG muscle belly was determined during the passive rotations over the same change in ankle angle. ²⁵ These analyses were repeated twice per measurement, and the mean value was adopted. The coefficient of variation of tendon stiffness analysis was 3.0 ± 2.0%.

Figure 2.

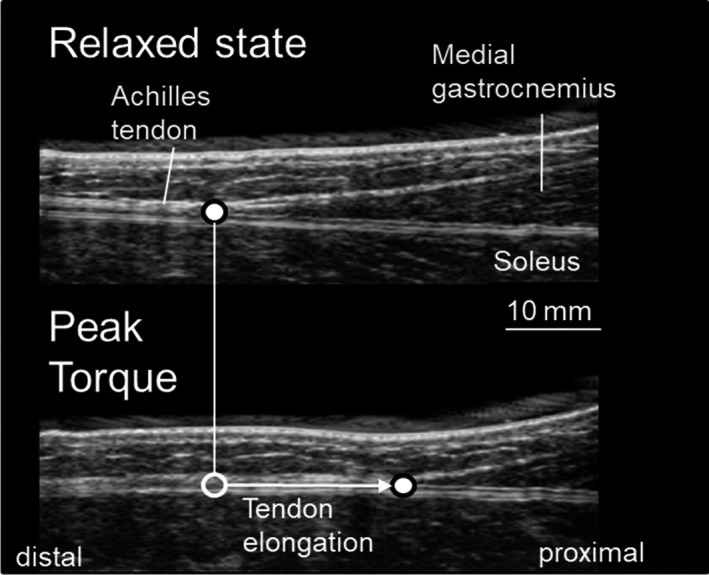
Ultrasonic images of Achilles tendon during isometric plantar flexion (upper; full point: distal end of muscle belly of medial gastrocnemius)

Figure 3.

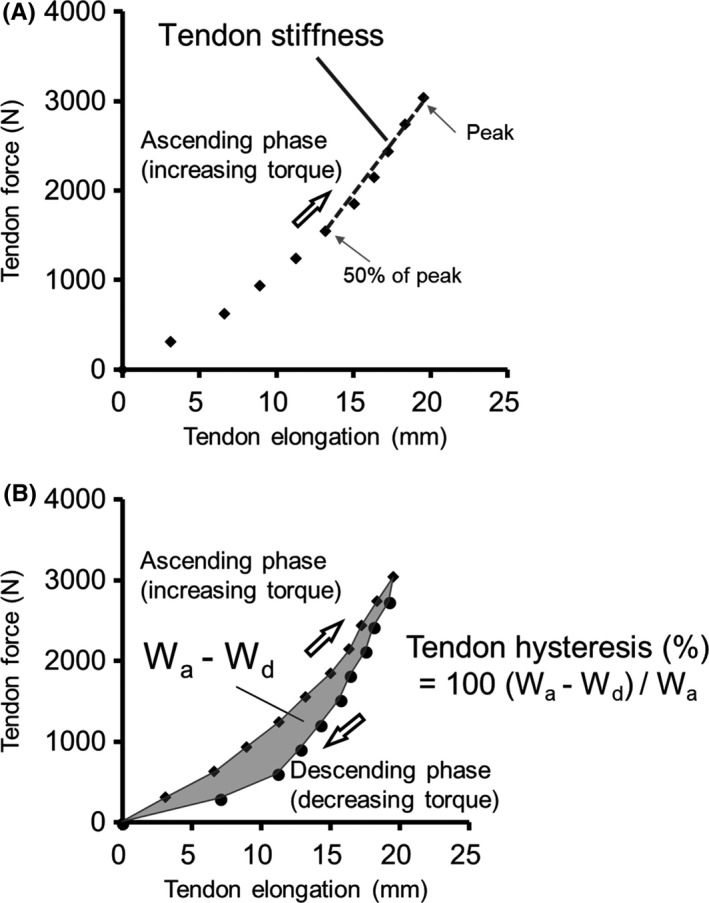
Typical example of Achilles tendon stiffness (A) and hysteresis (B) analyses

2.7. Muscle stiffness and ankle joint stiffness

To assess muscle stiffness, the shear wave velocity of the MG was determined using shear wave elastography (Aixplorer MSK mode, SuperSonic Imagine, France) (Figure 4). In reference to a previous study, ⁸ the ankle joint angles for measurement were set as follows: 0° (anatomical position), 10° dorsiflexion (DF), and 20° DF. Muscle stiffness was calculated from the shear wave velocity and joint angle. The slope of the shear wave velocity‐ankle joint angle relationship at 0°, 10°DF, and 20°DF was defined as muscle stiffness. The linear probe (frequency 4‐15 MHz, scan width 50 mm, SL15‐4; SuperSonic Imagine, France) was fixed on the skin at the center of the muscle belly (at 30% of the proximal length of the leg) using a double‐sided tape and a custom‐made guide consisting of a series of Styrofoam templates affixed by Velcro straps. The shear wave velocity was measured five times at each joint angle. The data of each joint angle were used to calculate the mean value of the three measurements, excluding the maximum and minimum measurements. The region of interest (ROI) was set to a circle with a diameter of 5 mm placed in the middle between the MG aponeuroses (superficial and deep) in the ultrasound image plane. ⁸ The ankle joint stiffness was calculated as the relationship between passive resistance torque and joint angle. ⁷ The passive resistance torque in the direction of plantar flexion was measured at the same three joint angles used for measurement of muscle stiffness (0°, DF 10°, and DF 20°). The passive resistance torque for each joint angle was analyzed as the mean value per second, once the torque had stabilized. The subjects were instructed to relax during these measurements.

Figure 4.

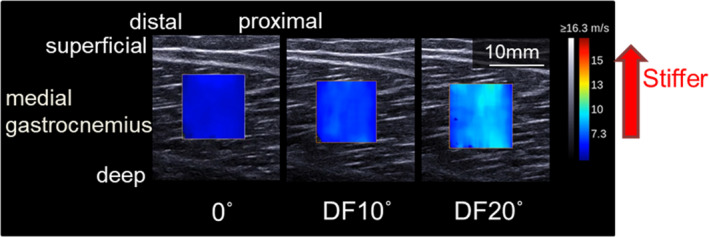
Typical example of muscle shear wave velocity measurement

To confirm that the muscles were in a passive state, neuromuscular activity of the MG, LG, SOL, and TA was measured using surface electromyography during muscle and joint stiffness measurements, as described above. Then, the mEMG (expressed as mean amplitude per second) was calculated for each muscle when the torque was stable for the above‐mentioned three ankle joint angles. The method used for data acquisition, filtering, and normalization were the same as those for the measurement of neuromuscular activity at the maximal isometric plantar flexion torque.

2.8. Statistical analysis

All data are expressed as mean ± standard deviation. For all measurement values of pre‐ and post‐intervention, we conducted two‐way repeated‐measures analysis of variance (ANOVA) on the intervention conditions (SS and MOS) and control condition × time (pre‐ and post‐intervention) using statistical software (IBM SPSS statistics 24, SPSS Japan, Japan). When interaction or the main effect of the time was observed, a paired t test was also performed as a post‐hoc test for each condition. The relative changes for each measurement variable for the pre‐ and post‐intervention were calculated, and one‐way repeated‐measures ANOVA was used to test for statistical differences between the intervention and control conditions. When a significant main effect was observed, a Tukey multiple comparison test was conducted. For effect size, ηp² (for ANOVA) and for the t test, Cohen's d was calculated as follows: d = M _diff/SD_pooled $\surd 2(1‐r)$, where M _diff was the difference between the mean value of the pre‐ and post‐measurement, and r was defined as the correlation between means. ²⁸ Effect size (Cohen's d) was defined as follows: 0.20‐0.50, small; 0.50‐0.80, medium; 0.80‐1.20, large; and >1.20 very large. ²⁹ , ³⁰ The absence of a significant difference among the conditions for each pre‐intervention value was confirmed by performing one‐way repeated‐measures ANOVA. A prior statistical power analysis (using G*power 3) revealed that 27 participants (9 for each condition) were required for this study design (repeated‐measures ANOVA within factors; effect size: 0.4, power: 0.8; alpha level: 0.05 ²⁹ ). The level of statistical significance was set at P < .05.

3. RESULTS

The ICC values for each measurement variable were 0.90 or more, indicating good reliability: maximal voluntary isometric plantar flexion torque, 0.99; mEMG of the MG, 0.96; mEMG of the LG, 0.94; mEMG of the SOL, 0.94; co‐contraction level of the TA, 0.96; RTD, 0.96; Achilles tendon stiffness, 0.97; Achilles tendon hysteresis, 0.98; ankle joint stiffness, 1.00; muscle stiffness, 0.99; shear wave velocities at 0°, 0.99; shear wave velocities at DF 10°, 0.98; and shear wave velocities at DF 20°, 1.00.

There was no interaction effect for maximal voluntary isometric plantar flexion torque (P = .12), whereas a main effect of time was observed (P < .01; ηp² = 0.59). As a result of post‐hoc test, maximal voluntary isometric plantar flexion torque significantly decreased after SS intervention (P = .016; d = 0.85) but not after MOS intervention (P = .26) or rest (control condition) (P = .85) (Table 1). At mEMG of the MG and LG during maximal voluntary isometric plantar flexion torque, no interaction effect was observed (MG: P = .61; LG: P = .094), whereas a main effect of time was observed (MG: P = .037, ηp² = 0.39; LG: P = .046, ηp² = 0.41). A post‐hoc test revealed that mEMG of the MG and LG decreased significantly after SS intervention (MG: P = .028, d = 0.79; LG: P = .017, d = 1.1), but not after MOS intervention (MG: P = .45; LG: P = .25) or rest (control condition) (MG: P = .34; LG: P = .98) (Table 1). There was no interaction or main effect for mEMG of the SOL and co‐contraction level of the TA (SOL: interaction: P = .46; main effect of time: P = .80) (TA: interaction: P = .73; main effect of time: P = .058). There was no interaction effect for RTD (P = .78), whereas a main effect of time was observed (P = .046; ηp² = 0.37). Post‐hoc tests revealed a significantly decreased RTD after SS intervention (P = .024; d = 0.96), but not after MOS intervention (P = .13), or rest (control condition) (P = .19) (Table 1).

Table 1.

Changes in isometric maximal voluntary plantar flexion torque (PFMVT), average electromyography amplitudes (mEMG) of the triceps surae (medial gastrocnemius: MG; lateral gastrocnemius: LG; soleus: SOL) and tibialis anterior (TA, co‐contraction level), rate of torque development (RTD), tendon stiffness, tendon hysteresis, ankle joint stiffness, muscle stiffness, and muscle shear wave velocities at three ankle joint angles (0° [anatomical position], dorsiflexion 10°, and 20°) in each condition

	Control		Static stretching		Minute oscillation stretching
	Pre	Post	Pre	Post	Pre	Post
PFMVT (Nm)	170 ± 47	171 ± 46	170 ± 52	156 ± 50*	178 ± 52	172 ± 50
mEMG during PFMVT
MG (mV)	0.117 ± 0.054	0.113 ± 0.045	0.134 ± 0.054	0.125 ± 0.057*	0.145 ± 0.066	0.141 ± 0.069
LG (mV)	0.120 ± 0.045	0.120 ± 0.045	0.126 ± 0.054	0.110 ± 0.047*	0.127 ± 0.050	0.121 ± 0.041
SOL (mV)	0.077 ± 0.018	0.079 ± 0.017	0.073 ± 0.020	0.069 ± 0.014	0.082 ± 0.019	0.082 ± 0.016
TA (%MVC)	11.8 ± 7.3	12.7 ± 7.6	11.0 ± 6.9	11.4 ± 6.3	12.4 ± 6.3	13.9 ± 7.9
RTD (Nm/s)	897 ± 308	860 ± 292	840 ± 200	790 ± 226*	950 ± 286	893 ± 274
Tendon stiffness (N/mm)	336 ± 94	333 ± 79	332 ± 84	288 ± 81*	335 ± 83	299 ± 68*
Tendon hysteresis (%)	16.9 ± 6.8	16.7 ± 7.7	17.1 ± 6.2	13.9 ± 5.2*	18.3 ± 6.3	13.7 ± 4.6*
Joint stiffness (Nm/°)	1.19 ± 0.40	1.17 ± 0.40	1.28 ± 0.38	1.19 ± 0.37*	1.24 ± 0.48	1.14 ± 0.45*
Muscle stiffness (m/s/°)	0.287 ± 0.053	0.287 ± 0.056	0.293 ± 0.050	0.287 ± 0.052*	0.297 ± 0.063	0.275 ± 0.068*
Shear wave velocities
0 °(m/s)	4.62 ± 0.74	4.61 ± 0.73	4.47 ± 0.66	4.46 ± 0.58	4.51 ± 0.73	4.45 ± 0.80
Dorsiflexion 10° (m/s)	6.97 ± 1.33	6.89 ± 1.33	6.85 ± 1.18	6.78 ± 1.02	7.01 ± 1.16	6.75 ± 1.04*
Dorsiflexion 20° (m/s)	10.37 ± 1.66	10.35 ± 1.69	10.33 ± 1.54	10.20 ± 1.49*	10.46 ± 1.65	9.95 ± 1.63*

^*Significantly changed compared with pre‐intervention (P < .05). Values are expressed as mean ± standard deviation (mEMG of LG and TA: n = 9).

An interaction effect was observed between the conditions and time for Achilles tendon stiffness (P = .015; ηp² = 0.37), with significant decreases both after the SS and MOS interventions (SS: P < .01, d = 1.4; MOS: P = .032, d = 0.86) but not after rest (control condition) (P = .63) (Figure 5A). For Achilles tendon hysteresis, an interaction effect was also observed between the conditions and time (P < .01; ηp² = 0.53), with significant decreases both after SS and MOS interventions (SS: P < .01; d = 2.6; MOS: P < .01, d = 1.7) but not after rest (control condition) (P = .84) (Figure 5B). For the relative changes in tendon stiffness and hysteresis, no significant differences were observed between the SS and MOS conditions (stiffness, SS: −13.4 ± 12.3%; MOS: −9.7 ± 11.5%; P = .70) (hysteresis, SS: −19.1 ± 8.0%; MOS: −23.5 ± 14.5%; P = .66).

Figure 5.

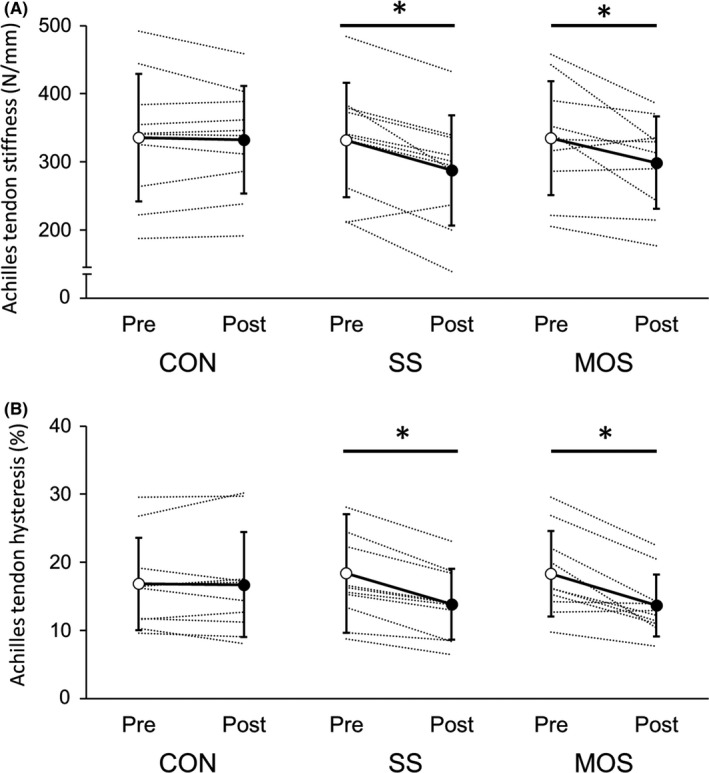
Changes in Achilles tendon stiffness (A) and hysteresis (B) in each condition. (CON: control; SS: static stretching; MOS: minute oscillation stretching. ^*Significantly changed compared with pre‐intervention (P < .05). Values are expressed as mean ± standard deviation

In ankle joint stiffness, no interaction effect was observed (P = .18), whereas a main effect of time was observed (P < .01; ηp² = 0.72). As a result of post‐hoc test, ankle joint stiffness decreased significantly after the SS and MOS interventions (SS: P = .010, d = 1.1; MOS: P = .039, d = 0.81), while there was no change after rest (control condition) (P = .10) (Table 1). An interaction effect between the conditions and time was observed in muscle stiffness (P < .01; ηp² = 0.43) and decreased significantly both after the SS and MOS interventions (SS: P = .021, d = 0.83; MOS: P = .016, d = 0.95), but not after rest (control condition) (P = .84) (Figure 6). The relative changes in muscle stiffness in the MOS condition (−7.9 ± 8.3%) was significantly greater than the SS condition (−2.3 ± 2.9%; P = .039). There was no interaction effect for shear wave velocities at DF 10° (P = .20), whereas a main effect of time was observed (P = .011; ηp² = 0.53). A post‐hoc test revealed that shear wave velocities at DF 10° decreased significantly after the MOS intervention (P = .020; d = 0.94) but not both after the SS intervention (P = .34) or rest (control condition) (P = .34) (Table 1). In addition, an interaction effect between the conditions and time was observed for shear wave velocities at DF 20° (P < .01; ηp² = 0.51), which decreased significantly both after the SS and MOS interventions (SS: P = .043, d = 0.79; MOS: P < .01, d = 1.3), while there was no change after the rest (control condition) (P = .25). The relative changes in shear wave velocities at DF 20° in the MOS condition (−4.9 ± 4.0%) were significantly greater than that of the SS condition (−1.3 ± 1.8%; P = .012). There was no interaction or main effect for shear wave velocities at 0° (interaction: P = .78; main effect of time: P = .26). The mEMG of the MG, LG, SOL, and TA during measurements of muscle stiffness and ankle joint stiffness showed no interaction or main effect at all ankle joint angles (Table 2).

Figure 6.

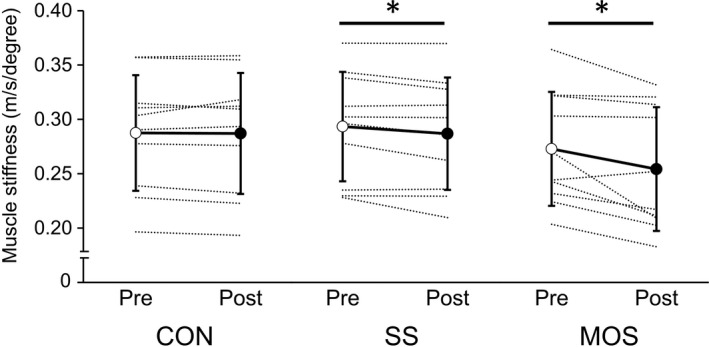
Changes in muscle stiffness in each condition (CON: control; SS: static stretching; MOS: minute oscillation stretching). ^*Significant change compared with pre‐intervention (P < .05). Values are expressed as mean ± standard deviation

Table 2.

Changes in mEMG of the triceps surae (medial gastrocnemius: MG; lateral gastrocnemius: LG; soleus: SOL) and tibialis anterior (TA, co‐contraction level) during ankle joint stiffness and muscle stiffness measurement in each condition

	Control		Static stretching		Minute oscillation stretching
	Pre	Post	Pre	Post	Pre	Post
0° (anatomical position)
MG (%MVC)	1.8 ± 1.4	1.7 ± 1.1	1.6 ± 1.2	1.6 ± 1.0	1.2 ± 0.6	1.2 ± 0.7
LG (%MVC)	1.5 ± 1.0	1.5 ± 1.1	1.4 ± 0.9	1.3 ± 0.7	1.3 ± 0.8	1.3 ± 0.7
SOL (%MVC)	2.3 ± 1.4	2.3 ± 1.4	2.3 ± 1.2	2.4 ± 1.9	2.0 ± 1.8	1.7 ± 1.4
TA (%MVC)	1.9 ± 2.5	0.9 ± 0.4	1.5 ± 1.9	1.5 ± 1.5	2.0 ± 1.8	1.7 ± 1.4
Dorsiflexion 10°
MG (%MVC)	1.8 ± 1.4	1.8 ± 1.4	1.7 ± 1.2	1.6 ± 1.1	1.6 ± 1.8	2.1 ± 3.1
LG (%MVC)	1.5 ± 1.1	1.5 ± 1.1	1.6 ± 1.4	1.3 ± 0.7	1.5 ± 1.1	1.4 ± 1.0
SOL (%MVC)	2.2 ± 1.2	2.3 ± 1.5	2.3 ± 1.1	2.6 ± 2.3	2.1 ± 2.2	1.8 ± 1.7
TA (%MVC)	2.2 ± 2.9	1.0 ± 0.5	1.5 ± 1.8	1.6 ± 1.6	1.0 ± 0.4	1.0 ± 0.4
Dorsiflexion 20°
MG (%MVC)	4.9 ± 5.8	4.0 ± 5.7	4.5 ± 4.7	3.8 ± 4.6	4.9 ± 7.9	4.2 ± 7.3
LG (%MVC)	1.6 ± 1.2	1.7 ± 1.6	1.5 ± 0.8	1.8 ± 1.9	2.1 ± 2.5	2.0 ± 2.1
SOL (%MVC)	2.8 ± 2.0	2.5 ± 1.9	3.7 ± 2.2	3.5 ± 3.3	3.8 ± 4.2	3.5 ± 5.0
TA (%MVC)	3.1 ± 5.2	2.3 ± 4.3	1.5 ± 1.9	1.6 ± 1.8	1.0 ± 0.3	0.9 ± 0.4

Values are expressed as mean ± standard deviation (mEMG of LG and TA: n = 9).

4. DISCUSSION

The present study investigated the effects of MOS to ankle plantar flexors on neuromuscular activity during force production, RTD, and on elastic properties of the triceps surae and Achilles tendon. The results demonstrated that MOS did not decrease neuromuscular activity during force production and RTD, unlike for SS, while Achilles tendon stiffness and hysteresis were reduced to a similar extent to that observed with the SS intervention. Moreover, this study clarified that muscle stiffness decreased after the MOS intervention, and this decrease was higher than that after the SS intervention.

In this study, ankle joint and musculo‐tendinous stiffness were decreased after the SS intervention, which was consistent with the results of previous studies. ⁵ , ⁶ , ⁷ , ⁸ Both ankle joint and musculo‐tendinous stiffness were decreased after MOS, although these results suggested that a potential mechanism of flexibility improvement by the MOS intervention could be a decrease in musculo‐tendinous stiffness as in SS. Additionally, the degree of decrease in muscle stiffness was significantly higher in the MOS than in SS, which indicated that the MOS intervention was more effective at reducing muscle stiffness than the SS intervention. Passive and repetitive stretch‐shortening cycles of the MTU (likely passive DS) result in a reduction in muscle stiffness. ³¹ , ³² Therefore, a reduction in muscle stiffness due to the MOS intervention appeared to be caused by passive and repetitive stretch‐shortening cycles of the MTU and dorsiflexing of the ankle into the final range of motion. The neuromuscular activities of the triceps surae during ankle joint and muscle stiffness measurements were quite low in all conditions (Table 2). Previous studies suggest that the SS intervention changes the neurophysiological properties (eg, efferent neural drive). ³ , ¹⁹ However, the MOS intervention did not change the neuromuscular activity during force production in this study. Therefore, it is unlikely that changes in neurophysiological properties are similar for MOS and SS interventions.

The Achilles tendon stiffness decreased after the MOS intervention, and its reduction was similar to that occurring after SS. This result suggests that MOS changed the material property of the tendinous tissue with an increase in its elongation. The tendinous tissue has been reported to increase in extensibility by being repeatedly exposed to a tension of approximately 80% maximal voluntary contraction (MVC). ¹⁷ However, acute tendinous tissue stiffness reduction (increased extensibility) is considered to be associated not only with the magnitude of load but also with the duration of exposure to the load. ³³ Although the load on the tendinous tissue was smaller than approximately 80% MVC, the MOS intervention continued the load for 5 minutes. Therefore, it was assumed that the extensibility of the tendinous tissue increased after MOS, and the stiffness decreased. Tendinous tissue stiffness has been reported not to decrease after a short duration (15 or 60 seconds) of SS. ³⁴ Further studies are required to clarify the effects of a short‐duration MOS on tendinous tissue stiffness.

Both the MOS and SS interventions caused a similar significant decrease in the Achilles tendon hysteresis. Achilles tendon hysteresis is an index of elastic energy recycling efficiency, and changes in hysteresis and stiffness have been suggested to affect the efficiency of stretch‐shortening cycle (SSC) movements such as running and hopping. ⁶ , ¹⁶ , ²⁵ , ³⁵ Future studies should examine the influence of MOS on the efficiency of SSC movements.

Achilles tendon stiffness was decreased both after SS and MOS, while the RTD decreased after SS but not after MOS interventions. The RTD is affected by maximal strength and time to reach a given force level, ²⁰ and the decrease in Achilles tendon stiffness might have a negative effect on the latter. Furthermore, maximal isometric strength significantly decreased after SS but not after MOS. Thus, we believe that the RTD is affected by maximal strength rather than tendinous stiffness and did not likely decrease after MOS. However, although not a significant decrease, the RTD reduced by approximately −5% after MOS intervention. It is suggested that there was a significant individual difference in the effect of MOS intervention on RTD, and it did not show a significant decrease (pre–post comparison [paired t test]: P = .13; d = 0.36). Higher tendon extensibility has also been reported to negatively affect electromechanical delay (EMD). ³⁶ The decrease in tendon stiffness due to stretching may cause EMD prolongation, which may affect the recruitment pattern of the motor units during RTD negatively. Further studies are required to clarify whether these peripheral changes are caused by MOS.

The maximal voluntary isometric plantar flexion torque and neuromuscular activity of gastrocnemii during torque exertion decreased after SS, and these results are supported by previous studies. ¹¹ , ¹⁸ , ¹⁹ In contrast, these parameters did not change after the MOS interventions. Overall, these results indicate that MOS did not reduce central nervous system input to the stretching target muscle group as occurs for SS, and hence, muscle function remained unchanged. Assuming that MOS has a combined effect of SS and DS on the MTU, it is possible that the decrease in neuromuscular activity caused by SS was offset by an increase in activity by DS. ¹¹ A previous study reported the effects of frequency of DS on muscle function. ³⁷ Although the modalities involved are completely different between MOS and general DS and cannot be directly compared, combinations of different stroke lengths and oscillation frequencies necessitate further studies.

One of the main mechanisms involved in muscle strength and RTD decrease after SS is the reduction in motor commands from the central nervous system to muscles. ⁴ , ¹⁸ , ¹⁹ The mechanism suggested to be involved in this phenomenon is the change in the ability to amplify motor commands at the spinal level (such as a decrease in persistent inward current in spinal motor neurons) that are induced by passive muscle stretching (stimulation to mechanoreceptors). ⁴ , ¹⁸ , ¹⁹ However, an acute increase in the corticospinal excitability is caused by oscillation. ³⁸ Therefore, an intervention that adds oscillation to stretching may offset the change in the ability to amplify motor commands by stretching and that the maximal strength and the RTD decrease do not occur. In addition, previous studies have also indicated that one of the factors involved in muscle strength and RTD reduction after SS is peripheral changes after excitation‐contraction coupling, involving intramuscular Ca²⁺ kinetics, modulation of the recruitment pattern of motor units, and musculo‐tendinous stiffness. ⁴ , ¹⁹ Although musculo‐tendinous stiffness was observed to be decreased after MOS interventions similar to SS, muscle strength and RTD remained unchanged. Additionally, the decrease in musculo‐tendinous stiffness reportedly occurs in the reduced muscle force output by doublet force summation. ³⁹ However, such an effect may have been minimal in the maximal voluntary force exertions in this study. Therefore, we believed that peripheral changes induced by MOS interventions do not affect muscle strength and RTD reduction to a significant degree.

There is an inherent limitation of the measurement of tendinous tissue stiffness using B‐mode ultrasonography; the approach ignores the contribution of other soft tissues, such as the arched structure of the foot, which may behave as a series elastic component, and does not consider the influence of synergist and antagonist muscles on joint torque. ⁴⁰ Hence, the Achilles tendon force might not be accurate. Because the ultrasound elastography method evaluated a small ROI of approximately 100‐300 mm², it should be noted that the results of this study are limited to a phenomenon observed at the center of the muscle belly. Measurement of tendinous tissue hysteresis using ultrasonography has been suggested to be subjected to methodological problems such as the sampling rate, which affects results ¹⁸ ; thus, our results might be dependent on the experimental setting of this study.

In conclusion, this study revealed the following effects of MOS, a newly developed stretching technique: (1) Neuromuscular activity during force production and RTD did not decrease unlike that following SS interventions, (2) Achilles tendon stiffness and hysteresis were reduced to a similar extent to that observed with SS, and (3) the reduction in muscle stiffness was greater than in the SS intervention.

5. PERSPECTIVE

In the present study, a stretching modality, MOS, providing repetitive small length changes to the plantar flexors undergoing passive stretch, improved flexibility by changing the muscle‐tendon compliance while retaining muscle function, unlike those that occurred following SS. These results demonstrated that MOS increased flexibility without reducing muscle function, and thus, MOS may be applied in sports and clinical fields. Our results showing that the MOS approach is more effective in reducing muscle stiffness than SS suggest that this may be a modality implicated in conditioning the MTU. Future research might lead to the development of more effective MOS applications in sporting and clinical fields, for example, examination of the effects of MOS in subjects with different characteristics (ie, sex, physical constitution, and sporting events) and the effect of warm‐up activities combining MOS with active exercises (such as running).

CONFLICT OF INTEREST

The authors declare no conflict of interest.

STATEMENT OF INSTITUTIONAL REVIEW BOARD APPROVAL OF THE STUDY PROTOCOL

This study was approved by the Ethics Review Committee on Human Research of Waseda University.

Ikeda N, Yonezu T, Kawakami Y. Minute oscillation stretching: A novel modality for reducing musculo‐tendinous stiffness and maintaining muscle strength. Scand. J. Med. Sci. Sports. 2021;31:104–114. 10.1111/sms.13830