Heating events in the nascent solar system recorded by rare earth element isotopic fractionation in refractory inclusions

The compositions of the first solar system solids were shaped by nonequilibrium condensation and evaporation.

Abstract

Equilibrium condensation of solar gas is often invoked to explain the abundance of refractory elements in planets and meteorites. This is partly motivated, by the observation that the depletions in both the least and most refractory rare earth elements (REEs) in meteoritic group II calcium-aluminum–rich inclusions (CAIs) can be reproduced by thermodynamic models of solar nebula condensation. We measured the isotopic compositions of Ce, Nd, Sm, Eu, Gd, Dy, Er, and Yb in eight CAIs to test this scenario. Contrary to expectation for equilibrium condensation, we find light isotope enrichment for the most refractory REEs and more subdued isotopic variations for the least refractory REEs. This suggests that group II CAIs formed by a two-stage process involving fast evaporation of preexisting materials, followed by near-equilibrium recondensation. The calculated time scales are consistent with heating in events akin to FU Orionis– or EX Lupi–type outbursts of eruptive pre–main-sequence stars.

INTRODUCTION

It is generally accepted that the building blocks of the terrestrial planets and other inner solar system objects experienced vaporization and homogenization at high temperature at the birth of the solar system (1). According to this view, solids and perhaps liquids condensed as the nebula cooled from gas of solar composition [e.g., (2, 3)]. The composition of the condensates evolved during cooling following a sequence, from more refractory phases rich in Ca, Al, and Ti to more volatile phases rich in K and Na. The condensation sequence is hinted at by the mineralogical and chemical compositions of partial condensed relicts known as refractory inclusions [or Ca-, Al-rich inclusions (CAIs)] that were isolated from the remaining nebular gas before major rock-forming elements such as Si, Mg, and Fe were fully condensed. This early isolation led refractory inclusions to preserve large enrichments in refractory elements such as the rare earth elements (REEs) and distinctively fractionated chemical abundance patterns. A readily identifiable fractionated REE pattern in CAIs is known as group II (4, 5), which is present in 40% of all CAIs, most notably those of fine-grained texture [70% of all CAIs have nonchondritic relative abundances of the REEs; (6)]. Group II CAIs have so far only been documented in carbonaceous chondrites, but this could be due to the scarcity of CAIs in noncarbonaceous chondrites. Group II REE patterns are marked by depletions in the least refractory (Eu and Yb) and most refractory REEs (Gd-Er and Lu), while REEs of intermediate refractoriness (La-Sm and Tm) are uniformly enriched. This pattern cannot be formed by evaporation alone and has been taken as evidence that condensation processes occurred in the early solar system.

The chemical fractionation of REEs recorded by CAIs was pervasive in the early solar system and affected planetary bodies at all scales. For example, the Earth and many meteorite parent-bodies display a resolvable depletion (~−4.5%) in the abundance of Tm relative to CI chondrites, which is interpreted to reflect the heterogeneous distribution in the solar nebula of refractory dust carrying group II REE patterns marked by large Tm excesses (~+200 to +5400%) relative to neighboring REEs Er and Lu (7, 8). After formation, CAIs experienced further processing, which is reflected in their textures and compositions. Some CAIs were reheated and experienced melting and partial vaporization, which obscured signatures arising from solar nebula condensation (9–12). Others, in particular, fine-grained CAIs, never experienced melting and provide a more faithful record of solar nebula condensation. Because of their porous nature, fine-grained CAIs experienced extensive alteration, which modified their composition and mineralogy (13). For elements that are fluid-immobile and refractory, this is, however, not a problem. Available Ti and Sr isotopic data of fine-grained inclusions indicate that kinetic processes were at play when fine-grained CAIs formed, but interpretation of these data in terms of evaporation/condensation processes is ambiguous (14–16).

REEs are ideally suited for testing the theory of equilibrium condensation of the solar nebula because they are relatively fluid immobile and display a range of 50% condensation temperatures that encompass the inferred formation temperature of CAIs (17), and their condensation behaviors are relatively well constrained. Although REE patterns can be explained by equilibrium condensation from gas of solar composition under a total H₂ pressure typical of the solar nebula of 10⁻⁵ to 10⁻³ bar (15, 18–20), abundances alone cannot easily tell if kinetic processes were at play during either evaporation or condensation. The reason is that evaporation kinetics are also governed by thermodynamics and equilibrium vapor pressures through the Hertz-Knudsen equation (21). Isotopes are highly sensitive to disequilibrium during evaporation and condensation (9, 22–28). They can therefore be used to understand the physicochemical pathway of CAI formation.

During condensation from a supersaturated medium, the light isotopes will impinge on mineral surfaces more frequently than the heavy isotopes, resulting in a light isotope enrichment in the condensed phase relative to the vapor. Conversely, during evaporation in an undersaturated medium, the light isotopes will be more readily lost than the heavy ones, resulting in a heavy isotope enrichment of the condensed phase relative to the vapor. If either evaporation or condensation takes place in a vapor whose partial pressure is in close equilibrium with the condensed phase, the fluxes in and out of the condensed phase will be nearly balanced and the vapor and solid/liquid will be in isotopic equilibrium. Given the elevated temperature involved in CAI formation, the expectation is that equilibrium isotopic fractionation should be small. As such, measuring the isotopic composition of REEs in CAIs displaying group II REE patterns should theoretically (i) reveal if these CAIs formed under equilibrium conditions and if they did not (ii) allow the roles played by evaporation and condensation processes to be resolved, which can help tie CAI formation and chemical fractionation of refractory elements to phenomena happening around young stellar objects.

Here, we report the isotopic compositions of eight REEs in eight CAIs (including seven fine-grained ones with group II REE patterns) from the Allende CV chondrite (Table 1). These measurements were made possible using a fluoropolymer pneumatic liquid chromatography (FPLC) system [fig. S1; (29, 30)] to separate the REEs from one another. The REEs analyzed are Ce, Nd, Sm, Eu, Gd, Dy, Er, and Yb. The other polyisotopic REEs La and Lu were not measured, as these have only two stable isotopes, one of which is extremely minor in abundance (0.09% for ¹³⁸La and 2.6% for ¹⁷⁶Lu). Furthermore, large ¹³⁸La anomalies of probably nucleosynthetic origin have been reported (31), which would hamper the detection of mass-dependent fractionation for La. In CAIs with group II REE patterns, we observe substantial stable isotopic fractionations of the REEs indicative of disequilibrium formation pathways.

Table 1. Stable isotopic fractionations of REEs in a geostandard and CAIs.

The δ^φE values are per mil/amu variations relative to terrestrial standards (OL-REEs) calculated using Eq. 1 applied to ¹⁴²Ce/¹⁴⁰Ce, ¹⁴⁶Nd/¹⁴⁴Nd, ¹⁵²Sm/¹⁴⁸Sm, ¹⁵³Eu/¹⁵¹Eu, ¹⁵⁸Gd/¹⁵⁶Gd, ¹⁶⁴Dy/¹⁶²Dy, ¹⁶⁸Er/¹⁶⁶Er, and ¹⁷⁴Yb/¹⁷²Yb ratios.

Isotopic fractionation (%/amu)
Sample	CAI name	δϕCe			#*	δϕNd			#*	δϕSm			#*	δϕEu			#*	δϕGd			#*	δϕDy			#*	δϕEr			#*	δϕYb
BCR-2		0.02	±	0.01	10	−0.01	±	0.02	15	0.00	±	0.01	9	0.01	±	0.03	4	0.02	±	0.03	10	0.02	±	0.03	8	−0.05	±	0.02	8	0.02	±	0.05
TS32		0.24	±	0.06	1	−0.03	±	0.05	4	−0.01	±	0.06	2	−0.19	±	0.05	2	−0.02	±	0.03	2	−0.02	±	0.07	3	−0.02	±	0.13	2	0.04	±	0.07
ME-3364-25.2	FG-FT-3	0.01	±	0.04	9	−0.14	±	0.01	9	−0.09	±	0.06	5	−1.09	±	0.05	1	−0.89	±	0.03	1	−1.04	±	0.07	1	−1.00	±	0.02	1	−0.33	±	0.07
ME-2639-16.2	FG-FT-4	0.81	±	0.04	12	1.1	±	0.01	8	0.8	±	0.06	3	−0.01	±	0.05	2	−0.61	±	0.03	2	−0.58	±	0.06	2	−2.89	±	0.11	1	−0.12	±	0.07
ME-2639-49.7	FG-FT-6	−0.03	±	0.02	9	−0.57	±	0.08	5	−0.37	±	0.06	2	−0.42	±	0.02	1	−1.03	±	0.07	3	−1.67	±	0.04	3	−2.02	±	0.05	1		±
ME-2639-51.1	FG-FT-7	0.42	±	0.03	9		±			0.07	±	0.06	5	−0.58	±	0.05	1	−1.28	±	0.03	3	−1.64	±	0.07	2	−2.24	±	0.02	1	−0.66	±	0.07
AL3S5	FG-FT-8	0.51	±	0.01	9	−0.02	±	0.03	8	0.07	±	0.01	9	0.04	±	0.11	2	−2.26	±	0.01	9	−3.07	±	0.01	9	−1.89	±	0.11	2	0.01	±	0.05
AL4S6	FG-FT-9	−0.82	±	0.01	9	−0.93	±	0.02	11	−0.97	±	0.02	9	0.73	±	0.04	6	−1.05	±	0.07	5	−1.10	±	0.02	7	0.08	±	0.04	6	−0.32	±	0.01
AL8S2	FG-FT-10	0.35	±	0.05	5	−0.16	±	0.08	3	−0.03	±	0.05	1	−1.03	±	0.11	1	−0.35	±	0.07	2	−0.53	±	0.04	1	−3.63	±	0.05	1		±
ME-2639-16.2	FG-FT-4†	0.96	±	0.07	5	0.68	±	0.04	8	0.88	±	0.06	5	0.03	±	0.06	4	−0.57	±	0.03	6	−0.55	±	0.02	6	−2.90	±	0.11	2	−0.12	±	0.05
AL3S5	FG-FT-8†	0.70	±	0.01	8	−0.01	±	0.01	15	0.11	±	0.01	11	0.13	±	0.06	3	−2.28	±	0.02	10	−3.08	±	0.02	8	−1.88	±	0.11	2	−0.05	±	0.15
AL4S6	FG-FT-9†	−0.62	±	0.07	5	−0.87	±	0.01	13	−0.84	±	0.02	7	0.82	±	0.06	3	−1.05	±	0.19	4	−1.15	±	0.06	5	0.01	±	0.11	5	−0.32	±	0.02

*Number of measurements.

†Replicates subjected to substantial loss of REEs during Mo chemistry and not used for data interpretation.

RESULTS

The abundances of Ce, Nd, Sm, Eu, Gd, Dy, Er, and Yb in group II CAIs [table 2 in (15)] are normalized to Sm abundance and CI chondrites (32) and plotted in Fig. 1A in order of increasing refractoriness according to their condensation temperatures under solar nebula conditions in 10⁻⁴ bar H₂ (see fig. S2 for the REE abundance pattern plotted as a function of atomic mass) (17). Both the least refractory (Eu and Yb) and most refractory REEs (Gd, Dy, and Er) are depleted, while the moderately refractory REEs (Ce, Nd, and Sm) display uniform enrichment relative to CI chondrites, which are characteristics of group II patterns (5).

Fig. 1. Abundances and stable isotopic fractionations of REEs ordered by increasing refractoriness (from the least refractory on the left to the most refractory on the right).

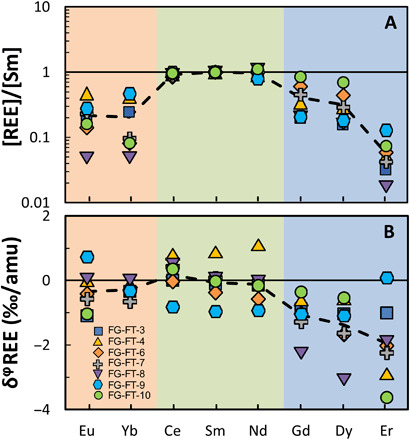
(A) Abundances of REEs in seven fine-grained CAIs with group II REE patterns [table 2 in (15)] normalized to Sm abundance and chondrites (7). Orange, green, and blue shades are least refractory, moderately refractory, and most refractory REEs, respectively. Each symbol corresponds to one CAI. The dashed line is the average abundance of seven CAIs for each REE. (B) Isotopic fractionations of REEs in the same seven CAIs (Table 1). The dashed line is the average isotopic fractionations of the seven CAIs analyzed. Note that one CAI with a flat REE abundance and isotopic pattern (TS32) is not plotted here (Table 1). See Table 1 caption and Eq. 1 for details on the δ^φ notation.

For a given REE, the isotopic fractionation is expressed as per mil per atomic mass unit (‰/amu) deviations relative to terrestrial standard solutions using the following notation (the φ superscript refers to the δ notation on a per atomic mass unit basis)

$${\mathrm{\delta }}^{\mathrm{\phi }}\mathrm{E} (‰/\text{amu})=[\frac{{({}_{ }{}^{\mathit{i}}\mathrm{E}/{}_{ }{}^{\mathit{j}}\mathrm{E})}_{\text{Sample}}}{{({}_{ }{}^{\mathit{i}}\mathrm{E}/{}_{ }{}^{\mathit{j}}\mathrm{E})}_{\text{Standard}}}-1]\frac{1000}{{\mathit{m}}_{\mathit{i}}-{\mathit{m}}_{\mathit{j}}}$$ (1)

where m_i and m_j are the atomic masses of isotopes ${}_{ }{}^{\mathit{i}}\mathrm{E}$ and ${}_{ }{}^{\mathit{j}}\mathrm{E}$, respectively (e.g., Ce isotopic fractionation δ^φCe in ‰/amu is reported as δ^142/140Ce divided by the atomic mass difference 142 – 140 = 2). The reason for expressing isotopic variations on a per mil per amu basis is that the magnitude of mass-dependent fractionation of an element scales to first order with the difference in mass of the isotopes involved, so this notation allows easier comparison of the isotopic variations of several REEs covering different mass ranges. The terrestrial standard solutions used for normalization in the δ^φE notation have isotopic compositions that are close to those of terrestrial basalts, which are presumably close to the bulk Earth values [Table 1; (33)]. The REE isotopic compositions in group II CAIs (Table 1) are plotted in order of increasing refractoriness in Fig. 1B. The most refractory REEs (Gd, Dy, and Er) show substantial negative stable isotopic fractionations ranging from 0 to −3.6‰/amu with averages approximately at −1.5‰/amu. The least refractory Eu and Yb and moderately refractory REEs Ce, Nd, and Sm range from −1 to +1‰/amu and define averages near 0. A CAI with a flat REE abundance pattern was also measured and shows isotopic compositions within ±0.25‰/amu of 0 for all REEs.

Although Sr has a nominal 50% condensation temperature (1464 K) that is ~100 K higher than that of Eu (1356 K) (17), the elemental enrichment factors of Eu and Sr in CAIs normalized to CI chondrites are tightly correlated, meaning that, during evaporation and condensation, the two elements have very similar behaviors (16). Comparing our new Eu isotopic analyses with previous Sr stable isotopic fractionation data measured on the same sample digestion using a double-spike approach shows that δ^φEu and δ^φSr also correlate closely over a range of δ^φSr values from −1.8 to +0.8‰/amu with a slope of 0.67 (Fig. 2A). This is further evidence that Eu and Sr have very similar behaviors during condensation in the nebula. Linear correlations between isotopic compositions were also observed for Ce and Nd versus Sm (with slopes of 0.90 and 1.13, respectively; Fig. 2B) and Dy versus Gd (with a slope of 1.40; Fig. 2C). The Ti stable isotopic fractionation in this set of CAIs was reported in (15) using sample-standard bracketing (SSB) and was remeasured in this study using the double-spike approach to achieve higher precision (table S1). The 50% condensation temperature of Ti is 1582 K under solar nebula conditions (at 10⁻⁴ bar total pressure of H₂), which overlaps with that of light REEs (LREEs) (17). The isotopic fractionation of Ti ranges from −0.2 to +1.0‰/amu but shows no clear correlation with any of the REEs. Because Ti is a major element in CAIs, its behavior during evaporation and condensation is likely to be decoupled from the REEs, which usually substitute into host minerals as trace elements. Correlation matrices showing all possible bivariate correlations for abundances and isotopes of the elements investigated in this set of CAIs are shown in fig. S3. The correlations highlighted above (Eu-Sr, Ce-Nd-Sm, and Dy-Gd) are the only ones that are statistically significant. Yb and Eu, which are among the least refractory REEs, are not correlated isotopically (Fig. 2D). The stable isotopic composition of U also does not correlate with that of any REE including Yb, whose abundance correlates with that of U [fig. S4; (34)].

Fig. 2. Relationship of stable isotopic fractionations of REEs.

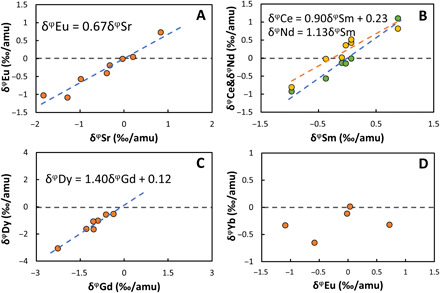
(A) Correlation of isotopic fractionations Eu versus Sr in seven CAIs with group II pattern. (B) Ce and Sm versus Nd. (C) Dy versus Gd. (D) Yb versus Eu. See Table 1 caption and Eq. 1 for details on the δ^φ notation. The REE data are from Table 1, while the Sr data are from (16).

Few data are available in the literature to compare with our results (33, 35). A previous study (33) reported measurements of the stable isotopic compositions of Er and Yb in a variety of terrestrial rocks and bulk meteorites. They found limited variations in meteorites, which span ~0.3‰/amu for δ^φYb and ~0.15‰/amu for δ^φEr. The isotopic variations documented in CAIs are much larger, spanning ~0.7 and ~3.6‰/amu for Yb and Er, respectively. The largest isotopic variations are found in the least REE-enriched CAIs, which contribute the least to the bulk inventory of these elements in CAI-bearing meteorites. This could explain the limited isotopic variations documented by (33) in bulk meteorites. Another study (35) reported measurements of the Sm and Eu isotopic compositions of several chondrites and CAIs. They found limited variations, with δ^φSm and δ^φEu values in CAIs that span ranges of less than 0.04 and 0.06‰/amu, respectively. The δ^φSm and δ^φEu values measured here span ranges of ~1.9 and 1.8‰/amu, respectively. The difference could be due to the fact that we focused on CAIs with highly fractionated REE patterns, while (35) may have measured samples with mostly flat (unfractionated) REE abundance patterns [the REE pattern is known for only one of the four CAIs that they measured and it is a flat group I (5)]. Highly fractionated group II REE patterns are most commonly found in fine-grained CAIs that are difficult to extract from meteorites, and it is conceivable that the CAIs studied by (35) were of the more readily extractable coarse-grained kind. We measured negligible isotopic fractionation in one CAI with a flat REE abundance pattern (TS32; Table 1).

DISCUSSION

A kinetic origin for the isotopic fractionation of the REEs

Although the various isotopic patterns for each group II CAI reflect their unique formation histories, they still share similarities, among which the most distinctive feature is that the most refractory REEs (Gd, Dy, and Er) are all enriched in the light isotopes with average isotopic compositions of approximately −1.5‰/amu. In contrast, the less refractory REEs show smaller isotopic variations, centered around 0 (Fig. 1B).

The isotopic fractionations of the REEs could be due to equilibrium or kinetic processes, or a combination of both during evaporation and condensation. The extent of equilibrium isotopic fractionation can be estimated based on thermodynamic considerations. During evaporation or condensation of CAIs under solar nebula conditions, REEs in minerals (presumably hibonite or perovskite) exist as 3+, except for Eu, for which a substantial fraction may exist as 2+ (36). In the vapor, La, Pr, Nd, Gd-Er, and Lu are predominantly composed of monoxides (2+), with less than 15% present as monatomic gas (0 oxidation state). Gaseous Eu and Yb are nearly all monatomic, while Sm and Tm are predominantly monatomic species with only moderate fractions (~30 and 5%, respectively) of monoxides. The majority (i.e., ~90%) of Ce exists in the vapor as CeO₂ (4+) and the rest as CeO (2+). There are no data or calculations that we are aware of to estimate equilibrium isotopic fractionation between Ce⁴⁺ in the vapor and Ce³⁺ in minerals, but we can estimate the other fractionation factors. All trivalent REEs have similar geochemical behavior, and it is likely that they form chemical bonds of similar strengths. The technique of nuclear resonant inelastic x-ray scattering (NRIXS) applied to the Mössbauer isotope ¹⁵¹Eu in solid EuO and Eu₂O₃ gives force constants of Eu²⁺ and Eu³⁺ of 114 and 196 N/m, respectively (37). The formula that relates reduced partition function ratio to force constant for Eu is [(37) and references therein]

$$1000\text{ln}{\mathrm{\beta }}_{\text{Eu}}^{\mathrm{\phi }}=193\frac{\langle {\mathit{F}}_{\text{Eu}}\rangle }{{\mathit{T}}^2}$$ (2)

where $1000\text{ln}{\mathrm{\beta }}_{\text{Eu}}^{\mathrm{\phi }}=1000\text{ln}{\mathrm{\beta }}_{\text{Eu}}^{1/({\mathit{m}}_{\mathit{i}}-{\mathit{m}}_{\mathit{j}})}$ is the equilibrium fractionation factor in ‰/amu in the compound of interest relative to monoatomic gas, 〈F_Eu〉 is the force constant in N/m, and T is the temperature in K. Using this formula, we estimate that at 1750 K, relevant to CAI formation, the equilibrium isotopic fractionation between Eu²⁺ and Eu³⁺ in solid and monatomic Eu in gas will be +0.007 and +0.012‰/amu, respectively. The force constant of gaseous EuO is not known but it can be calculated using the formula relating vibration wave number to bond force constant for diatomic molecules

$$\langle {\mathit{F}}_{\text{Eu}}\rangle =4{\mathrm{\pi }}^2{\mathit{m}}^{*}{\mathit{c}}^2{\mathrm{\nu }}^2$$ (3)

where ν = 668 cm⁻¹ is the vibration wave number of EuO in an argon matrix (38),c = 299792458 m/s is the speed of light, and m* = 2.404 × 10⁻²⁶kg/amu is the reduced mass of EuO. This gives a force constant of 381 N/m, corresponding to $1000\text{ln}{\mathrm{\beta }}_{\text{Eu}}^{\mathrm{\phi }}=+0.024‰/\text{amu}$ for gaseous EuO. The equilibrium isotopic fractionations between Eu²⁺ and Eu³⁺ in solid and EuO in gas at 1750 K are therefore −0.017 and − 0.012‰/amu, respectively. Regardless of the speciation of the REEs in the vapor or minerals, the expected equilibrium isotopic fractionation is always smaller than ~0.02‰/amu (Fig. 3). Even if a Rayleigh distillation was involved in the depletion of some REEs relative to others, the resulting isotopic fractionation would be 0.1‰/amu at most, assuming a depletion factor of 100. This calculation shows that equilibrium isotopic fractionation cannot explain the REE isotopic fractionation observed in group II CAIs, thus demonstrating that these CAIs are not simple solar nebula equilibrium condensates but that kinetic processes associated with evaporation and/or condensation played some role.

Fig. 3. Equilibrium isotopic fractionations of Eu.

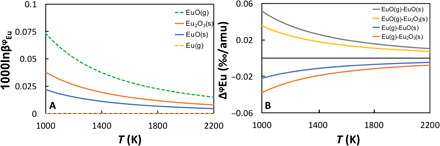
(A) Calculated reduced partition function ratios (in ‰/amu) of Eu compounds as a function of temperature. (B) Equilibrium isotopic fractionation between gas and solid phases of Eu as a function of temperature. See text for details.

Isotopic fractionation of the most refractory REEs

As discussed in the Introduction, the isotopic fractionation experienced by solids or liquids during evaporation and condensation is characteristic of the process involved and the saturation conditions. In the canonical view of the origin of the group II pattern, the most refractory REEs are sequestered in ultrarefractory grains that are removed from the system. Below, we discuss several scenarios for the removal of this ultrarefractory component and show how only the partial evaporation scenario (iii) is viable.

(i) If these ultrarefractory grains (distinct from the refractory CAIs investigated here) condensed under supersaturated conditions, the vapor left behind would have been isotopically heavy. This signature would have been imparted to the highly refractory REEs in CAIs condensed from this vapor, which is the opposite to what is observed (Fig. 1B).

(ii) An alternative scenario is that ultrarefractory grains formed in near-equilibrium conditions, leaving behind vapor depleted in the most refractory REEs but with near-normal isotopic composition. If group II CAIs condensed under supersaturated conditions from such unfractionated gas, the most refractory REEs could have acquired a light isotopic composition, consistent with observations. However, for isotopic fractionation to be expressed during condensation, mass balance requires that only a fraction of the refractory REEs should be condensed. In that case, we would expect a substantial fraction of the more volatile moderately refractory REEs (Ce, Nd, and Sm) to remain in the gas residue, leading to elemental depletion and isotopic fractionations of moderately refractory REEs in group II CAIs, which is not observed.

(iii) The most plausible scenario is therefore that the refractory heavy REE acquired their light isotope enrichments by near-complete condensation of vapor produced by a previous stage of nonequilibrium evaporation that left behind material akin to ultrarefractory grains.

The magnitude of stable isotopic fractionation during partial evaporation depends on the fraction of the target element left in the residue, the kinetic fractionation factor that describes the difference in evaporation rates for two isotopes, and the degree of undersaturation of the vapor (9, 22, 25). The finding of large kinetic isotopic fractionation for refractory REEs Gd and Dy means that evaporation took place in a medium that was undersaturated, which provides constraints on the time scale of this evaporation episode. If the surrounding vapor is highly undersaturated, evaporation is faster and isotopic fractionation is larger than when vapor is near equilibrium and the fluxes in and out the residue are nearly balanced.

To quantify the evaporation time scale, we have built a simple scenario (Fig. 4A; see the Supplementary Materials for details) whereby ultrarefractory grains of equal sizes are evenly distributed in space, and evaporation is driven by a temperature increase T(t) = T_h,0 + Φ_ht, where T_h,0 is the initial temperature and Φ_h is the heating rate (a positive number). The value of T_h,0 has little influence on the model result provided that it is low enough that all REEs are fully condensed at the beginning of the calculation. In our simulations, we adopt a value of 1350 K. Evaporation happens in a closed system until a time (temperature) when ultrarefractory grains are physically separated from the vapor. Some meteorites like Allende display a group II REE pattern at a bulk scale (7, 8, 39), supporting the view that ultrarefractory inclusions with REE patterns complementary to group II were physically separated at large scales from lower temperature refractory materials. We define the time scale of the evaporation event as the time span between 5% evaporation of the least refractory REE (Eu) and the time of ultrarefractory solid removal.

Fig. 4. Trajectories of chemical depletion and isotopic fractionation in the evaporation (left) and condensation (right) stages considered to explain the composition of group II CAIs (see text and the Supplementary Materials for details).

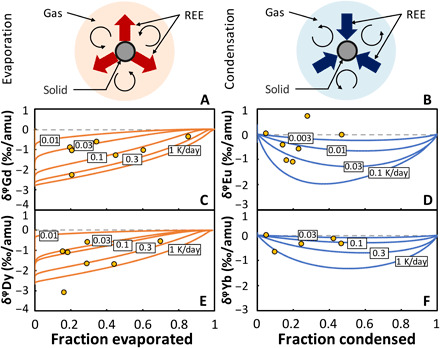
Schematics of the models envisioned for evaporation (A) and condensation (B). (C) Gd and (E) Dy isotopic fractionations and depletions during closed-system evaporation for different heating rates. (D) Eu and (F) Yb isotopic fractionations and depletions during closed-system condensation for different cooling rates. The labels on the curves are the heating rates (evaporation) and cooling rates (condensation) used in the calculations.

This model shares similarities with those applied to closed-system condensation/evaporation and chondrule formation (22–24, 26, 27). The chemical depletions and isotopic fractionations of the REEs evaporated depend on the heating rate and duration of the evaporation episode. If temperature increases slowly, the vapor pressure is always close to equilibrium, evaporation is slow, and isotopic fractionation approaches equilibrium, which is small at the temperatures considered. Conversely, if temperature increases rapidly, evaporation is fast but insufficient to keep up with the increasing equilibrium vapor pressure, leading to undersaturation and light isotope enrichment in the vapor (22). The two unknowns of the model are the heating rate and duration, which can be solved for using the two observables chemical depletion and isotopic fractionation of Gd and Dy—the two highly refractory REEs most affected by this evaporation episode.

The fractions of Gd and Dy evaporated from the ultrarefractory residue can be estimated for each CAI using their abundances normalized to chondrites over that of the moderately refractory REE Sm, which, like other moderately refractory REEs, would have been lost quantitatively from the precursors of ultrarefractory inclusions and fully condensed in the CAI. We have used thermodynamic modeling to calculate the isotopic fractionations and depletions of Gd and Dy for different heating rates and compare model predictions with our measurements (details of the modeling are provided in the Supplementary Materials). The thermodynamic data, oxygen fugacity, and number of moles of Ca condensed per unit volume of the system used for modeling evaporation are from (15) assuming that the mineral host of REEs during evaporation is hibonite. Although hibonite is rare in the fine-grained CAIs that we studied, we have adopted it as the REE solid carrier because (i) hibonite is the highest temperature mineral that incorporates substantial REEs, (ii) it is the only likely REE host phase over a 55-K range (40), (iii) it could have been present as a precursor mineral before disappearing by reaction with the vapor during cooling and is found in some ultrarefractory inclusions in CM chondrites (6), and (iv) perovskite is another option but it cannot explain Tm anomalies (19). We find that the heating rates of Gd (Fig. 4C) and Dy (Fig. 4E) in most CAIs fall in the range of ~0.03 to 0.3 K/day for a duration of the evaporation episode of hundreds to thousands of days.

Stable isotopic fractionation of the least refractory REEs (Eu and Yb)

The uniform enrichment of the moderately refractory REEs and the relative depletion of the least refractory REEs can potentially be explained by either evaporation or condensation. In the evaporation scenario, the vapor produced by evaporation of an ultrarefractory component as described above was quantitatively condensed in a second generation of refractory inclusions that were subsequently reheated and lost the least refractory REEs (Eu and Yb) to the vapor. In the condensation scenario, during cooling of the parcel of vapor produced by evaporation of an ultrarefractory component, the moderately refractory LREEs were quantitatively condensed but vapor was separated from the solid before the least refractory REEs condensed.

Overall, Eu and Yb show limited stable isotopic fractionations, with most Yb data displaying negative δ^φYb values consistent with partial condensation being the dominant process. A likely explanation for the limited isotopic variations but large elemental depletions of Eu and Yb is that their condensation took place under near-equilibrium conditions. The degree of isotopic fractionation during condensation (14, 22, 25, 28) is related to the degree of chemical depletion, the kinetic isotopic fractionation factor associated with condensation, and the degree of supersaturation. As with evaporation, isotopic fractionation during condensation can provide constraints on the time scale of this condensation episode. If the surrounding vapor is highly supersaturated, condensation is faster and isotopic fractionation is larger than when vapor is near equilibrium and the fluxes in and out the condensate are nearly balanced.

To quantify the condensation time scale, we have built a simple scenario (Fig. 4B; see the Supplementary Materials for details) whereby vapor produced during the first evaporation episode condenses in a second generation of refractory inclusions, and condensation is driven by a temperature decrease T(t) = T_c,0 + Φ_ct, where T_c,0 is the initial temperature and Φ_c is the cooling rate (a negative number). The value of T_c,0 has little influence on the model result provided that it is high enough that all REEs are fully evaporated at the beginning of the calculation. In our simulations, we adopt a value of 1700 K. Condensation happens in a closed system [also see (22, 26)] until a time or temperature at which the vapor is dissipated and the remaining least refractory REEs are lost. We define the time scale of the condensation event as the time span between 5% condensation of highly refractory REE (Dy) and the time of gas dissipation. The chemical depletions and isotopic fractionations of the condensed REEs depend on the cooling rate and duration of the condensation episode. If temperature decreases slowly, the vapor pressure is always close to equilibrium, condensation is slow, and isotopic fractionation approaches equilibrium, which is small at the temperatures considered. Conversely, if temperature decreases rapidly, condensation is fast but insufficient to keep up with the decreasing equilibrium vapor pressure, leading to oversaturation and light isotope enrichment in the condensate (22). Moderately refractory REEs are present in near-chondritic proportions and show little isotopic fractionations (Fig. 1A and fig. S1), so they were most likely quantitatively condensed during this stage. Highly refractory REEs were also quantitatively condensed. The only REEs affected by this stage are the least refractory Eu and Yb. Despite their large elemental depletions (Fig. 1A), these REEs show little isotopic fractionations (Fig. 1B), meaning that cooling must have been protracted and condensation occurred under near-equilibrium conditions. We calculated the cooling rate (Φ_c) and overall duration t (time of vapor dissipation after the onset of condensation) needed to explain the large depletions and small isotopic fractionations of Eu and Gd depletions (Fig. 4D). We find that the cooling rates relevant to most CAIs are lower than 0.1 K/day for Eu and 1 K/day for Yb (Fig. 4F). The duration of this condensation episode must have been at least a few hundreds of days.

Evaporation-condensation scenario inferred from REE isotope systematics

The isotopic compositions and depletions of the most refractory and least refractory REEs are most readily explained in the context of a two-stage evaporation-condensation scenario (Fig. 5).

Fig. 5. Schematics of group II REE fractionation in CAIs.

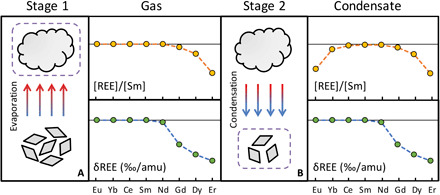
(A) Stage 1: Nebular dust with chondritic proportions of the REEs evaporates in an undersaturated medium, which leaves behind an ultrarefractory residue and produces vapor depleted in the heavy most refractory REEs that are also characterized by light isotope enrichments. (B) Stage 2: The vapor from stage 1 partially condenses. The highly refractory (HREEs except Tm and Yb) and moderately refractory (LREEs and Tm) are completely condensed. The least refractory REEs Eu and Yb remain in the gas and are not completely condensed. The condensation during that stage occurs in near-equilibrium conditions, resulting in minimal isotopic fractionation for Eu and Yb.

In the first stage, nebular dust with chondritic REE abundances was subjected to closed-system evaporation. The least refractory and moderately refractory REEs were quantitatively vaporized, and the vapor inherited the isotopic composition of the solid precursor for these elements. The most refractory REEs were only partially vaporized. The partial evaporation of the most refractory REEs induced substantial negative isotopic fractionations in the vapor because the lighter isotopes evaporated more readily than heavy ones during kinetically controlled evaporation.

In the second stage, vapor was physically isolated from the solid residue and subsequently condensed to form CAIs with group II REE pattern under near-equilibrium conditions. The most refractory REEs and the moderately refractory REEs were completely condensed at this stage and inherited the abundance and isotopic compositions from the gas phase. The least refractory REEs were only partially condensed, which resulted in their depletion in CAIs. The limited isotopic fractionations observed in the least refractory REEs required that the cooling that induced condensation must have been slow enough to maintain near-equilibrium conditions.

We have modeled the REE abundance and isotopic pattern predicted by this two-stage scenario and it can quantitatively reproduce the composition of group II CAIs. In this two-stage scenario, the elemental abundances of the most refractory REEs were fractionated due to previous isolation of an ultrarefractory component, which had an REE pattern complementary to group II pattern except for Eu and Yb. Such ultrarefractory REE patterns are rare but are found in a few refractory inclusions from carbonaceous chondrites such as Murchison and Ornans [e.g., (41, 42)]. A prediction of our model is that ultrarefractory inclusions should be evaporation residues, and as such, they should be enriched in the heavy isotopes of the REEs, especially for the least refractory and moderately refractory (Ce, Nd, Sm, Eu, and Yb). The view that ultrarefractory inclusions are evaporation residues agrees with the W depletion measured in an ultrarefractory inclusion from Ornans, as this feature requires relatively oxidizing condition, which is more easily achieved through evaporation than condensation of solar nebula gas (41). Analyzing the stable isotopic fractionation of REEs in these refractory inclusions will shed light on their genetic relationship with group II CAIs.

Early solar system events responsible for the formation of CAIs

According to the two-stage scenario outlined above, CAIs characterized by group II patterns were formed by heating above 1600 K for hundreds to thousands of days of dust with initially chondritic REE composition, followed by cooling of the vapor formed by the first evaporation stage for at least a few hundreds of days. It is difficult to tie this thermal history to specific early solar system events, but we speculate that such a heating history could have taken place during rapid solar outbursts when the young Sun went through EXor or FUor events akin to those documented in remote stars. EXors are a group of pre–main-sequence stars characterized by intense, episodic increases in brightness separated by quiescent periods (43). They are named after the prototype EX Lupi, which is a low-mass, Sun-like young star located at Lupus cloud (44). FUors are eruptions in FU Orionis objects, another group of pre–main-sequence stars that see episodes of brightening of higher intensity and longer duration than EX Lupi (43, 45). Observations of accretion tracers such as spectral lines and veiling indicate that these large, repetitive outbursts are caused by episodic increase of mass accretion from the protoplanetary disk onto the star. Taking EX Lupi as an example, the typical time scale of the outburst ranges from several months to a few years, during which the brightness of the star can increase by two to five magnitudes (44). The brightness during the outburst can fluctuate substantially, possibly leading to rapid temperature swings in the inner region of the protoplanetary disk.

The increase in brightness during FUors and EXor outbursts could be associated with large scale transport and intense thermal processing of preexisting dust in the central region of the protoplanetary disk (46, 47). This is supported by the discovery in 2008 of in situ formation of crystalline silicates in one of the largest outbursts that happened in the circumstellar disk of EX Lupi (48). Objects like CAIs might have been subjected to similar thermal processing near the protostar during FUors or EXor outbursts. The increased luminosity of the Sun and internal dissipation associated with higher mass flux through the disk would both lead to higher temperatures in the solar nebula. We propose that FUors and EXor outbursts or similar events happened in the early solar system and are responsible for the formation of group II CAIs and refractory dust of similar composition.

The periods of the optical light luminosity of FUors and EXor outbursts range from hundreds of days to 100 years (43, 45). The timescale for dust transport between cooler and hotter parts of the disk during FU Orionis events could be as low as tens of years (46, 47). These timescales are consistent with the time required for evaporation of the most refractory REEs and the condensation of the least refractory REEs. During the outburst or passage through the hot inner part of the disk, only part of the most refractory REEs (and all the least refractory and moderately refractory REEs) was vaporized at the peak luminosity, leading to substantial light isotope enrichments of the most refractory REEs in the vapor. When the outburst subsided or when CAIs moved outwards, most REE condensed except the least refractory REEs, prohibited by the relatively high ambient temperature. The chemical and isotopic compositions of CAIs with group II patterns might reflect the influence of several cycles of evaporation and condensation before final condensation in refractory inclusions. During or after the FUors or EXor outburst, newly formed CAIs would have been distributed to the outer regions of the solar system through extensive radial transport, where they were incorporated in comets and other low-temperature outer solar system objects (49–52).

MATERIALS AND METHODS

Sample selection and digestion

The samples used in this study were selected from a group of CAIs that were previously studied by (34). The sampling and digestion procedures are described in detail in the supplementary section of (34) and only briefly summarized here.

The CAIs studied by (34) were mostly fine-grained CAIs extracted from a few Allende slabs using a stainless steel dental tool. The mass of the CAIs ranged from 15 to 440 mg [table S2 in (34)]. A small chip of each CAI was taken and mounted in epoxy for chemical and petrographic characterization. Most minerals have a grain size on the order of about 10 μm and are mostly composed of spinel, pyroxene, sodalite, and nepheline, with minor and variable amounts of grossular, melilite, and olivine. One coarse-grained CAI (TS32) was characterized in a separate study and provided in powder form. It is primarily composed of melilite, pyroxene, anorthite, and spinel, with a small amount of grossular and perovskite (53). The main mineral carriers of REEs in TS32 are pyroxene, perovskite, and melilite (53). Fine-grained CAIs in Allende have been extensively altered, and to our knowledge, the distribution of REEs in these objects has not been studied.

Powdered samples were digested in a mixture of HF/HNO₃ in 3:1 proportion with a few drops of HClO₄ added. The samples in acid were placed on a hot plate at 160°C for 2 weeks, after which each sample was evaporated to dryness and redissolved in a 2:1 mixture of HCl:HNO₃ for 1 week on a hot plate. These steps were performed twice to ensure complete digestion. The samples were dried down and dissolved in concentrated HNO₃. All samples were then diluted in 3 M HNO₃ and centrifuged. No residue was visible. After dissolution, 80% of the sample was taken and processed through two U/TEVA columns for U extraction. The matrix cuts that contained all REEs were recovered after the U/TEVA chemistry.

REE extraction and FPLC elution

Approximately 30% of the matrix cut from the U/TEVA chemistry of (34) (equivalent to 24% fraction of the whole CAI) was passed through a prepacked TODGA column for extraction of the bulk REEs (32). The yields of this REE TODGA extraction step were near 100%. The bulk of the REE cut was then subjected to a two-step FPLC (29, 30) elution to separate REEs from each other.

Another 40% from the same matrix cut of the U/TEVA chemistry was passed through a separate Mo chemistry, during which 40 to 90% of LREEs and 20 to 40% of heavy REEs (HREEs) were lost on columns. We analyzed the matrix cuts after Mo chemistry of three group II CAIs (FG-FT-4, 8, and 9) as replicates and compare their results with the same CAIs using the 30% matrix cut so as to assess the reproducibility of the isotopic analyses (see the “Assessment of data accuracy” section). The results from these replicates agree with those from the 30% matrix cut. They are not used, however, for data interpretation to avoid unnecessary influence of stable isotopic fractionation potentially induced by Mo chemistry.

As REEs behave very similarly during chromatography, difficulties in separating individual REEs from one another have hampered their isotopic analyses, especially for mass-dependent fractionation. Previous stable isotopic fractionation measurement of REEs has therefore focused on either Sm + Eu (35) or Er + Yb (33). To separate all target REEs, we developed an FPLC system at the University of Chicago [fig. S1; (29, 30)]. The FPLC system was modified after publication of (29) to pneumatic actuation (30). Its most distinctive features are the following.

(i) The elution scheme is controlled by a computer via a LabView software interface, which allows one to run a fine-step gradient elution and mix as many as six reagents. Achieving a fine gradient elution like the one performed for the present study using traditional gravity-driven chromatography would be prohibitively labor intensive.

(ii) The liquid flow path is made of solid fluoropolymer. All parts related to elution are activated pneumatically using pressurized N₂ gas. Electronics are housed in a box that is positively pressurized and spatially isolated from the reagent reservoirs and the liquid flow path. This design reduces chances of contamination and ensures that the FPLC electronics are immune to acid fumes in the clean laboratory environment. A commercial high-performance liquid chromatography (HPLC) instrument would not survive under the harsh conditions needed to perform the elution.

(iii) The elution temperature can be adjusted from room temperature to 80°C through a water circulation system to achieve optimal and reproducible separation. The mixed reagents are forced through the column via pressurized high-purity N₂ gas (adjustable from 0 to 4 bar), making it possible to run elutions on extremely long columns (~70 cm for REE separation).

The sample solution is introduced into the FPLC system via a sample introduction loop composed of two fluoropolymer three-way valves. By changing the flow paths of the two valves, samples can be injected and stored in a tube between the two valves, to be later connected to the major flow path of elution. The column used for FPLC elution of the REEs is 70 cm in length and 1.6 mm in internal diameter, filled with 1.4 ml of 25- to 50-μm Ln-Spec resin (Eichrom). The overall affinity of REEs for Ln resin decreases exponentially with increasing acid molarity (29). The REEs are eluted sequentially from low (La) to high (Lu) atomic number by ramping up the HCl molarity (see fig. S5A for the gradient elution used). The REE elution is performed at 70°C and consists of 94 steps of 2 ml each of increasing molarity from 0.1 to 6 M HCl. The flow rate of the elution is 0.17 ml/min. The total elution volume is 188 ml, and an elution lasts 16 hours. The eluting acid is automatically made by mixing Milli-Q (MQ) water with any of three HCl reservoirs (0.5, 2, and 6 M, respectively). The volumes of acid and water needed are automatically calculated in the LabView software and transferred into a mixing chamber using pneumatic positive displacement diaphragm metering pumps made of fluoropolymer. The elution curve was calibrated, and the elution cuts were defined using 10-ppm (parts per million) SPEX CertiPrep Multi-Element standard solution (fig. S5B). The eluted fractions were collected in fluoropolymer beakers mounted on a 16-position plastic-made, pneumatically actuated, and computer-controlled stage. The whole elution is automated and does not require operator attention after the sample is introduced.

All samples were subjected to a two-step FPLC elution at 70°C. In the first step, the bulk of the REEs was dried in a 6-ml PTFE beaker on a hot plate to a tiny drop (<5 μl) and then dissolved in 350 μl of MQ water. The dissolved sample was then loaded onto the FPLC system and subjected to a complete elution. The eluted reagents were collected in 32 cuts ranging from 2 to 20 ml each defined by knowledge gained on a previous calibration. Approximately 5% of each cut was taken and diluted for concentration measurement by multicollector inductively coupled plasma mass spectrometry (MC-ICPMS). Elution cuts that contained a given REE were then combined based on the concentration measurements. The first elution was sufficient to separate most REEs with only minor overlap of Eu/Dy and Pr/Nd (<20% Dy in the Eu cut and 25% Pr in the Nd cut) and major overlap of Y/Er (fig. S5B), none of which showed clear isobaric and matrix effects according to our doping tests. To achieve a better separation, a second more specific elution step was performed. In this step, REEs that are not neighbor to each other were recombined and loaded onto the FPLC system. This involves four FPLC elutions (Ce, Nd, Sm/Gd/Er, and Eu/Dy/Yb) ranging from 92 to 188 ml (fig. S6). The overall yields of the two-step FPLC elution were higher than 95%. At the end of each elution, the column was cleaned by elution of 10 ml of 6 M HCl followed by 10 ml of MQ water at 70°C to eliminate cross contamination. The same resin batch was used for all the purifications with no adverse effect on the elution curves.

MC-ICPMS analysis and data reduction

The concentrations of all REEs and the isotopic compositions of Ce, Nd, Sm, Eu, Gd, Dy, Er, and Yb were analyzed at the University of Chicago on a Thermo Fisher Scientific MC-ICPMS upgraded to Neptune Plus specifications with the addition of an OnTool booster pump. The method for analyzing REE concentrations using an MC-ICPMS was discussed in detail in (32).

Isotopic analyses were done using the Neptune MC-ICPMS connected to an Apex Omega desolating nebulizer. A typical measurement consisted of 60-s take-up time, 60-s baseline, and 40 cycles with an integration time of 8.184 s per cycle. The cup configurations used for isotopic analyses of the REEs are provided in table S2. Most REEs were measured in static mode with the exception of Dy and Yb, for which a subconfiguration was used to monitor isobaric interferences. The subconfiguration was only measured twice at the beginning with integration times of 4.142 s each, and the average intensities were used to correct for isobaric interferences of the following 40 cycles in the main cup configuration after appropriate scaling. For example, correction of ¹⁶²Er and ¹⁶⁴Er on ¹⁶²Dy and ¹⁶⁴Dy, respectively, proceeds as follows: ¹⁶⁶Er is only measured in the subconfiguration, and ¹⁶³Dy is measured in both the main and subconfigurations. The ratio of intensities ¹⁶⁶Er/¹⁶³Dy in the two subconfigurations is calculated. This ratio is then multiplied by the ¹⁶³Dy intensity in the main configuration. The resulting main-configuration calculated ¹⁶⁶Er is then multiplied by the ratios ¹⁶²Er/¹⁶⁶Er and ¹⁶⁴Er/¹⁶⁶Er to subtract ¹⁶²Er and ¹⁶⁴Er from ion intensities at masses 162 and 164, which yields corrected ¹⁶²Dy and ¹⁶⁴Dy intensities.

To save sample solution, the idle time between cycles with the same configurations was set to zero, while the cycle after a configuration change was preceded by 10-s idle time. Isobaric effects from isotopes of neighboring elements were corrected by monitoring other isotopes of the same element, calculating intensities assuming natural abundance distribution, and subtracting the intensities from those of interfered isotopes.

As isotopic compositions of REEs in CAIs can be affected by nucleosynthetic anomalies and cosmogenic effects, we measured the isotopic composition of each REE through SSB. SSB is advantageous over the double-spike approach because one can distinguish mass-dependent fractionation from isotopic anomalies, which were observed in some REEs with more than two isotopes but are much smaller (and for the present purpose negligible) compared to mass fractionation. The overall yields on the columns were over 95%. Processing of a geostandard showed that no significant isotopic fractionation was introduced by column chemistry, at least compared to the large natural variations measured in the samples. As isotopic standards of REEs are not well established, especially for HREEs, we prepared isotopic standards (referred to as OL-REE series) using high-purity oxide in powder form from ESPI, which are available upon request from J.Y.H. or N.D. On average, LREEs were measured nine times bracketed by OL-REE isotope standard spaced apart by 300-s rinsing time. The concentration of the sample solution was usually 15 to 25 parts per billion (ppb), corresponding to a 3.5- to 10-V signal for the most abundant isotope (10 V for ¹⁴⁰Ce, 4 V for ¹⁴²Nd, and 3.5 V for ¹⁵²Sm). Eu and HREEs are depleted in CAIs with group II REE pattern and were usually measured one to six times at concentrations ranging between 1.5 and 10 ppb corresponding to 1.5 to 3 V for the most abundant isotope (2 V for ¹⁵¹Er and ¹⁵⁸Gd, 3 V for ¹⁶⁴Dy, and 1.5 V for ¹⁶⁶Er and ¹⁷⁴Yb). Exceptions are Er measurement in FG-FT-3, 6, 7, and 10, which, due to the extreme Er depletion, were analyzed at 1.5 ppb with a signal of 0.25 V for ¹⁶⁶Er. Despite the low abundance for some REEs in CAIs, the total procedure blanks were more than two orders of magnitude lower than the sample amount. The substantial isotopic fractionation of highly depleted Er in some CAIs is also evidence that the sample measurements were not plagued by contamination from matrix surrounding the CAIs, as this would have shifted these Er isotopic compositions toward that of bulk Allende (~0‰/amu).

Isotope data reduction was done by copying the raw data into a spreadsheet and correcting for background and isobaric interferences. As documented in previous studies (54), isotopic anomalies and cosmogenic effects are present for some REEs in CAIs but the variations are on the order of 0.1‰, which is negligible compared to the stable isotopic variations reported here. Therefore, the choice of ratio for reporting mass-dependent fractionation is not critical. For that purpose, we use in Eq. 1 the two most abundant isotopes for each REE.

The measurements were performed by SSB, whereby sample measurements (SMP) were interspersed with measurements of standards (STD) diluted to the same concentration as the sample, in the same acid as that used for diluting the sample. The reported δ^φE values were calculated based on 1 to 12 (typically 5) STD-SMP-STD bracketings, using each time the average isotopic ratio of the two STD measurements to normalize the isotopic ratio of SMP. The confidence intervals for the isotopic fractionations are reported as 95% confidence intervals using the Student’s t value and either the variability of sample δ^φE values (i.e., all the sequences STD-SMP-STD) for those with six or more values or the variability of the δ^φE values of standards bracketed by standards (i.e., all the sequences STD-SMP-STD-SMP-STD) for sample measurements with less than six values. The threshold of six repeat brackets is a trade-off between the following considerations: (i) It is difficult to robustly assess the SD based on a small number of bracketed sample measurements, and this can be estimated more robustly by using the isotopic dispersion of the numerous standard analyses performed during a session. (ii) The time between standard measurements is longer than that of samples bracketed by standards, which can detrimentally affect the SD, meaning that using the isotopic dispersion of the samples can be preferable.

Other than stable isotopic fractionation, REE isotopes in group II CAIs can be affected by isotopic anomalies induced by nucleosynthesis and, for isotopes with large neutron capture cross sections such as ¹⁴⁹Sm, ¹⁵⁵Gd, and ¹⁵⁷Gd, cosmogenic effects [see (54) for examples of cosmogenic effects in CAIs]. For the REEs that we analyzed in this study, all of them except Eu have four or more isotopes. Hence, we were able to monitor several pairs of isotope ratios and calculate isotopic anomalies by internal normalization using an exponential fractionation law. The isotopic anomalies recorded in the CAIs were all about two orders of magnitude smaller compared to the stable isotopic fractionation per amu for the same REE. Eu only has two isotopes (¹⁵¹Eu and ¹⁵³Eu). Thus, the stable isotopic fractionation of Eu cannot be distinguished from isotopic anomalies by monitoring different pairs of isotope ratios. ¹⁵¹Eu has a large thermal neutron capture cross section [9200 barns (b)], but it is much lower than that of ¹⁵⁷Gd (255,000 b), which shows cosmogenic effects that would correspond to a shift of 0.05‰/amu if misinterpreted in terms of isotopic fractionation. Both ¹⁵¹Eu and ¹⁵³Eu are mostly of r-process origin. Thus, we can safely assume that Eu isotopic variations are due to stable isotopic fractionation rather than nucleosynthetic anomalies or cosmogenic effects. The fact that Eu isotopic variations correlate with mass-dependent Sr isotopic fractionation (Fig. 2A) supports this view.

Approximately 5% of the matrix cut after U/TEVA chemistry (4% of the whole CAI) was pipetted out and used for analyzing Ti isotopic anomalies. Approximately 5% of the REE matrix cuts were also analyzed for Ti stable isotopic fractionation using the double-spike approach. The isotopic analyses and correction of isotopic anomalies followed (55) and (56).

Assessment of data accuracy

The data quality of REE isotopic analyses was controlled by measuring the total procedural blank, a terrestrial geostandard, and running replicates. The total procedural blanks for all REEs are less than 0.25 ng, which is negligible compared to the amounts of REEs in the samples. A geostandard (terrestrial basalt BCR-2) was processed with the CAIs. The stable isotopic fractionations normalized to the isotopic standards for all REEs are zero within error bars (typically less than 0.05‰/amu). The three replicates based on the matrix cut of Mo chemistry were found to yield generally consistent results compared to those of the same CAI that have not experienced substantial loss of REEs. The stable isotopic compositions of LREEs in the replicates are shifted to the heavy side by approximately 0.1‰/amu, while HREEs yield the same result within error bars (Table 1). The only exception is Nd in FG-FT-4, which is shifted by 0.4‰/amu. The stable isotopic fractionation of LREEs induced by Mo chemistry (possibly chromatography) is not negligible (~0.1‰/amu) but much less than the natural isotopic fractionations measured in these CAIs (−1 to +1‰/amu for LREEs).

Another piece of evidence demonstrating the accuracy of the data acquired is that our Eu isotopic variations correlate very tightly with Sr isotopic variations measured using a double-spike approach in a different laboratory, using a completely independent chemical procedure and a thermal ionization mass spectrometer (TIMS) for isotopic analysis (16).

The data quality of Ti isotope measurements was verified by measuring two geostandards BCR-2 and G-3, which yield values that are consistent with previous measurements (55, 57). The Ti double-spike measurements are also compared to the previous measurement on the same samples using SSB, which are generally consistent but are less precise (table S1).

SUPPLEMENTARY MATERIALS

Supplementary material for this article is available at http://advances.sciencemag.org/cgi/content/full/7/2/eabc2962/DC1