What the COVID-19 school closure left in its wake: Evidence from a regression discontinuity analysis in Japan

Abstract

To control the spread of COVID-19, the national government of Japan abruptly started the closure of elementary schools on March 2, 2020, but preschools were exempted from this nationwide school closure. Taking advantage of this natural experiment, we examined how the proactive closure of elementary schools affected various outcomes related to children and family well-being. To identify the causal effects of the school closure, we exploited the discontinuity in the probability of going to school at a certain threshold of age in months and conducted fuzzy regression discontinuity analyses. The data are from a large-scale online survey of mothers whose firstborn children were aged 4 to 10 years. The results revealed a large increase in children’s weight and in mothers’ anxiety over how to raise their children. On the outcomes related to marital relationships, such as the incidence of domestic violence and the quality of marriage, we did not find statistically significant changes. These findings together suggest that school closures could have large unintended detrimental effects on non-academic outcomes among children.

1. Introduction

During the COVID-19 pandemic in 2020, many countries closed schools in order to control infection. According to UNESCO (2020), more than 80% of students worldwide experienced school closures at the end of March 2020. Of course, there has been heated debate on the pros and cons of this policy. On the one hand, school closure has been seen as a natural policy response to the sudden outbreak of new respiratory diseases, since young children are extremely efficient at catching and passing them on, as has been found in the case of influenza (Cauchemez et al., 2008). However, opponents emphasize that children’s education is severely disrupted and their mental health may suffer in countries with national school closures. Several medical studies also show that school closure is not an efficient way to control COVID-19 infection, because unlike influenza, children are not among the populations that suffer greatly from COVID-19 (Armbruster and Klotzbücher, 2020, Iwata et al., 2020).

Despite the importance of understanding the various consequences of past school closures in the current policy debate, there has not been a sufficient number of studies that directly reveal the effects of school closure on children and their families. So far, some studies have explored the effects of anti-COVID-19 policies on children’s health outcomes and daily life, such as effects on obesity (Pietrobelli et al., 2020) and child maltreatment (Baron et al., 2020). Many studies also explore how lockdown affects the incidence of domestic violence (DV), as a prominent outcome that affects families (Piquero et al., 2020, Sanga and McCrary, 2020, Leslie and Wilson, 2020, Mohler et al., 2020, Campedelli et al., 2020, Payne and Morgan, 2020, Baron et al., 2020)1 .

Even with so much effort, it may be potentially impossible to identify the effects of school closures specifically in the countries that enforced a lockdown, because, in these countries, schools were closed jointly with the implementation of numerous other kinds of anti-COVID-19 policies, including stay-at-home orders and business suspension orders. Therefore, it still remains a challenge for researchers to isolate the effects of “school closure” from those of other anti-COVID-19 policies. For example, many papers on the effects of COVID-19 policies compare the trend of outcomes between 2019 and 2020 (Brodeur et al., 2021, Leslie and Wilson, 2020), but this strategy may not be adequate for the evaluation of school closures, as other concurrent policies may contribute to changes in the behavior of children and their families entirely.

In contrast, utilizing the experience of school closure in Japan, this study successfully estimates the pure impacts of school closure on the well-being of families comprehensively, which is our study’s largest contribution. This was made possible for the following two reasons: First, as we will see in Section 2, Japan is the rare country that experienced school closure without any heavy restrictions on daily life activities, which makes it possible to separate the effects of school closure from the effects of other anti-COVID-19 policies such as lockdown. Second, we utilize the prominent features of the Japanese school closure: all elementary schools were closed in March 2020, while preschools were exempted from this nationwide school closure. This enables us to compare the two groups of children and parents who faced totally different school closure situations even with only a very small difference in the timing of the children’s birth. Due to this small difference in birth timing, these children and their families are likely to have similar characteristics and experiences of other anti-COVID-19 policies. Thus, by comparing these two groups, we can identify the pure impact of school closure.

Another contribution of our study is the data collection approach. We implemented a large-scale online survey in a timely manner. By doing so, our study can explore the impacts of school closures on comprehensive outcomes before the memories of potential respondents fade, which prevents measurement error in their answers. Furthermore, by creating an original questionnaire covering almost all the potential impacts of school closure on families, including novel and unique questions, we could obtain valuable findings and implications that would not have been obtainable from readily available public data (Leslie and Wilson, 2020, Baron et al., 2020).

Further, as we will see in the conclusion section, the results we have obtained yielded very important policy implications, which are also among our contributions.

In this study, we explore how a marginal difference in the timing of children’s birth changed their experiences of school closure in March and eventually changed children’s and families’ outcomes, through a fuzzy regression discontinuity design (RDD) with an age-based threshold (Lee and Lemieux, 2010, Canaan, 2020). As results of our regressions, we gain several valuable findings, as follows: According to our fuzzy estimates of the impact of “non-schooling” due to school closure, the following two can be said to be the most conspicuous results: The fraction of mothers whose child(ren) gained weight rose by 14.4 to 15.4 percentage points, and mothers who worry over how to raise their children rose by 17.8 to 20.2 percentage points. Note that the magnitude of these numbers is non-negligible: these impacts are statistically significant even at a 1 % significance level. In contrast, we do not see any significant effect in other family outcomes, such as incidence of DV or quality of marriage index (Norton, 1983).

The remainder of this paper is constructed as follows: Section 2 offers an explanation of the natural experiment in Japan. Section 3 provides a description of the data and the main outcome variables. Section 4 explains the identification strategy and empirical methods. Section 5 reports the main results, and the results of the subsample analyses are presented in Section 6. Last, Section 7 concludes.

2. Background

As mentioned in Section 1, unlike in many other countries, in Japan, there have been no strict restrictions on daily life activities except for official requests to stay at home and not travel to other regions. This was due to the fact that COVID-19 did not spread rapidly at the time and the national government had no legal basis to implement a city-wide lockdown. Therefore, throughout February and early March in 2020, most economic activities went on as usual. However, following the rapid spread in nearby countries (China and South Korea), Prime Minister Shinzo Abe abruptly requested that all schools nationwide close as of March 2 (Cabinet Office, 2020), most likely in the hope that Japan would be able to host the Tokyo Olympics as planned (New York Times, 2020).

This sudden and unpredictable request for school closure is one of the most prominent features of Japan’s anti-COVID-19 measures. In fact, Japan’s nationwide closure was suddenly implemented despite the fact that the cumulative number of COVID-19 deaths was only three as of the day of the announcement. Because the school closure on March 2 was unexpected and implemented so abruptly, it caused substantial confusion to families. As a suggestive piece of evidence, we found a sharp increase in the number of Google searches for the word “divorce” on March 2—the first day of school closure—which is explained in Online Appendix A. This made us realize that it is necessary to more comprehensively and thoroughly investigate the impact of school closure on family well-being compared to existing studies because marital relationships can affect parents and children in many ways, which may include unexpected side effects.2

On the other hand, another surprising and sudden event occurred right after the announcement of the requested nationwide school closure by the prime minister: The Ministry of Health, Labour and Welfare announced that preschools were exempted from the nationwide school closure because of the potential impacts of the closure on working parents. Therefore, whether children were affected by school closure in March depended on children’s school grades. Specifically, given the school grade system in Japan, the first graders born in March, who are the youngest within the same school grade, experienced school closure in March 2020. In contrast, children born in April, who are the eldest preschoolers, did not experience school closure because preschools were generally open at that time, which implies that children very close in age-in-months were exposed to different schooling policies. These two groups seemed to experience a similar threat caused by the spread of COVID-19, and they were also exposed to other policies such as requests for physical distancing similarly3 , but whether they experienced school closure in March was totally different between them.4 Utilizing this natural experiment, we implemented a large-scale online survey to uncover the pure impact of school closure on the well-being of families comprehensively.

3. Data

3.1. Survey

For this study, we hired an Internet-survey company called Cross Marketing, Inc., and employed random sampling from about 4,790,000 people across the nation who had pre-registered as potential survey participants. The survey was implemented during the period from July 22, 2020, to August 19, 2020.

In the survey, we targeted married women whose co-resident firstborn child was born between April 2, 2010 and April 2, 2016, which roughly corresponds to 4–10 years old.5

In the actual implementation, we sent out invitations to our survey to 44,218 women, and among them, 22,553 mothers responded to our invitations and satisfied the requirements of the sample. We also included a question asking about their willingness to participate in the main survey after having explained that the main survey includes some sensitive questions such as inquiring about their mental health and the marital relationship. Through this question, 17,860 mothers eventually agreed to move on to our main survey. Ultimately, 15,836 mothers answered all the necessary questions, and thus, the number of the sample in the main analyses is 15,836.6

3.2. Descriptive statistics and representativeness

Next, we report descriptive statistics in our survey in comparison with other representative surveys. From the planning of the questionnaire, we made several questions the same as those in an already-existing survey in order to check the representativeness of our online internet survey afterward. To check the representativeness, we utilized two waves of the Japanese Study of Stratification, Health, Income and Neighborhood (J-SHINE), which were conducted in 2010 and 2012. The reason we use J-SHINE here is that it asks about the incidence of DVs in a solid manner proposed by Straus and Douglas (2004) and also includes other basic variables common to our covariates.7

The comparison with J-SHINE in Table 1 provides useful information about the representativeness of the respondents in our survey. First, we do not see any large difference between our data and J-SHINE in most of the mean values of basic covariates such as the age of respondents and the number of children.8

Table 1.

Descriptive statistics: comparison with J-SHINE.

	Our Survey		J-SHINE
	Mean	S.D.	Mean	S.D.
	(1)	(2)	(3)	(4)
Basic Characteristics
Age	37.31	5.25	36.97	4.72
Number of Children	1.89	0.71	1.88	0.73
Firstborn Child Is a Girl	0.49	0.50	0.50	0.50
4-Year College Graduates: Mother	0.40	0.49	0.28	0.45
4-Year College Graduates: Father	0.47	0.50	0.54	0.50
Working (Mother)	0.55	0.50	0.48	0.50
Regular Worker (Mother)	0.20	0.40	0.14	0.35
Domestic Violence
More than once  = 1
Physical DV	0.30	0.96	0.26	0.75
Physical DV by Wife	0.15	0.49	0.14	0.45
Physical DV by Husband	0.15	0.50	0.12	0.42
Frequently  = 1
Physical DV	0.11	0.59	0.14	0.59
Physical DV by Wife	0.05	0.30	0.06	0.32
Physical DV by Husband	0.05	0.31	0.08	0.35
N	15,836		746

Notes: The total score of DV measures is 10 at maximum because we asked 10 questions on DVs, and here we used dummies for each item of DV. The 10 DV items consist of five types of DVs (e.g., “ignoring” and “hitting”) and who did it (i.e., wife or husband). In addition to this, we measured the frequency of DVs in three categories (i.e., Never, Sometimes, and Frequently). Thus, For the results of “More Than Once = 1,” we count the number of DVs for which the respondent chose “Sometimes” or “Frequently.” For the results of “Frequently = 1” in our survey, we counted the number of DVs for which the respondent chose “Frequently” only, while the results of “Frequently = 1” in J-SHINE, we count the number of DVs in which the respondent chose “More than Twice,” since the J-SHINE survey counted the number of DVs in three categories (i.e., None, Once, and More than twice).

Next, the incidence of physical DVs, which is a useful indicator of marital quality, seems to be similar between our survey and J-SHINE: The total physical DV score was 0.3 in our survey and 0.26 in J-SHINE. This supports that our survey did not pick up a very specific population in terms of marital quality and family environment related to children.

3.3. Dependent variables

• • Changes Related to Children Caused by the COVID-19 Outbreak

  Our survey contains many yes-or-no questions about the changes in the respondent and her family members due to the COVID-19 outbreak. Among these questions, we report the results on the items regarding changes in the respondent’s child and the mother–child relationship caused by the COVID-19 outbreak.

• • Domestic Violence

  Following several studies (Straus and Douglas, 2004, Hidrobo and Fernald, 2013), we adopted multidimensional concepts of incidents of DVs that cover the emotional and physical aspects of DVs. For emotional DVs, we asked about incidents of “neglect or ignoring,” “insult,” and “behavior control.” For much more physical DVs, we asked whether a respondent has broken their spouse’s possessions or threatened their spouse by attempting to strike or actually striking them. For each positive response on DV, whether the violence was initiated by the father or the mother is noted. Thus, we asked 10 questions on DVs (i.e., 5 types and who did it). We also asked the frequency of the type of DV using the following three categories (i.e., 1. Never, 2. Sometimes, and 3. Frequently). Then, we calculated the total score of DVs by summing up the frequency measure over each of the 10 questions, which results in a score range from 10 to 30.

• • Satisfaction with Marriage

  As a convenient way to evaluate the quality of a marriage, we asked: “Are you satisfied with marital life?” with a 5-point Likert-type scale ranging from 1 for “not at all” to 5 for “very satisfied.”.

• • Risk of Divorce

  We measured the risk of divorce from four aspects: the incidence of 1. quarrel, 2. discussion of divorce with the spouse, 3. self-thinking of divorce, and 4. proposal of divorce from husband. The frequency of each item was evaluated on a 5-point Likert-type scale, ranging from “usually” for 5 to “none” for 1. We report the total score, which ranges from 4 to 20.

• • Quality of Marriage Index (QMI)

  QMI, which is one of the most popular indexes for evaluating marital quality in the academic literature, was developed by Norton (1983). In this study, we used Moroi (1996)’s marital quality scale, which incorporated and translated the concepts contained in Norton (1983)’s QMI into Japanese. Moroi (1996)’s marital quality scale consists of six items regarding marital life,9 and each question was answered on a 4-point scale. We report the total score, which ranges from 6 to 24. Note that a higher score indicates a higher quality of marriage.

4. Empirical strategy

We will explore the effects of school closure on family well-being during the outbreak of COVID-19. Here, note that we use the word “schooling” to refer to school-aged children (generally over seven years old) attending elementary school as well as preschool children (younger than seven years) going to nursery school or kindergarten as usual, despite the COVID-19 outbreak. Similarly, “non-schooling” refers to children not going to school, regardless of whether they belong to an elementary school or preschool.

4.1. Identification

As explained earlier, for school-aged children, elementary schools were completely closed as of March 2.10 In contrast, nursery schools and kindergartens were generally open at that time, following the announcement of the Ministry of Health, Labour and Welfare.

Interestingly, these governmental decisions on the status of school closures created two groups of children that faced totally different statuses of school closures even with a very small time difference in the matter of their births. More concretely, as of March 2020, children at the age of 89 months (at the time of the survey) belonged to the first grade at elementary school, while children at the age of 88 months were still preschool children in the highest grade in preschool facilities. Note that in spite of the fact that the age-in-months between the two groups differs by only one month–88 and 89–whether or not they could go to school differed between the two groups.

Based on these facts, we uncover the effects of proactive school closures by comparing several outcomes between mothers who had children barely below and barely above the threshold of the age-in-months of 89. This comparison is based on the idea that both the observable and unobservable factors that could potentially affect outcomes of interest (i.e., y) are continuous at the age-in-months of 89. Thus, if we find any discontinuity in y at the threshold, it can be interpreted as the “pure” impact of the school closures.

4.2. Fuzzy regression discontinuity design

Although it was announced that preschools were exempted from this nationwide school closure, in truth, not all preschools were open: The decision to close or open nursery schools and kindergartens was left to each facility and to the municipality where they were located.

In other words, exceeding the threshold age-in-months, that is, 89, did not mean that the probability of “non-schooling” changed from 0 to 1, since there were some preschools that also made a decision to close the facilities. Furthermore, even though preschools were not closed, children or their parents could choose whether to attend. Thus, being a preschool child did not necessarily mean “full schooling,” while school closure in elementary schools was enforced fully. Due to this imperfect compliance among preschools and the available option for preschool children and their parents whether to go to preschool, we apply a fuzzy RDD to estimate the causal effects of not going to school in March 2020.

Since our running variable is age-in-months, which is uncontrollable, there should in principle be no general manipulation problem around the threshold. Regarding another potential threat to identification, the timing of school entry cannot be manipulated, since school admission dates are strictly enforced (Kawaguchi, 2011). Also, grade retention is extremely rare in the Japanese school system.11

Finally, note that age-in-months of the firstborn child is used as the running variable. It is technically possible to use the age of the youngest child in the household as the running variable, but if that was used, it could lead to mistakenly treating some households with more than one child, for example households that consist of a preschool child whose preschool was open plus a school-aged child facing school closure, as those that did not face school closure at all. Note that this mistake cannot happen if we use the age-in-months of the firstborn child in the household as the running variable.

4.3. Local-linear regression

Since our identification framework is the fuzzy RDD, the treatment effect is recovered by dividing the marginal change of outcome variables around the threshold by the fraction of children who did not go to school due to the nationwide request of school closure in March 2020. Specifically, for a respondent (mother) i in our survey, we estimate a system of local-linear regressions of the following form. The first-stage specification is:

$$\mathit{Non}-{\mathit{Schooling}}_i={\alpha }_0+\alpha I(m_i⩾89)+{\alpha }_L(m_i-89)+({\alpha }_R-{\alpha }_L)I(m_i⩾89)(m_i-89)+{\epsilon }_i,$$ (1)

and the reduced-form specification is:

$$Y_i={\beta }_0+\beta I(m_i⩾89)+{\beta }_L(m_i-89)+({\beta }_R-{\beta }_L)I(m_i⩾89)(m_i-89)+{∊}_i,$$ (2)

where $89-b⩽m_i⩽89+b$ and b is the optimal bandwidth around the cutoff point. Next, $\mathit{Non}\mathit{-}{\mathit{Schooling}}_i$ is a binary variable which takes a value of one if mother i’s firstborn child did not go to school in March 2020, and $y_i$ is the outcome variable of mother i’s firstborn child or herself depending on the questions. $m_i$ represents age-in-months of the firstborn child. $I(m_i⩾89)$ is an indicator function that takes 1 if the firstborn child’s age-in-months is 89 or older and otherwise takes 0. Utilizing the estimates on coefficients of $I(m_i⩾89)$ separately obtained from these two equations, the fuzzy regression discontinuity (RD) estimate can be written as $\widehat{\beta }/\widehat{\alpha }$.

Note that the parameter ($\widehat{\beta }$) obtained from Eq. (2) corresponds to the estimate that will be obtained from a sharp RD regression, which is also equivalent to the magnitude of the discontinuity at the threshold in each figure of outcomes.

Concerning the actual implementation and presentation of this framework, in addition to reporting the results of the conventional local-linear regression, we also report results from the robust bias-corrected inference method (Calonico et al., 2014, Calonico et al., 2020). In the implementation, we use the triangular kernel function that weighs points near the threshold more heavily than those distant from the threshold. Regarding the choice of bandwidth, we use the mean square error optimal bandwidths proposed by the Calonico et al. (2014) (henceforth referred to as the CCT bandwidth).

In the estimations, we use heteroskedasticity-robust standard errors as suggested by Kolesár and Rothe (2018). They showed that the practice of clustering by the running variable does not resolve specification bias problems in discrete RDD settings and can even lead to CIs with substantially worse coverage properties than those based on the conventional heteroskedasticity-robust standard error. Recent papers such as Canaan (2020) have also tended to use the conventional robust standard error in response to the results of Kolesár and Rothe (2018), and so do we.

5. Empirical results

5.1. Checks for continuity assumption

Before presenting the empirical results, we check the validity of the continuity assumption in Online Appendix B. In the check for continuity assumption, we examined the continuity of observed covariates, such as the education level of respondents and age, and found that the basic characteristics of the respondents and their children are sufficiently continuous around the cutoff month. In addition to this, we checked how unobservable characteristics of respondents were distributed around the cutoff month by using the participation rate in the main survey after it was explained that the main survey included some sensitive questions, such as inquiring about negative impacts on family well-being. In short, we found the participation rate in the main survey was also continuous around the cutoff, suggesting that sample selection due to some sensitive questions was not so serious in our survey.

5.2. Impact of school closure on “Non-Schooling”

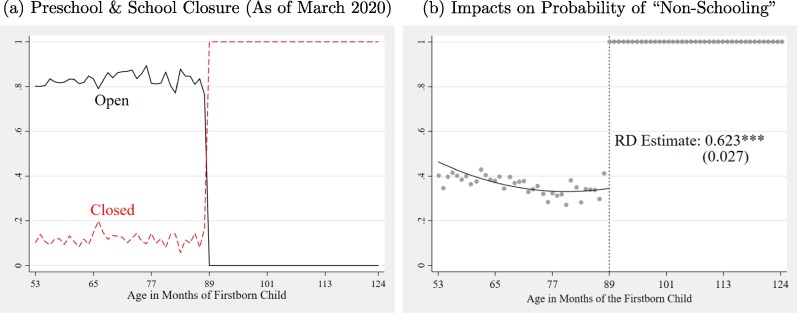
Fig. 1(a) shows the fraction of preschools and elementary schools in our sample that was available or unavailable as of March 15, 2020, for each of age-in-months. Since the cutoff value 89 corresponds to the age-in-months (evaluated in August 2020) for children to move from preschool to elementary school, the probability of school closure becomes suddenly 100% at this threshold.

Fig. 1.

The Impact of School Closures on “Non-Schooling”. Notes: The childcare facilities that were available as of March 15, including those with requests for voluntary restraint in the use of the childcare facility, are categorized as “Open” in Fig. 1(a). In Fig. 1(b), observations are averaged within bins using the mimicking variance evenly-spaced method described in Calonico et al. (2015). Fig. 1(b) also includes second-order global polynomial fits represented by the solid lines. The estimate reported inside the figure is a sharp-RD estimate obtained from the conventional local-linear regressions. Conventional heteroskedasticity-robust standard errors are reported in parenthesis. The CCT bandwidth selector proposed by Calonico et al. (2014) is used to calculate the optimal bandwidth. The same bandwidth is applied to the areas below and above the cutoff. A triangular kernel function is used to construct the estimators. The selected optimal bandwidth is 9.634, and the number of observations within the bandwidth is 4,003. ∗∗∗ p$<$0.01, ∗∗ p$<$0.05, and ∗ p$<$0.1.

Fig. 1(b) presents the RD estimate of the impact of being an elementary school student (becoming 89 age-in-months or older) on the probability of “non-schooling”: It is 0.623, and it is significantly positive even at the 1% significance level.

Thus, we can utilize the large increase in the probability of “non-schooling” around the threshold (caused by the difference in the status of school closures) to identify the effects of schooling.

Since we already confirmed that there was no gap in observable and unobservable factors between mothers who have children barely below and barely above the threshold in the Online Appendix B, if there was some gap in the outcome variables at the threshold, it should have been caused by the discontinuity in the probability of schooling as shown in Fig. 1(b).

5.3. Impact of school closure on family

5.3.1. Empirical results related to children

First, we explore the impact of the national school closure on variables related to children. To estimate the impact on variables related to children, we created yes-or-no questions about the changes in the respondent due to the COVID-19 outbreak. By asking questions focused on changes caused by the COVID-19 outbreak, it is expected that we can exclude the possibility that the estimates capture the effect of the difference in the children’s lifestyles over the past 12 months around the threshold.12

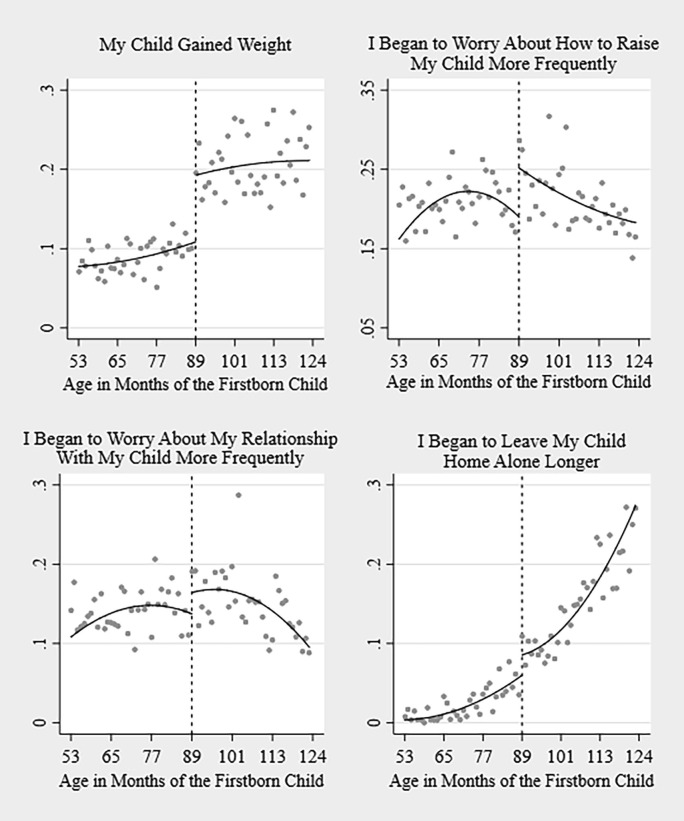
Both Fig. 2 and Table 2 report the results related to children from the yes-or-no questions about the changes due to the COVID-19 outbreak. Table 2 presents estimates from the conventional local-linear regression and those from the robust bias-corrected inference method (Calonico et al., 2014, Calonico et al., 2020). Although as indicated in the previous section, the framework of our study is the fuzzy RD design, we also report sharp-RD estimates as well as fuzzy RD estimates here because reporting the sharp estimates is helpful to compare the magnitude of the estimates with the mean values of each dependent variable as well as the magnitude of the discontinuity in Fig. 2. In contrast, the fuzzy estimates restore the causal effect of not going to school in March 2020.

Fig. 2.

RD Estimates on Changes Related to Children Caused by the COVID-19 Outbreak. Notes: Observations are averaged within bins using the mimicking variance evenly-spaced method described in Calonico et al. (2015). Each plot includes second-order global polynomial fits represented by the solid lines.

Table 2.

RD estimates for the impact of school closures on variables related to children.

	(1)	(2)	(3)	(4)	(5)	(6)	(7)
		Sharp (Reduced Form)		Fuzzy (IV)
Dependent Variable: 1 (Yes) or 0 (No)	Mean of Dep. Var.	Conventional	Bias-corrected	Conventional	Bias-corrected	Optimal Bandwidth	N
My child gained weight
	0.151	0.092∗∗∗	0.096∗∗∗	0.144∗∗∗	0.154∗∗∗	11.570	4,728
		(0.022)	(0.026)	(0.035)	(0.042)
I began to worry about how to raise my child more frequently
	0.218	0.110∗∗∗	0.124∗∗∗	0.178∗∗∗	0.202∗∗∗	7.805	3,189
		(0.032)	(0.036)	(0.053)	(0.059)
I began to worry about my relationship with my child more frequently
	0.157	0.064∗∗∗	0.075∗∗∗	0.101∗∗∗	0.120∗∗∗	9.769	4,003
		(0.025)	(0.028)	(0.039)	(0.044)
I began to leave my child home alone for a longer period of time (per day)
	0.070	0.046∗∗∗	0.052∗∗∗	0.073∗∗∗	0.084∗∗∗	9.898	4,003
		(0.017)	(0.019)	(0.027)	(0.031)

Notes:Table 2 presents estimates from the conventional local-linear regressions as well as estimates to which the robust bias-corrected inference method (Calonico et al., 2014, Calonico et al., 2020) is applied. Conventional heteroskedasticity-robust standard errors are reported in parentheses. For the estimates from the robust bias-corrected inference method, robust standard errors are reported. The CCT bandwidth selector proposed by Calonico et al. (2014) is used to calculate the optimal bandwidth. The same bandwidth is applied to the areas below and above the cutoff. A triangular kernel function is used to construct the estimators.∗∗∗ p$<$0.01, ∗∗ p$<$0.05, and ∗ p$<$0.1.

First, according to Fig. 2, the discontinuity in children’s weight gain is very conspicuous, and the existence of significant discontinuity at the threshold is undeniable. To see the exact magnitude of the discontinuity and statistical significance level, we will also check Table 2.

According to the mean value of the dependent variable from Table 2, about 15 % of respondents answered that their child gained weight. During the period of school closure, children were basically required to stay home, thus, this result makes sense. The sharp-RD estimates in Table 2 also suggest that there exists a very large discontinuity on children’s weight gain by 9.2 to 9.6 percentage points around the threshold and that the estimated coefficients are significantly positive even at the 1% significance level. The fuzzy estimates suggest “non-schooling” due to school closure increases the fraction of respondents whose child (children) gained weight by 14.4 to 15.4 percentage points, which are statistically significant even at the 1% significance level. Note that this estimate means the counter-factual effect of school closure, that is, if the probability of school closure was 0 for the left side of the threshold and 1 for the right side, the effect of school closure would be 14.4 to 15.4 percentage points.

The other conspicuous result is about the following item: “I began to worry about how to raise my child (children) more frequently.” The mean value of the dependent variable in Table 2 and the magnitude of the coefficient are the largest for this item. Concerning the mean value of the dependent variable, about one-fifth of respondents answered yes to this item. The fuzzy estimates indicate that mothers’ worrying over how to raise their children increased by 17.8 to 20.2 percentage points, which is also statistically significant even at the 1% significance level. From this result, we can see how parents became confused and were not ready for the school closure in March without any instruction on how to raise children who do not go to school. Accordingly, we find a large discontinuity in this variable at the cutoff in Fig. 2.

Regarding the remaining two variables: “I began to leave my child home alone for a longer period of time (per day)” and “I began to worry about my relationship with my child (children) more frequently,” although the magnitude of the discontinuity seems somewhat smaller than the first two variables, children’s weight gain and mothers’ worrying over how to raise their child (children), the discontinuities at the threshold are also clear in Fig. 2.

To check the statistical significance, we will move on to Table 2. Concerning the variable “I began to worry about my relationship with my child (children) more frequently,” the mean value of the dependent variable indicates that 15.7 % of respondents answered yes to this question, the number of which is almost the same level as that for children’s weight gain. Comparing this number with the sharp-RD estimates, it can be said that 6.4 to 7.5% of the increase in the fraction of those who answered yes to this question should have been caused by the school closure in March. In contrast, the fuzzy RD estimates indicate that a compulsory school closure that changes a probability of schooling from zero to one would increase the fraction who answer “I began to worry about my relationship with my child (children) more frequently” by 10.1–12.0 percentage points, which is not a small amount.

The modest but still significant effect among the four variables is the variable about leaving a child home alone, which potentially induces delinquency among children (Aizer, 2004, Blau and Currie, 2006). In Japan, since the school closure was announced abruptly and implemented within a short period, there should have been many parents who were not ready for it. For this reason, especially among working mothers who could not find any support for their children, there should have been many people who began to leave their child (or children) home alone. In Table 2, the mean value of the dependent variable is 7 %, and the sharp-RD estimates suggest that 4.6 to 5.2 % of the increase in the fraction of those who answered yes to the question,“I began to leave my child home alone for a longer period of time (per day)” can be due to the school closure that happened in March. In contrast, the fuzzy RD estimates suggest that if being a preschool child had exactly meant a “full schooling,” the magnitude of the effect of school closures on mothers’ leaving their child home alone would have been 7.3 to 8.4 percentage points.

5.3.2. Empirical results on parents

Note that in the Online Appendix A, we introduced evidence of a sharp increase in Google searches for the word “divorce” on March 2, when the school closure was suddenly announced. Does this lead to a situation in which marital relationships worsened in response to the confusion of the school closure and/or too great of a burden of childcare on parents?.

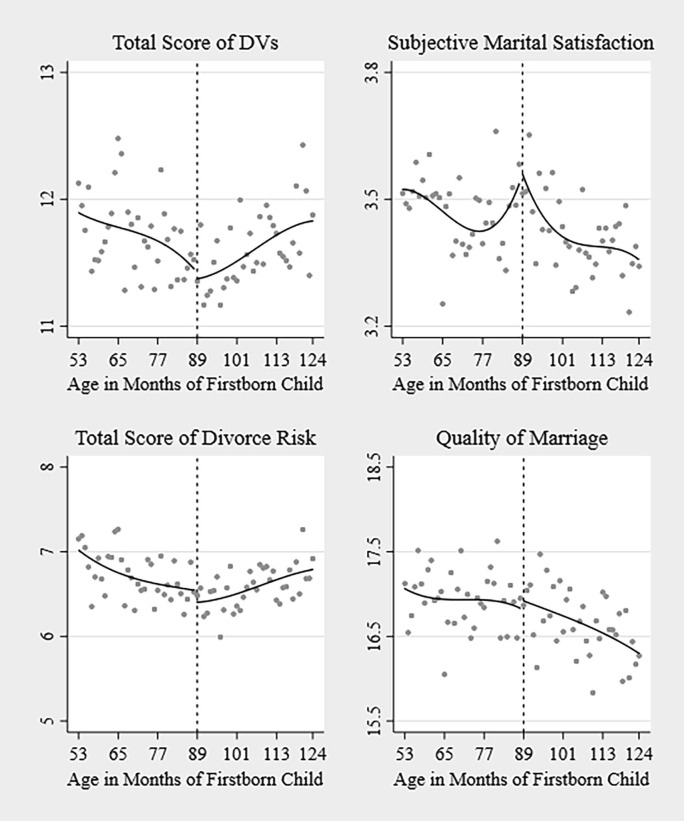
To answer this question, first, we examine the impact on DVs.13 Fig. 3 presents the results of total scores of DV behavior in August. Obviously, the discontinuity at the threshold for this variable is “hard-to-see,” and the scatter plots look very “noisy.” Indeed, according to Table C1 in the Online Appendix, this discontinuity turned out to be statistically insignificant.

Fig. 3.

RD Estimates for the Impact of School Closures on Parents in August. Notes: Observations are averaged within bins using the mimicking variance evenly-spaced method described in Calonico et al. (2015). Each plot includes second-order global polynomial fits represented by the solid lines.

Next, we will see how other measures of marital relationships were affected by the sudden school closure. Fig. 3 also reports the results on several measures of marital relationships, and we do not see clear discontinuities from any measure of marital relationships. The estimation results for these variables are presented in Table C1, and we confirmed that the estimates are all insignificant for these variables.14 Note that although the bias-corrected RD estimate of the impact on subjective marital satisfaction is close to zero, it should not be characterized as precisely zero, because the standard error is too large to rule out economically significant effects. The same trend can be seen for the other estimates in Table C1 as well.

Thus, although in Fig. 3, all the discontinuities at the threshold seem very tiny or negligible, indeed, we have confirmed that these “hard-to-see” or “invisible” gaps in the figures are truly statistically insignificant by Table C1. Thus, we have not obtained any significant results in marital relationship measures.

There might be a concern that the timing of the survey was too late to capture the impact on these measures. Thus, we also asked about situations related to marital relationship in March for each variable except for “Quality of Marriage Index,” which results in the March result being missed. Table C2 reports the comparison of impacts on DVs between August and March. As can be confirmed from the table, we can see the increase in the mean value of the dependent variable in March, but we do not observe any statistically significant results. Although due to the limited space, we do not include the comparison between March and August for other variables, and other measures of marital relationships also show a similar pattern, that is, we do not see any statistically significant results even in March.

5.3.3. Robustness check

In Online Appendix D, we present some robustness checks. First, in Table D1, we report results with local-quadratic specification. Second, in Table D2, we report results with another bandwidth selector type that focuses on delivering confidence intervals with optimal coverage error rates proposed by Calonico et al. (2018). From both robustness checks, we can confirm that our main results have been preserved, which indicates how robust our main results are.

6. Sub-sample analysis

In this subsection, by utilizing rich individual-level information included in our survey, we will explore a sub-sample analysis of children’s outcomes. We first focus on children who had the greatest potential to be negatively affected by the COVID-19 pandemic (Bacher-Hicks et al., 2020, Chetty et al., 2020, Adams-Prassl et al., 2020) —that is, those with mothers and fathers with low educational attainment. In this sub-sample analysis, mothers and fathers are categorized into the “high” education group if they graduated from college, and otherwise into the “low” group. Next, we explore the heterogeneity based on working status as of February 2020 and availability of informal support from grandparents as of February 2020 because sudden school closure may have detrimental effects on families with low availability of alternative childcare resources—for example, dual-income couples whose parents did not live nearby.

Finally, we additionally split the sample according to (1) prefecture of residence, (2) mother’s age, (3) gender of the child, and (4) the number of sibling(s). Out of (1) prefecture of residence, we constructed two groups according to whether the respondents lived in one of the seven prefectures where the state of emergency was declared proactively on April 7 because of the rapid spread of COVID-19. While elementary schools were closed nationwide, the local spread of COVID-19 may have affected how they coped with new daily life. For example, children in low infection regions could play together during March and April, but those in high infection regions could not, so they had to play alone, and the childcare burden, especially for mothers, might have been enormous.

Subsample results on changes in children due to the COVID-19 outbreak are reported in Fig. E1. In Fig. E1(a), we find a significant increase in home-alone hours among two-income households and households with boys. While the coefficient is statistically significant among households with children’s grandparents living nearby, the point estimates do not differ substantially by the grandparents’ proximity. In Fig. E1(b), we find suggestive evidence that the extent of children’s weight gain due to the COVID-19 outbreak differs according to the educational attainment of the fathers. When fathers have graduated from college, the fuzzy RD estimate is smaller by about 5 percentage points than that for children with non-college-educated fathers. This directly suggests that school closures have negative effects on children’s health, especially among those from low socioeconomic backgrounds. In addition to this, we found strong adverse effects among children without support from grandparents and without sibling(s). While there may be numerous stories to account for these findings consistently, one possibility is that school closure made children much more physically inactive when they had no close relatives. In fact, during March and April, it was generally difficult for children to meet and play with non-relatives so that the absence of relatives, especially siblings, leads to weight gain through a sharp reduction of physical activities.

Finally, we found a large increase in childcare anxiety measured by the item “I began to worry about how to raise my child more frequently” among mothers who lived in the seven prefectures with a high infection rate. On the subsample results on other outcome variables such as DVs and QMI, see Online Appendix E.

7. Conclusions

This study provides the first evidence in a comprehensive study design of how school closures without a strong lockdown policy affected children and parents. Unlike countries that implemented lockdown, the Japanese government did not implement strong anti-COVID-19 policies except for school closures. In addition to this, our research design enables us to compare the two groups of children and parents who faced totally different statuses of school closures even with a very small difference in the timing of the children’s birth. Due to the very small difference in the timing of their birth, they are likely to have similar characteristics and experiences of the same alternative anti-COVID-19 policies. Thus, this study successfully estimates the pure impacts of school closure on comprehensive outcome variables related to families.

As the most pronounced results, we have found a clear increase in children’s body weight, time spent home alone by children, and mothers’ worrying over how to raise their children. Quantitative impacts are also sizable: The fraction of mothers whose child (children) gained weight increased by 14.4 to 15.4 percentage points and mothers’ worrying over how to raise their children increased by 17.8 to 20.2 percentage points. Regarding the increase in body weight, the effects were prominent among children from low socioeconomic backgrounds.

Overall, this study implies that the school closure increased time spent home alone, and the reduction in physical activities might directly have resulted in the large weight gain among children. Furthermore, because of these negative effects on children, mothers began to worry about how to raise their children more frequently, which may lead to further deterioration of a healthy parent–child relationship in the long run.

Concerning the current policy debate on school closure, this paper provides clear insights on what we should know before we close schools during a pandemic. First, given the results presented in this paper, school closure may have unexpected side effects on non-academic health outcomes (i.e., weight gain). It is obvious that every day walking on school roads with friends itself is an exercise and that children are naturally kept away from eating unhealthy snacks by schools. School meal programs generally provide children with well-balanced meals. As a result of the sudden loss of these in their daily lives, many children experienced weight gain. Even if online education could offer a complete substitute for real in-person education in the future, in an academic sense, it should not be ignored that schools do not solely give academic education to children, but contribute to children’s healthy lives. Therefore, this aspect of real in-person schooling should not be ignored. Given that some epidemiological studies consistently find that school closure is not an effective tool to control COVID-19 (Armbruster and Klotzbücher, 2020, Iwata et al., 2020), we should pay closer attention to the adverse side effects of school closures carefully.

Second, if we have to close schools again due to the overwhelming spread of COVID-19, schools should provide families with adequate online education as well as guidelines on how children and parents can spend their time at home in more healthy and productive ways. Throughout the school closure during March and April, many parents in Japan worried about their relationships with their children because elementary schools did nothing except provide homework. It was really surprising that during March and April 2020, interactive online learning was provided in only five percent of all schools in Japan (MEXT, 2020a). Consistent with a lack of policy to support children’s education, our results show that school closure had negative effects on mothers’ worrying about how to raise their children. While this should be confirmed carefully, the deterioration of the mother–child relationship might have been alleviated if policymakers had provided much more effective measures to compensate for the sudden stop of schooling.

We hope to examine effects on children from other perspectives as well. For example, high-quality schools (Dobbie and Fryer, 2011) and intensive compulsory education (Kawaguchi, 2016) both contribute to equalizing the academic performance of children from different socioeconomic backgrounds; thus there is a possibility that this school closure may lead to the widening of the inequality of academic performance of children and hence the inequality of their future economic outcomes. To uncover these long run effects is left to future studies.

8. Declaration of Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Supplementary material

The following are the Supplementary data to this article: