Abstract
Nonlinear optical effects in layered two-dimensional transition metal chalcogenides have been extensively explored recently because of the promising prospect of the nonlinear optical effects for various optoelectronic applications. However, these materials possess sizable bandgaps ranging from visible to ultraviolet region, so the investigation of narrow-bandgap materials remains deficient. Here, we report our comprehensive study on the nonlinear optical processes in palladium diselenide (PdSe2) that has a near-infrared bandgap. Interestingly, this material exhibits a unique thickness-dependent second harmonic generation feature, which is in contrast to other transition metal chalcogenides. Furthermore, the two-photon absorption coefficients of 1–3 layer PdSe2 (β ~ 4.16 × 105, 2.58 × 105, and 1.51 × 105 cm GW−1) are larger by two and three orders of magnitude than that of the conventional two-dimensional materials, and giant modulation depths (αs ~ 32%, 27%, and 24%) were obtained in 1–3 layer PdSe2. Such unique nonlinear optical characteristics make PdSe2 a potential candidate for technological innovations in nonlinear optoelectronic devices.
Subject terms: Materials for optics, Nanoscale materials
Strong nonlinearities in 2D materials can lead to interesting applications in optoelectronics. Here the authors investigate the optical nonlinearity of palladium diselenide, determine the layer dependent two photon absorption efficiency and the saturable absorption modulation depth.
Introduction
Nonlinear optical (NLO) properties play an increasingly significant role in the development of laser technology, optical spectroscopy, and material structure analysis methodologies1–4. Recently, the study of NLO activity in two-dimensional (2D) layered materials (such as graphene, transition metal chalcogenides (TMDCs), and black phosphorus (BP)) has made significant progress5–12. The extraordinary nonlinearities of graphene, including saturable absorption (SA)13,14, optical limiting15 and harmonic generation16, have attracted great interest. However, the inherent properties of graphene, like its zero-bandgap and centrosymmetric crystal structure17, hinder the study of its NLO effects, including the second harmonic generation (SHG) and multiphoton absorption features. On the contrary, BP possesses intrinsic anisotropy, strong light-matter interaction, layer-dependent direct bandgap (0.3–2 eV), and a wide range of adjustable optical response18,19, leading to high SA modulation depths (~27.6% at 400 nm, ~12.4% at 800 nm20) and a large two photon absorption (TPA) coefficient ((−6.98 ± 0.6) × 103 cm GW−1 21). However, BP lacks sufficient air stability, resulting in the rapid degradation of its NLO properties. Meanwhile, the most studied TMDC materials (MX2, M = Mo, W, and X = S, Se, Te) that exhibit various fascinating NLO activities, such as SHG, SA and TPA, have sizable bandgaps ranging from visible to the ultraviolet region (1–2.5 eV) and there is a shortage of narrow-bandgap materials22. Interestingly, PdSe2 is a pentagonal 2D material with robust anisotropy, high carrier mobility, and air stability, that give it considerable advantages in nonlinear optoelectronic devices23. In addition, PdSe2 has a modifiable thickness-dependent bandgap from 0.03 to 1.37 eV24, filling the interspace between zero-gap graphene and large-gap TMDCs. Meanwhile, Xu et al. demonstrated the ultrafast SA performance of liquid-phase exfoliated PdSe2 that generated the dissipative soliton and pulse energy25. Zhang et al. experimentally investigated the mode-locked Er and Yb-doped operations in multilayer PdSe226. These works demonstrate the capacity of PdSe2 for broadband ultrafast photonic devices. However, to date, the current experimental investigation of the NLO activities of PdSe2 (especially the monolayer sample) is insufficient. Therefore, an extensive and in-depth study of the NLO behavior of PdSe2 is of great significance for the expansion of its optoelectronic applications.
Herein, we report the fabrication of large-size PdSe2 flakes with different layers and present a comprehensive investigation of thickness-dependent NLO properties of the PdSe2 flakes. Extraordinarily, owing to its unique layer-dependent inversion symmetry, PdSe2 exhibits a SHG behavior that is totally opposed to that in other 2D materials27,28. Moreover, the PdSe2 flakes exhibit giant TPA coefficients (β) that are two to three orders of magnitude larger compared to that of the conventional semiconductors29–31. Importantly, excellent saturable absorption was observed in 1–3 layer (L) PdSe2, with much higher modulation depths (αs) than that of the most other 2D materials32–34. These findings not only provide profound insights into the NLO features of this innovative nanomaterial, but also open an avenue for future nonlinear optoelectronic applications.
Results
Crystal structure and characterization of few-layer PdSe2 flakes
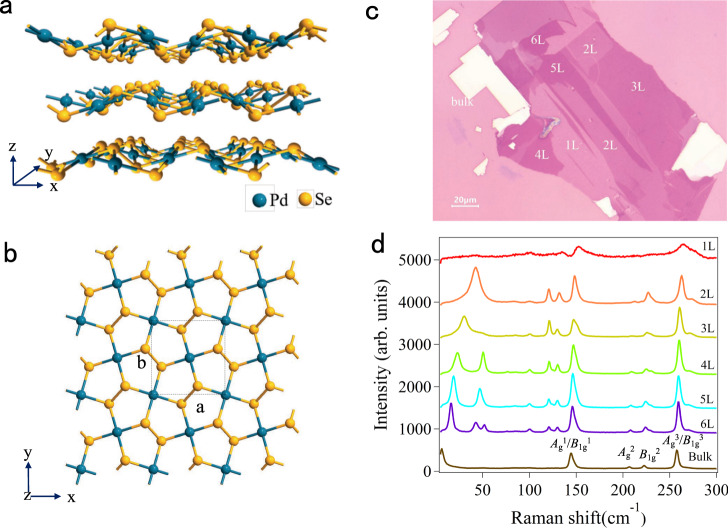
The unique optical characteristics of PdSe2 are correlated to its special structure. It has a stratiform structure that is identical to some of the other 2D materials (graphene, hexagonal boron nitride (hBN), BP, and TMDCs), as shown in Fig. 1a. The Pd and Se atoms combine to form a covalent bond within a monolayer, and the van der Waals (vdWs) force dominates the interactions between adjacent layers. In the side view, PdSe2 exhibits a unique plicate crystal structure with a monolayer vertical plicate height of 1.604 Å. In comparison to the traditional 2D materials with the highly symmetric hexagonal honeycomb structure, the Pd and Se atoms in PdSe2 constitute an asymmetric pentagonal structure (Fig. 1b). The ultrathin PdSe2 crystal displays robust interlayer coupling with a relatively large binding energy of 190 meV/atom35, as manifested in the process of mechanical exfoliation. Monolayer and few-layer PdSe2 flakes are difficult to be obtained by the conventional scotch tape method23,36. Instead, the gold-assisted exfoliation method was adopted to acquire the large-size monolayer and few-layer PdSe2, as shown in Fig. 1c on the Si/SiO2 substrates. The height profiles of the PdSe2 samples were obtained by atomic force microscopy (AFM) measurements (Fig. S1 in Supplementary Information). According to the measurements, the average thickness of a single layer PdSe2 is 0.7 nm. Recently, Raman technology has been demonstrated as an excellent approach in determining the layer number of various 2D materials37–40. Through analyzing the Raman spectrum, the layer number of a thin sample can be identified quickly, precisely and non-destructively. The Raman spectra of the 1–6 L and bulk PdSe2 samples at a wavenumber range of 0–300 cm−1 were obtained to estimate the layer numbers (Fig. 1d). Obviously, the Raman peak position varied gradually as the layer number increased. It is worth mentioning that the Raman peaks in the low-frequency region (0–100 cm−1) varied by a large margin. The low-frequency Raman modes indicate the occurrence of interlayer vibrations in the samples that were robust, and even exceeded those of the high-frequency modes (100–300 cm−1), which were attributed to the strong interlayer coupling of PdSe2. The peak positions of the low-frequency Raman modes have a specific relationship with the layer numbers, as shown in Fig. S2 in Supplementary Information. Because of the significant shifts of the low-frequency Raman modes, the layer number could be determined precisely. The feasibility and specific operation of such approach have been reported in detail in our previous work24.
Fig. 1. Crystallographic structure and optical characteristics of a few-layer PdSe2.
a The lattice structure of PdSe2 flakes with a puckered pentagonal. The unit cell vectors are denoted by the x, y, and z. Blue and yellow globes denote the Pd and Se atoms, respectively. b Top view of the atomic crystal structure of monolayer PdSe2. The black dotted frame represents a unit cell of PdSe2. The a and b denote the lattice constants along the x and y directions, respectively. c The optical microscope image of a few-layer PdSe2 on the silicon substrate with a 285 nm SiO2 layer. The numbers of sample layers containing 1–6 L were marked with white letters, and the scale bar is 20 μm. d Raman spectra of the 1–6 L and bulk PdSe2 flakes with the same intensity axis. The low-frequency Raman peaks (0−100 cm−1) were robust and even exceeded that of the high-frequency modes. The Raman vibration modes of bulk PdSe2 were marked as Ag1, Ag2, Ag3, B1g1, B1g2, and B1g3 mode.
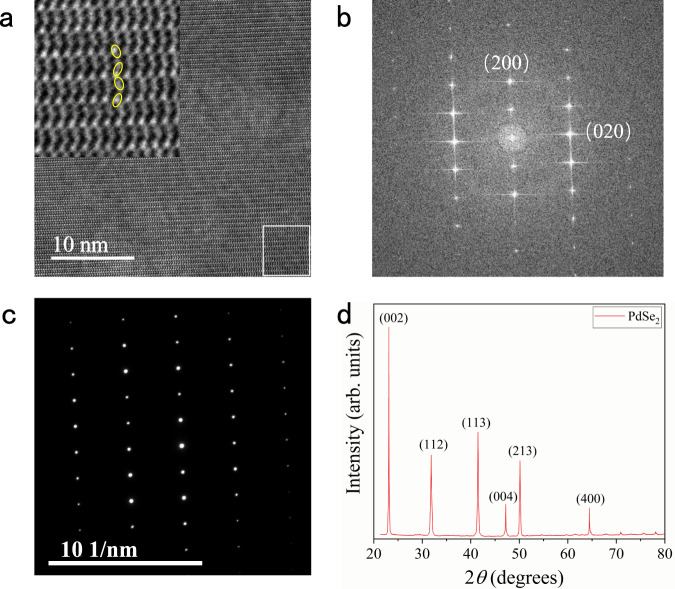
Furthermore, the high-resolution transmission electron microscopy (HRTEM) characterization was performed to reveal the atomic structure of PdSe2. The periodic atomic arrangement of PdSe2 could be distinctly observed, and the sample had a flat surface (Fig. 2a). No obvious defects were observed, indicating high crystallinity of the samples. Figure 2b shows the fast Fourier transform (FFT) diffraction patterns. The distinct diffraction spots correspond to the two principle crystal planes of (200) and (020). The selected-area electron diffraction (SAED) pattern was taken from the few-layer region of PdSe2 (Fig. 2c), indicating the high-quality single-crystal structure and confirming the isometric system of PdSe2. For the further investigation of our experimentally prepared PdSe2, X-ray diffraction (XRD) characterization was performed. As shown in Fig. 2d, the diffraction pattern exhibits six robust peaks at 23.1, 31.8, 41.5, 47.2, 50.14, and 64.4°, corresponding to (002), (112), (113), (004), (213), and (400) atomic planes of PdSe2, demonstrateing the high crystal quality of the sample41.
Fig. 2. TEM and XRD characterizations of PdSe2 flakes.
a HRTEM image of the few-layer region of PdSe2 flakes. The inset shows a zoom-in of the white square regions, revealing the atomic models. The scale is marked in the lower-left corner. b FFT diffraction patterns of the solid white areas highlighted on the image (a). c SAED pattern of the PdSe2. d XRD pattern of bulk PdSe2 is in good agreement with the standard PDF card (PDF#97-017-0327). The diffraction pattern exhibits six robust peaks at 23.1, 31.8, 41.5, 47.2, 50.14, and 64.4°, respectively.
SHG properties of PdSe2 flakes
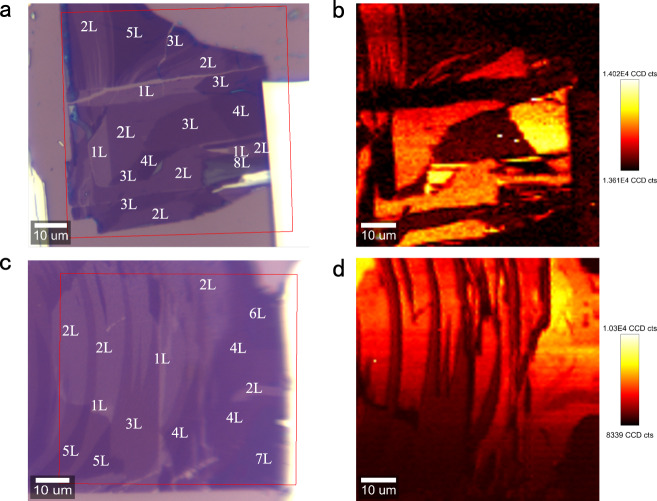
To better understand the NLO characteristics of the PdSe2 flakes, the SHG properties of few-layer PdSe2 at the excitation wavelength of 800 nm were studied in detail. For the monolayer region, the detected SHG signal was negligible. When the laser spot was switched to the bilayer area, strong signals could be observed at 400 nm. In order to further demonstrate the SHG signal dependence on the PdSe2 layer number, the spatial mapping was implemented. The red box in Fig. 3a indicates the scanning regions of the sample, containing 1–5 L, 8 L, and bulk PdSe2 flakes. Clearly, the SHG signals from even-numbered layers of PdSe2 were strong, while the signals from odd-numbered layers and the bulk PdSe2 were almost negligible (Fig. 3b). In order to ensure sufficient sampling, spatial scanning of another sample (consisting of 1–7 L and bulk PdSe2) was carried out and the same results were obtained, as can be seen in Fig. 3c, d. Such characteristics are exactly opposite to the SHG phenomenon displayed by other group VI 2D materials, where a strong SHG signal was only observed from the odd-numbered layers, and no signal was detected from even-numbered layers27,28,42. It is well known that strong SHG signals will occur only in 2D materials with a broken inversion symmetry43. Interestingly, the odd-numbered layers of PdSe2 belong to the C2h (2/m) point group and P21/c (No.14) space group, which have an inversion symmetry, while the even-numbered layers possess a C2v (mm2) point group and Pca21 (No. 29) space group with a broken inversion symmetry23. Meanwhile, the bulk PdSe2 has an orthogonal structure with a D2h point group and Pbca (No. 61) space group with an inversion symmetry23. These characteristics, that lead to the unique layer dependence of the SHG signal of PdSe2 flakes, are very unlike that of the other 2D materials that have an inversion symmetry in the even layers and a broken inversion symmetry in the odd layers42,44. Additionally, it was found that the PdSe2 samples were very uniform in thickness, attributing to the gold-assisted exfoliation method.
Fig. 3. SHG spatial mapping of few-layer PdSe2.
a, c The optical images of the PdSe2 flakes for the SHG characterization. The red boxes indicate the selected area for the SHG measurements. The layer numbers are labeled by the white numbers. The region elected in a contain 1–5 L and 8 L PdSe2, and the area selected in c includes 1–7 L PdSe2, ensuring sufficient sampling. The PdSe2 samples were excited by 800 nm wavelength using 50× objective with a NA value of 0.55. b, d The spatial mapping of the integrated SHG signals corresponding to the regions in a and c, respectively. The intensities of the SHG signals are different for different layers. The SHG signal is almost absent in the odd-layer PdSe2, while it is strong in the even-layer PdSe2. The color bars are shown on the right of the figure.
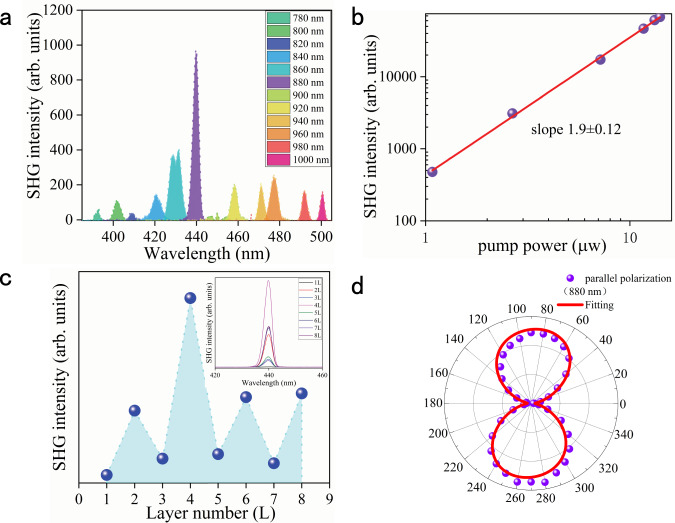
Generally, there are many factors that can influence the SHG intensity of PdSe2, and among those the excitation wavelength is relatively a significant one. To determine the wavelength that could generate the highest SHG signal, the SHG spectra were acquired by changing the excitation wavelengths from 780 to 1000 nm at a step length of 20 nm. As shown in Fig. 4a, there were strong excitation wavelength-dependent optical signals. The highest optical signal was observed under 880 nm excitation, which could be caused by the SHG effect. To explore the physical mechanism under excitation at 880 nm, the correlation between the detected optical intensity and the excitation intensity was measured. As presented in Fig. 4b, it was found that the optical intensity was quadratically dependent on the excitation power, and this was definitely the SHG signal. In addition, when excited with the same wavelength, the layer-dependent SHG signals of PdSe2 were measured. As expected, the odd-numbered layers (1, 3, 5, and 7 L) exhibited very low SHG intensities while the even-numbered layers (2, 4, 6, and 8 L) displayed much stronger SHG signals. The SHG intensities as a function of the layer numbers were plotted in Fig. 4c, and it can be seen that the highest SHG intensity occurred in 4 L PdSe2 (inset). The SHG intensity of PdSe2 is mainly affected by two factors, the asymmetry and absorption intensity28,45. The odd-numbered layers of PdSe2 (1, 3, 5, and 7 L) reserve the symmetry so that no distinct SHG signal is detected. However, as the layer number increased, the signal was weak and non-zero. This was due to the small but finite optical phase shift between the PdSe2 layers28. Additionally, in the even-numbered layers (2, 4, 6, and 8 L) of PdSe2, as the layer number increased, the asymmetry of PdSe2 increased, which caused a strong SHG signal in 4 L PdSe246. However, when the layer number was more than 4 L, the optical absorption intensity in PdSe2 became much stronger, leading to a lower collection efficiency of SHG47. Therefore, influenced by these two factors, the 4 L PdSe2 exhibited the strongest SHG intensity. In general, the NLO process in a crystal can be expressed by the relationship of polarization vector and the electric field of the incident light in the form of1,2:
| 1 |
where is the polarization vector, and is the permittivity in a vacuum, while is the electric susceptibility of the medium, and is the electric field component of the incident light. For the SHG process, the dominated term is . The second-order electric susceptibility, , reflects the SHG efficiency, and for 4 L PdSe2, it was determined to be 5.17 × 10−11 m V−1 at the excitation of 880 nm, according to Eqs. S1–3 in the Supplementary Information (Note S1). The values for different 2D materials are presented in Table S1. It can be seen that the value of PdSe2 is comparable to that of the other 2D materials.
Fig. 4. SHG characterization of the PdSe2 flakes.
a The SHG intensities excited at various wavelengths from 780 to 1000 nm, represented by different colors. The highest intensity appeared at the wavelength of 440 nm, manifesting the strongest SHG signal of excitation at 880 nm. b The excitation power dependence of SHG intensities in 4 L PdSe2. Both axes are shown on the log scale. The correlation of the pump power and SHG intensities exhibits was linear with a slope of 1.9 ± 0.12. c The SHG signal excited at the fixed wavelength of 880 nm in 1–8 L PdSe2. The integrated intensity of SHG varied with different layers, represented by the blue dots. The SHG signal was the strongest in the 4 L sample. The inset represents the SHG intensities versus the layer numbers of PdSe2. d The purple dots indicate the polar plot of integrated SHG intensities excited by the 880 nm laser in 4 L PdSe2. The corresponding fitting result is denoted by the solid red curve.
Besides, the polarization-resolved SHG signals were also acquired, with the aim of identifying the crystal orientation of the sample. In our measurement, the polarization orientations of the incident and detected lights were parallel (for more specific information, see Experimental details). The experimental configuration and the measured parameters of the SHG measurement are illustrated in Fig. S3 in the Supplementary Information. Under a parallel configuration, the polar plot of the SHG signals represented distinct anisotropy, embodied in a dumbbell shape (Fig. 4d). The SHG intensity had its minimum at 0°, then it gradually increased as the polarization orientation rotated, reaching the maximum at 90°. The slight skewing of the polar plot might be resulted from the distorted crystal lattice. The nonlinear dielectric susceptibility tensor was employed to analyze the polarization-resolved SHG signals. The SHG polarization vector, , is related to the electrical field, , of the incident light, and their relationship is expressed as46
| 2 |
where d is the dielectric susceptibility tensor. Under the three-dimensional geometric space, both and have three components in the x, y, and z directions. Likewise, other variables also have a three-dimensional form. Therefore, the above formula can be further expressed as follows
| 3 |
where dij is the element of the dielectric susceptibility tensor. For the experimental backscattering setup, the electrical field intensity of the incident light in the z-direction was negligible. Thus, the electrical field intensities in the x and y directions can be expressed as
| 4 |
where is the module of the electrical field intensity of the incident light, and is the angle between the x-axis of the crystal lattice of PdSe2 and the polarization orientation of the incident light. Under the parallel backscattering configuration, the eventual SHG intensity is only correlated with and , expressed as
| 5 |
where is the detected SHG intensity under the parallel configuration. Substitute Eq. (3) with Eq. (5), we obtained
| 6 |
Using this formula, the experimental SHG intensities were fitted appropriately, as shown by the solid red curve in Fig. 4d. It can be seen that the experimental results are entirely consistent with the theoretical analysis. Meanwhile, the polarization-resolved SHG measurements are expected to accurately identify the crystal orientation of PdSe2 at the macro scale.
TPA properties of PdSe2 flakes
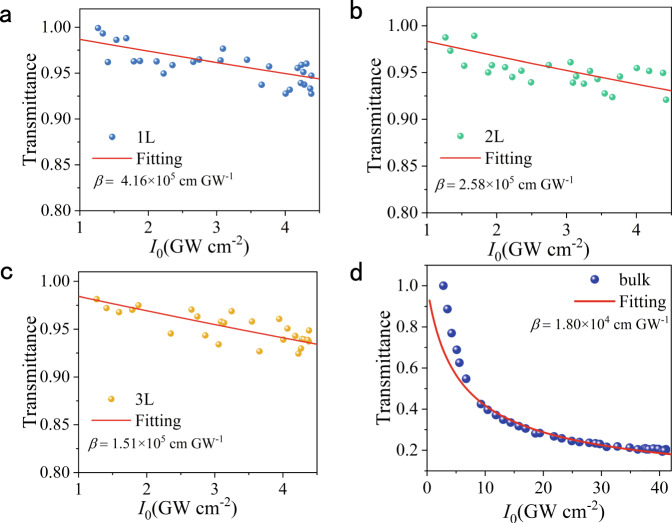
The NLO processes in 2D materials can be divided into two classifications, the parametric and non-parametric process48. The parametric process in 2D materials is mainly a scattering process, where the ground state is excited into a virtual state, and there is no electron transfer and absorption between the two virtual states, such as the SHG above. On the contrary, the non-parametric process is the excitation from the ground state to a real state, which involves absorption and electron transfer, mainly including TPA and SA. To deeply explore the NLO properties of PdSe2, its TPA was further investigated. Figure S4 illustrates the experimental setting of the home-built nonlinear transmittance system used to perform the TPA and SA measurements, together with the measured parameters. To perform the TPA measurement, the monolayer and few-layer PdSe2 flakes, obtained from the bulk crystal by gold-assisted exfoliation, were transferred to the transparent quartz substrates. After that, their TPAs were measured using the nonlinear transmittance method. According to our previous reports, PdSe2 exhibits distinct linear dichroism features under the excitation of 300–800 nm24. When the excitation wavelength was 800 nm, the linear absorption coefficients of 1, 2, and 3 L PdSe2 were estimated to be 3.9 × 103, 1.3 × 104 and 1.9 × 104 cm−1, respectively, according to the linear absorption measurement in Fig. S5 in Supplementary Information. Thus, the linear absorption was almost negligible in the solid film. Consequently, TPA could be predicted when excited by pulses at 800 nm. As intuitively described in Fig. 5, the transmittance of the PdSe2 flakes decreased with the increasing of the incident light intensity, exhibiting a typical TPA characteristic.
Fig. 5. TPA properties of 1–3 L and bulk PdSe2 flakes.
a–c Nonlinear transmittance of 1–3 L PdSe2. The blue, green, and yellow dots represent the experimental data, while the solid red lines show the fitted results using the TPA model. The TPA coefficients (β) of 1, 2, and 3 L PdSe2 are 4.16 × 105, 2.58 × 105, and 1.51 × 105 cm GW−1, respectively. d Nonlinear transmittance of bulk PdSe2. The blue dots represent the experimental results, while the solid red lines show the fitted results using the TPA model. The TPA coefficient (β) of bulk PdSe2 is 1.80 × 104 cm GW−1. All the above measurements were performed under 800 nm excitation.
Based on the nonlinear transmittance method, the TPA coefficients of the samples could be determined by the following formula49:
| 7 |
where is the transmittance of the PdSe2 sample, and is the transmitted light intensity of the sample, while is the incident irradiance intensity and β is the TPA coefficient with being the thickness of the PdSe2 sample. By fitting the experimental data with Eq. (7), the β values of 1, 2, 3 L, and bulk PdSe2 were determined to be 4.16 × 105, 2.58 × 105, 1.51 × 105, and 1.80 × 104 cm GW−1, respectively. These values are two to three orders of magnitude larger compared to those of other conventional semiconductors, such as MoS2, WS2, GaAs, CdS, and ZnO (Table S2 in Supplementary Information)29–31,50, meaning the great potential of the few-layer PdSe2 flakes for TPA-based applications. Such giant TPA coefficients might originate from the strong exciton effects in PdSe251. This was enhanced by the strong dielectric screening owing to the ultrathin structure of the monolayer23,52. In addition, it was found that the TPA coefficient of PdSe2 gradually decreased with the increasing of layers. Such a phenomenon was mainly attributed to the fact that the bandgap decreased with the increase of the number of layers, and there was a certain detuning between the formative exciton and the two-photon energy53.
SA properties of PdSe2 flakes
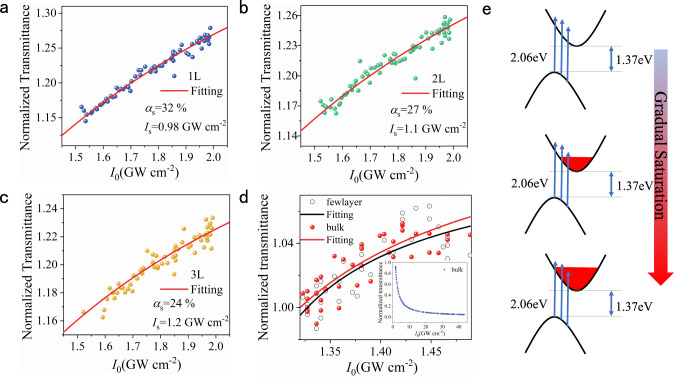
Considering that strong SA was widely reported in various two-dimensional TMDCs, including MoS254, WS255, WSe256, and MoSe257, a typical SA effect could also be expected in our PdSe2 in the case of 600 nm excitation. Figure 6 shows the trends of the normalized transmittance versus the incident intensity in 1–3 L and bulk PdSe2. The fitting could be performed according to a standard model of SA, as shown in the following formula58:
| 8 |
where is the modulation depth, and is the saturable intensity, while is the unsaturated absorption component. The obtained fitting parameters of the modulation depths (αs) of 1–3 L PdSe2 were 32%, 27% and 24%, respectively. The saturable intensities (usually the half of the highest optical absorption) of 1–3 L PdSe2 were 0.98, 1.1, and 1.2 GW cm−2, respectively. As the sample thickened, the saturable intensity gradually increased. The modulation depths are much higher than that of the most other 2D materials, such as SnSe32, MoSe233, MoS234, and PtSe259, which are promising for the application in ultrafast lasers. The high modulation depths mainly resulted from the low unsaturated loss, indicating a low defect density in the PdSe27. However, the modulation depths in (thinner) bulk and few-layer PdSe2 (more than 10 L, only 2.8%) were much lower than that in 1–3 L PdSe2, which might be attributed to the increased unsaturated loss induced by the strengthened scattering7. It is worth noting that there was no SA phenomenon for the thicker bulk PdSe2 since when the sample was thick enough, the light capacity was sufficient, and the saturation state would not be easily reached. The excellent SA property of 1–3 L PdSe2 endows its application prospect in the field of ultrafast lasers.
Fig. 6. SA properties of 1–3 L and bulk PdSe2 flakes.
a–c Nonlinear transmittance of 1–3 L PdSe2. The blue, green, and yellow dots represent the experimental data, while the solid red lines show the fitted results using the SA model. The SA intensities of 1, 2, and 3 L PdSe2 are 0.98, 1.1, and 1.2 GW cm−2, respectively. The modulation depths of 1, 2, and 3 L PdSe2 are 32%, 27%, and 24%, respectively. d Nonlinear transmittance of the bulk PdSe2. The white and red dots represent the experimental data, while the black and red solid lines show the fitting curves of few-layer and bulk PdSe2 using the SA model. The inset shows a reverse SA trend of the thick bulk PdSe2 with increased laser power. e The electronic transition process of SA. All the above measurements were performed under 600 nm excitation.
To understand the principle of the transmittance dependence on the incident irradiance, the electronic transition process in the momentum space should be clear. Figure 6e illustrates the electronic transition process of SA in PdSe2. The bandgap of the monolayer (bulk) PdSe2 was determined to be 1.37 (0.03) eV (Fig. S6 in Supplementary Information), indicating the unusual indirect bandgap characteristics. In our case, the energy of the incident photon (2.06 eV) was much higher than the bandgap of a few-layer PdSe2. For low excitation intensity, the electrons in the valence band jumped to the relatively high energy levels in the conduction band, as illustrated on the top panel of Fig. 6e. Then, the hot electrons relaxed to the lower energy band, and populated in the bottom of the conduction band. With the increase in incident optical intensity, the number of excited electrons also increased. Electron is a fermion, that cannot populate at the same energy level. Thus, the energy levels were gradually filled from the bottom to the top in the conduction band (middle panel in Fig. 6e). When the energy level of the conduction band was filled with electrons as the incident light intensity increased, the electronic transition process was impeded owing to the Pauli exclusion principle. The number of the absorbed photons then gradually approached saturation, as indicated on the bottom panel of Fig. 6e. This explains the gradually saturable transmittance when the incident optical intensity was progressively increased.
In summary, large-area PdSe2 flakes with different thicknesses were successfully fabricated on the Si/SiO2 and quartz substrates. Significantly, in contrast to that of the other 2D materials, robust broadband SHG properties in the even-numbered layers of PdSe2 were demonstrated, as a result of the unique anisotropic puckered pentagonal structure of PdSe2. Besides, the TPA and SA characteristics of the PdSe2 flakes were investigated. Under the excitation at 800 nm, 1–3 L and bulk PdSe2 exhibited giant TPA coefficients (β) of 4.16 × 105, 2.58 × 105, 1.51 × 105, and 1.80 × 104 cm GW−1, respectively, which are at least two orders of magnitude larger than those of the conventional semiconductors. Interestingly, when the excitation was switched to 600 nm, on account of the ultra-low unsaturable loss, strong SA with the modulation depths (αs) up to 32%, 27% and 24% for 1–3 L PdSe2 were observed. Compared to those of the other traditional 2D materials, these values are exceptionally large. Such prominent NLO activity of 2D PdSe2 provides great prospects for potential applications in optical switching, ultrafast lasers, saturation absorbers, optical limiters, and micro/nano optical modulation devices.
Methods
Sample preparation
The PdSe2 flakes were exfoliated from bulk single-crystal PdSe2 (HQ graphene) onto silicon and transparent quartz substrates, employing the gold-assisted exfoliation. The thicknesses of the 1, 2, 3 L, and bulk PdSe2 were 0.7, 1.4, 2.1, and 10.5 nm, respectively.
Raman single-spectrum characterization
A WITec Alpha 300 R was utilized to obtain the high-resolution spectra. A 532 nm laser was used to excite the PdSe2 flakes with a power of 0.25 mW, a 100× objective with NA = 0.9, and a laser spot size of 1 μm. The integration time was 5 s with accumulated ten times to obtain a high signal-to-noise ratio. The 1800 g mm−1 optical grating was used to improve the spectral resolution.
SHG measurements and characterization
The reflective geometry with normal incidence excitation was used to achieve the SHG measurements. The ultrashort 80 fs pulses with a 80 MHz repetition rate (Spectra-Physics Solstice Maitai HP) were used as the excitation source for the SHG measurements. For SHG mapping characterization, the regions of 40 × 40 μm2 and 50 × 50 μm2 containing 1–8 L PdSe2 flakes were selected. A fiber-based pulsed laser was used as the fundamental pump source with the center wavelength at 800 nm. The laser pulses were focused onto a spot size of 6 μm on the PdSe2 sample by a 50× objective with NA = 0.55. The same objective lens was used to collect the SHG signal scattered from the sample and then detected by the charged coupling device (CCD) spectrometer. The integration time of 0.5 s and the scan duration of 2 h was set to achieve the high-resolution mapping images.
For the measurements of layer number dependent SHG signal, the excitation wavelength was fixed at 880 nm, and the same excitation intensity was used. For SHG polarization measurements, a half-wave plate (analyzer) was placed in the incident (outgoing) light path. Only the light with its polarization orientation parallel to the optical axis of the analyzer could pass and be detected by the CCD spectrometer. A step size of 10° was adopted for the parallel configuration.
TPA and SA measurements
The TPA and SA of PdSe2 were measured using the nonlinear transmittance method in a home-built micro NLO system.The samples were excited at the wavelength of 600 nm (spectral width ~15 nm) or 800 nm (spectral width ~10 nm) by the laser pulses from an OPA combined with TOPAS (1000 Hz, 110 fs, Spectra-Physics, Inc.). The fs pulses were focused onto the sample by A 50× objective lens with NA = 0.55.
Supplementary information
Acknowledgements
The authors acknowledge support from the National Natural Science Foundation of China (Grant No. 61775241), the Youth Innovation Team (Grant No:2019012) of CSU, the Science and Technology Innovation Basic Research Project of Shenzhen (Grant No. JCYJ20180307151237242), Hunan Provincial Science Fund for Distinguished Young Scholars (Grant No: 2020JJ2059), and the Hunan province key research and development project (Grant No. 2019GK2233). The authors also are grateful for the support by the Project of State Key Laboratory of High-Performance Complex Manufacturing, Central South University (Grant No. ZZYJKT2020-12). Z.W.L. thanks the support from the Australian Research Council (ARC Discovery Project, DP180102976).
Author contributions
Y.P.L., A.L.P. and T.C.H. designed and managed the project. J.Y. fabricated the device and performed the Raman characterizations. J.Z.L., Z.X.S.Z. and Z.Y.L. performed the nonlinear optical measurements. Y.P.L., J.Y., X.F.K., C.Z., J.H.Z., L.C.C., Z.W.L. and T.C.H. provided a vital interpretation of the data. Y.P.L., T.C.H. and J.Y. drafted the paper. All authors read and contributed to the revising of the manuscript. J.Y., X.F.K. and J.Z.L. contributed equally to this work.
Data availability
All data generated during this study are included in this published article and its supplementary information files. All other relevant data are available from the corresponding authors on request.
Competing interests
The authors declare no competing interests.
Footnotes
Peer review information Nature Communications thanks Shenggui Fu and the other, anonymous, reviewer(s) for their contribution to the peer review of this work.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
These authors contributed equally: Juan Yu, Xiaofei Kuang, Junzi Li.
Contributor Information
Tingchao He, Email: tche@szu.edu.cn.
Anlian Pan, Email: anlian.pan@hnu.edu.cn.
Yanping Liu, Email: liuyanping@csu.edu.cn.
Supplementary information
The online version contains supplementary material available at 10.1038/s41467-021-21267-4.
References
- 1.Levenson M. The principles of nonlinear optics. IEEE J. Quantum Electron. 1985;21:400–400. doi: 10.1109/JQE.1985.1072657. [DOI] [Google Scholar]
- 2.Barry RM, Robert WB. Book review: nonlinear optics, 3rd edn. J. Biomed. Opt. 2009;14:1–4. [Google Scholar]
- 3.Shen YR. Surface properties probed by second-harmonic and sum-frequency generation. Nature. 1989;337:519–525. doi: 10.1038/337519a0. [DOI] [Google Scholar]
- 4.Bloembergen N. Nonlinear optics and spectroscopy. Rev. Mod. Phys. 1982;54:685–695. doi: 10.1103/RevModPhys.54.685. [DOI] [Google Scholar]
- 5.Xia F, Mueller T, Lin Y-m, Valdes-Garcia A, Avouris P. Ultrafast graphene photodetector. Nat. Nanotechnol. 2009;4:839–843. doi: 10.1038/nnano.2009.292. [DOI] [PubMed] [Google Scholar]
- 6.Ju L, et al. Graphene plasmonics for tunable terahertz metamaterials. Nat. Nanotechnol. 2011;6:630–634. doi: 10.1038/nnano.2011.146. [DOI] [PubMed] [Google Scholar]
- 7.Bao Q, et al. Atomic-layer graphene as a saturable absorber for ultrafast pulsed lasers. Adv. Funct. Mater. 2009;19:3077–3083. doi: 10.1002/adfm.200901007. [DOI] [Google Scholar]
- 8.Tsai D-S, et al. Few-layer MoS2 with high broadband photogain and fast optical switching for use in harsh environments. ACS Nano. 2013;7:3905–3911. doi: 10.1021/nn305301b. [DOI] [PubMed] [Google Scholar]
- 9.Liu W, et al. Tungsten diselenide for mode-locked erbium-doped fiber lasers with short pulse duration. Nanotechnology. 2018;29:174002. doi: 10.1088/1361-6528/aaae40. [DOI] [PubMed] [Google Scholar]
- 10.Wang H, Qian X. Giant optical second harmonic generation in two-dimensional multiferroics. Nano Lett. 2017;17:5027–5034. doi: 10.1021/acs.nanolett.7b02268. [DOI] [PubMed] [Google Scholar]
- 11.Zhou F, Ji W. Giant three-photon absorption in monolayer MoS2 and its application in near-infrared photodetection. Laser Photon. Rev. 2017;11:1700021. doi: 10.1002/lpor.201700021. [DOI] [Google Scholar]
- 12.Wang L, et al. Nonlinear optical signatures of the transition from semiconductor to semimetal in PtSe2. Laser Photon. Rev. 2019;13:1900052. doi: 10.1002/lpor.201900052. [DOI] [Google Scholar]
- 13.Sun Z, Hasan T, Ferrari AC. Ultrafast lasers mode-locked by nanotubes and graphene. Phys. E. 2012;44:1082–1091. doi: 10.1016/j.physe.2012.01.012. [DOI] [Google Scholar]
- 14.Martinez A, Sun Z. Nanotube and graphene saturable absorbers for fibre lasers. Nat. Photon. 2013;7:842–845. doi: 10.1038/nphoton.2013.304. [DOI] [Google Scholar]
- 15.Wang J, et al. Graphene and carbon nanotube polymer composites for laser protection. J. Inorg. Organomet. Polym. Mater. 2011;21:736–746. doi: 10.1007/s10904-011-9581-8. [DOI] [Google Scholar]
- 16.Kumar N, et al. Third harmonic generation in graphene and few-layer graphite films. Phys. Rev. B. 2013;87:121406. doi: 10.1103/PhysRevB.87.121406. [DOI] [Google Scholar]
- 17.Nair RR, et al. Fine structure constant defines visual transparency of graphene. Science. 2008;320:1308. doi: 10.1126/science.1156965. [DOI] [PubMed] [Google Scholar]
- 18.Tran V, Soklaski R, Liang Y, Yang L. Layer-controlled band gap and anisotropic excitons in few-layer black phosphorus. Phys. Rev. B. 2014;89:235319. doi: 10.1103/PhysRevB.89.235319. [DOI] [Google Scholar]
- 19.Li L, et al. Direct observation of the layer-dependent electronic structure in phosphorene. Nat. Nanotechnol. 2017;12:21–25. doi: 10.1038/nnano.2016.171. [DOI] [PubMed] [Google Scholar]
- 20.Lu SB, et al. Broadband nonlinear optical response in multi-layer black phosphorus: an emerging infrared and mid-infrared optical material. Opt. Express. 2015;23:11183–11194. doi: 10.1364/OE.23.011183. [DOI] [PubMed] [Google Scholar]
- 21.Chen R, Tang Y, Zheng X, Jiang T. Giant nonlinear absorption and excited carrier dynamics of black phosphorus few-layer nanosheets in broadband spectra. Appl. Opt. 2016;55:10307–10312. doi: 10.1364/AO.55.010307. [DOI] [PubMed] [Google Scholar]
- 22.Mak KF, Shan J. Photonics and optoelectronics of 2D semiconductor transition metal dichalcogenides. Nat. Photon. 2016;10:216–226. doi: 10.1038/nphoton.2015.282. [DOI] [Google Scholar]
- 23.Oyedele AD, et al. PdSe2: Pentagonal two-dimensional layers with high air stability for electronics. J. Am. Chem. Soc. 2017;139:14090–14097. doi: 10.1021/jacs.7b04865. [DOI] [PubMed] [Google Scholar]
- 24.Choi Y, et al. Complete determination of the crystallographic orientation of ReX2 (X = S, Se) by polarized Raman spectroscopy. Nanoscale Horiz. 2020;5:308–315. doi: 10.1039/C9NH00487D. [DOI] [Google Scholar]
- 25.Huanian Z, et al. Palladium selenide as a broadband saturable absorber for ultra-fast photonics. Nanophotonics. 2020;9:2557–2567. doi: 10.1515/nanoph-2020-0116. [DOI] [Google Scholar]
- 26.Nannan X, et al. Palladium diselenide as a direct absorption saturable absorber for ultrafast mode-locked operations: from all anomalous dispersion to all normal dispersion. Nanophotonics. 2020;9:4295–4306. doi: 10.1515/nanoph-2020-0267. [DOI] [Google Scholar]
- 27.Malard LM, Alencar TV, Barboza APM, Mak KF, de Paula AM. Observation of intense second harmonic generation from MoS2 atomic crystals. Phys. Rev. B. 2013;87:201401. doi: 10.1103/PhysRevB.87.201401. [DOI] [Google Scholar]
- 28.Li Y, et al. Probing symmetry properties of few-layer MoS2 and h-BN by optical second-harmonic generation. Nano Lett. 2013;13:3329–3333. doi: 10.1021/nl401561r. [DOI] [PubMed] [Google Scholar]
- 29.Zhang S, et al. Direct observation of degenerate two-photon absorption and its saturation in WS2 and MoS2 monolayer and few-layer films. ACS Nano. 2015;9:7142–7150. doi: 10.1021/acsnano.5b03480. [DOI] [PubMed] [Google Scholar]
- 30.Li Y, et al. Giant two-photon absorption in monolayer MoS2. Laser Photon. Rev. 2015;9:427–434. doi: 10.1002/lpor.201500052. [DOI] [Google Scholar]
- 31.Cirloganu CM, Padilha LA, Fishman DA, Webster S, Hagan DJ, Van Stryland ,EW. Extremely nondegenerate two-photon absorption in direct-gap semiconductors [Invited] Opt. Express. 2011;19:22951–22960. doi: 10.1364/OE.19.022951. [DOI] [PubMed] [Google Scholar]
- 32.Zhang C, et al. Anisotropic nonlinear optical properties of a SnSe flake and a novel perspective for the application of all-optical switching. Adv. Optical Mater. 2019;7:1900631. doi: 10.1002/adom.201900631. [DOI] [Google Scholar]
- 33.Luo Z, et al. Nonlinear optical absorption of few-layer molybdenum diselenide (MoSe2) for passively mode-locked soliton fiber laser [Invited] Photon. Res. 2015;3:A79–A86. doi: 10.1364/PRJ.3.000A79. [DOI] [Google Scholar]
- 34.Luo Z, et al. 1-, 1.5-, and 2-μm fiber lasers Q-switched by a broadband few-layer MoS2 saturable absorber. J. Lightwave Technol. 2014;32:4077–4084. doi: 10.1109/JLT.2014.2362147. [DOI] [Google Scholar]
- 35.Yuan H, et al. Polarization-sensitive broadband photodetector using a black phosphorus vertical p–n junction. Nat. Nanotechnol. 2015;10:707–713. doi: 10.1038/nnano.2015.112. [DOI] [PubMed] [Google Scholar]
- 36.Liang Q, et al. High-performance, room temperature, ultra-broadband photodetectors based on air-stable PdSe2. Adv. Mater. 2019;31:1807609. doi: 10.1002/adma.201807609. [DOI] [PubMed] [Google Scholar]
- 37.O’Brien M, et al. Mapping of low-frequency raman modes in CVD-grown transition metal dichalcogenides: layer number, stacking orientation and resonant effects. Sci. Rep. 2016;6:19476. doi: 10.1038/srep19476. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 38.Ferrari AC, et al. Raman spectrum of graphene and graphene layers. Phys. Rev. Lett. 2006;97:187401. doi: 10.1103/PhysRevLett.97.187401. [DOI] [PubMed] [Google Scholar]
- 39.Yu J, et al. Observation of double indirect interlayer exciton in WSe2/WS2 heterostructure. Opt. Express. 2020;28:13260–13268. doi: 10.1364/OE.392052. [DOI] [PubMed] [Google Scholar]
- 40.Shi W, et al. Raman and photoluminescence spectra of two-dimensional nanocrystallites of monolayer WS2 and WSe2. 2D Mater. 2016;3:025016. doi: 10.1088/2053-1583/3/2/025016. [DOI] [Google Scholar]
- 41.Soulard C, et al. Experimental and theoretical investigation on the relative stability of the PdS2- and pyrite-type structures of PdSe2. Inorg. Chem. 2004;43:1943–1949. doi: 10.1021/ic0352396. [DOI] [PubMed] [Google Scholar]
- 42.Ribeiro-Soares J, et al. Second harmonic generation in WSe2. 2D Mater. 2015;2:045015. doi: 10.1088/2053-1583/2/4/045015. [DOI] [Google Scholar]
- 43.Wang Y, Xiao J, Yang S, Wang Y, Zhang X. Second harmonic generation spectroscopy on two-dimensional materials [Invited] Opt. Mater. Express. 2019;9:1136–1149. doi: 10.1364/OME.9.001136. [DOI] [Google Scholar]
- 44.Mennel L, Paur M, Mueller T. Second harmonic generation in strained transition metal dichalcogenide monolayers: MoS2, MoSe2, WS2, and WSe2. APL Photon. 2018;4:034404. doi: 10.1063/1.5051965. [DOI] [Google Scholar]
- 45.Lin X, et al. Two-dimensional pyramid-like WS2 layered structures for highly efficient edge second-harmonic generation. ACS Nano. 2018;12:689–696. doi: 10.1021/acsnano.7b07823. [DOI] [PubMed] [Google Scholar]
- 46.Song Y, et al. Extraordinary second harmonic generation in ReS2 atomic crystals. ACS Photon. 2018;5:3485–3491. doi: 10.1021/acsphotonics.8b00685. [DOI] [Google Scholar]
- 47.Song Y, et al. Second harmonic generation in atomically thin MoTe2. Adv. Optical Mater. 2018;6:1701334. doi: 10.1002/adom.201701334. [DOI] [Google Scholar]
- 48.Wen X, Gong Z, Li D. Nonlinear optics of two-dimensional transition metal dichalcogenides. InfoMat. 2019;1:317–337. doi: 10.1002/inf2.12024. [DOI] [Google Scholar]
- 49.Qiu X, et al. Strong multiphoton absorption in chiral CdSe/CdS dot/rod nanocrystal-doped poly(vinyl alcohol) films. Opt. Lett. 2019;44:2256–2259. doi: 10.1364/OL.44.002256. [DOI] [PubMed] [Google Scholar]
- 50.Liu W, et al. Giant two-photon absorption and its saturation in 2D organic–inorganic perovskite. Adv. Optical Mater. 2017;5:1601045. doi: 10.1002/adom.201601045. [DOI] [Google Scholar]
- 51.Van Stryland EW, Woodall MA, Vanherzeele H, Soileau MJ. Energy band-gap dependence of two-photon absorption. Opt. Lett. 1985;10:490–492. doi: 10.1364/OL.10.000490. [DOI] [PubMed] [Google Scholar]
- 52.Drüppel M, Deilmann T, Krüger P, Rohlfing M. Diversity of trion states and substrate effects in the optical properties of an MoS2 monolayer. Nat. Commun. 2017;8:2117. doi: 10.1038/s41467-017-02286-6. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 53.Xie Y, et al. Layer-modulated two-photon absorption in MoS2: probing the shift of the excitonic dark state and band-edge. Photon. Res. 2019;7:762–770. doi: 10.1364/PRJ.7.000762. [DOI] [Google Scholar]
- 54.Wang K, et al. Ultrafast saturable absorption of two-dimensional MoS2 nanosheets. ACS Nano. 2013;7:9260–9267. doi: 10.1021/nn403886t. [DOI] [PubMed] [Google Scholar]
- 55.Wei C, et al. Passively Q-switched mid-infrared fluoride fiber laser around 3µm using a tungsten disulfide (WS2) saturable absorber. Laser Phys. Lett. 2016;13:105108. doi: 10.1088/1612-2011/13/10/105108. [DOI] [Google Scholar]
- 56.Bikorimana S, et al. Nonlinear optical responses in two-dimensional transition metal dichalcogenide multilayer: WS2, WSe2, MoS2 and Mo0.5W0.5S2. Opt. Express. 2016;24:26998–26998. doi: 10.1364/OE.24.026998. [DOI] [PubMed] [Google Scholar]
- 57.Chen B, et al. Q-switched fiber laser based on transition metal dichalcogenides MoS2, MoSe2, WS2, and WSe2. Opt. Express. 2015;23:26723–26737. doi: 10.1364/OE.23.026723. [DOI] [PubMed] [Google Scholar]
- 58.Ren C, et al. A near-infrared I emissive dye: toward the application of saturable absorber and multiphoton fluorescence microscopy in the deep-tissue imaging window. Chem. Commun. 2019;55:5111–5114. doi: 10.1039/C9CC02120E. [DOI] [PubMed] [Google Scholar]
- 59.Yuan J, et al. Few-layer platinum diselenide as a new saturable absorber for ultrafast fiber lasers. ACS Appl. Mater. Interfaces. 2018;10:21534–21540. doi: 10.1021/acsami.8b03045. [DOI] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Data Availability Statement
All data generated during this study are included in this published article and its supplementary information files. All other relevant data are available from the corresponding authors on request.