On the Calculation of TCID₅₀ for Quantitation of Virus Infectivity

Dear Editor

The most important property of a virus is its infectivity. To measure infectivity, one can assay viral replication in cells to obtain a titer for a given virus stock. A titer is defined as a given number of infectious viral units per unit volume, and an infectious unit is the smallest amount of virus that produces recognizable effects [e.g., cytopathic effect (CPE), dot blot immunoreactivity]. The median tissue culture infectious dose (TCID₅₀) is defined as the dilution of a virus required to infect 50% of a given cell culture.

Several methods have been developed to calculate the TCID₅₀ including the Spearman–Kärber method (Spearman 1908; Kärber 1931), the Reed–Muench method (Reed and Muench 1938), the improved Kärber method (Sun 1963), the Weil method (Meynell GG and Meynell E 1970), and probit/logit regression models (Finney 1971). In this letter, we analyze datasets using three popular methods for calculating the TCID₅₀ in Excel based calculator (Supplementary file 1). We also compared these results with those of probit/logit regression models and discuss the differences among these methods.

Reed–Muench Method

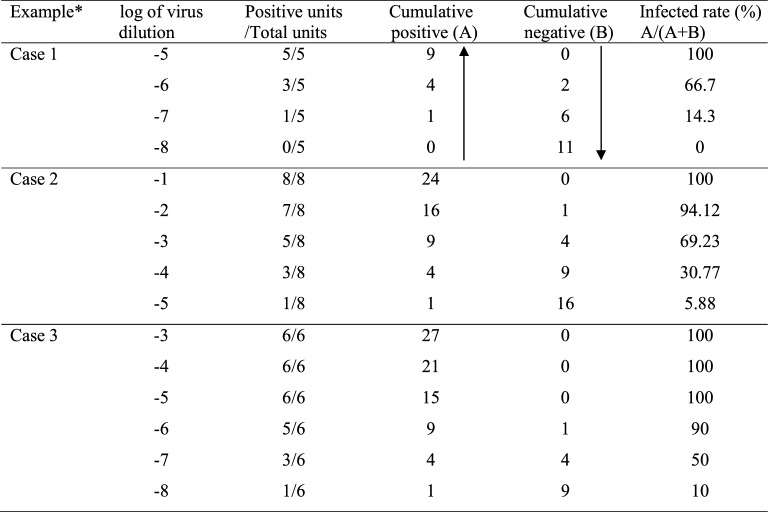
Table 1 shows typical data for titration of virus stocks available in the literature. To calculate the infection rate for case 1, the number of cumulative infected units (‘positive’) was calculated based on the assumption that the test unit that was infected at a 10⁻⁷ dilution of virus would also have been infected at a 10⁻⁶ dilution. Therefore, at 10⁻⁶ dilution of virus there would be 4 cumulative infected units (1 at 10⁻⁷ dilution and 3 at 10⁻⁶ dilution). Similarly, at 10⁻⁵ dilution of virus, the cumulative number of infected units would be 1 (at 10⁻⁷) + 3 (at 10⁻⁶) + 5 (at 10⁻⁵) = 9. The number of cumulative non-infected units was calculated in similar fashion based on the assumption that test units were not infected by a given dilution of virus would also be uninfected by a higher dilution of the virus (Burleson et al. 1992). The infection rate was calculated as:

$$\text{Infection}\text{rate}=\frac{\text{number}\text{of}\text{cumulative}\text{positive}\text{units}}{\text{number}\text{of}\text{cumulative}\text{positive}\text{units}+\text{number}\text{of}\text{cumulative}\text{negative}\text{units}}.$$ 1

Table 1.

Sample datasets used to calculate the 50% endpoint using the Reed–Muench method

^aCase 1 was derived from Table A1 in Burleson et al. (1992); case 2 from Table 2.1 in Hierholzer and Killington (1996); and case 3 from Figure 1 in Svensson et al. (1999)

The dilution corresponding to the 50% endpoint (ID₅₀) lay somewhere between the 10⁻⁶ (66.7% positive) and 10⁻⁷ (14.3% positive) dilutions. The proportionate distance (PD) between these two dilutions is calculated in the following manner:

$$\text{PD}=\frac{\%\text{positive}\text{above}50\%-50\%}{\%\text{positive}\text{above}50\%-\%\text{positive}\text{below}50\%}.$$ 2

For case 1 in Table 1, $\text{PD}=\frac{66.7-50}{66.7-14.3}=0.318$.

We used a compound function in Excel [IF(AND(J6 ≥ 0.5, J7 < 0.5), (J6-0.5)/(J6-J7), ““)] to discriminate the dilutions spanning the ID₅₀ laid and the remaining dilutions were shown as spaces (Supplementary file 1). Then, logID₅₀ = log(dilution with > 50% positive) + PD × (− log(dilution factor)).

For example, in case 1,

$${\text{logID}}_{50}=-6+0.318\times (-1)=-6.318.$$

Then, ID₅₀ = 10^−6.318. This is the end point dilution (i.e., the dilution that would infect 50% of test units inoculated). The reciprocal of this figure is the virus titer in terms of infectious doses per unit volume. If the viral inoculum was 0.1 mL, the titer of the virus stock would be: 1/10^−6.318/0.1 = 10^7.318 (2.08 × 10⁷) TCID₅₀/mL.

Spearman–Kärber Method

When using the Spearman–Kärber method, the following formula can be used to directly estimate the 50% end point (Kärber 1931):

$${\text{logID}}_{50}=log\left(\text{highest}\text{dilution}\text{giving}100\text{\%}\text{CPE}\right)+0.5-\frac{\text{total}\text{number}\text{of}\text{test}\text{units}\text{showing}\text{CPE}}{\text{number}\text{of}\text{test}\text{units}\text{per}\text{dilution}}.$$ 3

Again using the data for case 1,

$${\text{logID}}_{50}=-5+0.5-9/5=-6.3.$$

We also used a compound function in Excel [IF(AND(J25 = 1, J26 < 1), C25,”“)] to identify the highest dilution giving 100% CPE.

Here, the infection rate was calculated as the number of positive units/number of test units per dilution, unlike in Eq. 1 (Supplementary file 1).

The titer, given a viral inoculum of 0.1 mL, is therefore 1/10^−6.3/0.1 = 10^7.3 (2.0 × 10⁷) TCID₅₀/mL.

This result was similar to that obtained using the Reed–Muench method, and in many cases the results using the Spearman–Kärber method are identical to the output of the Reed–Muench method (Table 2).

Table 2.

Comparison of five methods for calculating TCID₅₀/mL

Examplea	Method of calculation (TCID50/mL)
	Reed–Muench	Spearman–Kärber	Modified Kärber (95% CI)	Probit regression (95% CI)	Logit regression (95% CI)
Case 1	2.08 × 107	2.00 × 107	2.00 × 107 (5.57 × 106, 7.15 × 107)	2.05 × 107 (2.89 × 106, 1.22 × 108)	1.98 × 107 (2.12 × 106, 1.91 × 108)
Case 2	3.16 × 104	3.16 × 104	3.16 × 104 (8.42 × 103, 1.19 × 105)	3.40 × 104 (7.54 × 103, 2.01 × 105)	3.42 × 104 (6.77 × 103, 2.31 × 105)
Case 3	2.00 × 108	2.00 × 108	2.00 × 108 (5.24 × 107, 7.63 × 108)	2.00 × 108 (4.10 × 107, 1.00 × 109)	2.01 × 108 (3.70 × 107, 1.17 × 109)

^aThe examples listed in Table 1 were used to calculate TCID₅₀/mL

Improved Kärber Method

Sun (1963) modified the Kärber method by incorporation of Bliss’s weighting method (Bliss 1938) to calculate the median lethal doses of chemicals in animals. This method gives the 50% endpoint as:

$$\begin{array}{cc}\hfill {\text{logID}}_{50}& =log\left(\text{dilution},,\text{giving},,\text{highest},,\text{CPE}\right)-log\left(\text{dilution}\text{factor}\right)\hfill \\ \hfill & \times \left(\Sigma \text{infected}\text{rate}\text{at}\text{each}\text{dilution}-0.5\right).\hfill \\ \hfill \end{array}$$ 4

Adapting his formula to case 1,

$${\text{logID}}_{50}=-5-1\times (1.8-0.5)=-6.300.$$

This result is identical to that using the Spearman–Kärber method, but the improved Kärber method can also provide a 95% confidence interval (95% CI) for the logID₅₀ by calculating its standard error (SE) as:

$$\text{SE}\left({\text{logID}}_{50}\right)=log\left(\text{dilution}\text{factor}\right)\times \sqrt{\sum \frac{p\left(1-p\right)}{n}},$$ 5

where p is the infection rate at each dilution and n is number of test units per dilution.

For case 1, $\text{SE}\left({\text{logID}}_{50}\right)=log\left(10\right)\times \sqrt{0.080}=0.283.$

Then, the 95% CI for logID₅₀ is:

$${\text{logID}}_{50}\pm 1.96\times \text{SE}\left({\text{logID}}_{50}\right).$$ 6

For case 1, the lower boundary of the 95% CI for logID₅₀ was − 6.3 − 1.96 × 0.283 = − 6.854, and the upper boundary was − 6.3 + 1.96 × 0.283 = − 5.746.

The titer, given a viral inoculum of 0.1 mL, was therefore 10^7.3 TCID₅₀/mL, with a 95% CI of 10^6.746–10^7.854 (5.57 × 10⁶, 7.15 × 10⁷) TCID₅₀/mL. Notice that the lower and upper boundaries of the titer (TCID₅₀/mL) corresponding to those of logID₅₀ were converted, as the TCID₅₀/mL was a reciprocal of the ID₅₀.

Using the improved Kärber method, it is not necessary that the lowest dilution (highest concentration of virus) gives 100% CPE (see Eq. 4), unlike the Spearman–Kärber method (see Eq. 3).

Probit/Logit Regression Method

Probit or logit-log(dose) regression is typically used to determine the median lethal doses of chemicals in animals (Finney 1971). These methods can also be used to calculate TCID₅₀ (LaBarre and Lowy 2001).

Defining p as the number of positive units/number of test units per dilution, a linear regression between the probit transition (Φ⁻¹) of p and the logarithm of the dilution can be established as:

$${\Phi }^{-1}\left(p\right)=\alpha +\beta \times log\left(\text{dilution}\right),$$ 7

where α is the intercept and β is the slope of the regression equation.

For the logit regression model, the equation is:

$$ln\left(\frac{p}{1-p}\right)=\alpha +\beta \times log\left(\text{dilution}\right).$$ 8

The above equations can be solved using statistical software such as SPSS (SPSS Inc. 2011) or Polo-Plus (LeOra Software 2007), and log(ID₅₀) with its associated 95% CI can be directly derived from the outputs of these programs.

Probit or logit-log(dilution) regressions yielded TCID₅₀s similar to those obtained using the methods described above. However, the 95% CIs for TCID₅₀ were wider than that given by the improved Kärber method (Table 2). To compare the TCID₅₀ values of two or more viruses on one cell line or of one virus against different cell lines, the potency ratio with 95% CI could be calculated and used to compare viruses or cell lines (Robertson et al. 2017; Lei and Sun 2018).

In conclusion, the Reed–Muench method is the most popular choice to estimate TCID₅₀ and uses a simple interpolation method. The improved Kärber method also gave an accurate TCID₅₀ and 95% CI. Both methods can be easily carried out using widely-available software (e.g., Microsoft Excel) or even by hand. Probit or logit-log(dilution) regression methods are professional statistical procedures and could be used to compare the ability of two or more viruses to infect a single cell line.

Electronic supplementary material

Below is the link to the electronic supplementary material.

Compliance with Ethics Standards

Conflict of interest

The authors declare that they have no conflict of interest.

Human and Animal Rights Statement

This article does not contain any studies with human or animal subjects performed by any of the authors.