Abstract
Density functional theory (DFT) based computation is performed on the endohedrally encapsulated Li3 cluster inside the B40 and C60 cages namely, Li3@B40 and Li3@C60. For both these systems, the Li-Li bond lengths are shorter than that in the free Li3 cluster. Due to confinement, the Li-Li vibrational frequencies increase in both the systems as compared to that in the free Li3 cluster. Thermodynamically, the formation of these two systems is spontaneous in nature as predicted by the negative values of Gibbs’ free energy changes (ΔG). For both the systems one non-nuclear attractor (NNA) is present on the middle of the Li3 cluster which is predicted and confirmed by the electron density analysis. The NNA population and the percentage localization of electron density at the NNA of the Li3@C60 system are higher than that in the Li3@B40 system. At the NNA the values of the Laplacian of electron density are negative and an electron localization function basin is present at the center of the Li3 cluster for localized electrons. Both systems show large values of nonlinear optical properties (NLO). Both the Li3 encapsulated endohedral systems behave as electrides. Electrides have low work function and hence have a great potential in catalytic activity toward the activation of small molecules (such as CO2, N2). Even some electrides have greater catalytic activity than some well-studied metal-loaded catalysts. As the systems under study behave as electrides, they have the power to show catalytic activity and can be used in catalyzing the activation of small molecules.
Keywords: endohedral encapsulation, electride, non-nuclear attractor, electron localization function basin, nonlinear optical properties properties
Introduction
Electrons trapped inside the cavity of some interesting ionic systems behave as anions giving rise to electrides (Dye, 2003; Garcia-Borrà;s et al., 2012; Postils et al., 2015; Zhao et al., 2016; Saha et al., 2019). In recent year’s electride properties of materials have generated great attention in experiments as well as in the theoretical studies. The inception of the concept of an electride took place during the study of the solvated electrons in the solution of alkali metal systems in ammonia (Greenlee and Henne, 1946; Zurek et al., 2009). Dye et al., 1970; Dye, 1990; Dye, 1991; Dye, 1997; Dye, 2003) provided valuable studies on electride materials. The trapped electrons are not attached to any particular atom but located at the cavities and the interstices of cryptands and solid crystals, respectively (Zhao et al., 2016; Dale and Johnson, 2018). The electron density analysis confirmed the presence of confined electrons in the cavities of solid alkali metals (Marqués et al., 2009; Mei et al., 1993). Ellaboudy et al. (1983) synthesized the first stable organic electride, Cs+(18-crown-6)2e–in the crystalline form and Matsuishi et al. (2003) synthesized the first stable inorganic electride, [Ca24Al28O64]4+·(4e–). In both cases, the excess electrons are confined in the empty area of those crystals. This is followed by the synthesis and characterization of six temperature and air-stable electride systems (Ward et al., 1988, Ward et al.,1990a; Ward et al., 1990b; Wagner et al., 1994; Huang et al., 1997; Xie et al., 2000; Redko et al., 2005). In these systems, the cryptand ligands or crown ethers are complexed with alkali metals. Electride materials are very sensitive to temperature and air (Sun et al., 2016). So, it becomes a challenging task to generate and characterize electride materials which are stable related to air and temperature. The presence of cavity trapped loose electrons causes a lowering of the work function of electrides so that they can be used as an electron donor in chemical reactions. Moreover, the presence of loose electrons is responsible in making the electride systems very important because of their potential applications for example the emitting diodes for organic light (Yanagi et al., 2009), reversible hydrogen storage materials (Kitano et al., 2012), catalyst for the CO2 activation (Toda et al., 2013), splitting of N2 molecule (Kitano et al., 2012, 2015; Lu et al., 2016), powerful reducing agents (Buchammagari et al., 2007; Choi et al., 2014; Kim et al., 2014), and superconductivity (Miyakawa et al., 2007). The experimental identification of the position of localized trapped electrons is very difficult because of the low density of these localized electrons. So, experimentalist used indirect evidence for its experimental characterization (Singh et al., 1993; Dye, 1997). Therefore, computational studies can be helpful for the identification of electride materials. For that purpose, people used different computational tools to characterize electride materials. One can in silico characterize a material to behave as an electride and the necessary conditions for the same are, 1) presence of non-nuclear attractors (NNA) of the electron density (Dye, 2003; Lee et al., 2013; Dale et al., 2014); 2) the Laplacian of electron density (∇2 ρ) should be negative at the NNAs; 3) existence of electron localization function (ELF) basin at the NNA region; 4) high values of NLO properties. Some molecules which do not possesses confined electrons in electronic structure can show one or more of the above-mentioned properties. Thus, none of these conditions alone can be used to characterize electride systems, unambiguously. Some previous studies reported some molecules as electride material based on large NLOPs are not considered to be materials with electride properties on these days. When all of these four criteria are simultaneously satisfied, we can say that a cavity-trapped electron is present within the structure of a molecule and it constitutes a real electride material. We will analyze all of the above criteria in detail to check whether they present systems qualify to be termed as electrides. Most recently one theoretical work has shown that binuclear sandwich complexes of Be and Mg atoms bonded with isoelectronic C5H5 −, N5 −, P5 −, As5 − ligands obeyed all these above-mentioned criteria to behave as electride materials (Das and Chattaraj, 2020).
After the discovery of buckminsterfullerene (C60) in 1985, people became interested in using its cavity for the encapsulations of metals, and gas molecules (Kroto et al., 1985). Endohedral fullerenes are very useful in biology (Cagle et al., 1996), in molecular electronics (Jaroš et al., 2019), in nuclear magnetic resonance (NMR) analysis, and in magnetic resonance imaging (Kato et al., 2003). The exterior surface of fullerenes has been used for various chemical reactions to take place (Levitt, 2013). The first experimentally synthesized endohedral fullerene is La@C60 (Heath et al., 1985). Experimentally, Hiroshi et al. reported the endohedral encapsulation of Li+ ion inside the C60 cage (Ueno et al., 2015). Experimentally Li, Ca, Pr, Y, Ba, Ce, Nd, Gd metals (Ding and Yang, 1996; Kubozono et al., 1996; Wan et al., 1998; Okada et al., 2012) and He, Ne, Ar, Kr, Xe noble gases (Saunders et al., 1993; Saunders et al., 1994; Ohtsuki et al., 1998) were kept inside the C60 cage. Using the “molecular surgery” approach, it is experimentally reported for the endohedral encapsulation of H2 (Komatsu et al., 2005; Murata et al., 2008), H2O (Kurotobi and Murata, 2011), HF (Krachmalnicoff et al., 2016), CH4 (Bloodworth et al., 2019) molecules inside fullerene. Several theoretical works have been reported for the encapsulation of different noble gases and metals inside the C60 cage (Andreoni and Curioni, 1996; Bühl et al., 1997; Strenalyuk and Haaland, 2008). Theoretically, Krapp and Frenking have studied the possibility of the encapsulation of noble gas dimers inside the C60 cage and showed the formation of noble gas-noble gas (Ng-Ng) ‘genuine’ chemical bond for Ar, Kr, and Xe, whereas weak interactions are present for He and Ne (Krapp and Frenking, 2007). Theoretically, Khatua et al. (2014a) studied the confinement of HF dimer inside the C60, C70, C80, and C90 cages. Using the ab-initio molecular dynamics study the movement of Ng2 dimer inside the C60 cage has been reported (Khatua et al., 2014b). Recently, endohedral encapsulation of Mg2 molecule inside the C60 cage and the bonding interactions therein have also been studied (Das et al., 2020).
Borospherene, the boron analogue of fullerene has achieved great attention to the scientist. The first reported borospherene is B40 having a cage-like structure (Zhai et al., 2014). After that several borospherenes such as B28, B38, B44, B46, B29 −, B37 −, B38 −, B39 −, B44 −, B39 +, B40 +, B41 +, B42 2+, and their various metal doped homologues have been reported experimentally as well as theoretically (Lv et al., 2014; Chen et al., 2015; Zhao et al., 2015; Li et al., 2016a; Li et al., 2016b; Tai and Nguyen, 2016; Tian et al., 2016; Li et al., 2017; Tai and Nguyen, 2017). Pan et al. (2018) studied the endohedral encapsulation of noble gas monomer and dimer inside the B40 cage and the bonding interactions between Ng-B and Ng-Ng using density functional theory (DFT). Furthermore, the endohedral encapsulation of noble gas dimer inside the cavitand of cucurbit[6]uril and octa acid has been reported (Pan et al., 2015; Chakraborty et al., 2016). Theoretically, Das and Chattaraj (2014) studied the encapsulation of alkali and alkaline earth metals inside an aza crown analogue, [(N4C2H2)4]2- and the bonding interactions therein.
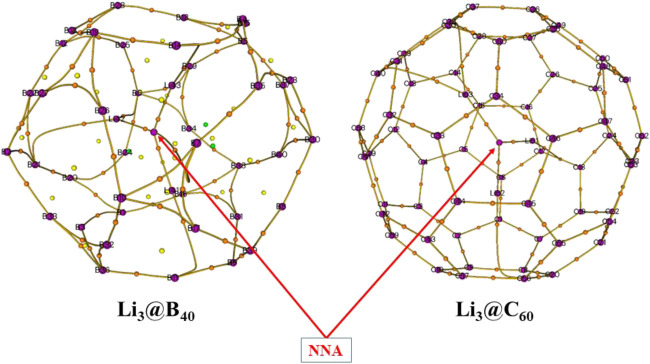
In this article we attempt to analyze molecules with electride property and for that purpose, we have encapsulated the Li3 cluster in two different cages, B40 and C60 and they are denoted as Li3@B40 and Li3@C60. We have used density functional theory (DFT) for the study of the structure, stability, and nature of bonding in these systems. We have computed the Gibbs’ free energy change for the formation of both the electride systems in gas phase as well as in toluene and benzene solvent phases. The molecular orbital analysis and the electron density analysis of both these systems have been performed. Then we have calculated and compared the NLO properties of these systems. Finally, the electride characteristics of these two systems have been analyzed and the same between them have been compared.
Computational Details
We have used BP86-D3/def2-TZVPP method (Perdew, 1986; Becke, 1988; Weigend and Ahlrichs, 2005; Grimme et al., 2010) for geometry optimization and subsequent frequency calculations. The real harmonic frequency values indicate that these are the energy minimum structures on their respective potential energy surfaces. The Gaussian 16 program package has been used for all the computations (Frisch et al., 2016).
The atom centered density matrix propagation (ADMP) simulation has been carried out at BP86/6–31G method to know about the dynamical behavior of our systems at 300 K and 500 K temperatures and 1 atm pressure over 700 fs of time.
We have carried out the natural bond orbital (NBO) analysis to know the charge distribution on each atom. The computation for this analysis has been carried out at BP86-D3/def2-TZVPPD//BP86-D3/def2-TZVPP level of theory using NBO 3.1 version (Reed et al., 1988; Glendening et al., 1990) as implemented in Gaussian 16.
Multiwfn program package (Lu and Chen, 2012) has been used for atoms-in-molecule analysis (AIM) (Bader, 1990) of electron density. We have used BP86-D3/def2-TZVPPD//BP86-D3/def2-TZVPP method for this analysis and various bond critical points (BCP) have been generated. Both AIM and ELF basin population have been analyzed.
For the calculation of the average polarizability (), first hyperpolarizability (), and second hyperpolarizability (), B3LYP/6-31+G(d)/6-31+G//BP86-D3/def2-TZVPP method has been used, where, 6-31+G(d) basis set is used for Li atoms and 6-31+G basis set is used for C and B atoms.
To compute the , and values the following equations have been used (Bishop and Norman, 2001),
Results and Discussion
Geometries and Energetics
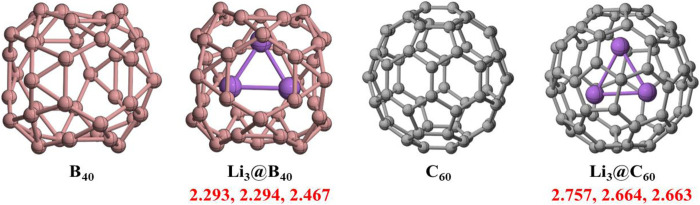
The optimized geometries of B40 and C60 cages and the Li3@B40 and Li3@C60 systems without any symmetry constraint are given in Figure 1. The B40 and C60 cages have D 2d and C 2h point groups of symmetry, respectively, and the Li3@B40 and Li3@C60 systems have C 1 point group of symmetry. The Li-Li bond lengths are 2.293 Å, 2.294 Å, and 2.467 Å for the Li3@B40 system, and for the Li3@C60 system, the Li-Li bond distances are 2.757 Å, 2.663 Å, and 2.664 Å. For both the systems the Li-Li bond lengths are shorter than that in the free Li3 cluster. The confinement effect of B40 and C60 cages can account for this short Li-Li bond length for Li3@B40 and Li3@C60 systems, respectively. For the Li3@B40 system, the Li-Li bond lengths are shorter than that in the Li3@C60 systems. This is because of the comparatively smaller size of the B40 cage than that of the C60 cage. The numerical values of vibrational frequencies of Li-Li bonds for both Li3@B40 and Li3@C60 systems are presented in Supplementary Table S1. From the numerical values of Li-Li vibrational frequencies for both the systems, it has been found that there is an increase in the vibrational frequencies as compared to that in the free Li3 cluster (139.6 cm−1, 185 cm−1, and 301.8 cm−1). Li3@B40 system has higher values of Li-Li vibrational frequency as compared to that of the Li3@C60 systems.
FIGURE 1.
The optimized geometries of B40, C60 cages, and Li3@B40 and Li3@C60 systems at BP86-D3/def2-TZVPP method. The values in red color indicate Li-Li bond lengths (rLi-Li). The bond lengths are in the Å unit.
For these endohedral encapsulation processes the Gibbs’ free energy changes (ΔG) in gas phase are −57.36 kcal/mol and −50.13 kcal/mol for Li3@B40 and Li3@C60 systems, respectively. For Li3@B40 system the ΔG values are −55.33 kcal/mol and −55.26 kcal/mol in toluene and in benzene solvents, respectively. However, for Li3@C60 system the ΔG values are −61.68 kcal/mol and −59.82 kcal/mol in toluene and in benzene solvents, respectively. The ΔG values are computed at BP86-D3/def2-TZVPP level of theory. The negative values of ΔG as shown in Figure 2 indicate the spontaneous formation of these endohedral systems in gas phase as well as in the solvents. So, both the hosts, B40 and C60 cages can hold and stabilize the guest Li3 cluster inside their cavity.
FIGURE 2.
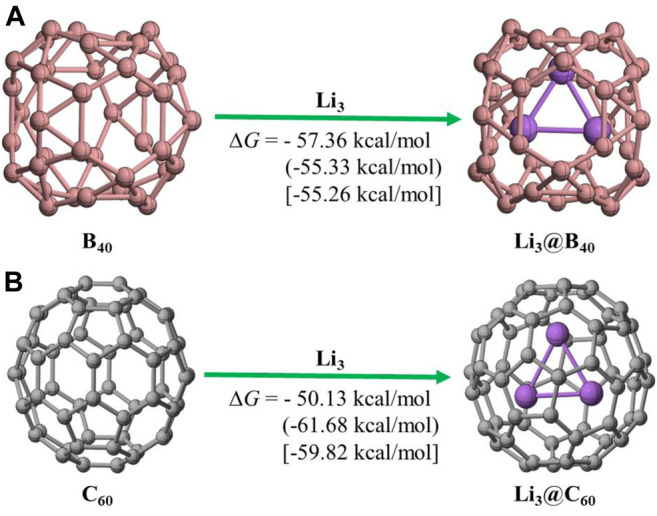
The Schematic representation of the reaction path for (A) Li3@B40; (B) Li3@C60 systems considered in the study. The values without parentheses are calculated ΔG values at gas phase. The values within parentheses and within square brackets are calculated ΔG values at toluene and benzene solvent phases, respectively.
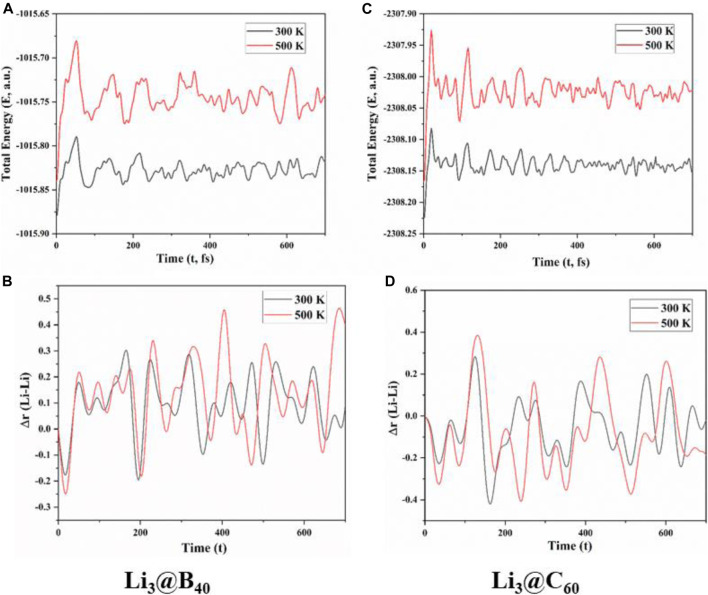
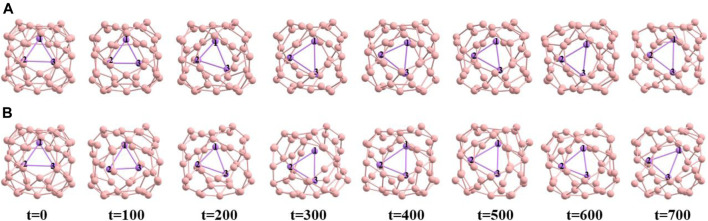
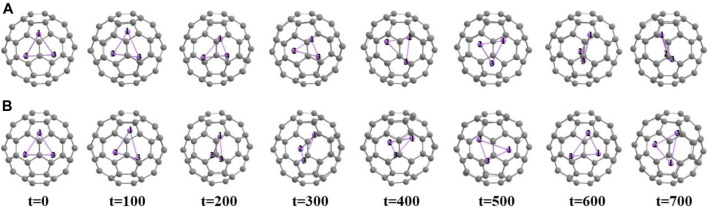
To know about the dynamical behavior of these systems we have carried out ADMP simulation at BP86/6-31G level of theory at both 300 K and 500 K temperature and at 1 atm pressure over 700 fs of time. We have presented the time evolution of the energy plots in Figures 3A,C for Li3@B40 and Li3@C60 systems, respectively. During structural deformation the nuclear kinetic energy of the systems increases which causes the oscillation in the time evolution of energy plots. From the time evolution plot of Li-Li bond length (Figures 3B,D for Li3@B40 and Li3@C60 systems, respectively) it is shown that the Li-Li bond lengths are fluctuating around the corresponding equilibrium values without disintegrating the systems. The different orientations of the Li3 cluster inside the B40 and C60 cages at these temperatures at different time steps are shown in Figures 4, 5, respectively. At both temperatures, the Li3 cluster is only moving inside the cages without breaking the cages. So we can say that these two systems are stable at room temperature as well as at 500 K temperature. So, the guest Li3 cluster can stay inside the B40 and C60 cages.
FIGURE 3.
(A) and (C) Time evolution of total energy for Li3@B40 and Li3@C60 systems, respectively, (B) and (D) change in the Li-Li bond lengths with respect to the corresponding optimized value (at t = 0) against time for Li3@B40 and Li3@C60 systems, respectively.
FIGURE 4.
Snapshots at different time steps (time in fs) (A) at 300 K temperature, (B) at 500 K temperature of Li3@B40 system.
FIGURE 5.
Snapshots at different time steps (time in fs) (A) at 300 K temperature, (B) at 500 K temperature of Li3@C60 system.
Nature of Bonding
Molecular Orbitals
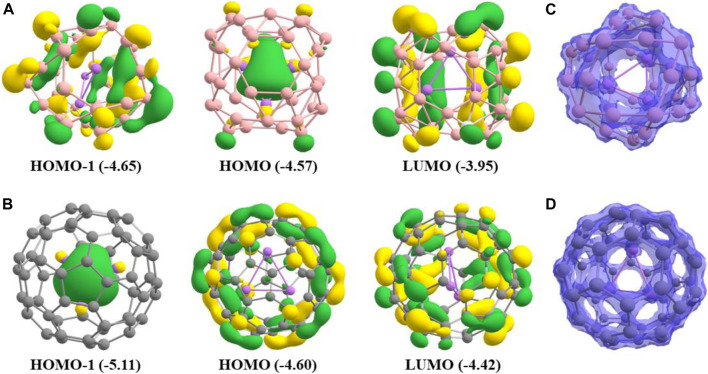
We have used BP86-D3/def2-TZVPP method for molecular orbital analysis of both systems. The highest occupied molecular orbital (HOMO), HOMO-1, and lowest unoccupied molecular orbital (LUMO) for the systems are presented in Figure 6. For the Li3@B40 system, the HOMO-1 and LUMO are distributed over the B40 cage but there is no contribution from the Li3 moiety. For the Li3@C60 system, the HOMO and LUMO are π-type of orbitals and are distributed over the C60 cage but there is no contribution from the Li3 moiety. The energy differences between HOMO and LUMO are 0.62 eV and 0.18 eV for Li3@B40 and Li3@C60 systems, respectively. The spin density plots are presented in Figures 6C,D for Li3@B40 and Li3@C60 system, respectively. The spin density plots show that the total spin density is distributed over the guest Li3 cluster and the host B40 and C60 cages.
FIGURE 6.
Plots of the HOMO-1, HOMO, and LUMO for (A) Li3@B40 system, (B) Li3@C60 system, (C) and (D) are spin density for Li3@B40 and Li3@C60 system, respectively. The values in the parenthesis are the energies of the corresponding orbitals in the eV unit.
Natural Bond Orbital Analysis
The charge distribution over the atoms in both the systems has been analyzed by natural bond orbital analysis. For Li3@C60 system the natural charges on Li atoms are 0.60 |e|, 0.60 |e|, and 0.55 |e|. While the charges on Li atoms are 0.60 |e|, 0.73 |e|, and 0.74 |e| for Li3@B40 system. The charges on the Li atoms are higher in case of Li3@B40 system as compared to that in the Li3@C60 system. In both the systems charge transfer takes place from the guest Li3 cluster to the host B40 and C60 cages. In Li3@B40 system, a greater extent of charge transfer takes place from Li3 to B40 cage as predicted by the greater positive charges on Li atoms for this system. It is reported that for La@C82 system 3 |e| transferred from the La atom to the C82 cage (Bethune et al., 1993). Again, in Sc3N@C80 system charge transfer occurs from Sc3N fragment to C80 cage by 6 |e| unit (Iiduka et al., 2005). But for F2@C60 system the charge transfer occurs in a reverse way i.e. from C60 cage to F2 molecule (Foroutan-Nejad et al., 2018).
Atoms in Molecule Analysis
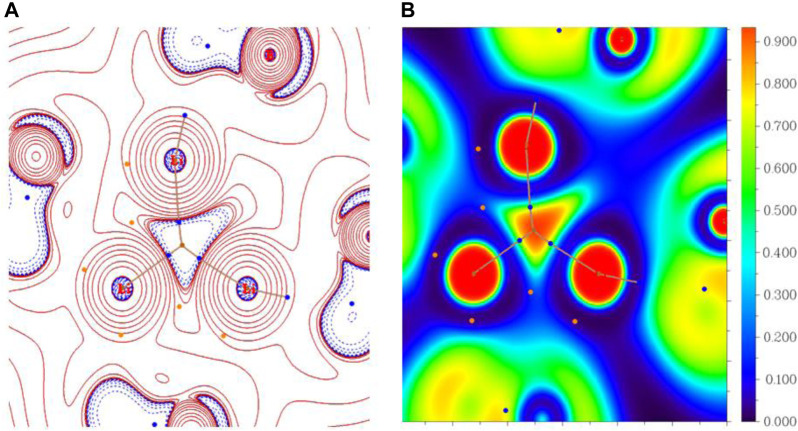
The electron density descriptors of both these systems have been computed at relevant bond critical points (BCPs) and the numerical values are given in Table 1. We have also generated the corresponding molecular graphs for these systems and are presented in Figure 7. From this analysis, it is confirmed that a non-nuclear attractor (NNA) [(3, −3) type of bond critical point] is present at the center of the Li3 cluster for both these systems. The negative values of Laplacian of electron density [∇2 ρ(r c)] at both the NNAs indicate the electron localization therein. We have found that both Li3@B40 and Li3@C60 systems contained three NNA-Li bond paths which are (3,-1) type of bond critical points. The contour plots of ∇2 ρ(r) for both systems are presented in Figure 8A, which indicates a portion of the electron localization at the center of the Li3 cluster. The NNA populations are 0.17 |e| and 0.59 |e| with 12% and 46% localization of electron density for Li3@B40 and Li3@C60 systems, respectively. The population of NNA and the percentage localization of electron density at the NNA for Li3@C60 system is higher than that of the Li3@B40 system. The electron-deficient nature of boron (B) atoms may cause the lowering of percentage of localization of electron density at the NNA for Li3@B40 system as compared to Li3@C60 system, where such an effect is absent. The B40 cage attracts the electron density from the Li3 cluster more toward itself and hence decreases the electron density at the center of the Li3 cluster.
TABLE 1.
Electron Density [ρ(rc)], Laplacian of Electron Density [∇2 ρ(rc)], Kinetic Energy Density [G(rc)], Potential Energy Density [V(rc)], Total Energy Density [H(rc)], Basin Population [N(pop)], Localization Index (LI), Percentage of Localization Index (% LI) at Different Critical Points (CP) of the Li3@B40 and Li3@C60 systems at BP86-D3/def2-TZVPPD//BP86-D3/def2-TZVPP level.
| Systems | CP | Type | ρ(r c) | ▿2 ρ(r c) | G(r c) | V(r c) | H(r c) | N(pop) | LI | %LI |
|---|---|---|---|---|---|---|---|---|---|---|
| Li3@B40 | NNA | (3,−3) | 0.016 | −0.015 | 0.001 | −0.006 | −0.005 | 0.17 | 0.02 | 12 |
| NNA−Li | (3,−1) | 0.015 | −0.004 | 0.003 | −0.008 | −0.005 | ||||
| Li3@C60 | NNA | (3,−3) | 0.018 | −0.018 | 0.000 | −0.005 | −0.005 | 0.59 | 0.27 | 46 |
| NNA−Li | (3,−1) | 0.015 | 0.004 | 0.005 | −0.008 | −0.004 |
FIGURE 7.
The plots of molecular graphs of Li3@B40 and Li3@C60 systems generated at BP86-D3/def2-TZVPPD//BP86-D3/def2-TZVPP level.
FIGURE 8.
The plots of (A) the Laplacian of electron density [∇2 ρ(r)], blue dashed and red solid lines indicate ∇2 ρ(r) < 0 and ∇2 ρ(r) > 0 regions, respectively; (B) the electron localization function (ELF) basin of Li3@B40 and Li3@C60 systems.
We have generated the electron localization function basin (ELF) plots for both the studied systems and are presented in Figure 8B. From the plot, it is shown that a basin is present at the center of the Li3 cluster for both systems. The basin population is 0.58 |e| with 22% localization of electron density for the Li3@B40 system. However, for the Li3@C60 system, the population of the basin is 1.01 |e| with 56% localization of electron density. The ELF basin population of the Li3@C60 system is higher than that of the Li3@B40 system. The lowering of the basin population for the Li3@B40 system is due to the electron-deficient nature of boron (B) atoms. From these results, it can be said that a portion of electron density is localized at the center of the Li3 cluster in both the systems. The higher values of NNA and ELF populations at the center of the Li3 cluster of Li3@C60 system as compared to the Li3@B40 system indicates a greater extent of localization of electron density in the Li3@C60 system.
Nonlinear Optical Property
As electride materials contain loosely bound excess electrons, they showed high values of NLO properties. For this purpose, we have computed average polarizability (), first hyperpolarizability (β), and second hyperpolarizability () for both the systems, and the numerical values are given in Table 2. Among both these systems Li3@C60 system shows higher values of and the Li3@B40 system shows higher values of β and. We have compared the NLO values of our systems with some previously reported known electride materials, for example, M@calix (M = Li, Na; calix = calix [4]pyrrole), Li@B10H14 (Muhammad et al., 2011), and M2 (TCNQ) (Li et al., 2008) (M = Li, Na; TCNQ = Tetracyanoquinodimethane) and are presented in Supplementary Table S2. Our systems show comparatively higher values of but lower values of β than the systems under comparison. The numerical values of of our systems are comparable with that of the systems being compared.
TABLE 2.
Average linear polarizability (), first hyperpolarizability (β), and second hyperpolarizability () of Li3@B40 and Li3@C60 systems.
| NLO property | Li3@B40 | Li3@C60 |
|---|---|---|
| 554.2 | 584.7 | |
| β | 129.4 | 79.9 |
| 3.6 × 105 | 2.1 × 105 |
Electride Properties
It has been observed that in both the systems an NNA is present in the middle of the Li3 cluster. An ELF basin has appeared in the position where the NNA is present and the values of ∇2 ρ are negative therein. Both the systems under study exhibit high values of NLO properties. All the criteria for an electride material have been satisfied by these systems. So, Li3@B40 and Li3@C60 systems can be classified as electrides. Li3@C60 system will show better electride characteristics than the Li3@B40 system.
Summary and Conclusion
The stability of Li3@B40 and Li3@C60 systems has been studied using density functional theory (DFT) based computations. The thermochemical results show the spontaneous formation of both the systems as predicted by the negative values of Gibbs’ free energy change (ΔG). Due to the confinement, the Li-Li bonds in both the systems are shorter than that in the free Li3 cluster and the Li-Li vibrational frequencies are increased on confinement. The Li-Li bonds are shorter in the Li3@B40 system as compared to that in the Li3@C60 system. The numerical values of Li-Li bond vibrational frequencies in the Li3@B40 system are higher than that in the Li3@C60 system. The results from the ADMP simulation showed that the systems are stable both at room temperature (300 K) and at 500 K temperature and 1 atm pressure. So, the host B40 and C60 cages can take the Li3 cluster inside their cavity and stabilize the cluster. The topological analysis of electron density shows the presence of an NNA at the center of the Li3 cluster of both these systems and a portion of electron density gets localized therein. The Laplacian of electron density is negative at the NNAs. Li3@C60 system has higher values of NNA and ELF population than that of Li3@B40 system. Our designed endohedral Li3@B40 and Li3@C60 systems behave as electride. Li3@C60 system shows better electride characteristics than Li3@B40 system. As the systems under study behave as electrides, they have the potential to show catalytic activity.
Acknowledgments
PKC thanks the topic editors, Professors Soumyajit Roy, Sailaja Krishnamurty and Wolfgang Schöfberger for inviting him to contribute to this special issue honoring Professor Sourav Pal on his sixty-fifth birth anniversary. He would also like to thank DST, New Delhi, India for the J. C. Bose National Fellowship, grant number SR/S2/JCB-09/2009. PD thanks to UGC, New Delhi, India for the Research Fellowship.
Data Availability Statement
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
Author Contributions
PD designed the complex systems under study and executed the computational work. He prepared the first draft of the manuscript. PKC supervised the complete work and critically scrutinized the manuscript.
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Supplementary Material
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fchem.2021.638581/full#supplementary-material.
References
- Andreoni W., Curioni A. (1996). Freedom and constraints of a metal atom encapsulated in fullerene cages. Phys. Rev. Lett. 77, 834. 10.1103/PhysRevLett.77.834 [DOI] [PubMed] [Google Scholar]
- Bader R. F. W. (1990). Atoms in molecules. A quantum theory. Oxford, United Kingdom: Oxford University Press; [Google Scholar]
- Becke A. D. (1988). Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A. 38, 3098. 10.1103/PhysRevA.38.3098 [DOI] [PubMed] [Google Scholar]
- Bethune D. S., Johnson R. D., Salem J. R., de Vries M. S., Yannoni C. S. (1993). Atoms in carbon cages: the structure and properties of endohedral fullerenes. Nature 366, 123–128. 10.1038/366123a0 [DOI] [Google Scholar]
- Bishop D. M., Norman P. (2001). Handbook of advanced electronic and photonic materials; Editor Nalwas H. S., San Diego, CA: Academic, Vol. 9, 1–240(.) [Google Scholar]
- Bloodworth S., Sitinova G., Alom S., Vidal S., Bacanu G. R., Elliott S. J., et al. (2019). First synthesis and characterization of CH4@C60 . Angew. Chem. Int. Ed. 58, 5038–5043. 10.1002/anie.201900983 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Buchammagari H., Toda Y., Hirano M., Hosono H., Takeuchi D., Osakada K. (2007). Room temperature-stable electride as a synthetic organic reagent: application to pinacol coupling reaction in aqueous media. Org. Lett. 9, 4287–4289. 10.1021/ol701885p [DOI] [PubMed] [Google Scholar]
- Bühl M., Patchkovskii S., Thiel W. (1997). Interaction energies and NMR chemical shifts of noble gases in C60 . Chem. Phys. Lett. 275, 14–18. 10.1016/s0009-2614(97)00733-1 [DOI] [Google Scholar]
- Cagle D. W., Thrash T. P., Alford M., Chibante L. P. F., Ehrhardt G. J., Wilson L. J. (1996). Synthesis, characterization, and neutron activation of holmium. Metallofullerenes. J. Am. Chem. Soc. 118, 8043–8047. 10.1021/ja960841z [DOI] [Google Scholar]
- Chakraborty D., Pan S., Chattaraj P. K. (2016). Encapsulation of small gas molecules and rare gas atoms inside the octa acid cavitand. Theor. Chem. Acc. 135, 119. 10.1007/s00214-016-1876-y [DOI] [Google Scholar]
- Chen Q., Li W-L., Zhao Y-F., Zhang S-Y., Hu H-S., Bai H., et al. (2015). Experimental and theoretical evidence of an axially chiral borospherene. ACS Nano 9, 754–760. 10.1021/nn506262c [DOI] [PubMed] [Google Scholar]
- Choi S., Kim Y. J., Kim S. M., Yang J. W., Kim S. W., Cho E. J. (2014). Hydrotrifluoromethylation and iodotrifluoromethylation of alkenes and alkynes using an inorganic electride as a radical generator. Nat. Commun. 5, 1038–1046. 10.1038/ncomms5881 [DOI] [PubMed] [Google Scholar]
- Dale S. G., Johnson E. R. (2018). Theoretical descriptors of electrides. J. Phys. Chem. A. 122, 9371–9391. 10.1021/acs.jpca.8b08548 [DOI] [PubMed] [Google Scholar]
- Dale S. G., Otero-de-la-Roza A., Johnson E. R. (2014). Density-functional description of electrides. Phys. Chem. Chem. Phys. 16, 14584–14593. 10.1039/C3CP55533J [DOI] [PubMed] [Google Scholar]
- Das P., Chattaraj P. K. (2020). Electride characteristics of some binuclear sandwich complexes of alkaline earth metals, M2(η5-L)2 (M = Be, Mg; L = C5H5 –, N5 –, P5 –, As5 –). J. Phys. Chem. A. 124, 9801−9810. 10.1021/acs.jpca.0c08306 [DOI] [PubMed] [Google Scholar]
- Das P., Saha R., Chattaraj P. K. (2020). Encapsulation of Mg2 inside a C60 cage forms an electride. J. Comput.Chem. 31, 1645–1653. 10.1002/jcc.26207 [DOI] [PubMed] [Google Scholar]
- Das R., Chattaraj P. K. (2014). Guest–host interaction in an aza crown analog. Int. J. Quan. Chem. 114, 708–719. 10.1002/qua.24648 [DOI] [Google Scholar]
- Ding J., Yang S. (1996). Isolation and characterization of Pr@C82 and Pr2@C80 . J. Am. Chem. Soc. 118, 11254–11257. 10.1021/ja961601m [DOI] [Google Scholar]
- Dye J. L., DeBacker M. G., Dorfman L. M. (1970). Pulse radiolysis studies. XVIII. Spectrum of the solvated electron in the systems ethylenediamine–water and ammonia–water. J. Chem. Phys. 52, 6251–6258. 10.1063/1.1672935 [DOI] [Google Scholar]
- Dye J. L. (1991). Electrides and alkalides-comparison with metal solutions. J. Phys. IV. 1, 259–282. 10.1051/jp4:1991531 [DOI] [Google Scholar]
- Dye J. L. (1997). Electrides: from 1D heisenberg chains to 2D pseudo-metals. Inorg. Chem. 36, 3816–3826. 10.1021/ic970551z [DOI] [Google Scholar]
- Dye J. L. (1990). Electrides: ionic salts with electrons as the anions. Science 247, 663–668. 10.1126/science.247.4943.663 [DOI] [PubMed] [Google Scholar]
- Dye J. L. (2003). Electrons as anions. Science 301, 607–608. 10.1126/science.1088103 [DOI] [PubMed] [Google Scholar]
- Ellaboudy A., Dye J. L., Smith P. B. (1983). Cesium 18-crown-6 compounds. A crystalline ceside and a crystalline electride. J. Am.Chem. Soc. 105, 6490–6491. 10.1021/ja00359a022 [DOI] [Google Scholar]
- Foroutan-Nejad C., Straka M., Fernàndez I., Frenking G. (2018). Buckyball difluoride F2 −@C60 +—a single‐molecule crystal. Angew. Chem. Int. Ed. 57, 13931–13934. 10.1002/anie.201809699 [DOI] [PubMed] [Google Scholar]
- Frisch M. J., Trucks G. W., Schlegel H. B., Scuseria G. E., Robb M. A., Cheeseman J. R., et al. (2016). Gaussian 16, Revision B.01. Wallingford, CT: Gaussian, Inc. [Google Scholar]
- Garcia-Borràs M., Solà M., Luis J. M., Kirtman B. (2012). Electronic and vibrational nonlinear optical properties of five representative electrides. J. Chem. Theor. Comput. 8, 2688–2697. 10.1021/ct300433q [DOI] [PubMed] [Google Scholar]
- Glendening E. D., Reed A. E., Carpenter J. E., Weinhold F. (1990). NBO 3.1 QCPE bulletin, 10, 58 [Google Scholar]
- Greenlee K. W., Henne A. L. (1946). Sodium amide. Inorg. Synth. 2, 128–135. 10.1002/9780470132333.ch38 [DOI] [Google Scholar]
- Grimme S., Antony J., Ehrlich S., Krieg H. (2010). A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 132, 154104. 10.1063/1.3382344 [DOI] [PubMed] [Google Scholar]
- Heath J. R., O’Brien S. C., Zhang Q., Liu Y., Curl R. F., Tittel F. K., et al. (1985). Lanthanum complexes of spheroidal carbon shells. J. Am. Chem. Soc. 107, 7779–7780. 10.1021/ja00311a102 [DOI] [Google Scholar]
- Huang R. H., Wagner M. J., Gilbert D. J., Reidy-Cedergren K. A., Ward D. L., Faber M. K., et al. (1997). Structure and properties of Li+(cryptand [2.1.1])e−, an electride with a 1D “spin-ladder-like” cavity-channel geometry. J. Am. Chem. Soc. 119, 3765–3772. 10.1021/ja9640760 [DOI] [Google Scholar]
- Iiduka Y., Ikenaga O., Sakuraba A., Wakahara T., Tsuchiya T., Maeda Y., et al. (2005). Chemical reactivity of Sc3N@C80 and La2@C80 . J. Am. Chem. Soc. 127, 9956–9957. 10.1021/ja052534b [DOI] [PubMed] [Google Scholar]
- Jaroš A., Bonab E. F., Straka M., Foroutan-Nejad C. (2019). Fullerene-based switching molecular diodes controlled by oriented external electric fields. J. Am. Chem. Soc. 141, 19644–19654. 10.1021/jacs.9b07215 [DOI] [PubMed] [Google Scholar]
- Kato H., Asakura K., Kudo A. (2003). Highly efficient water splitting into H2 and O2 over lanthanum-doped NaTaO3 photocatalysts with high crystallinity and surface nanostructure. J. Am. Chem. Soc. 125, 3082–3089. 10.1021/ja027751g [DOI] [PubMed] [Google Scholar]
- Khatua M., Pan S., Chattaraj P. K. (2014a). Confinement of (HF)2 in Cn(n = 60, 70, 80, 90) cages. Chem. Phys. Lett. 616–617, 49–54. 10.1016/j.cplett.2014.10.025 [DOI] [Google Scholar]
- Khatua M., Pan S., Chattaraj P. K. (2014b). Movement of Ng2 molecules confined in a C60 cage: an ab initio molecular dynamics study. Chem. Phys. Lett. 610–611, 351–356. 10.1016/j.cplett.2014.06.052 [DOI] [Google Scholar]
- Kim Y. J., Kim S. M., Hosono H., Yang J. W., Kim S. W. (2014). The scalable pinacol coupling reaction utilizing the inorganic electride [Ca2N]+·e− as an electron donor. Chem. Comm. 50, 4791–4794. 10.1039/C4CC00802B [DOI] [PubMed] [Google Scholar]
- Kitano M., Inoue Y., Yamazaki Y., Hayashi F., Kanbara S., Matsuishi S., et al. (2012). Ammonia synthesis using a stable electride as an electron donor and reversible hydrogen store. Nat. Chem. 4, 934–940. 10.1038/nchem.1476 [DOI] [PubMed] [Google Scholar]
- Kitano M., Kanbara S., Inoue Y., Kuganathan N., Sushko P. V., Yokoyama T., et al. (2015). Electride support boosts nitrogen dissociation over ruthenium catalyst and shifts the bottleneck in ammonia synthesis. Nat. Commum. 6, 6731–6739. 10.1038/ncomms7731 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Komatsu K., Murata M., Murata Y. (2005). Encapsulation of molecular hydrogen in fullerene C60 by organic synthesis. Science 307, 238–240. 10.1126/science.1106185 [DOI] [PubMed] [Google Scholar]
- Krachmalnicoff A., Bounds R., Mamone S., Alom S., Concistrè M., Meier B., et al. (2016). The dipolar endofullerene HF@C60 . Nat. Chem 8, 953–957. 10.1038/nchem.2563 [DOI] [PubMed] [Google Scholar]
- Krapp A., Frenking G. (2007). Is this a chemical bond? A theoretical study of Ng2@C60 (Ng=He, Ne, Ar, Kr, Xe). Chem. Eur. J. 13, 8256–8270. 10.1002/chem.200700467 [DOI] [PubMed] [Google Scholar]
- Kroto H. W., Heath J. R., Obrien S. C., Curl R. F., Smalley R. E. (1985). C60: buckminsterfullerene. Nature 318, 162–163. 10.1038/318162a0 [DOI] [Google Scholar]
- Kubozono Y., Maeda H., Takabayashi Y., Hiraoka K., Nakai T., Kashino S., et al. (1996). Extractions of Y@C60, Ba@C60, La@C60, Ce@C60, Pr@C60, Nd@C60, and Gd@C60 with aniline. J. Am. Chem. Soc. 118, 6998–6999. 10.1021/ja9612460 [DOI] [Google Scholar]
- Kurotobi K., Murata Y. (2011). A single molecule of water encapsulated in fullerene C60 . Science 333, 613–616. 10.1126/science.1206376 [DOI] [PubMed] [Google Scholar]
- Lee K., Kim S. W., Toda Y., Matsuishi S., Hosono H. (2013). Dicalcium nitride as a two-dimensional electride with an anionic electron layer. Nature 494, 336–340. 10.1038/nature11812 [DOI] [PubMed] [Google Scholar]
- Levitt M. H. (2013). Spectroscopy of light-molecule endofullerenes. Phil Trans. R. Soc. A. 371, 20120429. 10.1098/rsta.2012.0429 [DOI] [PubMed] [Google Scholar]
- Li H-R., Chen Q., Tian X-X., Lu H-G., Zhai H-J., Li S-D. (2016a). Cage-like B40 +: a perfect borospherene monocation. J. Mol. Model. 22, 124. 10.1007/s00894-016-2980-6 [DOI] [PubMed] [Google Scholar]
- Li H. R., Jian T., Li W. L., Miao C. Q., Wang Y. J., Chen Q., et al. (2016). Competition between quasi-planar and cage-like structures in the B29 − cluster: photoelectron spectroscopy and ab initio calculations. Phys. Chem. Chem. Phys. 18, 29147–29155. 10.1039/C6CP05420J [DOI] [PubMed] [Google Scholar]
- Li S., Zhang Z., Long Z., Qin S. (2017). Structures, stabilities and spectral properties of borospherene B44 − and metalloborospherenes MB44 0/− (M = Li, Na, and K). Sci. Rep. 7, 40081. 10.1038/srep40081 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Li Z.-J., Wang F.-F., Li Z.-R., Xu H.-L., Huang X.-R., Wu D., et al. (2008). Large static first and second hyperpolarizabilities dominated by excess electron transition for radical ion pair salts M2˙+TCNQ˙− (M = Li, Na, K). Phys. Chem. Chem. Phys. 11, 402. 10.1039/b809161g [DOI] [PubMed] [Google Scholar]
- Lu T., Chen F. (2012). Multiwfn: a multifunctional wavefunction analyzer. J. Comput. Chem. 33, 580–592. 10.1002/jcc.22885 [DOI] [PubMed] [Google Scholar]
- Lu Y., Li J., Tada T., Toda Y., Ueda S., Yokoyama T., et al. (2016). Water durable electride Y5Si3: electronic structure and catalytic activity for ammonia synthesis. J. Am. Chem. Soc. 138, 3970–3973. 10.1021/jacs.6b00124 [DOI] [PubMed] [Google Scholar]
- Lv J., Wang Y., Zhu L., Ma Y. (2014). B38: an all-boron fullerene analogue. Nanoscale 6, 11692–11696. 10.1039/C4NR01846J [DOI] [PubMed] [Google Scholar]
- Marqués M., Ackland G. J., Lundegaard L. F., Stinton G., Nelmes R. J., McMahon M. I., et al. (2009). Potassium under pressure: a pseudobinary ionic compound. J. Phys. Rev. Lett. 103, 115501. 10.1103/PhysRevLett.103.115501 [DOI] [PubMed] [Google Scholar]
- Matsuishi S., Toda Y., Miyakawa M., Hayashi K., Kamiya T., Hirano M., et al. (2003). High-density electron anions in a nanoporous single crystal: [Ca24Al28O64]4+(4e-). Science 301, 626–629. 10.1126/science.1083842 [DOI] [PubMed] [Google Scholar]
- Mei C., Edgecombe K. E., Smith V. H., Heilingbrunner A. (1993). Topological analysis of the charge density of solids: bcc sodium and lithium. International Journal Quantum Chemistry 48, 287–293. 10.1002/qua.560480503 [DOI] [Google Scholar]
- Miyakawa M., Kim S. W., Hirano M., Kohama Y., Kawaji H., Atake T., et al. (2007). Superconductivity in an inorganic electride 12CaO·7Al2O3:e- . J. Am. Chem. Soc. 129, 7270–7271. 10.1021/ja0724644 [DOI] [PubMed] [Google Scholar]
- Muhammad S., Xu H., Su Z. (2011). Capturing a synergistic effect of a conical push and an inward pull in fluoro derivatives of Li@B10H14 basket: toward a higher vertical ionization potential and nonlinear optical response. J. Phys. Chem. A. 115, 923–931. 10.1021/jp110401f [DOI] [PubMed] [Google Scholar]
- Murata M., Maeda S., Morinaka Y., Murata Y., Komatsu K. (2008). Synthesis and reaction of fullerene C70 encapsulating two molecules of H2. J. Am. Chem. Soc. 130, 15800–15801. 10.1021/ja8076846.Ohtsuki [DOI] [PubMed] [Google Scholar]
- Ohtsuki T., Ohno K., Shiga K., Kawazoe Y., Maruyama Y., Masumoto K. (1998). Insertion of Xe and Kr atoms into C60 and C70 fullerenes and the formation of dimers. Phys. Rev. Lett. 81, 967. 10.1103/PhysRevLett.81.967 [DOI] [Google Scholar]
- Okada H., Komuro T., Sakai T., Matsuo Y., Ono Y., Omote K., et al. (2012). Preparation of endohedral fullerene containing lithium (Li@C60) and isolation as pure hexafluorophosphate salt ([Li+@C60][PF6 −]). RSC Adv. 2, 10624–10631. 10.1039/C2RA21244G [DOI] [Google Scholar]
- Pan S., Ghara M., Kar S., Zarate X., Merino G., Chattaraj P. K. (2018). Noble gas encapsulated B40 cage. Phys. Chem. Chem. Phys. 20, 1953–1963. 10.1039/C7CP07890K [DOI] [PubMed] [Google Scholar]
- Pan S., Mandal S., Chattaraj P. K. (2015). Cucurbit[6]uril: a possible host for noble gas atoms. J. Phys. Chem. B. 119, 10962–10974. 10.1021/acs.jpcb.5b01396 [DOI] [PubMed] [Google Scholar]
- Perdew J. P. (1986). Density-functional approximation for the correlation energy of the inhomogeneous electron gas. Phys. Rev. B. 33, 8822. 10.1103/PhysRevB.33.8822 [DOI] [PubMed] [Google Scholar]
- Postils V., Garcia-Borràs M., Solà M., Luis J. M., Matito E. (2015). On the existence and characterization of molecular electrides. Chem. Commun. 51, 4865–4868. 10.1039/C5CC00215J [DOI] [PubMed] [Google Scholar]
- Redko M. Y., Jackson J. E., Huang R. H., Dye J. L. (2005). Design and synthesis of a thermally stable organic electride. J. Am. Chem. Soc. 127, 12416–12422. 10.1021/ja053216f [DOI] [PubMed] [Google Scholar]
- Reed A. E., Curtiss L. A., Weinhold F. (1988). Intermolecular interactions from a natural bond orbital, donor-acceptor viewpoint. Chem. Rev. 88, 899–926. 10.1021/cr00088a005 [DOI] [Google Scholar]
- Saha R., Das P., Chattaraj P. K. (2019). A complex containing four magnesium atoms and two Mg–Mg bonds behaving as an electride. Eur. J. Inorg. Chem. 2019 (38), 4105–4111. 10.1002/ejic.201900813 [DOI] [Google Scholar]
- Saunders M., Jimenez-Vazquez H. A., Cross R. J., Mroczkowski S., Gross M. L., Giblin D. E., et al. (1994). Incorporation of helium, neon, argon, krypton, and xenon into fullerenes using high pressure. J. Am. Chem. Soc. 116, 2193–2194. 10.1021/ja00084a089 [DOI] [Google Scholar]
- Saunders M., Jiménez-Vázquez H. A., Cross R. J., Poreda R. J. (1993). Stable compounds of helium and neon: He@C60 and Ne@C60 . Science 259, 1428–1430. 10.1126/science.259.5100.1428 [DOI] [PubMed] [Google Scholar]
- Singh D. J., Krakauer H., Haas C., Pickett W. E. (1993). Theoretical determination that electrons act as anions in the electride Cs+ (15-crown-5)2·e- . Nature 365, 39–42. 10.1038/365039a0 [DOI] [Google Scholar]
- Strenalyuk T., Haaland A. (2008). Chemical bonding in the inclusion complex of He in adamantane (He@Adam): the origin of the barrier to dissociation. Chem. Eur. J. 14, 10223–10226. 10.1002/chem.200800715 [DOI] [PubMed] [Google Scholar]
- Sun W. -M., Li X. -H., Li Y., Ni B. -L., Chen J. -H., Li C. -Y., et al. (2016). Theoretical study of the substituent effects on the nonlinear optical properties of a room-temperature- stable organic electride. Chem. Phys. Chem. 17, 3907–3915. 10.1002/cphc.201600970 [DOI] [PubMed] [Google Scholar]
- Tai T. B., Nguyen M. T. (2016). A new chiral boron cluster B44 containing nonagonal holes. Chem. Commun. 52, 1653–1656. 10.1039/C5CC09111J [DOI] [PubMed] [Google Scholar]
- Tai T. B., Nguyen M. T. (2017). Aromatic cage-like B46: existence of the largest decagonal holes in stable atomic clusters. RSC Adv. 7, 22243–22247. 10.1039/C7RA02870A [DOI] [Google Scholar]
- Tian W. J., Chen Q., Li H. R., Yan M., Mu Y. W., Lu H. G., et al. (2016). Saturn-like charge- transfer complexes Li4&B36, Li5&B36 +, and Li6&B36 2+: exohedral metalloborospherenes with a perfect cage-like B36 4− core. Phys. Chem. Chem. Phys. 18, 9922–9926. 10.1039/C6CP01279E [DOI] [PubMed] [Google Scholar]
- Toda Y., Hirayama H., Kuganathan N., Torrisi A., Sushko P. V., Hosono H. (2013). Activation and splitting of carbon dioxide on the surface of an inorganic electride material. Nat. Commun. 4, 2378–2385. 10.1038/ncomms3378 [DOI] [PMC free article] [PubMed] [Google Scholar]
- Ueno H., Nishihara T., Segawa Y., Itami K. (2015). Cycloparaphenylene‐based ionic donor–acceptor supramolecule: isolation and characterization of Li+@C60⊂[10]CPP. Angew. Chem. Int. Ed. 54, 3707. 10.1002/anie.201500544 [DOI] [PubMed] [Google Scholar]
- Wagner M. J., Huang R. H., Eglin J. L., Dye J. L. (1994). An electride with a large six-electron ring. Nature. 368, 726–729. 10.1038/368726a0 [DOI] [Google Scholar]
- Wan S. M., Zhang H. –W., Nakane T., Xu Z., Inakuma M., Shinohara H., et al. (1998). Production, isolation, and electronic properties of missing Fullerenes: Ca@C72 and Ca@C74 . J. Am. Chem. Soc. 120, 6806–6807. 10.1021/ja972478h [DOI] [Google Scholar]
- Ward D. L., Huang R. H., Dye J. L. (1988). Structures of alkalides and electrides. I. Structure of potassium cryptand[2.2.2] electride. Acta Crystallogr. Sect. C: Cryst. Struct. Commun. 44, 1374–1376. 10.1107/S0108270188002847 [DOI] [Google Scholar]
- Ward D. L., Huang R. H., Dye J. L. (1990a). The structures of alkalides and electrides. III. Structure of potassium cryptand[2.2.2] natride. Acta Crystallogr. Sect. C: Cryst. Struct. Commun. 46, 1833–1835. 10.1107/S0108270190001007 [DOI] [Google Scholar]
- Ward D. L., Huang R. H., Dye J. L. (1990b). The structures of alkalides and electrides. V. Structures of caesium bis (15-crown-5) kalide and rubidium bis (15-crown-5) rubidide. Acta Crystallogr. Sect. C: Cryst. Struct. Commun. 46, 1838–1841. 10.1107/S0108270190002293 [DOI] [Google Scholar]
- Weigend F., Ahlrichs R. (2005). Balanced basis sets of split valence, triple zeta valence and quadruple zeta valence quality for H to Rn: design and assessment of accuracy. Phys. Chem. Chem. Phys. 7, 3297–3305. 10.1039/B508541A [DOI] [PubMed] [Google Scholar]
- Xie Q., Huang R. H., Ichimura A. S., Phillips R. C., Pratt W. P., Dye J. L. (2000). Structure and properties of a new electride, Rb+(cryptand- [2.2.2])e− . J. Am. Chem. Soc. 122, 6971–6978. 10.1021/ja9943445 [DOI] [PubMed] [Google Scholar]
- Yanagi H., Kim K.-B., Koizumi I., Kikuchi M., Hiramatsu H., Miyakawa M., et al. (2009). Low Threshold Voltage and Carrier Injection Properties of Inverted Organic Light-Emitting Diodes with [Ca24Al28O64]4+(4e−) Cathode and Cu2−xSe Anode. J. Phys. Chem. C. 113, 18379–18384. 10.1021/jp906386q [DOI] [Google Scholar]
- Zhai H-J., Zhao Y-F., Li W-L., Chen Q., Bai H., Hu H-S., et al. (2014). Observation of an all- boron fullerene. Nat. Chem. 6, 727–731. 10.1038/nchem.1999 [DOI] [PubMed] [Google Scholar]
- Zhao J., Huang X., Shi R., Liu H., Sua Y., King R. B. (2015). B28: the smallest all- boron cage from an ab initio global search. Nanoscale 7, 15086–15090. 10.1039/C5NR04034E [DOI] [PubMed] [Google Scholar]
- Zhao S., Kan E., Li Z. (2016). Electride: from computational characterization to theoretical design. Wires Comput. Mol. Sci. 6, 430–440. 10.1002/wcms.1258 [DOI] [Google Scholar]
- Zurek E., Edwards P. P., Hoffmann R. (2009). A molecular perspective on lithium- ammonia solutions. Angew. Chem. Int. Ed. 48, 8198–8232. 10.1002/anie.200900373 [DOI] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Data Availability Statement
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.