Clear aligner orthodontic therapy of rotated mandibular round-shaped teeth: A finite element study

Abstract

Objective:

To evaluate, using the finite element method, the orthodontic rotational movement of a lower second premolar obtained with clear aligners, analyzing different staging and attachment configurations.

Materials and Methods:

A CAD model including a complete lower dental arch (with element 4.5 mesially rotated 30°) and the corresponding periodontal ligaments, attachments, and aligner was designed and imported to finite element software. Starting from the CAD model, six projects were created to simulate the following therapeutic combinations for correcting element 4.5 position: (1) without attachments, (2) single attachment placed on the buccal surface of element 4.5, (3) three attachments placed on the buccal surfaces of teeth 4.4 to 4.6. For each project, both 1.2° and 3° of aligner activation were considered.

Results:

All the analyzed configurations revealed a clockwise rotation movement of element 4.5 on the horizontal plane. Models with attachments showed a greater tooth displacement pattern than models without attachments. Simulations with attachments and 3° of aligner activation exhibited the best performance concerning tooth movement but registered high stresses in the periodontal ligaments, far from the ideal stress levels able to produce tooth rotational movement.

Conclusions:

The model with a single attachment and 1.2° of aligner activation was the most efficient, followed by the three attachment model with the same degree of activation. Aligner activation should not exceed 1.2° to achieve better control of movement and reasonable stress in periodontal structures.

INTRODUCTION

Despite the widespread use of clear aligner orthodontic therapy (CAT), several concerns still remain regarding the efficiency of these appliances in controlling all the possible orthodontic tooth movements. However, the evolution of thermoplastic materials, a better comprehension of applied biomechanics in conjunction with an increasing number of biomedical studies, have improved CAT reliability.1,2

One reason why this kind of treatment is still under debate could reside in the force transmission mechanisms.3,4 Forces originating from metal wire and bracket interactions are transmitted to tooth structures causing displacement; on the contrary, CAT outcomes are the result of a predetermined mismatch between tooth and aligner, which coincides with the desired position of the tooth.3,5 The final dental position is reached by sequential aligners worn by patient 22 h/d, which progressively reposition the teeth by small amounts.3,5,6 Additionally, several studies demonstrated that auxiliaries such as attachments and elastics are mandatory in CAT to achieve the predicted results.3

Tooth shape could influence the efficiency of CAT7,8 during correction of malalignment due to the geometric interaction between teeth and aligners. Rotation of round-shaped teeth remains one of the less predictable movements in CAT. According to the existing literature,9,10 the least accurate movement during CAT is premolar rotation. The reduced control of orthodontic tooth movement could be related to the lack of interproximal undercuts between premolars, producing an incorrect force distribution. The result is a loss of tracking of tooth surfaces with respect to the aligner shape.

However, the in vivo study of the mechanical perturbation induced by an appliance is quite challenging. An alternative way is to consider the creation of mathematical models to test the effects of interactions between the appliance and the teeth.

According to several authors, the finite element method (FEM) “represents a non-invasive, accurate method that provides quantitative and detailed data regarding the physiological responses occurring in tissues such as the periodontal ligament and the alveolar bone.” FEM is an engineering technique used to calculate stress and deformation developed on a geometric solid submitted to external forces and is widely accepted for medical purposes.11

FEM has been suggested as a solution for complex biomechanical questions and has been applied in several cases in orthodontics in order to assess the center of resistance, various biomechanical aspects of tooth movement, different fixed appliances, anchorage or surgical treatment modalities, debonding, and retention procedures. The reliability of FE analysis is dependent, not only on the loading configuration, but also on the geometry of the structure and the material properties. Experimental validation studies of FE analysis are also encouraged whenever possible.12

The aim of this study was to evaluate, through FEM, the orthodontic rotational movement of a round-shaped tooth with clear aligners, analyzing different staging and attachment configurations.

MATERIALS AND METHODS

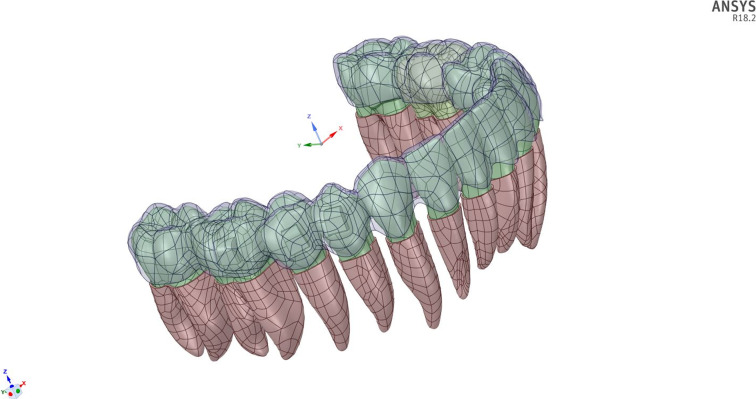
A CAD model of a mandibular arch including the periodontal ligament (PDL), teeth from 3.7 to 4.7 with the right second premolar (element 4.5) mesially rotated 30°, rectangular attachments, and dedicated orthodontic aligners, was designed with CAD software (SpaceClaim Corporation; Canonsburg, PA, USA). After CAD design, all of the components were imported in the FE software (ANSYS 18.2, Inc; Canonsburg, PA, USA) as shown in Figure 1.

Figure 1.

CAD model.

Teeth were designed based on ideal proportions and anatomy while the periodontal ligament (PDL) was modeled on root shape. Aligners were developed making an external offset from all teeth crowns and attachments in the simulations, which included attachments. Afterward, septa between teeth were manually removed and the contour of the aligner was refined to remove edges and undercuts. Plastic aligner thickness was set at 0.5 mm as a result of repeated measurements with a Micro-CT Scan (SkyScan 1172: Bruker-microCT; Kontich, Belgium) of Invisalign aligners (Align Technology, Inc., San Jose, CA, USA). The PDL was modeled with an average thickness of 0.25 mm, according to the scientific literature.13 Attachments were designed as vertical rectangular with 3 mm height, 2 mm width, and 1 mm thickness, with the shape derived from ClinCheck software (Align Technology, Inc., San Jose, CA, USA).

Material Properties (Table 1)

Table 1.

Material Properties

Component	Young's Modulus (MPa)	Poisson's Ratio
Teeth	1.96 × 104	0.30
Attachment	12.5 × 103	0.36
Plastic Aligner	528	0.36
PDL	See reference14	See reference14

^aPDL indicates periodontal ligament.

According to Gomez et al.,5 teeth, attachments, and aligners were considered as isotropic and homogeneous materials. Teeth and attachments were considered as a unique body with rigid stiffness behavior. The PDL was set as a hyperelastic material to simulate its real mechanical characteristics14 as closely as possible. The difference in rigidity between enamel and dentin was not considered relevant for the study and was not set.

Mesh size was set at 0.5 mm for teeth, 0.15 mm for aligners, and 0.1 mm for the PDL. The process of discretization produced a total of 1,280,700 nodes and 1,600,440 linear elements on average. Mesh sizes were defined after a convergence study was performed on a single tooth model.

Bonded contacts were set on the interface between the PDL and teeth, while frictionless contacts were applied between aligners and teeth, according to Barone et al.3 According to Barone et al., to simulate the effects of alveolar bone on teeth and PDL, fixed supports were applied on external surfaces of each periodontal ligament.3

Three experimental models were developed, considering different combinations of attachments:

• No attachments (NO ATT)

• 3 mm vertical rectangular attachment positioned on the buccal crown surface of the rotated right second premolar (ATT 4.5)

• 3 mm vertical rectangular attachments on buccal crown surface, from the right first premolar to the right first molar (ATT 4.4–4.6)

For each model, different amounts of clockwise rotation on the horizontal plane were considered: aligner activations of 1.2° and 3° were analyzed for every model, resulting in a total of six simulations. The 3° value was selected following the recommendations of some clear aligner manufacturers, while 1.2° was the mean aligner activation for tooth rotation according to Simon et al.15

Analyzed Outcomes Included

• Teeth displacement pattern

• Aligner deformation

• Equivalent stress of PDL

• Stress developed on aligner

RESULTS

During lower right premolar rotation, different behaviors and force systems were recorded among the simulations performed. Data for deformation and stress are reported in Tables 2 through 8.

Table 2.

Minimum Tooth Deformation and Location

Teeth Deformation	MIN (mm)	Location	Direction
NO ATT 1.2°	0.0000	Mesial root surface of element 4.3	Lingual
NO ATT 3°	0.0000	Lingual surface of element 4.3	Apical
ATT 4.5 1.2°	0.0000	Mesial root of element 3.7	Distal
ATT 4.5 3°	0.0000	Distal root of element 3.6	Mesial
ATT 4.4–4.6 1.2°	0.0000	Mesial root surface of element 4.3	Apical
ATT 4.4–4.6 3°	0.0000	Distal root of element 3.6	Mesial

Table 3.

Maximum Tooth Deformation and Location

Teeth Deformation	MAX (mm)	Location	Direction
NO ATT 1.2°	0.0123	Buccal surface of element 3.5	Buccal
NO ATT 3°	0.0217	Distal surface of element 4.5	Clockwise
ATT 4.5 1.2°	0.0277	Distal surface of element 4.5	Clockwise
ATT 4.5 3°	0.0499	Distal surface of element 4.5	Clockwise
ATT 4.4–4.6 1.2°	0.0252	Distal surface of element 4.5	Clockwise
ATT 4.4–4.6 3°	0.0602	Buccal surface of element 4.5	Clockwise

Table 4.

Maximum Tooth Deformation Expressed in Degrees of Rotation

Teeth Deformation	Degrees of Rotation
NO ATT 1.2°	0.08714°
NO ATT 3°	0.24748°
ATT 4.5 1.2°	0.26141°
ATT 4.5 3°	0.54208°
ATT 4.4–4.6 1.2°	0.16869°
ATT 4.4–4.6 3°	0.60594°

Table 5.

Minimum Aligner Deformation and Location

Aligner Deformation	MIN (mm)	Location	Direction
NO ATT 1.2°	0.01235	Lingual surface of element 4.6	Buccal
NO ATT 3°	0.02806	Occlusal surface between element 4.5-4.6	Mesial
ATT 4.5 1.2°	0.01859	Occlusal surface between element 4.5-4.6	Mesial
ATT 4.5 3°	0.05539	Occlusal surface of element 4.5	Mesial
ATT 4.4–4.6 1.2°	0.01844	Occlusal surface between element 4.5-4.6	Mesial
ATT 4.4–4.6 3°	0.06687	Occlusal surface of element 4.5	Mesial

Table 6.

Maximum Aligner Deformation and Location

Aligner Deformation	MAX (mm)	Location	Direction
NO ATT 1.2°	0.18150	Buccal surface of element 4.5	Buccal
NO ATT 3°	0.20982	Buccal surface of element 4.5	Buccal
ATT 4.5 1.2°	0.15433	Lingual surface of element 4.5	Lingual
ATT 4.5 3°	0.29481	Distal surface of element 4.7	Mesial and apical
ATT 4.4–4.6 1.2°	0.25403	Lingual surface of element 4.5	Lingual
ATT 4.4–4.6 3°	0.27780	Distal surface of element 4.7	Mesial and apical

Table 7.

PDL Stress

PDL Stress	MIN (g/cm2)	MAX (g/cm2)
NO ATT 1.2°	0.00	9.52
NO ATT 3°	0.00	30.60
ATT 4.5 1.2°	0.00	61.70
ATT 4.5 3°	0.00	418.00
ATT 4.4–4.6 1.2°	0.00	30.60
ATT 4.4–4.6 3°	0.00	560.00

Table 8.

Aligner Stress

Aligner Stress	MIN (N/mm2)	MAX (N/mm2)
NO ATT 1.2°	0.0000	1.8927
NO ATT 3°	0.0000	2.7504
ATT 4.5 1.2°	0.0000	2.1972
ATT 4.5 3°	0.0000	3.2816
ATT 4.4–4.6 1.2°	0.0002	3.7295
ATT 4.4–4.6 3°	0.0001	2.9521

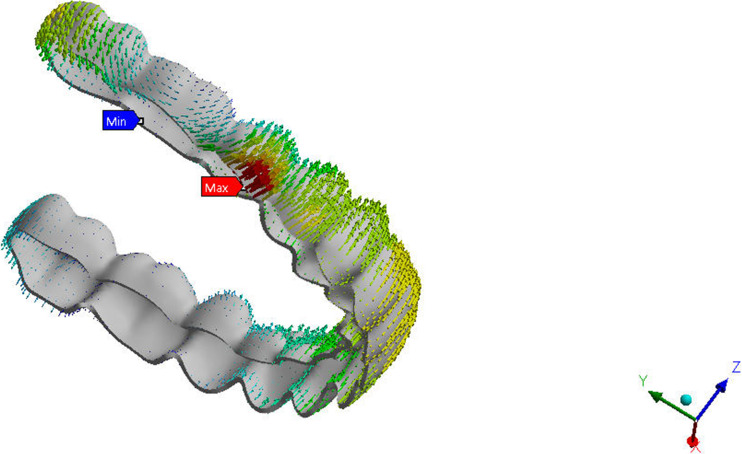
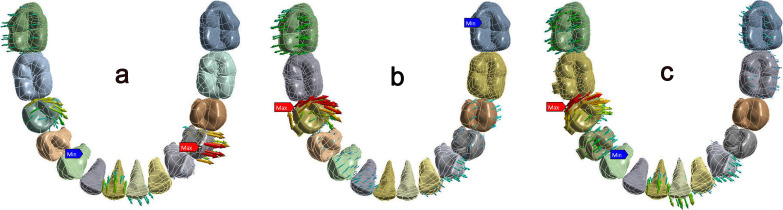
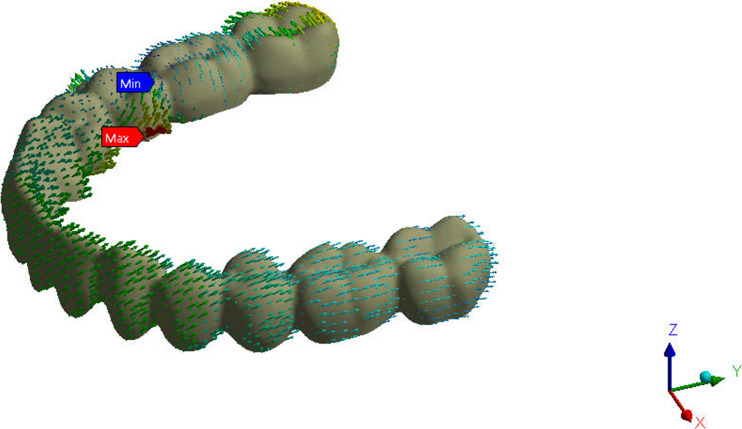
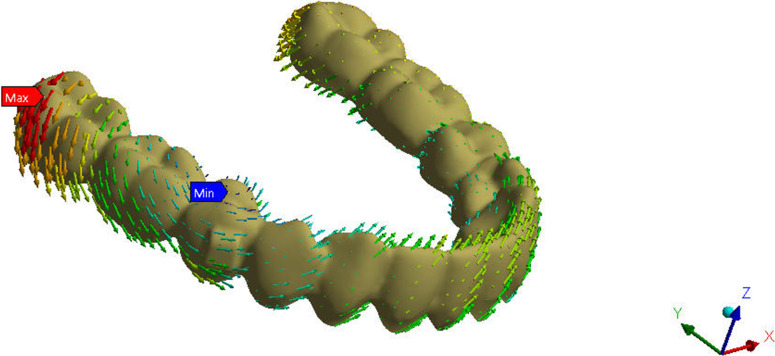
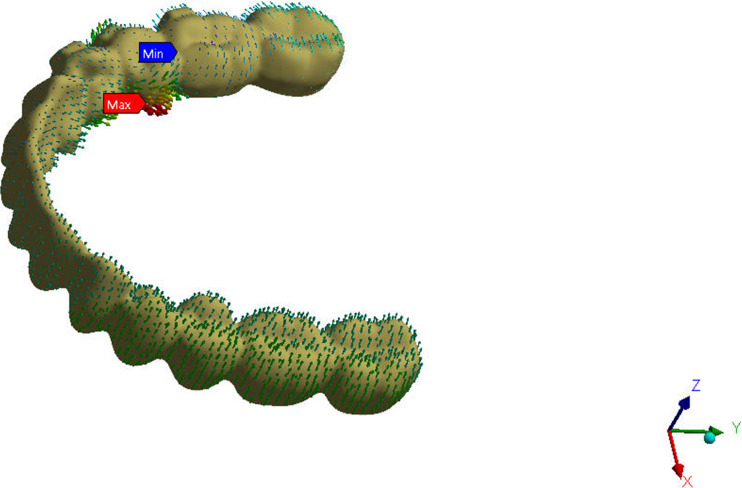
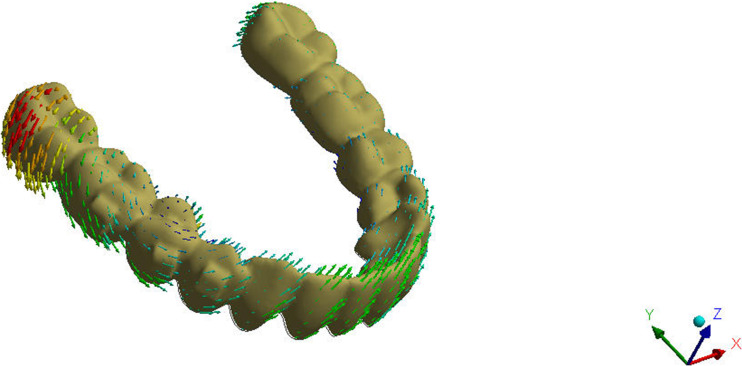
• NO ATT (1.2°): maximum aligner deformation (Figure 2) was located at the cervical-buccal area of element 4.5 (maximum values of 0.1815 mm). Focusing on tooth displacement pattern, the rotated premolar performed a clockwise rotation movement of 0.09°, while the element 3.4 experienced the area of maximum displacement, resulting in a buccal tipping movement (Figure 3a).

• NO ATT (3°): higher aligner deformations (Figure 4) were detected both at the buccal and lingual cervical areas corresponding to the rotated premolar. In particular, the buccal surface registered a maximum deformation of 0.21 mm. Regarding tooth displacement pattern, maximum displacement of 0.25° of rotation was located on the rotated premolar crown.

• ATT 4.5 (1.2°): maximum aligner deformation was shown on the lingual-cervical area of the rotated tooth while minimum values were on the occlusal surfaces of elements 4.5 and 4.6 (Figure 5). The rotated tooth performed a clockwise rotation of 0.26°, while the element 4.7 underwent a small buccal displacement (0.01 mm) (Figure 3b).

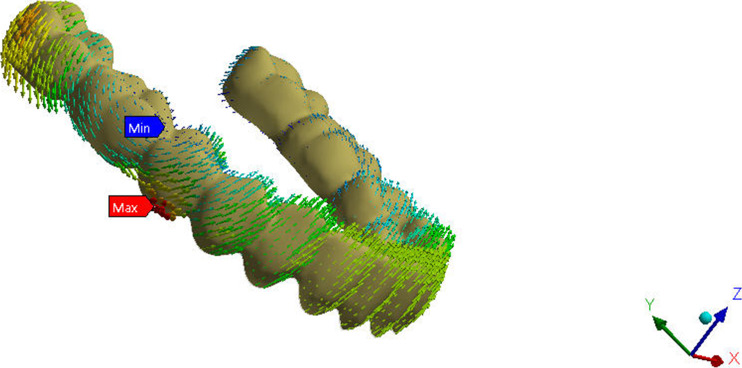
• ATT 4.5 (3°): highest aligner deformations were detected on the aligner distal surfaces corresponding to both elements 3.7 and 4.7 (Figure 6). Deformation resulting in intrusive displacement was observed on the right second molar area (4.7). Regarding tooth response, the element 4.5 performed a clockwise rotational movement of 0.54°, while elements 4.4 and 4.2 underwent lingual crown tipping (0.02–0.03 mm).

• ATT 4.4–4.6 (1.2°): the highest deformation on the aligner (Figure 7) was found on the cervical-lingual surface corresponding to the rotated element (maximum deformation values: 0.25 mm). Minimum deformation was located on the occlusal surface between elements 4.5 and 4.6. Teeth displacement pattern graphs presented maximum displacement of the rotated premolar, which performed a clockwise rotational movement of 0.17°. Other minimal forces were observed on the central incisors with little crown movements in the buccal direction for element 3.1 and in the lingual direction for element 4.1 (0.014 mm) (Figure 3c).

• ATT 4.4–4.6 (3°): the activation pattern identified the highest aligner deformation distal to element 4.7 (Figure 8). Focusing on teeth displacement pattern: maximum displacement of 0.06 mm was achieved by element 4.5, which registered a clockwise rotational movement of 0.61°. The adjacent first premolar underwent lingual displacement of 0.043 mm, while element 4.7 barely moved buccally.

Figure 2.

Aligner deformation of the NO ATT 1.2° simulation.

Figure 3.

Tooth displacement patterns: worst-case scenario: (a) NO ATT 1.2°; and best case scenarios: (b) ATT 4.5 1.2° and (c) ATT 4.4–4.6 1.2°.

Figure 4.

Aligner deformation of the NO ATT 3° simulation.

Figure 5.

Aligner deformation of the ATT 4.5 1.2° simulation.

Figure 6.

Aligner deformation of the ATT 4.5 3° simulation.

Figure 7.

Aligner deformation of the ATT 4.4–4.6 1.2° simulation.

Figure 8.

Aligner deformation of the ATT 4.4–4.6 3° simulation.

Regarding PDL stress, all the simulations showed maximum stress values around the coronal area of the right second premolar PDL, except for the NO ATT 1.2° configuration, in which the highest stress value was located at the apex of the contralateral first premolar PDL. Minimum stress was detected in different periodontal ligaments depending on the pattern of activation: the coronal area of the left second premolar PDL was registered in the two configurations ATT 4.5 3° and ATT 4.4-4.6 1.2°, while the coronal area of the left second molar PDL was registered in the NO ATT 1.2 and ATT 4.5 1.2° configurations.

Aligner stress resulted in similar values in all the simulations. Maximum stress areas were located on the occlusal surface between elements 4.5 and 4.6; only NO ATT 3° configuration displayed maximum stress area on the occlusal surface between elements 4.4 and 4.5.

Four simulations showed minimum stress areas located on the aligner portion corresponding to the left second molar (3.7) while minimum stress areas for the NO ATT 1.2° and ATT 4.4–4.6 1.2° configurations were identified on the lingual aligner surfaces corresponding to element 3.6 and element 3.5, respectively.

DISCUSSION

On the basis of the FE results, it was demonstrated that rotation of round-shaped lower teeth could be controlled with aligners and attachments. As described in previous FE studies,5,16 as well as in other trials on tooth movement with aligners,15,17 auxiliaries were shown to be mandatory to improve the expression of the prescribed tooth movement. As reported in the present study, rotation in groups with attachments was on average 0.23° higher than simulations without attachments. From a clinical perspective, the 30° rotation of a lower second premolar with 1.2° of staging would result in 25 aligners. The amount of prescribed rotation, which was lost because of aligner deformation and biomechanical inefficiency was 0.6° greater in simulations without attachments compared to those with attachments. Loss of tracking is the main cause of incomplete tooth movement, due to the decrease in controlling the movement itself. Previous studies reported that, if rotational control is reduced during CAT, the tooth tends to intrude.7,8

Regarding tooth movement, all aligner activations and combinations of attachments resulted in effective lower right second premolar clockwise rotation except NO ATT 1.2°. The ATT 4.4-4.6 3° configuration produced the greatest tooth movement (0.61°), followed by the ATT 4.5 3° model (0.54°).

Proclination was reported for at least one anterior tooth (from canine to canine) in all the models tested. Regarding simulations NO ATT 1.2°, 3°, and ATT 4.4–4.6 1.2°, a displacement pattern in which element 4.1 moved lingually and element 3.1 moved buccally was detected. On the other hand, the remaining configurations resulted in lingual displacement of the lateral incisors and canine (elements 4.2, 4.3). Despite the differences between incisor reactions in the different simulations, evidence emerged regarding the role of incisal area as an anchorage unit during premolar rotation with CAT. Isolated effects on adjacent teeth may be an artefact due to the nature of FE analysis; the displacement patterns available in these simulations were related to the initial activation and may change in a short time, but evolution of tooth displacement and CAT effects over a long time wearing the aligner were not the subject of this paper. Additionally, the different displacements on anterior teeth draw attention to the clinical aspects of biomechanical analysis, showing that undesired loads may be experienced by lower anterior teeth, thus this should be considered by orthodontists when planning aligner treatment.

All 3° aligner activation models detected anchorage loss on element 4.4, performing lingual displacement of 0.0085 mm for the NO ATT model, 0.035 mm for the ATT 4.5 model, and 0.043 mm for the ATT 4.4–4.6 model. Unpredicted vertical movements were detected on both the ATT 4.5 3° and ATT 4.4–4.6 3° configurations; these models showed intrusive forces focused on element 4.7, which moved 0.025 mm and 0.032 mm, respectively.

The lingual displacement of the mesial tooth as well as intrusion in the molar area during rotation in the 3° activations may be related to the amount of activation, which may have resulted in an excessive load on the active and adjacent units. The increase of mismatch between the target tooth and the aligner may lead to a stiffness increase of the aligner during wear, which could lead to undesired movements near the active unit. This assumption was also supported by the increase in 4.4 and 4.7 displacement with the increase the amount of attachments, which subsequently caused an increase in the aligner's stiffness. Thus, it seems reasonable to state that a key factor influencing CAT outcomes is the aligner's elasticity, which is influenced by several factors that are clinically and nonclinically dependent.

Focusing on stress applied on the PDL, three models (NO ATT 3°: 30.6 g/cm², ATT 4.5 1.2°: 61.7 g/cm², and ATT 4.4-4.6 1.2°: 30.6 g/cm²) were consistent with the definition of “light forces” described by various authors;18,19. The NO ATT 1.2° configuration expressed forces (9.52 g/cm²) that were considered not clinically relevant by the authors. Furthermore, the maximum PDL stress area registered on the PDL of tooth 3.4, as well as the maximum deformation of tooth 3.4 in the NO ATT 1.2° simulation, confirmed that tooth rotation is not efficient with CAT unless the right auxiliaries (eg, attachments) are during treatment planning. The remaining two simulations (ATT 4.5 3° and ATT 4.4.-4.6 3°) developed forces of 418 g/cm² and 560 g/cm², respectively, which could result in hyalinization of the PDL and these values were far from the ideal magnitude of force required for rotation.18,19 These stress areas could have been related to the 3° aligner activation, which transmitted greater forces to the teeth as compared to results for the 1.2° activation models, which showed better force distribution on the PDL. This is in agreement with the statement of main aligner companies to not exceed 2° of rotation for each aligner.

Concerning aligner deformation, no significant differences were reported between the attachment and no attachment models. Four simulations (NO ATT 1.2° and 3°, ATT 4.5 1.2°, ATT 4.4–4.6 1.2°) showed aligner maximum deformation around the rotated element. The two remaining groups (ATT 4.5 3°; ATT 4.4–4.6 3°) with attachments and 3° of activation detected highest deformation on the aligner covering element 4.7 (mean maximum aligner deformation of 0.28mm), with a buccal displacement of the involved tooth (mean displacement of 0.0285 mm). If it is considered that 10 aligners are required to control the 30° rotation, this would result in a second molar total buccal displacement of 0.28 mm. It can be argued that the greatest aligner deformation in the distal portion of the aligner was related to the shape and mechanical properties of the aligner material. This could be the reason why, as stated by Houle et al., aligners become less accurate going from the anterior to the posterior region.20

Considering the overall results obtained through FEM analysis, it could be stated that the most efficient configuration in displacement/anchorage loss/PDL stress ratio was ATT 4.5 with 1.2° of activation. Generally speaking, 1.2° of activation seemed to deliver force levels on the PDL, which better fit with the “optimal force paradigm” of biology of tooth movement.18,19 However, this kind of attachment setting seems to be pretty unrealistic, due to the multitude of simultaneous movements that involve each tooth during orthodontic treatment. On the basis of this limitation, and of FEM results, it could be reasonably stated that ATT 4.4–4.6 with 1.2° of activation is a reliable and efficient configuration for lower premolar rotation.

Limitations of the Study

FEM studies represent one of the best ways to analyze force systems delivered by orthodontic appliances. However, in vitro and clinical study results may differ. High quality clinical trials are required to confirm FEM-derived force systems. Additionally, the study could be improved and integrated by examining other possibilities such as attachments placed on every mandibular tooth or repeated simulations with other attachment designs.

CONCLUSIONS

• Initial lower premolar rotations of 0.2° per aligner is obtainable, on average, with vertical rectangular attachments.

• The configuration with the rectangular and vertical attachment on element 4.5 with 1.2° of activation seems to be the most efficient in rotating the tooth.

• Anterior teeth seem to work as anchorage units during rotation of a second premolar with clear aligners.

• 1.2° of activation seems to deliver force levels on the PDL, which better fit with the “optimal force paradigm” of biology of tooth movement.

• On the basis of the results of the present study, it seems reasonable to prescribe no more than 1.2° of rotation per aligner, in order to maintain good control while rotating round-shaped teeth.

• Aligner deformation is a key factor influencing CAT outcomes.