Towards quantifying the mass extinction debt of the Anthropocene

Abstract

To make sense of our present biodiversity crises, the modern rate of species extinctions is commonly compared to a benchmark, or ‘background,’ rate derived from the fossil record. These estimates are critical for bounding the scale of modern diversity loss, but are yet to fully account for the fundamental structure of extinction rates through time. Namely, a substantial fraction of extinctions within the fossil record occurs within relatively short-lived extinction pulses, and not during intervals characterized by background rates of extinction. Accordingly, it is more appropriate to compare the modern event to these pulses than to the long-term average rate. Unfortunately, neither the duration of extinction pulses in the geological record nor the ultimate magnitude of the extinction pulse today is resolved, making assessments of their relative sizes difficult. In addition, the common metric used to compare current and past extinction rates does not correct for large differences in observation duration. Here, we propose a new predictive metric that may be used to ascertain the ultimate extent of the ongoing extinction threat, building on the observation that extinction magnitude in the marine fossil record is correlated to the magnitude of sedimentary turnover. Thus, we propose that the ultimate number of species destined for extinction today can be predicted by way of a quantitative appraisal of humanity's modification of ecosystems as recorded in sediments—that is, by comparing our future rock record with that of the past. The ubiquity of habitat disruption worldwide suggests that a profound mass extinction debt exists today, but one that might yet be averted by preserving and restoring ecosystems and their geological traces.

1. Introduction

Concern continues to grow about the modern biodiversity crisis: the loss of habitats, decline of populations and ultimately the extinction of species. This concern arises, in part, from the recognition that times with elevated extinction rates during the past 540 Myr often coincided with the losses of key ecosystems and their functions globally [1–4]. The largest such events are often called ‘mass extinctions’, loosely defined as intervals during which species go extinct at a rate that far exceeds surrounding geological intervals [5–7]. The fear is that humanity is triggering a so-called ‘Sixth Mass Extinction’ [8].

In order to contextualize the severity of ongoing biotic crises within Earth's history, previous work has sought to identify a ‘background’ extinction rate, characterizing the majority of the fossil record, as a benchmark. However, the dynamics of biodiversity over most of Earth's history are resolved on multi-million-year timescales, in contrast with the century timescales of modern observations [8–10]. Such a disparate temporal resolution makes direct comparison between modern and ancient extinction rates challenging and/or misleading. Here, we identify and address two fundamental limitations which arise when comparing modern and prehistoric extinction events. Subsequently, we propose an alternative metric that is robust to these issues, while providing actionable targets for avoiding a catastrophic extinction event in the near future.

The first problem is that extinction rates within the fossil record are highly non-uniform, even among intervals without mass extinctions [3,11]. Specifically, poor time constraints on fossil compilations usually require their separation into individual stages lasting over a million years, and associated extinctions are, therefore, averaged over this timescale [12]. However, it has been shown that extinctions generally appear more concentrated into extinction ‘pulses’ [3], as opposed to occurring uniformly throughout stages. Thus, the concept of a single background extinction rate poorly captures the underlying temporal dynamics of prehistoric extinctions. Within this framework, it is more appropriate to compare the modern crisis to previous extinction pulses, as opposed to a background rate, in order to assess its relative size.

In addition to rate-determination, a second short-coming concerns extinction magnitude. That is, geological extinction events have ended, leaving a fraction of genera extinct. By contrast, the modern event is ongoing. Even if human interference ceased immediately, extinctions would continue owing to the phenomenon of ‘extinction debt’ [13]. Extinction debt describes the lag time between an initial perturbation and the extinction of species, as populations decline. Extinction debt has been observed in both modern and ancient studies [14–17], spanning a wide range of timescales; from decadal to centennial lags in mammals [18], island species [19–21] and plants [22], to multi-million-year lags subsequent to the closure of the Isthmus of Panama [23]. A full theoretical understanding of the relationship between perturbation, lag time and extinction magnitude, particularly in the fossil record, remains elusive.

Compounding the problem of extinction debt is uncertainty regarding the importance of secondary extinctions [2,4,24]. Secondary extinctions describe the cascading loss of species within an ecosystem, indirectly following on from a smaller number of initial extinctions. Understanding the importance of secondary extinctions during mass extinction events is hindered by coarse time resolution. Thus, the role played by secondary extinctions, via ecological cascades, in scaling minor extinctions into mass extinctions is uncertain. In other words, it is unclear if we are currently accruing a mass extinction debt (i.e. including secondary extinction) and, if so, how big it might be.

In short, another ruler is needed to assess the relative size of the current biodiversity crisis, and ideally one that is predictive, allowing us to possibly avoid the full magnitude of the event. We suggest such a ruler may be constructed by measuring the magnitude of environmental change as preserved in sediments and rock types, rather than extinction rate alone. The leading threats to species today include habitat loss and land-use change [10] such as agriculture, logging and mining. Crucially, land-use change would be translated to the fossil record as a change in the rocks laid down [25]. The marine fossil record reveals such a correlation between changes in the deposition of rocks and marine extinctions [26], hypothesized to exist because a common environmental perturbation drives both changes. Here, we propose to use analogous changes in ongoing global sediment deposition and rock types to assess the ultimate magnitude of the modern biotic crisis.

We begin by constructing a model of extinction that incorporates the effects of pulsed events in addition to background extinctions (§2). Using this framework, we address whether the current extinction rate is more consistent with background or extinction pulses (§3). We then discuss an environmental metric for measuring the relative size of the modern biodiversity crises (§§4 and 5).

2. Quantifying extinction rates

Extinction rates have been quantified through a variety of metrics [27,28]. Whereas modern assessments usually track species, the fossil record is more appropriately analysed at the level of genus, because of issues of sampling effort, spatial coverage, synonymy and age [12]. Accordingly, in this work, we consider extinction rates in terms of genera. In order to compare extinction rates between modern and ancient genera, we derive a metric quantifying the average extinction rate per taxon.

Suppose that a cohort of genera with diversity N(t₀) = N₀ are extant at some initial time t₀, but experience a time-dependent extinction rate per genus of μ(t), modelled as [29]

$$\frac{\mathrm{d}N(t)}{\mathrm{d}t}=-\mu (t)N(t).$$ 2.1

We can define the average per-taxon extinction rate $\mathcal{E}$ measured over a time Δt as

$$\mathcal{E}\equiv \frac{1}{\mathrm{\Delta }t}{\int }_{t_0}^{t_0+\mathrm{\Delta }t}\mu (t)\hspace{0.17em}\mathrm{d}t.$$ 2.2

The number of surviving genera at time t₀ + Δt is then equal to $N_0{\mathrm{e}}^{-\mathcal{E}t}$. Writing the number of extinctions as N_E = N₀ − N(t) we arrive at our chosen extinction metric:

$$\mathcal{E}=-\frac{1}{\mathrm{\Delta }t}\ln \left(1-\frac{N_E}{N_0}\right).$$ 2.3

The metric often used to quantify modern extinction rates measures the fraction of species going extinct divided by the timescale of observation (i.e. N_E/(N₀Δt)) [27,30]. Over modern timescales, it is usually the case that N_E ≪ N₀, such that the value of $\mathcal{E}$ is approximately equal to N_E/(N₀Δt), as in [8,27]. By contrast, the fossil record spans sufficiently long timescales that the fraction of genera going extinct (N_E/N₀), may approach unity.

In summary, we quantify extinction rates using metric (2.3), ensuring mathematical consistency between modern and fossil extinction. We refer to extinction rates in units of E MGY⁻¹, or ‘extinctions per million genus-years.’

(a). Geological extinction rates

At the resolution of fossil compilations, genus diversity N, is usually only known to the precision of a geological stage. This diversity is often split into four components [12], including those that enter and leave the interval X_bt, those that enter the interval but go extinct X_bL, genera that originate in the interval and leave it X_Ft and the ‘singletons’ X_FL; genera that are found only within the interval. (The subscripts ‘L’ and ‘F’ refer to last and first occurrences, while ‘b’ and ‘t’ refer to crossing the bottom and top boundaries, respectively.)

It is usually impossible to precisely pin down originations or extinctions within a stage. We partially accommodate this uncertainty by excluding singletons, as is typical in palaeontological biodiversity studies [28], and define the initial diversity as all genera that cross the bottom boundary of the interval: N₀ = X_bt + X_bL. Consistently, we ignore singletons in the total number of extinctions N_E = X_bL (see §3).

(b). Pulsed extinction model

Genus extinctions do not occur at a regular pace throughout time. Each generic extinction is a single event, stochastically distributed in time with rate parameter μ. Nevertheless, when the resolution of observations Δt far exceeds the time between extinctions, as for geological stages, multiple extinctions may be thought of as occurring at a single ‘background’ rate—an approach often adopted. However, this idea becomes misleading during modern times, when our timestep of resolution drops well below the expected time between genus extinctions. At such a high resolution, the concept of a background rate in some sense loses meaning. In comparing the modern to the past, it is important to consider how a time-variable extinction rate may be recorded in the fossil record.

Despite the long time-intervals of geological stages, a disproportionate number of last occurrences of fossil genera coincide with the upper strata of these stages [3,11]. This pattern suggests that the majority of genus extinctions throughout Earth's history have occurred within relatively brief timespans. Complications to this interpretation related to sequence stratigraphic biasing are well-known [31,32], and ongoing work continues to refine the statistical robustness of the pulsed signal [33]. Nevertheless, despite these biases, the fossil record of extinction dynamics favours a pulsed profile [3,11]. That is, long periods of relative quiescence are punctuated by intervals of heightened extinction rates.

As such, even the simplest model of extinctions must include a time-dependent per capita extinction rate that includes two components: a constant rate μ₀ (referred to as a ‘true’ background rate) and a number k of pulses situated at times τ_i, each removing a fraction A_i of biodiversity. We construct a model of extinction including both components by writing the per-taxon extinction rate as (e.g. [29,34]):

$$\mu (t)=\underset{\mathrm{background}}{\underbrace{{\mu }_0}}-\underset{\mathrm{pulses}}{\underbrace{{\sum }_{i=1}^k\ln (1-A_i)\delta (t-{\tau }_i)\hspace{0.17em}}},$$ 2.4

where δ(t − τ_i) is a Dirac delta function. Representing extinction pulses with a Dirac delta function assumes them to be infinitely brief, but we relax this assumption later. For illustration, the mean extinction rate (equation (2.2)) resulting from a single such pulse is given by

$$\mathcal{E}\approx {\mu }_0-\frac{\ln (1-A)}{\mathrm{\Delta }t}.$$ 2.5

This result depends upon the observation timescale (§3). However, without further information, the extinction pulse's contribution to $\mathcal{E}$ is indistinguishable from a heightened background extinction rate. Moreover, a large number of small pulses occurring close together grades into the same extinction dynamics as a single background rate [34]. Note that longer Δt dilutes the influence of pulses, implying that longer stages may better capture a ‘true’ background rate.

(c). A single extinction pulse

We now generalize from instantaneous pulses to those lasting a finite time σ. For illustrative purposes, we assume that the temporal evolution of extinction rates within a pulse takes a Gaussian form (figure 1), possessing standard deviation σ, centred at time t_p. We replace the Delta function in equation (2.4) with a Gaussian distribution to obtain the per-genus extinction rate within a single pulse,

$$\mu (t)={\mu }_0-\frac{\ln (1-A)}{\sqrt{4\pi {\sigma }^2}}\exp \left[-\frac{{(t-t_p)}^2}{4{\sigma }^2}\right].$$ 2.6

Figure 1.

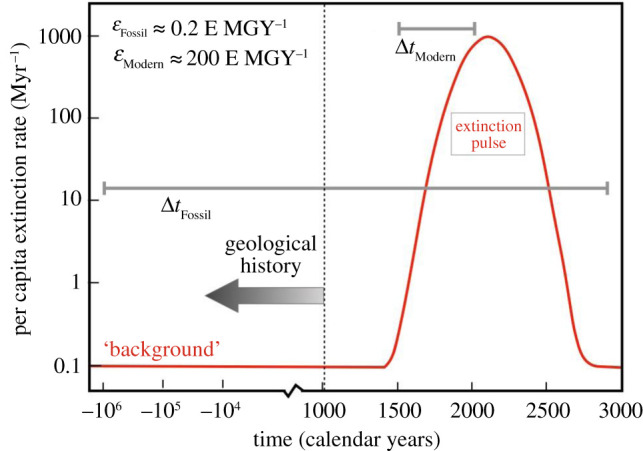
An illustrative Gaussian profile for an extinction pulse of magnitude A = 0.3, duration σ = 100 years and a peak in the year t₀ = 2100. Sampling intervals of Δt_Modern = 520 years and Δt_Fossil ≈ 10⁶ years are indicated with associated extinction rates ε_Modern = 200 E MGY⁻¹ and ε_Fossil = 0.2 E MGY⁻¹. The x-axis denotes time in calendar years, where the scale is linear to the right of the year 1000 and logarithmic to the left. This scale-change in time is included to illustrate the longer time over which geological extinction rates are averaged. The ‘true’ background rate μ₀ = 0.1 E MGY⁻¹ (see equation (2.6)).

When the time interval greatly exceeds the pulse duration (Δt ≫ σ; as in the fossil record), equation (2.6) reduces to the Delta function model of equation (2.4). That is, the fossil record removes all information regarding the temporal pattern of the pulse; only the ultimate fraction of extinctions (A) is known. By contrast, in the modern extinction crisis, only μ(t); the rate of extinctions at a given instant is known, not A.

For illustration (figure 1), suppose that the modern extinction crisis is characterized by A = 0.3, duration σ = 100 years and will peak at t₀ = 2100 years. Sampling with a time interval Δt_Modern = 520 years between years 1500 and 2020, assuming a background rate μ₀ = 0.1 E MGY⁻¹ yields an extinction rate $\mathcal{E}=200$ E MGY⁻¹. Within the fossil record, the same event sampled over Δt_Fossil = 2 Myr would record an extinction rate of $\mathcal{E}=0.2$ E MGY⁻¹, a factor of 1000 lower, simply owing to the timescale of measurement. Thus, a pulsed extinction profile contributes time-dependence to geological rates in addition to sedimentation and sampling biases [12,35].

3. Comparison to past extinction pulses

(a). Data and methods

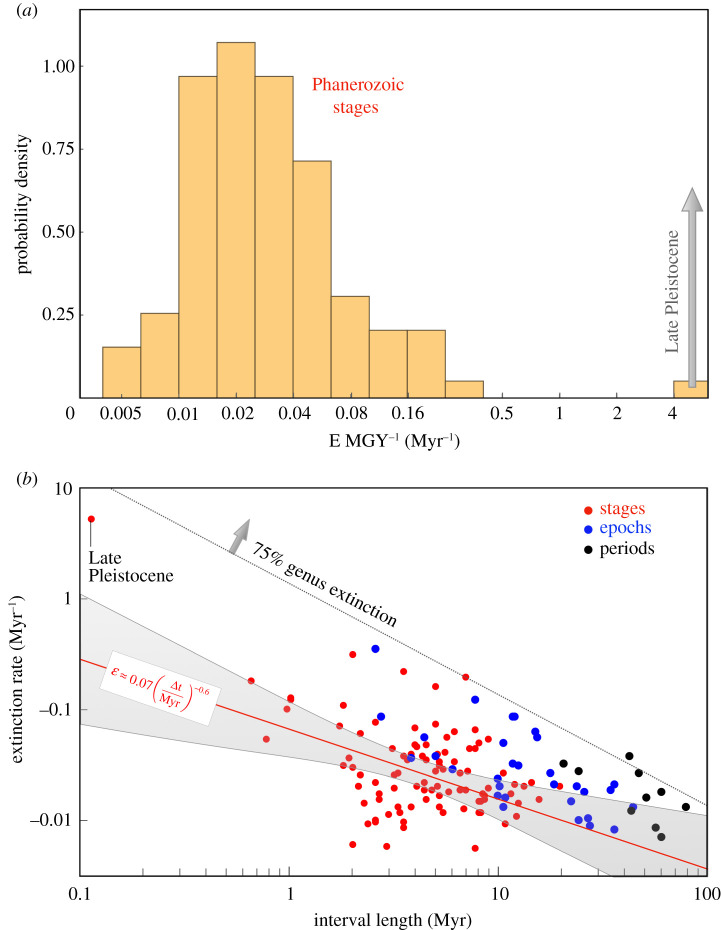
In order to compare extinction rates from modern and ancient ecosystems, we use three separate databases of taxon occurrence. The first is the Paleobiology Database (PBDB; http://paleo-biodb.org/). From the PBDB, we downloaded diversity data in five different formats. The first three included all animal genera, with occurrences divided by stage, epoch and period, respectively (figure 2). The final two formats include Cenozoic genera, with one including all animal genera and the other restricted to mammalian genera (figure 3). The data was downloaded on 25 January 2021.

Figure 2.

(a) The distribution of extinction rates across stages within the PBDB. From the perspective of the PBDB alone, the Late Pleistocene appears highly anomalous. (b) Extinction rate of all animal genera versus the lengths of stages (red), epochs (blue) and periods (black) on a logarithmic scale. A linear regression to the stage-level data is show as a red line, while the extinction rate associated with the loss of three quarters of all genera is shown for illustration with a dotted line. (Online version in colour.)

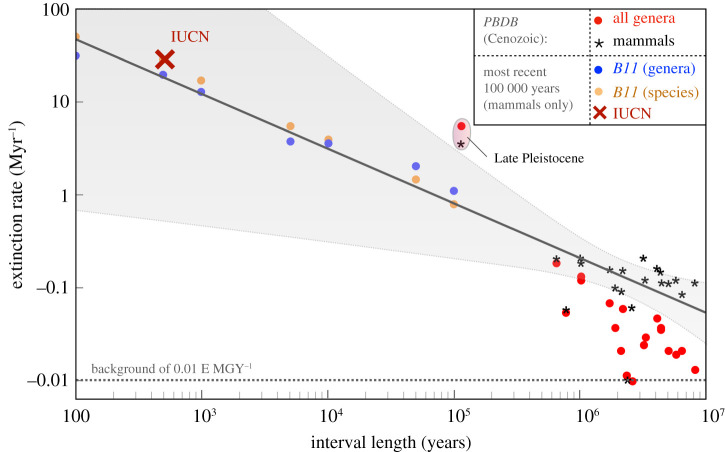
Figure 3.

The extinction rate as measured (following equation (2.3)) across geological stages of varying duration, given by the x-axis. Red circles represent all Cenozoic genera, with black stars the mammal-only subset of those genera. Blue points are extinction rates at the genus level as compiled by B11 over the past 100 000 years, with orange points the equivalent species extinction rates. The black line is a linear regression to the mammal only PBDB data with associated confidence limits in grey. The IUCN Red List information as of 2019 is given by the red cross. (Online version in colour.)

The PBDB is currently the most comprehensive database covering the Phanerozoic (i.e. the last 541 Myr), but is designed to consider diversity dynamics primarily on million year-long intervals or longer. To connect these longer timescales with the modern day, we incorporate data from the compilation described in [8] (abbreviated as B11), which extends back to include the most recent approximately 10⁵ years. The B11 data draws from a number of compilations, with global coverage, but is restricted to mammalian genera. Finally, we draw from the International Union for Conservation of Nature (IUCN) Red List extinction data dealing with the last 500 years [10]. Full details of our datasets are published online at (https://doi.org/10.5061/dryad.tdz08kpzt) [36]. Together, this data allows us to assess the impact of time resolution on modern–ancient comparisons of extinction rates.

We begin our comparison by using the PBDB alone. The distribution of Stage-level extinction rates across all genera is displayed in figure 2a. The Late Pleistocene stands as an outlier, with an extinction rate well above the rest of the distribution. While this conclusion is consistent with the globally detrimental effect of the megafaunal extinction event [37,38], it ignores the abnormally short length of the Late Pleistocene as a stage (approx. 10⁵ years).

Rates of various geological processes, such as sedimentation [35], temperature variation [39] and even extinction rates [12], depend upon the time interval over which they are measured. In order to explore this effect, we plot the stage, epoch and period-level generic extinction rates as a function of time interval duration (figure 2). We fit the stage-level data (red points) with a power law of the form

$$\mathcal{E}{|}_t=E_0{\left(\frac{t}{\mathrm{Myr}}\right)}^{-\gamma }\hspace{0.17em}\mathrm{E}\hspace{0.17em}\mathrm{MG}{\mathrm{Y}}^{-1}.$$ 3.1

A linear regression yields E₀ ≈ 0.07 ± 0.02 and slope γ ≈ 0.6 ± 0.2. The equivalent computations with singletons included yield E₀ ≈ 0.11 ± 0.03 and γ ≈ 0.5 ± 0.2. Thus, excluding singletons does not significantly affect the slope, but slightly modulates E₀.

The approximate extrapolation above predicts extinction rates of $\mathcal{E}$ ∼ 0.3 E MGY⁻¹ over the 100 000 years of the Late Pleistocene, an order of magnitude lower than the PBDB value of 5.3 E MGY⁻¹. Thus, the Late Pleistocene is characterized by an anomalously high extinction rate in spite of its short duration. This is not a new result by any means (e.g. [8,40,41]). However, as many extinctions within geological stages probably arrive in pulses [3,11], the Pleistocene may simply be a better recorder of the extinction pulse than other intervals owing to its short duration. Accordingly, it may be more appropriate to compare modern extinction rates to previous extinction pulses than to the (much-lower) background.

(b). Background or extinction pulse?

The PBDB data exemplify the importance of accounting for time interval duration in comparing modern and ancient extinction rates. In this section, we bridge the gap between the multi-million-year timescales of the PBDB with the modern day. We use the compilation B11, which records mammalian extinctions in the last 100 000 years in discrete time steps (spanning 100 000, 10 000, 5000, 1000 and 500 years, as of 2010). This dataset only includes mammals, and so we compare it to mammals within the PBDB. These data are displayed in figure 3.

Data from B11 include both species and genus-level extinctions and so we plot both on figure 3 (in orange and blue, respectively). Extinction rates at species and genus levels are similar to a factor of approximately 1.5, consistent with previous results [42]. Almost half of the mammalian genera are monospecific [43] suggesting that the fractional extinction of species and genera should be within a factor of approximately 2, only differing substantially if a bias existed for (or against) the extinction of single-species genera.

The relationship between genera and species in the fossil record is less certain, owing to the tendency for fossilized genera to have experienced higher diversification rates at origination (a ‘push of the past’; [34,44]) as compared to unfossilized genera. Translating between species and genus extinction rates requires a careful consideration of such biases. Nevertheless, their approximate correspondence in recent records suggests that the time-dependence of extinction rates in fossil genera also applies to species.

Notably, the B11 rate is lower than the PBDB rate in the Late Pleistocene (Δt = 10⁵ years). This discrepancy probably arises from the inclusion of all modern-day mammalian genera by B11 but not in the PBDB (which includes fossil occurrences only). Including modern genera increases the total standing diversity of mammals in the Late Pleistocene to 1397 genera in contrast to 581 genera in the PBDB. This factor of 2.4 approximately accounts for the disagreement.

Considered together, the PBDB and B11 data suggest mammal extinction rates are marginally higher than that for all genera in the fossil record (E₀ ≈ 0.2 for mammals; E₀ ≈ 0.07 for all genera; figure 2), but exhibit a similar slope γ ∼ 0.6 ± 0.2. The best-fit line for PBDB mammalian data passes, within 95% confidence bounds, through all shorter-timescale points except the Late Pleistocene. Considering modern extinction rates, the IUCN has declared 17 out of 1258 (or 1.4%) mammal genera extinct since 1500 [10], yielding a rate of 27 E MGY⁻¹ (red cross, figure 3), much higher background rates [8,40,41]. However, this extinction rate is within the confidence limits extrapolated to the short observation time frame (grey region, figure 3). If all currently threatened mammal species were to go extinct (1404 species from 5932; [10]), the inferred extinction rate of approximately 500 E MGY⁻¹ would reach the upper edge of the (wide) confidence bounds. The regression uncertainty is sufficiently large that it is unclear how anomalous the present is.

The problems associated with observation interval on rate estimates are well-known [12,35,39], and are central to previous attempts to correct for the effect (e.g. [8,27,40], but the poor time resolution of the fossil record makes it difficult to faithfully project geological extinction rates to shorter, modern timescales. In addition to problems of time-step duration, the fossil record is incomplete, with an estimated 38% of modern marine genera appearing in the fossil record [45]. Genera preserved are preferentially long-lived and widespread [46,47], specious and initially rapidly diversifying [29,34,44], and are found in habitats with higher preservation potential (e.g. [48]). Such taphonomic biases make threatened modern mammal genera about half as likely to display a fossil record as those that are not [42].

Perhaps most problematically, the significance of rate comparisons is unclear because they do not include future extinctions, either through primary extinction debts or secondary cascades. At present, we lack theoretical or empirical knowledge for predicting how minor extinction crises scale into major ones, or how long our current extinction event will last (figure 1). In summary, we might benefit from using metrics other than extinction rates to compare modern and past biotic crises, ones that are predictive and actionable. In the next section, we propose one such metric, related to the degree to which humans have disrupted sedimentary sequences and lithologies globally.

4. Towards a sedimentary proxy of mass extinction debt

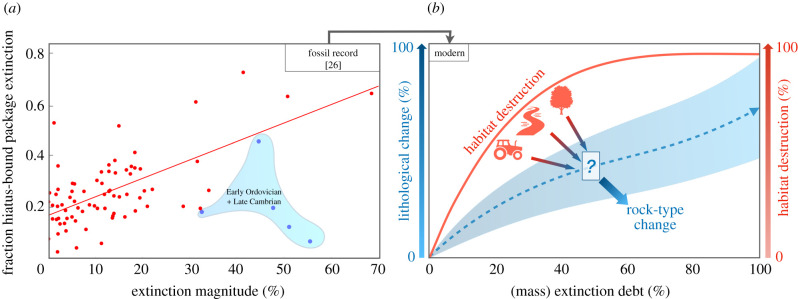
Human activity has already exterminated 100s of species, but an uncertain number are bound for extinction through extinction debt and secondary cascades. By contrast, the fossil record would compress an extinction debt of centuries into a single geological boundary—extinctions and rock-type changes are coeval. At least in the marine fossil record, well-documented temporal correlation exists between extinction magnitude and hiatuses (disruptions to sedimentation) within North American sedimentary sequences [49–52]. Using data acquired from Heim & Peters [26] we illustrate this correlation by comparing the fractional genus extinction within each stage (as of 2011) to hiatuses in sedimentary sequences (figure 4). This correlation has been suggested as causal; the loss of shallow-marine shelf habitat (typically via sea-level variation) drives marine extinctions through species–area relationships [26].

Figure 4.

(a) The relationship between the fractional turnover in hiatus-bound sediment packages and percentage genera extinctions within geological stages (data from Heim & Peters [26]). Points more recent than the Early Ordovician are denoted in red, illustrating that extinctions of genera and hiatuses in marine packages are well-correlated. This correlation suggests that the fractional loss of genera during an extinction pulse may be related to lithological changes. (b) We propose several modern analogies to sedimentary hiatuses that may provide constraints for the current crisis. Specifically, the x-axis, mass extinction debt, is the percentage of genera that will go extinct if no efforts are made to return sedimentary processes to a pre-human state. In red on the y-axis is the per cent habitat destruction, linked via a species–area relationship. The left axis, in blue, is the equivalent lithological change resulting from the habitat loss. We propose that quantifying the relationship between the right and left axes serves as the most direct comparison to geological extinction events. (Online version in colour.)

Critically, these relationships between rocks and life imply that, from a theoretical point of view, changes to the rock record precede extinction pulses, and in principle encode the full magnitude of the coming extinction event. Thus, we propose that an analogous tracer of humanity's global influence upon sedimentation and rock-formation may serve as the most appropriate metric for geological-modern comparisons. However, numerous theoretical and practical difficulties must be overcome if such a metric is to become quantitative and actionable.

First, in contrast to the marine realm, sedimentary hiatuses are not a good candidate in terrestrial environments (where erosion dominates rock quantity, e.g. [52,53]) or for modern-historical comparisons. Sea-level (and sea-level variations) is responsible for much of the quantity and continuity of preserved shallow-marine sediment over the Phanerozoic [54] and is not the primary dynamic we are seeking to trace today. Instead, a sedimentary tracer of anthropogenic environmental change might be better linked to turnover in lithology, that is, to changes in the composition of the rock itself (figure 4).

Lithological tracers serve as signals of ecosystem turnover analogous to those acting out in the modern day (e.g. [55]). Moreover, evidence for lithological turnover underscore events such as the Permian–Triassic mass extinction (e.g. [56,57]), the Cretaceous–Palaeogene mass extinction (e.g. [58,59]) and the Palaeocene–Eocene thermal maximum (e.g. [60–62]). Nevertheless, careful consideration is needed regarding what aspect of sedimentary change is most informative, the sedimentary environments to include in such assessments, and the mechanistic reasons why such a proxy might work.

(a). Anthropogenic rock record

Human activity has altered an estimated 75% of Earth's terrestrial ice-free surface [63], profoundly altering how modern environments will be recorded in our currently forming rock record (e.g. [64–66]). In figure 4, we present a schematic of how lithologic change may be used as a like-for-like comparison to the fossil record. Specifically, a well-known relationship exists between habitat area H, and the number of species it contains S [67], of the form S ∝ H^k. A habitat loss ΔH, will be associated with an extinction debt, ΔS, as shown in figure 4b (red line), where we chose k = 0.25 [68]. The timescale over which the extinction debt is paid is usually unknown, but the habitat loss ΔH will correspond with a nearly coeval global stratigraphic signal.

Significant benefits would come from a predictive metric that forecasts a predicted ΔS from an observed ΔH. However, many challenges stand in the way before such a computation may be used (e.g. [65]). Crucially, each regime of environmental disruption would require its own geomorphological modelling in order to translate human modification into basin-scale alterations (shown schematically in figure 4; [64,69]), which will then become encoded within the geological record [70,71]. These sedimentary signals may be spatially displaced from the associated environment, and many critical environments are simply not (or only very rarely) recorded in the rock record. Accordingly, such a metric may be best suited to assessing the global (rather than regional, or habitat specific) magnitude of the current biotic crisis.

A further difficulty pertains to what or when to define as a pre-human state (e.g. [72]). Humans have exerted global influence upon sedimentation for millennia [63,69,73,74]. For example, prior to extensive damming centuries ago, much of the river system of North America supported widespread wetlands, now buried beneath metres of post-settlement alluvium (destined to be recorded in the rock record [75]), and devoid of the historical fauna. Similarly, a century ago, agricultural practices had already transformed the Southern California shelf into relatively homogeneous mud-ground [76]. Declines in populations, habitats and species during recent centuries span a wide range of taxa and habitats [10], but began even earlier (e.g. [37]). Thus, any lithological comparison between the modern and the geological record carefully infer the time humans first began altering the rock record.

5. On mass extinction debt

The passage of time erodes and distorts the signals of past species and environments preserved in rocks. Despite this, geologists have recognized that extinction is episodic, and that extensive biotic disturbances often coincide with profound disruptions to the sedimentary record [7,12,26,50,51]. Here, we have used these facts to propose a quantitative metric that might be better suited to assessing the ultimate magnitude of the modern extinction crisis.

Eventually, all genera go extinct, with or without humanity's influence, but their durations are being cut short by the widespread disruption of ecosystems and their functions wrought by humanity [77,78]. In tandem, such anthropogenic influences are reflected in comparable evidence for pervasive disruption to the sedimentary record of today [21,71,72,74,76,79]. Although we need a quantitative assessment and comparison of the sedimentary change in modern and ancient strata, the extent of sedimentary disruption as compared to the pre-human state suggests that we may already be incurring a profound mass extinction debt. Thus, there is a real need to move from qualitative to quantitative assessments of modern and ancient sedimentary change to assess whether and to what extent this may be true.

By shifting the scientific focus to assessing our mass extinction debt, rather than relative extinction rates, we hope to provide a path towards avoiding the full effects of a mass extinction. The separation of extinction from the ultimate driver by many years provides the time to restore ecosystems and their many vital functions, so that biodiversity might yet be saved. Thus, while we are proposing that the full extent of the modern biodiversity crisis may be indicated by the extent of environmental change as recorded in the rock, we also recognize that the end result is not a foregone conclusion. Strange as it may sound, if we aim to ‘save the rocks’ we may yet save the species and ecosystems on which we depend.

Data accessibility

The data used in this work is publicly available from the Dryad Digital Repository: https://doi.org/10.5061/dryad.tdz08kpzt [36]. It draws from publications of Heim & Peters [26], Barnosky et al. [8], the IUCN Red List [10] and from the paleobiology database (https://paleobiodb.org/).

Authors' contributions

Both authors conceived of the idea to compare modern and fossil extinction dynamics using an alternative proxy. P.M.H. suggested basing such a proxy on the work of Heim & Peters [26]. C.S. conceived of the extinction dynamics modelling. Both authors contributed to writing the manuscript.

Competing interests

The authors declare no competing interests.

Funding

This work was supported by a Sloan Ocean Fellowship (P.M.H.) and the Heising-Simons Foundation grant no. 2017-0608 (C.S.).