Self-organization in natural swarms of Photinus carolinus synchronous fireflies

Flash bursts relay around vegetation across the swarm, illuminating the role of the environment in shaping self-organization.

Abstract

Fireflies flashing in unison is a mesmerizing manifestation of animal collective behavior and an archetype of biological synchrony. To elucidate synchronization mechanisms and inform theoretical models, we recorded the collective display of thousands of Photinus carolinus fireflies in natural swarms, and provide the first spatiotemporal description of the onset of synchronization. At low firefly density, flashes appear uncorrelated. At high density, the swarm produces synchronous flashes within periodic bursts. Using three-dimensional reconstruction, we demonstrate that flash bursts nucleate and propagate across the swarm in a relay-like process. Our results suggest that fireflies interact locally through a dynamic network of visual connections defined by visual occlusion from terrain and vegetation. This model illuminates the importance of the environment in shaping self-organization and collective behavior.

INTRODUCTION

The spontaneous synchronization of thousands of flashing fireflies is a natural spectacle that elicits fascination, even bewilderment (1). Early scientists investigating popular accounts of firefly synchrony often dismissed it as an illusion, a statistical accident, or an observational artifact, such as the observer’s blinking eyelids or the sudden alignment of the fireflies’ lanterns (light-producing organs) from the wind (2). Skepticism might have persisted for a few decades because these displays are quite rare, and as a natural occurrence, synchronous patterns can be complex and noisy. But careful studies over the past 50 years, facilitated by new imaging techniques and analytical tools, have confirmed that precise synchrony does occur in swarms of specific species under proper circumstances (3–6).

Fireflies use flashes for species recognition and courtship (7). Typically, males advertise their fitness by flying and flashing, while females respond selectively from the ground (8). In a few species, and generally associated with a high swarming density, males tend to synchronize their rhythmic flashing with their peers. Synchronous flashing is a compelling display of collective behavior and a readily accessible example to study synchrony in natural systems. This is why firefly synchrony has often been cited as an inspiration for the theoretical study of systems of coupled oscillators (9, 10), such as the Integrate-and-Fire, Winfree, or Kuramoto models (11, 12), which have generated an abundant literature (13). However, although synchronous fireflies are directly observable, the connection between theory and natural patterns has rarely been attempted rigorously (14). In fact, spatiotemporal data currently available show that these models in their current form are unable to explain a wide variety of natural features of firefly synchrony (5, 6).

RESULTS

To reconcile theory with empirical observations, we video-recorded the collective flashing display of Photinus carolinus fireflies in Great Smoky Mountain National Park during peak mating season in June 2020. The fireflies’ primary natural habitat are the densely forested creeks of the Elkmont, TN area of the park. We positioned our cameras at the edge of a small forest clearing, facing a steep ridge (Fig. 1A). Using stereoscopic recordings, flash occurrences were localized in three-dimensional (3D) space (Fig. 1B). After camera calibration and flash triangulation (see Materials and Methods), we were able to reconstruct, for each night, a cone-shaped portion of the swarm (30 m long and up to 10 m wide) containing up to half a million space-time coordinates (Fig. 1C). It appears that flashes tend to correlate strongly with terrain geometry, indicating that fireflies localize primarily in a thin layer about 1 m above ground (Fig. 1D), in agreement with our previous observations (6). This layer is crowded with bushes and short vegetation. Therefore, this camera placement provides an external, global view of the swarm that is quite different from the perspective of a single swarming firefly. As the natural swarm extends over hundreds of meters, and visual occlusion from vegetation is substantial, these reconstructions also constitute only partial renderings of the swarm.

Fig. 1. Three-dimensional reconstruction of a natural swarm and density-dependent collective flashing.

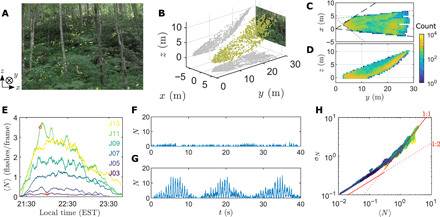
(A) Movie frame showing P. carolinus flashes in their natural habitat (composite image; Photo credit: Peleg Lab, CU Boulder). A steep ridge covered by dense vegetation is visible in the background. (B) Using a second camera to record the same scene, flashes can be located in 3D (yellow dots; 2D projections in gray). The x and y axes define the horizontal plane, with y pointing away from the cameras and toward the ridge; the z axis is vertical upward. (C and D) Spatial distribution of flashes. Colors indicate number of flashes within (0.5 m)² bins. Horizontal projection shows the swarm from above (C). Because of the cameras’ limited FoV (dashed and dotted lines), only a cone-like portion of the swarm can be reconstructed. Vertical projection perpendicular to the ridge (D) shows fireflies localized mainly in a 1-m layer above ground. (E) Moving averages (5 min) of the number of flashes per frame, 〈N〉, for each night (3 to 13 June; only odd nights shown for clarity). Density increases until peak is reached (10 to 13 June). (F and G) N time series for a short interval around 21:45 [red circles in (E)], for a low-density [(F); 3 June] and a high-density [(G); 11 June] night. At low 〈N〉, flashes occur uniformly with little fluctuations. At high 〈N〉, N exhibits large fluctuations, with flash occurrences clustering at specific times. Fireflies flash synchronously every ∼0.5 s during periodic bursts repeated every ∼12 s. (H) Scaling of the standard deviation σ_N with the mean 〈N〉 [same nights as (E)]. All available data collapse on a single curve, with two regimes of scaling σ_N ∼ 〈N〉^α and a turnaround point around 〈N〉 ≃ 0.3. At small 〈N〉, σ_N ∼ 〈N〉^1/2 (least-squares fit: α = 0.53 for 〈N〉 < 0.2, R² = 0.96). At large 〈N〉, σ_N ∼ 〈N〉 (least-squares fit: α = 0.86 for 〈N〉 > 0.8, R² = 0.91). Red lines of slope 1/2 and 1 are shown as a guide.

P. carolinus fireflies produce, individually, flashes of 100 to 150 ms repeated up to eight times, while either immobile or in flight (5, 6). They are active for approximately 3 hours every night after sunset during about 2 weeks in early summer (8). We recorded from 3 June, when a few rare flashes started to be seen, to 13 June, the fourth consecutive night of peak activity, as evidenced by the moving average of the number of flashes N in a given frame (Fig. 1E), detected by pixel intensity thresholding. Synchronous flashing appears to necessitate a critical density of fireflies to occur. When only few fireflies are active (3 to 5 June, and early or late in the night), collective flashing appears incoherent (Fig. 1F). During peak nights, flashes tend to cluster at specific times (Fig. 1G), as N exhibits a doubly periodic pattern of synchronous flashes every 0.55 s during repeated bursts lasting about 10 s (incidentally, this intermittent synchrony is incompatible with numerous models describing asymptotic phase convergence of continuously coupled oscillators). These two features have well-defined frequencies, as seen in the frequency power spectrum of N(t) (fig. S1). However, while Fourier transforms reveal periodicity, they do not inform about synchrony. To quantify the onset of synchrony, we study the distribution of N over 5-min time intervals (Fig. 1H). At low density, the standard deviation of N, σ_N, scales sublinearly with the mean 〈N〉, and approximately as ${\mathrm{\sigma }}_N\sim \sqrt{\langle N\rangle }$, suggesting that flashes are randomly distributed. Past a certain density threshold, however, the scaling becomes linear, σ_N ∼ 〈N〉, indicating clustering (15). This marks synchronous behavior.

This density-dependent transition from disorder to order has been observed in various animal systems exhibiting collective behavior and is a feature of common mathematical models (16). The underlying mechanism is often believed to stem from an increased impulse to follow the behavior of the peers when they are numerous. However, the fireflies’ cooperative behavior at high density does not seem to require multiple converging influences, because even two fireflies in isolation attempt to flash in synchrony, as previously demonstrated in controlled experiments (6). Rather, we hypothesize that fireflies interact at short range; therefore, a high density is necessary to receive and relay the flash information across the swarm.

To explore this hypothesis, we investigate the popular observation that the collective flashing of P. carolinus appears to be “propagating” or “cascading” across the terrain (17). Specifically, as flash bursts start and end with only a few flashers [Fig. 1G and (6)], it has often been reported, but never measured, that bursts tend to originate and terminate at distinct locations many meters apart, often the top and bottom of a ridge. Flashes are sometimes perceived to move progressively from one location to the other over the course of a burst, then repeat the same path during a few minutes. Hence, we look for evidence of this flash propagation in our 3D data. Because the signal is noisy but periodic, we first calculate each flash’s relative timing within a burst. For each burst, we define the time with the maximum N as the origin (see the Supplementary Materials). Each flash occurrence within this burst is then labeled by a “phase” ϕ, roughly between −5 s and +5 s, corresponding to its relative time in the burst (Fig. 2A). Although as defined ϕ is not strictly a phase, having units of time, its interpretation is similar, and conversion to an angular phase φ is straightforward: φ = πϕ/T_b, with T_b the burst period. By doing so, we were able to identify certain time intervals of a few minutes that show a clear propagation up and down the ridge over the course of ≃10 s, when averaged over ≃50 bursts (movies S1 and S2). In Fig. 2B, for example, early flashes are concentrated at the bottom of the ridge, and late ones at the top. The distribution of ϕ along the direction perpendicular to the ridge (y axis) follows a linear progression (Fig. 2C).

Fig. 2. Flash propagation across the swarm.

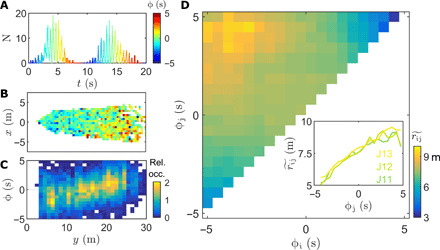
(A) Flash occurrences are associated with a phase ϕ indicating their relative timing within a burst. The center of the burst (highest peak) is defined as ϕ = 0 s. (B and C) Example of flash propagation along the y axis over a 10-min interval (11 p.m., 10 June). On average, early flashes are located at the bottom of the ridge (close to the cameras), while late flashes are located at the top. (B) Average ϕ in 0.5 m × 0.5 m space bins, same colors as (A). Bins close to the cameras show a negative phase, while bins far have a positive phase. (C) Distribution of ϕ along the y axis. Colors indicate relative occurrence. (D) Flash propagation over 150 min, 11 June. For each pair of intraburst flashes occurring at (ϕ_i, ϕ_j) corresponds a distance r_ij. In each bin of the (ϕ_i, ϕ_j) matrix, the distributions of distances are represented by their median value, $\widetilde{r_{\text{ij}}}$, ranging from 3 to 10 m. The diagonal has been removed to avoid intraflash self-correlations. (Inset) Median distance $\widetilde{r_{\text{ij}}}$ as a function of ϕ_j for ϕ_i = 5 ± 0.25 s (leftmost column in the larger plot), for 11 to 13 June. The increase in $\widetilde{r_{\text{ij}}}$ (about 5 m over 10 s) is approximately linear in time up to ϕ_j = 2.5 s. Linear least-square fits for ϕ_j < 2.5 s return slopes of 0.48, 0.58, and 0.49 m/s (R² = 0.94, 0.98, and 0.98 s) for 11 to 13 June, respectively.

However, flash propagation does not always follow a specific terrain orientation. To characterize flash propagation in general and without relying on a specific coordinate system, we calculate the distribution of distances r_ij between pairs of intraburst flashes occurring at ϕ_i and ϕ_j (ϕ_j > ϕ_i; fig. S2). When all flash occurrences from a night’s recording are processed together (Supplementary Materials), a pattern emerges: the median distance $\widetilde{r_{\text{ij}}}$ between flashes increases linearly over time, especially relative to early flashes (fig. 2D). This defines a propagation velocity for the burst activation front. The small value of $\widetilde{r_{\text{ij}}}$ at ϕ_i, ϕ_j ≃ − 5 s and ϕ_i, ϕ_j ≃ + 5 s indicates that very early and very late flashes are strongly localized. The same trend is observed for all data corresponding to peak nights (Fig. 2D and fig. S3) and for 360° recordings made at other locations within 300 m of the ridge area (fig. S3). This analysis suggests that flash propagation occurs, on average, at constant speed of about 0.5 m/s. Importantly, flash propagation is not associated with any significant flow of flashing fireflies along the propagation path (fig. S4). This demonstrates that flash propagation occurs through a relay-like process, similar to a wave, whereby information, not matter, is transported.

Therefore, burst propagation suggests that active fireflies interact with the swarm locally, rather than globally. This is common and well documented in animal groups. Local interactions have been proposed to be either metric (18), where individuals interact with peers within a certain distance, or topological (19), where interactions occur through a set number of nearest neighbors. In flocks, schools, and crowds, local interactions have been shown to result in a linear (constant velocity), underdamped propagation of information (20–23).

What is remarkable within P. carolinus swarms is that information propagation is linear only on average. In fact, simultaneous flashes, even early and late ones, can be spatially distant. In other words, the distributions of r_ij(ϕ_i, ϕ_j) are broad (fig. S2), in stark contrast to bird flocks or human crowds where the time-distance relationship is very narrow (20–23). This suggests that local interactions may extend beyond each firefly’s immediate geometric vicinity.

As further evidence, we find that, although collective flashing is symmetric within a burst (Fig. 3A), firefly movement is not. We consider streaks, defined as the spatial path of a flash as it appears on successive frames (typically five to eight frames). We find that early streaks (ϕ < 0) move significantly faster than late ones (ϕ > 0), as seen on Fig. 3B. This is similar to what had been observed previously in controlled experiments within an unobstructed confining volume, where the burst leader was flashing longer and flying farther than followers (6). Most importantly, motion asymmetry suggests that at least some fireflies are able to perceive the global state of the swarm (i.e., relative delay in the burst), and not simply their local environment. Otherwise, purely local sensing would not create collective behavior disparities during bursts.

Fig. 3. Firefly movement during bursts.

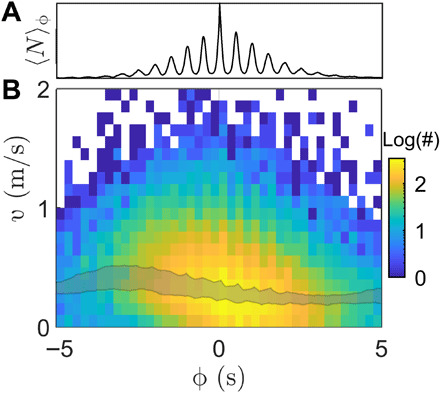
(A) Average burst 〈N〉_ϕ, obtained by averaging N over the ϕ-space. Flash distribution is almost perfectly symmetric. (B) Distribution of streak velocities as a function of streak phase ϕ, 10 June. Colors indicate count, in log₁₀ scale. Early streaks (ϕ < 0) are significantly faster than late ones (ϕ > 0). Median velocities for peak nights (10 to 13 June) all fall within the shaded area (0.31 to 0.51 m/s for ϕ = − 2.5 s and 0.19 to 0.28 m/s for ϕ = + 2.5 s).

DISCUSSION

The combination of flash propagation and velocity asymmetry leads us to hypothesize that local interactions may have a complex structure, and notably involve a wide range of distances. To explain this framework, we consider the line-of-sight premise: Fireflies can only interact with peers that are directly in their field of view (FoV), i.e., when a pair is connected by an unobstructed line. In dense groups of large animals, visual occlusion is created by nearest neighbors, which are generally concentrated at a characteristic distance. Therefore, local interactions, whether metric or topological, must have a narrow distribution of distances. In dilute swarms, however, there is no such screening, and lines of sight may be broadly distributed (24, 25). For example, pair interactions in mosquito swarms typically do not follow a characteristic scaling (24).

P. carolinus congregations present an intermediate situation: The swarm is dilute, but the environment creates significant visual occlusion. Elevated fireflies have access to a wider view of the swarm, but they are few. Most fireflies are located among the vegetation and may have their FoV significantly obstructed.

To elucidate the type of interactions that fireflies can establish, we used local swarm reconstructions obtained from 360° cameras placed directly within the vegetation, 0.6 m above ground (Supplementary Materials).

This setup offers an immersive view from the perspective of an “average” swarming firefly. In particular, it allows to characterize the distribution and range of line-of-sight interactions (within a scaling factor relating the light sensitivity of the cameras and of the firefly’s eyes). From these local reconstructions, we find that the distribution of accessible flashes is peaked at short distance, but is also long-tailed and extends much further in certain directions (fig. S5). Terrain and vegetation also create large variations in the number and range of accessible interactions depending on the firefly’s orientation.

In conclusion, this work investigates firefly collective behavior by approaching the swarm as a macroscopic entity and examining the internal structure of social interactions. We demonstrate that firefly density induces a transition from uncorrelated flashing to synchrony and that information waves can propagate across the swarm. Although the swarm spans hundreds of meters within a dense hardwood forest and it is not possible to probe it exhaustively, our data show identical spatiotemporal patterns over seven nights of recordings spanning three different locations (fig. S3). Our results suggest that although P. carolinus males synchronize their rhythmic flashing with their peers locally, a global swarm synchronization is only possible if enough fireflies are active to transport the collective pace information. Firefly local interactions, rather than metric or topological in nature, are possibly supported by a dynamic network of visual connections defined by relative orientations and visual occlusion from terrain and vegetation (26). This results in a mixture of short-range and long-range interactions. While this distinctive paradigm still requires further examination, this self-organization would allow for the possibility for an individual to position itself to be more or less connected, for example, by flying above the swarm to be more visible and carry flashing information further. This, in turn, might enable social differentiation. Indeed, it seems a priori paradoxical that a group of males competing for female attention exhibits this strong mimicry. While convincing explanations for the ecological function of synchronous flashing have been proposed (27, 28), it is possible to assume that males would use subtle variations in their behavior to distinguish themselves. In particular, further analysis should attempt to understand why and how flash bursts originate at specific locations.

MATERIALS AND METHODS

Experimental sites

Field experiments took place in the Elkmont, TN area of Great Smoky Mountain National Park (USA) after approval by the National Park Service. In accordance with Park policies, the exact location of the experimental sites will not be disclosed publicly but may be indicated upon request. The general area for field experiments encompasses over 1 mile (1.6 km) of trails through densely forested areas, and video recordings took place at several different locations with various camera setups, including, in particular, the ridge area described in Fig. 1, and sites FS and TC for 360° recordings (see below) mentioned in Supplementary Text. Extreme care was taken to respect local vegetation and wildlife.

Three-dimensional reconstruction of the ridge area

For stereoscopic recordings of the ridge area, we used two Sony α7R4 cameras, with the following settings: 60 frames per second (fps); exposure time 1/60 s; maximum aperture (f/1.8); maximum ISO (32,000); and focus to infinity. The cameras were positioned about 4 m apart, and their relative orientation was adjusted to optimize the overlap of their FoV. Using landscape markers such as trees, we made sure that each camera’s FoV was the same for each night. Spatial calibration was performed using 5 to 10 pictures of a checkerboard (25 mm square side length) placed at different locations and using the MATLAB stereo calibration toolbox. Temporal calibration (frame synchronization) was based on both a short artificial light signal at the beginning of the recording and cross-correlation of the temporal patterns between both cameras, which returned the same results. After extraction of the flash positions in each frame using global pixel intensity thresholding, triangulation was performed using MATLAB’s triangulate function to compute the 3D coordinates of recorded flashes. About 60% of the flashes recorded in each camera could be triangulated. Some mild postprocessing was then applied to eliminate about 1% of outlier points, for example, those falling at a distance much greater than 30 m from the cameras or at a negative elevation.

3D reconstruction using 360° cameras

The details of the principle and implementation of stereovision using 360° cameras are described in (6). Briefly, two GoPro Fusion 360° cameras recording at 30 fps were placed on the ground at a set distance (0.9 or 1.8 m) facing the same direction. The trajectory of a small light was used for calibration, and triangulation was performed using the algorithm provided in (6). The cameras were started at around 9:30 p.m. EST (Eastern Standard Time) each night at various locations across the experimental area and recorded for about 100 min.

SUPPLEMENTARY MATERIALS

Supplementary material for this article is available at http://advances.sciencemag.org/cgi/content/full/7/28/eabg9259/DC1