Abstract
Bipolar membranes (BPMs) are gaining interest in energy conversion technologies. These membranes are composed of cation- and anion-exchange layers, with an interfacial layer in between. This gives the freedom to operate in different conditions (pH, concentration, composition) at both sides. Such membranes are used in two operational modes, forward and reverse bias. BPMs have been implemented in various electrochemical applications, like water and CO2 electrolyzers, fuel cells, and flow batteries, while BPMs are historically designed for acid/base production. Therefore, current commercial BPMs are not optimized, as the conditions change per application. Although the ideal BPM has highly conductive layers, high water dissociation kinetics, long lifetime, and low ion crossover, each application has its own priorities to be competitive in its field. We describe the challenges and requirements for future BPMs, and identify existing developments that can be leveraged to develop BPMs toward the scale of practical applications.
Renewable energy conversion technologies, including water electrolyzers, fuel cells, and photoelectrolytic cells, have rapidly gained interest in the past decades. These electrochemical technologies often use ion-exchange membranes as an electrolyte that has three main functions: (1) allowing passage of ionic charge carrier species, (2) separating reactants and/or products between the anode and cathode, and (3) providing a controlled environment for electrode reactions.1,2 An ion-exchange membrane contains immobilized ionic groups, facilitating the transport of, e.g., proton cations in the case of cation-exchange membranes (CEMs) or hydroxide anions in the case of anion-exchange membranes (AEMs). A third category of ion-exchange membranes encompasses bipolar membranes (BPMs), which were first introduced in the electrochemical field by Frilette (1956),3 were traditionally applied to electrodialysis applications, and have been receiving increasing attention in the past decade for energy technology applications.4,5 A BPM is composed of a cation-exchange layer (CEL, transporting, e.g., H+) and an anion-exchange layer (AEL, transporting, e.g., OH–), which are laminated together. The abrupt transition from CEL to AEL at the interface of the BPM involves a chemical process, e.g., dissociation or association of the two active charge carriers, H+ and OH–. The BPM prevents transport of ions across both layers of the BPM, which provides the freedom to operate in distinct electrolytes at either side.6,7 The interface layer (IL) between the two membrane layers features a catalyst to promote the dissociation/association process in order to maintain the supply or consumption of the ionic charge carriers from either layer of the BPM.
Several electrochemical energy technologies have successfully implemented a bipolar membrane (BPM), such as CO2 reduction, fuel cells, water electrolyzers, photoelectrochemical cells, flow batteries, and resource recovery, but none of the BPM-facilitated energy technologies has reached industrial scale.
Several applications in electrochemical energy conversion technologies have implemented a BPM as electrolyte, such as CO2 reduction,8,9 fuel cells,10 water electrolyzers,11 photoelectrochemical cells,12,13 flow batteries,14 and resource recovery, e.g., ammonia and carbon dioxide via BPM electrodialysis.15,16 The choice for a BPM is made due to its intrinsic advantages compared to a monopolar membrane (stability of electrolytes as total charge is maintained, improved separation of products and/or reactant), but often the available BPMs have some imperfections, leading to unwanted behavior (ion crossover, blistering, high resistance, slow kinetics; see further on). An ideal BPM should feature (1) high conductivity of the individual bulk layers, (2) if applicable, fast chemical (dissociation or association of water) kinetics at the interface, (3) high water permeability, (4) long lifetime under operational current densities, and (5) low parasitic (ion) crossover (see Figure 1a). As the BPM was originally developed for producing acids and bases in, e.g., BPM electrodialysis, the membrane properties have not been geared toward optimization in energy technologies. Hence, embedding a BPM in electrochemical cells for energy conversion is limited to the lab-scale stage.
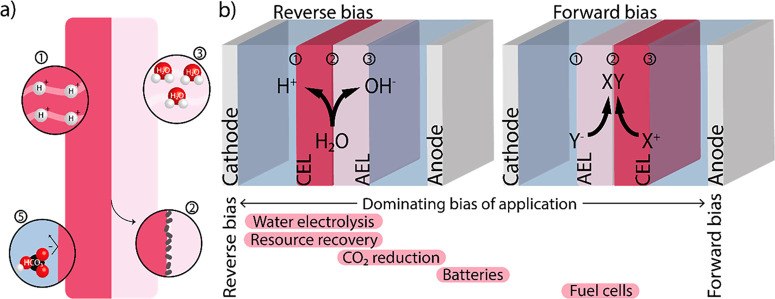
Figure 1.
(a) Schematic of components of an ideal bipolar membrane (BPM), with (1) high conductivity of the individual bulk layers, (2) fast chemical kinetics at the interface via deposition of catalyst, (3) high water permeability, (4) long lifetime under operational current densities (not shown here), and (5) low ion crossover. (b) The BPM can operate in two modes: reverse and forward bias. A BPM comprises three interfaces of interest: two with the electrolyte/electrode and membrane layer (1 and 3) and one between the membrane layers (2). At each interface, a potential difference is created due to the change in charge density, as described in the literature.17,18 A BPM can also be used in a zero gap configuration (not shown here), in which the membrane layer is in contact with the electrode. The bars at the bottom of the image indicate the different applications and their relative usage of each orientation (e.g., water electrolysis is only performed in the reverse bias orientation, while CO2 reduction is predominantly used in reserve bias but has also been studied in forward bias).
At the same time, a BPM can offer a unique advantage to emerging energy technologies, as it allows passage of protons through the CEL on one side and hydroxide through the AEL on the other. In this way, the BPM is capable of solving incompatibility issues: as for electrochemical applications, like water and CO2 electrolysis, the optimal pH differs for the two electrodes, and the BPM can bridge these variations, allowing optimal conditions. Adding this degree of freedom to the process setup favors individual optimization of separate compartments and tuning of electrode chemistries and will, in the end, speed up the development of industrial applications.
Even though an ideal BPM for energy technologies complies with the same five characteristics as an ideal BPM for acid/base production, the optimized realistic BPMs can be quite different. For example, the high current density in water electrolyzers (200–400 mA cm–2 for alkaline and 1–2 A cm–2 for PEM type) sets extremely high standards for the water dissociation activity, water diffusion rate, and ion conductivity, while these parameters are less stringent for resource recovery and photoelectrochemistry that operate typically at 2 orders of magnitude lower current density. Similarly, reducing (cat)ion crossover is key to mitigate salt formation and ensure operational stability in the case of CO2 electrolysis,19 while imperfect BPM selectivity would be acceptable for water electrolysis, as the water dissociation is 2 orders of magnitude larger than the co-ion crossover at high current densities typical for electrolyzers,20 and no crossover at all would occur for gas-fed electrolyzers.
The recent reviews on BPMs by Pärnamäe et al. (2021)21 and Giesbrecht and Freund (2020)22 provide an excellent overview of the recent achievements in the field, but do not address the improvements that are required to implement BPMs in energy conversion technology applications at industrially relevant conditions. In this Perspective, we analyze what developments in BPM materials are already available, and what will be still needed to successfully apply BPMs in electrochemical energy technologies. We outline the BPM modes as applied in energy technologies and provide a roadmap for improvements from a materials point of view and in terms of operational conditions. Having established that conditions and requirements of BPMs are strongly application-dependent, we apply the outlined opportunities for improvement to the applications of water electrolysis, CO2 electrolysis, resource recovery, fuel cells, and batteries.
Bipolar membranes, being made of two opposite-charged layers, can be operated at two modes of operation, depending on the direction of the ion flow (Figure 1b). When the transport of cations and anions is directed toward the interfacial layer, the operational mode is called forward bias. In the opposing mode, a reverse bias is applied across the BPM, with an outward transport of cations and anions. The terms forward and reverse bias were, in analogy to the n-p junction, adopted for electrodialysis, referring to the enrichment and depletion of ions in the IL, respectively. For energy conversion technologies, the concerned cations and anions are typically protons and hydroxides, respectively. The two operating modes are hence accompanied by water formation (forward bias) and dissociation (reverse bias) in the IL, as shown in Figure 1b. In some cases in the literature where forward bias is used, other ionic species are transported to the IL, and salts are formed.18 For both biases, a membrane–membrane voltage is established at the IL, given by the Gibbs energy for water dissociation and the proton/hydroxide activities. At standard conditions (i.e., protons at unit activity in the CEL and hydroxide at unit activity in the AEL), the interfacial potential is 0.83 V. However, the total (electrostatic) equilibrium potential of the membrane depends on the surrounding electrolytes, as Donnan potentials exist at each membrane interface (electrode/electrolyte–membrane layer twice and IL).23 The underlying thermodynamics has been recently rationalized for the use of BPMs in water electrolysis24 and for fuel cells.25
A reverse bias is traditionally applied to BPMs, as this leverages the enhanced water dissociation. In the reverse bias mode, ions are removed from the IL, depleting the membrane of the mobile charges. In order to maintain charge neutrality and to supply the required ion current, the depletion of ions (proton and hydroxide) triggers further water dissociation, in line with Le Chatelier’s principle. The reverse bias has been demonstrated in acid/base production,4 water electrolysis,23 CO2 electrolysis,8 and resource recovery via pH swing.16,26 The ion transport mechanism in reverse bias has been well studied, in particular for extreme pH gradients (i.e., pH 0 vs pH 14) and unbuffered (initially neutral) solutions.17,23
In forward bias mode, an energy gain in the cell voltage can be acquired due to recombination reactions at the IL, with products like salts18 or water.25,27,28 Potentially, the membrane voltage obtained from water recombination is the same as the thermodynamic potential for water dissociation and can be harvested as electrical energy in, e.g., fuel cells or acid/base batteries.14,25,29 Other studies opted for the forward bias mode to mitigate CO2 crossover in water/CO2 electrolysis cells.30 Potential challenges in forward bias mode are reduced stability (blistering), particularly in the case of gas evolution at the interface, and decreasing ionic strength in the electrolyte as charged species neutralize each other.13
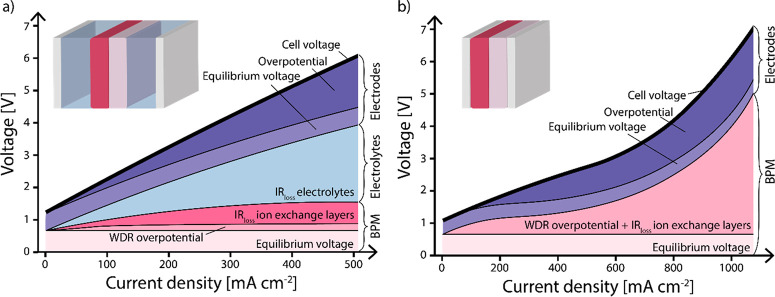
The membrane voltage consists of three components: the earlier discussed equilibrium potential, water dissociation reaction (WDR) overpotential (in case of reverse bias), and ohmic losses of the individual bulk layers (see Figure 2a). While the equilibrium potential depends on the environment, the two other components should be as small as possible. For the individual bulk layers, this implies maximizing the ion conductivity of the CEL and AEL. At present, commercial BPMs show a significantly higher resistance (typical area-specific resistances of 3–10 Ω cm2) than low-resistance membranes in electrolyzers. This is 2 orders of magnitude higher than the total area-specific resistance the individual layers could provide.31 At 60–80 °C, the ion conductivity of a fully hydrated state-of-the-art CEL (e.g., perfluorosulfonic acid membranes) in the H+ form is typically above 0.1 S cm–1,32 while that of an AEL (e.g., quaternary ammonium-functionalized membranes) in the OH– form is slightly lower.33 One of the explanations for this discrepancy between the BPMs and low-resistance (monopolar) membranes is that the thickness of commercial BPMs is almost an order of magnitude larger than 25 μm. With a thickness of 25 μm of the individual layers and a conductivity of 0.1 S cm–1 for both layers (which translates to a total area-specific resistance for the bulk layers of 50 mΩ cm2), an ohmic voltage drop of just 10 mV across the bulk layers of the BPM at a current density of 100 mA cm–2 is obtained. Further increasing the current density to 500 and 1000 mA cm–2 would result in a voltage drop of 50 and 100 mV, respectively. Also, there is partial neutralization of the ionic groups due to support electrolyte entering the membrane, which seems to be supported by the observation that the area-specific resistance under reverse bias decreases with increasing current density as a result of the higher rate of H+ and OH– formation at the interface.31 A third explanation is that additional contributions to the resistance are in play that appear ohmic from the linearity of the polarization curve but are connected to, e.g., kinetic losses or mass transport limitations related to the WDR under reverse bias operation. Electrochemical impedance spectroscopy studies have shed light on the complexity of the polarization losses associated with the water dissociation and ionic separation.31,34 From an engineering point of view, making thinner CELs and AELs using the latest technologies with high conductivity and selectivity for H+ and OH–, respectively, would be readily available to boost the BPM performance.
Figure 2.
Voltage distribution of an electrochemical cell (a) in a liquid–liquid environment with extreme pH (favoring equilibrium voltage) and (b) in a zero gap configuration. Membrane voltage consists of equilibrium voltage, water dissociation reaction (WDR) overpotential, and ion-exchange layers (data obtained from Chen et al., 2020, for (a)36 and Shen et al., 2017, for (b),37 where no data was available to discriminate the WDR overpotential from ohmic losses of the ion-exchange layers). The membrane contributions, together with the ohmic losses of the electrolytes (if applicable) and voltage of the electrodes, result in the cell voltage.
Besides optimizing the resistance contribution of the individual layers, also the IL requires improvement, as it contributes in a similar order to the total membrane voltage by performing WDR when applied in reverse bias (see Figure 2). To obtain a BPM that can support currents in a technologically relevant range (>100 mA cm–2) at a reasonable cell voltage, introduction of water dissociation catalysts at the IL is necessary to further improve the kinetics. This was clearly demonstrated by Oener et al. (2020),35 based on a screening of a large number of different metal oxides. Both membrane layers at the IL have their own local pH and therefore optimal catalyst in the form of metal-oxide nanoparticles (e.g., IrO2 at CEL and NiO at the AEL interface side). This then lowers the water dissociation overpotential to 10 mV at 20 mA cm–2.35
To put the BPM-based energy losses in perspective, as in the case of a finite gap electrolysis cell (Figure 2a), reducing energy losses in the electrolyte and electrode is even more important than lowering the BPM voltage. Especially at higher current densities, the ohmic losses in the electrolyte take a significant amount of the energy losses. Here, zero gap configurations in combination with a membrane electrode assembly should be standard procedure for some applications like water electrolysis to optimize the overall performance (Figure 2b).36 The need for novel BPMs remains a necessity in these configurations, where on top of the ohmic losses and WDR overpotentials, the water diffusion gets limited at high current density and makes the membrane voltage increase rapidly.
At higher current densities, the diffusion rate of water has to be sufficient in order to avoid mass-transfer limitations, requiring a high water permeance. Commercial BPMs show a limiting current density of approximately 600 mA cm–2, which is equivalent to a water flux of 6.2 μmol s–1 cm–2.37 As applications like water electrolysis may operate at higher current densities, newly designed BPMs require a higher cutoff. The most straightforward route to tune the water permeance is by the membrane thickness: thinner layers increase the limiting water flux. In addition, a combination of highly hydrophilic membrane surfaces with a highly active membrane interface could alleviate the water transport limitations.
To improve the lifetime of BPMs, the AEL presents significantly more challenges than the CEL because of the intrinsic instability of common quaternary ammonium groups in alkaline environments. The challenge originates from the basicity and nucleophilicity of the hydroxide ion, which lead to different degradation mechanisms depending on conditions and particular structure. Hoffmann β elimination and different substitution or rearrangement reactions are commonly reported.38 This has triggered tremendous research toward stable quaternary ammonium head groups within the AEM community. The most successful degradation mitigation strategies include steric hindrance39 and integration of cyclic configurational or geometric features that increase the activation barrier of common degradation pathways.40,41 Backbone stability is another concern, particularly for AELs based on poly(arylene ethers) or other ether-linked backbone chemistries.42 The recent development in the field is therefore focusing on all-carbon-linked structures devoid of labile ether linkages, such as polyphenylenes,43 polycarbazoles,44 polyfluorenes,45 poly(arylene alkylenes),41 or aliphatic polymers.46
Another aspect of the chemical stability of the BPM is the interfacial compatibility between the AEL and CEL, which is an essential factor that needs to be considered in the design phase of novel BPM structures. First of all, good adhesion between the layers is needed to avoid delamination and blistering. Interfacial compatibility is also needed to be able to control and tune the depth, morphology, and composition of the boundary region where the WDR occurs, which is the key to develop high-performing BPMs.35,37 Given that the AEL represents the biggest challenge from a polymer electrolyte stability perspective, a rational way forward to improve the interfacial properties is to develop a CEL that is compatible with the most promising AEL chemistries that are available. Using CELs based on perfluorosulfonic acid derivatives is indeed attractive from a conductivity and stability point of view, but interfacial compatibility and adhesion to high-performing AEL chemistries are challenges. One way to mitigate adhesion limitations involves the development of 3D CEL-AEL interfaces, which not only increases the active contact area and overall water dissociation rate but also physically anchors the individual layers and thereby improves interfacial stability.36,37
The final important feature of an ideal BPM composition is low ion crossover, i.e., unwanted transport of ionic species present in the support electrolytes across both membrane layers. This lowers the selectivity of the WDR and, therefore, reduces the chemical potential of the surrounding electrolytes.20 However, this cannot be tuned independently from the previously discussed material properties, in particular the catalyst (favoring WDR), morphology of the interface, and thickness of the BPM. Tuning, e.g., ion-exchange capacity, swelling, and nanomorphology will therefore also impact the other components of an ideal BPM. In addition, operational parameters (like surrounding environment, thickness, and current density) also have a great impact.
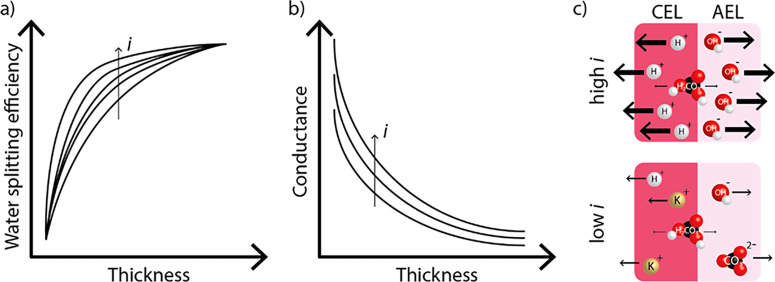
Lowering the thickness of the membrane layers increases the conductance and water permeance toward the IL, both enhancing the performance of the BPM at high current densities. However, a thin membrane layer also increases the ion crossover.47 If the thickness is doubled, the ion crossover through BPMs is more than halved.48 This requires a trade-off, as shown in Figure 3. Because cation crossover usually dominates anion crossover, asymmetry in the membrane layer thickness is a potential lever to further balance the water permeance and conductance at one hand, and ion crossover at the other hand. In particular, the CEL can be made very thin while still maintaining a high performance in terms of ion crossover, as recently demonstrated via simulations.17 The thickness of the AEL can also be reduced to boost the current density,49 but that affects the rejection of co-ions by the BPM.17 The recent pioneering works by Mayerhöfer et al. (2020)49 and Oener et al. (2021)50 show that highly asymmetric BPMs can support remarkably high currents, even in pure water, when the BPM junctions are installed near the electrodes. This mitigates mass transport limitations related to slow water diffusion, and sufficiently high water dissociation rates can thus be reached to maintain the steep pH gradient across the BPM interface, even in pure water.
Figure 3.
Effect of the thickness of the BPM (or its individual layers). Thicker membranes negatively impact the conductance while improving the water-splitting efficiency in favor of the WDR at the interface layer (see the Supporting Information for further explanation). Both parameters are influenced by the current density, as the flux of H+ and OH– increases faster than the flux of co-ions (schematically shown in (c)), resulting in a higher water-splitting efficiency and conductance.
As shown in Figure 3, the trade-off between rejection and conductance can be influenced by a different operational parameter: current density. As increasing the current density reduces the relative ion crossover significantly,20 the optimal trade-off will be favored toward lower thicknesses for applications that run at high current densities. Another operational parameter is the environment surrounding the membrane. Depending on the application, the membrane is contacted with a support electrolyte on zero, one, or both sides of the membrane. If no support electrolyte is used, substantial humidification of feed gases is needed to provide enough water for the cell reactions (e.g., CO2 reduction) and to keep conducting ionic groups dissociated. The type of electrolyte (or the absence of it) determines the local environment and has a major influence on the membrane potential. While neutral pH electrolytes result in a low thermodynamic potential, a high overpotential for the WDR is generally created.23 High concentrations of ions other than H+ and OH– result in a complex distribution of ionic species across the membrane, which compromises the Donnan potentials at the membrane–electrolyte interface. Moreover, these additional electrolytes also affect the ion conductivity of the individual layers of the membrane and the transference number for the different ionic species.23,51
To mitigate the lack of a sudden concentration jump at the electrolyte–membrane interface (resulting in high overpotentials) at near-neutral pH of the surrounding electrolyte, two strategies can be applied: (1) The diffusion of ionic species into the membrane layers can be accepted and therefore shift the boundary of the interface toward the membrane–membrane interface. This requires that catalysts at the BPM interface are geared toward near-neutral pH conditions, for instance, using catalysts based on graphene oxide52 or metal–organic frameworks.53 (2) Alternatively, efforts should be directed toward maintaining a sudden jump in pH at the membrane–electrolyte interfaces, which directly yields a Donnan potential that compensates the WDR potential. This sudden pH-jump at the membrane–electrolyte interface requires flow strategies to reduce the concentration polarization and membrane material with extremely high affinity for protons and hydroxide ions over other ions. In principle, such a membrane material exists in the form of an ice-based proton membrane but has obviously limited practical (liquid) water possibilities.54 Another option is operating with a pure water feed, avoiding the presence of ionic species in the membrane layer and circumventing the challenge to have strong relative affinity in multi-ionic systems.49,50
The surrounding electrolytes affect not only the membrane potential but also the ion crossover across the membrane. This exchange of ionic species compromises the water dissociation efficiency. This co-ion transport can be reduced by the type of electrolytes based on their ionic properties (valence, diffusion coefficient, size, etc.). Ions with higher valence or ionic size show significantly lower crossover, but the electrolytes with a higher ionic size typically feature a lower conductivity and solubility.20 Concentration profiles of different ionic species in the BPM and their diffusion and migration behavior as a function of current, temperature, and electrolyte composition are limited to early efforts.7,34 Electrochemical impedance spectroscopy in combination with ion speciation31 at different conditions would allow for investigating such properties.
As the current density is one of the most influential operational parameters for tuning the BPM crossover and conductance, we have mapped the different BPM applications on their typical current density. Figure 4 shows the different applications using a BPM with their relative current density and technology readiness level (TRL), which is determined based on the number of publications and conditions of BPM-facilitated systems in literature and industry (see Table SI1 in the Supporting Information). For the latter, only BPM electrodialysis qualifies as an industrially developed technology. The highest applied current densities are found in water electrolysis (>500 mA cm–2),37 while photoelectrochemistry operates typically at 2 orders of magnitude lower.55
Figure 4.
Schematic of the different applications in function of the current density and technology readiness level. The applications are, in order of increasing current density, photoelectrochemistry (PEC), flow batteries, bipolar membrane electrodialysis (BPMED, resource recovery), CO2 electrochemical reduction (CO2R), fuel cells (FC), and water electrolysis (HER).
The motivation for using a BPM in water electrolysis is directly associated with the lack of consensus about the optimal pH in electrolyzers; both acidic (PEM) and alkaline electrolyses are developed, each with corresponding electrocatalysts. In the realm of Earth-abundant materials, highly active oxygen evolution catalysts (e.g., Ni-based) operate almost exclusively in alkaline media. For the hydrogen evolution reaction in an acidic environment, platinum remains the state-of-the-art catalyst material, although transition metal phosphides, for example, have been demonstrated as potential substitutes.56 BPM-based electrolyzers have successfully been demonstrated to combine hydrogen evolution catalysts in acidic environments with oxygen evolution catalysts in alkaline environments at laboratory scale. However, as industrial electrolyzers typically operate between 100 and 1000 mA cm–2, the commercial BPMs fail to provide sufficient water dissociation kinetics to achieve energy-efficient water splitting. With thinner and highly asymmetric BPMs, an opportunity is available for BPM development for electrolysis, as demonstrated by Mayerhöfer et al. (2020).49 A cell with a BPM electrode assembly (where the anode, integrated with an thin AEL, is in contact with a Nafion membrane) achieved current densities as high as 8 A cm–2 at 2.2 V. This points to further exploration of highly asymmetric BPMs as a rational way forward.49
As an alternative to targeting high current density electrolysis, photo-driven systems can be considered, which usually operate at a low current density (typically 10 mA cm–2) due to the limited solar radiation flux. For those cases when the operating currents are very small, the high internal resistance of the BPMs is no longer troublesome. However, three other challenges appear. First, when making use of photoelectrodes in the system, a near-neutral pH is often required to provide a realistic electrode lifetime. As explained before, a near-neutral pH compromises the WDR efficiency and membrane conductivity. Moreover, to allow the use of both a photocathode and a photoanode, or a single photoelectrode with a non-transparent photovoltaic cell behind, frontal illumination is needed, which requires a transparent BPM. Such a transparent membrane has been presented in literature already, with a transmission of 75%.57 Third, at these low current densities, the ion crossover can be up to 10% of the charge for near-neutral pH electrolytes, which can be reduced by selecting electrolytes with high ionic sizes, as the low conductivity has a limited effect on the performance.20
Under CO2 electrolysis conditions, the electrochemical setup is similar to that of a water electrolyzer (including the benefit of the anode catalyst optimization), with the addition of a CO2-rich feed, e.g., using a gas diffusion electrode and CO2 in the vapor phase, allowing us to overcome the mass transport limitations in aqueous conditions.58 One of the biggest challenges with CO2 electrolyzers using traditional AEMs is the parasitic CO2 crossover. When using AEMs, bicarbonate ions are continuously generated at the cathode from hydroxide ions (released from the electrochemical reaction) and CO2 (from the cathode feed). In CO2 electrolyzers constructed around a BPM, on the other hand, parasitic CO2 transport can be completely eliminated, since the current is supported by the water dissociation and ionic separation, as shown experimentally in a liquid–liquid environment.1 The use of a (bicarbonate) buffer in the catholyte improves the CO2 reduction efficiency by suppressing the HER, but increases the thickness of the reactor. Recent work shows that, by reducing the acidity of the CEL, the buffer can be omitted as the HER is suppressed (up to 40% increase in efficiency).59 A remaining challenge is to reach faradaic efficiencies (FEs) similar to those in systems with an AEM, demanding further optimization of the CEL. While attempts to directly deposit the catalyst on the membrane layer reported a FE of 60% for CO at 25 mA cm–2, possibly suffering from the high acidity of the CEL,18 a viable method is to integrate a buffer (in various forms) between the cathode and CEL in combination. One report showed high FEs at current densities above 100 mA cm–2.60 Another showed a similar setup, reaching 90% FE toward formate at 500 mA cm–2.61 Important in these cases is to minimize the thickness of these buffer layers up to the order of 10 μm to reduce ohmic losses. Another approach to suppress the competing hydrogen evolution reaction at the cathode side under CO2 reduction conditions is to adjust the local pH of the CEL near the electrode by adding a weak-acid polymer layer.59 In these designs, the high membrane voltage remains a challenge, similarly as discussed in the resource recovery section.
In addition to usage of BPMs in electrolyzers, BPMs can be utilized for obtaining raw materials for energy technologies. Bipolar membrane electrodialysis (BPMED) processes for recovery of resources like ammonia15 and capture of CO216,62 present a promising alternative to the existing technologies. The recovery of ammonia and CO2 ultimately leverages the ability of BPMs to create a different pH in the concentrate stream compared to a diluate stream, which makes it possible to combine concentration and conversion to the desired product (e.g., NH3 and CO2(g)). At the same time, such a system is limited by the undesired crossover of neutral species and proton/hydroxide crossover, showing a current efficiency barely above 50% for the NH3 example.15 This is mainly associated with poor selectivity of membranes and the necessity to recirculate solutions to reach a high effluent concentration.26 The diffusion of neutrally charged species like NH3, H3PO4, and CO2 (H2CO3) cannot be solved with the same strategy of using asymmetric BPMs like in other applications,63 as charge selectivity is invalid in this case.15 Although the concentration of ionic species can be larger than that of the neutral species (especially for CO2 which has mediocre solubility in aqueous solutions), the unhindered crossover of neutrally charged species via diffusion can still exceed the crossover of ionic species at low current densities (up to 40 mA cm–2 for the example of 0.5 M phosphoric acid).20 As the crossover of such neutrally charged products like NH3 and CO2 is exclusively driven by diffusion, the crossover can be balanced at the expense of conductance by tuning the degree of cross-linking and thickness of the BPM.47
Although the energy consumption at lab scale is competitive with that of other technologies (e.g., 19 kJ/gN for NH3 recovery with BPMED15,26 against 30.6 kJ/gN with the classical ED64), further reducing the energy input is required to compete with fossil routes for NH3 and C-based raw materials. Strategies would involve improving stack design using thinner flow channels and, to some extent, using BPMs with reduced overpotential at the IL for the near-neutral operating pH in resource recovery. In BPMED systems for CO2 capture (e.g., from seawater), the extra energy constraint due to undesired water-splitting at the electrodes can be reduced by combined capture and conversion cells65 or reversible redox couples (e.g., K3/K4[Fe(CN)6]) at the electrodes.62
BPM fuel cells operating in the reverse bias mode are impractical from electrode kinetics and catalyst material points of view.10 Instead, the forward bias mode is a natural option for BPM fuel cells, where hydrogen oxidation takes place near the CEL and oxygen reduction near the AEL. The distinct proton concentrations in the CEL and AEL are favorable for both reactions in terms of electrode kinetics and catalyst selection. With the anode side at pH 0 and the cathode side at pH 14, the standard redox potential difference of a fuel cell operating in forward bias mode is 0.4 V, using both the electrode potentials for their respective local pH. On top of that, the potential across the interface within the BPM at a pH difference of 14 is 0.83 V, which constitutes a positive bias to the cell voltage so that a thermodynamic voltage of 1.23 V is obtainable.25 Open-circuit voltages close to those normally obtained for cells based on monopolar AEM and CEM chemistries (0.9–1.0 V) have been achieved when the BPM interfacial junctions were placed very near the electrode surfaces.10,25 In practice, such cell designs have been obtained by, e.g., introducing the AEL in the high-pH cathode catalyst layer and thereafter assembling the cell with a thick CEL based on a conventional Nafion membrane.10
Operation of fuel cells in the forward bias meets an issue of parasitic H2O transport and management. Water is supplied with oxygen on the cathode in order to generate OH– and with hydrogen on the anode to form hydrated protons (e.g., H3O+), which are the charge carriers through the AEL and CEL, respectively. As a result, the amount of water produced in the IL is at least 3 times that of the fuel cell reaction product. This, on the one hand, opens the possibility to eliminate the humidification, as demonstrated by Peng et al. (2015).66 On the other hand, ineffectual removal of the water from the IL may cause flooding and BPM delamination. However, this application has good potential, because there are no other ions that could cross over, as both sides have gas feed. The lack of published fuel cell data with symmetric BPMs points toward the development of highly asymmetric structures as the most rational way forward.
The forward bias mode is, alternating with the reverse bias mode, also used in flow batteries. The first implementation of a BPM in flow batteries was demonstrated in vanadium-metal hydride semi-flow systems.67 Such systems benefited from the unique operability of BPMs in maintaining a pH gradient, resulting in higher operating voltages of up to 2.4 V compared to the conventional all-vanadium redox flow battery systems utilizing monopolar membranes (∼1.2–1.3 V).68 However, the current density is low (<10 mA cm–2), which results in ion crossover, calling for highly selective membrane designs. Moreover, aqueous redox flow batteries sometimes produce highly oxidative species, such as VO2+, Ce4+, and Br2, which directly oxidize the functional groups in membranes, particularly the AEMs. Hence, the use of hydrocarbon BPMs with highly functional and stable AELs can essentially enhance the cycle life and bring down the capital cost.
A more recent route for BPM-based batteries is the acid/base flow battery system, using a BPM in reverse bias to charge the fluids and in forward bias for discharging.14,29,69,70 Being charged from a neutral NaCl solution into HCl and NaOH, the acid/base flow batteries benefit from more abundant resources compared to the vanadium redox flow batteries.69 The discharge mode (in forward bias) requires a membrane that is prone to water formation at the BPM interface, similar to the conditions in fuel cells. However, as the current densities in acid/base flow batteries are typically at least an order of magnitude smaller than in fuel cells (Figure 4), and as opportunities for membrane thickness tuning exist, this seems realistic for future BPM architectures. Another challenge is imposed by the supporting electrolyte involved in this system, as co-ion leakage can occur, particularly when working at high acid/base concentrations, potentially forming salts in the BPM interface. Also, specific to this technology is the relatively low power density of acid/base flow batteries (4–200 W m–2) compared to other flow battery systems,14,29 which means this technology calls for highly conductive and low-cost BPM materials. The priority for each membrane characteristic strongly differs per application, due to different electrolyte conditions and typical current densities that vary more than 2 orders of magnitude.
In conclusion, the various electrochemical energy applications demand an equally varying set of properties of the bipolar membrane. Although BPMs have been used for decades in acid/base production, no single energy conversion technology with a BPM has reached industrial application. In general, the BPM should feature highly conductive individual bulk layers, fast water dissociation or recombination kinetics at the interface, a long lifetime, high water permeability, and a low ion crossover. For each of these membrane characteristics, improvement strategies are already available, via material enhancements or tuning operational conditions, albeit often compromising another parameter. However, the priority for each membrane characteristic strongly differs per application, due to different electrolyte conditions and typical current densities that vary more than 2 orders of magnitude. While fuel cells, water, and CO2 electrolysis require fast kinetics at the interface layer, low ion crossover is more important to batteries and resource recovery. Hence, a dedicated approach to design BPMs for each application is needed, to fabricate new BPM designs that may be successfully implemented in industrial applications.
Acknowledgments
This research received funding from The Netherlands Organization for Scientific Research (NWO) under project number 733.000.008 in the framework of the Solar to Products programme co-funded by Shell Global Solutions International B.V., European Union’s Horizon 2020 research and innovation program under the Marie Sklodowska-Curie action (713683, COFUND fellows DTU), and Independent Research Fund Denmark (0217-00074B.)
Biographies
Marijn A. Blommaert is a Ph.D. candidate in the Smith group and Vermaas group at Delft University of Technology, in The Netherlands. His research focuses on the implementation of bipolar membranes in electrochemical applications. He graduated cum laude with his M.Sc. in chemical engineering from the University of Leuven in 2017.
David Aili received his Ph.D. in polymer chemistry and technology from the Technical University of Denmark (DTU) in 2011, and returned to DTU after a period in the polymer industry. He is currently a Senior Researcher and is active in the areas where organic chemistry meets materials science and sustainable energy technology.
Ramato Ashu Tufa is a double awardee of Marie Skłodowska-Curie Fellowship, currently at the Technical University of Denmark and formerly at the University of Chemistry and Technology Prague (UCTP). He received multiple Ph.D.s from the University of Calabria, University of Twente, and UCTP (2016). He explores membrane materials and processes for clean energy.
Qingfeng Li is a full professor at the Technical University of Denmark. His research areas include proton conducting electrolytes, catalysts, and energy technologies, particularly fuel cells and electrolyzers. He received his Ph.D. in electrochemistry from Northeastern University, China, in 1990, and was awarded the Doctor Degree of Technices at DTU in 2006.
Wilson A. Smith is an Associate Professor at TU Delft and the University of Colorado Boulder and a Senior Scientist at the U.S. National Renewable Energy Lab (NREL). His research focuses on scaling electrochemical processes from the nanoscale to MW-size systems.
David A. Vermaas is an assistant professor at TU Delft and leads the Electrochemical Flow Systems Lab. He obtained his Ph.D. degree in membrane technology (University of Twente), studied photoelelectrochemical systems afterwards, and now focuses on mass transport in electrochemical systems, including electrolysis, CO2 capture, redox flow batteries, and water technology.
Supporting Information Available
The Supporting Information is available free of charge at https://pubs.acs.org/doi/10.1021/acsenergylett.1c00618.
The authors declare no competing financial interest.
Supplementary Material
References
- Ma M.; Kim S.; Chorkendorff I.; Seger B. Role of ion-selective membranes in the carbon balance for CO2 electroreduction via gas diffusion electrode reactor designs. Chem. Sci. 2020, 11, 8854–8861. 10.1039/D0SC03047C. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Luo T.; Abdu S.; Wessling M. Selectivity of ion exchange membranes: A review. J. Membr. Sci. 2018, 555, 429–454. 10.1016/j.memsci.2018.03.051. [DOI] [Google Scholar]
- Frilette V. J. Preparation and characterization of bipolar ion-exchange membranes. J. Phys. Chem. 1956, 60, 435–439. 10.1021/j150538a013. [DOI] [Google Scholar]
- Wilhelm F. G.; Pünt I.; Van Der Vegt N. F. A.; Wessling M.; Strathmann H. Optimisation strategies for the preparation of bipolar membranes with reduced salt ion leakage in acid-base electrodialysis. J. Membr. Sci. 2001, 182, 13–28. 10.1016/S0376-7388(00)00519-6. [DOI] [Google Scholar]
- Ramírez P.; Rapp H. J.; Mafé S.; Bauer B. Bipolar membranes under forward and reverse bias conditions. Theory vs. experiment. J. Electroanal. Chem. 1994, 375, 101–108. 10.1016/0022-0728(94)03379-X. [DOI] [Google Scholar]
- Simons R.; Khanarian G. Water dissociation in bipolar membranes: Experiments and theory. J. Membr. Biol. 1978, 38, 11–30. 10.1007/BF01875160. [DOI] [Google Scholar]
- Wilhelm F. G.; Van Der Vegt N. F. A.; Strathmann H.; Wessling M. Current-voltage behaviour of bipolar membranes in concentrated salt solutions investigated with chronopotentiometry. J. Appl. Electrochem. 2002, 32, 455–465. 10.1023/A:1016317503435. [DOI] [Google Scholar]
- Vermaas D. A.; Smith W. A. Synergistic Electrochemical CO2 Reduction and Water Oxidation with a Bipolar Membrane. ACS Energy Lett. 2016, 1, 1143–1148. 10.1021/acsenergylett.6b00557. [DOI] [Google Scholar]
- Li Y. C.; Zhou D.; Yan Z.; Gonçalves R. H.; Salvatore D. A.; Berlinguette C. P.; Mallouk T. E. Electrolysis of CO2 to Syngas in Bipolar Membrane-Based Electrochemical Cells. ACS Energy Lett. 2016, 1, 1149–1153. 10.1021/acsenergylett.6b00475. [DOI] [Google Scholar]
- Unlu M.; Zhou J.; Kohl P. A. Hybrid Polymer Electrolyte Fuel Cells: Alkaline Electrodes with Proton Conducting Membrane. Angew. Chem. 2010, 122, 1321–1323. 10.1002/ange.200906021. [DOI] [PubMed] [Google Scholar]
- FCH JU . Development of Water Electrolysis in the European Union, 07/02/2014; https://www.fch.europa.eu/node/783.
- Schüttauf J.-W.; Modestino M. A.; Chinello E.; Lambelet D.; Delfino A.; Dominé D.; Faes A.; Despeisse M.; Bailat J.; Psaltis D.; Moser C.; Ballif C. Solar-to-Hydrogen Production at 14.2% Efficiency with Silicon Photovoltaics and Earth-Abundant Electrocatalysts. J. Electrochem. Soc. 2016, 163, F1177–F1181. 10.1149/2.0541610jes. [DOI] [Google Scholar]
- Vargas-Barbosa N. M.; Geise G. M.; Hickner M. A.; Mallouk T. E. Assessing the utility of bipolar membranes for use in photoelectrochemical water-splitting cells. ChemSusChem 2014, 7, 3017–3020. 10.1002/cssc.201402535. [DOI] [PubMed] [Google Scholar]
- Xia J.; Eigenberger G.; Strathmann H.; Nieken U. Flow battery based on reverse electrodialysis with bipolar membranes: Single cell experiments. J. Membr. Sci. 2018, 565, 157–168. 10.1016/j.memsci.2018.07.073. [DOI] [Google Scholar]
- van Linden N.; Bandinu G. L.; Vermaas D. A.; Spanjers H.; van Lier J. B. Bipolar membrane electrodialysis for energetically competitive ammonium removal and dissolved ammonia production. J. Cleaner Prod. 2020, 259, 120788. 10.1016/j.jclepro.2020.120788. [DOI] [Google Scholar]
- Sharifian R.; Wagterveld M.; Digdaya I. A.; Xiang C.; Vermaas D. A. Electrochemical carbon dioxide capture to close the carbon cycle. Energy Environ. Sci. 2021, 14, 781–814. 10.1039/D0EE03382K. [DOI] [Google Scholar]
- Bui J. C.; Digdaya I.; Xiang C.; Bell A. T.; Weber A. Z. Understanding Multi-Ion Transport Mechanisms in Bipolar Membranes. ACS Appl. Mater. Interfaces 2020, 12, 52509–52526. 10.1021/acsami.0c12686. [DOI] [PubMed] [Google Scholar]
- Blommaert M. A.; Sharifian R.; Shah N.; Nesbitt N. T.; Smith W. A.; Vermaas D. A. Orientation of bipolar membrane determines the dominant ion and carbonic species transport in membrane electrode assemblies for CO2 reduction. J. Mater. Chem. A 2021, 9, 11179. 10.1039/D0TA12398F. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Leonard M. E.; Clarke L. E.; Forner-Cuenca A.; Brown S. M.; Brushett F. R. Investigating Electrode Flooding in a Flowing Electrolyte, Gas-Fed Carbon Dioxide Electrolyzer. ChemSusChem 2020, 13, 400–411. 10.1002/cssc.201902547. [DOI] [PubMed] [Google Scholar]
- Blommaert M. A.; Verdonk J. A. H.; Blommaert H. C. B.; Smith W. A.; Vermaas D. A. Reduced Ion Crossover in Bipolar Membrane Electrolysis via Increased Current Density, Molecular Size, and Valence. ACS Appl. Energy Mater. 2020, 3, 5804–5812. 10.1021/acsaem.0c00687. [DOI] [Google Scholar]
- Pärnamäe R.; Mareev S.; Nikonenko V.; Melnikov S.; Sheldeshov N.; Zabolotskii V.; Hamelers H. V. M.; Tedesco M. Bipolar membranes: A review on principles, latest developments, and applications. J. Membr. Sci. 2021, 617, 118538. 10.1016/j.memsci.2020.118538. [DOI] [Google Scholar]
- Giesbrecht P. K.; Freund M. S. Recent Advances in Bipolar Membrane Design and Applications. Chem. Mater. 2020, 32, 8060–8090. 10.1021/acs.chemmater.0c02829. [DOI] [Google Scholar]
- Vermaas D. A.; Wiegman S.; Nagaki T.; Smith W. A. Ion transport mechanisms in bipolar membranes for (photo)electrochemical water splitting. Sustain. Energy Fuels 2018, 2, 2006–2015. 10.1039/C8SE00118A. [DOI] [Google Scholar]
- Oener S.; Ardo S.; Boettcher S. W. Ionic Processes in Water Electrolysis: The Role of Ion-selective Membranes. ACS Energy Lett. 2017, 2, 2625–2634. 10.1021/acsenergylett.7b00764. [DOI] [Google Scholar]
- Ünlü M.; Zhou J.; Kohl P. A. Hybrid anion and proton exchange membrane fuel cells. J. Phys. Chem. C 2009, 113, 11416–11423. 10.1021/jp903252u. [DOI] [Google Scholar]
- Rodrigues M.; De Mattos T. T.; Sleutels T.; Ter Heijne A.; Hamelers H. V. M.; Buisman C. J. N.; Kuntke P. Minimal Bipolar Membrane Cell Configuration for Scaling up Ammonium Recovery. ACS Sustainable Chem. Eng. 2020, 8, 17359–17367. 10.1021/acssuschemeng.0c05043. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Reiter R. S.; White W.; Ardo S. Communication—Electrochemical Characterization of Commercial Bipolar Membranes under Electrolyte Conditions Relevant to Solar Fuels Technologies. J. Electrochem. Soc. 2016, 163, H3132–H3134. 10.1149/2.0201604jes. [DOI] [Google Scholar]
- Daud S. S.; Norrdin M. A.; Jaafar J.; Sudirman R. The effect of material on bipolar membrane fuel cell performance: A review. IOP Conf. Ser.: Mater. Sci. Eng. 2020, 736, 032003. 10.1088/1757-899X/736/3/032003. [DOI] [Google Scholar]
- van Egmond W. J.; Saakes M.; Noor I.; Porada S.; Buisman C. J. N.; Hamelers H. V. M. Performance of an environmentally benign acid base flow battery at high energy density. Int. J. Energy Res. 2018, 42, 1524–1535. 10.1002/er.3941. [DOI] [Google Scholar]
- Pătru A.; Binninger T.; Pribyl B.; Schmidt T. J. Design Principles of Bipolar Electrochemical Co-Electrolysis Cells for Efficient Reduction of Carbon Dioxide from Gas Phase at Low Temperature. J. Electrochem. Soc. 2019, 166, F34–F43. 10.1149/2.1221816jes. [DOI] [Google Scholar]
- Blommaert M. A.; Vermaas D. A.; Izelaar B.; in ’t Veen B.; Smith W. A. Electrochemical impedance spectroscopy as a performance indicator of water dissociation in bipolar membranes. J. Mater. Chem. A 2019, 7, 19060–19069. 10.1039/C9TA04592A. [DOI] [Google Scholar]
- Yandrasits M. A.; Lindell M. J.; Hamrock S. J. New directions in perfluoroalkyl sulfonic acid-based proton-exchange membranes. Curr. Opin. Electrochem. 2019, 18, 90–98. 10.1016/j.coelec.2019.10.012. [DOI] [Google Scholar]
- Zhegur-Khais A.; Kubannek F.; Krewer U.; Dekel D. R. Measuring the true hydroxide conductivity of anion exchange membranes. J. Membr. Sci. 2020, 612, 118461. 10.1016/j.memsci.2020.118461. [DOI] [Google Scholar]
- Hurwitz H. D.; Dibiani R. Experimental and theoretical investigations of steady and transient states in systems of ion exchange bipolar membranes. J. Membr. Sci. 2004, 228, 17–43. 10.1016/j.memsci.2003.09.009. [DOI] [Google Scholar]
- Oener S. Z.; Foster M. J.; Boettcher S. W. Accelarating water dissociation in bipolar membranes and for electrocatalysis. Science (Washington, DC, U. S.) 2020, 369, 1099–1103. 10.1126/science.aaz1487. [DOI] [PubMed] [Google Scholar]
- Chen Y.; Wrubel J. A.; Klein W. E.; Kabir S.; Smith W. A.; Neyerlin K. C.; Deutsch T. G. High-Performance Bipolar Membrane Development for Improved Water Dissociation. ACS Appl. Polym. Mater. 2020, 2, 4559–4569. 10.1021/acsapm.0c00653. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Shen C.; Wycisk R.; Pintauro P. N. High performance electrospun bipolar membrane with a 3D junction. Energy Environ. Sci. 2017, 10, 1435–1442. 10.1039/C7EE00345E. [DOI] [Google Scholar]
- Marino M. G.; Kreuer K. D. Alkaline Stability of Quaternary Ammonium Cations for Alkaline Fuel Cell Membranes and Ionic Liquids. ChemSusChem 2015, 8, 513–523. 10.1002/cssc.201403022. [DOI] [PubMed] [Google Scholar]
- Fan J.; Wright A. G.; Britton B.; Weissbach T.; Skalski T. J. G.; Ward J.; Peckham T. J.; Holdcroft S. Cationic Polyelectrolytes, Stable in 10 M KOHaq at 100 °C. ACS Macro Lett. 2017, 6, 1089–1093. 10.1021/acsmacrolett.7b00679. [DOI] [PubMed] [Google Scholar]
- Dang H. S.; Jannasch P. A comparative study of anion-exchange membranes tethered with different hetero-cycloaliphatic quaternary ammonium hydroxides. J. Mater. Chem. A 2017, 5, 21965–21978. 10.1039/C7TA06029G. [DOI] [Google Scholar]
- Olsson J. S.; Pham T. H.; Jannasch P. Poly(arylene piperidinium) Hydroxide Ion Exchange Membranes: Synthesis, Alkaline Stability, and Conductivity. Adv. Funct. Mater. 2018, 28, 1702758. 10.1002/adfm.201702758. [DOI] [Google Scholar]
- Mohanty A. D.; Tignor S. E.; Krause J. A.; Choe Y.-K.; Bae C. Systematic Alkaline Stability Study of Polymer Backbones for Anion Exchange Membrane Applications. Macromolecules 2016, 49, 3361–3372. 10.1021/acs.macromol.5b02550. [DOI] [Google Scholar]
- Park E. J.; Maurya S.; Hibbs M. R.; Fujimoto C. H.; Kreuer K.-D.; Kim Y. S. Alkaline Stability of Quaternized Diels–Alder Polyphenylenes. Macromolecules 2019, 52, 5419–5428. 10.1021/acs.macromol.9b00853. [DOI] [Google Scholar]
- Cha M. S.; et al. Poly(carbazole)-based anion-conducting materials with high performance and durability for energy conversion devices. Energy Environ. Sci. 2020, 13, 3633–3645. 10.1039/D0EE01842B. [DOI] [Google Scholar]
- Pham T. H.; Allushi A.; Olsson J. S.; Jannasch P. Rational molecular design of anion exchange membranes functionalized with alicyclic quaternary ammonium cations. Polym. Chem. 2020, 11, 6953–6963. 10.1039/D0PY01291B. [DOI] [Google Scholar]
- Wang L.; Peng X.; Mustain W. E.; Varcoe J. R. Radiation-grafted anion-exchange membranes: The switch from low- to high-density polyethylene leads to remarkably enhanced fuel cell performance. Energy Environ. Sci. 2019, 12, 1575–1579. 10.1039/C9EE00331B. [DOI] [Google Scholar]
- Tedesco M.; Hamelers H. V. M.; Biesheuvel P. M. Nernst-Planck transport theory for (reverse) electrodialysis: III. Optimal membrane thickness for enhanced process performance. J. Membr. Sci. 2018, 565, 480–487. 10.1016/j.memsci.2018.07.090. [DOI] [Google Scholar]
- Moussaoui R. El; Pourcelly G.; Maeck M.; Hurwitz H. D.; Gavach C. Co-ion leakage through bipolar membranes. Influence of I-V responses and water-splitting efficiency. J. Membr. Sci. 1994, 90, 283–292. 10.1016/0376-7388(94)80078-2. [DOI] [Google Scholar]
- Mayerhöfer B.; McLaughlin D.; Böhm T.; Hegelheimer M.; Seeberger D.; Thiele S. Bipolar membrane electrode assemblies for water electrolysis. ACS Appl. Energy Mater. 2020, 3, 9635–9644. 10.1021/acsaem.0c01127. [DOI] [Google Scholar]
- Oener S. Z.; Twight L. P.; Lindquist G. A.; Boettcher S. W. Thin Cation-Exchange Layers Enable High-Current-Density Bipolar Membrane Electrolyzers via Improved Water Transport. ACS Energy Lett. 2021, 6, 1–8. 10.1021/acsenergylett.0c02078. [DOI] [Google Scholar]
- Münchinger A.; Kreuer K.-D. Selective ion transport through hydrated cation and anion exchange membranes I. The effect of specific interactions. J. Membr. Sci. 2019, 592, 117372. 10.1016/j.memsci.2019.117372. [DOI] [Google Scholar]
- Sun H.; Liu S.; Zhou G.; Ang H. M.; Tadé M. O.; Wang S. Reduced graphene oxide for catalytic oxidation of aqueous organic pollutants. ACS Appl. Mater. Interfaces 2012, 4, 5466–5471. 10.1021/am301372d. [DOI] [PubMed] [Google Scholar]
- Wang Q.; Wu B.; Jiang C.; Wang Y.; Xu T. Improving the water dissociation efficiency in a bipolar membrane with amino-functionalized MIL-101. J. Membr. Sci. 2017, 524, 370–376. 10.1016/j.memsci.2016.11.056. [DOI] [Google Scholar]
- Sleutels T.; Kaniadakis I.; Oladimeji O.; van der Kooi H.; Ter Heijne A.; Saakes M. An acid-doped ice membrane for selective proton transport. Int. J. Energy Res. 2021, 45, 8041–8048. 10.1002/er.6322. [DOI] [Google Scholar]
- Vermaas D. A.; Sassenburg M.; Smith W. A. Photo-assisted water splitting with bipolar membrane induced pH gradients for practical solar fuel devices. J. Mater. Chem. A 2015, 3, 19556–19562. 10.1039/C5TA06315A. [DOI] [Google Scholar]
- Ge Z.; Fu B.; Zhao J.; Li X.; Ma B.; Chen Y. A review of the electrocatalysts on hydrogen evolution reaction with an emphasis on Fe, Co and Ni-based phosphides. J. Mater. Sci. 2020, 55, 14081–14104. 10.1007/s10853-020-05010-w. [DOI] [Google Scholar]
- Chabi S.; Wright A. G.; Holdcroft S.; Freund M. S. Transparent Bipolar Membrane for Water Splitting Applications. ACS Appl. Mater. Interfaces 2017, 9, 26749–26755. 10.1021/acsami.7b04402. [DOI] [PubMed] [Google Scholar]
- Tufa R. A.; Chanda D.; Ma M.; Aili D.; Demissie T. B.; Vaes J.; Li Q.; Liu S.; Pant D. Towards highly efficient electrochemical CO2 reduction: Cell designs, membranes and electrocatalysts. Appl. Energy 2020, 277, 115557. 10.1016/j.apenergy.2020.115557. [DOI] [Google Scholar]
- Yan Z.; Hitt J. L.; Zeng Z.; Hickner M. A.; Mallouk T. E. Improving the efficiency of CO2 electrolysis by using a bipolar membrane with a weak-acid cation exchange layer. Nat. Chem. 2021, 13, 33–40. 10.1038/s41557-020-00602-0. [DOI] [PubMed] [Google Scholar]
- Salvatore D. A.; Weekes D. M.; He J.; Dettelbach K. E.; Li Y. C.; Mallouk T. E.; Berlinguette C. P. Electrolysis of Gaseous CO 2 to CO in a Flow Cell with a Bipolar Membrane. ACS Energy Lett. 2018, 3, 149–154. 10.1021/acsenergylett.7b01017. [DOI] [Google Scholar]
- Chen Y.; Vise A.; Klein W. E.; Cetinbas F. C.; Myers D. J.; Smith W. A.; Deutsch T. G.; Neyerlin K. C. A Robust, Scalable Platform for the Electrochemical Conversion of CO2 to Formate: Identifying Pathways to Higher Energy Efficiencies. ACS Energy Lett. 2020, 5, 1825–1833. 10.1021/acsenergylett.0c00860. [DOI] [Google Scholar]
- Digdaya I. A.; Sullivan I.; Lin M.; Han L.; Cheng W. H.; Atwater H. A.; Xiang C. A direct coupled electrochemical system for capture and conversion of CO2 from oceanwater. Nat. Commun. 2020, 11, 4412. 10.1038/s41467-020-18232-y. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Pronk W.; Biebow M.; Boller M. Treatment of source-separated urine by a combination of bipolar electrodialysis and a gas transfer membrane. Water Sci. Technol. 2006, 53, 139–146. 10.2166/wst.2006.086. [DOI] [PubMed] [Google Scholar]
- Tarpeh W. A.; Barazesh J. M.; Cath T. Y.; Nelson K. L. Electrochemical Stripping to Recover Nitrogen from Source-Separated Urine. Environ. Sci. Technol. 2018, 52, 1453–1460. 10.1021/acs.est.7b05488. [DOI] [PubMed] [Google Scholar]
- Li T.; Lees E. W.; Goldman M.; Salvatore D. A.; Weekes D. M.; Berlinguette C. P. Electrolytic Conversion of Bicarbonate into CO in a Flow. Cell. Joule 2019, 3, 1487–1497. 10.1016/j.joule.2019.05.021. [DOI] [Google Scholar]
- Peng S.; Xu X.; Lu S.; Sui P. C.; Djilali N.; Xiang Y. A self-humidifying acidic-alkaline bipolar membrane fuel cell. J. Power Sources 2015, 299, 273–279. 10.1016/j.jpowsour.2015.08.104. [DOI] [Google Scholar]
- Weng G.-M.; Li C.-Y. V.; Chan K.-Y. High Voltage Vanadium-Metal Hydride Rechargeable Semi-Flow Battery. J. Electrochem. Soc. 2013, 160, A1384–A1389. 10.1149/2.035309jes. [DOI] [Google Scholar]
- Liu C.; Chi X.; Han Q.; Liu Y. A High Energy Density Aqueous Battery Achieved by Dual Dissolution/Deposition Reactions Separated in Acid-Alkaline Electrolyte. Adv. Energy Mater. 2020, 10, 1903589. 10.1002/aenm.201903589. [DOI] [Google Scholar]
- Pärnamäe R.; Gurreri L.; Post J.; van Egmond W. J.; Culcasi A.; Saakes M.; Cen J.; Goosen E.; Tamburini A.; Vermaas D. A.; Tedesco M. The acid–base flow battery: Sustainable energy storage via reversible water dissociation with bipolar membranes. Membranes (Basel, Switz.) 2020, 10, 409. 10.3390/membranes10120409. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Morales-Mora M. A.; Pijpers J. J.H.; Antonio A. C.; Soto J. d. l. C.; Calderon A. M. A. Life cycle assessment of a novel bipolar electrodialysis-based flow battery concept and its potential use to mitigate the intermittency of renewable energy generation. J. Energy Storage 2021, 35, 102339. 10.1016/j.est.2021.102339. [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.