Fig. 4. Error budgeting repetition and surface codes.

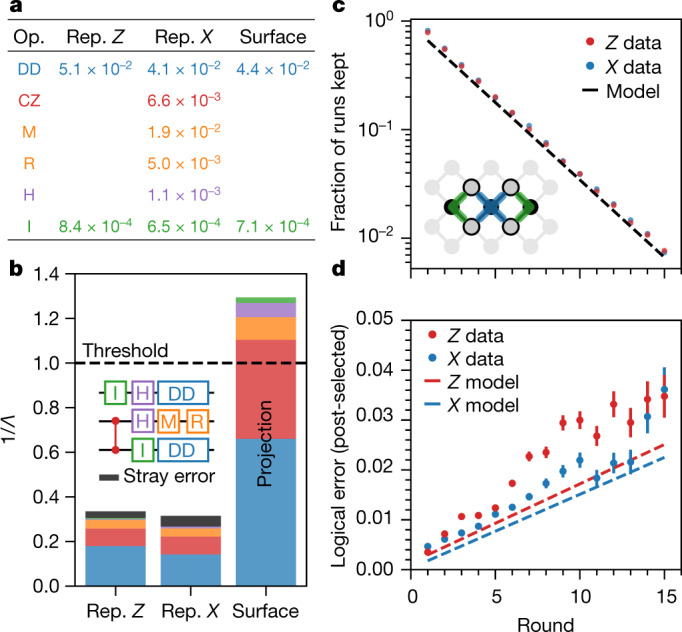
a, Depolarizing error probability (bit flip errors for M and R) for various operations in the stabilizer circuit, derived from averaging quantities in Fig. 1b. Note that the idle gate (I) and dynamical decoupling (DD) values depend on the code being run because the data qubits occupy different states. Op., operation; Rep., repetition. b, Estimated error budgets for the bit-flip and phase-flip codes, and projected error budget for the surface code, based on the depolarizing errors from a. The repetition code budgets slightly underestimate the experimental errors, and the discrepancy is labelled stray error. For the surface code, the estimated 1/Λ corresponds to the difference in ε_L between a d = 3 and d = 5 surface code, and is ~4 times higher than in the repetition codes owing to the more stringent threshold for the surface code. Rep., repetition c, For the d = 2 surface code, the fraction of runs that had no detection events versus number of rounds, plotted with the prediction from a similar error model as the repetition code (dashed line). Inset: physical qubit layout of the d = 2 surface code, seven qubits embedded in a 2D array. d, Probability of logical error in the surface code among runs with no detection events versus number of rounds. Depolarizing model simulations that do not include leakage or crosstalk (dashed lines) show good agreement. Error bars for c (not visible) and d are estimated standard error from binomial sampling with 240,000 experimental shots, minus the shots removed by post-selection in d.