The pnictogen bond: a quantitative molecular orbital picture

Abstract

We have analyzed the structure and stability of archetypal pnictogen-bonded model complexes D₃Pn⋯A⁻ (Pn = N, P, As, Sb; D, A = F, Cl, Br) using state-of-the-art relativistic density functional calculations at the ZORA-M06/QZ4P level. We have accomplished two tasks: (i) to compute accurate trends in pnictogen-bond strength based on a set of consistent data; and (ii) to rationalize these trends in terms of detailed analyses of the bonding mechanism based on quantitative Kohn–Sham molecular orbital (KS-MO) theory in combination with a canonical energy decomposition analysis (EDA) and Voronoi deformation density (VDD) analyses of the charge distribution. We have found that pnictogen bonds have a significant covalent character stemming from strong HOMO–LUMO interactions between the lone pair of A⁻ and σ* of D₃Pn. As such, the underlying mechanism of the pnictogen bond is similar to that of hydrogen, halogen, and chalcogen bonds.

Our quantitative molecular orbital analyses show that pnictogen bonds are not solely electrostatic phenomena, but also have a strongly stabilizing covalent component, just like chalcogen-, halogen-, and hydrogen bonds.

Introduction

The term pnictogen for the elements of the nitrogen group (group 15) was first proposed by van Arkel in the early 1950s.¹ Its etymology derives from the Ancient Greek root πνιγ (“choke”) and is a reference to the Dutch and German names for nitrogen, stikstof and Stickstoff, respectively, which literally mean “suffocation substance”. The trivalent pnictogen atom of a Lewis-acidic pnictogen-bond donor D₃Pn (Pn = group 15 atom) can engage in an intermolecular interaction, coined a pnictogen bond, with a Lewis-basic pnictogen-bond acceptor A⁻.² One of the first indications of Pn bonding appeared from stacked distibines and dibismuthines in crystal structures^3a and from intramolecular N⋯P contacts in hypervalent phosphorus compounds.^3b Later, a weak P⋯P interaction was identified via through-space coupling by NMR of phosphanyl-ortho-carbaboranes.^3c–e Since then, Pn bonding has flourished and emerged as a tool for coordination chemistry⁴ and catalysis.⁵ The nature of pnictogen bonds (similar to that of chalcogen and halogen bonds) is in general considered predominantly electrostatic,⁶ although the bonding mechanism of weak interactions is still under debate.^2b,7,8

In this study, we have computationally analyzed a range of pnictogen-bonded D₃Pn⋯A⁻ complexes (Pn = N, P, As, Sb; D, A = F, Cl, Br; see Scheme 1), using relativistic density functional theory (DFT) at the ZORA-M06/QZ4P level. One purpose of our work is to provide a set of consistent structural and energy data from which reliable trends can be inferred for a wide range of model systems. From these data, we have constructed a unified framework to rationalize the nature of pnictogen bonds, chalcogen bonds, halogen bonds, and hydrogen bonds, by studying the associated electronic structure and bonding mechanism.⁸

Scheme 1. Pnictogen-bonded D₃Pn⋯A⁻ model complexes (Pn = N, P, As, Sb; D, A = F, Cl, Br).

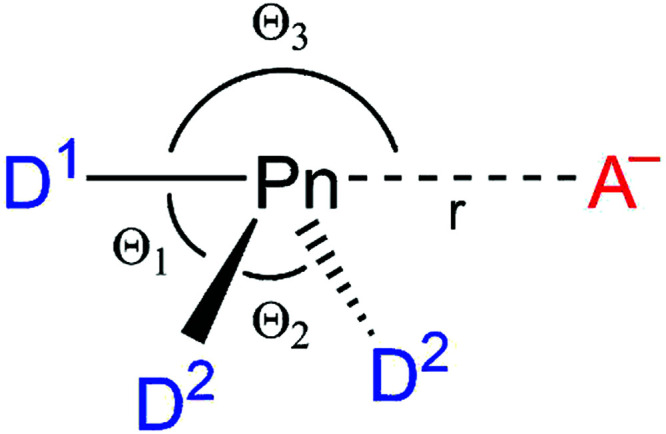
To this end, the pnictogen atom (Pn), the substituent (D), or the pnictogen bond accepting Lewis base (A⁻) are systematically varied to assess how the geometries and energies of our model complexes D₃Pn⋯A⁻ are affected. Activation strain analyses⁹ are performed on the formation of the pnictogen-bond complexes to understand the origin of the computed trends. As part of these analyses, the underlying bonding mechanism is elucidated in the context of Kohn–Sham molecular orbital (MO) theory in combination with a matching energy decomposition analysis (EDA) as implemented in the Amsterdam Density Functional (ADF) program.^10,11 Our analyses along the entire reaction profile for each of the pnictogen-bond complexation reactions demonstrate that pnictogen bonds are not at all purely electrostatic phenomena. Instead, they are, to a substantial extent, covalent in nature, very similar to chalcogen bonds, halogen bonds, and hydrogen bonds.

Theoretical methods

Computational details

All calculations were carried out using the Amsterdam Density Functional (ADF) 2017.103 program.¹¹ The equilibrium geometries and energies of pnictogen-bonded complexes were computed at the DFT level using the meta-hybrid functional M06.¹² A large uncontracted relativistically optimized QZ4P Slater type orbital (STO) basis set containing diffuse functions was used. The QZ4P all-electron basis set,¹³ no frozen-core approximation, is of quadruple-ζ quality for all atoms and has been augmented with the following sets of polarization and diffuse functions: two 3d and two 4f on nitrogen and fluorine, three 3d and two 4f on phosphorus and chlorine, two 4d and three 4f on arsenic and bromine, and one 5d and three 4f on antimony and iodine. The molecular density was fitted by the systematically improvable Zlm fitting scheme. Scalar relativistic effects were accounted for using the zeroth-order regular approximation (ZORA) Hamiltonian.¹⁴

Analysis of the bonding mechanism

Insight into the bonding mechanism is obtained through activation strain analyses of the various pnictogen bond formation reactions. These complexation reactions are computationally modeled by decreasing the distance between A⁻ and the Pn atom of the D₃Pn fragment, allowing the system to geometrically relax at each point. The D₃Pn⋯A⁻ distance is increased, starting from the equilibrium geometry in the pnictogen-bonded complex (r_Pn⋯A), to a value of 5.300 Å. Thus, each analysis starts from an optimized D₃Pn⋯A⁻ complex, which is then transformed into the D₃Pn molecule and a halide at a relatively large distance.

These complexation reactions are analyzed using the activation strain model. The activation strain model of chemical reactivity⁹ is a fragment-based approach to understand the energy profile of a chemical process in terms of the original reactants. Thus, the potential energy surface ΔE(ζ) is decomposed along the reaction coordinate ζ (or just at one point along ζ) into the strain energy ΔE_strain(ζ), which is associated with the geometrical deformation of the individual reactants as the process takes place, plus the actual interaction energy ΔE_int(ζ) between the deformed reactants [eqn (1)].

ΔE(ζ) = ΔE_strain(ζ) + ΔE_int(ζ) 1

In the equilibrium geometry, that is, for ζ = ζ_eq, this yields an expression for the bond energy ΔE(ζ_eq) = ΔE_strain + ΔE_int. The PyFrag program was used to facilitate the analyses along the reaction coordinate ζ of the bond formation processes.¹⁵ The interaction energy ΔE_int(ζ) between the deformed reactants is further analyzed in the conceptual framework provided by the quantitative Kohn–Sham MO model.¹⁰ To this end, it is decomposed into three physically meaningful terms [eqn (2)] using a quantitative energy decomposition analysis (EDA) as implemented in ADF.^10,11

ΔE_int(ζ) = ΔV_elstat(ζ) + ΔE_Pauli(ζ) +ΔE_oi(ζ) 2

The usually attractive term ΔV_elstat corresponds to the classical Coulomb interaction between the unperturbed charge distributions of the deformed reactants and has four components [eqn (3)]: (i) the electrostatic repulsion between the electron densities of fragments 1 and 2, ΔV_{elstat,ρ1ρ2}; (ii) the electrostatic attraction between the nucleus of fragment 1 and the electron density of fragment 2, ΔV_elstat,n1ρ2; (iii) the electrostatic attraction between the electron density of fragment 1 and the nucleus of fragment 2, ΔV_elstat,ρ1n2; and (iv) the electrostatic repulsion between the nuclei of fragments 1 and 2, ΔV_elstat,n1n2.

ΔV_elstat(ζ) = ΔV_{elstat,ρ1ρ2}(ζ) + ΔV_elstat,n1ρ2(ζ) + ΔV_elstat,ρ1n2(ζ) + ΔV_elstat,n1n2(ζ) 3

The Pauli repulsion energy (ΔE_Pauli) comprises the destabilizing interactions between occupied orbitals of one reactant and those of another reactant and is responsible for steric repulsion. The orbital-interaction energy (ΔE_oi) accounts for charge transfer, that is, the interaction between occupied orbitals of one fragment and unoccupied orbitals of the other fragment, including the interactions of the highest occupied and lowest unoccupied MOs (HOMO–LUMO), and polarization, that is, empty–occupied orbital mixing on one fragment, due to the presence of another fragment.

The electron density distribution is analyzed using the Voronoi deformation density (VDD) method for computing atomic charges.¹⁶ The VDD atomic charge on atom X in a molecule (Q^VDD_X) is computed as the (numerical) integral of the deformation density in the volume of the Voronoi cell of atom X [eqn (4)]. The Voronoi cell of atom X is defined as the compartment of space bounded by the bond midplanes on and perpendicular to all bond axes between nucleus X and its neighboring nuclei.

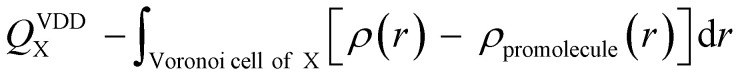4

Here, the deformation density is the difference between ρ(r), i.e., the electron density of the overall molecule or complex, and ρ_promolecule(r) = ∑_Yρ_Y(r), i.e., the superposition of spherical average-of-configuration atomic densities ρ_Y(r) of each atom Y in the fictitious promolecule without chemical interactions, in which all atoms are considered neutral. The interpretation of the VDD charge Q^VDD_Pn is rather straightforward and transparent: instead of measuring the amount of charge associated with a particular atom Pn, Q^VDD_Pn directly monitors how much charge flows out of (Q^VDD_Pn > 0) or into (Q^VDD_Pn < 0) the Voronoi cell of atom Pn due to chemical interactions.

The VDD scheme can also be used to directly compute how much charge flows into or out of an atomic Voronoi cell X in an overall complex (e.g., [D₃Pn⋯A]⁻) relative to two (poly)atomic molecular fragments (e.g., D₃Pn and A⁻), instead of spherical atoms, as shown in eqn (5).

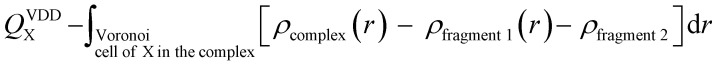5

ΔQ^VDD_X is a measure of how the atomic charge of atom X changes due to the bonding between the fragments. In this work, eqn (5) is used to compute the flow of electrons from the halide A⁻ to the pnictogen-bond donating molecule D₃Pn (see 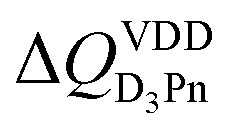in Tables 1 and 3).

Activation strain analyses (in kcal mol⁻¹) of a representative set of D₃Pn⋯A⁻ at the equilibrium geometries (in Å, deg.)^a.

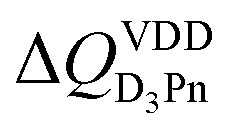
D3Pn⋯A−	ΔE	ΔEstrain	ΔEint		r Pn⋯A	ΔrD1–Pn	ΔrD2–Pn	Θ 3	ΔΘ1
F3N⋯F−	−11.8	32.5	−44.3	−0.30	1.859	0.503	−0.017	170.3	−8.9
F3N⋯Cl−	−3.5	0.8	−4.3	−0.01	3.239	0.048	−0.009	168.3	−1.5
F3N⋯Br−	−2.9	0.5	−3.5	0.00	3.484	0.040	−0.008	166.8	−1.2
Cl3N⋯F−	−30.4	55.7	−86.1	−0.65	1.416	1.490	0.001	170.7	−25.7
Cl3N⋯Cl−	−5.6	22.9	−28.5	−0.36	2.328	0.575	0.004	146.6	−7.8
Cl3N⋯Br−	−6.2	3.5	−9.7	−0.18	2.920	0.149	0.024	145.6	−2.8
Br3N⋯F−	−30.2	53.2	−83.3	−0.67	1.411	1.417	0.022	165.7	−24.0
Br3N⋯Cl−	−8.0	2.0	−10.0	−0.18	2.813	0.111	0.026	149.4	−2.8
Br3N⋯Br−	−7.0	17.2	−24.2	−0.44	2.322	0.416	0.066	127.2	−3.1
F3P⋯F−	−48.9	17.4	−66.4	−0.35	1.753	0.189	0.044	189.4	−10.6
F3P⋯Cl−	−16.0	4.4	−20.4	−0.12	2.700	0.083	0.016	183.4	−5.6
F3P⋯Br−	−12.9	3.0	−15.9	−0.09	2.982	0.067	0.012	181.6	−4.6
Cl3P⋯F−	−67.4	31.8	−99.3	−0.52	1.649	0.572	0.048	181.4	−11.8
Cl3P⋯Cl−	−25.5	14.4	−39.8	−0.31	2.370	0.315	0.034	174.6	−8.7
Cl3P⋯Br−	−20.5	10.7	−31.3	−0.26	2.617	0.263	0.030	172.9	−7.6
Br3P⋯F−	−71.0	30.2	−101.2	−0.56	1.637	0.647	0.045	175.4	−11.3
Br3P⋯Cl−	−28.5	15.4	−43.9	−0.37	2.312	0.382	0.038	172.0	−8.9
Br3P⋯Br−	−23.4	11.9	−35.3	−0.32	2.550	0.323	0.034	170.6	−7.9
F3Sb⋯F−	−72.0	8.8	−80.8	−0.31	2.037	0.144	0.037	193.9	−8.9
F3Sb⋯Cl−	−38.9	5.8	−44.7	−0.21	2.643	0.112	0.030	188.3	−7.3
F3Sb⋯Br−	−33.6	5.0	−38.7	−0.19	2.840	0.103	0.028	186.7	−6.8
Cl3Sb⋯F−	−77.1	12.3	−89.4	−0.38	2.017	0.301	0.051	183.7	−7.6
Cl3Sb⋯Cl−	−42.7	8.9	−51.7	−0.28	2.592	0.244	0.045	178.4	−6.5
Cl3Sb⋯Br−	−37.3	8.0	−45.3	−0.27	2.780	0.229	0.043	177.0	−6.1
Br3Sb⋯F−	−77.7	11.5	−89.2	−0.41	2.014	0.332	0.049	181.2	−7.2
Br3Sb⋯Cl−	−43.5	8.5	−52.0	−0.31	2.580	0.272	0.046	175.6	−6.1
Br3Sb⋯Br−	−38.1	7.7	−45.8	−0.30	2.766	0.256	0.045	174.2	−5.8

^aComputed at the ZORA-M06/QZ4P level. For a full set of data, see Tables S1 and S2 in the ESI.

Energy decomposition analyses (in kcal mol⁻¹) of a representative set of D₃Pn⋯A⁻ at the equilibrium geometries^a.

D3Pn⋯A−	ΔEint	ΔVelstat	ΔEPauli	ΔEoi	ε(5a′)	〈5a′|npy〉	〈4a′|npy〉	Pop5a′	Popnpy
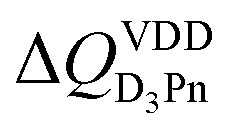
F3N⋯F−	−44.3	−66.9	89.6	−67.0	−5.1	0.12	0.05	0.40	1.68	−0.30
F3N⋯Cl−	−4.3	−5.8	4.5	−3.1	–0.2	0.10	0.05	0.02	1.99	−0.01
F3N⋯Br−	−3.5	−4.7	3.5	−2.3	−0.1	0.10	0.04	0.02	2.00	0.00
Cl3N⋯F−	−86.1	−208.3	431.6	−309.5	−6.5	0.13	0.08	1.24	1.34	−0.65
Cl3N⋯Cl−	−28.5	−41.8	69.2	−55.9	−5.2	0.11	0.06	0.54	1.59	−0.36
Cl3N⋯Br−	−9.7	−11.9	18.8	−16.7	−3.7	0.09	0.03	0.26	1.75	−0.18
Br3N⋯F−	−83.3	−204.1	439.6	−318.8	−6.0	0.12	0.07	1.25	1.38	−0.67
Br3N⋯Cl−	−10.0	−10.3	19.2	−18.9	−3.6	0.08	0.04	0.23	1.86	−0.18
Br3N⋯Br−	−24.2	−51.1	95.0	−68.1	−4.6	0.12	0.08	0.67	1.60	−0.44
F3P⋯F−	−66.4	−167.7	221.7	−120.3	−2.1	0.15	0.19	0.28	1.75	−0.35
F3P⋯Cl−	−20.4	−40.4	46.0	−25.9	−1.2	0.22	0.17	0.17	1.87	−0.12
F3P⋯Br−	−15.9	−29.1	30.7	−17.4	–1.1	0.25	0.16	0.18	1.81	−0.09
Cl3P⋯F−	–99.3	−222.6	312.1	−188.7	−4.1	0.16	0.13	0.48	1.70	−0.52
Cl3P⋯Cl−	−39.8	−84.8	119.2	−74.2	−3.1	0.20	0.12	0.40	1.73	−0.31
Cl3P⋯Br−	−31.3	−64.2	86.5	−53.6	−2.9	0.20	0.08	0.36	1.75	−0.26
Br3P⋯F−	−101.2	−228.4	331.2	−204.1	−4.2	0.15	0.12	0.53	1.69	−0.56
Br3P⋯Cl−	−43.9	−95.9	141.9	−89.8	−3.4	0.19	0.13	0.45	1.72	−0.37
Br3P⋯Br−	−35.3	−73.7	104.8	−66.5	−3.2	0.20	0.12	0.42	1.72	−0.32
F3Sb⋯F−	−80.8	−148.9	143.6	−75.5	−3.0	0.16	0.15	0.20	1.82	−0.31
F3Sb⋯Cl−	−44.7	−82.6	83.4	−45.4	−2.7	0.23	0.18	0.21	1.83	−0.21
F3Sb⋯Br−	−38.7	−70.7	70.9	−38.9	−2.7	0.24	0.18	0.21	1.84	−0.19
Cl3Sb⋯F−	−89.4	−158.0	161.0	−92.4	−3.4	0.16	0.11	0.26	1.79	−0.38
Cl3Sb⋯Cl−	−51.7	−93.3	100.9	−59.2	−3.2	0.22	0.11	0.28	1.80	−0.28
Cl3Sb⋯Br−	−45.3	−81.1	87.3	−51.5	−3.1	0.23	0.10	0.28	1.82	−0.27
Br3Sb⋯F−	−89.2	−157.4	166.1	−97.9	−3.4	0.16	0.10	0.28	1.79	−0.41
Br3Sb⋯Cl−	–52.0	−95.2	106.8	−63.5	−3.2	0.21	0.11	0.29	1.81	−0.31
Br3Sb⋯Br−	−45.8	−83.2	92.7	−55.3	−3.2	0.22	0.11	0.30	1.81	−0.30

^aComputed at the ZORA-M06/QZ4P level; ε(5a′) = 5a′ orbital energy of the prepared D₃Pn fragment (in eV); 〈Φ|np〉 = overlap between the Φ orbital of the D₃Pn fragment (see Fig. 2) and one of the np orbitals of the halide A⁻; Pop = Gross population (in electrons) of the indicated orbital. For a full set of data, see Table S2 in the ESI.

Results and discussion

Pnictogen bond strength and structure

Table 1 summarizes the results of our ZORA-M06/QZ4P calculations for a representative selection of nitrogen-, phosphorus-, and antimony-bonded model complexes D₃Pn⋯A⁻, covering D, A = F, Cl, and Br (for the complete dataset see Tables S1 and S2, ESI†). These model reactions go with a single-well potential energy surface (PES), that is, there is no energy barrier separating the reactants from their resulting product. In the cases where D ≠ A, C_S symmetric complexes with D¹–Pn bond lengths different from the Pn⋯A⁻ bond and with bond angles Θ₁ ≠ Θ₂ are formed. For the cases where D = A, C_2v symmetric complexes with equal bond distances r_D¹–Pn = r_Pn⋯A are formed (see Table 1).

The pnictogen bonds D₃Pn⋯A⁻ become stronger and longer upon descending group 15 in the periodic table, going from N to Sb. The pnictogen bonds become weaker and longer as the accepting halide (A⁻) goes down group 17, from F⁻ to Br⁻. The elongation of the bonds descending the periodic table originates from the increase in the effective size of the atoms involved. In the case of the antimony-bonded complexes Br₃Sb⋯A⁻, for example, ΔE weakens from a value of −78 kcal mol⁻¹ for A⁻ = F⁻ to −38 kcal mol⁻¹ for A⁻ = Br⁻ (see Table 1). The associated Sb⋯A⁻ bond elongates from around 2.0 Å for A⁻ = F⁻ to around 2.8 Å for A⁻ = Br⁻. From A⁻ = F⁻ to Br⁻, the nitrogen bond in Br₃N⋯A⁻ weakens from a value of −30 kcal mol⁻¹ to −7 kcal mol⁻¹. The associated N⋯A⁻ bond elongates from a value of around 1.4 Å for A⁻ = F⁻ to around 2.3 Å for A⁻ = Br⁻. The reason behind the trends in stability will be discussed later.

The strengths of the heavier pnictogen bonds D₃Pn⋯A⁻ are minimally affected upon variation of the substituent D. For example, along the series from F₃Sb⋯F⁻ to Br₃Sb⋯F⁻, the bond strength varies only from −72.0 to −77.7 kcal mol⁻¹, the antimony bond distance r_Pn⋯A decreases slightly from 2.037 to 2.014 Å, and the stretch Δr_D¹–Pn upon bond formation increases from 0.144 to 0.332 Å. The nitrogen bonds D₃N⋯A⁻ behave differently and become significantly stronger and shorter as D is varied from F to Br (see Table 1). For example, along the series from F₃N⋯F⁻ to Br₃N⋯F⁻, the nitrogen bond strengthens from a ΔE value of −11.8 to −30.2 kcal mol⁻¹, the nitrogen bond distance r_Pn⋯A decreases in value from 1.859 to 1.411 Å, and the stretch Δr_D¹–Pn significantly increases from 0.503 to 1.417 Å. A comprehensive analysis of the origin of these trends is provided in the following.

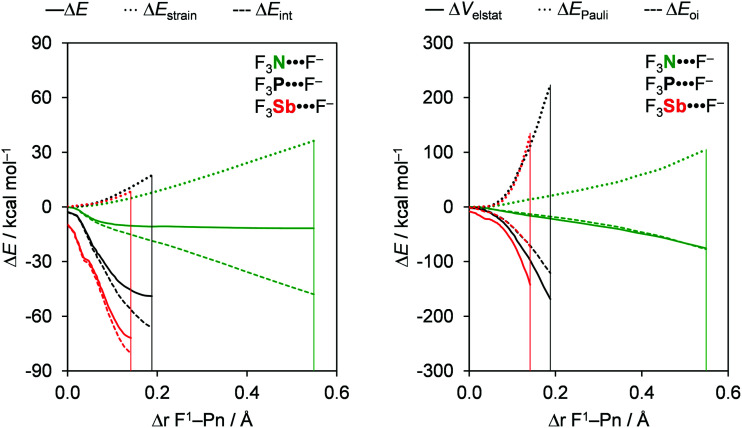
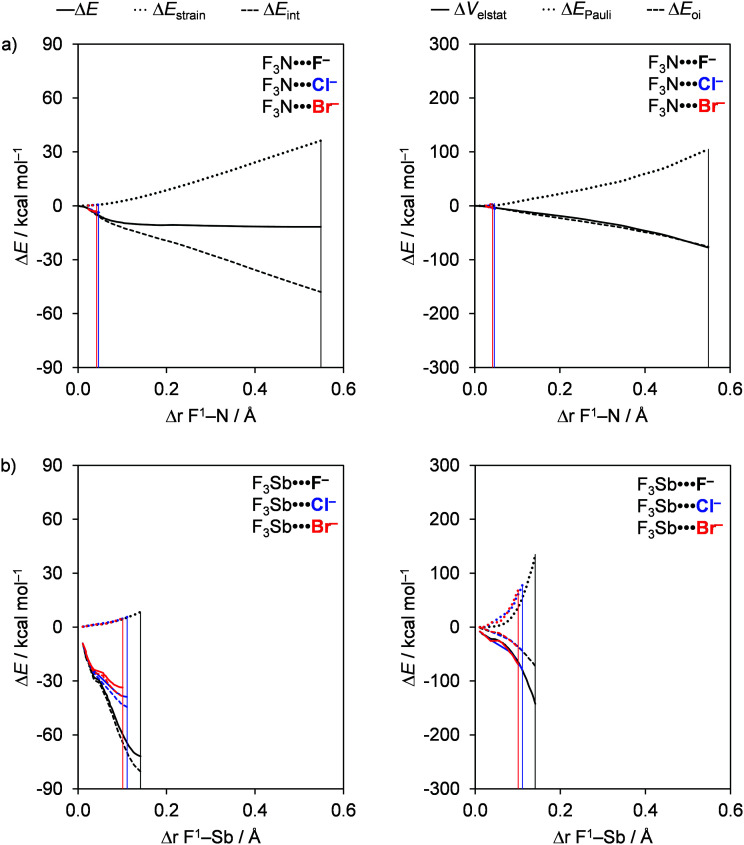
Bond analyses with the variation of Pn

The pnictogen bond D₃Pn⋯A⁻ strength ΔE increases as Pn varies along N, P, As, and Sb when the donating atom (D) and the accepting halide (A⁻) remain unchanged and the trend in ΔE is mainly set by the interaction energy ΔE_int. For example, from F₃N⋯F⁻ to F₃Sb⋯F⁻, ΔE is strengthened from a value of −11.8 to −72.0 kcal mol⁻¹ and ΔE_int is strengthened from a value of −44.3 to −80.8 kcal mol⁻¹ (see Table 1). The trend in ΔE is reinforced by the strain energy (ΔE_strain), which becomes less destabilizing from 32.5 to 8.8 kcal mol⁻¹ along the same series. We extend our analysis to the entire reaction coordinate ζ, projected onto the stretch in the D¹–Pn bond, Δr_D¹–Pn, that occurs as the pnictogen-bond accepting A⁻ atom approaches the D₃Pn molecule (see the Theoretical methods section). The activation strain and energy decomposition diagrams (ASD and EDD) for a representative example series, namely F₃N⋯F⁻ to F₃Sb⋯F⁻, are given in Fig. 1 (for the complete dataset, see Tables S1 and S2 in the ESI†). Notably, the trend in bond energy ΔE(ζ) is in fact determined by ΔE_int(ζ), which strengthens when going from Pn = N to Sb (Fig. 1, left), whereas the ΔE_strain(ζ) curves are relatively similar. In fact, only in the equilibrium geometries, the strain term ΔE_strain(ζ_eq) become less destabilizing from Pn = N to Sb. The reason is that as the interaction curve becomes steeper along the series, it pulls the equilibrium geometry [which results from the balance between ΔE_strain(ζ) and ΔE_int(ζ)] to an earlier stage along the reaction coordinate, at which the system is less distorted (i.e., a less expanded F¹–Pn bond in the F₃Pn fragment) and thus less strained, as reflected by ΔE_strain(ζ_eq) (see Table 1).

Fig. 1. Activation strain (left panel) and energy decomposition (right panel) analyses of a representative set of F₃Pn⋯F⁻ pnictogen-bonded complexes (green, Pn = N; black, Pn = P; red, Pn = Sb). The vertical lines indicate the position of the stationary points. For a full set of data, see Fig. S2 in the ESI.†.

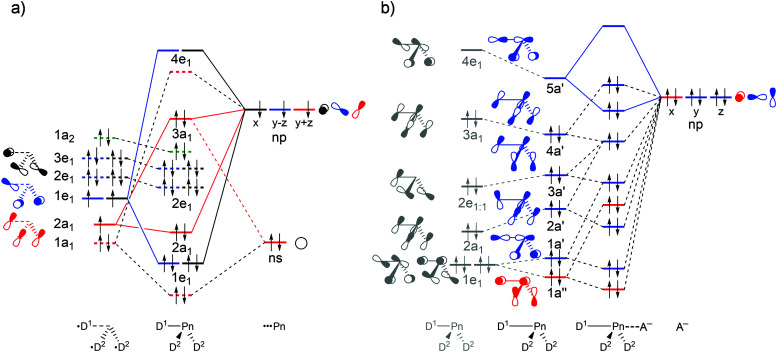
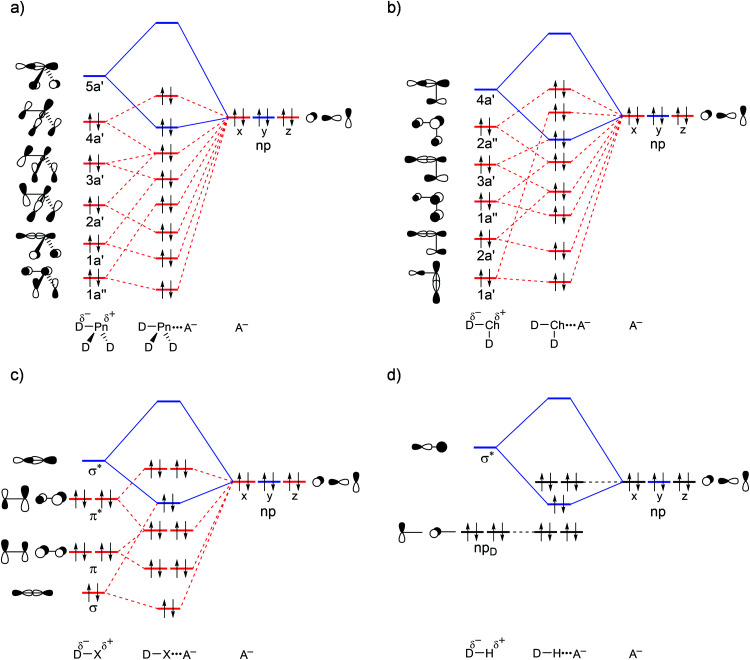
To understand the trends in ΔE_int(ζ), we further decomposed ΔE_int into the individual energy components (Fig. 1, right; for a full set of data, see Fig. S2 in the ESI†). The strengthening of ΔE_int(ζ) and, consequently, the increasing stabilization of D₃Pn⋯A⁻ as Pn varies along N, P, As, and Sb is caused by the rising electronegativity difference across the D–Pn bonds as Pn descends in the periodic table. Firstly, this causes the Pn atom to become increasingly positive along N, P, As, and Sb (see the VDD atomic charges in Table 2), resulting in the ΔV_elstat(ζ) curves being the least stabilizing for Pn = N and the most stabilizing for Pn = Sb. For example, the VDD atomic charge on Pn in F₃N, F₃P, F₃As, and F₃Sb amounts to +0.21, +0.33, +0.51, and +0.57 a.u., respectively. Secondly, this causes, among other effects that will be explained later, the σ* D–Pn antibonding 5a′ acceptor orbital to have higher amplitude on Pn (see Fig. 2), resulting in stronger HOMO–LUMO overlap and thus more stabilizing orbital interactions. These features are also observed for chalcogen bonds D₂Ch⋯A⁻, halogen bonds DX⋯A⁻, and hydrogen bonds DH⋯A⁻, which makes them similar to pnictogen bonds.⁸

Bond lengths (in Å), bond angle (in deg.), VDD charge (in a.u.), orbital energies (in eV) and the homolytic bond dissociation energy without ZPE (in kcal mol⁻¹) of isolated D₃Pn fragments^a.

D3Pn	r D1–Pn	r D2–Pn	Θ 1	Θ 2	Q VDD Pn	ε(1e1)	ε(2a1)	ε(3a1)	ε(4e1)	BDED–Pnb
F3N	1.356	1.356	101.9	101.9	0.21	−18.2	−16.8	−10.3	0.6	59.4
Cl3N	1.753	1.753	107.7	107.7	0.01	−14.4	−13.0	−8.2	−1.3	35.6
Br3N	1.906	1.906	108.5	108.5	−0.09	−13.1	−11.8	−7.6	−1.4	30.2
F3P	1.564	1.564	97.5	97.5	0.33	−16.1	−15.1	−9.3	−0.5	133.6
Cl3P	2.055	2.055	100.4	100.4	0.32	−12.8	−11.7	−8.3	−1.4	79.0
Br3P	2.227	2.227	100.9	100.9	0.25	−11.8	−10.8	−7.9	−1.7	60.8
F3As	1.717	1.717	95.8	95.8	0.51	−14.7	−13.8	−10.1	−1.3	116.4
Cl3As	2.179	2.179	99.1	99.1	0.44	−12.2	−11.2	−8.7	–1.7	76.4
Br3As	2.342	2.342	99.7	99.7	0.37	−11.3	−10.4	−8.1	−1.9	59.7
F3Sb	1.893	1.893	94.3	94.3	0.57	−13.5	−12.8	−9.7	−1.9	117.9
Cl3Sb	2.348	2.348	97.0	97.0	0.55	−11.5	−10.6	−8.5	−2.1	82.8
Br3Sb	2.510	2.510	97.7	97.7	0.49	−10.7	−9.9	−8.0	−2.2	66.2

^aComputed at the ZORA-M06/QZ4P level.

^bEnergy for the reaction D₃Pn → D₂Pn˙ + D˙.

Fig. 2. Schematic molecular orbital diagram for (a) isolated D₃Pn fragments with C_3v symmetry (red: a₁; green: a₂; blue: e_1:1; black: e_1:2) and (b) D₃Pn⋯A⁻ complexes. The first column in (b) refers to the isolated D₃Pn fragment and the second column refers to the D₃Pn fragment deformed to its C_s symmetric geometry in the complex (blue: a′; red: a′′), in which one D–Pn bond has been elongated. See Fig. S1 in the ESI† for computed 3D isosurfaces of the orbitals.

Our analyses reveal that the pnictogen bonding mechanism is not purely electrostatic but, instead, has a relatively large covalent component (ΔE_oi), stemming mainly from the HOMO–LUMO interaction between the occupied halide np_y atomic orbital (AO) and the σ* D–Pn antibonding 5a′ acceptor orbital (see Fig. 2). For the pnictogen-bonded complexes, the orbital-interaction term ranges from 34% for F₃Sb⋯F⁻ to as much as 65% for Br₃N⋯Cl⁻ of the total bonding interactions (ΔE_oi + ΔV_elstat; see Table 3), and the orbital interaction curves ΔE_oi(ζ) become more stabilizing from Pn = N to Sb (Fig. 1, right). The stronger orbital interaction for the heavier pnictogens is the result of the larger LUMO–HOMO overlap (i.e. 〈5a′|np_y〉; see Fig. 2 for the MO diagram that depicts the np_y orbital of A⁻ oriented towards the D¹–Pn bond of the D₃Pn fragment) as Pn becomes more electropositive. For example, in the Cl₃Pn⋯Cl⁻ series, 〈5a′|np_y〉 increases from 0.11 to 0.20 to 0.22 along Pn = N, P, and Sb in the equilibrium geometry (see Table 3). 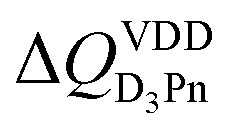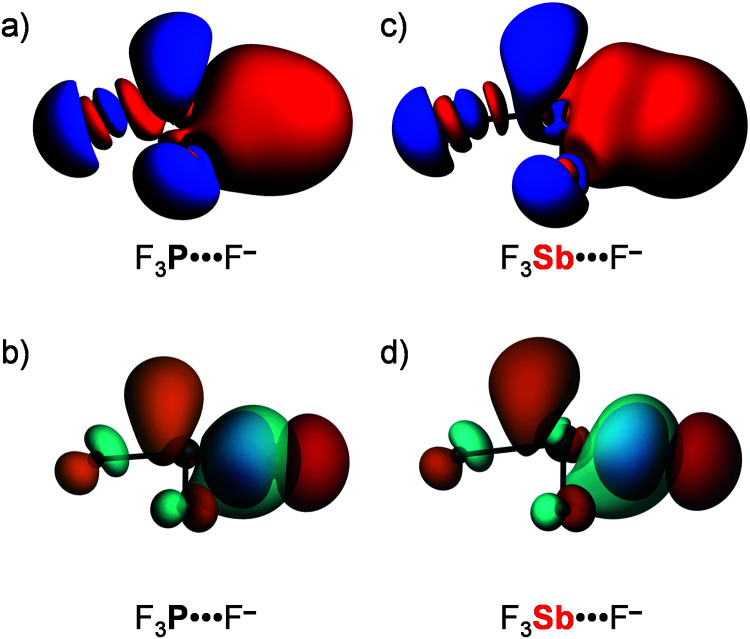The associated charge transfer from A⁻ to D₃Pn is reflected by the VDD charge of the D₃Pn fragment in the complex, , which is negative (see Table 1). Thus, D₃Pn gains charge from A⁻ upon complexation, for all D₃Pn⋯A⁻ complexes. For example, 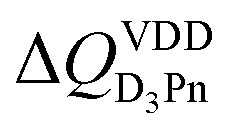is −0.30 a.u. for F₃N⋯F⁻ and −0.31 a.u. for F₃Sb⋯F⁻. The HOMO–LUMO charge transfer nature of the pnictogen bond is also reflected by the 3D plots of the deformation densities associated with pnictogen-bond formation in F₃P⋯F⁻ and F₃Sb⋯F⁻ (see Fig. 3). Note the charge depletion in the region of the HOMO on the Lewis base F⁻ (and within the Pn⋯F⁻ bond due to Pauli repulsion^10a) and the charge accumulation in the region of the LUMO on D₃Pn.

Fig. 3. Deformation density (Δρ(r) = ρ_{[D3Pn⋯A⁻]}(r) − ρ_D3Pn(r) − ρ_A⁻(r); red = depletion; blue = accumulation) plot (a and c) and HOMO–LUMO interaction (b and d) for a representative series of D₃Pn⋯A⁻ pnictogen bonds.

The ΔE_oi(ζ) curves become more stabilizing if one goes from nitrogen to the heavier pnictogen bonds, but, interestingly, the orbital interaction ΔE_oi(ζ_eq) at the stationary point of the complex turns out to be comparable in magnitude for all pnictogens (see Table 3). The reason is the significantly more pronounced stretch in the F¹–Pn bond for Pn = N than for the heavier pnictogens. This phenomenon causes ΔE_oi(ζ_eq) for nitrogen to occur at a later point at which the intrinsically less stabilizing ΔE_oi(ζ) curve has achieved a more stabilizing value that, as mentioned above, is comparable to the value of the other pnictigen bonds that do not feature this strong F¹–Pn bond stretch.

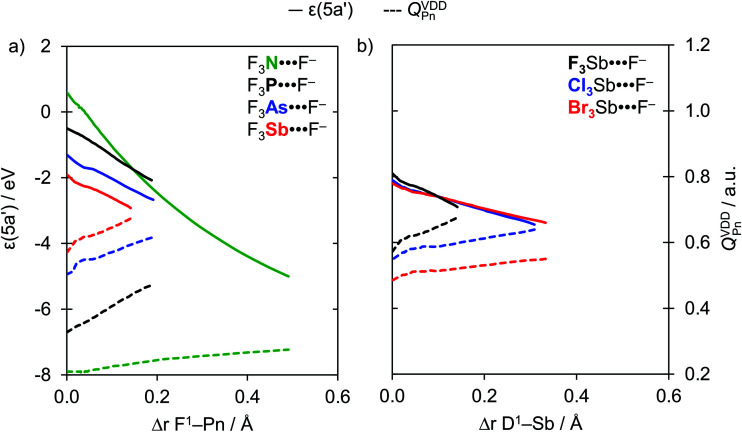
The reason that F¹–Pn streches more for Pn = N than for the heavier pnictogens is its lower polarity and thus weaker bond strength (see Table 2) which translates into less strain when it streches upon complexation with the Lewis base (see Fig. 1). Thus, as the F¹–Pn bond in the F₃Pn⋯F⁻ complexes expands the most, the σ* D–Pn antibonding 5a′ acceptor orbital drops significantly in energy and, due to a smaller HOMO–LUMO gap, enters into a more stabilizing donor–acceptor orbital interaction ΔE_oi(ζ_eq) (see Fig. 2b). This effect can be observed in Fig. 4a, which shows the energies of the σ* F–Pn antibonding 5a′ acceptor orbitals, as well as the VDD atomic charge on Pn in the F₃Pn fragments, along the reaction coordinate. The F¹–Pn bond in the F₃Pn⋯F⁻ complexes expands less from Pn = N to Sb and leads to a smaller stabilization of the σ* D–Pn antibonding 5a′ acceptor orbital and the decrease in the HOMO–LUMO gap becomes smaller from Pn = N to Sb, resulting in less stabilization by orbital interactions. For example, for Pn = Sb, the energy of the σ* F–Sb antibonding 5a′ acceptor orbital is up to −3.0 eV in F₃Sb at the equilibrium geometry of the F₃Sb⋯F⁻ complex. Due to the weaker F–N bond compared to F–Sb (e.g. BDE_F–N = 59.4 kcal mol⁻¹ and BDE_F–Sb = 117.9 kcal mol⁻¹; see Table 2), the F¹–N bond expands to a higher extent and the σ* F–N antibonding 5a′ acceptor orbital quickly drops to a value of −5.1 eV.

Fig. 4. Energy of the 5a′ orbital (in eV) and the VDD charge on the Pn atom (in a.u.) in the neutral fragment D₃Pn projected onto (a) the F¹–Pn bond stretch (green, Pn = N; black, Pn = P; blue, Pn = As; red, Pn = Sb) and (b) the D¹–Sb bond stretch (black, D = F; blue, D = Cl; red, D = Br).

Nevertheless, the nitrogen-bonded complexes remain the weakest as the high stabilization of the σ* F–N antibonding 5a′ acceptor orbital is counteracted by its poor orbital overlap with the np_y donor orbital due to the absence of radial nodes in and, thus, the very compact nature of the nitrogen 2p valence AOs (see Table 3).

In addition, note that, as the F¹–Pn bond in the F₃Pn⋯F⁻ complexes expands, the pnictogen atom in the D₃Pn fragment becomes more positive (Fig. 4a), resulting in a more stabilizing electrostatic ΔV_elstat(ζ_eq) (see Table 3). However, the significant expansion of the F¹–N bond is not enough to make the highly electronegative N atom as positive as the heavier pnictogens, making the nitrogen-bonded complexes also the least stabilized by electrostatic attraction.

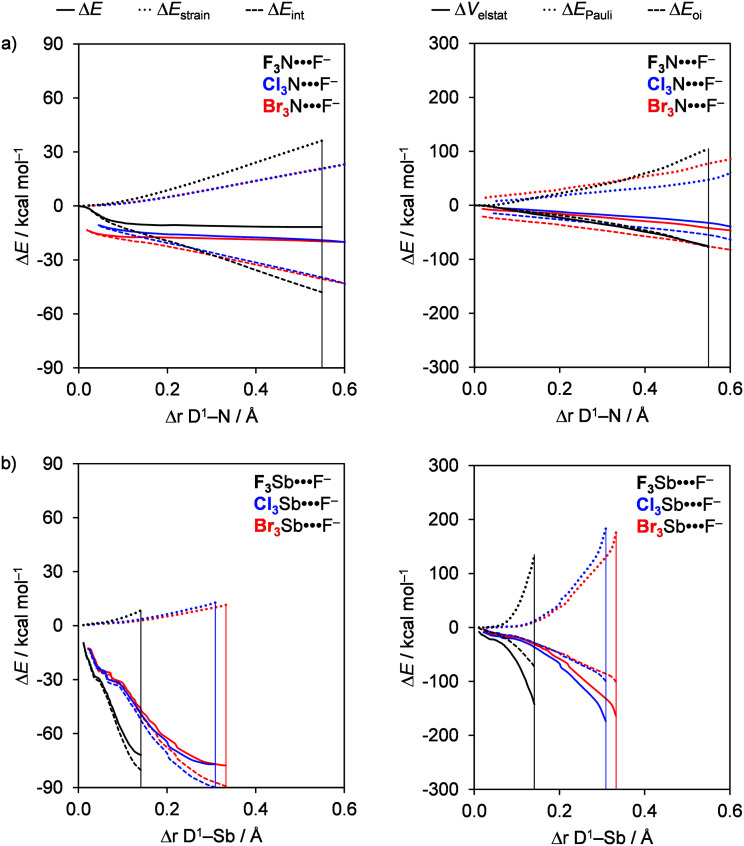
Bond analyses with the variation of A⁻

Our analyses show that the pnictogen bonds D₃Pn⋯A⁻ become weaker as the Lewis basicity of the A⁻ halide decreases from F⁻ to Br⁻.¹⁷ Again, this is equivalent to what was found for chalcogen bonds D₂Ch⋯A⁻, halogen bonds DX⋯A⁻, and hydrogen bonds DH⋯A⁻.⁸ As aforementioned, pnictogen bonds have both an electrostatic component (ΔV_elstat) and a covalent component (ΔE_oi) stemming mainly from the HOMO–LUMO interaction between the occupied halide np atomic orbital (AO) and the σ* D–Pn antibonding 5a′ acceptor orbital, shown schematically in Fig. 2. The electron-donating capacity of the halides is reduced as the halide np AOs become more diffuse and lower in energy from A⁻ = F⁻ to Br⁻, thus weakening ΔE_oi.^8,17b This will also result in longer bonds and weaker electrostatic attraction. As a result, the interaction energy (ΔE_int) and, thus, the net pnictogen-bond strength ΔE become less stabilizing along A⁻ = F⁻ to Br⁻ (see Table 1 and Table S1 in the ESI†).

Activation strain analyses reveal that the trend A⁻ = F⁻ to Br⁻ in the total energy ΔE(ζ) is directly determined by the trend in the corresponding interaction energies, that is, ΔE_int(ζ) weakens from A⁻ = F⁻ to Br⁻. This is nicely seen in the left diagrams in Fig. 5, which shows the formation of the pnictogen bonds D₃Pn⋯A⁻, with A⁻ = F⁻, Cl⁻, and Br⁻, for a representative series of F₃N and F₃Sb molecules. Note that the ΔE_strain curves coincide (because they stem from the same molecule with the same F–N bond being stretched as the complexation reaction progresses) and, thus, do not affect the trends in ΔE(ζ). For the nitrogen bonds involving the Lewis bases A⁻ = Cl⁻ and Br⁻, the bond strength is particularly weak because the interaction is too weak to significantly stretch the D¹–N bonds. Consequently, the D₃N fragment achieves a much weaker electron-accepting capacity in this less distorted equilibrium geometry and so the eventual interaction and bond energy become relatively weak (see Fig. 5a).

Fig. 5. Activation strain (left panel) and energy decomposition (right panel) analyses of (a) F₃N⋯A⁻ and (b) F₃Sb⋯A⁻ (black, A⁻ = F⁻; blue, A⁻ = Cl⁻; red, A⁻ = Br⁻). The vertical lines indicate the position of the stationary points.

The trend in ΔE_int(ζ) is again dictated by the bonding components ΔV_elstat and ΔE_oi. This can be seen in the right panel of Fig. 5, which shows that both ΔV_elstat(ζ) and ΔE_oi(ζ) become more stabilizing from A⁻ = F⁻ to Br⁻. The key to understand these trends is of course related to the factors that enhance the strength of the bonding components and, thus, ΔE_int: firstly, an approaching halide A⁻ with a higher lying HOMO and, secondly, a weak D–Pn bond that is easily stretched resulting in a σ* D–Pn antibonding 5a′ acceptor orbital that quickly drops in energy as the D¹–Pn bond elongates (see Fig. 4). These factors are the driving force for D¹–Pn stretching in D₃Pn⋯A⁻ since they generate stronger orbital interactions and, therefore, stronger pnictogen bonds. For F₃N⋯A⁻, the 5a′ acceptor orbital energy in the equilibrium geometry of the complex adopts significantly lower values for weaker Lewis bases (A⁻ = Cl⁻ or Br⁻) than for A⁻ = F⁻ (i.e., −5.1 eV for A⁻ = F⁻, −0.2 eV for A⁻ = Cl⁻, and −0.1 eV for A⁻ = Br⁻; see Table 3). Indeed, D¹–Pn stretching is most pronounced if this bond in the neutral fragment is weaker (e.g., ca. 59 kcal mol⁻¹ for F–N, ca. 35 kcal mol⁻¹ for Cl–N, and ca. 30 kcal mol⁻¹ for Br–N; see Table 2). For example, the D¹–N stretching in the nitrogen-bonded complexes is longer in Br₃N⋯F⁻, for which Δr_D¹–Pn is 1.4 Å, and less pronounced in F₃N⋯F⁻, for which Δr_D¹–Pn is 0.5 Å (see Table 1). As a result, the bonding components, ΔV_elstat and ΔE_oi, become significantly stronger, up to −300 kcal mol⁻¹, in the Cl₃N⋯A⁻ and the Br₃N⋯A⁻ series. However, the bonding components are significantly weakened to ca. −60 kcal mol⁻¹ for weaker Lewis bases, in which case Δr_D¹–Pn varies between only 0.04 and 0.6 Å (see Table 1).

Bond analyses with the variation of D

The strength of the heavier D₃Pn⋯A⁻ pnictogen bonds (Pn = P, As, Sb) slightly strengthens when the substituent D varies from F to Br. Based on the purely electrostatic picture of the σ-hole model, one might expect just the opposite, that is, a weakening of the pnictogen bond in D₃Pn⋯A⁻ as D varies from F to Br due to a decrease in the positive molecular electrostatic potential of the σ-hole at the Pn atom in the D₃Pn fragment (V_S,max).^6a,18 This apparent discrepancy from the σ-hole model is traced to the trend that D–Pn bonds become weaker along F–Pn, Cl–Pn and Br–Pn (see Table 2). And, the weaker the D–Pn bonds, the more they elongate in the eventual equilibrium geometry of the corresponding D₃Pn⋯A⁻ complex. Therefore, the latter is reached at a later stage along the reaction coordinate Δr_D¹–Pn. A consequence of this D–Pn bond elongation is a more electropositive Pn atom and a lower energy of the σ* D–Pn antibonding 5a′ acceptor orbital due to a reduction in antibonding character (see Fig. 4b). This situation translates into more stabilizing ΔV_elstat(ζ) and ΔE_oi(ζ) curves as D varies from F to Cl to Br (see Fig. 6, right). For the D₃N⋯A⁻ nitrogen bonds, these effects are most pronounced because the D–N bonds in D₃N are the weakest halogen–pnictogen bonds. Thus, stronger D₃N⋯A⁻ complexes with a more pronounced D–N stretch occur if we go from F₃N to Cl₃N and Br₃N.

Fig. 6. Activation strain (left panel) and energy decomposition (right panel) analyses of (a) D₃N⋯F⁻ and (b) D₃Sb⋯F⁻ (black, D = F; blue, D = Cl; red, D = Br). The vertical lines indicate the position of the stationary points.

In the following, we exemplify the above with a few concrete examples. For the heavier pnictogen bonds (Pn = P, As, Sb), we find that the trend in bond energy curves ΔE(ζ) is set by the interaction energy curves ΔE_int(ζ), that is, they become less stabilizing along D = F, Cl, and Br. However, as the D¹–Pn bond expands more for D = Cl and Br compared to D = F, both ΔE(ζ_eq) and ΔE_int(ζ_eq) in the equilibrium geometry are slightly more stabilizing for D = Cl and Br. This trend in the interaction energy curves ΔE_int(ζ) is a direct consequence of the electrostatic attraction ΔV_elstat and orbital interactions ΔE_oi. The curves for the bonding components ΔV_elstat(ζ) and ΔE_oi(ζ) are the most stabilizing for D = F because of the larger difference in electronegativity across the D–Pn bonds (vide supra). Nevertheless, the Cl–Pn and Br–Pn bonds are substantially weaker than the associated F–Pn bond (e.g. BDE_F–Sb = 117.9 kcal mol⁻¹, BDE_Cl–Sb = 82.8 kcal mol⁻¹, and BDE_Br–Sb = 66.2 kcal mol⁻¹; see Table 2), and the Cl–Pn and Br–Pn bonds expand to a greater degree. Consequently, ΔE_oi(ζ) becomes more stabilizing for D = Cl and Br because the σ* D–Pn antibonding 5a′ acceptor orbital is strongly stabilized and can engage in stronger donor–acceptor interactions with the np-type lone pair HOMO on A⁻ (see Fig. 4b). In parallel, the VDD atomic charge on Pn becomes increasingly more positive as the D¹–Pn bond expands (see Fig. 4b), which translates into a more stabilizing ΔV_elstat(ζ) for D = Cl and Br. This results in the slight strengthening of ΔV_elstat(ζ_eq) and ΔE_oi(ζ_eq), and thus ΔE_int(ζ_eq), in the equilibrium geometry along D = F, Cl, Br.

The D₃N⋯A⁻ complexes, on the other hand, show somewhat deviating behavior as compared to the heavier pnictogen bonding complexes exemplified above. Thus, for the D₃N⋯A⁻ complexes, ΔE(ζ) becomes significantly more stabilizing along D = F, Cl, and Br instead of remaining relatively constant. This is because the D¹–N bonds are all much weaker (e.g. BDE_F–N = 59.4 kcal mol⁻¹, BDE_Cl–N = 35.6 kcal mol⁻¹, and BDE_Br–N = 30.2 kcal mol⁻¹; see Table 2), and the stretch Δr_D¹–Pn for all nitrogen-bonded complexes is much more pronounced, that is, the complexes occur later in the reaction coordinate. For example, the D¹–N stretch has variation between 0.5 and 1.4 Å in D₃N⋯F⁻, whereas the D¹–Sb stretch varies only between 0.1 and 0.3 Å in D₃Sb⋯F⁻ from D = F to Br (see Table 1). As a result, the D₃N⋯A⁻ complexes show more significant strengthening of ΔV_elstat(ζ_eq) and ΔE_oi(ζ_eq) along D = F to Br in the equilibrium geometries, and, therefore, a significant increase in stability along the same series.

Comparison of pnictogen-, chalcogen-, halogen-, and hydrogen bonds

Our analyses highlight that pnictogen bonds share strong similarities with the corresponding chalcogen bonds (ChB), halogen bonds (XB), and hydrogen bonds (HB).⁸ We find that these bonds have considerable covalency on top of electrostatic attraction and can range in strength roughly between −3 and −78 kcal mol⁻¹ (see Fig. 7). The contribution of the covalent component ΔE_oi to the total bonding components (ΔV_elstat + ΔE_oi) is up to 97%, 76%, and 65% for XB, ChB, and PnB, respectively, whereas it is up to 66% for HB.^8a,b The same bonding mechanism with a substantial covalent component is also observed for the archetypal DM⋯A⁻ alkali- and coinage-metal bonds (MB) that have even more pronounced polarization in the D–M bonds.^8c

Fig. 7. Generic molecular orbital diagrams for (a) D₃Pn⋯A⁻ pnictogen bonds, (b) D₂Ch⋯A⁻ chalcogen bonds, (c) DX⋯A⁻ halogen bonds, and (d) DH⋯A⁻ hydrogen bonds.

Our findings consolidate earlier work and support the charge-transfer character of pnictogen bonds by providing a causal bonding mechanism.^2b,7 PnB, ChB, and XB are generally stronger than HB due to more stabilizing orbital interactions (see Table S3 for bond energies ΔE of a representative series of ChB, XB, and HB). On the other hand, hydrogen bonds have less destabilizing Pauli repulsion because there is no overlap between the np_D HOMO of the D–H fragment and the higher-lying occupied np AOs of A⁻ (see Fig. 7). Our analyses also show that PnB, ChB, and XB, but also MB, have even stronger electrostatic attraction than HB.^8a,b Note that this cannot be straightforwardly explained by the σ-hole model, which, based on hydrogen having the highest V_S,max, erroneously suggests that HB should have the stronger electrostatic attraction.⁶

Conclusions

The pnictogen bonds in D₃Pn⋯A⁻ range between 3 and 78 kcal mol⁻¹ in strength, becoming stronger as the pnictogen atom becomes more electropositive, along Pn = N, P, As and Sb, and also as the halide becomes a stronger Lewis base, along A⁻ = Br⁻, Cl⁻ and F⁻. The trend upon variation of the substituent along D = F, Cl, Br is less pronounced, as are all trends for the relatively weak nitrogen bonds. This follows from our bonding analyses based on relativistic density functional theory.

Our activation-strain and quantitative Kohn–Sham MO bonding analyses reveal that the pnictogen bonds in D₃Pn⋯A⁻ have a considerable covalent component ΔE_oi, ranging from 34% to 65% of the bonding components (ΔV_elstat + ΔE_oi), stemming from HOMO–LUMO interactions between the np-type lone pair HOMO on A⁻ and the σ* D–Pn antibonding LUMO on D₃Pn. The D₃Pn⋯A⁻ pnictogen bond becomes stronger as Pn descends in the periodic table along N, P, As and Sb. One reason is the increasing polarization towards Pn of the σ* LUMO and the associated increase in the LUMO–HOMO overlap with A⁻ (along P, As, and Sb this trend is reinforced by the drop in the σ* LUMO energy). Another reason is the higher positive charge on Pn which goes with more stabilizing electrostatic interactions with the Lewis base.

Finally, it appears that the pnictogen bonds in D₃Pn⋯A⁻ are similar in nature to the chalcogen bonds in D₂Ch⋯A⁻, halogen bonds in DX⋯A⁻, and hydrogen bonds in DH⋯A⁻ (Pn = N, P, As, Sb; Ch = O, S, Se, Te; D, X, A = F, Cl, Br). Our work constitutes a unified picture of all these interactions, which appear to be far from solely electrostatic phenomena. We conclude that the often-used designation of “noncovalent interactions” for these types of bonds is not consistent with their significant covalent nature. Instead of this term, we propose to refer to such bonds as (weak or strong) intermolecular interactions.

Conflicts of interest

There are no conflicts to declare.