Abstract
K0.5Na0.5NbO3 is considered as one of the most promising lead-free piezoelectric ceramics in the field of wearable electronics because of its excellent piezoelectric properties and environmental friendliness. In this work, the temperature-dependent longitudinal piezoelectric coefficient was investigated in K0.5Na0.5NbO3 single crystals via the Landau–Ginzburg–Devonshire theory. Results show that the piezoelectric anisotropy varies with the temperature and the maximum of deviates from the polar direction of the ferroelectric phase. In the tetragonal phase, parallels with cubic polarization direction near the tetragonal-cubic transition region, and then gradually switches toward the nonpolar direction with decreasing temperatures. The maximum of in the orthorhombic phase reveals a distinct varying trend in different crystal planes. As for the rhombohedral phase, slight fluctuation of the maximum of was observed and delivered a more stable temperature-dependent maximum and its corresponding angle θmax in comparison with tetragonal and orthorhombic phases. This work not only sheds some light on the temperature-dependent phase transitions, but also paves the way for the optimization of piezoelectric properties in piezoelectric materials and devices.
Keywords: piezoelectric, anisotropy, K0.5Na0.5NbO3, phase, temperature
1. Introduction
With the gradual deepening and prosperity of the smart wearable industry revolution, piezoelectric-based flexible electronics have attracted considerable attention because of their promising applications in robotics [1], human–machine interaction (HMI) [2], energy harvesters [3], and internet of things (IOT) [4]. Lead-based perovskites, such as Pb(ZrxTi1−x)O3 (PZT) [5] and Pb(Mg,Nb)O3 (PMN) [6] ceramics, possess huge piezoelectric properties but cause severe environmental and health concerns owing to their toxicity. As a promising alternative to lead zirconate titanate (PZT), the K0.5Na0.5NbO3, a lead-free ferroelectric material, has exhibited outstanding piezoelectric performance near the polymorphic phase boundary (PPB) [7] and attracted massive attention worldwide in recent years due to its environmental friendliness [8,9,10,11,12,13]. Owing to its unique merits of a high piezoelectric coefficient (d33), excellent ferroelectric properties, and a high Curie temperature (Tc = 420 °C), KNN has been widely utilized in energy-harvesting devices, transducers, actuators, and sensors [14,15,16,17,18,19,20,21]. Although pristine KNN ceramics possess relatively low piezoelectricity (d33~80 pC/N), they can be remarkably improved by tuning sintering conditions [22], domain engineering [23], phase boundary engineering [24,25], texturing [26], and so on. Furthermore, since the intrinsic piezoelectric response is intimately associated with spontaneous polarization rotation, the anisotropy of piezoelectric capability plays a crucial role in the application of piezoelectric materials [27]. Anisotropy of piezoelectric properties had attracted massive attention in the 1980s, and unprecedented large piezoelectric anisotropy was observed in lead titanate ceramics with random grain orientations [28,29]. However, limited works have focused on the piezoelectric anisotropy of K0.5Na0.5NbO3 single crystals.
The main purpose of this work is to study the piezoelectric anisotropy of K0.5Na0.5NbO3 single crystals as a function of temperature and to unravel the impact of phase transitions on the orientation and amplitude of the longitudinal piezoelectric coefficient. Landau–Ginzburg–Devonshire (LGD) theory was utilized to calculate the three-dimensional surface of the longitudinal piezoelectric coefficient for KNN single crystals in three ferroelectric phases as a function of temperature. Temperature-dependent free energy and spontaneous polarization of KNN has also been investigated to interpret the derivation of piezoelectric coefficients stemmed from temperature variation.
2. Materials and Methods
The ferroelectric capabilities of KNN single crystals were systematically investigated through the Landau–Ginzburg–Devonshire (LGD) function. To achieve more convenience in expressing piezoelectric coefficients in light of the coordinate system for each ferroelectric phase, the cubic paraelectric phase was selected as the reference. The thermodynamic potential function G of the KNN single crystal can be written as [30,31]:
| (1) |
where the Landau energy density is given by
| (2) |
Here, α denotes the Landau coefficients determined under the stress-free condition [28], σi denotes the ith component of stress in Voigt notation, s11, s12, and s44 denote the elastic compliance constants of a cubic phase [29,30,31], and Q11, Q12, and Q44 denote the corresponding electrostrictive coefficients between polarization and stress [32,33].
In this work, , , and denote the polarization, dielectric susceptibility, and piezoelectric coefficient in the KNN single crystal for each phase, respectively. For studying the orientational dependence of piezoelectric coefficients, Euler angle is utilized to quantitatively describe the rotation in terms of ferroelectric phase coordinates.
The Landau coefficients, elastic compliance constants, and electrostrictive coefficients are taken from Jianjun’s previous work [34]. The dielectric constant possesses a positive proportional relationship with relative dielectric stiffness (). K0.5Na0.5NbO3 endures a series of phase transition (cubic→tetragonal→orthorhombic→rhombohedral) in the process of cooling from the paraelectric phase. The following relations were utilized to calculate the temperature-dependence of piezoelectric coefficients and dielectric susceptibility coefficients of KNN crystals as a function of spontaneous polarization,
| (3) |
| (4) |
| (5) |
| (6) |
where Aji and Δ refer to the cofactor and determinant of the χij matrix.
3. Results and Discussion
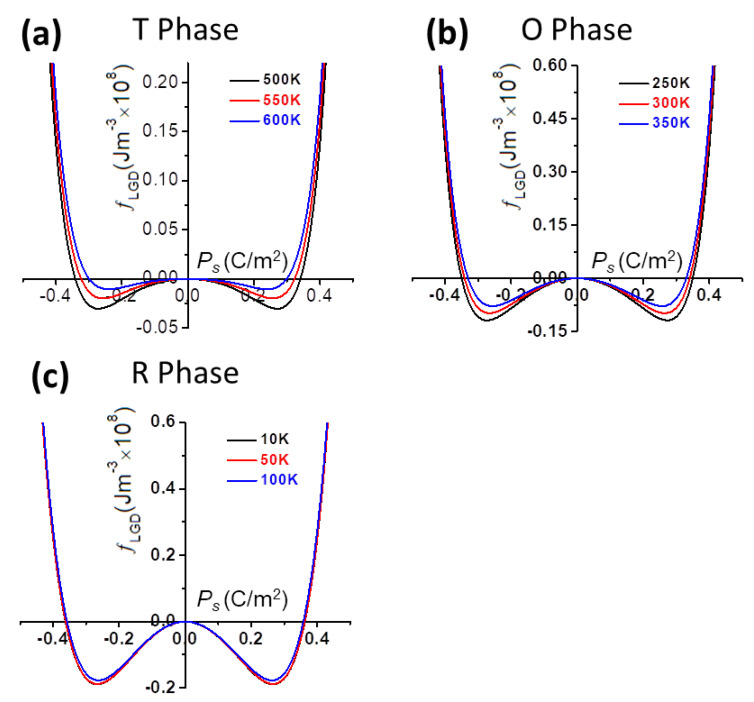
The LGD-free energy density of the tetragonal phase, orthorhombic phase, and rhombohedral phases, respectively, is plotted as a function of polarization at various temperatures, as shown in Figure 1a–c.
Figure 1.
The Calculated LGD-free energy as a function of polarization (Ps) in (a) tetragonal phase, (b) orthorhombic phase, (c) rhombohedral phase at various temperatures.
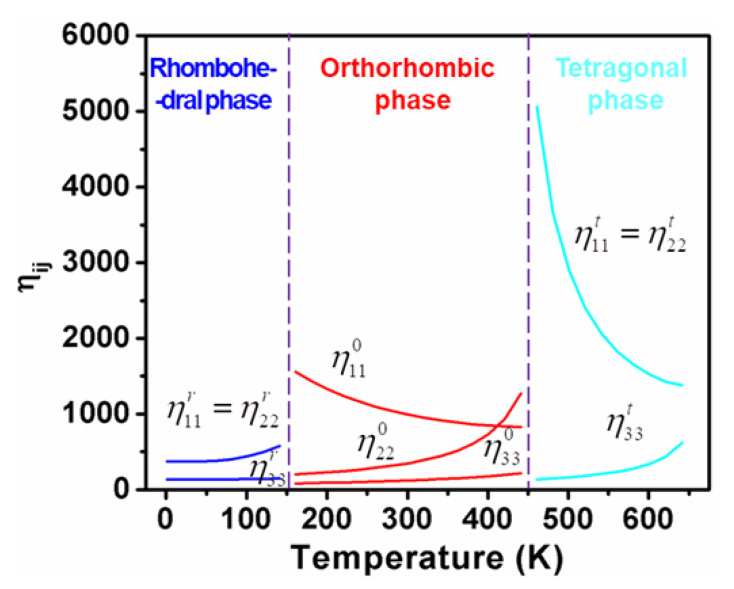
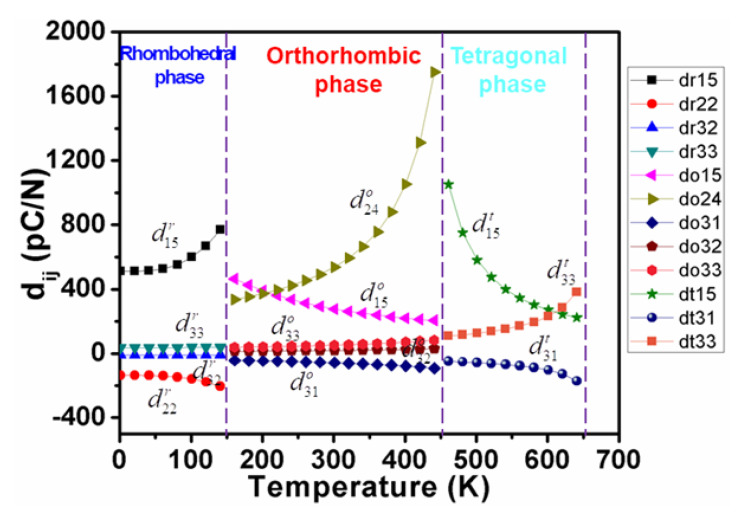
Figure 2 and Figure 3, respectively, elucidate the calculated dielectric susceptibility coefficients and piezoelectric coefficients for K0.5Na0.5NbO3 single crystals as a function of temperature in all three ferroelectric phases, respectively.
Figure 2.
Calculated dielectric susceptibility coefficients for K0.5Na0.5NbO3 single crystals as functions of temperature in all three ferroelectric phases.
Figure 3.
Calculated piezoelectric coefficients for K0.5Na0.5NbO3 single crystals as functions of temperature in all three ferroelectric phases.
According to the Landau–Ginsburg–Devonshire theory, the polarization, i.e., the second derivative of thermodynamic potential function G, can be acquired as a function of temperature by minimizing the total free energy in terms of polarization. Figure 4 illustrates the temperature-dependent spontaneous polarization of K0.5Na0.5NbO3 single crystals in the three phases. It can be clearly seen that polarization goes up with the cooling process for all the ferroelectric phases. Sudden rises were observed at the regions of phase transitions, where 648 K for cubic to tetragonal, 469 K for tetragonal to orthorhombic, and 130 K for orthorhombic to rhombohedral, which is consistent with the experimental results of 694 K, 468 K and 125 K, respectively, from Egerton et al. [35]. It is worth noting that the calculated polarization becomes zero when the temperature is approaching Curie temperature, implying that the system switches to paraelectric phase. Figure 5 displays the free energy (ΔG) of K0.5Na0.5NbO3 single crystals in the three phases as a function of temperatures.
Figure 4.
Spontaneous polarization of K0.5Na0.5NbO3 single crystals as a function of temperatures.
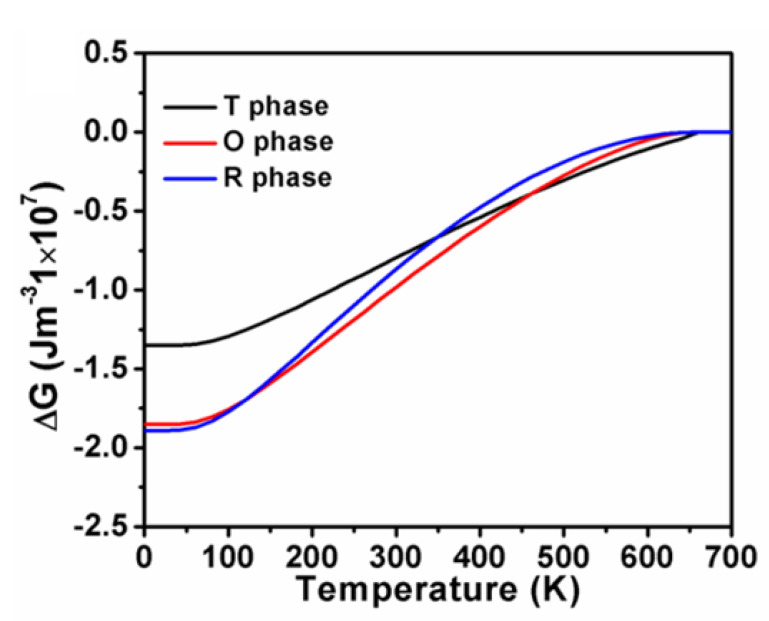
Figure 5.
Free energy (ΔG) of K0.5Na0.5NbO3 single crystals in the three phases as a function of temperatures.
The piezoelectric properties are proportional to the flattening of the free energy profile. It can be clearly seen that the tetragonal-cubic phase transition causing an enhanced (Figure 3) can also be explained by the flattening of the free energy profile (Figure 1a). Consequently, the delayering of the free energy profile favors the enhancement of dielectric susceptibility. It is obvious that in the orthorhombic phase, the increasing temperature flattens the LGD-free energy well and makes it shallower with the heating-up process (Figure 1b), giving rise to the increase in dielectric susceptibility, and thus the increase in its piezoelectric response (Figure 1b). As for the temperature-dependent free energy for the rhombohedral phase in Figure 1c, the delayering of the free energy arising from the temperature rising also contributes to the enhancement of piezoelectric coefficients lying along a no-polar direction (Figure 3).
In the tetragonal phase, the value of for K0.5Na0.5NbO3 crystals in the rotated coordinate along an arbitrary direction can be expressed as:
| (7) |
where angle θ denotes rotation always from [100]t. In the tetragonal phase, (P1 = P2 = 0, ).
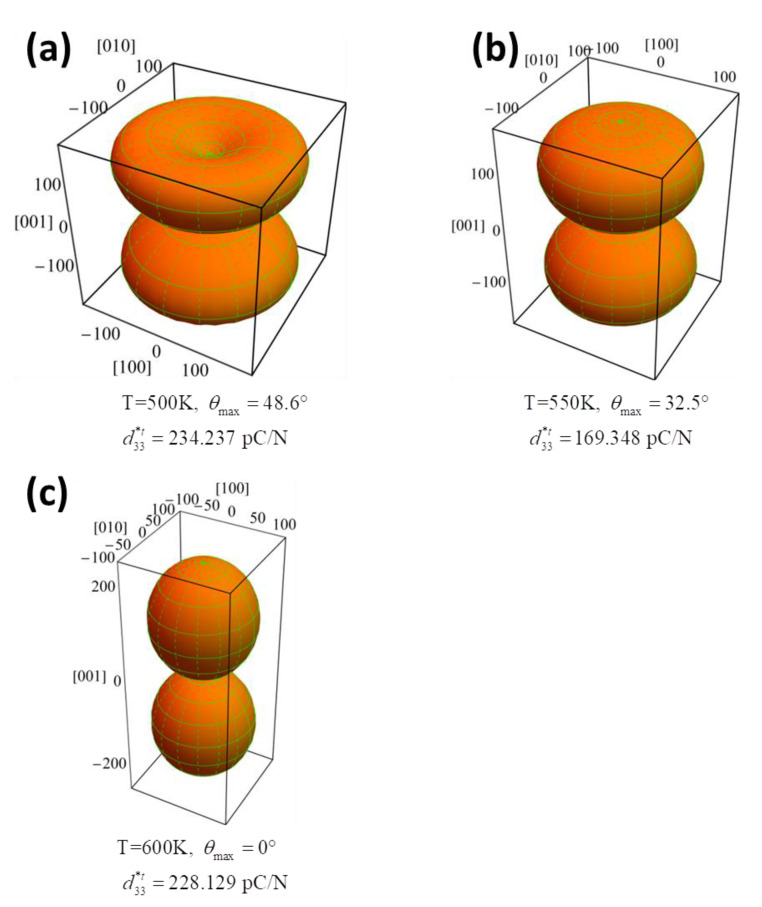
Therefore, by using Equation (7), the three-dimensional profile of calculated of the tetragonal phase for three selected temperatures 500, 550, and 600 K is displayed in Figure 6a–c, respectively. As the temperature goes down from the cubic phase to the orthorhomibic phase, the surface of varies during the cooling process. The direction of the largest lies along [001]c direction at 600 K then switches to θmax = 32.5° at 550 K, and finally to θmax = 48.6° at 500 K.
Figure 6.
The orientation dependence of piezoelectric coefficient of K0.5Na0.5NbO3 single crystals in the tetragonal phases at the temperature of (a) 500 K, (b) 550 K, (c) 600 K.
Attributed to the expression of in Equation (7), it is obvious that is determined by three parameters , , and .
As shown in Figure 3, and changes rapidly near the temperature range of tetragonal-cubic and tetragonal-orthorhombic phase transitions, respectively, while little change in was observed in comparison with and . The behaves like the dielectric permittivity (,) in the cubic phase as a function of temperature and increases with the process of cooling down toward the ferroelectric phase. When the temperature rises toward to the cubic phase (Figure 3), surpasses obviously.
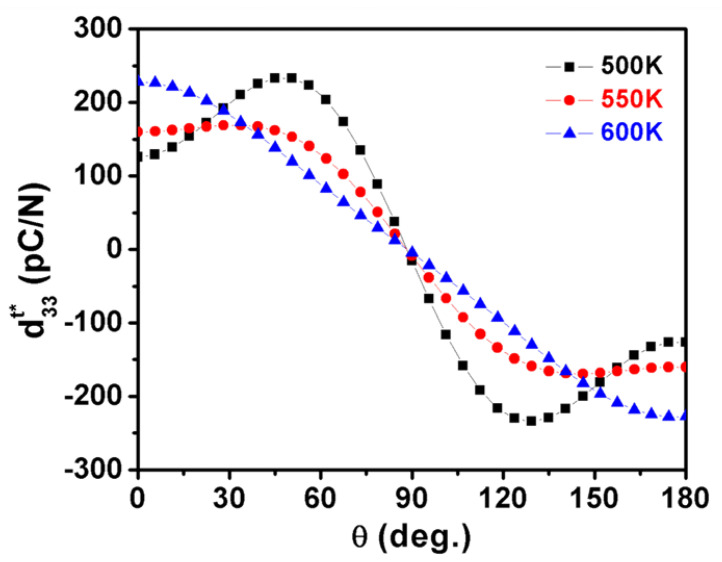
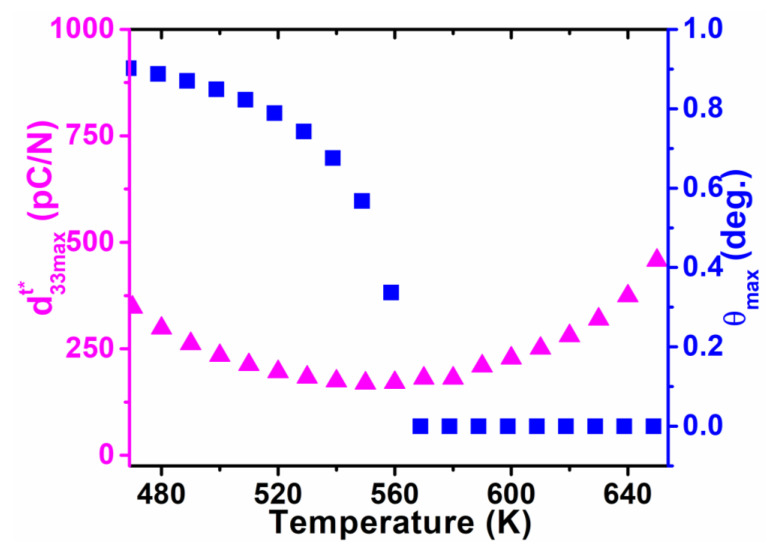
As shown in Figure 2, calculated dielectric constants and vary in opposite tendencies in the whole tetragonal phase temperature range, which gives rise to a maximum along the polar direction in the high-temperature range. As displayed in Figure 7 and Figure 8, the corresponding angle θ for the maximum value of varies as a function of temperatures, which clearly demonstrates the influence of temperature on the phase transition. It can be seen that the maximum stays along [001]t at high temperatures but deviates from [001]t to the nonpolar direction when the temperature goes down, near the orthorhombic-tetragonal point. At the temperature T = 450 K, the maximum = 521.9 pC/N lies along the direction defined by θmax = 52.98°. The maximum decreases at first, and then rises with increasing temperature, leading to a minimum at 550 K. This is because keeps increasing while keeps decreasing during the cooling process, leading to the orientation variation and amplitude change of maximum . It is reported that the pure KNN at 433 K exhibits a piezoelectric coefficient of 108 pC/N [36], which is similar to our calculated results (Figure 7) along the polar direction at 500 K. As shown in Figure 8, the angle θmax for the maximum in tetragonal K0.5Na0.5NbO3 deviates away from 0° once the temperature is below 560 K. It should be noted that the of KNbO3 (KNO) single crystals follows a similar tendency as that for the KNN single crystal because they have the same phase transition sequences and structures [37]. As for the PbTiO3 single crystal [38], the maximum lies along the polar direction at all temperatures because its shear coefficient is too small at all temperatures to rotate d33 away from the polar direction, which is quite different from the change trend for KNN.
Figure 7.
The piezoelectric coefficient in the tetragonal K0.5Na0.5NbO3 as a function of angle θ at various temperatures.
Figure 8.
Maximum of piezoelectric coefficient and its corresponding angle θmax as a function of temperature for the tetragonal K0.5Na0.5NbO3.
In the orthorhombic phase, more complex behaviors were observed in the variation of piezoelectric coefficients as a function of temperature in K0.5Na0.5NbO3 (Figure 3). Compared with ,, and that are relatively insensitive to temperature, the two different shear coefficients play a key role in piezoelectricity, where the shear piezoelectric coefficient declines with increasing temperature, while follows an opposite tendency, as shown in Figure 3. As a consequence, the piezoelectric coefficient in K0.5Na0.5NbO3 exhibits a more sophisticated temperature-dependent trend compared with the tetragonal phase:
| (8) |
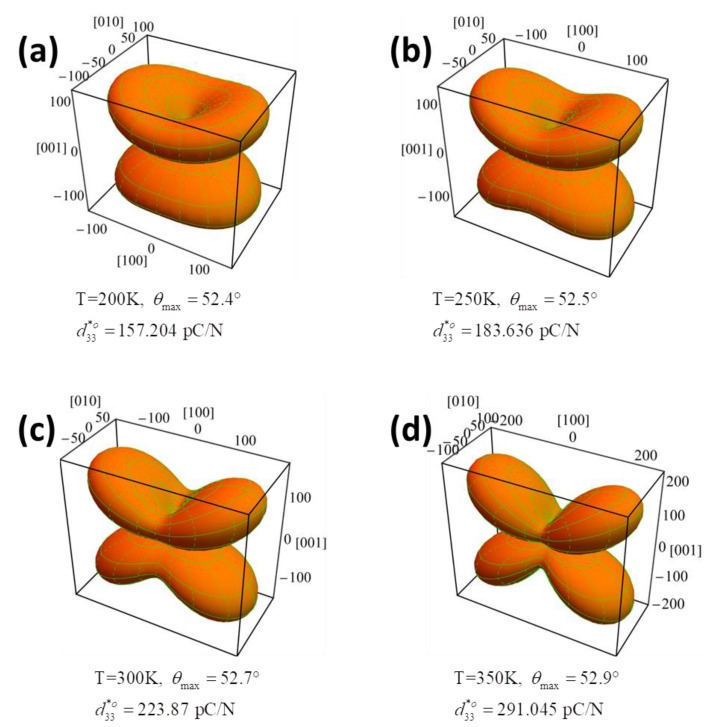
For the orthorhombic phase with P1 = 0, , the surface of the piezoelectric coefficient at four chosen temperatures of 200 K, 250 K, 300 K, and 350 K are respectively shown in Figure 9a–d. With a decreasing temperature, the direction of the maximum was slightly changed from the polar direction [001]°. The distinct changing tendencies of and are apparently responsible for the 90° rotation of the direction of the maximum in the cooling process. For instance, at 200 K, the maximum of 157.204 pC/N lies along the direction defined by θ = 52.4° and φ = π/2 (Figure 9a), but switches to the direction defined by θ = 52.9° and φ = 0 at 350 K with an amplitude of 291 pC/N (Figure 9d). Note that the reaches up to 223.87 pC/N at 300 K, which is very closed to the experimental results of 218 pC/N, indicating the accuracy and reliability of our modeling and calculation [39].
Figure 9.
The orientation dependence of piezoelectric coefficient of K0.5Na0.5NbO3 single crystals in the orthorhombic phases at the temperature of (a) 200 K, (b) 250 K, (c) 300 K, (d) 350 K.
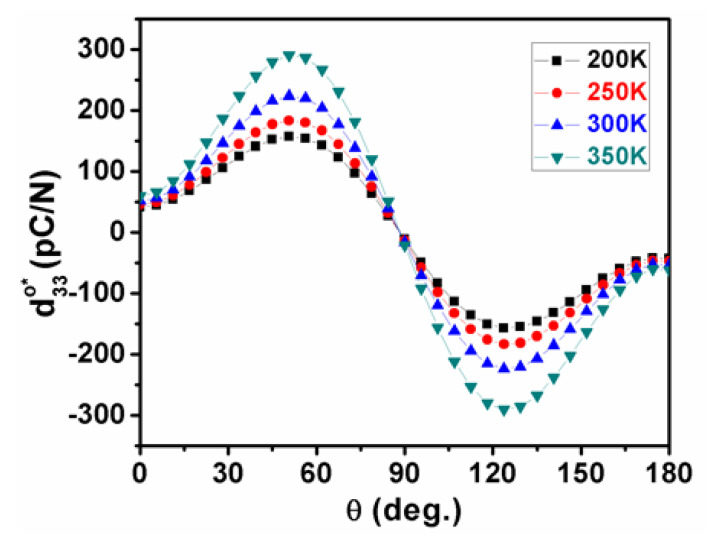
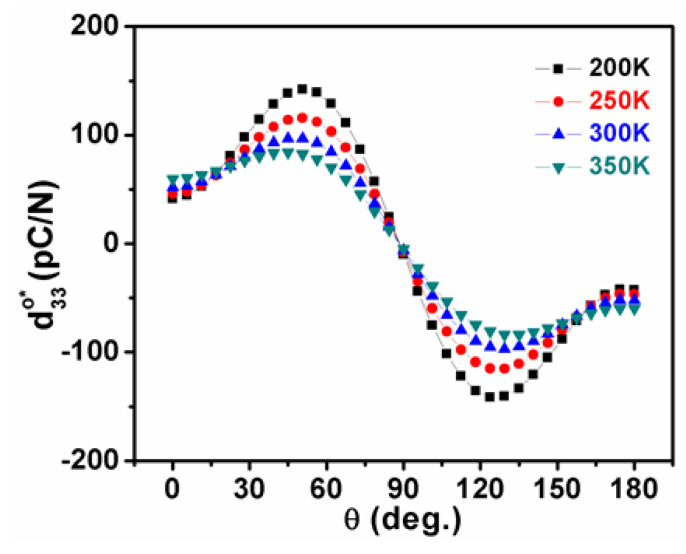
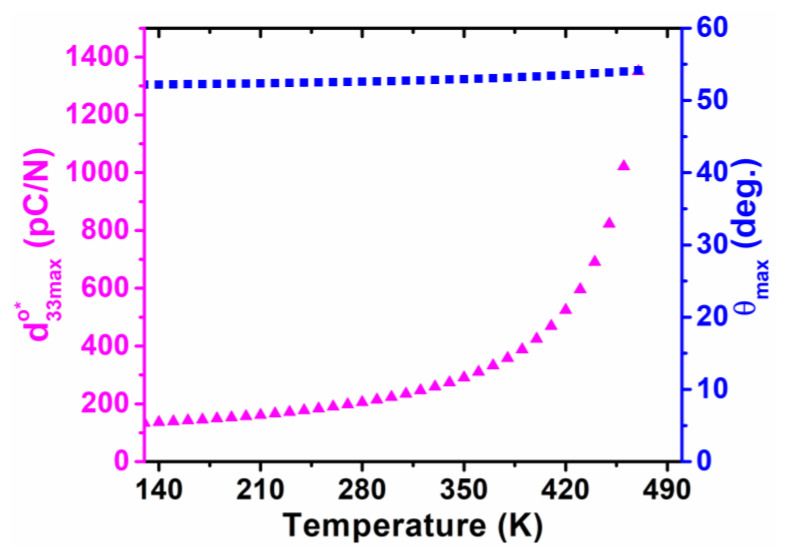
As unraveled in Figure 10, Figure 11, Figure 12 and Figure 13, the temperature-dependent maximum and its corresponding angle θmax are illustrated in planes of φ = 0 and φ = π/2 to systematically indicate the piezoelectric anisotropy of the orthorhombic K0.5Na0.5NbO3, where the direction of the maximum is rotated by 90° with an increasing temperature, and its amplitude attains the largest value in the high-temperature range when approaching the tetragonal phase.
Figure 10.
The piezoelectric coefficient in the orthorhombic K0.5Na0.5NbO3 as a function of angle θ under various temperatures in planes of φ = 0.
Figure 11.
The piezoelectric coefficient in the orthorhombic K0.5Na0.5NbO3 as a function of angle θ under various temperatures in planes of φ = π/2.
Figure 12.
Maximum of piezoelectric coefficient and its corresponding angle θmax as a function of temperature for the orthorhombic K0.5Na0.5NbO3 in planes of φ = 0.
Figure 13.
Maximum of piezoelectric coefficient and its corresponding angle θmax as a function of temperature for the orthorhombic K0.5Na0.5NbO3 in planes of φ = π/2.
According to the calculated LGD-free energy-polarization relationship as plotted in Figure 1b, the flattening of the LGD-free energy well arising from temperature growth enhances dielectric susceptibility, as well as piezoelectric response. This tendency further endows stronger effects on the piezoelectric response of KNN single crystals in the tetragonal-orthorhombic transition instead of the orthorhombic-rhombohedral transition.
As for the low-temperature rhombohedral phase, the orientation dependence of can be given by
| (9) |
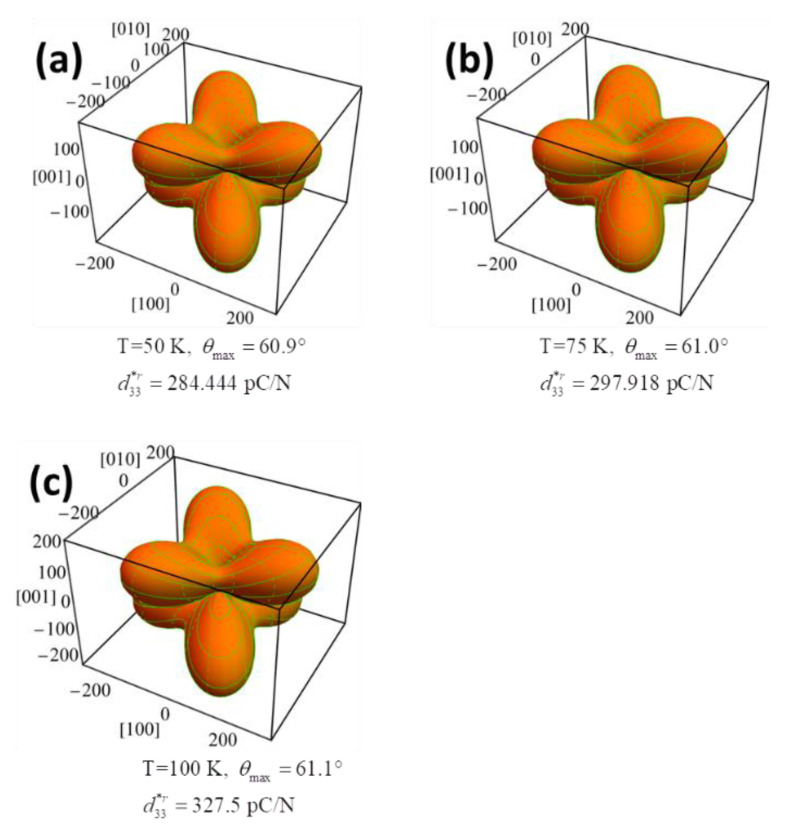
As displayed in Figure 14a–c, similar three-dimensional surfaces of were observed at temperatures of 50 K, 75 K and 100 K, implying that the temperature variation in the rhombohedral phase causes less impact on the three-dimensional surface of in comparison with those for the tetragonal and orthorhombic phase. Since no lower-symmetry phase exists as the temperature is approaching toward 0 K, the rhombohedral phase is the most stable one, and the three-dimensional surface of remains unchanged when the K0.5Na0.5NbO3 single crystal was gradually frozen during the cooling process.
Figure 14.
The orientation dependence of coefficient of K0.5Na0.5NbO3 single crystals in the rhombohedral phases at the temperature of (a) 50 K, (b) 75 K, (c) 100 K.
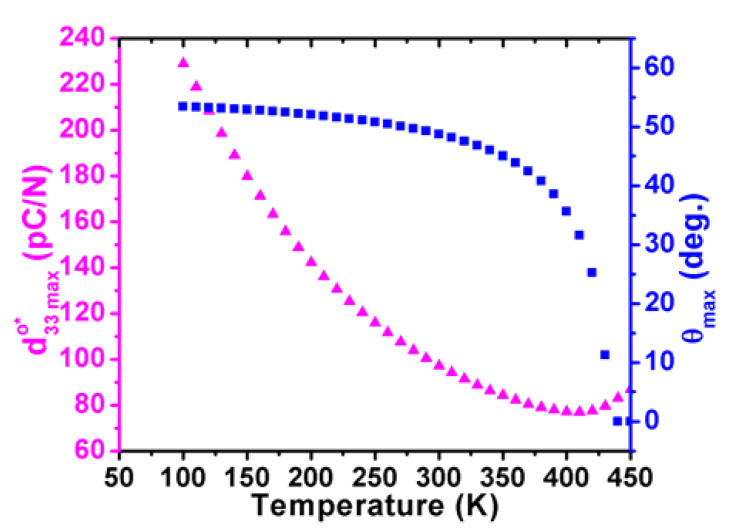
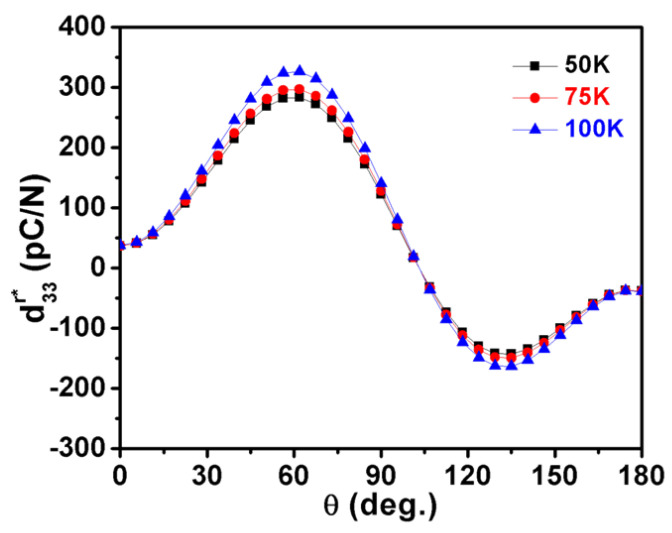
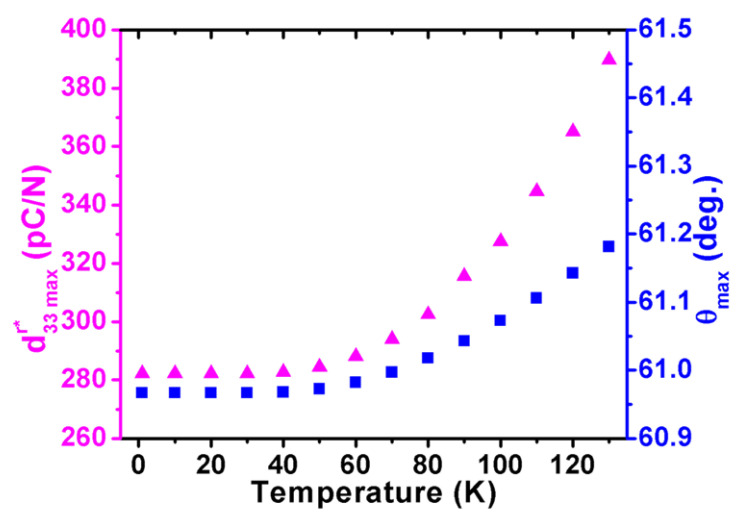
It is found that , (negative value) are proportional to the temperature, while and are almost temperature independent in the rhombohedral phase (Figure 3). As a result, the three-dimensional surface of slightly varies with varying temperatures, as shown in Figure 14a–c. At 50 K, the maximum of 284.444 pC/N lies along the direction defined by θ = 60.9° and φ = 0 (Figure 14a), while at 100 K, the maximum switches to the direction defined by θ = 61.1° and φ = 0 with an amplitude of 327.5 pC/N (Figure 14c). The dependence of the piezoelectric coefficient in KNN on the θ under various temperatures is revealed in Figure 15. The direction of the maximum value of varies with rising temperatures from 0 K to 130 K in the rhombohedral phase (Figure 16). Both maximum and its corresponding angle θmax are proportional to the temperature. The angles θ are higher than θ = 54.73°, which is consistent with the [001]c (or [111]r) direction.
Figure 15.
The piezoelectric coefficient in the rhombohedral K0.5Na0.5NbO3 as a function of angle θ at various temperatures.
Figure 16.
Maximum and its corresponding angle θmax as a function of temperature for the rhombohedral K0.5Na0.5NbO3.
According to the expression of in Equation (9), reveals an asymmetry attribute with respect to the axis defined by θ = 90°. It is obvious that the enhanced dielectric susceptibility, which is perpendicular to the polar direction, renders the large change in . The polarization rotation near the phase transition region gives rise to the increase of piezoelectric response upon the heating process, which brings about the rotation of the maximum and the slight variation in . Furthermore, with respect to the LGD-free energy profile (Figure 1c), the delayering of the free energy well with increasing temperatures indicates that the piezoelectric coefficients enhance toward a no-polar direction.
4. Conclusions
In summary, LGD thermodynamic theory was utilized to investigate the temperature-induced phase transition and evolution of three-dimensional surface in KNN single crystals. The dielectric softening along the direction perpendicular to the polarization axis is responsible for the direction change of the maximum under various temperatures. During the ferroelectric phase transition, the increase of shear piezoelectric coefficients renders a significant enhancement in along the non-polar direction. This work not only looks into the fundamental understanding of the temperature-dependent phase transitions, but also paves the way for the optimization of piezoelectric properties in ferroelectric materials.
Acknowledgments
The authors would like to thank the National Natural Science Foundation of China (Grant No. 62074027).
Author Contributions
Conceptualization, Y.S. and G.X.; methodology, Y.S.; software, Y.S.; validation, Y.S. and G.X.; formal analysis, W.L. and C.C.; investigation, W.L.; resources, W.L.; data curation, W.L. and Y.S.; writing—original draft preparation, W.L. and Y.S.; writing—review and editing, Y.S.; visualization, W.L. and C.C.; supervision, Y.S. and G.X.; project administration, Y.S.; funding acquisition, Y.S. All authors have read and agreed to the published version of the manuscript.
Funding
This research was funded by the National Natural Science Foundation of China (Grant No. 62074027).
Data Availability Statement
The data presented in this study are available on request from the corresponding author.
Conflicts of Interest
The authors declare no conflict of interest.
Footnotes
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
- 1.Han M., Wang H., Yang Y., Liang C., Bai W., Yan Z., Li H., Xue Y., Wang X., Akar B., et al. Three-dimensional piezoelectric polymer microsystems for vibrational energy harvesting, robotic interfaces and biomedical implants. Nat. Electron. 2019;2:26–35. doi: 10.1038/s41928-018-0189-7. [DOI] [Google Scholar]
- 2.Lim S., Son D., Kim J., Lee Y.B., Song J.K., Choi S., Lee D.J., Kim J.H., Lee M., Hyeon T., et al. Transparent and Stretchable Interactive Human Machine Interface Based on Patterned Graphene Heterostructures. Adv. Funct. Mater. 2015;25:375–383. doi: 10.1002/adfm.201402987. [DOI] [Google Scholar]
- 3.Wang X., Song J., Liu J., Wang Z.L. Direct-Current Nanogenerator Driven by Ultrasonic Waves. Science. 2007;316:102–105. doi: 10.1126/science.1139366. [DOI] [PubMed] [Google Scholar]
- 4.Chorsi M.T., Curry E.J., Chorsi H.T., Das R., Baroody J., Purohit P.K., Ilies H., Nguyen T.D. Piezoelectric biomaterials for sensors and actuators. Adv. Mater. 2019;31:1802084. doi: 10.1002/adma.201802084. [DOI] [PubMed] [Google Scholar]
- 5.Deng Y., Wang J.L., Zhu K.R., Zhang M.S., Hong J.M., Gu Q.R., Yin Z. Synthesis and characterization of single-crystal PbTiO3 nanorods. Mater. Lett. 2005;59:3272–3275. doi: 10.1016/j.matlet.2005.05.056. [DOI] [Google Scholar]
- 6.Li F., Cabral M.J., Xu B., Cheng Z., Dickey E.C., LeBeau J.M., Wang J.L., Luo J., Taylor S., Hackenberger W., et al. Giant piezoelectricity of Sm-doped Pb(Mg1/3Nb2/3)O3-PbTiO3 single crystals. Science. 2019;364:264–268. doi: 10.1126/science.aaw2781. [DOI] [PubMed] [Google Scholar]
- 7.Wang K., Malič B., Wu J. Shifting the phase boundary: Potassium sodium niobate derivates. MRS Bull. 2018;43:607–611. doi: 10.1557/mrs.2018.178. [DOI] [Google Scholar]
- 8.Helden L., Bogula L., Janolin P., Hanke M., Breuer T., Schmidbauer M., Ganschow S., Schwarzkopf J. Huge impact of compressive strain on phase transition temperatures in epitaxial ferroelectric KxNa1−xNbO3 thin films. Appl. Phys. Lett. 2019;114:232905. doi: 10.1063/1.5094405. [DOI] [Google Scholar]
- 9.Schmidbauer M., Braun D., Markurt T., Hanke M., Schwarzkopf J. Strain engineering of monoclinic domains in KxNa1-xNbO3 epitaxial layers: A pathway to enhanced piezoelectric properties. Nanotechnology. 2017;28:24LT02. doi: 10.1088/1361-6528/aa715a. [DOI] [PubMed] [Google Scholar]
- 10.Wang K., Li J.F. Domain Engineering of Lead-Free Li-Modified (K, Na)NbO3 Polycrystals with Highly Enhanced Piezoelectricity. Adv. Funct. Mater. 2010;20:1924–1929. doi: 10.1002/adfm.201000284. [DOI] [Google Scholar]
- 11.Hollenstein E., Davis M., Damjanovic D., Setter N. Piezoelectric properties of Li- and Ta-modified (K0.5Na0.5)NbO3 ceramics. Appl. Phys. Lett. 2005;87:82905. doi: 10.1063/1.2123387. [DOI] [Google Scholar]
- 12.Matsubara M., Kikuta K., Hirano S. Piezoelectric properties of (K0.5Na0.5)(Nb1−xTax)O3−K5.4CuTa10O29 ceramics. J. Appl. Phys. 2005;97:114105. doi: 10.1063/1.1926396. [DOI] [Google Scholar]
- 13.Mgbemere H.E., Hinterstein M., Schneider G.A. Structural phase transitions and electrical properties of (KxNa1−x)NbO3-based ceramics modified with Mn. J. Eur. Ceram. Soc. 2012;32:4341–4352. doi: 10.1016/j.jeurceramsoc.2012.07.033. [DOI] [Google Scholar]
- 14.Shirane G., Newnham R., Pepinsky R. Dielectric Properties and Phase Transitions of NaNbO3 and (Na,K)NbO3. Phys. Rev. 1954;96:581. doi: 10.1103/PhysRev.96.581. [DOI] [Google Scholar]
- 15.Jiang M., Zhang J., Rao G., Li D., Randall C.A., Li T., Peng B., Li L., Gu Z., Liu X., et al. Ultrahigh piezoelectric coefficient of a lead-free K0.5Na0.5NbO3-based single crystal fabricated by a simple seed-free solid-state growth method. J. Mater. Chem. C. 2019;7:14816–14844. doi: 10.1039/C9TC05143K. [DOI] [Google Scholar]
- 16.Liu H., Veber P., Rödel J., Rytz D., Fabritchnyi P.B., Afanasov M.I., Patterson E.A., Frömling T., Maglione M., Koruza J. High-performance piezoelectric (K,Na,Li)(Nb,Ta,Sb)O3 single crystals by oxygen annealing. Acta Mater. 2018;148:499–507. doi: 10.1016/j.actamat.2018.02.026. [DOI] [Google Scholar]
- 17.Zhu B., Zhu Y., Yang J., Ou-Yang J., Yang X., Li Y., Wei W. New Potassium Sodium Niobate Single Crystal with Thickness-independent High-performance for Photoacoustic Angiography of Atherosclerotic Lesion. Sci. Rep. 2016;6:39679. doi: 10.1038/srep39679. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 18.Ma J., Xue S., Zhao X., Wang F., Tang Y., Duan Z., Wang T., Shi W., Yue Q., Zhou H., et al. High frequency transducer for vessel imaging based on lead-free Mn-doped (K0.44Na0.56)NbO3 single crystal. Appl. Phys. Lett. 2017;111:092903. doi: 10.1063/1.4990072. [DOI] [Google Scholar]
- 19.Koruza J., Liu H., Höfling M., Zhang M.-H., Veber P. (K,Na)NbO3-based piezoelectric single crystals: Growth methods, properties, and applications. J. Mater. Res. 2020;35:990–1016. doi: 10.1557/jmr.2019.391. [DOI] [Google Scholar]
- 20.Rodel J., Li J.-F. Lead-free piezoceramics: Status and perspectives. MRS Bull. 2018;43:576–580. doi: 10.1557/mrs.2018.181. [DOI] [Google Scholar]
- 21.Li J.F., Wang K., Zhu F.Y., Cheng L.Q., Yao F.Z. (K,Na)NbO3-Based Lead-Free Piezoceramics: Fundamental Aspects, Processing Technologies, and Remaining Challenges. J. Am. Ceram. Soc. 2013;96:3677–3696. doi: 10.1111/jace.12715. [DOI] [Google Scholar]
- 22.Li J.-F., Wang K., Zhang B.-P., Zhang L.-M. Ferroelectric and Piezoelectric Properties of Fine-Grained Na0.5K0.5NbO3 Lead-Free Piezoelectric Ceramics Prepared by Spark Plasma Sintering. J. Am. Ceram. Soc. 2006;89:706–709. doi: 10.1111/j.1551-2916.2005.00743.x. [DOI] [Google Scholar]
- 23.Yao F., Wang K., Cheng L., Zhang X., Zhang W., Zhu F., Li J. Nanodomain Engineered (K,Na)NbO3 Lead-Free Piezoceramics: Enhanced Thermal and Cycling Reliabilities. J. Am. Ceram. Soc. 2015;98:448–454. doi: 10.1111/jace.13265. [DOI] [Google Scholar]
- 24.Lv X., Zhang J., Liu Y., Li F., Zhang X., Wu J. Synergetic Contributions in Phase Boundary Engineering to the Piezoelectricity of Potassium Sodium Niobate Lead-Free Piezoceramics. ACS Appl. Mater. Interfaces. 2020;12:39455–39461. doi: 10.1021/acsami.0c12424. [DOI] [PubMed] [Google Scholar]
- 25.Wu J., Xiao D., Zhu J. Potassium–sodium niobate lead-free piezoelectric materials: Past, present, and future of phase boundaries. Chem. Rev. 2015;115:2559–2595. doi: 10.1021/cr5006809. [DOI] [PubMed] [Google Scholar]
- 26.Li P., Zhai J., Shen B., Zhang S., Li X., Zhu F., Zhang X. Ultrahigh piezoelectric properties in textured (K, Na) NbO3-based lead-free ceramics. Adv. Mater. 2018;30:1705171. doi: 10.1002/adma.201705171. [DOI] [PubMed] [Google Scholar]
- 27.Uchino K., OH K. Piezoelectric anisotropy and polarization sublattice coupling in perovskite crystals. J. Am. Ceram. Soc. 1991;74:1131–1134. doi: 10.1111/j.1151-2916.1991.tb04354.x. [DOI] [Google Scholar]
- 28.Takeuchi H., Jyomura S., Yamamoto E., Ito Y. Electromechanical properties of (Pb, Ln) (Ti, Mn) O3 ceramics (Ln= rare earths) J. Acoust. Soc. Am. 1982;72:1114–1120. doi: 10.1121/1.388319. [DOI] [Google Scholar]
- 29.Newnham K., Skinner D., Cross L. Connectivity and piezoelectric-pyroelectric composites. Mat. Res. Bull. 1978;13:525–536. doi: 10.1016/0025-5408(78)90161-7. [DOI] [Google Scholar]
- 30.Damjanovic D. Ferroelectric, dielectric and piezoelectric properties of ferroelectric thin films and ceramics. Rep. Prog. Phys. 1998;61:1267. doi: 10.1088/0034-4885/61/9/002. [DOI] [Google Scholar]
- 31.Pohlmann H., Wang J., Wang B., Chen L. A thermodynamic potential and the temperature-composition phase diagram for single-crystalline K1-xNaxNbO3 (0 ≤ x ≤ 0.5) Appl. Phys. Lett. 2017;110:102906. doi: 10.1063/1.4978360. [DOI] [Google Scholar]
- 32.Wang B., Chen N., Wang J., Chen L. Ferroelectric domain structures and temperature-misfit strain phase diagrams of K1-xNaxNbO3 thin films: A phase-field study. Appl. Phys. Lett. 2019;115:092902. doi: 10.1063/1.5116910. [DOI] [Google Scholar]
- 33.Cao G., Huang H., Liang D., Ma X. A phenomenological potential and ferroelectric properties of BaTiO3–CaTiO3 solid solution. Ceram. Int. 2017;43:6671–6676. doi: 10.1016/j.ceramint.2017.02.058. [DOI] [Google Scholar]
- 34.Zhou M., Wang J., Chen L., Nan C. Strain, temperature, and electric-field effects on the phase transition and piezoelectric responses of K0.5Na0.5NbO3 thin films. J. Appl. Phys. 2018;123:154106. doi: 10.1063/1.5027505. [DOI] [Google Scholar]
- 35.Tagantsev A.K. Landau Expansion for Ferroelectrics: Which Variable to Use? Ferroelectrics. 2008;375:19–27. doi: 10.1080/00150190802437746. [DOI] [Google Scholar]
- 36.Li L., Gong Y., Gong L., Dong H., Yi X., Zheng X. Low-temperature hydro/solvothermal synthesis of Ta-modified K0.5Na0.5NbO powders and piezoelectric properties of corresponding ceramics. Mater. Des. 2012;33:362–366. doi: 10.1016/j.matdes.2011.03.023. [DOI] [Google Scholar]
- 37.Liang L., Li Y., Hu S., Chen L., Lu G. Piezoelectric anisotropy of a KNbO3 single crystal. J. Appl. Phys. 2010;108:094111. doi: 10.1063/1.3511336. [DOI] [Google Scholar]
- 38.Budimir M., Damjanovic D., Setter N. Piezoelectric anisotropy–phase transition relations in perovskite single crystals. J. Appl. Phys. 2003;94:6753–6761. doi: 10.1063/1.1625080. [DOI] [Google Scholar]
- 39.Long C., Li T., Fan H., Wu Y., Zhou L., Li Y., Xiao L., Li Y. Li-substituted K0.5Na0.5NbO3-based piezoelectric ceramics: Crystalstructures and the effect of atmosphere on electrical properties. J. Alloy. Compd. 2016;658:839–847. doi: 10.1016/j.jallcom.2015.10.245. [DOI] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Data Availability Statement
The data presented in this study are available on request from the corresponding author.