CaMn₃^IVO₄ Cubane Models of the Oxygen Evolving Complex: Spin Ground States S < 9/2 and the Effect of Oxo Protonation

Abstract

We report single crystal XRD and MicroED structure, magnetic susceptibility, and EPR data of a series of CaMn₃^IVO₄ and YMn₃^IVO₄ complexes as structural and spectroscopic models of the cuboidal subunit of the OEC. The effect of changes in heterometal identity, cluster geometry, and bridging oxo protonation on spin state structure was investigated. In contrast to previous computational models, we show that the spin ground state of CaMn₃^IVO₄ complexes and variants with protonated oxo moieties need not be S = 9/2. Desymmetrization of the pseudo-C₃ symmetric Ca(Y)Mn₃^IVO₄ core leads to a lower S = 5/2 spin ground state. The magnitude of the magnetic exchange coupling is attenuated upon oxo protonation, and an S = 3/2 spin ground state is observed in CaMn₃^IVO₃(OH). Our studies complement the observation that the interconversion between the low spin and high spin forms of the S₂ state is pH dependent, suggesting that (de)protonation of bridging or terminal oxygen atoms in the OEC may be connected to spin state changes.

Graphical Abstract

To better describe the electronic structure of the S_n state intermediates of Photosystem II, a ferromagnetically coupled CaMn₃^IVO₄ subunit with an S = 9/2 ground state has been proposed. This assignment has played a key role in the mechanism of water oxidation, but structure–electronic structure studies of CaMn₃^IVO₄ complexes remain rare. Through cluster desymmetrization or oxo protonation, lower spin ground states are found to be accessible, challenging prior models.

Introduction

Biological water oxidation is catalyzed at the oxygen evolving complex (OEC) of Photosystem II.^[1-3] The OEC has been characterized by crystallography, revealing a heterometallic CaMn₃O₄ cubane motif binding to a fourth Mn center via bridging (hydr)oxo moieties.^[4-6] Mechanistic studies have been performed within the context of the Joliot-Kok cycle of S_n (n = 0~4) states.^[7-8] Starting from the dark-stable S₁ state (Mn₂^IIIMn₂^IV), light-induced one e⁻ oxidation leads to the formation of the S₂ state, and numerous studies have been performed to better understand the (electronic) structure of the S₂ state and the requirements needed to advance to the higher S_n states.^[9-19] In the absence of unambiguous and direct structural data concerning the O─O bond forming S₄ intermediate, structural and spectroscopic characterization of lower S_n state intermediates influence mechanistic proposals for O─O bond formation.^[20-24]

Connected to Mn oxidation state and structural changes, each of the S_n state intermediates adopts a unique electronic structure with a characteristic spin ground state S_G:^[25] S_G(S₀) = 1/2,^[26] S_G(S₁) = 0^[27] or higher^[28-29], S_G(S₂) = 1/2 or 5/2,^[30-32] S_G(S₃) = 3.^{[15, 33-35]} The interconversion between the S_G = 1/2 and S_G = 5/2 forms of the S₂ state is notable.^[30] One interpretation invokes structural changes in the CaMn₄ core centered around the location of a particular O(5) oxo moiety, with concomitant changes in the magnetic coupling interactions resulting in a different S_G.^[13] In the so-called “closed-cubane” structure with S_G = 5/2, ferromagnetic coupling between Mn(1), Mn(2), and Mn(3) is proposed to lead to an S = 9/2 spin state for the CaMn₃^IVO₄ cuboidal subunit; antiferromagnetic coupling to the dangling Mn(4) would lead to the observed S_G = 5/2 (Figure 1). In the “open-cubane” structure where O(5) bridges Mn(3) and Mn(4) instead, an internal valence redistribution is proposed, with the CaMn^IIIMn₂^IV subsite now proposed to have an S = 1 spin state; antiferromagnetic coupling to the dangling Mn(4) would lead to the observed S_G = 1/2.^{[13, 25]} The above structural isomerism model helps explain the high- and low-spin forms of the S₂ state. Importantly, however, the model depends on the closed-cubane motif (region within the red box, Figure 1) having a ferromagnetically coupled S = 9/2 spin state. At room temperature, under turnover conditions, only the open-cubane form has been observed crystallographically thus far.^{[6, 36]} While experimental data remains very limited, two synthetic CaMn₃^IVO₄ model complexes have been reported with S_G = 9/2 (Figure 2),^[37-39] supporting the coupling scheme for the closed-cubane structure and the possible structural flexibility of the OEC core as an explanation for the spin state interconversion observed in the S₂ state. Subsequent XFEL structural studies show the incorporation of a sixth oxygen O(6) in the cleft between Mn(1) and Mn(4), a possible site for O─O bond formation (Figure 1).^[6] A similar magnetic coupling scheme has been advanced for the S₃ state (Mn₄^IV), featuring a ferromagnetically coupled CaMn₃^IVO₄ subunit with an S = 9/2 spin state; antiferromagnetic coupling to Mn(4) would lead to the observed S_G = 3 (Figure 1).^{[15, 40]} Similar to the S₂ state, a structural interpretation for the observed spectral heterogeneity in the S₃ state is the subject of ongoing investigation.^[34] An alternative interpretation for the spin state interconversion in the S₂ state is based on the observation that this process is pH dependent, with a higher pH favoring the high-spin ground state.^{[24, 41]} Notably, other effects such as low temperature irradiation of the S₁ state and the introduction of F⁻ favor formation of the high-spin state.^[42-43] Insofar as the pH effect, the aquo moiety bound to Mn(4) was hypothesized to be the site that is deprotonated following the observation that no pH dependent behavior is observed when NH₃ is bound to this aquo site.^{[17, 24, 41]} Structural changes to the OEC through either oxo exchange or (de)protonation would be accompanied by concomitant changes in the nature and magnitude of the magnetic exchange coupling J, which in turn affect not only the S_G of the cluster but also other spectroscopic properties such as the sign and magnitude of the projected ⁵⁵Mn hyperfine coupling constants.^{[15, 39, 44]} In a related computational study, protonation of the bridging oxo O(4) (Figure 1) in the S₂ state is proposed to lead to a high spin state, while maintaining the open-cubane structure.^[24] XAS studies performed on the high-spin form of the S₂ state indeed show structural differences to the low-spin form, but extracted Mn-Mn distances do not appear consistent with the closed-cubane structure.^[18] Such computational and experimental discrepancies highlight the need for data on model compounds in which mechanistic hypotheses can be tested and the effects of structural changes on the electronic structure of the OEC can be evaluated.

Figure 1.

Proposed or observed structures of the inorganic CaMn₄ core of the OEC in the S₂ and S₃ states. Trinuclear subsites boxed for emphasis, especially the proposed closed cubane motif in red, which is the focus of this study. Nature of the calculated magnetic exchange coupling interactions shown in red (antiferromagnetic) and blue (ferromagnetic) dashed lines. Coupling between Mn(4) and the trinuclear subsite leads to the observed spin ground states S_G.

Figure 2.

Previously reported complexes featuring a CaMn₃^IVO₄ core.

Despite significant synthetic efforts to prepare heterometallic complexes that mimic the OEC in terms of structure and redox state,^{[37, 45-58]} the effect of structural distortions or protonation state on the electronic structure of multimetallic Mn complexes is not well understood, and can be summarized as follows.^[59-63] In a series of dinuclear complexes featuring Mn₂^IVO₂, Mn₂^IVO(OH), and Mn₂^IV(OH)₂ cores, the antiferromagnetic coupling is strongest in the fully deprotonated form, with successive oxo protonations resulting in weaker antiferromagnetic coupling.^[60] A complete shift from ferromagnetic to antiferromagnetic coupling is observed upon a single protonation of the adamantane-shaped [Mn₄^IVO₆]⁴⁺ core.^[59] While representing incomplete models of the OEC, CaMn₃^IVO₄ complexes offer an opportunity to better understand the magnetic properties of the OEC, as it relates to current discussions on the geometry and the spin state of the S₂ state. Any changes to the spin state of the cubane portion would result in a different total spin of the cofactor when magnetically coupled to the dangler Mn(4). The ground state of both the pseudo-C₃ symmetric and the Ca-bound asymmetric Ca₂Mn₃^IVO₄ model complexes has been assigned to S_G = 9/2 on the basis of magnetic, spectroscopic, and computational data (Figure 2).^{[37-38, 49, 64]} Subsequent computational studies concluded that the spin ground state of cuboidal CaMn₃^IVO₄ complexes and all possible protonated analogues CaMn₃^IVO_n(OH)_(4–n) (n = 0~4) is S_G = 9/2.^[64] Studies that probe the effect of distinct structural changes or oxo protonation on the electronic structure of CaMn₃^IVO₄ complexes have not been reported.

Herein, we report single crystal XRD and MicroED structure, magnetic susceptibility, and EPR data of a series of cuboidal complexes featuring CaMn₃^IVO₄, YMn₃^IVO₄, and CaMn₃^IVO₃(OH) cores. Our results show that the electronic structure of CaMn₃^IVO₄ complexes is highly sensitive to changes promoted by the nature of the supporting ligands (reminiscent to studies of Mn^IIIMn₃^IVO₄ complexes)^[65] and the protonation state. We show that ground states such as S_G = 5/2 and S_G = 3/2 are possible in CaMn₃^IVO₄ complexes, which challenge the proposed model that a closed cubane such as that in the S₂ state of the OEC must be S_G = 9/2.

Results and Discussion

Synthesis and crystal structure.

The acetate-bridged complex 1-Ca was used as a precursor for other complexes (Scheme 1).^[37] Magnetic susceptibility and computational studies on 1-Ca indicate an S_G = 9/2 ground state.^{[38, 64]} Treatment of 1-Ca with Y(OTf)₃ leads to the analogous complex 1-Y.^[50] Magnetic studies show that 1-Y has a similar S_G = 9/2.^[66] Desymmetrization of the pseudo-C₃ symmetric complexes 1-Ca and 1-Y was achieved via substitution of two acetate moieties with a chelating bis-oxime proligand (H₂N₄O₂). Treatment of 1-Ca with H₂N₄O₂ results in the formation of 2-Ca via a protonolysis reaction.^[67] Similarly, treatment of 1-Y with H₂N₄O₂ results in the formation of the analogous complex 2-Y.^[58] Due to its limited solubility, suitable samples of 2-Ca for single crystal X-ray diffraction studies could not be obtained. The structure of 2-Ca was determined using MicroED.^[68] A powder sample of 2-Ca was judiciously treated with MeCN to obtain a TEM grid with non-aggregated particles of 2-Ca without loss of crystallinity. Complex 2-Ca dissolves in MeCN only sparingly, which is critical in obtaining a TEM grid with dispersed but intact microcrystals. The resolution and refinement parameters of 2-Ca were similar to those obtained for other MicroED organometallic structures (Table S4).^[68] Importantly, the MicroED structure of 2-Ca shows that the CaMn₃O₄ core is preserved, with a coordination sphere that is analogous to that of 2-Y.^{[58, 67]} The CaMn₃O₄ core of 2-Ca can be further desymmetrized via treatment with 2,6-lutidinium triflate, which results in the protonation of a unique oxo in between the oximate moieties to afford a complex with a CaMn₃O₃(OH) core (3-Ca).

Scheme 1.

Synthesis of complexes studied in this work.

Toward expanding the series of CaMn₃O₄ complexes with chelating ligands of similar basicity to the oximate moieties, an amidate-bridged complex was targeted. Treatment of 1-Ca with a triethylene glycol derived bis-amide (H₂diam) in the presence of NaO^tBu leads to the formation of 4-Ca. The crystal structure of 4-Ca is consistent with the LCaMn₃O₄(diam)(OAc) formulation in which two acetate moieties of 1-Ca are replaced by the chelating bis-amidate moiety (Figure 3). Overall, 1-Ca(Y), 2-Ca(Y), 3-Ca, and 4-Ca represent a unique series of complexes mimicking the cuboidal substructure of the OEC in which cluster symmetry, heterometal identity, bridging ligand, and oxo protonation state is systematically varied.

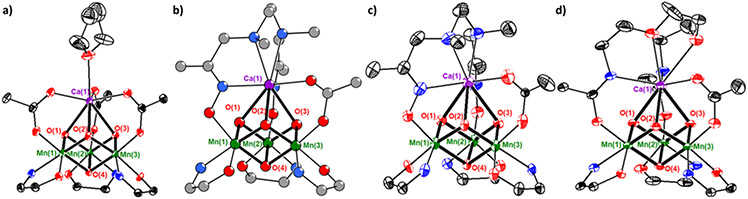
Figure 3.

Truncated crystal structures of a) 1-Ca,³⁷ b) 2-Ca, c) 3-Ca,⁶⁷ d) 4-Ca. Ellipsoids at 50% probability except for 2-Ca (ball and stick model).

Metal-oxo distances were compared across the series to understand the effect of chelating ligands and oxo protonation on structure (Table S1). Within each complex 1-Ca or 4-Ca, Ca-oxo, Mn-Mn, and M-O(4) distances are all similar to each other; the other six Mn-oxo distances alternate between 1.83 and 1.87 Å along the pseudo-C₃ axis of symmetry parallel to the Ca-O(4) vector. A similar pseudo-C₃ symmetry is observed in 1-Y and in other known analogues such as 1-Gd.^[50] The high symmetry of the metal-oxo core is broken with the oximate-bridged complexes. Because precise structural metrics could not be obtained from the MicroED structure of 2-Ca, the structure of 2-Y was used as a surrogate for the structure of 2-Ca for structural comparisons. Notably, the structure of 2-Y and the Gd analogue 2-Gd are very similar to each other, with Mn-oxo distances within 0.01 Å, indicating that general structural trends are observable. In 2-Y, the Y-O(1) distance is significantly shorter by 0.1 Å than the other two Y-oxo distances. O(1) is the unique oxo that is located between the two oximate donors, suggesting that the metal-oxo core has been structurally and electronically desymmetrized. While the difference is smaller, the Mn-oxo distances trans to the Mn-oximate bonds are slightly elongated compared to the corresponding Mn-oxo distance trans to the Mn-acetate/amidate bond. Similarly, Mn-Mn distances in 2-Y also distort in a manner that is consistent with a pseudo-C_S symmetry with the mirror plane containing the Y(1)–O(1) vector and bisecting the Mn(1)–Mn(2) vector. On the basis that the three-fold symmetry of 1-Y(Gd) is broken upon substitution of two acetates with the bridging oxime H₂N₄O₂, a similar structural distortion is expected in 2-Ca, away from the pseudo-C₃ symmetry of 1-Ca. The structure of 3-Ca is also consistent with a pseudo-C_S symmetry, with Mn-(μ³-OH) distances that are slightly elongated by roughly 0.1 Å in comparison to the corresponding Mn-(μ³-O) distances in 1-Ca. Overall, distinct structural changes are observed depending on the nature of the bridging ligands and the protonation state of bridging oxos; these relatively small structural changes across the series have a significant influence in the electronic structure of the CaMn₃^IVO₄ core.

Magnetometry.

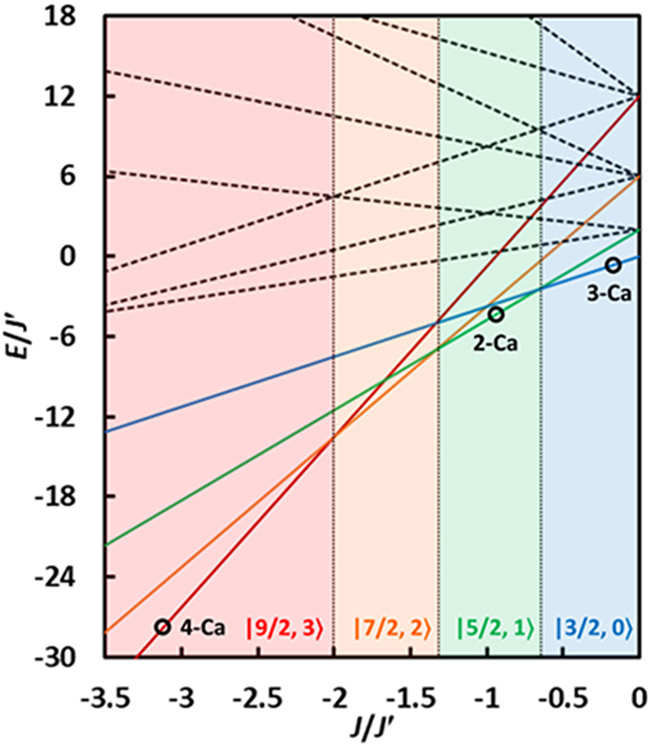
To obtain insight into the electronic structure of the series of CaMn₃^IV complexes, magnetic susceptibility studies were performed (Figure 4). For a pseudo-C_S symmetric Mn₃^IV core, an isotropic spin exchange Hamiltonian (eq 1) with two distinct magnetic interactions can be employed, with a unique J' = J₁₂ and J = J₁₃ = J₂₃ (subscripts follow the Mn numbering scheme in Figure 3).^[69] For Mn₃^IV systems with local spin S_i = 3/2, application of the vector coupling model S' = S₁ + S₂, S_T = S' + S₃ gives rise to twelve ∣S_T, S'⟩ states, in which S' varies in integer increments from 0 to 2S_i (i.e. S' = 0, 1, 2, 3); for each value of S', S_T varies in integer increments from ∣S' − S_i∣ to S' + S_i (i.e. for S' = 3, S_T = 3/2, 5/2, 7/2, 9/2). The energies of the individual ∣S_T, S'⟩ states can be expressed as shown in eq 2. By incorporating the energies of the twelve ∣S_T, S'⟩ states into the Van Vleck equation, an analytical solution for the magnetic susceptibility X can be obtained. Qualitatively, one can regard X to be derived from the sum of the individual ∣S_T, S'⟩ states weighed by their Boltzmann populations. At sufficiently low temperatures where only the ground state is significantly populated, reduced magnetization studies can be performed to obtain information about zero field splitting. Finally, while the relative energies of the individual states depend on the sign and magnitude of J and J', the ground state is more easily determined from the ratio of J/J' (Figure 5).^{[49, 64]}

$$\widehat{H}=-2J(S_1{S}_3+S_2{S}_3)-2J^{\prime }{S}_1{S}_2$$ (1)

$$E\mid S_{\tau },S^{\prime }\rangle =-J{S}_{\tau }(S_{\tau }+1)-(J^{\prime }-J)S^{\prime }(S^{\prime }+1)$$ (2)

Figure 4.

a) XT vs. T plot of complexes 2-Ca, 3-Ca, and 4-Ca with corresponding fits to the data. b)–d) Reduced magnetization plot of 2-Ca, 3-Ca, and 4-Ca, respectively. See text for fit parameters.

Figure 5.

Relative energies E/J' of the ∣S_T, S'⟩ states as a function of J/J′ (for J′ < 0). Each colored region represents a different spin ground state. Experimental points shown as black circles.

Structurally most similar to 1-Ca, 4-Ca was studied by magnetometry (Figure 4a). The XT value (units of emu K mol⁻¹) of 5.60 at 300 K increases with cooling to reach a maximum XT value of 11.98 at 2 K. This maximum value is in good agreement with the expected value of 12.375 for S_G = 9/2 (g = 2). Previous magnetic studies on 1-Ca show that while the structure is more consistent with a pseudo-C₃ symmetry, the data is better fit with two values J = 3.5 cm⁻¹ and J' = −1.8 cm⁻¹.^[38] The data for 4-Ca was similarly fit with two values J = 5.0 cm⁻¹, J' = −1.6 cm⁻¹, and g = 1.97. Using the fitted J values, the relative energies of the individual electronic states can be calculated. The ∣9/2, 3⟩ ground state is separated from the ∣7/2, 2⟩ first excited state by 5.5 cm⁻¹ and from the ∣5/2, 1⟩ second excited state by 8.8 cm⁻¹. The small separation between the ground and excites states is evident in the fact that XT decreases sharply from the maximum value upon warming. The J/J' ratio of −3.125 is fully consistent with S_G = 9/2 and comparable to the value of −3.75 obtained for the asymmetric Ca₂Mn₃^IVO₄ complex.^[49] The reduced magnetization data for 4-Ca (Figure 4d) shows little deviation from the ideal Brillouin function for S = 9/2, indicating the presence of a small zero-field splitting estimated at D = −0.1 cm⁻¹ by EPR. Magnetic studies on 4-Ca show that changing the bridging ligands from acetates to amidates does not result in significant changes to the electronic structure of the complex in the absence of notable structural distortions to the metal-oxo core.

Featuring a lower symmetry, distorted metal-oxo core compared to 1-Ca and 4-Ca, 2-Ca was studied by magnetometry (Figure 4a). The XT value of 4.526 at 300 K decreases slowly to a local minimum XT value of 4.313 at 150 K. Upon further cooling, the XT value increases slowly to reach a value of 4.405 at 15 K, in good agreement with the expected value of 4.375 for S_G = 5/2 (g = 2). Further decrease in XT with temperature can be attributed to intermolecular antiferromagnetic interactions and/or zero-field splitting. The temperature dependence of XT observed in 2-Ca is indicative of an irregular spin state structure where the first excited state is S ≤ 3/2 and the second excited state is S ≥ 5/2. Similar magnetic behavior has been observed in other trinuclear systems.^[70-73] On the basis of the small curvature of the XT vs. T curve, the expected separation between the spin ground state and the first excited state is in the order of hundreds of wavenumbers. To simulate the susceptibility data, the following parameters were used: g = 1.99, J = 250 ± 50 cm⁻¹, J' = −280 ± 50 cm⁻¹. Due to the small curvature of the XT vs. T curve, a relatively large variance is reported for the J values, which should not be treated as exact values but as estimates. However, the range in the J/J' ratio is narrower, between −0.90 and −0.93, and falls within the predicted region for S_G = 5/2 (Figure 5).^[64] The ∣5/2, 1⟩ ground state is separated from the ∣3/2, 0⟩ first excited state by 210 ± 50 cm⁻¹ and from the ∣7/2, 2⟩ second excited state by 350 ± 50 cm⁻¹. Thermally well-isolated spin ground states have been observed in multinuclear complexes, with values of ∣J∣ in the range of 160~900 cm⁻¹.^[74-76] Such systems behave as Curie paramagnets, with no temperature dependence of XT. In the absence of double exchange or close metal-metal contacts, the physical nature of the large increase of J in 2-Ca remains unclear, but we provide the following hypothesis. In 1-Ca and 4-Ca where each Mn(IV) ion is coordinated by mostly σ-only ligands, the principal symmetry axis for each Mn center is ill-defined, and thus several competing exchange pathways can be envisioned between d_xy, d_xz, and d_yz, resulting in the overall weak coupling observed. In 2-Ca and 2-Y, in contrast, Mn(1) and Mn(2) are coordinated by oximates which may act as pπ-donors (also more basic σ-donors than carboxylates). This defines the Mn-O(oxime) vector as the z axis for Mn(1) and Mn(2). Because d_xz and d_yz may be involved in dπ-pπ bonding with the oximates, contributions from exchange pathways such as (d_xy)¹∣O∣(d_xz)¹ that weaken the overall magnitude of the antiferromagnetic coupling may be reduced. The exchange pathway through the geometrically “aligned” d_xy orbitals on Mn(1) and Mn(2) may explain the larger J. For 2-Ca, the reduced magnetization isofield at 7 T reaches a value of 4.71 N_Aμ_B at 1.8 K, consistent with S_G = 5/2 (Figure 4b). Reduced magnetization isofields were simulated assuming a single value of D = +1.46 cm⁻¹ or −1.17 cm⁻¹. In many cases, powder susceptibility data is insensitive to the sign of D.^[77] This value of D is inconsistent with the value obtained from EPR, at 0.3 cm⁻¹ (Figure 6a). Using this value instead and incorporating the mean field model of intermolecular antiferromagnetic interaction zJ, a satisfactory fit can be obtained (Figure S7). The magnetic data on 2-Y, for which a high resolution crystal structure has been obtained, is also consistent with S_G = 5/2, showing that the change in the spin ground state from S_G = 9/2 to S_G = 5/2 is also observed going from 1-Y to 2-Y (Figure S8). The data on 2-Y was simulated with the following parameters: g = 2.0, J = 280 ± 50 cm⁻¹, J' = −315 ± 55 cm⁻¹, J/J' = −0.89. Importantly, magnetic studies on 2-Ca show that an intact CaMn₃^IVO₄ cubane moiety need not necessarily have an S_G = 9/2 ground state.

Figure 6.

X-band EPR spectra collected at 5 K (black traces) and corresponding simulations (dashed red traces). a) 2-Ca, S = 5/2, g = 1.96, D = 0.3 cm⁻¹, E/D = 0.06, fwhm E/D distribution 0.03. b) 3-Ca, S = 3/2, g = 1.98, D = 0.28 cm⁻¹, E/D = 0.33. c) 4-Ca, S = 9/2, g = 1.99, D = −0.1 cm⁻¹, E/D = 0.11.

Featuring a bridging hydroxo moiety, 3-Ca was studied by magnetometry (Figure 4). The XT value of 3.856 at 300 K decreases monotonically with temperature to reach a value of 1.886 at 10 K, in good agreement with the expected value of 1.875 for S_G = 3/2 (g = 2). To simulate the susceptibility data, the following parameters were used: g = 2.0, J = +11 cm⁻¹, J' = −55 cm⁻¹. The J/J' ratio of −0.2 falls within the predicted region for S_G = 3/2 (Figure 5).^[64] The ∣3/2, 0⟩ ground state is separated from the ∣5/2, 1⟩ first excited state by 77 cm⁻¹ and from the ∣3/2, 1⟩ second excited state by 132 cm⁻¹. Similar to 4-Ca, magnetization studies show little deviation from the ideal Brillouin function for S = 3/2, indicating the presence of a small zero-field splitting (Figure 4c). Importantly, magnetic studies on 3-Ca show that protonation of a single bridging oxo moiety has a strong influence in attenuating the magnitude of the magnetic coupling interactions.^[60] While protonation of 2-Ca does not change the nature (sign) of the magnetic coupling interactions in 3-Ca, the relative magnitudes of J and J' result in a change of spin ground state, from S_G = 5/2 in 2-Ca to S_G = 3/2 in 3-Ca. In a tetranuclear Mn₄ system, a complete reversal from ferromagnetic to antiferromagnetic interactions has been reported.^[59] With a structure that closely resembles the closed-cubane subunit of the OEC, the spin state of 3-Ca differs from the predicted S_G = 9/2, and an S_G = 3/2 is observed.^[64] To obtain magnetostructural insight for the observed changes in spin ground state, the geometry of the Mn(1)-O(1/4)-Mn(2) subsite was closely examined. Mn(1)-O-Mn(2) angles increase slightly going from the pseudo-C₃ symmetric complex 1-Ca to the desymmetrized complex 2-Y (Table S2). A comparably more dramatic increase in the Mn-O-Mn angles is observed in the protonated complex 3-Ca. The observed trend of increasing Mn-O-Mn angles is consistent with the decrease in spin ground state within the series, and can be explained in the context of an increased antiferromagnetic exchange for a more linear Mn-O-Mn linkage. Complex 3-Ca with the widest Mn-O-Mn angles therefore has the smallest J/J' ratio and the lowest spin state. Most importantly, magnetic studies show that spin states such as S_G = 5/2 and 3/2 are possible for a closed-cubane structure.

EPR spectroscopy.

To obtain further insight into the electronic structure of complexes 2-Ca, 3-Ca, and 4-Ca, X-band EPR studies were conducted (Figure 6). Qualitatively, the spectrum of 2-Ca suggests that the weak field regime is operative, (D ≫ hv ≈ 0.3 cm⁻¹ at X-band), where D is the axial component of the zero-field splitting (ZFS) parameter. In such cases, as illustrated in the rhombograms for half-integer spin states S > 1/2, peak positions are primarily determined by the E/D ratio where E is the transverse component of the ZFS parameter. The spectrum of 2-Ca features three transitions at g = 6.3 (108 mT), g = 4.3 (160 mT), and g = 2 (340 mT) that can be assigned to the ∣±1/2⟩ Kramers doublet of an axial (E/D ≈ 0) S = 5/2. The spectrum of 2-Ca can be approximated using parameters that are consistent with this qualitative analysis (Figure 6a). The broad spectrum of 2-Ca was simulated with a Gaussian distribution of E/D centered at 0.06 with a full width at half-maximum of 0.03 (Figure S9). The spectrum of 2-Y is similar to that of 2-Ca, consistent with the magnetic data showing that both have an S_G = 5/2 ground state (Figure S10). The spectrum of 3-Ca features two main transitions at g = 5.1 (133 mT) and g = 2.1 (330 mT) that can be assigned to the ∣±1/2⟩ Kramers doublet of a rhombic (E/D ≈ 0.3) S = 3/2 (Figure 6b). While not amenable for a simple qualitative analysis, the spectrum of 4-Ca suggests a higher S_G = 9/2 with multiple overlapping transitions from the five Kramers doublets and is reminiscent of the spectrum of the Ca₂Mn₃O₄ complex with an S_G = 9/2 ground state (Figure 6c).^[49] The ZFS parameters of 4-Ca were obtained by simultaneously fitting spectra obtained at X- and D-band (130 GHz). At higher microwave frequencies, the electron Zeeman term that splits the energy levels of a spin system in an applied magnetic field may be appreciably larger than that of the ZFS parameter. This is the case for 4-Ca at 130 GHz, where hv >> D. In such cases, the resulting EPR spectrum is centered around the g value that defines the total spin system. The width of the spectrum is determined by D, while the spacing of the EPR transitions is determined by E/D. The D-band electron-spin echo EPR spectrum of 4-Ca (Figure S11) displays approximately nine transitions centered about g = 1.99 and spans approximately 1.5 T. The transitions that appear at magnetic fields lower than 4.67 T (g = 1.99) are evenly spaced by approximately 200 mT, while those at fields higher than 4.67 T are unevenly spaced. This is consistent with a small, negative ZFS parameter (D = −0.1 cm⁻¹) that is axial (E/D = 0.11). Overall, EPR spectroscopic studies of 2-Ca~4-Ca are consistent with the electronic structure and spin ground state description obtained from magnetic susceptibility studies.

Conclusion

In summary, a series of CaMn₃^IVO₄ cuboidal complexes has been synthesized and characterized by XRD/MicroED, magnetometry and EPR spectroscopy. To our knowledge this is the first set of experimental studies that directly addresses the effect of systematic changes in cluster geometry and bridging oxo protonation on the spin state structure of CaMn₃^IVO₄ cubane models of the OEC. With implications in the interpretation of OEC spectroscopic properties, our benchmarking results show that the electronic structure of the CaMn₃^IV core is highly sensitive to small geometric changes, nature of the bridging ligands, and protonation state of the bridging oxo moieties. Even in the absence of large oxo movements proposed to account for the high spin and low spin signals of the S₂ state of the OEC, we find that spin ground states such as S_G = 3/2, 5/2, or 9/2 are accessible for the CaMn₃^IV subsite (Figure 4). Importantly, desymmetrization of pseudo-C₃ symmetric complexes 1-Ca(1-Y) and 4-Ca with S_G = 9/2 lead to a lower, S_G = 5/2 in 2-Ca(2-Y). Protonation of a single bridging oxo moiety in 2-Ca has a strong influence in attenuating the magnitude of the magnetic exchange coupling interactions, and S_G = 3/2 is observed in 3-Ca. The nature of the magnetic exchange interactions does not need to change to result in a different spin ground state; differences in the relative ratio of J values can lead to different ground states (Figure 5). Our results strongly argue against the idea that the ground state of CaMn₃^IVO_n(OH)_(4−n) (n = 0~4) complexes must necessarily be S_G = 9/2 as previously reported; the protonation of a single bridging oxo may result in spin ground state changes in tetranuclear complexes.^[64] Nevertheless, while the present results demonstrate that closed CaMn₃^IVO_n(OH)_(4−n) cubane motifs can have spin states different from S_G = 9/2, they do not rule out such an interpretation for the CaMn₃^IV subunit of the OEC. However, they do add support to mechanistic proposals that do not require structural distortions of the overall cluster for the observation of additional spin states. For example, recent pH dependence studies show that the S_G = 1/2 form of the S₂ state converts to the S_G = 5/2 form at high pH.^[41-42] On the basis of the results presented in this study, we support an interpretation based on spin state changes following deprotonation of a bridging hydroxo or terminal aquo. Most importantly, our results indicate that interpretation of EPR signals and subsequent structural assignments should not be limited to an S = 9/2 spin state for the CaMn₃^IVO₄ subsite of the OEC.