Peak shifts and extinction under sex-specific selection

Abstract

A well-known property of sexual selection combined with a cross-sex genetic correlation (r_mf) is that it can facilitate a peak shift on the adaptive landscape. How do these diversifying effects of sexual selection + r_mf balance with the constraints imposed by such sexual antagonism, to affect the macroevolution of sexual dimorphism? Here, I extend existing quantitative genetic models of evolution on complex adaptive landscapes. Beyond recovering classical predictions for the conditions promoting a peak shift, I show that when r_mf is moderate to strong, relatively weak sexual selection is required to induce a peak shift in males only. Increasing the strength of sexual selection leads to a sexually concordant peak shift, suggesting that macroevolutionary rates of sexual dimorphism may be largely decoupled from the strength of within-population sexual selection. Accounting explicitly for demography further reveals that sex-specific peak shifts may be more likely to be successful than concordant shifts in the face of extinction, especially when natural selection is strong. An overarching conclusion is that macroevolutionary patterns of sexual dimorphism are unlikely to be readily explained by within-population estimates of selection or constraint alone.

1. Introduction

A long-standing dilemma in evolutionary biology lies in understanding how populations can evolve from one phenotypic optimum to another. When a population is under net-stabilizing selection and in the vicinity of the optimum trait value (a ‘peak’), selection will pull the population towards the nearby optimum [1], leaving alternative optima seemingly inaccessible [2]. For a peak shift to occur, some force must allow a population mean phenotype to transcend the pull of the nearby optimum and cross a fitness valley to climb a peak beyond [3–6].

Candidate phenomena that may facilitate crossing a natural-selection valley include genetic drift, a change in the environment or sexual selection. Wright famously proposed a key role for drift in valley crossing [3,5,6], although drift alone will only facilitate such a crossing with exceptional rarity, requiring very weak selection (a shallow valley) and small population size [7–9]. A change in the environment seems a likely explanation, although also an incomplete one as rates of phenotypic macroevolution do not seem to be obviously coupled to environmental upheaval [10,11]. Finally, sexual selection can readily pull a population off a viability optimum [12], resulting in a peak shift even across quite deep valleys [13]. If only one sex is under significant sexual selection, whether or not a peak shift occurs in the other sex depends on the magnitude of the cross-sex genetic correlation (r_mf) for the trait [13]. If r_mf is high enough, sexual selection in one sex will pull both sexes off of their optimum, leading to a peak shift in both sexes. More generally, directional selection on any trait can induce a peak shift in other, genetically correlated traits that themselves reside on an optimum [9]. In this way, sexual selection coupled with cross-sex genetic correlations has been proposed as a likely mechanism facilitating peak shifts and thus promoting the origin of diversity [14].

Two open questions remain in light of sexual selection's likely role in driving peak shifts. First, how do sexual selection-induced peak shifts manifest the evolution of sexual dimorphism, when adaptive landscapes are complex; that is, when sexually dimorphic and sexually monomorphic trait optima exist, what are the conditions that promote or constrain the likelihood of a peak shift to each optimum? Second, how do we reconcile the diversifying effects of sexual selection and r_mf with the constraining effects that r_mf is expected to have on male and female adaptation? Put another way, although we know sexual selection + r_mf can lead to a peak shift, we also know that this condition of sexual conflict constrains adaptation.

In this note, I extend Lande's [12] model of directional sexual selection and stabilizing natural selection towards a single optimum to the case of multiple optima. This model is similar to one analysed by Lande & Kirkpatrick [13], but is agnostic to female preference evolution, a major focus of their model. My analysis reveals two underappreciated features of peak shift models. First, large regions of parameter space (r_mf) exist in which relatively weak sexual selection is required to induce a sex-specific peak shift; strong sexual selection is expected to lead to evolution along with a line of sexual monomorphism at the macroevolutionary scale. Second, the latter type of peak shift is severely limited by extinction. Although these effects are consistent with results of previous work [9,13], to my knowledge, they have rarely been appreciated, particularly in the context of macroevolution of sexual dimorphism.

2. The model: two-sex ‘twin peaks’

Lande [12] considered a model of the evolution of sexual dimorphism by natural and sexual selection, in which natural selection favours a single optimum value for the male trait z and female trait y. His model of natural selection corresponds to a single adaptive peak on the two-sex adaptive landscape (where $\overline{W}={\overline{W}}_m{\overline{W}}_f$, figure 1a). Directional sexual selection acts independently of natural selection to redistribute fitness across individuals without changing mean absolute fitness. This scenario represents a constant mating bias for one sex, arising from, e.g. female mating preference or male–male competition. The Lande model captures several features that are expected to be common in real populations: viability selection that favours an intermediate value of a trait (e.g. tail feather length, coloration and body size) in addition to sexual selection that favours exaggerated values of the trait in males.

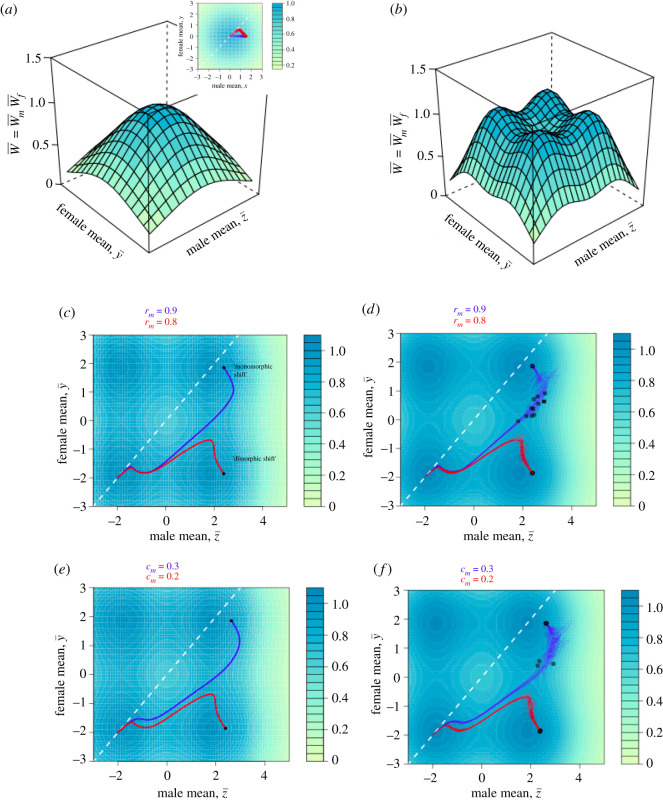
Figure 1.

Models of stabilizing selection in two sexes and peak shifts induced by sexual selection. Panels illustrate the natural selection (e.g. viability) adaptive landscape, the function ${\overline{W}}_m{\overline{W}}_f$_. In Lande's [12] original model of the evolution of sexual dimorphism by natural and sexual selection, a single multivariate optimum, corresponding to one optimum for the male trait and one for the female trait, is assumed (a). In this model, sexual selection leads to a displacement of males from their optimum (inset trajectories are shown starting from the optimum; one where r_mf = 0, blue and one where r_mf = 0.9, red), the magnitude of which is proportional to the strength of stabilizing natural selection. Extending the Lande and twin peaks model to both sexes results in two optima for each trait, or four multivariate optima on the two-sex adaptive landscape (b). As parameterized in this manuscript, the two-sex twin peaks model contains two sexually monomorphic optima, and two sexually dimorphic optima. Of interest is when and how peak shifts from an ancestral monomorphic optimum occur; (c) shows two deterministic trajectories, starting from the lower left optimum, corresponding to two different values of the cross-sex genetic correlation r_mf. Although the sexual selection is strong enough to induce a peak shift, which new optimum reached depends upon the value of r_mf; (d) illustrates 20 replicates of stochastic evolution (i.e. with drift; see electronic supplementary material for details) under the same parameter values as C and assuming female demographic dominance $(N_{t+1}=k\hspace{0.17em}{\overline{W}}_f{N}_t)$. Round circles illustrate the mean values after 10 000 generations of evolution; extinction events are denoted with a crossed circle; (e) contrasts trajectories under two strengths of sexual selection c_m, with otherwise identical parameter values; (f) illustrates stochastic evolution under the same parameter values as (e). White dashed line illustrates the line of sexual monomorphism, for reference. In (e,d), c_m = 0.2; (e,f) r_mf = 0.8; other parameter values as described in text.

We can expand the natural-selection fitness function of the Lande model to consider a scenario where more than one optimum for a trait exists, using a mixture of Gaussian functions [15] termed the ‘twin peaks’ model by Price et al. [9]. For males, this leads to the following function relating population mean fitness ${\overline{W}}_m$ to population mean phenotype $\overline{z}$:

$${\overline{W}}_m\propto \exp \{-{(\overline{z}-{\theta }_{z,1})}^2{({\omega }_{z,1}+{\mathrm{P}}_z)}^{-1}\}+\exp \{-{(\overline{z}-{\theta }_{z,2})}^2{({\omega }_{z,2}+{\mathrm{P}}_z)}^{-1}\}$$ 2.1

where ${\theta }_{z,1}$ and ${\theta }_{z,2}$ are two phenotypic optima, P_z is the phenotypic variance, and ${\omega }_{z,1}$ ${\omega }_{z,2}$ are the strengths of stabilizing selection (assumed equal throughout). This model is bimodal, with optima in the vicinity of $\theta$, for $2{\theta }^2>\omega +\mathrm{P}$. If females also have two optima, using an expression analogous to equation 1, we obtain the ‘two-sex twin peaks’ model, illustrated in figure 1b. The two-sex twin peaks model corresponds to a scenario where more than one viability optimum exists for both sexes; for example, multiple bill or body size optima driven by a complex resource distribution [16].

Given ${\beta }_m={\mathrm{\nabla }}_m\ln$ ${\overline{W}}_m+c_m$ [12], where ${\mathrm{\nabla }}_m$ is the gradient $\mathrm{\partial }/\mathrm{\partial }\overline{z}$ and c_m is sexual selection arising from some mating bias independent of the strength of natural selection, we can define ${\beta }_m$ under the twin peaks model in equation (2.1) as

$$\begin{array}{ccc}{\beta }_m& =& {\overline{W}}_m^{-1}[2\frac{({\theta }_{z,1}-\overline{z})}{({\omega }_{z,1}+{\mathrm{P}}_z)}\exp \left\{-\frac{{({\theta }_{z,1}-\overline{z})}^2}{({\omega }_{z,1}+{\mathrm{P}}_z)}\right\}\\ \hspace{0.17em}& \hspace{0.17em}& +2\frac{({\theta }_{z,2}-\overline{z})}{({\omega }_{z,2}+{\mathrm{P}}_z)}\exp \left\{-\frac{{({\theta }_{z,2}-\overline{z})}^2}{({\omega }_{z,2}+{\mathrm{P}}_z)}\right\}]+c_m\end{array}$$ 2.2

with an analogous expression for ${\beta }_f$. For simplicity and consistency with past work, I focus on the scenario of sexual selection in males only $(c_f=0,\hspace{0.17em}{c}_m\ge 0)$. Evolution of the male and female mean phenotypes, $\overline{z}$ and $\overline{y}$, depends not only on selection within each sex but also on a correlated response to selection in the other sex mediated by the cross-sex genetic correlation r_mf :

$$\begin{array}{rl}\mathrm{\Delta }\overline{z}=\hspace{0.17em}& \hspace{0.17em}\frac{1}{2}{\beta }_m{G}_m+\hspace{0.17em}\frac{1}{2}{\beta }_{f}B\\ \mathrm{\Delta }\overline{y}=\hspace{0.17em}& \hspace{0.17em}\frac{1}{2}{\beta }_f{G}_f+\hspace{0.17em}\frac{1}{2}{\beta }_{m}B\end{array}\}$$ 2.3

where G_m and G_f are the male and female genetic variances and $r_{mf}=B/\surd (G_m{G}_f)$.

(a) . Population growth

Population growth can be considered a function of mean fitness, such that maladaptation carries demographic cost. Following Lande [12], we can describe change in census population size N as

$$N_{t+1}=k\hspace{0.17em}{\overline{W}}_m{\overline{W}}_f{N}_t,$$ 2.4

where k is a constant or function defining per capita birth rates. For simplicity, I assume k as a constant corresponding to density-independent population growth. In equation (2.4), adaptation in both sexes contributes to population growth, corresponding to a biological scenario where, for example, parental care is shared across the sexes. Alternatively, in many species, male adaptation may contribute little to population growth rates, for example, in species where males provide no parental care, and we can instead define a change in census size as

$$N_{t+1}=k\hspace{0.17em}{\overline{W}}_f{N}_t,$$ 2.5

which assumes that there are always at least enough males to fertilize the population. Equations (2.4) and (2.5) represent two ends of a continuum in which male adaptation may contribute to population growth rates.

I used numerical simulations to explore how sexual selection and r_mf influence peak shifts and extinction. In order to understand how sexual dimorphism evolves from an ancestral condition of sexual monomorphism, each simulation was started with the population mean at a sexually monomorphic optimum of $\overline{z}=\overline{y}={\theta }_{z,2}={\theta }_{y,2}=-2$, with ${\theta }_{z,1}={\theta }_{y,1}=2$. In this scenario, there are three unoccupied optima, two of which are accessible deterministically under positive sexual selection and r_mf; one new optimum is along a line of male–female isometry and represents a shift from one sexually monomorphic peak to another (henceforth, ‘sexually-monomorphic’, or concordant, peak shift). A second accessible peak involves a male-only shift and so represents a ‘sexually dimorphic’ peak shift. All ω were assumed equal, and phenotypic variance was set at unity and G = P/2, assumed constant (most consistent with an infinitesimal genetic model). Starting N was set to 10 000; population growth was assumed as in equation 3 or 4, although population size was capped at 10 000 with an arbitrary extinction threshold of N = 20. The growth constant k was assumed 1.05. Thus, growth was exponential and density independent up to the upper bound. Assuming unbounded growth, changing population size, k, or genetic parameters did not change qualitative conclusions. Complete R script is provided as electronic supplementary material.

3. Results

When there is only a single optimum, sexual and natural selection jointly determine the equilibrium trait value for males, which are displaced from their peak proportional to sexual selection c_m and the strength of stabilizing selection ω (figure 1a; [12]). Female equilibrium trait values are unaffected by sexual selection in males, but their path towards their optimum is affected by r_mf (figure 1a). Thus, the Lande model is consistent with intuition [17] that sexual selection can result in the displacement of one sex from a viability optimum.

When multiple optima exist, the combination of sexual selection and r_mf determines if a peak shift occurs and which alternative optimum is reached. Two scenarios are shown in figure 1c,e, illustrating that increasing the strength of sexual selection while holding r_mf constant, and vice versa, have similar effects. Unlike the single optimum case, when multiple optima exist, female equilibrium trait values depend on sexual selection in males and r_mf; for a peak shift to occur in females, their product must be high enough for the female mean phenotype to be displaced beyond the critical value required for a peak shift. Note that a ‘monomorphic peak’ shift (figure 1c) still entails some sexual dimorphism in the traits will be observed at equilibrium (because males will be displaced from their optimum). Valley crossing carries substantial demographic costs, illustrated in electronic supplementary material, figure S1.

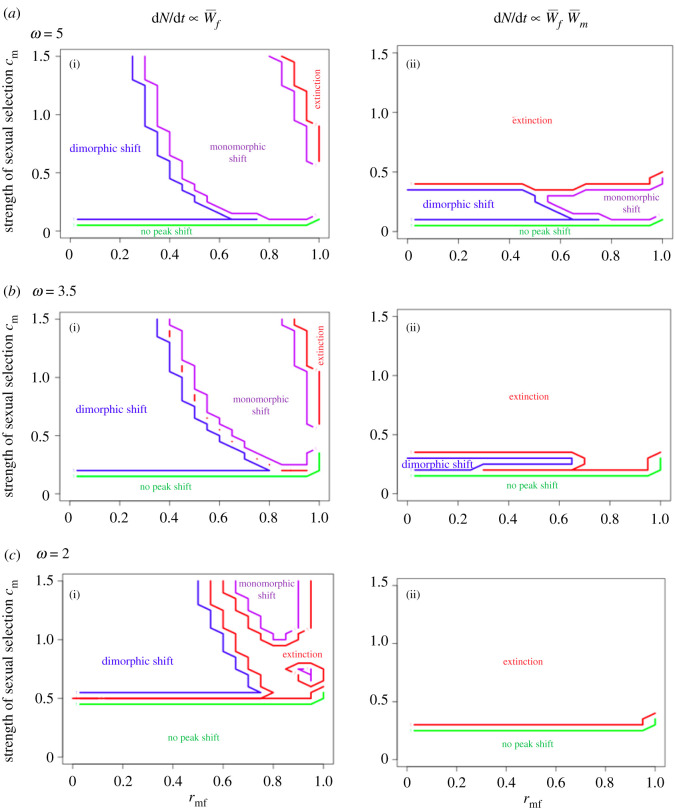
Whether a peak shift occurs for males depends only on the strength of sexual selection c_m. However, whether the shift is dimorphic (males only) or monomorphic depends also on r_mf, such that for wide ranges of moderate to strong genetic correlations, increasing the strength of sexual selection while holding r_mf constant yields first a transition from no peak shift to a dimorphic shift, and then to a monomorphic shift, and then in some cases extinction (figure 2a(i),b(i),c(i)). Increasing the strength of stabilizing selection (smaller ω) exacerbates these effects. These effects hold regardless of the demographic model assumed, although extinction is far more likely to be observed when population growth rates are a function of both male and female adaptation (figure 2a(ii),b(ii),c(ii)). These conclusions remain qualitatively unchanged when accounting for genetic drift, although the outcomes become probabilistic due to the stochastic nature of drift in the fitness valley (electronic supplementary material, figure S2).

Figure 2.

Deterministic outcomes as a function of sexual selection and r_mf. Panels show deterministic (i.e. no drift) outcomes of 1000 generations of evolution, calculated numerically, corresponding to three different strengths of stabilizing selection; (i) assume female demographic dominance, (ii) assume male and female fitness both determine population growth rate. ‘Dimorphic shift’ refers to a male-only peak shift, ‘monomorphic shift’ refers to a peak shift in both sexes (illustrated in figure 2a). No peak shift means the lineage remains in the vicinity of the ancestral optimum. Small gaps between regions represent plotting limitations of overlaid contour plots.

4. Discussion

Although the broad factors that may possibly affect peak shifts are well understood, how these factors jointly determine the probability of actual peak shifts remains a puzzle [6]. Sex-specific selection, in particular sexual selection, provides one example of the uncertainties around peak shifts. Sexual selection and genetic correlations may be key in facilitating peak shifts [12,13], yet this diversifying effect [14] is seemingly at odds with the constraining role that such a condition must also manifest [12,18]. The point of this paper is to explore how sexual selection and r_mf interact to influence the likelihood of a peak shift occurring, as well as which alternative optima are most likely to be reached. This modest revisit to classical peak shift models yields two underappreciated phenomena that have important implications for the evolution of sexual dimorphism.

First, although increasing the strength of sexual selection increases the likelihood of a peak shift, whether that peak shift results in substantial sexual dimorphism depends somewhat counterintuitively on the magnitude of sexual selection. For wide ranges of r_mf, weaker sexual selection produces a peak shift in males only; increasing sexual selection further increases the correlated response in females to the point where females also shift peaks (see also fig. 4A in Price et al. [9]). The implication is that when sexually monomorphic optima exist, strong sexual selection is expected to lead to peak shifts along a line of male–female isometry when r_mf is non-zero. Only when sexual selection is weak enough in magnitude that its interaction with r_mf fails to push females beyond the critical displacement required for a peak shift, yet strong enough to directly push males beyond their own critical displacement, will dimorphic optima be reached. This suggests that macroevolutionary patterns of sexual dimorphism may depend far more on nuances of the two-sex adaptive landscape than on the magnitude of within-population sexual selection.

Second, the demographic cost of valley crossing affects which peaks can be reached. When population growth rates depend on adaptation in both sexes, or when natural selection is strong, sex-specific peak shifts are more likely to occur successfully in the face of extinction than are concordant (monomorphic) shifts. This demographic effect is the result of higher demographic costs for crossing a valley in both sexes. When population growth rates depend only on female adaptation, valley crossing in males only carries no demographic cost, and so may be more likely to be observed. These effects are amplified under strong selection (deep valleys) and indicate that extinction-generated survivorship bias [19] may make sex-specific (dimorphic) peak shifts more likely to be observed than monomorphic shifts.

These two features of sex-specific peak shifts may explain several puzzling phenomena observed in macroevolutionary studies of sexual dimorphism. First, a large number of studies have investigated the link between proxies for the strength of within-population sexual selection and among-lineage patterns of sexual dimorphism. Often, these studies find only weak relationships between these sexual selection proxies and the magnitude of sexual dimorphism [20–23]. This finding of little correlation between the magnitude (yet some correlation between the sign; [24]) of sexual dimorphism and sexual selection proxies is consistent with the results presented here. Concomitantly, any association between r_mf and the strength of sexual selection within populations (as some data implies could be the case; [25,26]) could have important macroevolutionary consequences.

Further, when male adaptation contributes little to the population growth rate, this may lead to a male bias in macroevolutionary rate if male valleys are easier to cross without extinction. In many clades, males are observed to exhibit higher rates of body size evolution than females [27–30]. Although the opposite (higher female rates) is observed in some groups [31], it does appear to be rarer.

A major caveat is the assumption of constant genetic (co)variances. r_mf may itself evolve in response to sex-specific selection, altering the dynamics of peak shifts, although antagonistic selection can maintain r_mf under some conditions [32]. The deep-time evolutionary dynamics of r_mf remain somewhat of a puzzle and an important avenue of future work.

Evolution on real adaptive landscapes, with mixtures of peaks of varying height, varying distance from each other, will be more complex than the model presented here. Nonetheless, whether a peak shift to a dimorphic or monomorphic optimum occurs will depend in part on interactions between sexual selection and genetic correlations that may decouple the strength of sexual selection from the magnitude of resulting sexual dimorphism, and crossing two valleys at once may be demographically costly.

Data accessibility

This article has no additional data. The data are provided in the electronic supplementary material [33].

Competing interests

I declare I have no competing interests.

Funding

Funding was provided by grants from the Swedish Research Council (VR registration number 2019- 03706) and Kungl. Fysiografiska Sällskapet i Lund to S.P.D.