Twofold symmetry of c-axis resistivity in topological kagome superconductor CsV₃Sb₅ with in-plane rotating magnetic field

Abstract

In transition metal compounds, due to the interplay of charge, spin, lattice and orbital degrees of freedom, many intertwined orders exist with close energies. One of the commonly observed states is the so-called nematic electron state, which breaks the in-plane rotational symmetry. This nematic state appears in cuprates, iron-based superconductor, etc. Nematicity may coexist, affect, cooperate or compete with other orders. Here we show the anisotropic in-plane electronic state and superconductivity in a recently discovered kagome metal CsV₃Sb₅ by measuring c-axis resistivity with the in-plane rotation of magnetic field. We observe a twofold symmetry of superconductivity in the superconducting state and a unique in-plane nematic electronic state in normal state when rotating the in-plane magnetic field. Interestingly these two orders are orthogonal to each other in terms of the field direction of the minimum resistivity. Our results shed new light in understanding non-trivial physical properties of CsV₃Sb₅.

The recently discovered class of kagome metals AV₃Sb₅, where A stands for K, Rb, Cs, has been shown to host a variety of exotic phases. Here, the authors report the two-fold rotational symmetry of superconductivity and signatures of an in-plane nematic electronic state in CsV₃Sb₅ under in-plane magnetic field.

Introduction

Materials with a kagome lattice structure can host a rich variety of exotic states including spin liquid^1–3, spin density wave⁴, charge density wave (CDW)^5,6, and superconductivity^4,5,7,8. In addition, these kagome materials provide a novel platform to investigate topological electronic states^9–15. Recently, a new family of kagome metals AV₃Sb₅ (A = K, Rb, or Cs) has been discovered¹⁶, and shortly afterwards, superconductivity is reported in this system^17–19. The superconducting transition temperature (T_c) in CsV₃Sb₅ can be easily enhanced by the applied pressure^20–23. Although the superconductivity is argued to be a strong-coupling one^24–26 probably with triplet pairing²⁷, the gap symmetry of this superconductor remains controversial^{21,24–26,28–32} and requires further study. Besides the superconducting state, there is also a CDW transition in AV₃Sb₅^16–19. The CDW order has been investigated experimentally^33–36 and theoretically^37–40; it is probably driven by the nesting of saddle points near M points^18,35,39. The electronic states of CDW are also investigated on the atomic scale^{24,25,41–44}. Furthermore, the existence of Z₂ topologically non-trivial states in AV₃Sb₅ has been evidenced by the observation of symmetry-protected Dirac crossing bands^17,19,45,46, giant anomalous Hall effect^47,48, a chiral charge order⁴¹, and the possible existence of Majorana zero mode in the vortex core²⁵.

The nematic electronic state breaks the symmetry of the crystal structure in many strongly correlated electron systems⁴⁹, including cuprates^50,51, iron-based superconductors^52,53, ultra-clean quantum Hall systems⁵⁴, Sr₃Ru₂O₇⁵⁵, etc. The twofold in-plane electronic anisotropy breaks the symmetry of the underlying lattice in these materials. Superconductivity with twofold symmetry seems to be a common feature in topological superconductors. Such feature is observed by different kinds of measurements in doped Bi₂Se₃^56–61 and heterostructures constructed by Bi₂Te₃ and high-temperature superconductors^62,63. The nematic superconductivity is explained theoretically as a consequence of superconducting order parameter with odd parity derived from the spin−orbital coupling and the multi-orbital effect in these materials^64,65.

In this work, by measuring the c-axis resistivity (ρ_c) using a Corbino-shape-like electrode configuration with in-plane rotating magnetic field, we observe a twofold rotational symmetry of angular dependent ρ_c(θ) both in the superconducting state and the normal state of the topological kagome metal CsV₃Sb₅. The field direction for the minimum resistance in the superconducting state is along one pair of crystalline axes of the lattice (a-axis), which suggests a superconducting gap maximum in this direction. By applying a very strong in-plane magnetic field, we also observe a twofold rotational symmetry of ρ_c(θ) curves in the normal state but with an orthogonal direction of the minimum resistivity comparing to that in the superconducting state. The twofold rotational symmetry of ρ_c(θ) curves may be related to the in-plane nematic electron state with the assistance of strong magnetic fields. Furthermore, we find a six-fold oscillation component in the ρ_c(θ) curve, which is due to the six-fold symmetry of the lattice. These findings contribute to a better understanding of the electronic state and the superconductivity in this topological kagome metal.

Results

Experimental configuration and superconducting characterization

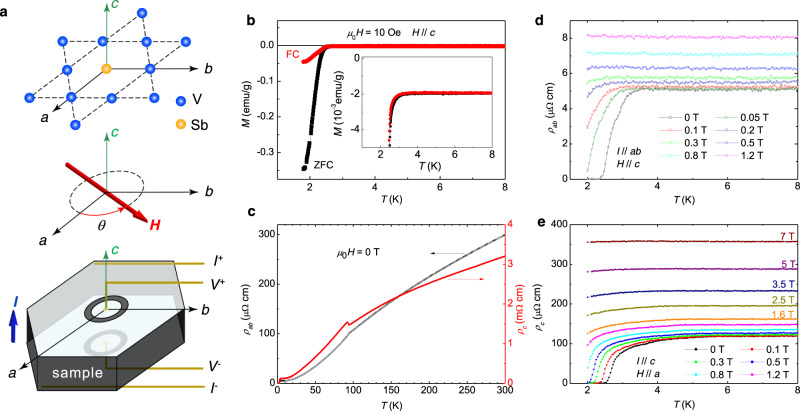
In order to detect the possible in-plane electronic anisotropy of the topological kagome metal CsV₃Sb₅, we measure the c-axis resistivity by using a Corbino-shape-like electrode configuration (Fig. 1a and Supplementary Fig. 3). The advantage of using this configuration is that a major part of the current is flowing along the c-axis, and thus is always perpendicular to the in-plane rotating magnetic field. This would avoid the undesired angle dependence of the in-plane resistivity due to the flux flow if the current were applied along ab-plane. Thus, the observed angle-dependent variation of the c-axis resistivity with the in-plane rotating field can be safely attributed to some anisotropic electronic property in the material. The superconducting transition of the CsV₃Sb₅ single crystal is characterized by magnetization measurements (Fig. 1b), and the onset transition temperature is about 3.5 K determined from the enlarged view shown in the inset of Fig. 1b.

Fig. 1. Measurement configuration of c-axis resistivity and characterization on superconductivity.

a The c-axis resistivity of CsV₃Sb₅ is measured by using a Corbino-shape-like electrode configuration. The electric current is applied mainly along c-axis of the single crystal, and the magnetic field is applied parallel to and rotated in the ab-plane. Single crystals usually have naturally formed edges with the angle of about 120° for neighboured edges, and these edges are along directions of crystallographic axes (see Supplementary Note 2). b Temperature-dependent magnetization measured with the zero-field-cooling (ZFC) and the field-cooling (FC) modes. c Temperature dependence of c-axis and in-plane resistivity which are measured with different configurations (Supplementary Fig. 3). Temperature dependence of in-plane (d) and c-axis (e) resistivity measured near the superconducting transition temperature at different magnetic fields.

Figure 1c shows the temperature dependence of the in-plane (ρ_ab) and the c-axis resistivity. The normal-state resistivity shows a large anisotropy of α = ρ_c/ρ_ab = 23 at 8 K, which suggests considerable two-dimensionality of the material. The CDW transition can be clearly seen at about 95 K as an anomaly of resistivity. However, there is an obvious step-like increase in the ρ_c(T) curve with decreasing temperature before the drop of resistivity. This feature is different from that measured by the in-plane resistivity which only exhibits a monotonic drop crossing the CDW transition. This difference has also been observed in the sister compound of RbV₃Sb₅¹⁹. Figure 1d, e shows the temperature dependence of in-plane and c-axis resistivity, respectively; they are measured near the superconducting transition under different magnetic fields. The superconducting feature starts at about 3.5 K in ρ_ab(T) at zero applied field, and the superconductivity can be easily killed by a field of about 0.8 T at 2 K. All the results of ρ_ab(T) are similar to those in previous reports^21,29. In the ρ_c(T) curve measured at 0 T, the detected zero-resistance temperature is the same as that obtained in the ρ_ab(T) curve. However, a superconducting-fluctuation-like behaviour can be seen obviously in the ρ_c(T) curve at temperatures up to about 5.5 K. Furthermore, the resistivity drop at 2 K can be even seen under 7 T. This contrasting behaviour between ρ_ab(T) and ρ_c(T) near T_c is quite interesting and deserves further study.

Angular dependent of c-axis resistivity

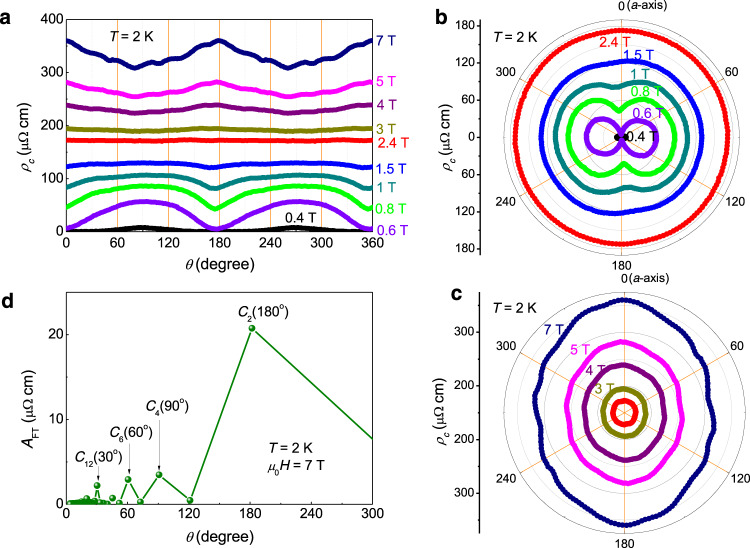
During the in-plane rotation of the magnetic field, the initial field direction (θ = 0°) is set to be parallel to one of the sample edges (Fig. 1a), and it is found that this direction is just along one pair of in-plane crystallographic axes of the single crystal determined by Laue diffraction (see Supplementary Note 2). The angle-dependent resistivity at 2 K and different magnetic fields (Fig. 2a) shows obvious twofold symmetry. At a field below 2.4 T, ρ_c(θ) curves show local minima near θ = 0° or 180° which is in the direction of one of the principal axes, namely a-axis. Since the resistivity minimum touches zero in the ρ_c(θ) curve measured at 0.4 T, the twofold symmetry of ρ_c(θ) curves is supposed to be induced by the anisotropic properties of the superconducting state. Here, on one particular curve of angle-dependent resistivity, the minimum resistivity reflects a relatively larger upper critical field (μ₀H_c2). A simple consideration based on the Ginzburg−Landau theory and the Pippard definition of the coherence length ξ ≈ ℏv_F⁄πΔ tells that ${\mu }_0{H}_{\mathrm{c}2}\propto {\mathrm{\Delta }}^2/v_{\mathrm{F}}^2$ with ℏ the reduced Planck constant, ∆ the superconducting gap, and v_F the Fermi velocity⁶⁰. Therefore, the gap maximum may be along a-axis, which suggests the possible existence of a twofold symmetry of superconductivity in CsV₃Sb₅.

Fig. 2. Twofold symmetry of angular dependent c-axis resistivity under in-plane magnetic field.

a Angular dependence of c-axis resistivity measured at different in-plane magnetic fields. The featureless ρ_c(θ) curve measured at 2.4 T separates two sets of ρ_c(θ) curves holding phase-reversed oscillations with twofold symmetry. Angular dependent c-axis resistivity plotted in polar coordinate measured with rotating in-plane magnetic field of the magnitude (b) below and (c) above 2.4 T. Local minima in curves measured at μ₀H < 2.4 T change to local maxima in curves at μ₀H > 2.4 T in the direction along a-axis. In addition, ρ_c(θ) curves measured at μ₀H > 2.4 T show extra oscillation of six-fold symmetry besides the major twofold signal. d FT result to the ρ_c(θ) curve measured at 7 T.

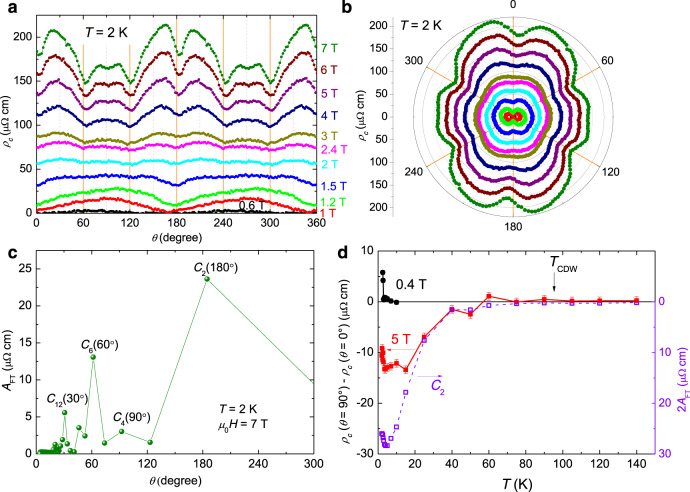
In contrast, when the magnetic field exceeds 2.4 T, the field orientation corresponding to the minimum resistivity becomes roughly orthogonal to that of superconducting state (below 2.4 T), i.e., ρ_c(θ) near the angles for the minimum of resistivity below 2.4 T now shows local maximum above 2.4 T instead (Fig. 2a). This contrasting behaviour can be easily seen in the polar illustrations in Fig. 2b, c for the curves measured at fields below and above 2.4 T, respectively. Although the ρ_c(T) curve shows superconducting-fluctuation-like behaviour at fields stronger than 2.5 T at 2 K (Fig. 1e), the suppression of resistivity due to this effect is very weak when compared to the magnetoresistance. Thus the twofold symmetry of ρ_c(θ) curves at μ₀H > 2.4 T should be dominated by normal-state properties. In addition, some extra oscillations can be seen in ρ_c(θ) curves measured at very high field (Fig. 2a, c), see the curve measured at 7 T for example. Figure 2d shows the result of the Fourier transformation (FT) to the ρ_c(θ) curve measured at 7 T, and the FT amplitude (A_FT) peak at 180° (with the C₂ symmetry) combined with that at 90° (C₄) suggests the nematic electronic state, and the peaks at 60° (C₆) and 30° (C₁₂) suggest other six-fold symmetries. Actually, the ρ_c(θ) oscillates by every 30°, which is strongly correlated with the arrangement of vanadium atoms in the lattice¹⁷ (detailed analysis see Supplementary Note 3).

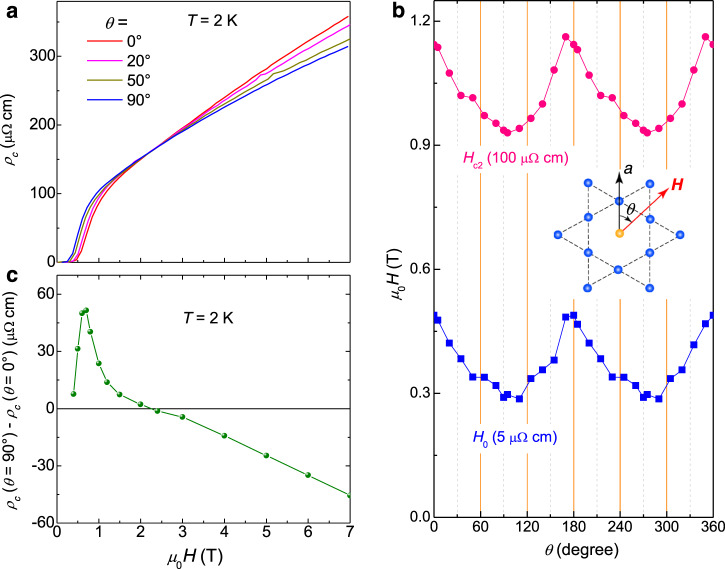
The two kinds of ρ_c(θ) curves below and above 2.4 T are roughly orthogonal to each other in terms of the field direction of the extremum resistivity. This tendency can be clearly seen from the field-driven evolution of the angle-dependent ρ_c(μ₀H) curves (Fig. 3a). By using certain criterions, namely ρ_c(μ₀H) = 100 and 5 μΩ·cm, we determined the angle-dependent upper critical field μ₀H_c2 and zero-resistance field μ₀H₀ (Fig. 3b). Now the peak position corresponds to the minimum resistivity in the superconducting state. The in-plane anisotropy of μ₀H_c2 is about 1.2−1.3, which is consistent with the value determined from temperature-dependent resistivity measurements (Supplementary Fig. 7d). The difference between the resistivity measured along two typical directions (θ = 0° and 90°) at 2 K is plotted in Fig. 3c with the variation of field. One can see that there is a clear sign change at the field of about 2.4 T. However, when T = 10 K, we see no cross of ρ_c(μ₀H) curves (Supplementary Fig. 7e). Now if we take the characteristic fields μ₀H^* with the criterions of ρ_c(μ₀H^*) = 300 or 320 μΩ·cm, we see again the twofold feature of μ₀H^* (Supplementary Fig. 7f), but now μ₀H^*(θ) shows oscillations with opposite phase as that of μ₀H_c2(θ).

Fig. 3. Magnetic-field induced phase reverse of ρ_c(θ) curves with twofold symmetry.

a Magnetic field dependence of c-axis resistivity measured with different angles between field and the a-axis at T = 2 K. b Angular dependent upper critical field and zero-resistance field (μ₀H₀) by using different criterions of c-axis resistivity. c Field dependence of the c-axis-resistivity difference between θ = 0° and 90°. The resistivity difference changes its sign at a field of about 2.4 T.

Temperature evolution of twofold feature

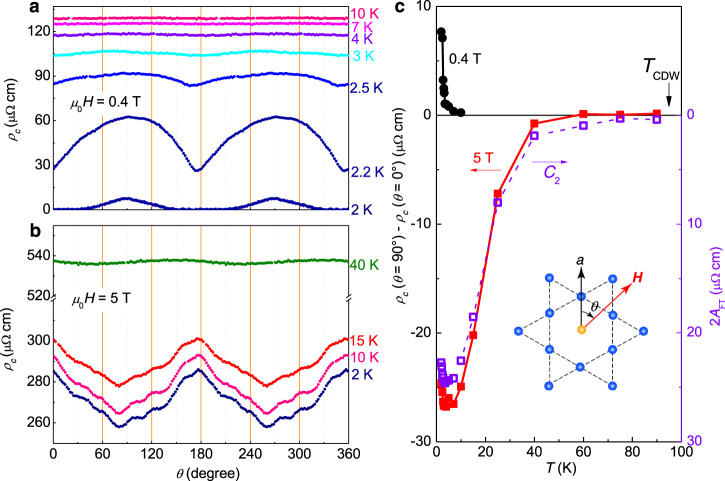
Figure 4a, b shows the temperature evolution of ρ_c(θ) curves at two different fields of 0.4 and 5 T, respectively. Obviously, the twofold feature of the ρ_c(θ) curve at 0.4 T weakens quickly with the increase of temperature. When T reaches about 4 K, this oscillation is greatly diminished. This indicates that the twofold symmetry of ρ_c(θ) is just induced by the flux flow dissipation in the superconducting state. However, at roughly the same angles for the minimum resistivity in the superconducting state, the resistivity peaks up when μ₀H = 5 T (Fig. 4b). Figure 4c shows the difference of ρ_c(θ = 90°) − ρ_c(θ = 0°) as an indicator to show the nematicity. Here the filled circles and squares represent the data for 0.4 and 5 T, respectively. With the increase of temperature, the twofold anisotropy of ρ_c(θ) curves measured at 0.4 T quickly vanishes around T_c, but that measured at 5 T changes much gently with the increase of temperature. We carry out the FT to ρ_c(θ) curves measured at different temperatures under 5 T, and the result is shown in Supplementary Fig. 8a. The FT amplitudes for the 180° peak are also plotted in Fig. 4c as another indicator to show the nematicity. One can see clearly that the twofold symmetry at 5 T progressively weakens with the increase of temperature and disappears near the CDW transition temperature (T_CDW) of about 95 K. In Fig. 5, we show the control experimental results carried out in another sample. One can also see the twofold symmetry of ρ_c(θ). The temperature evolution of the twofold feature is almost the same as the results shown in Fig. 4. The observations are very similar in these two samples.

Fig. 4. Temperature evolution of the nematicity of c-axis resistivity.

Angular dependent c-axis resistivity measured at different temperatures under a magnetic field of a 0.4 and b 5 T. c Temperature dependence of the nematicity. The solid symbols represent the c-axis resistivity difference between θ = 0° and 90° at 0.4 and 5 T, while the open symbols show twice the amplitude of the 180° (or C₂) peak (2A_FT,C2) from FT results (Supplementary Fig. 8a) to ρ_c(θ) curves measured at different temperatures under 5 T. One can see that the latter is more sensitive to show the C₂ symmetry which seems ending near T_CDW.

Fig. 5. Control experiment of ρ_c(θ) curves with twofold symmetry of in another sample.

a,b Angular dependence of c-axis resistivity measured at different in-plane magnetic field shown by a rectangular and a polar coordinate, respectively. The inverse of the local extrema can be observed when the magnetic field crosses about 2 T. c FT result to the ρ_c(θ) curve measured at 7 T. d Temperature dependence of the nematicity. The solid symbols are derived from the c-axis resistivity difference between θ = 0° and 90° at 0.4 and 5 T, while the open symbols show twice the amplitude of the 180° (or C₂) peak (2A_FT,C2) from FT results (Supplementary Fig. 8c) to ρ_c(θ) curves measured at different temperatures under 5 T. The error bars in the figure are determined by the noise in the resistivity measurement and the error transfer theory. The relatively small thickness and large surface area make the resistance very small with a relatively large noise.

Discussion

Now we discuss the origin of the twofold symmetry observed in ρ_c(θ) curves. One may argue that this can be induced by the misalignment between the current direction and c-axis or that between the field and the ab-plane. Indeed, although we cannot avoid this misalignment, however, this is unlikely for our results because of the following reasons. Firstly, for the ρ_c(θ) curve measured at 7 T and 2 K, ρ_c(θ = 90°)/ρ_c(θ = 0°) = 0.85 suggests a big difference. If the anisotropic magnetoresistance were induced by the misalignment between the magnetic field and the ab-plane, and the magnetoresistance was only induced by the ab-plane component of field which is perpendicular to the current, this anisotropy would correspond to a misalignment angle of about 32° (cos 32° = 0.85) between the field and the ab-plane. In the experiment, we can guarantee that the misalignment angle of the ab-plane to the field is less than 3°, thus it is impossible for such a big angle misalignment. Secondly, a relatively large normal-state resistance at 0° and 180° in the normal state would mean a large component of field perpendicular to the current (since we have a positive magnetoresistance), which would suppress superconductivity more severely and also induce a larger flux-flow resistivity at the same angle. But this contradicts the observations. Thirdly, we have repeated the experiments in the same sample and in the re-cleaved sample (Supplementary Fig. 9), and we have also carried out the experiments in another sample (Fig. 5). These control experiments all show similar behaviours, indicating a high reproducibility. Although the directions of superconducting gap maximum are all along one pair of crystalline axes, these two directions have an intersect angle of about 60° (Supplementary Fig. 3) for the two samples which are mounted on the same sample holder. This excludes the possible error from the experimental setup.

In iron-based superconductors, the normal-state nematic electronic order was detected by measuring in-plane resistance with a detwinning setup at zero field⁵². However, this kind of in-plane resistive measurements for checking the C₂ symmetry cannot be easily done in the present CsV₃Sb₅ system. The reason is that the crystal structure has a fourfold symmetry in iron-based superconductors, and the sample shape is usually rectangular. Therefore, it is easy to measure the resistivity in a- or b-axis direction by using the standard four-probe technique on one sample, and the fourfold symmetry breaking can be easily obtained by the analysis of resistivity. However, in CsV₃Sb₅, the crystal structure has a six-fold symmetry, and the shape of the sample is usually hexagonal, with some corners of enclosed angles of about 120°. Hence, it is difficult to measure the exact resistivity along three crystalline directions in one sample mounted in a detwinning setup. Here, we detect the in-plane symmetry of the sample by measuring the c-axis resistivity with a rotating in-plane field, and the twofold symmetry in ρ_c(θ) curves may not reflect directly the nematic electron state in the ab plane. However, the twofold symmetry nature of ρ_c(θ) curves in the normal state only show up when the magnetic field is higher than 2.4 T at 2 K. In the presence of a magnetic field, the mobile electrons will possess a circular momentum in the plane perpendicular to the field direction. Then the c-axis resistivity measured in this configuration should contain the contribution of the in-plane electronic states or the in-plane mobility component along the direction perpendicular to the magnetic field⁶⁶. For example, if the in-plane Fermi velocity has a two-fold symmetry, that will induce a twofold symmetric feature of the in-plane scattering rate, which can be detected by the c-axis resistivity with an in-plane magnetic field.

The twofold symmetry of ρ_c(θ) curves measured at high fields may suggest the in-plane nematic electronic state in the material. Based on the FT results obtained at high temperatures across T_CDW (Supplementary Fig. 8d, f), one can see that the amplitude of the 180° (or C₂) peak is reduced to the background signal when the temperature is increased to that near T_CDW, and the amplitude is always in the noise level at high temperatures above T_CDW. Consequently, the first scenario comes to our mind is that it may be related to the CDW state. We have been aware that there is an additional 4a₀ unidirectional charge order besides the tri-directional charge order with a 2a₀ period in this material^24,42,44. This unidirectional charge order pattern corresponds to CDW stripes along a-axis, which may be explained based on the picture of topological CDW⁶⁷. These CDW stripes induce a twofold symmetry of in-plane electronic properties, which has been detected by our c-axis resistivity when the magnetic field is rotated within the V−Sb planes. In addition, for the tri-directional charge order with a 2a₀ period, the intensities of three sets of peaks have pronounced intensity anisotropy^24,41–44, which exactly shows the feature as the nematic CDW state. Besides, theoretically, it was predicted that a chiral flux phase may exist and break the time-reversal symmetry in the c-axis⁶⁸, but this model cannot predict a symmetry breaking in the ab plane. Although the six-fold rotational symmetry is supposed to be preserved in a single V−Sb kagome layer, the stacking of these layers along the c-axis may induce the C₆ symmetry breaking. The three-dimensional (3D) 2a₀ × 2a₀ × 2c₀ CDW order^25,34 or 2a₀ × 2a₀ × 4c₀ one³⁶ was identified by different kinds of experiments. In this 3D CDW configuration, there is a π phase shift between neighboured V−Sb kagome layers, i.e., a misalignment of a₀ emerges along one of three in-plane crystalline axes for the same characteristic in-plane CDW patterns in neighboured layers. Therefore, the phase shift in addition to the inter-layer coupling between the neighboured layers lower the six-fold symmetry to a twofold one^39,40. This picture does not require any additional type of order, and it can naturally explain the twofold symmetry of ρ_c(θ) curves with an in-plane rotating magnetic field in the normal state. A simple understanding is that the 3D CDW phase affects the in-plane mobility or the scattering rate along and perpendicular to the symmetry breaking axis. It should be noted that the ρ_c(θ,Β) curve at a fixed angle θ should be an even function of the magnetic field⁶⁶, then the calculated resistivity difference ρ_c(θ = 90°) − ρ_c(θ = 0°) is likely to be proportional to B² in the low field region. This fact is demonstrated in Supplementary Fig. 10, the nematicity effect appears with the presence of a small field and it is enlarged by a high magnetic field.

The twofold feature of ρ_c(θ) curve in the superconducting state may be intimately related to the feature in the normal state. A simple picture is that the CDW phase with twofold symmetry would gap out the density of states at the Fermi level leading to a truncated Fermi surface with twofold symmetry, and this leads to a twofold symmetry of the superconducting gap or H_c2. By now the existence of the gap anisotropy is still hard to be detected directly from experiments²⁵. Alternatively, concerning the fact that the nematic electronic state is observed with the help of a strong in-plane magnetic field with the absence of superconductivity, while the twofold symmetry of H_c2(θ) is observed in superconducting transition region with very small field: these two kinds of twofold symmetries may have different origins. We have noticed that the material is supposed or partly proved to be a topological superconductor^{17,25,45,46,48}, thus the superconductivity with twofold symmetry may originate from the superconducting order parameter with odd parity. This scenario has recently been well proved^{56–61,64,65} in topological superconductors Cu_xBi₂Se₃, Sr_xBi₂Se₃, or Nb_xBi₂Se₃. Beside these two possibilities, a 4a₀/3 bidirectional pair density wave²⁴ or the spin-triplet superconductivity²⁷ may be extra possible reasons of the twofold symmetry of ρ_c(θ) curves at small magnetic field in the superconducting state, thus quantitative analyses based on these models are highly desired.

As mentioned above, the natural explanation for the twofold symmetry of ρ_c(θ) curves is the 3D CDW configuration, and the π phase shift between neighboured V−Sb kagome layers emerges along one of three in-plane crystalline axes. Based on this picture, there may be three kinds of nematic domains, and the angle between every two kinds of domains is 60°. The twinned C₂ electronic state may exist in the samples, and the existence of multiple nematic domains may be supported by the angle deviation between twofold and six-fold components derived from ρ_c(θ) curves (Supplementary Fig. 6). If there were quite a lot of randomly distributed domains, the twofold symmetric ρ_c(θ) curves would not be observed. However, we do observe the twofold nature of ρ_c(θ) curves both in superconducting and normal states. In addition, these two kinds of orders are orthogonal to each other in terms of the field direction of the minimum resistivity. Nevertheless, one kind of the nematic domain dominates the electronic transport as seen from the ρ_c(θ) curves. It should be noted that the nematic superconductivity is also observed in some topological superconductors by different kinds of experiments without the detwinning operation^56–60, and the multi-domain effect cannot induce the equal concentrations of different domains. The nematic electronic state is also observed in ultra-clean quantum Hall systems⁵⁴ and Sr₃Ru₂O₇⁵⁵ without detwinning. In our point of view, the situation in CsV₃Sb₅ is similar to that in the materials referenced above. Based on our experimental data, we argue that the predominant contribution of one kind of domain may be fixed by the subtle stress in the sample due to the different thermal shrinkage coefficients of the sample and the grease used to attach the sample on the sample holder. With the increase of temperature, although the twofold symmetry of ρ_c(θ) curves remains, the phase of the twofold component changes in all the samples (Supplementary Figs. 11−13). This means that the contribution from other domains increases. That may be the reason why the anisotropy disappears at about 60 K if we use ρ_c(θ = 90°) − ρ_c(θ = 0°) as the indicator of the nematicity. However, the twofold symmetry feature does disappear near T_CDW as seen from the measured ρ_c(θ) curves (Supplementary Figs. 11−13) or the temperature-dependent FT amplitude of the C₂ peak (Figs. 4c, 5d, and Supplementary Fig. 13l), which confirms a very close relationship between the twofold symmetry of ρ_c(θ) curves and 3D CDW transition.

In summary, we have observed twofold symmetry of superconductivity and the anti-phase oscillation of c-axis resistivity in the normal state with respect to the in-plane magnetic field, and these observations will shed new light in the study of this fascinating kagome and topological material.

Methods

Single-crystal growth and preparation

High-quality single crystals of CsV₃Sb₅ were grown by a self-flux method with Cs−Sb binary eutectic mixture as the flux^16,44. High-purity elements of Cs, V, and Sb were mixed in a molar ratio of 7:3:14 in an alumina crucible, and then the alumina crucible was sealed inside an evacuated quartz tube. The loaded tube was put into a muffle furnace and heated slowly to 1000 °C, and this temperature was kept for 20 h. After that, the tube was cooled down to 200 °C at a rate of 3 °C/h. Flake-like single-crystalline samples have the c-axis orientation. The analysis of the structure and the stoichiometry to CsV₃Sb₅ single crystals can be seen in Supplementary Note 1. The crystal orientation was determined by the Laue X-ray crystal alignment system (Photonic Science Ltd.). Some single crystals have naturally formed edges with the angle of about 120° for neighboured edges, and these edges are proved to be crystallographic axes by Laue diffraction measurements (see Supplementary Note 2).

Resistivity measurements

Resistance measurements were carried out in a physical property measurement system (PPMS, Quantum Design). Samples were cleaved along the Van der Waals layers, and some edge(s) were cut in order to form the hexagon structure by following the naturally formed edges (see Supplementary Fig. 3). The c-axis resistance was measured by the four-electrode method with the Corbino-shape-like configuration⁶⁰. To eliminate the influence of the slight Hall signals on the raw data of angular dependence of resistivity, the resistivity taken at every angle has been averaged with positive and negative magnetic fields. The in-plane resistivity ρ_ab was measured by the standard four-electrode method with remade electrodes on the same sample, and the current was in the ab-plane of the sample.

Author contributions

Y.L., Z.W, and Y.Y. grew single crystals and characterized the structure and stoichiometry of samples. Q.L. and W.X. measured and analyzed the crystal orientation. Y.X., H.Y., and H.-H.W. carried out resistivity measurements. H.Y., H.-H. W., Y.X., and Q.L. analyzed the data and wrote the manuscript which was proof-read and agreed by all authors.

Data availability

All the data that support the findings of this paper are available from the corresponding authors upon reasonable request.

Competing interests

The authors declare no competing interests.

Supplementary information

The online version contains supplementary material available at 10.1038/s41467-021-27084-z.