Polymorph exploration of bismuth stannate using first-principles phonon mode mapping

Abstract

Accurately modelling polymorphism in crystalline solids remains a key challenge in computational chemistry. In this work, we apply a theoretically-rigorous phonon mode-mapping approach to understand the polymorphism in the ternary metal oxide Bi₂Sn₂O₇. Starting from the high-temperature cubic pyrochlore aristotype, we systematically explore the structural potential-energy surface and recover the two known low-temperature phases alongside three new metastable phases, together with the transition pathways connecting them. This first-principles lattice-dynamics method is completely general and provides a practical means to identify and characterise the stable polymorphs and phase transitions in materials with complex crystal structures.

Using a phonon mode-mapping approach, we recover the known experimental phases of the ternary oxide Bi₂Sn₂O₇ and identify three new metastable phases, highlighting the utility of the method for polymorph prediction on many other complex materials.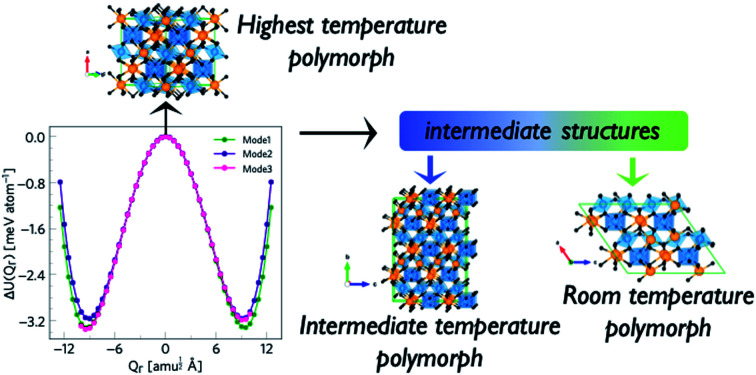

1. Introduction

Predicting polymorphism using first-principles methods is a fundamental challenge in materials discovery.^1–3 The ability to search for and identify polymorphs computationally both helps avoid experimental complications from poor-quality samples and low-resolution characterisation, and also accelerates the development of functional materials.

Group-theoretical methods have successfully been used to characterise the lower-symmetry phases derived by distorting the archetypal cubic ABX₃/A₂BB′X₆ perovskite phase by tilting and distorting the BX₆/B′X₆ octahedra.^4–8 Predicting polymorphs in complex multi-component systems presents a major challenge, as the number of degrees of freedom often makes ab initio exploration prohibitively expensive.

In this study, we remap the problem of identifying polymorphs of the ternary metal oxide Bi₂Sn₂O₇ from a search over atom positions in real space to collective atomic displacements in phonon space.⁹ This results in an efficient and unbiased computational approach to predicting the polymorphs of the ternary oxide Bi₂Sn₂O₇, which is generally applicable to other complex systems where conventional approaches such as random searching or genetic algorithms are not practical.^10–14

1.1. Crystal chemistry of bismuth stannate

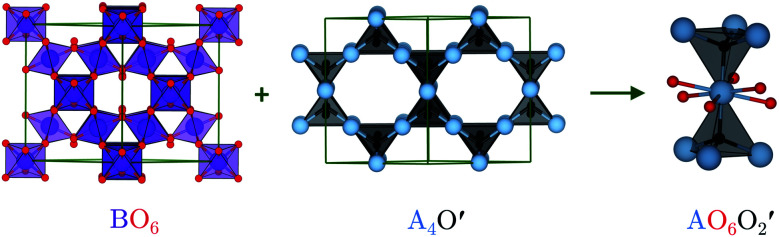
The ideal A₂B₂O₆O′ pyrochlore structure in the Fd3̄m space group has eight formula units in the unit cell. The four unique atomic sites, viz. the A-site cation (+2 or +3), B-site cation (+4 or +5), and two inequivalent oxygen sites (O and O′), are present at the 16c, 16d, 48f and 8a Wyckoff positions, respectively.¹⁵ The inequivalent O sites can be understood as two interpenetrating oxide sublattices.¹⁶ The B-site cation forms corner-sharing BO₆ octahedra, while the A-site cation adopts an anti-cristobalite geometry based on corner-sharing A₄O′ tetrahedra with linear O′–A–O′ bonds. The interpenetration of the oxide sublattices results in distorted hexagonal bipyramidal coordination at the A-site, with six longer equatorial A–O bonds and two shorter axial A–O′ bonds (Fig. 1).

Fig. 1. Pyrochlore structure showing the two oxide sublattices forming a corner-sharing network of BO₆ octahedra and an anti-cristobalite network of corner-sharing A₄O′ tetrahedra. Interpenetration of the two networks results in a bipyramidal hexagonal A-site coordination environment with 6 equatorial O and two axial O′. The atoms are coloured as follows: A-site – blue, B-site – purple, O – red, and O′ – black. The images were generated using VESTA.¹⁷.

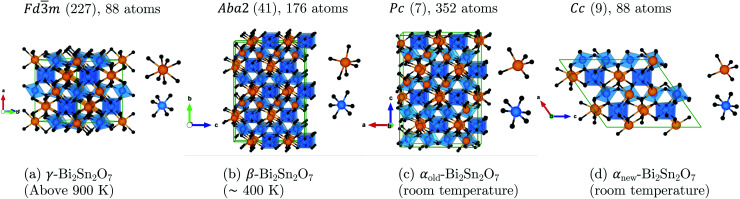
Bi₂Sn₂O₇ is unique in undergoing symmetry-lowering phase transitions on cooling and has three well-characterised polymorphs: the high-temperature cubic γ phase undergoes a second-order phase transition to β-Bi₂Sn₂O₇ above 900 K, followed by a first-order phase transition to α-Bi₂Sn₂O₇ around 390 K.¹⁸ γ-Bi₂Sn₂O₇ possesses the standard cubic pyrochlore structure with the Bi³⁺ cations displaced from the ideal crystallographic sites.¹⁹ While the structure of γ-Bi₂Sn₂O₇ is undisputed, characterising the structures of β- and α-Bi₂Sn₂O₇ has been a significant challenge.

The first structural model for α-Bi₂Sn₂O₇ was a non-centrosymmetric monoclinic P1c1 structure with 352 atoms per cell²⁰ (α_old). Three assumptions were used to solve the structure: (1) that β-Bi₂Sn₂O₇ has a face-centred cubic structure, (2) that β- and α-Bi₂Sn₂O₇ are second-harmonic generation active; and (3) that the three phases have group–subgroup relations.¹⁸

New insight was obtained very recently from high-resolution X-ray and neutron powder diffraction.¹⁶ Though the neutron data showed β to be metrically cubic, small peak splittings in the X-ray data indicated a lower-symmetry structure. An exhaustive symmetry-adapted distortion mode approach was used to enumerate all the candidate structures between the high-symmetry γ parent and a base P1 child structure using ISODISTORT.^21,22 All the candidate structures were then tested against room-temperature and ∼470 K X-ray and neutron data, based on which an orthorhombic Aba2 structure with 176 atoms in the conventional cell was suggested for β-Bi₂Sn₂O₇ together with a simplified Cc structure with 88 atoms per cell for α-Bi₂Sn₂O₇ (α_new). The four structures, viz. γ, β, α_old and α_new, are shown in Fig. 2.

Fig. 2. Unit cells of (a) the cubic pyrochlore γ-Bi₂Sn₂O₇ structure with statistically averaged Bi³⁺ displacements and the distorted (b) β, (c) α_old, and (d) α_new structures. All four structures are aligned along the SnO₆ octahedra to show the increasingly-irregular Bi chains as the temperature decreases. The insets show the cation coordination in each structure. The atoms are coloured as follows: Bi – orange, Sn – blue, O and O′ – black. These images were generated using VESTA.¹⁷.

2. Results and discussion

2.1. Phonon mode mapping procedure

Starting from the high-temperature γ structure, we employ an ab initio approach to systematically identify the energy minima on the structural potential-energy surface (PES) of Bi₂Sn₂O₇.

The harmonic phonon dispersion of γ-Bi₂Sn₂O₇ is evaluated using the supercell finite-displacement method^23,24 with the Phonopy package.²⁵ Imaginary harmonic modes in the dispersion indicate dynamical instabilities in the form of collective atomic displacements that lower the energy. We use the ModeMap code²⁶ to map the energy as a function of the normal-mode coordinate Q (distortion amplitude) along the imaginary-mode eigenvectors and to locate the energy minimum along the mode. This structure is then relaxed and a subsequent phonon calculation performed to check for additional imaginary modes. Repeating this procedure iteratively across all the imaginary modes in the parent γ and child structure(s) until each branch terminates in a dynamically-stable structure with no imaginary modes allows the sequence of intermediate structures connecting the γ and low-temperature α-phases to be systematically enumerated while also identifying other (meta-)stable polymorphs.

We use plane-wave density-functional theory with the PBEsol functional for the force calculations, as PBEsol has been shown to give accurate structures and phonon frequencies.^26–30 Full details of the computational modelling and mode-mapping procedure are given in the ESI.†

2.2. Distorting the cubic aristotype

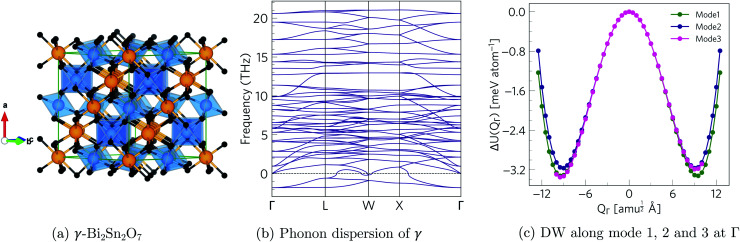
The phonon dispersion of γ-Bi₂Sn₂O₇ shows imaginary modes at four phonon wavevectors, viz. a triply-degenerate mode at Γ, doubly-degenerate modes at W and X, and single- and a doubly-degenerate modes at L (Fig. 3). The wavevector defines the periodicity of the distortion, and the q_Γ = (0, 0, 0) wavevector is commensurate with the unit cell and the distortions therefore do not change the cell volume. We therefore began our exploration by mapping the three Γ-point modes to obtain the typical double-well potential-energy curves shown in Fig. 3. The three imaginary modes lead to minima 3.17–3.35 meV per atom lower in energy than γ-Bi₂Sn₂O₇.

Fig. 3. (a) Crystal structure of γ-Bi₂Sn₂O₇. (b) Phonon dispersion of γ-Bi₂Sn₂O₇ showing imaginary frequencies at the Γ, L, W and X phonon wavevectors. (c) Double-well (DW) potential-energy surface along the three Γ-point imaginary modes.

After relaxation we obtain an orthorhombic Ima2 structure with 44 atoms per cell. The dispersion of this structure has imaginary modes at four wavevectors, viz. Γ, R, X and T. Mapping the Γ-point instability leads to a 44-atom monoclinic Cc structure with a single imaginary mode at 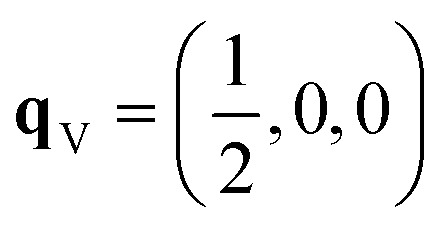(Fig. S3 and S4†). For this mode, a complete distortion period requires a 2 × 1 × 1 supercell, and mapping yields the 88-atom Cc α_new structure.¹⁶ (Calculated: a = 13.108 Å, b = 7.559 Å, c = 13.115 Å and β = 109.6°; measured:¹⁶a = 13.155 Å, b = 7.541 Å, c = 13.146 Å and β = 110.04°.) Our method therefore yields the room-temperature α_new polymorph from the high-temperature γ phase in three mode-mapping steps. The phonon dispersion of α_new has no imaginary modes, indicating it to be a dynamically-stable energy minimum.

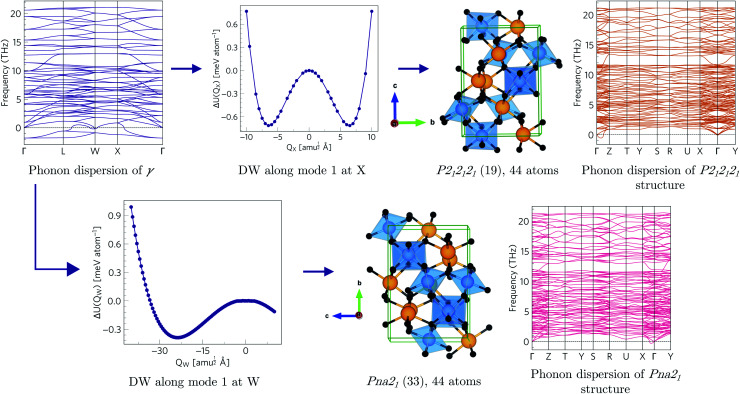
Since each of the instabilities in γ-Bi₂Sn₂O₇ represents different possible transition pathways, we mapped all the independent imaginary modes to try and locate the β phase or other stable polymorphs. The instabilities at X and W were mapped in 2 × 1 × 2 and 2 × 4 × 4 supercells, respectively, commensurate with the 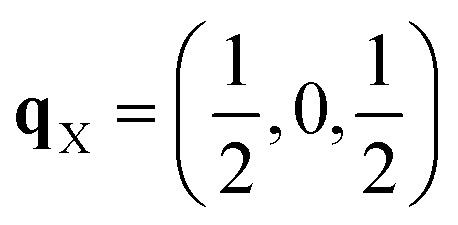and 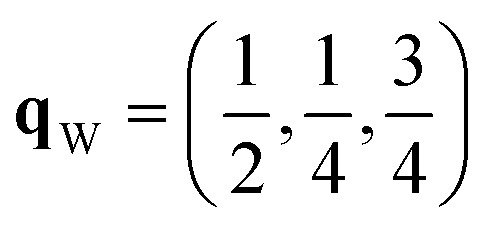wavevectors (Fig. 4). The instability at X leads in a single step to a dynamically-stable P2₁2₁2₁ structure with 44 atoms per cell via a double-well with a depth of ∼0.714 meV per atom. The W-point instability leads to a dynamically-stable Pna2₁ structure via a double-well potential with a depth of ∼0.388 meV per atom, again with 44 atoms per cell. Neither polymorph has yet been identified experimentally, and we refer to the P2₁2₁2₁ and Pna2₁ structures as δ-Bi₂Sn₂O₇ and ε-Bi₂Sn₂O₇ respectively. The δ and ε phases are 0.87 and 1.45 meV per atom higher in energy than α_new and are thus metastable.

Fig. 4. Transition pathways starting from the X- and W-point instabilities in the γ-Bi₂Sn₂O₇ dispersion (top left). The X-point instability leads to a P2₁2₁2₁ structure (top row) while the W-point mode leads to a Pna2₁ structure (bottom row). Both structures are dynamically stable with no imaginary modes in the dispersions. (The imaginary acoustic mode along the Γ → Z path in the P2₁2₁2₁ is an interpolation artefact – see ESI.†).

Finally, mapping the L-point instability in γ-Bi₂Sn₂O₇ in a 2 × 2 × 2 supercell also leads to the α_new structure in two steps via an intermediate R3̄c (167) phase with 132 atoms per unit cell (Fig. S6†).

We next proceeded to analyse the other instabilities present in the intermediate structures (Fig. S7–S10†). Several pathways end in α_new, while several others lead to the intermediate-temperature β phase.¹⁶ More pathways lead to α_new, suggesting it may be more accessible on the PES.

The dispersion of β-Bi₂Sn₂O₇ has imaginary modes at the Γ and Y wavevectors. Both double-well potentials have a very shallow depth of ∼7 × 10⁻³ meV per atom and lead to a stable Cc structure with 176 atoms per cell (ζ-Bi₂Sn₂O₇; Fig. S11†). This indicates that the β phase is likely a thermal average of equivalent lower-symmetry ζ structures.

To investigate this, we performed Rietveld analysis of a series of powder diffraction patterns collected from 300–1100 K in which the isotropic atomic displacement parameters (ADPs) of the inequivalent Bi sites were independently refined (4 in α, 5 in β, 1 in γ). The ADPs can provide valuable structural information,^31–33 and while this analysis pushes the information content of powder diffraction to its limits, we find that the Bi4 and Bi5 atoms, which disorder during the phase transition, have ADPs in the β phase comparable to those in γ-Bi₂Sn₂O₇ and much larger than the other Bi atoms (Fig. S12†). Similarly, the calculated ADPs for β-Bi₂Sn₂O₇ predict large-amplitude displacements of these atoms.

We further tested the model against the data in ref. 16 with a Rietveld methodology such that the R-factors are directly comparable. Splitting the Bi4 site produces an excellent fit to the neutron and X-ray powder diffraction data and reduces R_wp from 4.355% without splitting to 4.176%. This is also lower than the 4.201% obtained by reducing the symmetry to monoclinic. This provides further evidence that the site is dynamically disordered, as predicted by our calculations, and indicates that ζ-Bi₂Sn₂O₇ represents the true local structure of the intermediate-temperature phase.

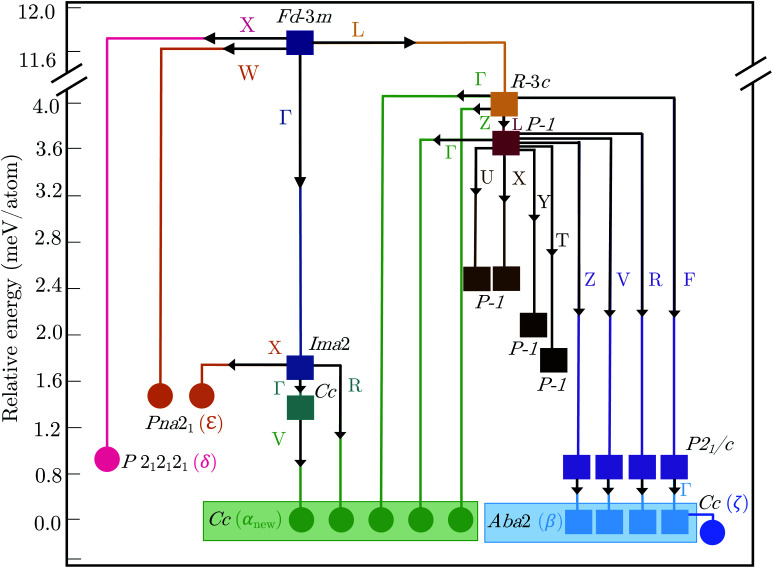
2.3. Polymorph map

Fig. 5 shows the complete polymorph map for Bi₂Sn₂O₇ as a tree diagram. Each branch connects intermediate structures linked by continuous atomic displacements and terminates at structures with no dynamical instabilities. The energies decrease along all pathways with no energetic barriers. Apart from the two new metastable δ and ε phases, all other branches lead to α- and β-/ζ-Bi₂Sn₂O₇. A series of intermediate P1̄ structures with dynamical instabilities were also identified, but the instabilities were not mapped. From their position on the diagram, however, we can infer that they would likely lead to α- or β-Bi₂Sn₂O₇.

Fig. 5. Tree diagram showing the polymorphism in Bi₂Sn₂O₇ starting from the high-temperature γ polymorph (purple square). Energies are shown relative to the α_new-phase (green circle). Branches trace the transition pathways obtained by distorting along single imaginary harmonic modes at each step. Circles and squares indicate dynamically stable and unstable structures, respectively, with the space groups as marked. The wavevectors of the imaginary modes connecting pairs of structures are indicated next to the solid lines.

The small energy separations between minima highlight the complexity of the PES and indicate that different synthesis conditions may yield different polymorphs by changing the free-energy landscape during crystal formation.³⁴ With the exception of β- and ζ-Bi₂Sn₂O₇, all the structures are higher in energy than α_new. However, these energies are the lattice energies from athermal electronic-structure calculations, and free-energy contributions from lattice vibrations and temperature effects are likely to change the energetic ordering. β- and ζ-Bi₂Sn₂O₇ are predicted to be only 0.1 and 0.19 meV per atom lower in energy than the α phase, respectively, and taken together with the experimental data this suggests that α_new is the global energy minimum on the PES.

Future work may employ the quasi-harmonic approximation (QHA) to compute the temperature-dependent Gibbs free energy G and obtain a more accurate free-energy landscape, improved relative stabilities, and predicted phase-transition temperatures.³⁵

2.4. Chemical explanation of distortions

The structural distortions underlying the polymorphism in Bi₂Sn₂O₇ are driven by the stereochemically-active Bi 6s lone pair. Interaction of the Bi 6s and O 2p states produces filled antibonding orbitals at the top of the valence band, which facilitate coupling to the metal 6p states.³⁶ This hybridisation is symmetry forbidden in the cubic γ phase, but instantaneous symmetry breaking from vibrations allows the Bi 6p and Bi 6s/O 2p states to couple. This stabilises the filled antibonding orbital and offsets the increase in energy from the structural distortion, thereby favouring lower-symmetry structures.^37–40

This model is supported from the symmetry-mode analysis of the diffraction data by the larger amplitudes of distortion modes associated with Bi₄O′ tetrahedra compared to those of SnO₆ octahedra.¹⁶ This is also consistent with the eigenvectors of the imaginary modes involved in the γ → α_new transition path (Fig. S13†), which show coupled rotations of the Bi and O′ atoms with very little movement of Sn.

3. Conclusions

We have employed a first-principles lattice-dynamics approach to systematically explore the structural PES of Bi₂Sn₂O₇ starting from the high-temperature cubic pyrochlore aristotype. We recover the known phases from diffraction measurements and identify three new dynamically-stable polymorphs that may be targets for future experimental syntheses. We further demonstrate that the intermediate-temperature β polymorph is a crystallographic average over a lower-symmetry ζ minimum, the structure of which is supported by the diffraction data.

Bi₂Sn₂O₇ is the most complex system yet to be solved using a computational approach, highlighting the strength of our mode-mapping technique for complex multiternary structures, and this approach could be readily extended to other materials with high-symmetry parent structures such as leucite/pollucite,⁴¹ moganite⁴² and andorite.⁴³

Conflicts of interest

There are no conflicts to declare.