Creation of an unexpected plane of enhanced covalency in cerium(III) and berkelium(III) terpyridyl complexes

Abstract

Controlling the properties of heavy element complexes, such as those containing berkelium, is challenging because relativistic effects, spin-orbit and ligand-field splitting, and complex metal-ligand bonding, all dictate the final electronic states of the molecules. While the first two of these are currently beyond experimental control, covalent M‒L interactions could theoretically be boosted through the employment of chelators with large polarizabilities that substantially shift the electron density in the molecules. This theory is tested by ligating Bk^III with 4’-(4-nitrophenyl)-2,2’:6’,2”-terpyridine (terpy*), a ligand with a large dipole. The resultant complex, Bk(terpy*)(NO₃)₃(H₂O)·THF, is benchmarked with its closest electrochemical analog, Ce(terpy*)(NO₃)₃(H₂O)·THF. Here, we show that enhanced Bk‒N interactions with terpy* are observed as predicted. Unexpectedly, induced polarization by terpy* also creates a plane in the molecules wherein the M‒L bonds trans to terpy* are shorter than anticipated. Moreover, these molecules are highly anisotropic and rhombic EPR spectra for the Ce^III complex are reported.

Studying how the ligand design influences the bonding of f-block complexes is crucial to control their properties. Here, the authors report the preparation of Bk(III) and Ce(III) complexes featuring a terpyridyl ligand; structural, spectroscopic, electrochemical, and theoretical analysis reveal that the ligand induces unusual bonding by creating a plane of enhanced bond covalency.

Introduction

Unexpected properties, structures, and reactivities emerge in heavy elements because their high nuclear charge accelerates surrounding electrons to relativistic speeds, altering orbital shapes and energies and the nature of chemical bonds¹. This in turn, leads to abrupt changes in behavior between neighboring elements^2–8 and a breakdown of simple descriptions of electronic structure that can be used to explain emerging properties^1,9–17. Examples of these discontinuities include the large volume expansion between α-Pu and α-Am, and the corresponding localization of 5f electrons that leads to superconductivity in α-Am at low temperatures¹⁸, as well as the diminishment of redox activity that occurs at this same juncture in the actinide series¹⁹. Moreover, between berkelium and californium a second transition occurs whereby the divalent state becomes metastable in both the pure elements and in compounds^2,15. Understanding the origin of these step functions between neighboring actinides has been at the forefront of research since the dawn of the Atomic Age.

In a more general sense, many electronic factors arise in magnitude in a nonlinear manner in heavy elements. For example, between hydrogen (Z = 1) and bismuth (Z = 83) there is only a 25% increase in the relative mass of the 1s electrons induced by acceleration afforded by nuclear charge. In contrast, between bismuth and uranium (Z = 92), the perceived mass increases by an additional 25% even though Z has only increased by 9²⁰. Spin–orbit coupling, a consequence of relativistic effects, scales as Z⁴¹, and is large enough in magnitude to mix L and S states together in the traditional Russell–Saunders coupling scheme²¹. Moreover, the spin–orbit splitting not only affects the ground state but also the excited states. In the actinide series, the splitting is large enough to mix ground and excited configurations giving rise to multi-reference states^19,22,23. In Bk(IO₃)₃, for example, the ground state consists of ~70% the LS term (⁷F₆) and ~30% the first excited state (⁵G₆). Thus, the magnetic properties of Bk(IO₃)₃ would be expected on this basis alone to differ from the ostensibly isoelectronic Tb^III analog, and this is observed⁶. Similar differences are found between Bk(Hdpa)₃ and Tb(Hdpa)₃ (dpa = dipicolinate; 2,6-pyridinedicarboxylate)⁸. In addition to magnetic susceptibility, optical properties and even bond lengths not only differ between formally isoelectronic ions (e.g., Dy^III and Cf^III) but also between neighboring actinides in a non-systematic way as observed in the aforementioned breaks between plutonium and americium and again between berkelium and californium^2–8.

In actinide compounds, the frontier orbitals (5f, 6p, 6d, 7s, 7p) can contribute to bonding to a greater extent than occurs in corresponding lanthanide systems despite f-element–ligand bonds being dominated by electrostatic interactions^24–26. This can also lead to deviations in chemical and physical properties between the 4f and 5f series that manifests in the adoption of different structures with distinct physical properties emerging³. It is also now established that ligand-field splitting is larger than expected beyond curium, and examples in both berkelium and californium systems exist where this splitting is ca. 2000 cm^‒15,6. Coupling these features together with the decreased e^‒···e^‒ repulsion between 5f electrons vs. those in 4f orbitals^27,28 leads to the so-called intermediate coupling regime where no single electronic effect (interelectronic repulsion, ligand field, spin–orbit coupling) dominates, and predicting the physico-chemical properties of actinide molecules becomes quite challenging^2,6,19.

Thus, the question arises as to whether the electronic structures of actinide complexes can be substantially altered through the design of specific electronic attributes of the ligands surrounding it given the complexities of the metal ions in these systems. While there are certainly numerous examples of the use of ligands to create specific symmetries^29,30, large binding constants^31,32, and open-coordination sites around actinides that lead to unique reactivities^12,33–39, substantial changes in bonding might also be achievable in actinide complexes by using ligands that create large dipole moments. Guidance on how to achieve this effect exists from the large body of work for designing organic nonlinear optical materials⁴⁰. Herein, we show that a terpyridyl ligand with a large polarizability, 4’-(4-nitrophenyl)-2,2’:6’,2”-terpyridine (terpy*), can be used to create unusual bonding and rare spectroscopic features in a berkelium(III) complex. To the best of our knowledge, this is only the sixth berkelium compound for which a single crystal structure has been solved, thus the opportunity to compare it to its closest electrochemical analog, Ce^III, was also undertaken in this work.

Results and discussion

Synthesis

²⁴⁹Bk has a half-life of 330 days and therefore has an unusually high specific activity. This is especially apparent when compared to earlier actinide isotopes such as ²³⁸U that possesses t_½ = 4.5 × 10⁹ years. Even a few milligrams of ²⁴⁹Bk creates Ci levels of radiation. Recoil from the β decay of ²⁴⁹Bk is in the keV range and creates local disruption of chemical bonds. Moreover, its rapid decay to ²⁴⁹Cf (t_½ = 351 years) creates an α emitter with energies above 5 MeV that again leads to further sample destruction. Substantial degradation of solvents, ligands, and compounds occurs within a few days because, in addition to the damage from nuclear recoil, and the damage paths from the trajectories of the α and β particles, reactive radiolytic products, such as hydroxyl radical, create undesirable side reactions that yield intractable mixtures of products. Crystals of targeted compounds must therefore be grown, isolated, and fully characterized within hours of preparation or Coulombic explosions occur that render them into nanocrystalline or amorphous solids that are difficult to characterize further.

²⁴⁹Bk decays to ²⁴⁹Cf at a rate of ~1.5% week⁻¹. This necessitates the separation of ²⁴⁹Cf from ²⁴⁹Bk immediately prior to synthesis. ²⁴⁹Bk was isolated from an aged mixture of ²⁴⁹Bk/²⁴⁹Cf that had a ratio of ca. 1:5 via the oxidation of Bk^III to Bk^IV under slightly basic conditions using 30% H₂O₂. This vigorous reaction results in the precipitation of Bk(OH)₄ as a deep red solid and leaves Cf^III behind as an emerald green solution. This product was subsequently converted to Bk(NO₃)₃·nH₂O by gentle fuming in 8 M HNO₃.

The reaction of freshly-prepared Bk(NO₃)₃·nH₂O or Ce(NO₃)₃·nH₂O with 4’-(4-nitrophenyl)-2,2’:6’,2”-terpyridine (terpy*) in tetrahydrofuran (THF) yields golden columns of Bk(terpy*)(NO₃)₃(H₂O)·THF and Ce(terpy*)(NO₃)₃(H₂O)·THF (Bk1 and Ce1, respectively) within a few hours. The corresponding Ce^III complex lacking the appended 4-nitrophenyl group, Ce(terpy)(NO₃)₃(H₂O)·THF (Ce2), was also synthesized for comparison by similar methods. Further synthetic details for Bk1, Ce1, and Ce2 can be found in the Supplementary Information in the “Supplementary Methods” section.

Structural characterization

Single crystal X-ray diffraction data from crystals of Bk1, Ce1, and Ce2 were measured from samples cooled to 28 K using a helium cryostat. While such data collections are fraught with technological woes, such as rapid and severe icing, they potentially allow for significant improvements in the precision of bond distances (by an order of magnitude), increased diffraction intensities, and reduced thermal motion of atoms⁴¹. The latter reduction means that the measured bond distances have substantially less libration^42,43 and are much closer to libration-free interatomic distances.

Bk1 and Ce1 are isomorphous and adopt the same structure as found with other trivalent lanthanides and actinides as we recently reported for Am^III44. The structure of Bk(terpy*)(NO₃)₃(H₂O) with the co-crystallized THF molecule omitted is shown in Fig. 1a and contains a Bk^III cation bound by three bidentate nitrate anions, one tridentate terpy* ligand, and one water molecule yielding a ten-coordinate environment. These bond distances are tabulated in the Supplementary Materials, but some critical features are noted below. They can also be used to calculate the ionic radius of ten-coordinate Bk^III, of which this is the first example, and yield a value of 1.19 Å that parallels that of Sm^III44.

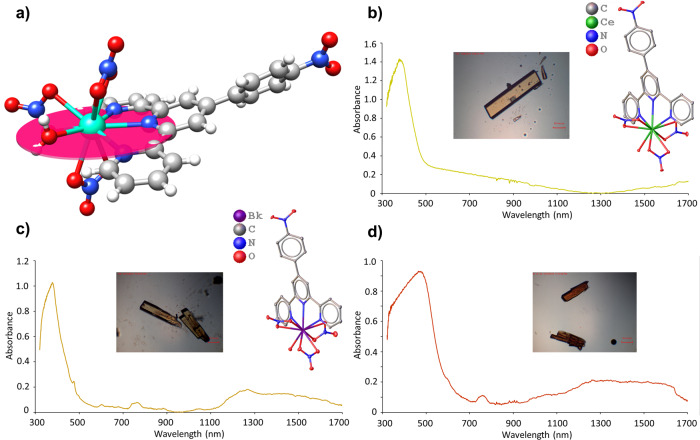
Fig. 1. Characterization of Ce^III and Bk^III terpyridine complexes.

a Depiction of the plane defined by the terpyridine derivative, water molecule, and equatorial nitrate ligand. The optimization of the plane was performed using the coordinates of the terpyridine nitrogen atoms, metal center, and water oxygen atom. b Absorption spectrum, crystal structure, and single crystal picture of Ce1. c Absorption spectrum, crystal structure, and single crystal pictures of Bk1 the day they were collected and d 3 days later.

The key feature of the M(terpy*)(NO₃)₃(H₂O) (M = Bk, Ce) molecules is that they contain a nearly planar moiety composed of terpy*, the bound water molecule, and one of the nitrate molecules (Fig. 1a). This nitrate anion is bisected by the polarization plane. Two additional nitrate anions that bind the metal centers are also present above and below this plane. The simplest way to illuminate the differences between ligands in this plane vs. those out of the plane is achieved by comparing the asymmetry of Bk‒O bond lengths with the nitrate anions. In Bk1, the deviation between the two Bk‒O bond lengths of the nitrate anions are 0.088(3) and 0.062(3) Å above and below the plane, respectively; whereas the Bk‒O bond lengths to the nitrate anion trans to the terpy* are more similar and differ by 0.023(3) Å. Similarly, in Ce1 the differences in the Ce‒O bond lengths of the nitrate anions above and below the plane of the terpy* are 0.031(2) and 0.034(2) Å; while the difference between the Ce‒O bond lengths of the nitrate molecule trans to the terpy* is 0.013(2) Å. For Bk1, this gives an average difference of 0.075(3) Å axially and 0.023(3) Å in the plane. Likewise, Ce1 gives an average difference on 0.033(2) Å axially and 0.013(2) Å in the plane.

A similar observation is made when examining the M‒OH₂ bond distances in these molecules. Here a comparison is made between the structure of Ce1 and Ce2 (Supplementary Fig. 17) where the latter lacks the 4-nitrophenyl moiety. In Ce2, the water molecule is not co-planar with the terpy ligand and the Ce‒OH₂ bond distance is 2.5267(6) Å. In contrast, in both Bk1 and Ce1 the bound water molecule is co-planar with the terpy* ligand, and the Ce‒OH₂ bond is statistically shorter (3σ) than found in Ce2 with a distance of 2.491(2) Å. The difference in conformations between Bk1/Ce1 and Ce2 is likely a consequence of the polarization by terpy* (vide infra). Bk1, Ce1, and Ce2 all contain an outer-sphere THF molecule that interacts with the bound water molecule through hydrogen bonding. The disparate placement of the water molecule in Ce2 could alternatively be attributed to crystal packing, as it lacks the nitrophenyl group present in Ce1 and Bk1.

Gas-phase studies

To further understand the strength of the interaction of Ce^III with terpy and terpy*, collision-induced dissociation of gas-phase coordination complexes was carried out (“Additional Discussion” section in Supplementary Information). These studies were compared to the previously reported Eu1 structure⁴⁴. Our results reveal that in gas-phase complexes both terpy and terpy* bind more strongly to Ce^III than Eu^III and that both Ce^III and Eu^III bind more strongly to terpy than terpy*. This measurement is consistent with the terpy* being a weaker σ-donor than terpy as would be anticipated from the electron-withdrawing nature of the 4-nitrophenyl group. It is noteworthy that these results cannot be correlated to the formation of a plane of interaction due to the crystal packing effects vs. gas-phase molecular geometries. Distinctive processes corresponding to oxidation to Ce^IV and reduction to Eu^II, which directly reflect condensed-phase redox properties, are revealed upon dissociation of gas-phase complexes.

Optical and magnetic circular dichroism (MCD) spectroscopy

Ultraviolet–visible (UV-vis)–near-infrared spectra were collected from single crystals of Ce1 and Bk1, and again for Bk1 3 days after the crystals were formed (Fig. 1b–d). All three spectra show a broad band centered near 400 nm that is assigned to intra-ligand transitions for the terpy* complexes. For Bk1, the characteristic f–f transitions for Bk^III are observed⁴⁵, confirming that Bk^III has not been oxidized to Bk^IV (Fig. 1c, d).

To aid in the assignment of the absorption spectrum of Ce1, the 5K MCD spectrum was obtained in the UV-vis region (Supplementary Fig. 16). C-term MCD spectroscopy provides higher resolution and the benefit of both positive and negative sign transitions that aids in separating and assigning overlapping transitions like those observed in Ce1. The spectrum could be fit to multiple transitions, as predicted by computational analysis that were subsequently assigned to a series of 4f ⟶ ligand and ligand ⟶ 4f charge–transfer transitions (Supplementary Table 5).

Cyclic voltammetry of Ce1 and Bk1

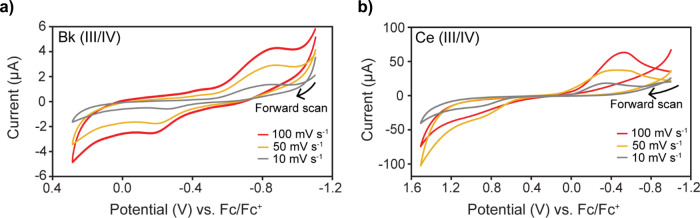
The Ce1 and Bk1 complexes exhibit similarly quasi-reversible electrochemical behavior, with very wide peak separations evident in the cyclic voltammograms (CVs) in Fig. 2. The Bk1 complex was more reversible than the Ce1 complex, as demonstrated by the smaller peak-to-peak separation (0.729 vs. 1.542 V, Table 1). A variety of cerium complexes have previously been shown to exhibit low reversibility⁴⁶, and varying degrees of quasi-reversibility have been exhibited in selected lanthanide cryptates in THF in a previous study⁴⁷. Cerium undergoes a potential shift of ~300 mV upon complexation with terpy* (Table 1) when compared to cerium nitrate (i.e., prior to Ce1 complex formation). The cerium and berkelium complexes had (IV/III) reduction peak potentials, E_p,c, of −0.522 and −0.887 V, respectively, differing by about 0.350 V, and (III/IV) oxidation peak potentials, E_p,a, of 1.020 and −0.158 V, respectively, differing by 1.180 V. The very wide peak-to-peak separations, an indication of poor reversibility, are much greater than those found in previous work^5,46.

Fig. 2. Cyclic voltammograms of Ce^III/Ce^IV and Bk^III/Bk^IV terpyridine derivatives in THF and electrochemical data.

Potential scan rates of 10 (gray), 50 (yellow), and 100 (red) mV s⁻¹ were used. a Voltammograms showing the oxidation (III/IV) and reduction (IV/III) of the Bk/Terpy* complex, in 0.1 M BArF (supporting electrolyte). b Voltammograms showing the oxidation (III/IV) and reduction (IV/III) of the Ce/Terpy complex, in 0.1 M TBA PF₆ (supporting electrolyte). All data have been corrected to zero volts vs. an internal Fc/Fc⁺ reference.

Table 1.

Electrochemical data.

Complex	E1/2 (vs. Fc/Fc+) (V)	Ep,a (vs. Fc/Fc+) (V)	Ep,c (vs. Fc/Fc+) (V)	Ep,a − Ep,c (V)	∆Ep,c for complex vs. non-complexed (V)
Ce1	0.249	1.020	−0.522	1.542	−0.277
Bk1	0.207	−0.158	−0.887	0.729	N/A

All data have been corrected to zero volts vs. an internal Fc/Fc⁺ reference and was recorded at 100 mV s⁻¹.

All voltammograms showed the presence of water, for which no effort was made to remove, since water is coordinated to the metal center of the complex. The sharp rise in anodic current at the end of the forward sweep is indicative of water oxidation, and the initially large cathodic current can also be explained by the reduction of water. Since the amount of water in the sample is likely to have varied between experiments, these currents were also variable. The anodic current was close to the oxidation of Ce^III to Ce^IV, making the peak less prominent. The solvent window of water is much smaller than that of THF, so the presence of water posed a challenge for the observation of the anodic peak⁴⁸. Peak identity was confirmed using different concentrations of the complex, with all other variables kept the same (Supplementary Information Fig. 19). This result also confirmed that, as well as increased complex concentration, the addition of more complex also resulted in more water present in the solution.

A potential scan rate-dependent current response was observed for the voltammetry, as expected when using a macroelectrode. CVs were recorded at 10, 50, and 100 mV s⁻¹, which resulted in increasing current magnitudes for both the reduction and oxidation peaks of Ce1 and Bk1 (Fig. 2 and Table 1). Lower scan rates resulted in more clearly defined oxidation peaks, with lower current. These data were collected for freshly prepared complexes in THF solution. Data were collected at similar times after complex formation, to minimize the effects of solvent loss due to evaporation, which would increase peak current magnitude.

Electron paramagnetic resonance (EPR) and magnetism

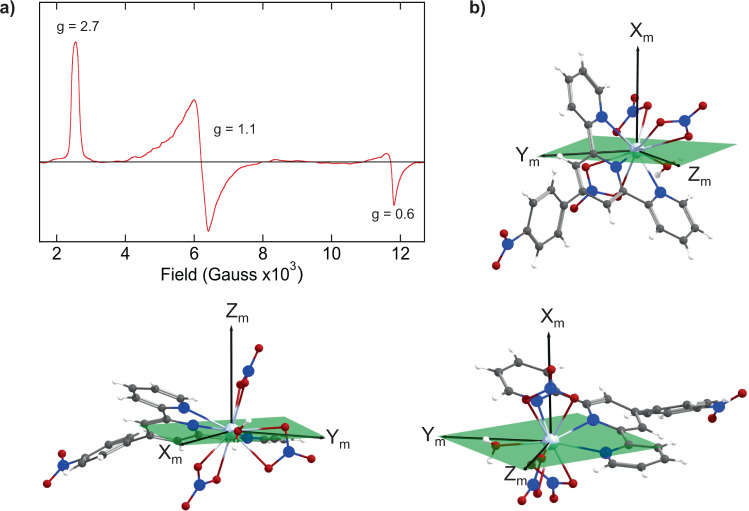
Ce^III has a 4f ¹ configuration that has been shown to exhibit anisotropic EPR spectra in multiple molecular systems^49,50. According to the 5K EPR spectrum of a powder sample of Ce1 (Fig. 3a), Ce^III displays an anisotropic signal with three distinct g values, 2.7, 1.1, and 0.6. This anisotropy reflects the different binding of the terpy*, aquo, and nitrate ligands to Ce^III resulting in three distinct molecular axes and a rhombic system.

Fig. 3. Electron paramagnetic resonance and g-factors for Ce1.

a Experimental EPR spectrum and the corresponding g-factors. b Quantization axes and magnetization planes for the three KDs ordered by increasing energy from left to right.

The f-block complexes commonly exhibit large deviations of the g-factors from the spin only value (g_e ~ 2) and pronounced magnetic anisotropy. These effects are produced by an orbital contribution to the magnetic moment that result from the spatial degeneracy of an open shell in combination with spin–orbit interaction and covalent interactions with the ligands. Owing to the effect of the ligand field, the ²F_5/2 ground multiplet of Ce^III splits into three Kramers doublets (KDs) characterized by a pseudospin S = 1/2. This approximation refers to a spin acting in a model space of eigenfunctions $\mid M_{\mathrm{S}}⟩$ for the pseudospin projection onto a quantization axis that is useful to interpret our results. The calculated energies and g-factor components of these three KD states are presented in Table 2.

Table 2.

g-factors for Ce1.

E (cm−1)	gx	gy	gz	<Lz>	<Sz>	<Lx>	<Sx>	<Ly>	<Sy>
0.0	0.789	1.372	2.671	−1.887	0.275	−0.409	0.007	−0.990	0.152
131.0	0.378	0.969	2.535	−1.708	0.219	−0.254	0.032	−0.601	0.058
299.7	2.640	1.787	0.859	−0.628	0.099	−1.817	0.248	−1.139	0.123

Theoretical energies, g-factors, and the orbital and spin angular momentum expectation values obtained from spin–orbit CAS(1,7) wave functions for the three Kramer’s doublets (KDs) derived from the ²F_5/2 ground multiplet.

The calculated g-factors for the ground state agree with the experimental values (Fig. 3a and Table 2), given the quantization axes shown in Fig. 3b. An important contribution of angular momentum was observed for the three KDs that also have an opposite sign to the spin contribution as expected for an 4 f¹ configuration (less than half-filled shell) (Table 2). It is interesting to note that the g-factors describe a magnetization plane for KD1 (yz), KD2 (yz), and KD3 (xy), where x, y, and z represent the quantization axes for each KD. Furthermore, this is accompanied by significant contributions from the components of the orbital angular momentum defined on these planes of magnetization. A more detailed analysis (Table 2) shows that these planes are formed by the water molecule, the terpy* nitrogen atoms, and the equatorial nitrate group. The observation of this magnetic plane along with the significant angular momentum contribution to the g-factor may be related to the presence of a plane of covalency between Ce^III and the ligands sitting on this plane⁵¹, though further studies would be required to confirm this. On the other hand, the shape of the 4f electron density that is directly related to the occupation of the 4f natural orbitals shows an oblate nature, where the electron density is distributed preferentially in the plane (KD1 in Supplementary Fig. 3). This can be correlated with the spin magnetization that is distributed equally in the magnetization plane with an oblate shape. Since the total splitting of the ²F_5/2 ground term into M_J substates (~300 cm⁻¹) matches the same magnitude as kT at room temperature (~210 cm⁻¹), the three KDs are populated (according to the Boltzmann distribution), and therefore all have a direct influence in the observed magnetic properties at room temperature.

For Bk1, the ground state corresponds to a non-KD derived from the ground multiplet ⁷F₆ according to the Bk^III free ion with contributions from the excited multiplet ⁵G₆ (9%) due to spin–orbit coupling. This enables us to analyze this state using a pseudo-spin ½ Hamiltonian. Unlike Ce1, this ground state exhibits a large magnetic anisotropy with g_z = 17.7 and g_x = g_y = 0.0, owing to an important contribution of angular momentum (L_z = 2.858) and spin (S_z = 2.993) that is expected for a 5f⁸ configuration (more than half-filled shell). At room temperature, the calculated magnetic moment is 9.677 μ_B, close to the expected value for a pure ⁷F₆ multiplet 9.72 μ_B. The predicted magnetic susceptibility (χT) that reaches a value of 11.39 cm³ K mol⁻¹ at room temperature decreases slowly even at low temperatures because of contributions of low-lying states. This behavior does not differ significantly from that previously observed in other Bk^III compounds⁶.

Examination of chemical bonding

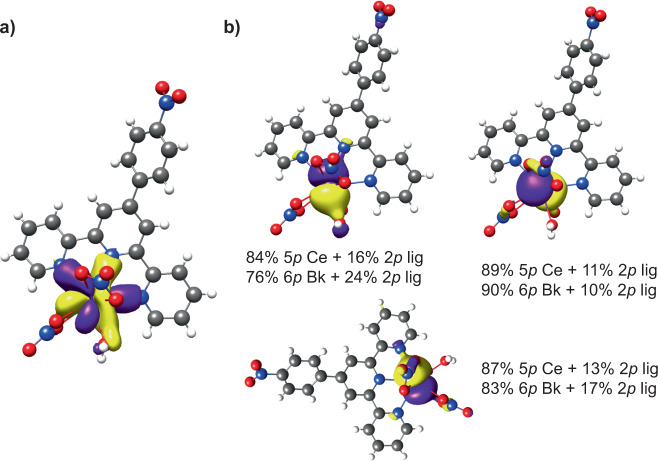
From the molecular orbital perspective, two main aspects are to be emphasized to elucidate the nature of this plane of enhanced interactions: (i) the observation of one f orbital featuring the interaction with terpy* and aquo ligands (Fig. 4a), and (ii) the role of the 5p/6p semi-core orbitals in bonding to expose the 4f/5f shells. The latter is exemplified in the mixing of these metal orbitals with 2p-ligand orbitals (Fig. 4b). It is important for the reader to note that these orbital interactions represent subtle effects compared to the dominant force in the bond formation, i.e., electrostatic interactions. Further discussion on the electronic structure is found in the Supplementary Information (see “Theory” in the “Additional Discussion” section).

Fig. 4. CASSCF natural orbitals and ligand-field DFT parameters.

Natural orbitals derived from state-specific scalar-relativistic CAS(1,7) and CAS(8,7) for Ce1 and Bk1. a For both complexes, there is an f-orbital similar to the f_±3 orbital that shows a preferential orbital interaction between the metal center, terpy*, and the water molecule. This orbital is crucial to define the plane of covalency. b Orbital mixing between the 5p/6p orbitals with 2p-ligand orbitals showing the participation of the core in chemical bonding.

Ligand-field density functional theory⁵² was used to evaluate the expansion of the 5p/6p radial functions through the reduction of the inter-electron repulsion for the Ce1 and Bk1 complexes with respect to their corresponding free ions⁵³. Our results show that semi-core 5p/6p electrons are involved in covalent interactions due to the observed reduction in the inter-electron repulsion as well as the effective spin–orbit coupling parameter. The more polarizable character of the 6p shell in Bk^III, than the 5p in Ce^III, is evidenced in the increased reduction observed in Bk1 (50% in F^k and 31% in ζ_SO) compared to Ce1 (42% in F^k and 25% in ζ_SO) (Table 3). These results show that electron repulsion between semi-core electrons is overcome by the covalent interactions with the coordinating ligands. Furthermore, the involvement of the semi-core orbitals have been associated previously with the inverse trans influence (ITI)^54–56 that is now offered in a broader sense to understand covalency. The difference resides in that for ITI the semi-core 6p orbitals “push from below” by hybridizing with the 5f-orbitals, whereas in Ce1 and Bk1 this occurs by direct mixing (i.e., hybridization) with the ligand 2p orbitals.

Table 3.

Ligand-field DFT parameters.

5p parameters	Ce3+	Ce1	Reduction	6p parameters	Bk3+	Bk1	Reduction
F0 (5p,5p)	14.23	8.23	42%	F0 (6p,6p)	14.51	7.24	50%
F2 (5p,5p)	7.74	4.45	42%	F2 (6p,6p)	8.03	3.99	50%
ζSO (5p)	1.75	1.31	25%	ζSO (6p)	6.62	4.60	31%

Slater–Condon inter-electron repulsion and effective spin–orbit coupling parameters of the 5p and 6p shells obtained for the Ce^III and Bk^III free ions and Ce1 and Bk1 along with their corresponding reductions due to covalent interactions.

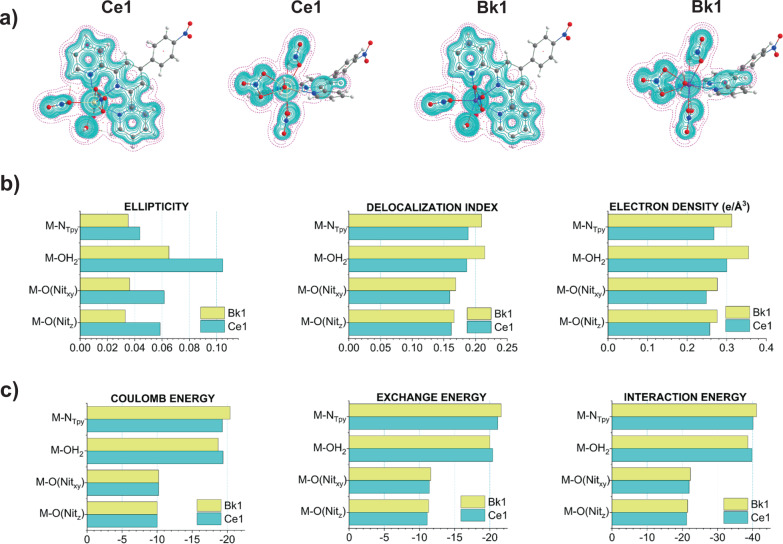
To shed light on this plane of covalency, the quantum theory of atoms in molecules (QTAIM)^57,58 was used to map the electron density at the interatomic region and derive useful metrics, such as delocalization indices (pairs of shared electrons), energy densities, and ellipticities. These metrics are helpful to describe the nature of the bond in terms of concentration of electron density ρ(r) at the so-called bond critical point (BCP) (see “Additional Discussion” section in the Supplementary Information).

It is well known that trivalent lanthanides are considered to be hard Lewis acids or at least harder than actinides. Therefore, it should be expected that terpy* would bind significantly more strongly to Bk^III than to Ce^III, which is not the case. Although it is possible to see a difference in M–N bond metrics, the most striking difference is observed for both M–OH₂ bonds. From Carnall’s work on the spectroscopy of lanthanides and actinides, the aquo complexes are considered a “diluted ion,” thus implying that their properties should resemble those of the free ion^59,60. This approximation does not hold for Ce1 and Bk1, where their M–aquo bonds are shown to display more significant covalent interactions and their ρ_BCP(r) values are approximately of the same order as that of the metal–terpy* bonds (Fig. 5b and Supplementary Tables 6 and 7). It is important to note that differences in the accumulation of ρ_BCP(r) are a direct measure of the strength of the bond, and therefore the orbital overlap between the two atoms involved. Despite this, only for Bk1 this interaction is shown to be significantly covalent based on the H(r) values (Supplementary Tables 6 and 7), while Ce1 displays a negative, but close to zero, value. The formation of the plane of covalency is qualitatively shown in Fig. 5a, where the solid cyan lines represent regions where the total energy density is negative. To highlight this increased covalent character on Ce1 and Bk1, we have previously reported the Eu1 and Am1 structures where the Eu–OH₂ bond displays a positive H(r) value and the Am–OH₂ ca. −5 kJ mol⁻¹ Å⁻³⁴⁴. In contrast, Ce1 and Bk1 predict more excess of potential energy density, and therefore covalent character, with values −1.8 and −22.8 kJ mol⁻¹ Å⁻³ (Supplementary Tables 6 and 7).

Fig. 5. Bonding features of the plane of covalency based on the CASSCF electron densities.

a Plots of the total energy density, H(r), in the preferential plane of interaction for Ce1 (Ce = yellow sphere) and Bk1 (Bk = purple sphere) and perpendicular to this plane. The solid cyan lines denote the regions where H(r) is negative (covalent character), while pink dashed lines represent areas where H(r) is positive (purely ionic). The water molecule as well as the terpy* N atoms display covalent interactions with the metal centers in both Ce1 (cyan bars) and Bk1 (yellow bars) compared to the nitrate ligands. b QTAIM metrics for Ce1 and Bk1; the ellipticity describes the deviations from a cylindrical single bond as values differ from zero, while delocalization indices and electron densities describe the shared pairs of electrons and accumulation of electrons in the bond critical points, respectively. c Interacting quantum atom (IQA) energy decomposition analysis in kJ mol⁻¹. The total energy of interaction is decomposed into Coulomb or electrostatic and exchange or covalent energy components. M–N_terpy correspond to average metrics of the three metal–terpy* bonds; M–O(Nit_xy) and M–O(Nit_z) refer to metal–nitrate bonds in the plane (equatorial) and out of the plane (axial), respectively. Tables with detailed information of QTAIM and IQA can be found in the Supplementary Information.

An alternative to the QTAIM approach is the interacting quantum atom (IQA) method⁶¹ that provides an estimation of the interaction without employing the concept of BCP by integrating the electron density and providing a scheme of energy decomposition based on QTAIM. In principle, this natural partition of the molecule provides a more reliable estimation of the interacting densities between the metal and the ligands. However, we cannot fully rely on these numbers due to the approximation introduced to calculate the two-electron interactions (Supplementary Information). Figure 5c shows the decomposition of the M–L interaction into Coulombic (electrostatic) and exchange (covalent) components (Supplementary Table 8). The latter directly relates to the strength of the interaction and therefore is the parameter to consider. The results overall agree with the QTAIM metrics except for the description of the M–OH₂ interaction that suggest the Ce–OH₂ interaction to be stronger than the Bk–OH₂ bond (Fig. 5c). This difference in strength is rather unexpected from the increased covalency one would expect for actinides over lanthanides. The origin could reside in the fact that lanthanides are more oxophilic than actinides, and therefore Bk^III generally displays a preference toward N-donor ligands, whereas Ce^III toward O-donor molecules. Regardless, all of our theoretical results support that the unusual behavior of the water molecule is attributed to the effect of the terpy* ligand on the metal center that causes the formation of a preferential plane of covalency.

In summary, the combination of structural, spectroscopic, electrochemical, and theoretical analysis of M(terpy*)(NO₃)₃(H₂O) (M = Bk, Ce) all support that the large polarizability of the terpy* ligand creates a plane where M–L interactions are enhanced and a highly anisotropic electronic environment around the metal centers exists. More generally, Bk1 shows greater involvement of the frontier orbitals in forming chemical bonds than occurs in Ce1, and this in turn is reflected in improved quasi-reversibility of electrochemical processes. These compounds represent proof of concept that the principles used to guide the synthesis of organic nonlinear optical materials, i.e., donor–acceptor molecules, can also be used to create ligands that enhance the involvement of frontier orbitals in forming chemical bonds in the 5f series. A large and diverse family of compounds should be achievable.

Author contributions

A.N.G., F.D.W., J.M.S., S.R.S., T.N.P., and T.E.A.-S. conceived, designed, and carried out the synthetic, spectroscopic, electrochemical, and crystallographic experiments with Bk1. C.C.-B., M.J.B.-L., and D.P.-H. carried out the theoretical studies. T.J. and J.K.G. conducted the gas-phase experiments. N.J.W. and M.L.N. performed the EPR and MCD experiments. Electrochemical studies of Ce1 were conducted by N.J.J., A.J.R., and R.A.L. R.E.B. conducted the magnetism studies. D.G.M. conducted IR spectroscopic measurements. All authors discussed and co-wrote the manuscript.

Peer review information

Nature Communications thanks the anonymous reviewer(s) for their contribution to the peer review of this work. Peer reviewer reports are available.

Data availability

The data that support the findings of this study have been deposited in the CCDC database with the accession codes 1857536, 2050447, and 2050448 that contain the supplementary crystallographic data for this paper. These data can be obtained free of charge via www.ccdc.cam.ac.uk/data_request/cif, or by emailing data_request@ccdc.cam.ac.uk, or by contacting The Cambridge Crystallographic Data Centre, 12 Union Road, Cambridge CB2 1EZ, UK; fax: +44 1223 336033.

Competing interests

The authors declare no competing interests.

Supplementary information

The online version contains supplementary material available at 10.1038/s41467-021-27576-y.