Fetal Loss and Feminine Sex Ratios at Birth in Sub-Saharan Africa

Abstract

A wealth of demographic research explores the determinants of sex ratios at birth. Few studies consider the role of fetal loss, or spontaneous abortion, in producing feminine sex ratios. One challenge is measuring the occurrence of fetal loss, which is difficult to recognize and report in survey research. This study uses the length of the birth interval as a proxy for fetal loss; fetal loss restarts the clock on time to conception and lengthens the birth interval. Using Demographic and Health Survey data on second births to women in 17 Sub-Saharan African countries, results show that longer second birth intervals are significantly related to lower odds of a male second birth and with feminine sex ratios at birth. These findings suggest that high levels of fetal loss, which could signal underlying poor maternal health in a population, have dramatic effects on the sex ratio at birth

Introduction

Demographers have long been interested in sex-selective abortion as a strategy parents undertake to avert a female birth. This conscious behavior creates the phenomenon of missing girls and skews sex ratios toward males at the population level (Sen 1992, 2003; Bongaarts and Guilmoto 2015). Yet spontaneous fetal loss—an unconscious occurrence—remains a relatively unexplored determinant of sex ratios, specifically its role in producing feminine sex ratios at birth, or those lower than the natural sex ratio of 105 to 107 males born for every 100 females (Bongaarts and Guilmoto 2015). A number of studies suggest that various maternal stressors can lead to spontaneous miscarriage, and that these fetal losses are disproportionately male (McMillen 1979; Kellokumpu-Lehtinen and Pelliniemi 1984; Byrne et al. 1987; Kraemer 2000). High rates of fetal loss in a population could therefore produce skewed, feminine sex ratios at birth.

One issue limiting demographic research in this area is difficulty measuring spontaneous abortion, or fetal loss. Several recent studies have exploited exogenous shocks or exposures, such as famines, terrorist attacks, and earthquakes, and have examined their impact on feminine sex ratios at birth in various settings (e.g., Catalano 2003; Valente 2015). The underlying assumptions are that such events produce maternal stress that increases the likelihood of fetal loss, and that these fetal losses are disproportionately male. Fetal loss is unobserved; nevertheless, researchers presume it is a major biological pathway operating to reduce the number of male births, resulting in feminine sex ratios at birth.

In contrast to studies that focus on extreme events or exposures, we examine the association between fetal loss and sex ratios at birth under more common and widespread conditions. Our interest is in the continent of Sub-Saharan Africa, where sex ratios have historically been skewed toward females. A challenge to our analysis, however, is measuring fetal loss at the individual level. Mothers’ self-reports are unreliable, and clinical detection is difficult to implement in large-scale surveys. Instead, we employ a unique strategy and use the length of the birth interval to proxy for the occurrence of fetal loss. A fetal loss resets the clock on time to conception, thereby increasing the length of the birth interval, ceteris paribus. We hypothesize that women who suffer fetal loss are more likely to successfully carry a female fetus to term, and thus longer birth intervals (which proxy for fetal loss) will be associated with decreased odds of a subsequent male birth and feminine sex ratios at birth.

Our study has two main goals. First, we provide the first test of our hypothesis using a large population-based sample of births in Sub-Saharan Africa. Second, we use our micro-level results to estimate population sex ratios at birth by various birth interval lengths. Our results reveal that longer birth intervals are significantly related to lower odds of a subsequent male birth, as expected, and with increasingly feminine sex ratios at birth. These findings suggest that high levels of fetal loss in a population could have dramatic effects on the sex ratio at birth.

We use Demographic and Health Survey (DHS) data from 17 countries in Sub-Saharan Africa for the analyses. Compared to a natural sex ratio of 105–107 males born for every 100 females, the sex ratio at birth in Sub-Saharan Africa is 103:100 (Kaba 2008; United Nations Population Division 2019), and many African countries’ populations dip even lower (Garenne 2002). For example, Namibia, Rwanda, Togo, and Mozambique have consistently recorded sex ratios at birth at or lower than 102:100 (United Nations Population Division 2019). Although these differences appear minor, they translate into large differences in absolute numbers of births. Given the birth rate in Sub-Saharan Africa each year, the difference between a sex ratio at birth of 103:100 and 106:100 is approximately 250,000 male births annually. To use the language of the sex-selection literature: there are 250,000 missing boys in Sub-Saharan Africa each year. These missing boys could signal an underlying reproductive health problem in Sub-Saharan Africa—elevated rates of fetal loss.

Background

Exposure Studies and Male Fetal Loss

A body of research across the biological, social, and health sciences links exogenous shocks or exposures to feminine sex ratios at birth across multiple contexts. For example, studies have documented the feminization of sex ratios at birth following traumatic events, such as civil conflict in Nepal (Valente 2015), terrorist attacks, such as 9/11 and the 2004 Madrid and 2005 London bombings (Catalano et al. 2006; Masukume et al. 2017), and earthquakes (Fukuda et al. 1998, 2019; Saadat 2008; Torche and Kleinhaus 2012; Catalano et al. 2013). Longer-term exogenous stressors, such as famines and food shortages (Williams and Gloster 1992; Andersson and Bergstrom 1998; Gibson and Mace 2003; Cai and Feng 2005; Song 2012; Hernández-Julián et al. 2014) as well as exposure to environmental pollutants (Williams, Lawson, and Lloyd 1992; Terrell, Hartnett, and Marcus 2011; Sanders and Stoecker 2015; Pavic 2019) have been associated with feminine sex ratios.

The key assumptions in this literature are twofold: First, these shocks or exposures cause maternal stress in various forms, which compromises the pregnancy, increasing the occurrence of fetal loss. Although the exact mechanisms are not well understood, maternal stressors are hypothesized to produce changes in pregnant women’s hormone levels or immunotolerance, for example, which can lead to spontaneous abortion (Fukuda et al. 2019; Pavic 2019). The second assumption is that male fetuses are more vulnerable than female fetuses, and thus fetal loss is disproportionately male.

The latter assertion, that fetal loss is disproportionately male, is not settled in the biological and medical sciences literatures, however. Scholars agree that the natural sex ratio at birth is approximately 105–107 male:100 female live births. There is debate, however, about the primary sex ratio (the sex ratio at conception) and fluctuations in the sex ratio of fetuses throughout the period of gestation (Boklage 2005; Austad 2015).

One interpretation supports the assumption that fetal loss is male on average, and the female survival advantage begins in utero. Scholars holding this view believe that the primary sex ratio is skewed toward males and could be as high as 164:100, although more conservative estimates generally range around 115–130:100 (McMillen 1979; Kellokumpu-Lehtinen and Pelliniemi 1984; Waldron 1998; Pergament, Todydemir, and Fiddler 2002; Tarín et al. 2014). Although more males are conceived than females, male fetuses are more frail and disproportionately lost throughout gestation (McMillen 1979; Kellokumpu-Lehtinen and Pelliniemi 1984; Byrne et al. 1987; Kraemer 2000; Di Renzo et al. 2007; Tarín et al. 2014), resulting in a natural sex ratio of 105–107:100. As such, this interpretation is consistent with research arguing that maternal stressors produce excess male fetal loss, thus feminizing, or lowering, the sex ratio further.

A second interpretation based on recent clinical evidence is that the primary sex ratio is 100:100, with females as likely to be conceived as males. Overall, more females are lost in utero than males on average; however, the timing of the losses differs by sex. Losses in the first weeks after conception are more likely to be male, feminizing the fetal sex ratio, with losses disproportionately female into the second trimester (until about 18 weeks), masculinizing the sex ratio to levels well above 100:100. Thereafter, losses are more likely to be male for about 4 weeks, and then the sex ratio plateaus at the end of the second trimester until losses become male-biased once more in the last weeks of pregnancy. This brings the sex ratio down to 105–107:100 at birth (Boklage 2005; Orzack et al. 2015). Although fetal loss is female on average, this interpretation could also be consistent with observed feminine sex ratios at birth. In this case, maternal stressors could have disproportionate impact in the latter half of gestation, which would result in excess male fetal loss, feminizing the sex ratio at birth.

Studies of exogenous shocks and exposures are insightful and have brought needed attention to the links between various causes of maternal stress, fetal loss, and feminine sex ratios. Nevertheless, such studies are limited to extreme or uncommon events. In contrast, we focus on the association between fetal loss and the decreased odds of a male birth using population-based data from Sub-Saharan Africa, where sex ratios have historically been feminine.

Measuring Fetal Loss with the Length of the Birth Interval

A major challenge to carrying out our inquiry is the measurement of fetal loss at the individual level. Clinical studies are intensive and expensive to carry out for larger samples. For example, studies that follow pregnant women over time to record any spontaneous abortions and their sex are intensive (Kellokumpu-Lehtinend and Pelliniemei 1984; Byrne and Warburton 1987). Survey methods that ask women to self-report spontaneous abortion are prone to reporting error. For example, clinical studies estimate that a large portion of losses occurs in the first seven weeks of pregnancy—well before most mothers confirm their pregnancies (Boklage 1990; Macklon 2002; Jauniaux and Burton 2005; Benagiano, Farris, and Gedis 2010). Thus, women are likely to interpret early fetal loss as delayed menstruation rather than recognize and report it as fetal loss. By some estimates, over 90% of pregnancy losses occur without the knowledge of the mother (Edmonds et al. 1983). Accordingly, rates of fetal loss are much higher in clinical studies (12–70% of all pregnancies (Wood 1994)) than for survey estimates based on self-reports (3–15% of pregnancies (Casterline 1989)). A rigorous demographic analysis of the relationship between fetal loss and the sex ratio requires an indicator of fetal loss that is not dependent on self-reports of its occurrence.

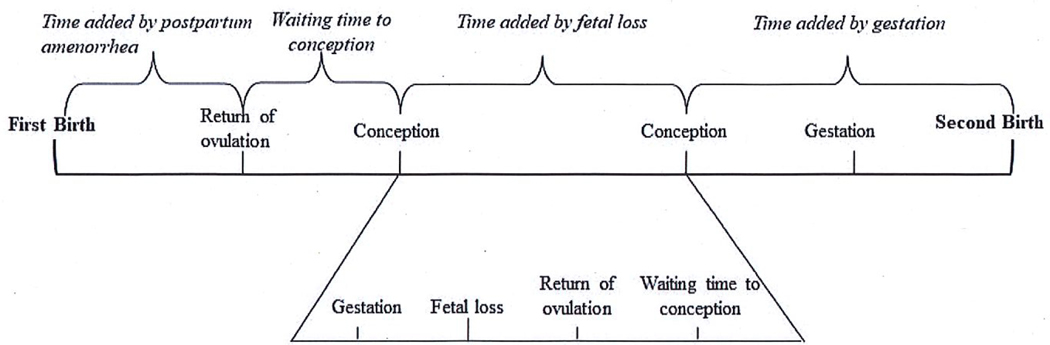
Our strategy is to use the length of the birth interval to infer the occurrence of fetal loss. The length of a birth interval is the sum of four components: postpartum amenorrhea from the previous birth (if any), waiting time to conception, fetal loss (if any), and the period of gestation (see Figure 1) (Potter 1963; Bongaarts and Potter 1983). A fetal loss resets the clock on time to conception and can add a substantive amount of time to the length of a birth interval, ceteris paribus. We hypothesize that women who suffer fetal loss are more likely to successfully carry a female fetus to term, and thus longer birth intervals—which proxy for fetal loss—will be associated with decreased odds of a subsequent male birth and feminine sex ratios at birth.

Figure 1.

Components of the second birth interval

The challenge to using the birth interval as a proxy for fetal loss, however, is isolating the component due to fetal loss. While the duration of gestation is considered constant, the other three components of the birth interval vary between women and between populations. Several demographic and clinical studies have estimated the separate contribution of each of these components to the length of the birth interval (Potter 1963; Stoeckel and Choudhury 1974; Pullum and Williams 1977; Hebert et al. 1986; R.M. Youssef 2005). We attempt to isolate the component due to fetal loss from other determinants of the birth interval through our choices of setting, sample, and control variables, described below.

Data and Methods

Data and Sample

Our main aim is to isolate the occurrence of fetal loss within a birth interval and relate it to the sex of the subsequent birth in a population-based sample. We choose to study Sub-Saharan Africa due to its history of feminine sex ratios at birth. This setting is also advantageous because there is little conscious manipulation of the sex of the fetus. Previous studies have found no evidence of sex-selective fertility behaviors (Filmer, Friedman, and Schady 2009; Basu and De Jong 2010; Fuse 2010; Rossi and Rouanet 2015) or son preference in breastfeeding behaviors (Garenne 2003) in this region. In addition, examinations of survey questions on sex preferences for children confirm that a balanced preference or no preference dominates responses in Sub-Saharan Africa (Fuse 2010).

We utilized data from IPUMS Demographic and Health Surveys (DHS) in multiple Sub-Saharan African countries. The DHS is a cross-sectional survey of a representative sample of women of reproductive age (ages 15–49) that collects information on fertility and maternal and child health, including a complete fertility history. We chose the most recent survey in the last 10 years for all countries in Sub-Saharan Africa that included key variables, including Benin (2011), Burkina Faso (2010), Cameroon (2011), Cote d’Ivoire (2011), Ghana (2008), Guinea (2012), Kenya (2008), Madagascar (2008), Malawi (2010), Mali (2012), Mozambique (2011), Niger (2012), Nigeria (2013), Rwanda (2010), Uganda (2011), Zambia (2013), and Zimbabwe (2010).

We restricted the sample to second live births, which are our units of analysis. Using the second birth limits the amount of selection bias in the sample. In the Sub-Saharan African context, almost all women who have a first birth go on to have a second birth. With each higher birth interval, however, the sample of women becomes less representative, particularly due to infecundity. Use of the first birth interval, by contrast, is imprudent because it is difficult to determine the beginning of the interval (i.e., the onset of exposure to regular intercourse). Both the date of first sex and first union are imperfect markers of the commencement of regular intercourse in the Sub-Saharan African context (Brown 2000). The first birth, however, provides a clearly defined beginning of the exposure period to a second pregnancy. We model the sex of the second birth as a function of the length of the interval between the first and second births.

Across all countries included in the analysis, there were N=281,221 second births. Our first concern was the possibility of event displacement, or misreported dates of first and/or second births. In particular, very short birth intervals could be particularly inaccurate because they are unlikely to occur naturally and could be the result of displacement. Therefore, we dropped second births that corresponded to second birth intervals less than 8 months, which produced an analytic sample of N=279,461 second births.

We then restricted our sample to second births that occurred within 12 months of the survey to ensure that reports of women’s characteristics (such as wealth and urban/rural residence) were contemporaneous to the second birth interval, resulting in N=8,049 second births.

Our next decision was to drop second births to women who practiced conscious spacing behavior in the preceding interval, which would artificially lengthen the birth interval. The DHS does not contain information on recent contraceptive use for all countries in the study; therefore, we relied on information on ever use of contraception. We excluded second births to women who had ever used modern methods of contraception and also to those who reported they ever used breastfeeding, abstinence, withdrawal, or any traditional method as a means to plan their childbearing. After this exclusion, the final analytic sample included N= 4,470 second births.

Variables

The dependent variable in our analysis was the sex of the second live birth (male = 1, female = 0). The main independent variable was the length of the second birth interval, measured by the time between a woman’s first and second births in months.

We chose control variables that would serve to isolate the occurrence of fetal loss. As noted above, a birth interval is composed of four components: postpartum amenorrhea, waiting time to conception, fetal loss, and the gestation period. Assuming the gestation period is constant across women, we needed to account for portions of the birth interval due to postpartum amenorrhea and time to conception. The remaining portion can be attributed to fetal loss.

Postpartum amenorrhea is the period of infertility following each pregnancy, and a large contributor to its variance is the duration of breastfeeding. Unfortunately, information on breastfeeding across DHS data sets is not uniform, and the only common measure was whether the respondent was currently breastfeeding. There was no information recorded on the duration and intensity of breastfeeding following the first birth (during the second birth interval). Therefore, to account for individual-level variation in the duration of breastfeeding, we included an indicator for whether a woman’s first child died before age one or not. The death of an infant leads to cessation of breastfeeding and has been shown to significantly decrease the average length of birth intervals through shortening of the amenorrheic period (Chowdhury, Khan, and Chen 1976; Preston 1976; Grummer-Strawn, Stupp, and Mei 1998). Of the women who had second births in the final analytic sample, survival of the first birth explained 56% of the variation in whether women were currently breastfeeding.

We also included several variables to capture variation in the duration and intensity of breastfeeding practices that results from differences in social norms across countries (Page and Lesthaeghe 1981; Brown 2007), including mothers’ educational attainment, economic status, religion, and urban residence. Educational attainment was measured in three categories: no schooling, primary schooling, and secondary or higher. We categorized economic status with wealth quintiles based on the cumulative living standard index (constructed from selected household assets) included in the DHS data sets. Religion was categorized as Muslim, Catholic, Protestant, Other Christian, Other, and No religion. Protestant included Protestant, Anglican, Methodist, and Other Protestant responses, and all other Christian designations, including Pentecostal and Seventh Day Adventist, were included in the Other Christian category. We included Traditional/spiritual, Traditional, Animist, and Voodoo in Other. We used the DHS categories of residence as urban or rural. We also included a dummy variable for each country.

We accounted for variance in the time to conception by including measures related to fecundability and coital frequency. Fecundability is the monthly probability of conception in the absence of contraception. Fecundability declines with age (Wood 1989; Weinstein, James, and Chang 1993), and we controlled for the age of the mother in years. Limiting the sample to second births and considering the second birth interval also accounted for parity-specific effects on fecundity (Wood 1994). There is no information on coital frequency over time in the DHS surveys. The DHS includes information on time since last sexual intercourse; however, we do not use this variable. Women who had second births in our analytic sample had given birth in the last 12 months, and coital frequency in this postpartum period cannot be generalized to coital frequency during the second birth interval. Instead, we included control variables that are correlated with coital frequency, including mothers’ current marital status, partner residency, and the duration of the union (James 1983; Weinstein et al. 1993; Brewis and Meyer 2005). Marital status included the following categories: monogamous marriage, polygamous marriage, non-marital union, and no union. Residential partner was coded dichotomously as yes/no. Union duration was measured in years.

Although, as noted, evidence suggests there is no sex selective fertility behavior in Sub-Saharan Africa, we nevertheless controlled for a woman’s ideal proportion of sons as well as the sex of the first birth. We used ideal proportion of sons rather than ideal number of sons, because ideal number of sons was highly correlated with ideal number of daughters. In other words, women who wanted many sons appeared to want many children in general. Thus, ideal proportion of sons captures sex preferences rather than family size preferences. We categorized ideal proportion of sons as none, more than none/less than half, half, more than half/less than three quarters, and more than three quarters. The sex of the first birth was coded male = 1, female = 0.

There were few variables with missing values in the data set. Among the original sample of 281,221 second births, there were no missing values on the dependent or main independent variables. Among the controls, religion had the highest number of missing values (over 17,000 observations) and marital status the second highest (over 2,000 observations). Ever use of contraception had four missing values and education had 30. The remaining controls had no missing values. To address item non-response, we used multiple imputation assuming multivariate normal distributions for missing variables (Allison 2001). We imputed before applying our exclusion restrictions. We generated 25 imputations.

Plan of Analysis

We carried out our analyses in several steps. First, we produced descriptive statistics for our analytic sample as well as for all second births for comparison purposes. Second, we visually examined the relationship between the sex of the second birth and the duration of the second birth interval with a histogram. Third, we estimated a logistic regression of the probability of a male birth by the length of the birth interval, adding controls in a stepwise manner for indicators of the length of postpartum amenorrhea, coital frequency, fecundity, sex preferences, and country. Fourth, to illustrate the implications of the results for population-level sex ratios at birth, we translated the predicted odds of a male birth into predicted sex ratios at birth for various lengths of the birth interval. Lastly, we performed multiple robustness checks.

Results

Table 1 presents descriptive statistics for our analytic sample of second births and for the full sample of second births. Among all second births, 51.1% are male, translating into a sex ratio at birth of 104.5:100, which is largely consistent with literature finding lower than natural sex ratios at birth in Sub-Saharan Africa. Among the second births in the analytic sample, 51.2% are male, indicating a sex ratio of 105:100. The mean birth interval for second births is 38.6 months (3 years, 2 months).

Table 1:

Descriptive statistics of intitial and analytic sample

	Initial Sample (All second births)		Final sample (second births born to women who had not used contraception or spaced births, and interviewed within 12 months after birth)
	Mean	SE	Mean	SE
Sex of second birth	0.511	0.500	0.512	0.500
Second birth interval (in months)	36.681	33.006	38.601	23.298
Death of first birth (within 1st year)	0.118	0.323	0.109	0.311
Mother’s education
No education	0.354	0.478	0.505	0.500
Primary	0.325	0.469	0.306	0.461
Secondary plus	0.321	0.467	0.189	0.392
Mother’s wealth quintile
Poorest	0.183	0.387	0.241	0.428
Poorer	0.185	0.388	0.225	0.418
Middle	0.192	0.394	0.203	0.402
Richer	0.205	0.404	0.184	0.387
Richest	0.235	0.424	0.147	0.354
Mother’s religion
Muslim	0.306	0.461	0.442	0.497
Catholic	0.206	0.405	0.184	0.388
Protestant	0.184	0.387	0.126	0.331
Other Christian	0.238	0.426	0.169	0.375
Other	0.032	0.176	0.035	0.183
No religion	0.034	0.181	0.044	0.205
Urban	0.345	0.476	0.274	0.446
Age	28.498	9.462	23.314	4.222
Age at 2nd birth	22.146	4.067	22.978	4.184
Mother’s marital status
Monogamous	0.431	0.495	0.598	0.490
Polygamous marriage	0.168	0.374	0.192	0.394
Non marital union	0.076	0.266	0.114	0.318
Not in a union	0.325	0.468	0.096	0.295
Duration of union (in months)	157.981	106.641	62.438	33.637
Sex of first birth	0.514	0.500	0.513	0.500
Mother’s ideal proportion of sons:
None	0.150	0.357	0.157	0.364
Less than 1/2	0.158	0.365	0.154	0.361
1/2	0.431	0.495	0.418	0.493
More than 1/2	0.180	0.384	0.201	0.401
More than 3/4	0.017	0.128	0.017	0.129
Non-numeric response	0.065	0.246	0.052	0.223
Mother’s total children ever born	2.961	2.830
Mother’s ever use of contraception	0.368	0.482
Total N	281,221		4,470

With respect to additional characteristics in Table 1, mothers of second births in the analytic sample are younger, poorer, less educated, more likely to be in a union, and more likely to be Muslim than mothers of all second births, likely because the second birth sample is restricted to women who had recently had a second birth and have never used contraception. In addition, mothers in both samples appear to display little son preference; approximately 42% desire equal numbers of sons and daughters, 31% desire more daughters than sons, and 21% more sons than daughters.

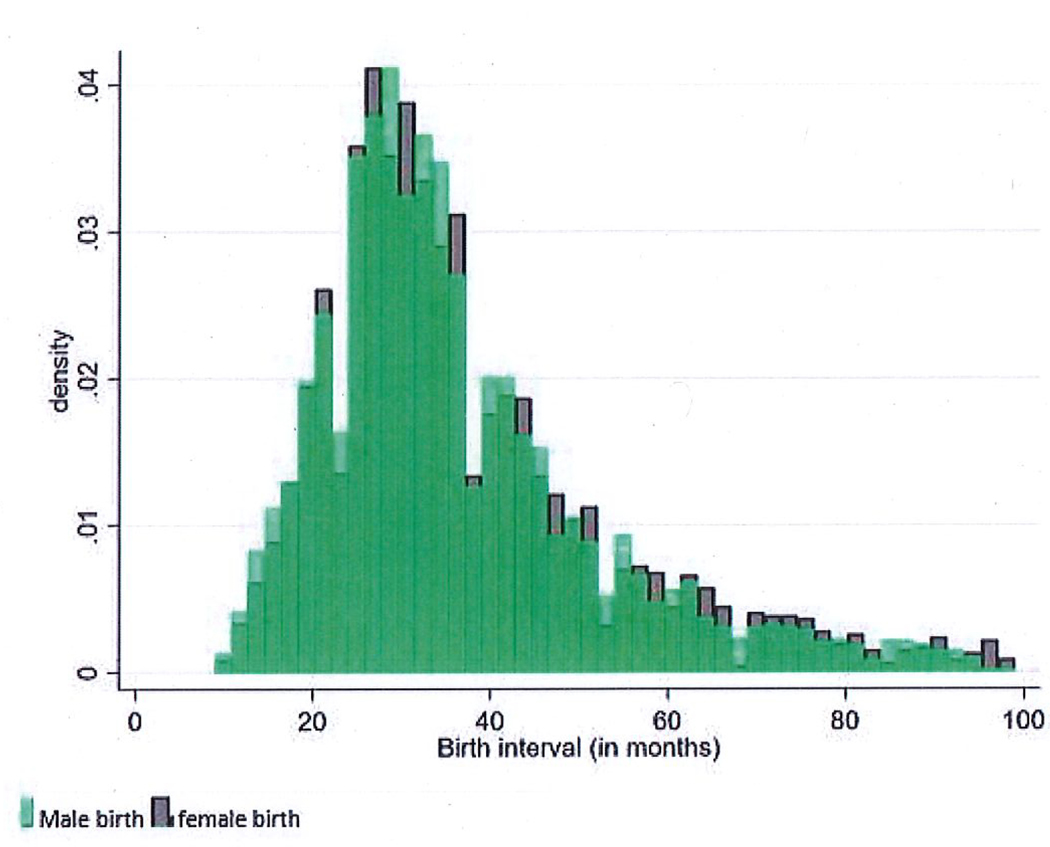
Figure 2 shows a histogram of second birth intervals from the analytic sample for male and female second births. Recall, we limited our sample to second birth intervals that were 8 months or longer. We find a unimodal and slightly right-skewed distribution of birth interval lengths overall. There is a higher proportion of male births with short birth intervals (approximately 8 to 24 months), near parity for births between approximately 24 and 56 months, and a higher proportion of female births at very long birth intervals (56+ months).

Figure 2.

Histogram of male and female births by duration of birth interval

Table 2 presents results of logistic regression models of the association between the length of the second birth interval and the sex of the second birth. Results are presented as odds ratios. An odds ratio less than one indicates lower odds of a male birth. The bivariate association between the duration of the birth interval and the likelihood of a male birth in Model 1 is negative but not statistically significant. The remaining models add sets of control variables: Model 2 introduces control variables relating to postpartum amenorrhea, Models 3 adds controls for waiting time to conception, Model 4 adds controls for sex preferences, and Model 5 adds country of residence dummies. Across Models 2 to 5, the relationship between length of the birth interval and odds of a male birth is negative and statistically significant. We also estimated specifications with various functional forms of the birth interval to test for nonlinearities in the relationship between length of the birth interval and odds of a male birth. We found that squared and cubed terms of the birth interval variable were not significant, however.

Table 2:

Logit models predicting odds of a male birth to women who have had a 2nd birth in last 12 months and never used contraception

	Model 1		Model 2		Model 3		Model 4		Model 5
	OR	SE	OR	SE	OR	SE	OR	SE	OR	SE
Birth interval	0.998	0.001	0.997	0.001 *	0.995	0.002 **	0.995	0.002 **	0.995	0.002 **
Post partum amenorrhea
Death of first birth (within 1st year)			0.831	0.085	0.852	0.090	0.846	0.090	0.850	0.090
Mother’s education (no education=reference)
Primary			1.100	0.088	1.151	0.095	1.167	0.097	1.177	0.107
Secondaiy plus			1.015	0.101	1.036	0.108	1.048	0.110	1.095	0.126
Mother’s wealth quintile (poorest = reference)
Poorer			1.128	0.104	1.109	0.105	1.090	0.103	1.101	0.105
Middle			1.092	0.106	1.112	0.110	1.079	0.108	1.087	0.110
Richer			1.006	0.109	0.943	0.104	0.906	0.101	0.899	0.103
Richest			0.931	0.125	0.901	0.126	0.858	0.121	0.850	0.124
Mother’s religion (Muslim = reference)
Catholic			0.867	0.079	0.862	0.084	0.850	0.083	0.832	0.091
Protestant			0.995	0.108	1.006	0.117	1.000	0.117	0.959	0.136
Other Christian			0.992	0.096	1.007	0.103	0.997	0.103	0.947	0.107
Other			0.854	0.150	0.852	0.154	0.840	0.153	0.794	0.153
No religion			0.796	0.127	0.832	0.138	0.810	0.135	0.761	0.142
Urban			1.115	0.102	1.133	0.108	1.161	0.111	1.154	0.113
Time to conception
Age at second birth					1.011	0.009	1.010	0.009	1.007	0.010
Mother’s marital status (monogamous marriage = reference)
Polygamous marriage					0.951	0.082	0.942	0.082	0.954	0.084
Non marital union					0.973	0.101	0.969	0.101	1.000	0.111
Not in a union					0.817	0.115	0.813	0.115	0.821	0.119
Duration of Union					1.001	0.001	1.001	0.001	1.001	0.001
Mother’s sex preferences
Sex of first birth							0.881	0.057	0.873	0.057
Desired proportion of sons (half = reference)
None							0.987	0.071	1.002	0.074
Less than 1/2							0.748	0.053 **	0.760	0.054 **
More than 1/2							1.262	0.084 **	1.288	0.087 **
More than 3/4							3.083	0.723 **	3.027	0.714 **
Non-numeric response							0.892	0.115	0.899	0.117
Mother’s country (Nigeria = reference)
Cameroon									0.777	0.125
Benin									1.141	0.160
Ghana									1.176	0.315
Guinea									1.060	0.159
Cote d’Ivoire									0.860	0.164
Kenya									1.815	0.466 *
Madagascar									1.071	0.211
Malawi									1.011	0.181
Mali									0.875	0.128
Mozambique									1.110	0.167
Rwanda									0.987	0.241
Zimbabwe									0.880	0.376
Uganda									0.872	0.174
BurkinaFaso									0.998	0.129
Zambia									1.010	0.234
Constant	1.155	0.068	1.153	0.105	0.908	0.177	0.951	0.206	1.015	0.230

¹Niger was dropped from this analysis due to multicollinearity.

Note: OR is the odds ratio; SE is the standard error.

Source: As for Table 1.

^*p < 0.05

^**p < 0.01.

Overall, few controls are significant with the exception of Kenya, where second births are more likely to be male than in Nigeria, the reference category, and sex preferences. Compared to mothers who desired an equal number of sons and daughters, those who wanted more sons are more likely to have had a male birth, and those who wanted fewer sons are less likely to have had a male birth; those who wanted no sons or gave a non-numeric response are no different than the reference category. A likely explanation for the relationship between sex preferences and the odds of a male birth is post factum rationalization, whereby women make an adjustment in their reported sex preferences that is closer or equal to the actual sex composition of their children (Bongaarts 1990, Gibby and Luke 2019).

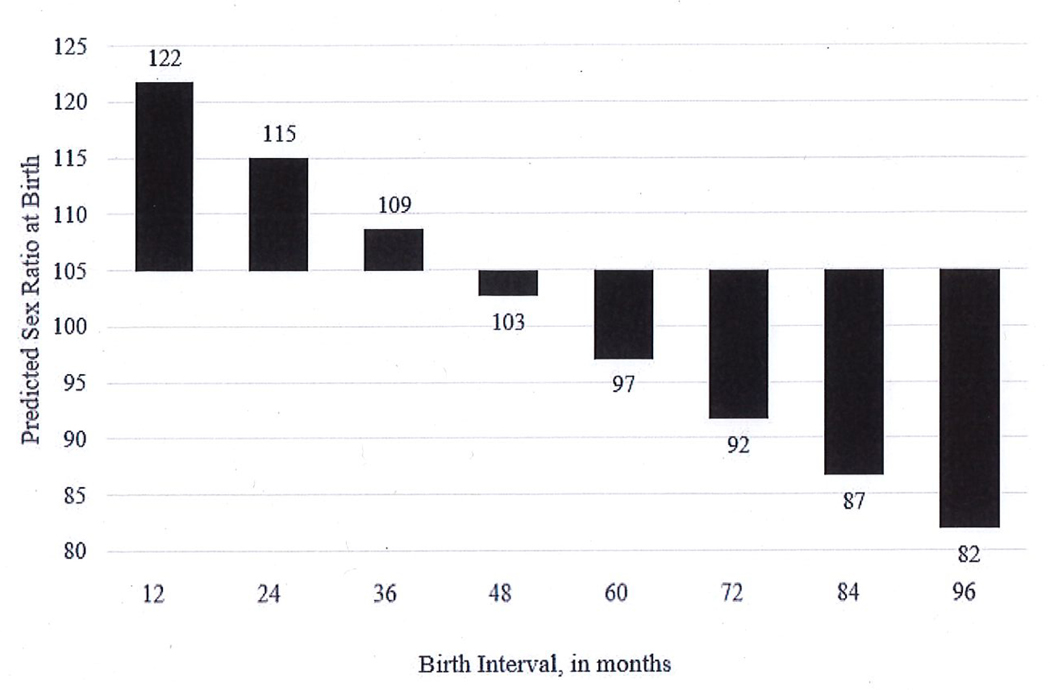
With respect to the key independent variable, Model 5 reveals that an increase in the birth interval of one month decreases the likelihood of a male birth by 0.5%. This seemingly small effect per month has a large impact on the sex ratio at birth at the population level. We converted the predicted odds of a male birth from Model 5 into predicted sex ratios at birth, (pr(male)/pr(female))*100, for various lengths of birth intervals in Figure 3.

Figure 3.

Predicted values of the sex ratio at birth at various lengths of the birth interval, based on Model 5

Assuming a short birth interval of 12 months, which has a low probability of fetal loss, we estimate the sex ratio at birth as 122 males:100 females. The ratio is quite high; however, the rarity of births at this short interval prevents the average sex ratio in the population from reaching this level. The predicted sex ratio at birth for the mean birth interval (38 months) is 105:100 which is close to the sex ratio at birth for the sample. For birth intervals longer than approximately four years, the predicted sex ratio at birth drops below the natural sex ratio at birth 105–107:100. A birth interval of eight years (96 months) produces a predicted sex ratio of 82:100.

Robustness Checks

We were concerned that our results could be sensitive to the restrictions we placed on our analytic sample. Indeed, we see in Table 1 that in our analytic sample, mothers are generally of lower socioeconomic status than mothers of all second births. It could be that the analytic sample represents the types of women who are more likely to experience maternal stress or other conditions that produce fetal loss, which results in a more feminine sex ratio at birth than in the general population. We carried out multiple additional analyses to investigate this possibility. In each, we used the same specification as Table 2 Model 5.

First, our analytic sample was restricted to second births that occurred within 12 months of the interview to ensure that mothers’ and birth characteristics were contemporaneous. This also reduced reporting error due to recall bias. We relaxed this restriction and estimated models for second births up to 24 months before the interview (N= 7,765) as well as no restriction on length of the reference period (N=279,461). We tested these alternate samples with imputed data. We found that the coefficient on the length of the birth interval is similar in magnitude and consistent in direction across all samples. The statistical significance, however, varied across the samples. We also used a sample with list-wise deleted, non-imputed data and found the same relationship between birth interval and the likelihood of a male birth.

Second, our analytic sample was also restricted to second births from mothers who had never used contraception or means of birth spacing, whereas in the full sample of second births, approximately 37% of mothers had used some method (Table 1). We relaxed this restriction (N= 7,083 second births) and found that the coefficient on the length of the birth interval is similar in magnitude and maintained significance as in the original analysis. We had expected precision to decrease once we added contraceptive users to the sample, given that associated birth intervals could thereby be artificially lengthened; however, the addition of contraceptive users almost doubled the sample size, which resulted in an apparent countervailing increase in precision. Taken together, these robustness tests regarding sample restrictions support the view that our results are generalizable to the entire sample of second births.

A related question is the role of socioeconomic factors and the possibility that women of lower education or economic position, for example, are more likely to suffer from maternal stressors that lead to male fetal loss and the decreased likelihood of a male birth. Previous research has found that lower levels of maternal education decrease the likelihood of male births (Almond and Edlund 2007; Grech 2018). To investigate this possibility in Sub-Saharan Africa, we estimated models that included interaction terms between both education levels and wealth quintiles and the length of the birth interval. None of the interaction terms in these specifications are statistically significant and the standard errors are too large to indicate directionality of the coefficients.

Discussion

A large body of demographic research focuses on the masculinization of sex ratios at birth in countries across East, South, and Western Asia (Gupta, Chung, and Shuzhuo 2009; Alkema et al. 2014; Bongaarts and Guilmoto 2015). Less attention has been paid to contexts displaying feminine sex ratios at birth. A recent body of work examines the role of fetal loss, or spontaneous abortion, in producing feminine sex ratios at birth by considering the impact of exogenous occurrences, such as famines or terrorist attacks, which are believed to cause maternal stress that prompts male fetal loss. These studies focus on more extreme conditions, and therefore raise concerns about the generalizability of their findings (Maccini and Yang 2009).

Our contribution is to examine the role of fetal loss in producing feminine sex ratios at birth under more common or widespread conditions. We use population-based data from 17 Sub-Saharan African countries, where sex ratios have historically been skewed toward females. We also employ a unique strategy and use the length of the birth interval to proxy for the occurrence of fetal loss at the individual level. We find a negative and statistically significant relationship between the length of the second birth interval and the odds of a subsequent male birth, which suggests that fetal loss has a meaningful impact on the sex ratio of live births. We used our micro-level results to estimate population sex ratios at birth by various birth interval lengths. We find that short birth intervals are associated with more masculine sex ratios at birth, while longer intervals produce increasingly feminine sex ratios at birth.

Our results suggest that longer birth intervals are associated with male fetal loss and that women who experience longer birth intervals are more likely to successfully carry a subsequent female fetus to term. These findings appear to uphold the view that fetal loss is disproportionately male; however, several recent studies suggest that fetal loss is female on average, skewed by disproportionate female loss early in gestation, with male fetal loss more common in the later stages. It is likely the case that excess female fetal loss in early gestation has little impact on birth interval length, and thus our measure chiefly picks up male fetal deaths that occur at moderate to long birth interval lengths. Future research should continue to explore fetal loss and its determinants at various gestational lengths and resulting fetal sex ratios.

The current study is not without limitations. One issue is the accuracy of second birth interval lengths, which are based on mothers’ retrospective reports of first and second birth dates. Analysis of birth date data in the DHS has found overall accurate reporting, particularly for more recent surveys, although surveys in Sub-Saharan Africa tend to have the highest levels of misreporting (Pulman and Becker 2014; Shoumaker 2014). Our concern, however, is if misreporting systematically differs by the sex of the second birth, which could bias our estimates. A study of two Bangladeshi data sets, including the DHS, found no significant differences in displacement of birth dates by sex of the live birth (Espeut and Donna 2015), however, which supports the view that misreporting of birth dates does not vary by sex.

We aimed to isolate the portion of the second birth interval due to fetal loss by controlling for other birth interval components such as postpartum amenorrhea and waiting time to conception. However, DHS surveys do not contain direct measures of the length of postpartum amenorrhea or of coital frequency following the first birth. Therefore, we used available controls for related demographic characteristics of mothers and their unions and households. More accurate measures of postpartum amenorrhea, breastfeeding, fecundity, and coital frequency could more precisely estimate the relationship between the length of the birth interval and the odds of a male birth.

A related issue is that coital frequency has been linked to the sex ratio at birth (e.g., James 2008). Several scholars posit that couples who have intercourse near the day of ovulation are more likely to conceive males (Shettles and Rorvik 2006). Higher coital frequency would increase the likelihood of male conceptions and sex near ovulation and also reduce the time to conception, and hence shorten the birth interval. Therefore, an alternative explanation for our findings is that coital frequency determines birth interval length and odds of a male birth, not fetal loss. The literature on coital frequency and sex ratios at birth is contested, however. Additional research in this area finds that conception near the day of ovulation produces more females (France et al. 1984), and others find no relationship (Wilcox, Weinberg, and Baird 1995). Further studies with accurate data linking coital behavior to sex of the fetus would help determine if this alternative explanation is plausible.

We also uncovered a minority of second birth intervals that were quite long despite assumed exposure to regular intercourse and nonuse of contraception. Fourteen percent of intervals were longer than five years and were thereby associated with a very low likelihood of a male birth. One potential explanation for these long birth intervals is low fecundity, which we did not entirely control for with women’s age in our models. In other words, some women could have longer birth intervals because they are less fecund and not because of a higher likelihood of fetal loss. Some research suggests, however, that a large portion of what appears to be infecundity or sterility results not from the inability to conceive but rather from repeated fetal loss (Leridon 1976; Macklon 2002). Other work also suggests that fetal loss and low fecundity coincide ( Wood 1994; Hakim, Gray, and Zacur 1995). Further, there is no evidence that we know of linking low fecundity with increased odds of a female birth. Thus, it is likely that very long birth intervals occur to women who suffer repeated fetal loss and have particular difficulty carrying a male fetus to term. This interpretation also bolsters our argument that male fetal loss is associated with a subsequent female birth.

We also tested for variation in the relationship between birth interval length and the odds of a male birth by women’s education levels and household wealth, under the assumption that very poor socioeconomic conditions would produce heightened maternal stress. We did not find statistically significant relationships by these socioeconomic measures, however. Several studies reveal parallel findings among African Americans and Native Americans in the U.S., who display lower sex ratios at birth than native Whites (Matthews and Hamilton 2005; Grech 2017) but show no differences across education levels within these groups (Grech 2019). Taken together, these results suggest that these minority populations in the U.S. as well as women across Sub-Saharan Africa face a host of contextual issues that lead to widespread poor maternal health and stress, which results in higher levels of fetal loss within these populations.

Several scholars have argued that disproportionately low sex ratios at birth are a sentinel health indicator (Bruckner, Catalano, and Ahern 2010; Terrell et al. 2011; Masukume et al. 2017) for poor maternal and fetal health. We did not measure the maternal stressors that could cause elevated fetal loss in Sub-Saharan Africa; however, our results suggest that documented chronic conditions, such as malnutrition or lack of adequate prenatal care, or shorter-term stressors, such as armed conflicts or forced migration, should be examined for their connections to fetal loss. The results of such research as well as our own would help justify policies and programs aimed at improving maternal health before and during pregnancy across the continent.