Abstract
Porous germanium is a promising material for sensing applications in the mid-infrared wavelength range due to its biocompatibility, large internal surface area, open pores network and widely tunable refractive index, as well as its large spectral transparency window ranging from 2 to 15 μm. Multilayers, such as Bragg reflectors and microcavities, based on porous germanium material, are designed and their optical spectra are simulated to enable SF6 gas-sensing applications at a wavelength of 10.55 µm, which corresponds to its major absorption line. The impact of both the number of successive layers and their respective porosity on the multilayer structures reflectance spectrum is investigated while favoring low layer thicknesses and thus the ease of multilayers manufacturing. The suitability of these microcavities for mid-infrared SF6 gas sensing is then numerically assessed. Using an asymmetrical microcavity porous structure, a sensitivity of 0.01%/ppm and a limit of detection (LOD) around 1 ppb for the SF6 gas detection are calculated. Thanks to both the porous nature allowing gases to easily infiltrate the overall structure and Ge mid-infrared optical properties, a theoretical detection limit nearly 1000 times lower than the current state of the art is simulated.
Keywords: porous germanium materials, mid-infrared detection, Bragg reflector, optical microcavity
1. Introduction
Germanium (Ge) is a very promising material for spectroscopy and sensing applications in the mid-infrared (Mid-IR) wavelength range. Ge is advantageous because of its special physico-chemical properties [1,2]. In particular, its large spectral transparency window ranging from 2 to 15 μm, covering the whole molecule fingerprint, and its high refractive index allows the implementation of devices with reduced footprint. In recent years, numerous Ge-based fundamental devices have been developed using Ge-on-silicon [3,4], Ge-on-silicon-on-insulator [5], Ge-on-Si3N4 [6], and germanium-on-insulator [7] platforms.
Porous materials are attractive materials for many different sensing applications because of their large internal surface area [8,9], open pores network [10], and widely tunable refractive index [11]. Several structures using porous materials, especially porous silicon (PSi), have been demonstrated, such as omnidirectional mirrors [12], multilayers [13], microcavities [14], and waveguides [15]. The potential application areas of porous materials are mainly in the fields of biotechnology [16], microelectronics [17], and energy conversion [18]. These porous materials are promising candidates for environmental monitoring applications as they enable increased interaction between the wave and the molecules to be detected, which enhances sensor performance [19,20,21]. Porous silicon (PSi) sensing devices have been demonstrated to display greater sensitivities and lower limits of detection compared to massive materials in the near infrared (NIR) wavelength range [22,23]. Compared to silicon (Si), whose transparency window ranges from 1 to 8 μm, porous Ge (PGe) benefits from the larger transparency window of Ge extending from 2 to 15 μm. Furthermore, the strong light–matter interaction, conferred by its pores network, has attracted a growing interest for its use in various integrated detection applications [15,24].
This work is a contribution to the spectroscopic sensing of sulfur hexafluoride (SF6) gas whose Mid-IR signature monitoring could enable the quantification of its releases in the atmosphere [25]. This gas is mainly released by the electrical industry, which uses it as a gaseous insulator, and its concentration is rapidly increasing in the atmosphere. It is a powerful greenhouse gas listed in the Kyoto Protocol [26]. In its gaseous form, SF6 has a main absorption peak in the mid-infrared at 10.55 μm [27]. Using 2D material-based sensors, several techniques have been applied to SF6 gas detection: absorption of Ru-doped MoS2 (Ru-MoS2) [28], detection and absorption of InN doped with Ru (Ru-InN) [29], and detection Ni-doped C3N (Ni-C3N) [30]. An Ni-modified carbon nanotube (Ni-CNT) gas sensor was also implemented for SF6 detection and demonstrated a 1 ppm experimental limit of detection [31].
Leveraging from PGe advantages for Mid-IR sensing applications, the aim of the paper is to numerically study vertical optical structures based on PGe such as Bragg reflectors and microcavities dedicated to SF6 gas sensing. The study is first carried out on Bragg mirrors by varying both the number of layers N and the contrast between the low and high porosities of these layers to achieve a maximum reflectance R. Then, the study is focused on microcavities, which are the superposition of Bragg mirrors already studied, in order to define the numbers and porosities of the layers to get an optimal microcavity spectral response. In particular, the use of asymmetric microcavities is studied. Finally, a SF6 gas sensing study is performed based on the designed microcavities. Theoretical sensitivity and limit of detection are finally calculated and compared to the state of the art.
2. Modeling
The optical response of porous layers and their sensitivity to SF6 absorption strongly depend on the properties of a single layer (thickness, refractive index related to porosity, and pores size), and on the chosen optical multilayer structure (Bragg reflector or microcavity) fabricated using a stack of two different porous layers. The transfer matrix method was used to calculate the reflectance spectrum and to study the optical response as a function of the porous layer physical parameters (porosity, thickness, and number of layers) [32].
The refractive index of each layer is a key element to obtain the reflectance spectrum of the multilayered structures. A PGe layer of porosity p consists of germanium crystallites and open pores into which air infiltrates. The SF6 present in the air will therefore also penetrate the porous layer. Consequently, the complex refractive index of the PGe layer depends on its porosity p and on the superstrate consisting of air and SF6 with a concentration C. The presence of SF6 just influences the imaginary part k of the complex refractive index of the superstrate. In the presence of gas, nsup, which is the superstrate refractive index, is written in the form:
| (1) |
with , where λ is the wavelength, and ε(λ) is the absorption coefficient of SF6 in air.
The absorbance of SF6 in the Mid-IR wavelength range for different SF6 concentrations was extracted from the Hitran database [33]. The mid-IR complex refractive index of Ge, nGe, was taken from [34,35].
The PGe refractive index, n, calculation was then performed, as a function of the PGe porosity and for different SF6 concentrations, using the Bruggeman model [36]:
| (2) |
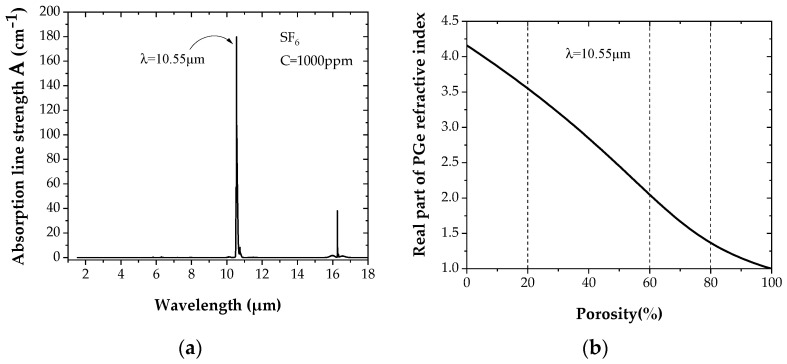
The absorbance (A = Cε) spectrum for a SF6 concentration in air of C = 1000 ppm [37] is reported in Figure 1a. A strong SF6 absorption peak at a wavelength of 10.55 μm is observed. The transparency of germanium for such wavelengths is expected, hence validating its selection instead of silicon.
Figure 1.
(a) Mid-IR absorbance spectrum of SF6 (for a SF6 concentration of 1000 ppm in air). (b) Dependence of the real part of the PGe refractive index on porosity.
Figure 1b reports the strong dependence on the real part of n, the PGe refractive index on layer porosity at 10.55 μm, which allows multilayers with a high refractive index contrast to be obtained thereafter. The refractive index n obviously decreases with porosity. By selecting a given degree of porosity, a precise corresponding refractive index value n can be obtained from Figure 1b.
2.1. Bragg Reflector Theory
Bragg reflectors consist of periodic dielectric layers, with a quarter wavelength-optical path length for each layer giving them important properties and making them suitable for optoelectronics applications such as filters or laser cavity. It consists of a stack of alternating thin dielectric layers with high and low refractive indices. The reflectivity of the mirror is characterized by a stop band of high reflectivity. The pattern is formed by two layers of high and low porosities (named HP and LP, respectively) which is repeated N times. Each layer is characterized by its thickness e and its porosity, i.e., its refractive index. The Bragg reflector is characterized by its central wavelength λ0 (at normal incidence) and by the reflection bandwidth Δλ which is determined mainly by the index contrast. These two parameters are defined, respectively, by Equations (3) and (4).
| (3) |
| (4) |
| (5) |
where nHP and eHP are, respectively, the refractive index and the thickness for the HP layer, nLP and eLP for the LP layer, and .
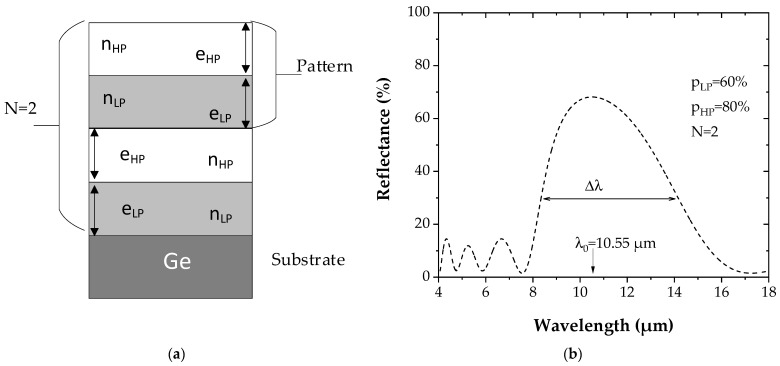
Figure 2a represents a schematic Bragg reflector with a pattern composed of low and high porosity successive layers and repeated twice (N = 2).
Figure 2.
(a) Schematic of a PGe Bragg reflector made of a repeated pattern constituted by alternating a layer of low porosity (refractive index nLP and thickness eLP) and a layer of high porosity (refractive index nHP and thickness eHP). In this case, the pattern is repeated twice (N = 2). (b) Calculated reflectance spectrum of the corresponding PGe Bragg reflector, with values of 60% and 80% for the low and the high porosities, respectively.
The reflectance R of a Bragg mirror structure corresponding to Figure 2a with LP and HP layers having, respectively, porosities pLP of 60% (corresponding to refractive index nLP = 2.048) and a pHP of 80% (corresponding to refractive index nHP = 1.367), is reported in Figure 2b. Its maximum value depends on the refractive index of each layer and on the number N of the patterns according to:
| (6) |
2.2. Microcavity Theory
PGe planar microcavities have also been simulated. The microcavity is constituted of two distributed Bragg reflectors (DBRs) with a Fabry–Perot cavity thickness of λ0/2 in the middle (Figure 3a). Several alternating patterns of PGe layers of different refractive indices, repeated N times, constitute the DBRs. For the DBRs, the optical thickness of each single-layer equals λ0/4, according to the following relation:
| (7) |
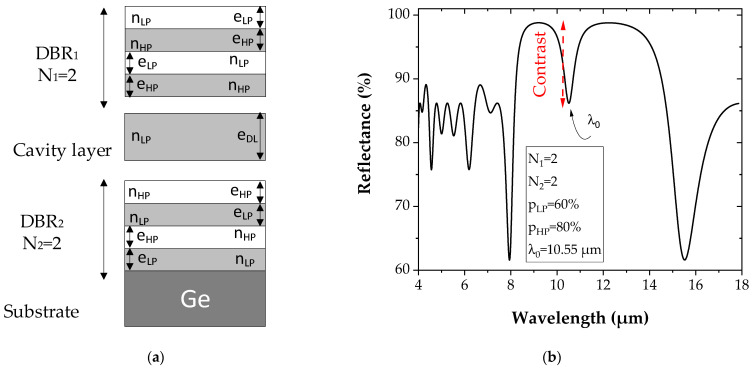
Figure 3.
(a) Schematic of a PGe microcavity reflector constituted of a Fabry–Perot layer cavity of low porosity (refractive index nLP and thickness eDL) sandwiched by two DBRs. The two DBRs are made of a pattern of low (60%) and high porosity (80%) layers with a refractive index nLP and nHP and a thickness eLP and eHP, respectively, and repeated in this case two times. (b) Calculated reflectance spectrum of the PGe microcavity reflector with values of 60% and 80% for the low and the high porosities, respectively.
In the case of a microcavity, the relation becomes:
| (8) |
A typical reflectance spectrum of a microcavity (Figure 3b) is characterized by a sharp dip in the stop band of the DBR, corresponding to the resonant wavelength λ0 of the cavity. This difference between the reflectance level represents the contrast, which is defined by the difference between the maximum (Rmax = 99%) and minimum (Rmin = 86%) of the reflectance.
The resonance properties of the microcavity is characterized by the quality factor of the structure. In order to study this parameter, the classical Fabry–Perot can be used [38]. The quality factor (Q) is thus given by the following equation:
| (9) |
where n0 is the refractive index of the resonant cavity layer that separates the two Bragg mirrors, , the thickness of the cavity layer, λ0 is the expected resonant wavelength equal to 10.55 µm, A is the absorption of the molecule, and R is the reflectance value.
3. Results and Discussion
3.1. Bragg Reflector
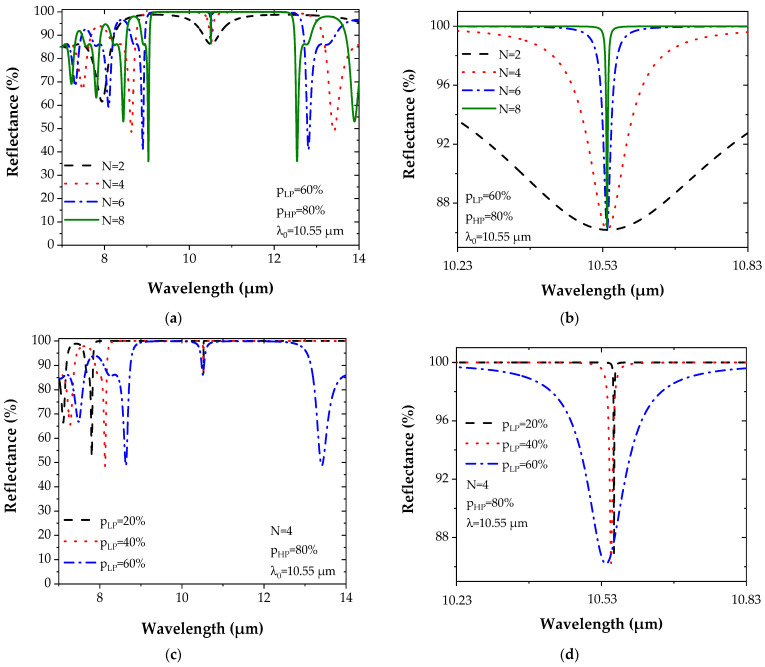
The objective of this part is to study the influence of two parameters on reflectance: the contrast between the high and low porosities of the multilayers and the number N of layers. The upper layer of the period is the one which has the higher porosity.
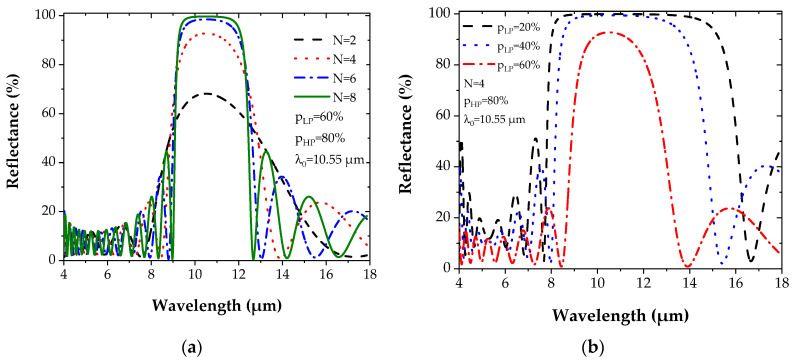
Two structures are proposed. The first structure is composed of pairs of layers whose high porosity is pHP = 80%, while the low porosity is pLP = 60% and the number N of layers is varied to study its impact on the reflectance evolution (Figure 4a). For the second structure, the number N of layers is fixed to four, while the low porosity value pLP varies from 20% to 60%, whereas pHP is constant and equal to 80% (Figure 4b). To keep the optical thickness of each layer constant and have a central wavelength λ0 at 10.55 µm (Equation (7)) in all simulations, the thicknesses and the refractive indices are chosen according to Table 1:
Figure 4.
(a) The Bragg reflector for PGe materials with (a) porosities pLP = 60% and pHP = 80% while varying N. (b) Reflectance spectrum when N is fixed to 4, porosities pHP = 80%, and varying pLP.
Table 1.
The calculation of the thickness e and the refractive index n for different values of porosity p to obtain λ0/4 with λ0 = 10.55 µm.
| p (%) | 20 | 40 | 60 | 80 |
| n | 3.549 | 2.844 | 2.048 | 1.367 |
| e (nm) | 743 | 927 | 1288 | 1929 |
Table 1 shows that the layer thickness e increases with the layer porosity.
Thus, pHP is set to 80%, and the Bragg reflector total thickness is smaller for lower pLP, and also for a high porosity contrast Δp between the two pattern layers. These results also highlight the interest in Ge which, in addition to having a wider transparency, offers much lower layer thicknesses than low refractive indices materials [34].
Figure 4 represents the evolution of the reflectance spectrum for the Bragg mirror by varying either the number N of layers or the degree of porosity pLP (pHP fixed at 80%) and by taking into account the Ge complex refractive index dispersion [35].
The variation of the number N of layers (2 to 8) induces an increase of the maximum reflectance Rmax, which approaches 100% for N = 8 while narrowing the band (Figure 4a). The same observation is noticed with the increase of the contrast between the two porosities pLP and pHP, but with a widening of the band (Figure 4b).
From Figure 4a, it can also be noticed that a maximum reflectance (100%) is quickly reached with a small number of layers when the contrast Δp between the two porosities pHP and pLP is high. These results show the enormous potential of PGe for the fabrication of optical devices based on multilayer structures. Compared to multilayers based on other materials, the maximum reflectance was achieved for a much lower number of layers [34,39,40].
3.2. Microcavity
3.2.1. Optimization of the Structure
The microcavities are made up of Bragg reflected mirrors studied in the previous section and of a resonant cavity centered at 10.55 µm for SF6 gas detection.
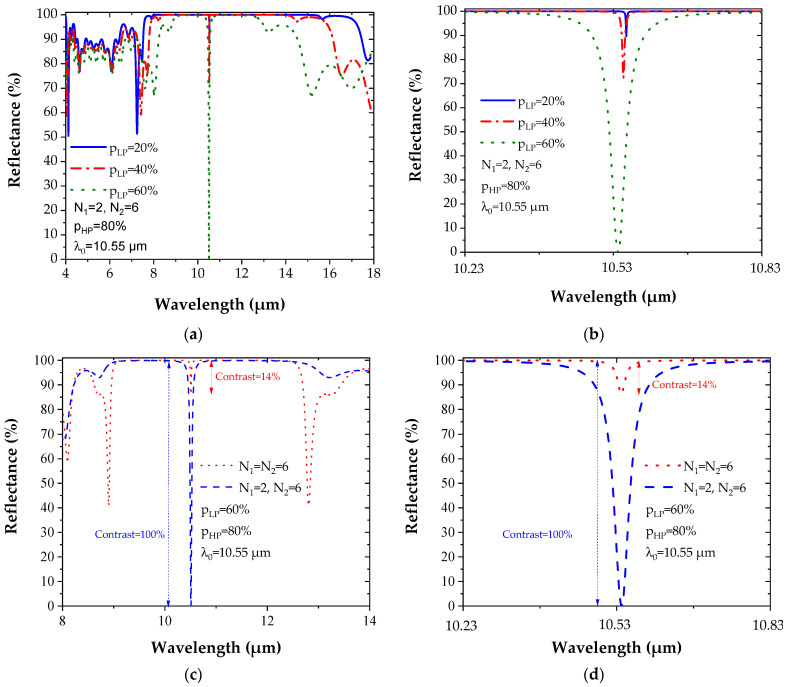
Figure 5 reports the reflectance spectrum for symmetric Bragg mirrors with different values of N with N = N1 = N2, where N1 and N2 the number of layers of the upper and lower Bragg mirrors, respectively. The increase of the number N of layers allowed the increase of the maximum reflectance R (Figure 5a,b). A reflectance of 100% was reached for N = 4 (Figure 5a,b). The decrease in Δp is accompanied by a narrowing of the band Δλ (Figure 5c,d). The contrast is about 14% and is not influenced by the variation of these previously mentioned parameters (Figure 5c,d).
Figure 5.
Reflectance spectrum of microcavities based on symmetric PGe Bragg mirrors with: (a,b) porosities pLP = 60% and pHP = 80% while varying N; (c,d) N fixed to 4, porosities pHP = 80% and varying pLP; (d) the magnification of the spectra around the resonance wavelength.
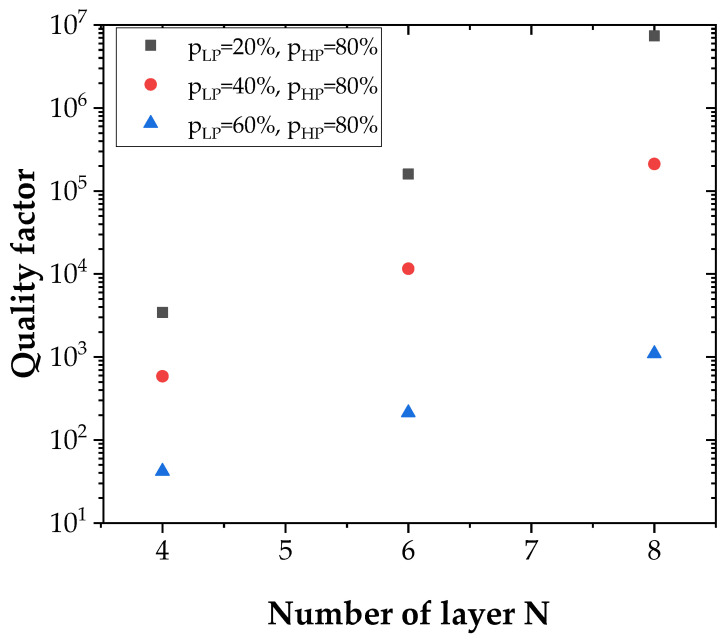
Equation (9) was used to calculate the quality factor Q. The results, for different configurations, are shown in Figure 6.
Figure 6.
Quality factor Q as a function of layer number N for the microcavities studied.
If the increase of the number of layers N allows a slight increase of the quality factor Q, values are multiplied by several orders of magnitude when increasing Δp increases. The influence of Δp on the Q factor is therefore larger than that of the number of layers N. The maximum value of Q is reached for N = 8, pLP = 20% and pHP = 80%, and is of the order of 7.39 × 106.
In symmetrical microcavities, the narrow peak of Figure 5a–d, which is centered at the wavelength of 10.55 µm, does not go down sufficiently (low contrast), which could limit the detection dynamics. This is due to the strong reflectivity of the top Bragg mirror.
In order to improve the resonance contrast, an asymmetric microcavity composed of two Bragg mirrors whose upper part is less reflective than the lower part has also been studied. To obtain a reflectance difference of between the two DBRs, the number of layers N1 (for the upper DBR) and N2 for the lower DBR are no longer chosen to be equal.
Figure 7a,b shows the reflectance spectrum of an asymmetric microcavity varying Δp and using N1 = 2 and N2 = 6. The contrast of reflectance is maximum (100%) for pLP = 60% and pHP = 80%. Figure 7c,d shows a comparison between the reflectance spectrum of the symmetrical in red (contrast = 14%) and asymmetrical microcavities in blue (contrast = 100%). The asymmetric structure associated with the choice of porosities of pLP = 60% and pHP = 80% gives a better contrast. That is the reason why it is used in the next part for SF6 gas detection.
Figure 7.
(a,b) Reflectance spectrum of asymmetric microcavities: N1 = 2 and N2 = 6, porosities pHP = 80% and varying pLP. (c,d) Comparison between the reflectance spectrum of the symmetric (N1 = N2 = 6) and asymmetric microcavities (N1 = 2 and N2 = 6) for pHP = 80% and pLP = 60%.
3.2.2. Theoretical SF6 Gas Detection
The asymmetric microcavity structure used has the following parameters: pLP = 60%, pHP = 80% for a respective number of layers on both sides, N1 = 2 and N2 = 6.
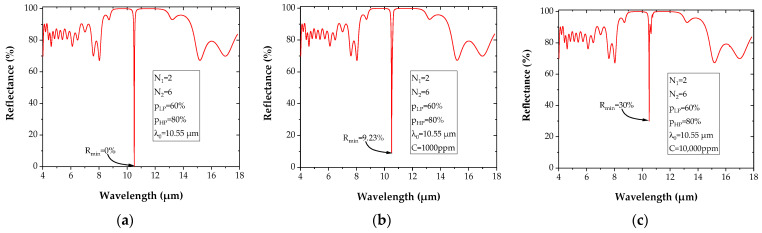
The chosen asymmetric microcavity is first tested in the absence of gas molecules (nsup = nair = 1). In the presence of SF6 gas, the imaginary part k in the superstrate (Equation (2)) is included in the simulation. The SF6 concentration C increase leads to an increase of the reflectance Rmin, which corresponds to the lowest reflectance level at resonance. Rmin is null when there is no gas molecule (Figure 8a) while it reaches about 9% for a SF6 concentration of 1000 ppm (Figure 8b), and 30% when C = 10,000 ppm (Figure 8c).
Figure 8.
Reflectance spectrum of asymmetric microcavity, for PGe materials: without gas (a) and with SF6 concentrations in air of (b) 1000 ppm, and (c) 10,000 ppm.
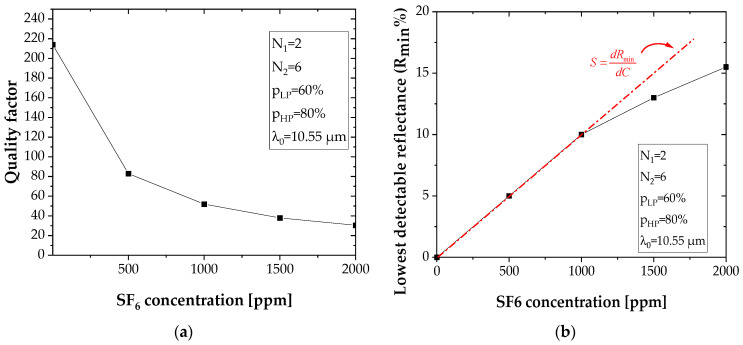
A degradation of the quality factor Q is observed as the concentration C of the gas to be detected becomes important (Figure 9a). The opposite phenomenon is observed for the lowest detectable reflectance Rmin (Figure 9b).
Figure 9.
(a) Quality factor Q as a function of SF6 concentration. (b) The lowest detectable reflectance as a function of concentration of target molecules.
The sensitivity , which is assimilated to the tangent to the curve for SF6 concentrations inferior to C = 1000 ppm in air (threshold value), is equal to S = 0.0093 ± 0.0014%/ppm.
The limit of detection (LOD), which is the minimum SF6 concentration detectable in air is defined by:
| (10) |
where (ΔRmin)min is the minimal reflectance variation measurement. Taking into consideration a typical resolution using attenuated total reflectance Fourier transform infrared spectroscopy of 0.00001% [41], a detection limit of 1.0 ± 0.2 ppb could be theoretically estimated, on the assumption of the linear dependence of Rmin on gas concentration, for the detection of low concentration of SF6 under 1000 ppm. This is a promising value since it is 1000 times larger than the state of the art value of 1 ppm obtained by Yingang et al. [31].
4. Conclusions
This work presents the use of PGe in multilayers for the SF6 mid-IR detection in mid-IR. First, the study focused on the influence of two parameters (number N of layers and the degree of Ge porosity) and on the reflectance R in a structure based on the Bragg mirror. A reflectance of 100% was reached for a number of layers, N = 4, for degrees of porosity pLP = 20% pHP = 80%. Using a microcavity consisting of Bragg mirrors previously optimized, a 100% reflectance and a high-quality factor Q = 7.39 × 106 were obtained for a number of symmetrical Bragg mirrors, N1 = N2 = 8. However, to improve the 14% contrast, an asymmetrical microcavity, consisting of a less reflective top DBR with N1 = 2 (68%) and a more reflective bottom DBR with N2 = 6 (99.7%), is designed. In these conditions, a contrast of 100% is achieved, which would enable a greater detection dynamic at the resonant wavelength of the microcavity corresponding to an SF6 absorption wavelength to insure the sensing selectivity. Theoretical sensing performances of this asymmetrical structure were numerically evaluated for SF6 gas detection, and a sensitivity of the order of S = 0.01%/ppm with an estimated LOD of =1 ppb was inferred, outperforming SF6 sensing detection limits reported in the literature [31]. As asymmetrical PGe vertical structures are quite easy to elaborate and their use in sensing provide a very rapid response, PGe is a very promising material to be explored for the implementation of multilayer optical sensors for Mid-IR gas detection.
Author Contributions
R.Z. carried out the simulations, wrote the paper, and prepared the original draft; N.L. and J.C. proposed the research directions. R.Z., N.L., S.M., Y.D., L.B., M.G., A.Z., J.C. contributed to the organization of the paper, writing, and proofreading. All authors have read and agreed to the published version of the manuscript.
Funding
This work was supported by the French National Research Agency under the MID-VOC ANR project (ANR-17-CE09-0028-01), and the Algerian Ministry of Higher Education and Scientific Research. The PhD grants of S. Meziani by the French Higher Education Ministry and Lannion Trégor Community are gratefully acknowledged.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Not applicable.
Conflicts of Interest
The authors declare no conflict of interest.
Footnotes
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
- 1.Hasan D., Lee C. Hybrid Metamaterial Absorber Platform for Sensing of CO2 Gas at Mid-IR. Adv. Sci. 2018;5:1700581. doi: 10.1002/advs.201700581. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 2.Chen C., Mohr D.A., Choi H.-K., Yoo D., Li M., Oh S.-H. Waveguide-Integrated Compact Plasmonic Resonators for On-Chip Mid-Infrared Laser Spectroscopy. Nano Lett. 2018;18:7601–7608. doi: 10.1021/acs.nanolett.8b03156. [DOI] [PubMed] [Google Scholar]
- 3.Mashanovich G.Z., Nedeljkovic M., Soler-Penades J., Qu Z., Cao W., Osman A., Wu Y., Stirling C.J., Qi Y., Cheng Y.X., et al. Group IV Mid-Infrared Photonics [Invited] Opt. Mater. Express. 2018;8:2276–2286. doi: 10.1364/OME.8.002276. [DOI] [Google Scholar]
- 4.Della Torre A., Sinobad M., Armand R., Luther-Davies B., Ma P., Madden S., Mitchell A., Moss D.J., Hartmann J.-M., Reboud V., et al. Mid-Infrared Supercontinuum Generation in a Low-Loss Germanium-on-Silicon Waveguide. APL Photonics. 2021;6:016102. doi: 10.1063/5.0033070. [DOI] [Google Scholar]
- 5.Kang J., Takenaka M., Takagi S. Novel Ge Waveguide Platform on Ge-on-Insulator Wafer for Mid-Infrared Photonic Integrated Circuits. Opt. Express. 2016;24:11855–11864. doi: 10.1364/OE.24.011855. [DOI] [PubMed] [Google Scholar]
- 6.Lai J., Yuan J., Cheng Y., Mei C., Zhou X., Wu Q., Wu Q., Wu Q., Sang X. Dispersion-Engineered T-Type Germanium Waveguide for Mid-Infrared Supercontinuum and Frequency Comb Generations in All-Normal Dispersion Region. OSA Contin. 2020;3:2320–2331. doi: 10.1364/OSAC.399941. [DOI] [Google Scholar]
- 7.Lin Y., Lee K.H., Bao S., Guo X., Wang H., Michel J., Tan C.S. High-Efficiency Normal-Incidence Vertical p-i-n Photodetectors on a Germanium-on-Insulator Platform. Photonics Res. 2017;5:702–709. doi: 10.1364/PRJ.5.000702. [DOI] [Google Scholar]
- 8.Liu S., Pan M., Feng Z., Qin Y., Wang Y., Tan L., Sun T. Ultra-High Adsorption of Tetracycline Antibiotics on Garlic Skin-Derived Porous Biomass Carbon with High Surface Area. New J. Chem. 2020;44:1097–1106. doi: 10.1039/C9NJ05396D. [DOI] [Google Scholar]
- 9.Minhas R.S., Antunez E.E., Guinan T.M., Gengenbach T.R., Rudd D.A., Voelcker N.H. Fluorocarbon Plasma Gas Passivation Enhances Performance of Porous Silicon for Desorption/Ionization Mass Spectrometry. ACS Sens. 2020;5:3226–3236. doi: 10.1021/acssensors.0c01532. [DOI] [PubMed] [Google Scholar]
- 10.Altaf H., Vorhauer N., Tsotsas E., Vidaković-Koch T. Steady-State Water Drainage by Oxygen in Anodic Porous Transport Layer of Electrolyzers: A 2D Pore Network Study. Processes. 2020;8:362. doi: 10.3390/pr8030362. [DOI] [Google Scholar]
- 11.Ocier C.R., Richards C.A., Bacon-Brown D.A., Krueger N.A., Clawson M.K., Soares J.A.N.T., Braun P.V. Optically Anisotropic Porous Silicon Microlenses with Tunable Refractive Indexes and Birefringence Profiles. Opt. Mater. Express. 2020;10:868–883. doi: 10.1364/OME.385013. [DOI] [Google Scholar]
- 12.Palavicini A., Wang C. Ab-Initio Determination of Porous Silicon Refractive Index Confirmed by Infrared Transmittance Measurements of an Omnidirectional Multilayer Reflector. Appl. Phys. B. 2018;124:65. doi: 10.1007/s00340-018-6938-5. [DOI] [Google Scholar]
- 13.Shubair T., Eljamal O., Khalil A.M.E., Matsunaga N. Multilayer System of Nanoscale Zero Valent Iron and Nano-Fe/Cu Particles for Nitrate Removal in Porous Media. Sep. Purif. Technol. 2018;193:242–254. doi: 10.1016/j.seppur.2017.10.069. [DOI] [Google Scholar]
- 14.Robbiano V., Paternò G.M., La Mattina A.A., Motti S.G., Lanzani G., Scotognella F., Barillaro G. Room-Temperature Low-Threshold Lasing from Monolithically Integrated Nanostructured Porous Silicon Hybrid Microcavities. ACS Nano. 2018;12:4536–4544. doi: 10.1021/acsnano.8b00875. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 15.Zegadi R., Lorrain N., Bodiou L., Guendouz M., Ziet L., Charrier J. Enhanced Mid-Infrared Gas Absorption Spectroscopic Detection Using Chalcogenide or Porous Germanium Waveguides. J. Opt. 2021;23:035102. doi: 10.1088/2040-8986/abdf69. [DOI] [Google Scholar]
- 16.Porous Silicon-Based Photonic Biosensors: Current Status and Emerging Applications/Analytical Chemistry. [(accessed on 5 January 2021)]. Available online: https://pubs.acs.org/doi/full/10.1021/acs.analchem.8b05028?casa_token=Se1TDJUjhlsAAAAA:qvQshbSJOtIC5jB9AR5gsABJWndCCCnb5nEGZNaFDfDbPtTmH1KQ4prL0zyWLb_mXoRZpp9BQJA5PCE. [DOI] [PubMed]
- 17.Hirschman K.D., Tsybeskov L., Duttagupta S.P., Fauchet P.M. Silicon-Based Visible Light-Emitting Devices Integrated into Microelectronic Circuits. Nature. 1996;384:338–341. doi: 10.1038/384338a0. [DOI] [Google Scholar]
- 18.Figueiredo J.L. Nanostructured Porous Carbons for Electrochemical Energy Conversion and Storage. Surf. Coat. Technol. 2018;350:307–312. doi: 10.1016/j.surfcoat.2018.07.033. [DOI] [Google Scholar]
- 19.Zhang B., Cheng M., Liu G., Gao Y., Zhao L., Li S., Wang Y., Liu F., Liang X., Zhang T., et al. Room Temperature NO2 Gas Sensor Based on Porous Co3O4 Slices/Reduced Graphene Oxide Hybrid. Sens. Actuators B Chem. 2018;263:387–399. doi: 10.1016/j.snb.2018.02.117. [DOI] [Google Scholar]
- 20.Zhang M., Zhao C., Gong H., Niu G., Wang F. Porous GaN Submicron Rods for Gas Sensor with High Sensitivity and Excellent Stability at High Temperature. ACS Appl. Mater. Interfaces. 2019;11:33124–33131. doi: 10.1021/acsami.9b09769. [DOI] [PubMed] [Google Scholar]
- 21.Yang X., Li H., Li T., Li Z., Wu W., Zhou C., Sun P., Liu F., Yan X., Gao Y., et al. Highly Efficient Ethanol Gas Sensor Based on Hierarchical SnO2/Zn2SnO4 Porous Spheres. Sens. Actuators B Chem. 2019;282:339–346. doi: 10.1016/j.snb.2018.11.070. [DOI] [Google Scholar]
- 22.Azuelos P., Girault P., Lorrain N., Dumeige Y., Bodiou L., Poffo L., Guendouz M., Thual M., Charrier J. Optimization of Porous Silicon Waveguide Design for Micro-Ring Resonator Sensing Applications. J. Opt. 2018;20:085301. doi: 10.1088/2040-8986/aad01b. [DOI] [Google Scholar]
- 23.Girault P., Azuelos P., Lorrain N., Poffo L., Lemaitre J., Pirasteh P., Hardy I., Thual M., Guendouz M., Charrier J. Porous Silicon Micro-Resonator Implemented by Standard Photolithography Process for Sensing Application. Opt. Mater. 2017;72:596–601. doi: 10.1016/j.optmat.2017.07.005. [DOI] [Google Scholar]
- 24.Bioud Y.A., Boucherif A., Belarouci A., Paradis E., Fafard S., Aimez V., Drouin D., Arès R. Fast Growth Synthesis of Mesoporous Germanium Films by High Frequency Bipolar Electrochemical Etching. Electrochim. Acta. 2017;232:422–430. doi: 10.1016/j.electacta.2017.02.115. [DOI] [Google Scholar]
- 25.Quantification of the SF6 Lifetime Based on Mesospheric Loss Measured in the Stratospheric Polar Vortex-Ray-2017—Journal of Geophysical Research: Atmospheres—Wiley Online Library. [(accessed on 18 June 2021)]. Available online: https://agupubs.onlinelibrary.wiley.com/doi/full/10.1002/2016JD026198.
- 26.Occhipinti Z., Verona R. Kyoto Protocol (KP) In: Leal Filho W., Azul A.M., Brandli L., Özuyar P.G., Wall T., editors. Climate Action. Springer International Publishing; Berlin/Heidelberg, Germany: 2020. pp. 605–617. [Google Scholar]
- 27.Lu Q., Li Q., Hu L., Huang L. An Effective Low-Contrast SF6 Gas Leakage Detection Method for Infrared Imaging. IEEE Trans. Instrum. Meas. 2021;70:1–9. doi: 10.1109/TIM.2021.3118092. [DOI] [Google Scholar]
- 28.Zhang G., Wang Z., Zhang X. Theoretical Screening into Ru-Doped MoS2 Monolayer as a Promising Gas Sensor upon SO2 and SOF2 in SF6 Insulation Devices. Mol. Phys. 2021:e2018517. doi: 10.1080/00268976.2021.2018517. [DOI] [Google Scholar]
- 29.Cui H., Liu T., Zhang Y., Zhang X. Ru-InN Monolayer as a Gas Scavenger to Guard the Operation Status of SF6 Insulation Devices: A First-Principles Theory. IEEE Sens. J. 2019;19:5249–5255. doi: 10.1109/JSEN.2019.2899966. [DOI] [Google Scholar]
- 30.Cui H., Yan C., Jia P., Cao W. Adsorption and Sensing Behaviors of SF6 Decomposed Species on Ni-Doped C3N Monolayer: A First-Principles Study. Appl. Surf. Sci. 2020;512:145759. doi: 10.1016/j.apsusc.2020.145759. [DOI] [Google Scholar]
- 31.Gui Y., Zhang X., Lv P., Wang S., Tang C., Zhou Q. Ni-CNT Chemical Sensor for SF6 Decomposition Components Detection: A Combined Experimental and Theoretical Study. Sensors. 2018;18:3493. doi: 10.3390/s18103493. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 32.Katsidis C.C., Siapkas D.I. General Transfer-Matrix Method for Optical Multilayer Systems with Coherent, Partially Coherent, and Incoherent Interference. Appl. Opt. 2002;41:3978–3987. doi: 10.1364/AO.41.003978. [DOI] [PubMed] [Google Scholar]
- 33.Gordon I.E., Rothman L.S., Hill C., Kochanov R.V., Tan Y., Bernath P.F., Birk M., Boudon V., Campargue A., Chance K.V., et al. The HITRAN2016 Molecular Spectroscopic Database. J. Quant. Spectrosc. Radiat. Transf. 2017;203:3–69. doi: 10.1016/j.jqsrt.2017.06.038. [DOI] [Google Scholar]
- 34.Duris M., Guendouz M., Lorrain N., Pirasteh P., Bodiou L., Raiah W., Raiah W., Coffinier Y., Thomy V., Charrier J. Vertical Multilayer Structures Based on Porous Silicon Layers for Mid-Infrared Applications. Opt. Mater. Express. 2020;10:1921–1930. doi: 10.1364/OME.396343. [DOI] [Google Scholar]
- 35.Refractive Index of Ge (Germanium)—Amotchkina. [(accessed on 14 November 2021)]. Available online: https://refractiveindex.info/?shelf=main&book=Ge&page=Amotchkina.
- 36.Astrova E.V., Voronkov V.B., Remenyuk A.D., Shuman V.B., Tolmachev V.A. Variation of the Parameters and Composition of Thin Films of Porous Silicon as a Result of Oxidation: Ellipsometric Studies. Semiconductors. 1999;33:1149–1155. doi: 10.1134/1.1187885. [DOI] [Google Scholar]
- 37.Tsai W.-T. The Decomposition Products of Sulfur Hexafluoride (SF6): Reviews of Environmental and Health Risk Analysis. J. Fluor. Chem. 2007;128:1345–1352. doi: 10.1016/j.jfluchem.2007.06.008. [DOI] [Google Scholar]
- 38.Zhang W., Xiong B., Shao B., Lei X., Chen W. A Demodulation Model of Dynamic Low-Finesse Fabry-Perot Cavity Based on the Instantaneous Frequency. IEEE Access. 2020;8:71074–71082. doi: 10.1109/ACCESS.2020.2987466. [DOI] [Google Scholar]
- 39.Zhang H., Lv J., Jia Z. Detection of Ammonia-Oxidizing Bacteria (AOB) Using a Porous Silicon Optical Biosensor Based on a Multilayered Double Bragg Mirror Structure. Sensors. 2018;18:105. doi: 10.3390/s18010105. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 40.Toanen V., Symonds C., Benoit J.-M., Gassenq A., Lemaıtre A., Bellessa J. Room-Temperature Lasing in a Low-Loss Tamm Plasmon Cavity. ACS Photonics. 2020;7:2952–2957. doi: 10.1021/acsphotonics.0c00781. [DOI] [Google Scholar]
- 41.Khoshmanesh A., Dixon M.W.A., Kenny S., Tilley L., McNaughton D., Wood B.R. Detection and Quantification of Early-Stage Malaria Parasites in Laboratory Infected Erythrocytes by Attenuated Total Reflectance Infrared Spectroscopy and Multivariate Analysis. Anal. Chem. 2014;86:4379–4386. doi: 10.1021/ac500199x. [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Data Availability Statement
Not applicable.