Liquid-liquid phase separation [1, 2] occurs not only in bulk liquid, but also on surfaces. In physiology, the nature and function of condensates on cellular structures remain unexplored. Here, we study how the condensed protein TPX2 behaves on microtubules to initiate branching microtubule nucleation [3–5], which is critical for spindle assembly in eukaryotic cells [6–10]. Using fluorescence, electron, and atomic force microscopies and hydrodynamic theory, we show that TPX2 on a microtubule reorganizes according to the Rayleigh-Plateau instability, like dew droplets patterning a spider web [11,12]. After uniformly coating microtubules, TPX2 forms regularly spaced droplets from which branches nucleate. Droplet spacing increases with greater TPX2 concentration. A stochastic model shows that droplets make branching nucleation more efficient by confining the space along the microtubule where multiple necessary factors colocalize to nucleate a branch.
Branching microtubule nucleation plays a major role in spindle assembly and chromosome segregation in dividing eukaryotic cells, where it is required to generate microtubules in the spindle for kinetochore fiber tension, spindle bipolarity, and cytokinesis [6–10]. Its malfunction has been linked to a worse prognosis in cancer [13,14]. The nucleation of a new microtubule from the side of a preexisting microtubule requires TPX2, the augmin complex, and the γ-tubulin ring complex (γ-TuRC) [3]. The first component to bind to the preexisting microtubule is TPX2 [4], which forms a liquid-like condensate on the microtubule that recruits tubulin and increases branching nucleation efficiency [5]. Other proteins also form condensed phases when associated with microtubules, such as Tau [15,16] and BugZ [17]. Yet, how these proteins behave on the microtubule surface and how this behavior translates to biological function remain unexplored. Here, we investigate the dynamics of condensed TPX2 on the microtubule. We find that the hydrodynamic Rayleigh-Plateau instability causes TPX2 to form regularly spaced droplets along the microtubule. Then, microtubule branches nucleate from these droplets.
We first studied the dynamics of TPX2 binding to microtubules in vitro using total internal reflection fluorescence (TIRF) microscopy (Fig. 1a, Fig. 1b, Methods). GFP-TPX2 at a concentration of 1 μM formed an initially uniform coating along microtubules within seconds. This coating then broke up into a periodic pattern of droplets over tens of seconds with size 0.5 ± 0.1 μm and spacing 0.6 ± 0.2 μm (mean ± standard deviation, N = 35 microtubules) along the microtubules (Movie 1, Fig. S1). Similar patterns of condensed protein have also been previously observed on single microtubules for TPX2 [5] and microtubule bundles for Tau [15], BugZ [17], and LEM2 [19]. We next performed the same experiment at a lower, physiological concentration of TPX2, 0.1 μM [5,20]. We observed a uniform coating but no visible droplet formation (Fig. S2a). In contrast, at higher resolution, electron microscopy (Methods) revealed that regularly spaced droplets do form at 0.1 μM, with size 0.29±0.03 μm and spacing 0.46±0.11 μm (mean ± standard deviation, N =2 microtubules) (Fig. 1c). This indicates that these droplets can exist below the diffraction limit of visible light. We then reconstituted branching microtubule nucleation in vitro using purified proteins [18] (Methods) and observed that branches originate from TPX2 droplets colocalized with augmin and γ-TuRC (Fig. 1d, Movie 2). 75 ± 18% of TPX2 droplets nucleated branches (mean ± standard deviation, N = 7 microtubules, Table S1). Droplet formation always happened before the nucleation of a branch, and no branches nucleated from areas that did not have droplets. Finally, in meiotic cytosol (Methods), microtubules also nucleate from a TPX2-coated microtubule to form a branched network (Fig. S2b, Movie 3). These results suggest that droplet formation from condensed TPX2 may be important for branching microtubule nucleation.
Figure 1: TPX2 uniformly coats microtubules and then forms periodically spaced droplets that can nucleate branches.
(a) Initial films and subsequent droplets of TPX2 on microtubules visualized using TIRF microscopy (Movie 1). 1 μM GFP-TPX2 was spiked onto a passivated glass surface coated with Alexa568-labeled microtubules. Scale bars are 1 μm. (b) Large field of view of a TIRF experiment after droplets have formed along microtubules. GFP-TPX2 concentration is 1 μM. Microtubules with a droplet pattern are marked with a number. Scale bars are 5 μm. (c) TPX2 droplets on microtubules imaged using electron microscopy. 0.1 μM GFP-TPX2 was incubated with microtubules bound to a carbon grid. Scale bars are 100 nm. (d) Branched microtubules nucleating from TPX2 droplets formed along the initial mother microtubule, assembled in vitro as in [18] (Movie 2). Recombinant GFP-augmin and γ-TuRC purified from X. laevis meiotic cytosol were included. Arrows indicate branched microtubules. Scale bars are 5 μm (top) and 1 μm (bottom). Only the soluble Cy5-tubulin channel (magenta) was imaged over time to enable a higher frame rate. The GFP-TPX2 and GFP-augmin channel (cyan) and the A568 template microtubule channel (red) were only imaged at the start at 60 s.
We wished to study the dynamics of droplet formation of TPX2 alone at higher spatial resolution than available by fluorescence microscopy and with temporal resolution not accessible by electron microscopy. Therefore, we turned to atomic force microscopy (AFM) to measure the topography of the initial coating and subsequent beading up of TPX2 on microtubules (Methods). By scanning the AFM tip over the sample every 4 minutes, with a resolution of ≃ 8 nm in the sample plane and ≃ 1 nm in height, we measured the height of a bare microtubule on a mica surface to be 25 ± 2 nm (mean ± standard deviation) (Fig. 2a −5 min, Fig. 2b black line), consistent with the known diameter of 25 nm [21]. We then added TPX2 at a concentration of 0.2 μM. After addition of TPX2, the height signal uniformly increased to 41 ± 3 nm (mean ± standard deviation) as the condensed protein coated the microtubule (Fig. 2a 0 min, Fig. 2b blue line). The film of TPX2 then proceeded to bead up into a periodic pattern of droplets along the microtubule with spacing 250± 35 nm (mean ± standard error of the mean) (Fig. 2a 60 min, Fig. 2b red line, Movie 4). The white carets in the 60 minute topography in Fig. 2a mark the droplets. The longer time scale to form droplets and the different spacings between droplets in AFM experiments compared to fluorescence and electron microscopy experiments is due to the different biochemical conditions and components used in each experimental method (Table S2). The emergent periodicity of the condensate is evident in the power spectrum of the raw height profile along the microtubule averaged over many samples (Fig. 2c). Power spectra rely on the Fourier transform to identify the frequency components of a signal buried in noise (Methods). Peaks in a power spectrum indicate the presence of a periodic pattern amidst noise; a monotonic power spectrum is expected for data that lacks periodicity. The power spectrum (Fig. 2c) shows no characteristic length scale before and immediately after coating with TPX2, whereas a peak with wavelength 260±20 nm (mean ± standard deviation) has emerged by 60 minutes. Thus, the topography of condensed TPX2 on microtubules exhibits systematic emergent periodicity.
Figure 2: AFM measurements reveal condensed TPX2 dynamics on microtubules.
(a) Time-lapse AFM height topographies of TPX2 uniformly coating and then forming regularly spaced droplets on a microtubule. GFP-TPX2 at 0.1 μM was spiked onto microtubules adhered to a mica surface during data acquisition. This is the entire measured span of the microtubule in the top left of Movie 4. The topography was smoothed using a 40 nm × 40 nm median filter. White carets mark droplets. Scale bar is 100 nm. (b) Height profiles centered on the microtubule long axis before coating (black), just after coating (blue), and when droplets have formed on the microtubule surface (red). Height profiles were smoothed using a moving-average window of 40 nm. The raw height profiles and their power spectra are shown in Fig. S3. (c) Averaged power spectra calculated from the raw height profiles across many microtubules for uncoated (black, N = 22 microtubules), uniformly coated (blue, N = 23 microtubules), and droplet-patterned microtubules (red, N = 17 microtubules). A peak is seen only in the data for droplet-patterned microtubules, corresponding to a droplet spacing of 260 ± 20 nm (mean ± standard deviation). Shaded regions are 95% bootstrap confidence intervals.
Fluids that coat a solid fiber are known to form droplets via the Rayleigh-Plateau instability [11]. Surface tension causes the film to be unstable due to the curvature of the filament surface and the surface area is minimized by forming periodically spaced droplets along the fiber [12,22–24]. Following Goren [22], but working directly at low Reynolds number as is appropriate for our experimental system, we solved a linear stability problem for the growth rate σ of the droplet pattern as a function of the wave number k = 2π/λ, where λ is the pattern wavelength (Fig. 3a, Fig. S4, Supplement 1.1). We find that for a given ratio of the microtubule radius to the outer film radius, ri/ro, there is a wavelength λmax that grows with the largest growth rate σmax (Fig. 3b). This wavelength will grow exponentially faster than all other wavelengths, leading to a periodic interface with wavelength λmax. Thus, we identify the thicker regions of the AFM height profiles as droplets formed by this instability.
Figure 3: Hydrodynamic theory predicts TPX2 droplet formation on a microtubule surface.
(a) Schematic of the Rayleigh-Plateau instability. TPX2 initially coats the microtubule uniformly with thickness h = ro – ri. This film breaks up into droplets with spacing λmax due to capillary forces on a time scale roμ/γ, where μ is the condensate viscosity and γ is the surface tension. (b) Dispersion relation showing the growth rate versus wave number for different aspect ratios ri/ro. The most unstable mode (black circles and line) grows most quickly and corresponds to the observed droplet spacing λmax. (c) Theoretical prediction for λmax (solid orange line). Overlaid are AFM measurements of the average film thickness and droplet spacing for each TPX2 concentration. The average droplet spacing corresponds to the peak of the averaged power spectra at a given concentration (Fig. S7, Table S3). There are no fit parameters. The shaded area encompasses the wavelengths that grow within 10% of the maximum growth rate for each h. In order of increasing concentration, N = 25; 17; 23; and 21 microtubules. Error bars are standard deviations for h and bootstrapped standard deviations for λmax.
We tested the ability of this theory to explain droplet formation on microtubules by measuring film thicknesses and subsequent droplet spacings at different bulk concentrations of TPX2 (Fig. S5). The radius of the microtubule is fixed at ri = 25 nm. However, the thickness of the initial TPX2 film depends on its bulk concentration in solution and the density of microtubules (Fig. S6, Supplement 1.2). Capitalizing on this experimental fact, we changed the initial film thickness from h = 13 ± 2 nm at 0.1 μM TPX2 to h =22 ± 1 nm at 0.8 μM TPX2 (mean ± standard deviation) for a fixed microtubule density. The lower concentrations are physiological in healthy cells [5, 20]. The higher concentrations may reflect overexpression in cancer tumor cells, in which TPX2 often has higher genetic copy number [25] and transcript and protein expression [13,26], and can be a negative prognostic indicator [14]. TPX2 formed regularly spaced droplets along microtubules with consistently larger spacings λmax as its bulk concentration increased, following theory (Fig. 3c, Fig. S7, Table S3).
Our data exhibit spread for two reasons. First, the dispersion relation we calculate (Fig. 3b) has a broad peak, which means that wavelengths near the maximum growth rate λmax will grow nearly as fast (Fig. 3c, shaded area). Therefore, the discrepancy between the orange curve in Fig. 3c and the measured λmax is a natural consequence of the hydrodynamic theory. Second, low-force (25–40 pN), nanometer-scale AFM in fluid is highly susceptible to thermal noise. This is apparent in the raw height profiles (Fig. S3a) and power spectra (Fig. S3b) of the microtubule shown in Fig. 2a and Fig. 2b, as well as the power spectra of individual microtubules across the other TPX2 concentrations (Fig. S5, right column).
The theory is purely geometric and has no free parameters. The predicted wavelength does not depend on the material properties of the TPX2 condensate such as viscosity or surface tension, which only set the timescale for pattern formation. We note that the higher microtubule density used in AFM experiments (Fig. 2, Fig. 3c) leads to thinner condensed films than in the EM and reconstitution experiments (Fig. 1) and hence smaller droplet sizes, even at similar TPX2 concentrations (Table S2, Supplement 1.2).
In addition, we measured the growth rate of λmax to be exponential at early times, as expected for a linear instability (Fig. S8). At later times, the periodicity has already been selected as the droplet pattern has set in. Thus, the spectral power versus time stops changing. We also see that the time to form droplets is orders of magnitude greater than the time to grow the initial film. The film grows more quickly because the timescale for its growth is set by fast diffusion of protein in the bulk, whereas the timescale for droplet formation is limited by the slow capillary velocity γ/μ of the condensate (Supplement 1.2). As a control, kinesin-1, a motor protein that does not exist as a condensed phase in any known physiological context and whose binding site on the microtubule is structurally known [27, 28], did not exhibit hydrodynamic behavior on the microtubule as measured by AFM (Fig. S7).
How might TPX2 droplets facilitate branching microtubule nucleation? Noting that the process requires the coordinated action in time and space of at least two additional factors, augmin and γ-TuRC, we first imaged the localization of γ-TuRC on microtubules in the presence of TPX2 and augmin using electron microscopy. We found that the ratio of γ-TuRC on microtubules to γ-TuRC on the grid surface was 0.05 ± 0.05 without TPX2 and augmin (mean ± standard deviation, N = 3 microtubules, Fig. S9, Table S4). With TPX2 and augmin, this ratio was 0.48 ± 0.04 (mean ± standard deviation, N = 4 microtubules, Table S5), confirming that TPX2 and augmin preferentially localize γ-TuRC to microtubules. We observed that multiple 7-TuRCs cluster inside TPX2 droplets spaced 0.25 ± 0.09 μm (mean ± standard deviation, N = 4 microtubules) apart along microtubules (Fig. 4a), consistent with a recent report [18]. The ratio between the number of γ-TuRCs inside TPX2 droplets to the number on bare regions of the same microtubules was 4.8 ± 2.0 (mean ± standard deviation, N = 3 microtubules). Although this is an underestimate, given the difficulty of counting γ-TuRCs in TPX2 droplets, these results demonstrate that γ-TuRC preferentially localizes to TPX2 droplets along microtubules.
Figure 4: A stochastic model predicts that TPX2 droplets enhance the efficiency of branching microtubule nucleation.
(a) Electron microscopy images show ring-shaped γ-TuRCs (black arrows) localizing to regularly spaced TPX2 droplets along the microtubule in the presence of augmin. Scale bars are 100 nm. (b) Schematic of branching factors binding to a microtubule coated with a uniform TPX2 layer versus periodic TPX2 droplets. (c) Monte Carlo simulations show that droplets colocalize two necessary factors faster than a uniform coating. Here, konl/koff = 10. These results are not sensitive to this parameter choice (Fig. S10). Each datapoint is the average of 107 independent simulations.
The first step in branching is the binding of TPX2 to the microtubule, which then localizes the other factors [4]. As such, we hypothesized that regularly spaced TPX2 droplets lead to more efficient colocalization of factors than a uniform coating (Fig. 4b). For a uniform coating, multiple factors must search a greater length along the microtubule before finding each other to nucleate a new branch. With regularly spaced droplets, the explored distance is shorter, which reduces the search time. We performed kinetic Monte Carlo simulations [29] for two factors binding to (with rate kon) and unbinding from (with rate koff) a microtubule of length l with a uniform TPX2 coating and a periodic pattern of TPX2 droplets (Supplement 1.3). These results show that the time to colocalize τ on the microtubule, and hence for nucleation of a new branch, is smaller for droplets than for a uniform layer (Fig. 4c, Fig. S10). As a negative control for this model, we used AFM to measure the topography of a C-terminal fragment of TPX2 on microtubules. This fragment is known to be less efficient at nucleating branches in cytosol [5]. Consistent with our model, it did not form droplets on microtubules (Fig. S7). Thus, synergistic with TPX2’s ability to recruit tubulin [5] and its high concentration as a condensate (Supplement 1.2), its organization into droplets partitions the microtubule so that multiple factors can more easily find each other. Taken together, TPX2’s phase behaviour enhances reaction kinetics via droplet patterning, condensate concentration and tubulin recruitment.
It is important to think about our model in a cellular context. During cell division, TPX2 is released as a gradient in the vicinity of chromosomes [30]. The typical TPX2 concentration in X. laevis is 90 nM [20] and the typical gradient length is ~ 10 μm [30]. This gives ~ 2 × 105 TPX2 molecules that are available to condense on microtubules near chromosomes, assuming a spherical volume. We estimate the concentration of TPX2 in the condensed phase to be 104 μm−3 using our AFM experiments (Methods). There are then ~ 80 TPX2 molecules needed to form a 10 nm condensed film on a typical 7-μm-long, 25-nm-diameter microtubule [31]. Therefore, TPX2 can coat ~ 3 × 103 microtubules during cell division. Given that the density of microtubules in the metaphase spindle is ~ 2 μm−3 within 10 μm of chromosomes [31], ~ 8 × 103 microtubules lie in the vicinity of chromosomes. Thus, TPX2 can coat ~ 40% of the metaphase microtubule mass near chromosomes at this film thickness. We hypothesize that the Rayleigh-Plateau instability is most relevant during early spindle assembly in order to accelerate the generation of microtubules, as TPX2 is responsible for creating most of the spindle microtubules via branching nucleation [32], in particular during early stages of spindle assembly [8].
As the study of liquid-like protein condensates has intensified [1,2], the physical phenomenology has been dominated by optical observations of droplets in solution or on microtubules. Here, we quantitatively demonstrate the emergence of non-trivial hydrodynamic features such as films and spatiotemporal periodic instabilities that arise when a condensate interacts with a filament. In future work, it will be interesting to explore how multiple proteins, such as TPX2 and BuGZ [17], condense on the microtubule, as multi-protein condensates in solution have been reported [33]. We suspect that interfacial physics could manifest itself in other ways when condensates interact with cellular filaments, such as via elastocapillary effects [34] that could produce forces between cytoskeletal filaments or other semi-flexible macromolecules such as RNA and DNA [35].
Extended Data
Extended Data Fig. 1. Statistics of droplet patterned microtubules imaged with TIRF microscopy.
(a) Histogram of droplet sizes and spacings for TIRF experiments at 1 uM GFP-TPX2. N=35 microtubules were analyzed with a mean size of 0.5 +/− 0.1 um and spacing 0.6 +/− 0.2 um (mean +/− standard deviation). (b) Average power spectrum of GFP-TPX2 fluorescence intensities of droplet patterns for TIRF experiments at 1 uM GFP-TPX2 (N=35 microtubules). The peak indicates the emergence of a periodic pattern with wavelength lambda_max = 0.6 +/− 0.1 um (mean +/− standard deviation), in agreement with the histogram analysis. Shaded regions are 95% bootstrap confidence intervals. For calculation details, see Methods.
Extended Data Fig. 2. TPX2 on the microtubule can appear uniform when imaged via optical microscopy.
(a) TIRF microscopy time lapses showing that a 0.1 uM TPX2 coating does not break up into visible droplets like the 1 uM TPX2 coating does. (b) Branching microtuble nucleation visualized by TIRF microscopy in X. laevis meiotic cytosol at 0.1 uM TPX2, indicating that branching can occur from diffraction limited droplets.
Extended Data Fig. 3. Raw and smoothed AFM height profiles, and power spectra of raw height profiles.
(a) Raw height profiles of the topographies in Fig. 2a. The smoothed profile from Fig. 2b is shown again for reference. (b) Power spectra of the raw height profiles in (a). The red curve shows the mean +/− standard error of the mean over nine topographies of the microtubule after the droplet pattern had formed. The frequency f at which the peak in the red curve occurs gives the droplet spacing measured for this microtubule, according to lambda = 1/f.
Extended Data Fig. 4. Schematic of the Rayleigh-Plateau instability.
The viscosity of the condensed film is mu, gamma is the surface tension of the interface, and p_infinity is the far field pressure provided by the solvent. The microtubule has radius r_i Initially, the interface is flat at xi(z, t=0) = r_o, but this scenario is unstable against the capillary pressure gamma/r_o, so xi(z, t) will evolve to a lower energy state. The unit normal n and unit tangent t track the geometry of the interface during its evolution.
Extended Data Fig. 5. AFM height profiles and power spectra at additional TPX2 concentrations.
For 0.1 +/− 0.05 uM, the power spectrum is averaged over N=5 topographies after the droplet pattern had formed. For 0.6 +/− 0.3 uM, N = 3. For 0.8 +/− 0.4 uM, N = 4; the uncoated height profile for this specific microtubule is unavailable because the sample moved after TPX2 addition. Height profiles were smoothed using a moving-average window of 40 nm. All power spectra after droplet formation show mean +/− standard error of the mean.
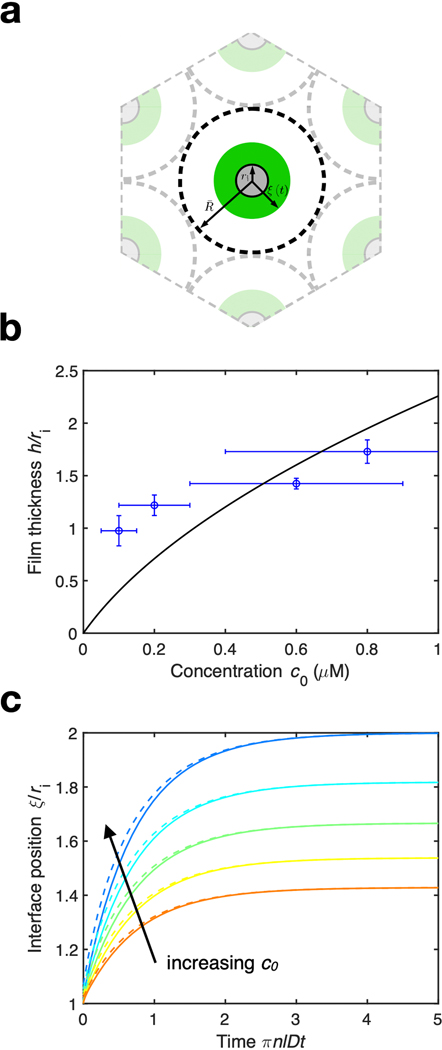
Extended Data Fig. 6. Growth of the condensed film.
(a) Schematic of the model for growth of the condensed protein film. Microtubules of radius r_i are spaced periodically by a distance 2R, where R = 1/sqrt(pi * n * l) where l is the typical microtubule length and n is the number density of microtubules. Soluble protein phase separates from solution and nucleates a spatially uniform condensed film on the microtubule surface, whose interfacial position we denote by r = xi(t). (b) Final film thickness h versus initial concentration c_0 as measured by atomic force microscopy (blue) and as predicted by equation (19) (black) using a least-squares fit. (c) Evolution of the interfacial position of the film xi/r_i over time T for S = r_i/r_o between [0.5, 0.7], which is our experimentally observed range of S. Solid lines are the exact solution and dashed lines are the asymptotic formula (34b).
Extended Data Fig. 7. Average power spectra from AFM data for all concentrations of TPX2 and for uncoated, initially TPX2-coated, C-terminal-TPX2-bound, and kinesin-1-bound microtubules; the growth of the instability for early times is exponential.
Peaks indicate characteristic wavelengths that correspond to a typical droplet spacing (Table S3) (N= 25, 17, 23, and 21 microtubules, respectively, for increasing TPX2 concentration). Also included are average power spectra for uncoated microtubules (N = 29 microtubules), microtubules initially coated uniformly with TPX2 (N = 25 microtubules), kinesin-bound microtubules (N = 19 microtubules), and C-terminal-TPX2-bound microtubules (N = 4 microtubules)—none of which show any characteristic spatial features. For kinesin-bound microtubules, h = 2.9 +/− 2.0 nm, consistent with what one would expect for the kinesin construct used. For C-terminal-TPX2-bound microtubules, h = 3.7 +/− 1.8 nm. Heights are mean +/− standard deviation. Shaded regions represents 95% bootstrap confidence intervals.
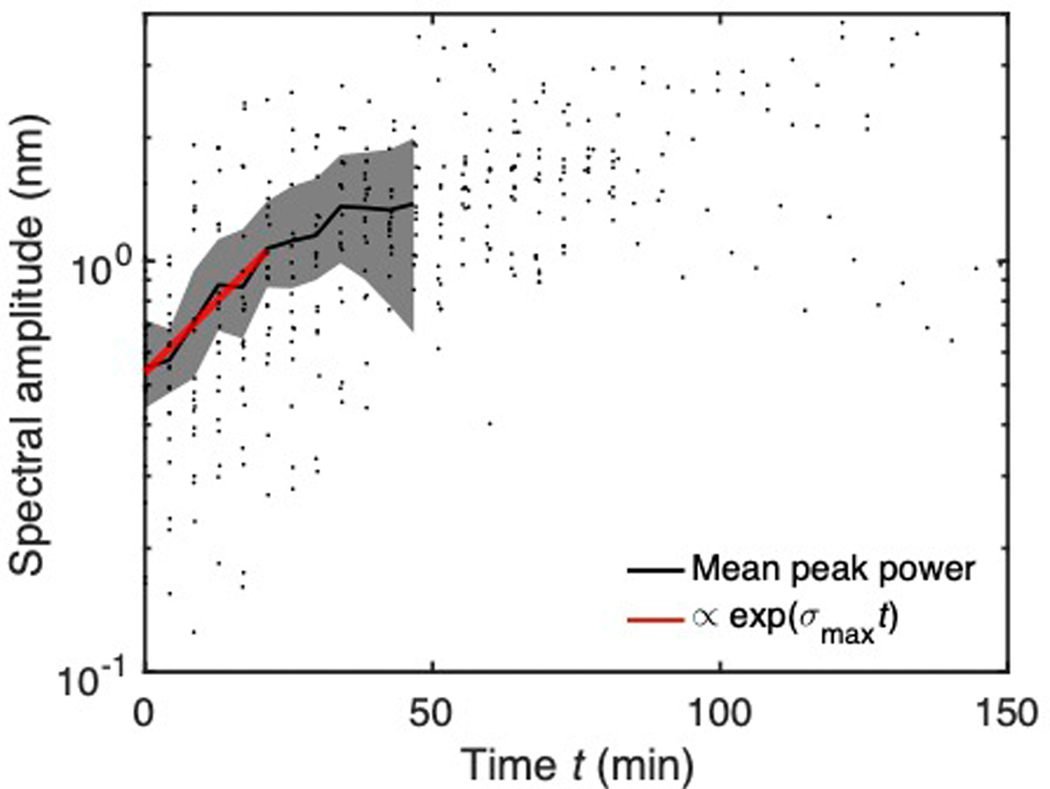
Extended Data Fig. 8. The growth of the instability for early times is exponential.
The average spectral amplitude at the most unstable frequency grows exponentially for early times (black line, N=21 microtubules). Spectral amplitude = sqrt(spectral power). Individual measurements are black dots. Shaded region represents 95% bootstrap confidence intervals. At later times, the spectral amplitude levels off due to nonlinear forces as the pattern sets in. For the exponential fit (red), sigma_max = 0.03 1/min, with R2 = 0.75.
Extended Data Fig. 9. g-TuRC localization on bare microtubules.
Typical electron microscopy experiment with just g-TuRC and microtubules. Clearly, the localization of g-TuRC to the microtubule without TPX2 and augmin is negligible (Table S4). Scale bar is 100 nm.
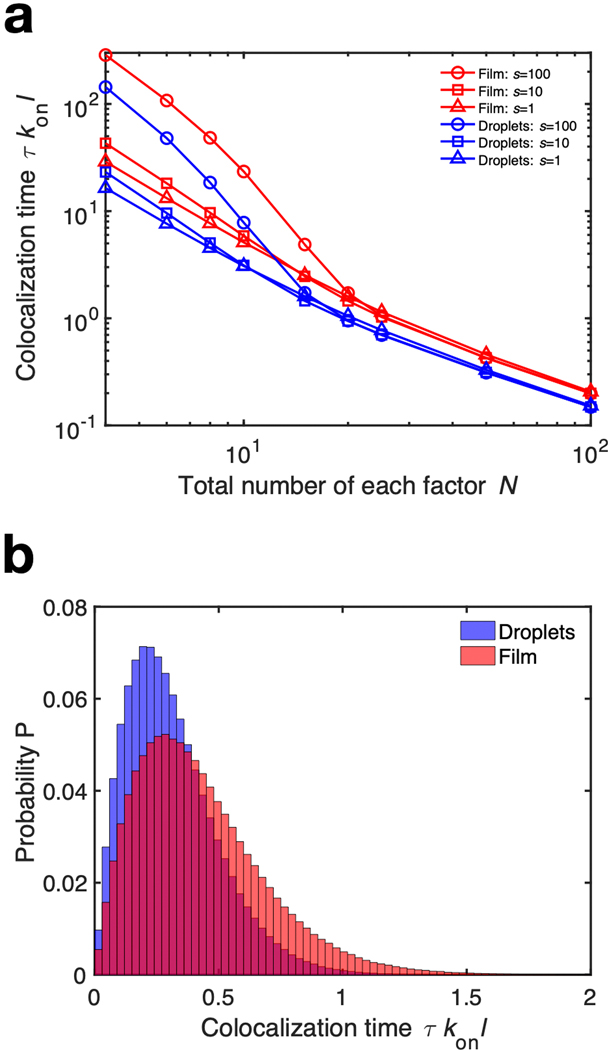
Extended Data Fig. 10. Parametric study of Monte Carlo simulations.
(a) Time tau to colocalize two distinct factors, and hence form a branch, as a function of N and s for a uniform and periodic protein coating. For a given s, the periodic coating is uniformly more efficient at colocalizing well-mixed factors. Each data point is the average of 107 independent simulations. (b) Typical histogram of 107 independent simulations for F = 2, N = 50, and s = 10.
Supplementary Material
Movie 3. Branching microtubule nucleation visualized using TIRF microscopy. 0.1 μM TPX2 is added to X. laevis meiotic cytosol puri_ed from eggs. TPX2 coats the mother microtubule from which daughter microtubules then nucleate, leading to an autocatalytic branched network. Frame dimensions are 16 μm × 22 μm.
Movie 4. Rayleigh-Plateau instability of TPX2 on microtubules probed using AFM. 0.2 ± 0.1 μM GFP-TPX2 was spiked onto a mica surface covered with microtubules. The uniform film of TPX2 is established at t = 0 s, after which it breaks up into droplets. Frame dimensions are 2 μm × 2 μm.
Movie 1. Rayleigh-Plateau instability of TPX2 on microtubules visualized using TIRF microscopy. 1 μM GFP-TPX2 was spiked onto a passivated glass surface covered with Alexa568-labeled microtubules at t = 0 s. TPX2 coats the microtubule and then proceeds to break up into droplets. Scale bar is 1 μm.
Movie 2. Microtubule branches nucleating from TPX2 droplets on a preexisting microtubule. During acquisition, only the soluble tubulin channel was imaged to enable capturing nucleation and polymerization of branched microtubules at high temporal resolution. Scale bar is 5 μm.
Acknowledgments
We thank Drs. Stephanie Lee, Tseng-Ming Chou, and Matthew Libera at Stevens Institute of Technology for access to their AFM; Drs. Ian Armstrong and Samrat Dutta at Bruker for access to and support for their AFM; Drs. Matthew King, Ben Bratton, Mohammad Safari, Matthias Koch, Pierre Ronceray, and Ned Wingreen for helpful discussions; Akanksha Thawani for purification of TPX2; Henry Ando, Caroline Holmes, physiology students Valentina Baena, Linda Ma, Davis Laundon, and the Physiology Course at the Marine Biological Lab for assisting with the first AFM trials; and Princeton’s Imaging and Analysis Center, which is partially supported by the Princeton Center for Complex Materials, an NSF-MRSEC program (DMR-1420541).
B.G. was supported by PD Soros and NSF GRFP. S.S. was supported by NIH NCI NRSA 1F31CA236160 and NHGRI training grant 5T32HG003284. This work was funded by NIH NIA 1DP2GM123493, Pew Scholars Program 00027340, Packard Foundation 2014-40376, and CPBF NSF PHY-1734030.
Footnotes
Competing interests
The authors declare no competing interests.
Ethics
Data, code, and materials used are available upon request.
Animal care was done in accordance with recommendations in the Guide for the Care and Use of Laboratory Animals of the NIH and the approved Institutional Animal Care and Use Committee (IACUC) protocol 1941-16 of Princeton University.
References
- [1].Shin Y. & Brangwynne CP Liquid phase condensation in cell physiology and disease. Science 357 (2017). [DOI] [PubMed] [Google Scholar]
- [2].Alberti S, Gladfelter A. & Mittag T. Considerations and challenges in studying liquid-liquid phase separation and biomolecular condensates. Cell 176, 419–434 (2019). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [3].Petry S, Groen A, Ishihara K, Mitchison T. & Vale R. Branching microtubule nucleation in xenopus egg extracts mediated by augmin and tpx2. Cell 152, 768–777 (2013). URL http://www.sciencedirect.com/science/article/pii/S0092867413000159. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [4].Thawani A, Stone HA, Shaevitz JW & Petry S. Spatiotemporal organization of branched microtubule networks. eLife 8, e43890 (2019). URL 10.7554/eLife.43890. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [5].King MR & Petry S. Phase separation of tpx2 enhances and spatially coordinates microtubule nucleation. Nature Communications 11, 270 (2020). URL 10.1038/s41467-019-14087-0. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [6].Uehara R. & Goshima G. Functional central spindle assembly requires de novo microtubule generation in the interchromosomal region during anaphase. The Journal of cell biology 191, 259–267 (2010). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [7].Uehara R. et al. The augmin complex plays a critical role in spindle microtubule generation for mitotic progression and cytokinesis in human cells. Proceedings of the National Academy of Sciences 106, 6998–7003 (2009). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [8].Petry S, Pugieux C, Nedelec FJ & Vale RD Augmin promotes meiotic spindle formation and bipolarity in xenopus egg extracts. Proceedings of the National Academy of Sciences 108, 14473–14478 (2011). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [9].Lawo S. et al. Haus, the 8-subunit human augmin complex, regulates centrosome and spindle integrity. Current Biology 19, 816–826 (2009). [DOI] [PubMed] [Google Scholar]
- [10].Hotta T. et al. Characterization of the arabidopsis augmin complex uncovers its critical function in the assembly of the acentrosomal spindle and phragmoplast microtubule arrays. The Plant Cell 24, 1494–1509 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [11].Rayleigh L. On the instability of jets. Proceedings of the London mathematical society 1, 4–13 (1878). [Google Scholar]
- [12].Quéré D. Fluid Coating on a Fiber. Annual Review of Fluid Mechanics 31, 347–384 (1999). [Google Scholar]
- [13].Pérez de Castro I. & Malumbres M. Mitotic stress and chromosomal instability in cancer: the case for tpx2. Genes & cancer 3, 721–730 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [14].Uhlen M. et al. A pathology atlas of the human cancer transcriptome. Science 357 (2017). URL https://science.sciencemag.org/content/357/6352/eaan2507. https://science.sciencemag.org/content/357/6352/eaan2507.full.pdf. [DOI] [PubMed] [Google Scholar]
- [15].Hernández-Vega A. et al. Local Nucleation of Microtubule Bundles through Tubulin Concentration into a Condensed Tau Phase. Cell Reports 20, 2304–2312 (2017). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [16].Siahaan V. et al. Kinetically distinct phases of tau on microtubules regulate kinesin motors and severing enzymes. Nature Cell Biology 21, 1086–1092 (2019). [DOI] [PubMed] [Google Scholar]
- [17].Jiang H. et al. Phase Transition of Spindle-Associated Protein Regulate Spindle Apparatus Assembly. Cell 163, 108–122 (2015). URL 10.1016/j.cell.2015.08.010. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [18].Alfaro-Aco R, Thawani A. & Petry S. Biochemical reconstitution of branching microtubule nucleation. eLife 9, e49797 (2020). URL https://www.biorxiv.org/content/10.1101/700047v1.full. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [19].von Appen A. et al. Lem2 phase separation promotes escrt-mediated nuclear envelope reformation. Nature 1–4 (2020). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [20].Wühr M. et al. Deep proteomics of the xenopus laevis egg using an mrna-derived reference database. Current biology 24, 1467–1475 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [21].Desai A. & Mitchison TJ Microtubule polymerization dynamics. Annual review of cell and developmental biology 13, 83–117 (1997). [DOI] [PubMed] [Google Scholar]
- [22].Goren SL The instability of an annular thread of fluid. Journal of Fluid Mechanics 12, 309–319 (1962). [Google Scholar]
- [23].Boulogne F, Pauchard L. & Giorgiutti-Dauphine F. Instability and morphology of polymer solutions coating a fibre. Journal of Fluid Mechanics 704, 232–250 (2012). [Google Scholar]
- [24].Haefner S. et al. Influence of slip on the Plateau-Rayleigh instability on a fibre. Nature Communications 6, 4–9 (2015). 1501.02194. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [25].Tonon G. et al. High-resolution genomic profiles of human lung cancer. Proceedings of the National Academy of Sciences 102, 9625–9630 (2005). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [26].Neumayer G, Belzil C, Gruss OJ & Nguyen MD Tpx2: of spindle assembly, dna damage response, and cancer. Cellular and Molecular Life Sciences 71, 3027–3047 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [27].Cao L. et al. The structure of apo-kinesin bound to tubulin links the nucleotide cycle to movement. Nature communications 5, 5364 (2014). [DOI] [PubMed] [Google Scholar]
- [28].Shang Z. et al. High-resolution structures of kinesin on microtubules provide a basis for nucleotide-gated force-generation. Elife 3, e04686 (2014). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [29].Gillespie DT Exact stochastic simulation of coupled chemical reactions. The journal of physical chemistry 81, 2340–2361 (1977). [Google Scholar]
- [30].Caudron M, Bunt G, Bastiaens P. & Karsenti E. Spatial coordination of spindle assembly by chromosome-mediated signaling gradients. Science 309, 1373–1376 (2005). [DOI] [PubMed] [Google Scholar]
- [31].Brugués J, Nuzzo V, Mazur E. & Needleman DJ Nucleation and transport organize microtubules in metaphase spindles. Cell 149, 554–564 (2012). [DOI] [PubMed] [Google Scholar]
- [32].Decker F, Oriola D, Dalton B. & Brugués J. Autocatalytic microtubule nucleation determines the size and mass of xenopus laevis egg extract spindles. Elife 7, e31149 (2018). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [33].Feric M. et al. Coexisting liquid phases underlie nucleolar subcompartments. Cell 165, 1686–1697 (2016). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [34].Duprat C, Protiere S, Beebe A. & Stone HA Wetting of flexible fibre arrays. Nature 482, 510 (2012). [DOI] [PubMed] [Google Scholar]
- [35].Li Q. et al. Llpsdb: a database of proteins undergoing liquid-liquid phase separation in vitro. Nucleic Acids Research (2019). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [36].Taylor N. et al. Biophysical characterization of organelle-based RNA/protein liquid phases using microfluidics. Soft Matter 12, 9142–9150 (2016). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [37].Jawerth LM et al. Salt-dependent rheology and surface tension of protein condensates using optical traps. Physical review letters 121, 258101 (2018). [DOI] [PubMed] [Google Scholar]
- [38].Shell MS Thermodynamics and statistical mechanics: an integrated approach (Cambridge University Press, 2015). [Google Scholar]
- [39].Berry J, Brangwynne CP & Haataja M. Physical principles of intracellular organization via active and passive phase transitions. Reports on Progress in Physics 81 (2018). [DOI] [PubMed] [Google Scholar]
- [40].Greenberg MD Foundations of applied mathematics (Courier Corporation, 2013). [Google Scholar]
- [41].Alfaro-Aco R, Thawani A. & Petry S. Structural analysis of the role of tpx2 in branching microtubule nucleation. The Journal of Cell Biology 216, 983–997 (2017). URL http://jcb.rupress.org/content/216/4/983. http://jcb.rupress.org/content/216/4/983.full.pdf. [DOI] [PMC free article] [PubMed] [Google Scholar]
- [42].Song J. et al. Mechanism of how augmin directly targets the 7-tubulin ring complex to microtubules. J Cell Biol 217, 2417–2428 (2018). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [43].Murray AW & Kirschner MW Cyclin synthesis drives the early embryonic cell cycle. Nature 339, 275 (1989). [DOI] [PubMed] [Google Scholar]
- [44].Hannak E. & Heald R. Investigating mitotic spindle assembly and function in vitro using xenopu s laevi s egg extracts. Nature protocols 1, 2305 (2006). [DOI] [PubMed] [Google Scholar]
- [45].Case RB, Pierce DW, Hom-Booher N, Hart CL & Vale RD The directional preference of kinesin motors is specified by an element outside of the motor catalytic domain. Cell 90, 959–966 (1997). [DOI] [PubMed] [Google Scholar]
- [46].King M. & Petry S. Visualizing and analyzing branching microtubule nucleation using meiotic xenopus egg extracts and tirf microscopy. In The Mitotic Spindle, 77–85 (Springer, 2016). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [47].Gell C. et al. Microtubule dynamics reconstituted in vitro and imaged by single-molecule fluorescence microscopy. In Methods in cell biology, vol. 95, 221–245 (Elsevier, 2010). [DOI] [PubMed] [Google Scholar]
- [48].Schindelin J. et al. Fiji: an open-source platform for biological-image analysis. Nature methods 9, 676 (2012). [DOI] [PMC free article] [PubMed] [Google Scholar]
- [49].Hamon L, Curmi PA & Pastré D. High-resolution imaging of microtubules and cytoskeleton structures by atomic force microscopy. In Methods in cell biology, vol. 95, 157–174 (Elsevier, 2010). [DOI] [PubMed] [Google Scholar]
- [50].Nečas D. & Klapetek P. Gwyddion: an open-source software for spm data analysis. Open Physics 10, 181–188 (2012). [Google Scholar]
- [51].Thevenaz P, Ruttimann UE & Unser M. A pyramid approach to subpixel registration based on intensity. IEEE transactions on image processing 7, 27–41 (1998). [DOI] [PubMed] [Google Scholar]
- [52].Riback JA et al. Composition-dependent thermodynamics of intracellular phase separation. Nature 581, 209–214 (2020). [DOI] [PMC free article] [PubMed] [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Supplementary Materials
Movie 3. Branching microtubule nucleation visualized using TIRF microscopy. 0.1 μM TPX2 is added to X. laevis meiotic cytosol puri_ed from eggs. TPX2 coats the mother microtubule from which daughter microtubules then nucleate, leading to an autocatalytic branched network. Frame dimensions are 16 μm × 22 μm.
Movie 4. Rayleigh-Plateau instability of TPX2 on microtubules probed using AFM. 0.2 ± 0.1 μM GFP-TPX2 was spiked onto a mica surface covered with microtubules. The uniform film of TPX2 is established at t = 0 s, after which it breaks up into droplets. Frame dimensions are 2 μm × 2 μm.
Movie 1. Rayleigh-Plateau instability of TPX2 on microtubules visualized using TIRF microscopy. 1 μM GFP-TPX2 was spiked onto a passivated glass surface covered with Alexa568-labeled microtubules at t = 0 s. TPX2 coats the microtubule and then proceeds to break up into droplets. Scale bar is 1 μm.
Movie 2. Microtubule branches nucleating from TPX2 droplets on a preexisting microtubule. During acquisition, only the soluble tubulin channel was imaged to enable capturing nucleation and polymerization of branched microtubules at high temporal resolution. Scale bar is 5 μm.