Steady-State Nitrogen Isotope Effects of N₂ and N₂O Production in Paracoccus denitrificans

Abstract

Nitrogen stable-isotope compositions (δ¹⁵N) can help track denitrification and N₂O production in the environment, as can knowledge of the isotopic discrimination, or isotope effect, inherent to denitrification. However, the isotope effects associated with denitrification as a function of dissolved-oxygen concentration and their influence on the isotopic composition of N₂O are not known. We developed a simple steady-state reactor to allow the measurement of denitrification isotope effects in Paracoccus denitrificans. With [dO₂] between 0 and 1.2 μM, the N stable-isotope effects of NO₃⁻ and N₂O reduction were constant at 28.6‰ ± 1.9‰ and 12.9‰ ± 2.6‰, respectively (mean ± standard error, n = 5). This estimate of the isotope effect of N₂O reduction is the first in an axenic denitrifying culture and places the δ¹⁵N of denitrification-produced N₂O midway between those of the nitrogenous oxide substrates and the product N₂ in steady-state systems. Application of both isotope effects to N₂O cycling studies is discussed.

The importance of denitrification in microbial ecology, N₂O production, agricultural N loss and wastewater treatment has prompted a large body of research over the last 25 years. Although the basic pathway is well known (36),

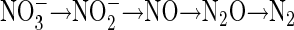1

the regulation and distribution of denitrification remain poorly understood. The sensitivity of denitrification to oxygen tension is of particular interest due to (i) the recent demonstration of denitrification under aerobic conditions (40, 41), (ii) increased N₂O production under these conditions (12, 20, 28), (iii) the importance of linked nitrification-denitrification in N cycling in natural environments (11, 14), and (iv) development of “single-sludge” wastewater treatment as a low-cost alternative to traditional strategies that employ separate aerobic and anoxic reactors (26, 34, 47).

The natural-abundance ¹⁵N ratios of nitrogenous materials have been used to identify or quantify denitrification activity in low-oxygen environments, including the marine water column (24, 53, 54, 55), groundwater (15), sediments (1), and soil (25). These studies exploit the variation in the ratio of ¹⁵N to ¹⁴N in nitrogenous material that results from the isotopic discrimination of denitrification, in which ¹⁴N reacts faster than ¹⁵N. Thus, natural-abundance ¹⁵N ratios provide a small (≈0.366% ¹⁵N) but endogenous in situ tracer of denitrification activity, in contrast to large ¹⁵N additions (50 to 99% ¹⁵N) traditionally used to trace biological N fixation and other N transformations. However, published estimates of the extent of isotopic discrimination, or isotope effect (ɛ), of denitrification range from 13 to 40‰, reflecting the variety of experimental methods and denitrifying cultures used (5, 6, 10, 13, 27, 50, 52). Because the ɛ of denitrification lies between those of other N transformations, such as N assimilation at 10‰ (9, 19, 30) and nitrification at 13 to 16‰ in situ (21) or 30 to 60‰ in vitro (27, 52), it is desirable that ɛ be better constrained. In addition, possible variation in ɛ as a function of oxygen tension has not been investigated heretofore.

We measured ɛ of denitrification in pure cultures of Paracoccus denitrificans under dissolved-oxygen concentrations between 0 and 1.2 μM. We also measured a unique ɛ for N₂O reduction in these cultures. For these experiments, we developed a simple, steady-state reactor which does not require a dedicated mass spectrometer or online sample preparation system, thus offering a flexible approach to investigators who do not routinely use stable-isotope techniques. The reactor was also used to measure oxygen isotope effects, which are reported elsewhere (4). This information expands the utility of stable isotope studies of denitrification in low-oxygen (<10 μM) environments. Here we describe the necessary steady-state fractionation models and the reactor configuration and performance and report the N stable-isotope effects for denitrification.

MATERIALS AND METHODS

Experimental design.

Continuous cultures were used to control the dissolved-oxygen concentration ([dO₂]) more easily and to exploit the simplicity of steady-state fractionation models, which relate kinetic ɛ values directly to the isotopic compositions of reactants and products. The isotopic composition of nitrogenous material is commonly expressed as a δ-value relative to atmospheric N₂:

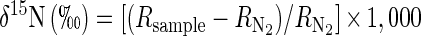2

where R = ¹⁵N/¹⁴N. In a single first-order reaction, in which the substrate pool is infinitely large, ɛ closely approximates the difference between the δ values of the substrate and product (16). The isotopic dynamics of steady-state anoxic denitrification may be idealized as such a one-step process:

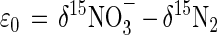3

where ɛ₀ is the overall ɛ of denitrification. Alternatively, this pseudo-ɛ may be partitioned: ɛ1, δ1ɛ2, δ2 δ¹⁵NO₃⁻ → δ¹⁵N₂O → δ¹⁵N₂ (4)

where ɛ₁ and ɛ₂ are the ɛ values of NO₃⁻ and N₂O reduction, respectively, and δ₁ and δ₂ are the isotopic compositions of the instantaneous products of the two reactions. At steady state, δ¹⁵N₂O and δ¹⁵N₂ are constant; thus, δ₁ and δ₂ both equal δ¹⁵N₂. Applying the principle of equation 3 to equation 4 yields the following relationships:

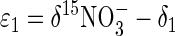5a

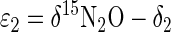5b

When δ¹⁵NO₃⁻, δ¹⁵N₂O, and δ¹⁵N₂ are measured experimentally and δ¹⁵N₂ is substituted for δ₁ and δ₂, equations 5a and 5b may be solved for ɛ₁ and ɛ₂, respectively. Note that ɛ₁ = ɛ₀, which is generally true for unbranched, nonreversible reaction pathways (37).

The approach described above allows the calculation of ɛ₁ and ɛ₂ by measuring δ¹⁵N of three species at a single steady state. Independent checks of ɛ₁ were calculated from isotopic mass balances of the reactor at multiple steady states. Because denitrification intermediates constituted very small fractions of reactor N at steady state, N isotope mass balances were written as follows:

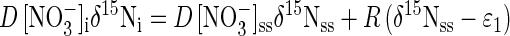6

where D is reactor dilution rate (time⁻¹), R is the denitrification rate ([N] time⁻¹), δ¹⁵N is the δ value of NO₃⁻, and the subscripts “i” and “ss” refer to initial and steady state, respectively. Equation 6, like equation 3, implies that ɛ₁ is equal to the steady-state difference between δ¹⁵NO₃⁻ and δ¹⁵N₂. Because R equals the product of D and the concentration of substrate consumed in a continuous culture (chemostat) at steady state, equation 6 can be rewritten to eliminate R:

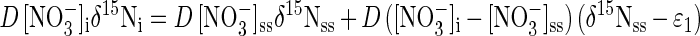7

and may be rearranged into linear form:

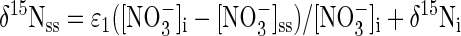8a

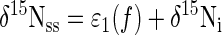8b

where f is the fraction of NO₃⁻ consumed at steady state and ɛ₁ equals the slope of steady-state δ¹⁵NO₃⁻ as a function of f (17). Different values of f were achieved by manipulating the dissolved-oxygen concentration ([dO₂]). Experimental [dO₂] treatments were 0, 0.1, 0.3, and 1.2 μM.

Reactor configuration.

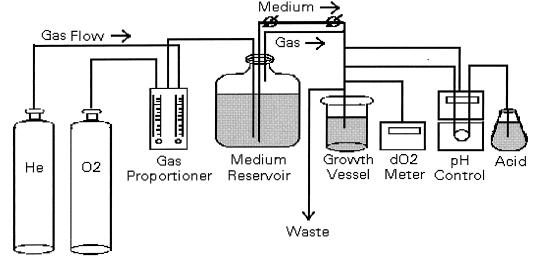
The reactor system consisted of a medium reservoir, growth chamber, waste carboy, and connecting tubing and flow controls (Fig. 1). The 20-liter Pyrex carboy in which media were sterilized also served as the reservoir. A heavy-gauge aluminum lid and rubber gasket were secured to the reservoir with a collar and screws. The lid contained ports for gas entry, gas and medium exit to the growth chamber, and venting. Gas mixing and flow to the reservoir were controlled by a gas proportioner (Alltech, Deerfield, Ill.). Gas was conducted to the reservoir in 1/8-in. stainless steel tubing, through a 0.5-μm nominal matrix filter (Nupro, Willoughby, Ohio) and a sparging stone. Medium flow from the reservoir to the growth chamber was caused by positive pressure in the reservoir, which was in turn controlled by the sparging rate. Two needle valves (Nupro) were added to enable finer control of medium flow rate to the growth chamber. This mode of medium delivery was chosen over pumping due to the difficulty of maintaining absolutely anoxic connections between steel tubing and peristaltic pump tubing.

FIG. 1.

Reactor configuration.

The growth chamber was a 2-liter Pyrex cylinder equipped with a magnetic stirrer and a stainless steel lid similar to the reservoir lid. In addition to gas and liquid entry ports, it contained a septum port for sampling by syringe, ports to accommodate a pH probe (Orion, Boston, Mass.), and a dO₂ probe (Ingold, Wilmington, Mass.), a 3/8-in. port for gas and liquid exit to the waste carboy, and a 3/8-in. port fitted with a shutoff valve for headspace sampling. The pH probe was connected to a pH controller (Cole Parmer, Chicago, Ill.), which activated a peristaltic pump equipped with microbore tubing to introduce HCl into the growth chamber. This tubing entered the growth chamber through the septum port. The waste port was situated to give the growth chamber a working volume of 1.75 liters. Waste liquid and gas were forced by positive pressure into the 20-liter vented waste carboy through 3/8-in. stainless steel tubing.

Organism and media.

P. denitrificans ATCC 17741, a relatively oxygen-sensitive, classic denitrifier, was chosen for the experiments (2). Cultures were purchased from the American Type Culture Collection (Rockville, Md.) and reconstituted in nutrient broth (Difco, Detroit, Mich.) at 30°C. Subcultures were frozen in glycerol and stored at −20°C until needed. The defined medium for continuous-culture experiments contained 30 mM nitrate and 20 mM acetate, which was the sole electron donor, carbon source, and limiting substrate (39). The composition of denitrification medium was as follows (in grams per liter): KNO₃, 3.03; CH₃COONa · 3H₂O, 2.72; K₂HPO₄, 0.8; KH₂PO₄, 0.3; NH₄Cl, 0.4; MgSO₄ · 7H₂O, 0.4; trace-elements solution, 2 ml liter⁻¹. The trace-elements solution was modified from that of Vishniac and Santer (48) and contained (in grams per liter) EDTA, 50.0; ZnSO₄, 2.2; CaCl₂, 5.5; MnCl₂ · 4H₂O, 5.06; FeSO₄ · 7H₂O, 5.0; (NH₄)₆Mo₇O₂₄ · 4H₂O, 1.1; CuSO₄ · 5H₂O, 1.57; CoCl₂ · 6H₂O, 1.61.

Reactor operation and sampling.

Denitrification medium was autoclaved in 16-liter batches at 121°C and 15 lb/in² for 80 min. Beginning immediately after sterilization, the reservoir was sparged with ultra-high-purity helium or O₂ in helium (Med-Tech Gases, Medford, Mass.). The growth chamber, dO₂ probe, liquid-sampling needle, waste vessel, and tubing were autoclaved and connected while hot. The pH probe was calibrated with standard buffers (Fisher Scientific, Fair Lawn, N.J.), surface sterilized with 70% ethanol, and inserted into the growth chamber. The chamber was filled to working volume with medium, which was sampled with a syringe when cool. The culture was then inoculated with 30 ml of stationary-phase P. denitrificans and grown in batch mode at 30°C to approximately 10⁸ cells ml⁻¹. Medium was then added at an appropriate dilution rate, and the pH was maintained at 8.0 by automatic addition of 1 M HCl. The [N₂O] of the headspace gas was monitored daily until it stabilized within a few ppm (by volume). At this point, the reactor was assumed to have reached steady state (see below).

Once steady state was established for a given experimental [dO₂] treatment, samples of each type were taken in triplicate. Liquid samples were drawn with a syringe and processed for either dissolved inorganic nitrogen concentrations (DIN) or cell N analysis. Samples (10 ml) for DIN analyses were filtered through 0.2-μm-pore-size cartridges (Gelman, Ann Arbor, Mich.), split into subsamples, and frozen until analysis of DIN or δ¹⁵N. Unfiltered liquid samples for cell counts were preserved in 5% formalin and stored at 10°C until analysis. Unfiltered samples for direct cell N measurement were processed immediately after sampling, as described below.

Gas samples were collected in preevacuated, U-shaped Pyrex tubes (34-ml volume) fitted on either end with vacuum stopcocks (Ace Glass, Vineland, N.J.). For N₂ collection, the U-tubes were coupled to the gas-sampling port of the growth chamber by using compression fittings with Teflon front ferrules and nylon back ferrules (Swagelok, Solon, Ohio). Each N₂ collection tube contained several granules of silica gel for cryogenic absorption of N₂ gas (33). With the growth chamber waste vent closed, U-tubes were opened and flushed with outgoing headspace gas (100 ml min⁻¹) for at least 5 min. Each grab sample was then isolated by closing first the stopcock near the sampling port and then the outlet stopcock. This order was necessary to maintain atmospheric pressure and to enable back-calculation of the N₂ production rate. Samples were stored in U-tubes for up to 2 days until purified and used for manometric determination of N₂.

N₂O was quantified by gas chromatography. Samples for gas chromatography were collected by flushing a 30-ml serum bottle with outgoing headspace gas via the gas sampling port. N₂O samples for δ¹⁵N and δ¹⁸O determination were collected by trapping N₂O out of the outgoing gas stream. This was necessary because the headspace [N₂O] was too low (≈0.1 μM) for grab samples of reasonable volume to yield the 2 to 6 μmol of N required for mass spectrometry. The N₂O trap was a U-tube packed with borosilicate glass beads, which increased the trap surface area and dispersed the gas flow enough to trap N₂O when chilled in liquid nitrogen (LN₂). The efficiency of the N₂O trap was verified by measuring zero N₂O in the trap effluent. CO₂ was removed from N₂O samples by a scrubber in line between the growth chamber and the N₂O trap. The scrubber consisted of a standard gas purification cartridge (Alltech) packed with a 3-cm layer of Carbosorb granules (Elemental Microanalysis, Manchester, Mass.) between two layers of indicating silica gel.

Cryogenic distillation.

Gas samples were purified by standard cryogenic techniques (7). U-tubes were first immersed in LN₂ to freeze the N₂ or N₂O sample onto silica gel or glass beads, respectively. The large overburden of helium carrier gas was then removed with a vacuum pump. N₂ samples were further purified of CO₂ and H₂O by a LN₂-cooled trap; O₂ was removed by passing the sample over copper filings at 550°C. The purified N₂ was quantified by using a capacitance manometer (MKS Baratron). N₂O samples were further purified of H₂O by using a glass trap cooled in an ethanol-dry ice slurry. Each N₂ or N₂O sample was refrozen in a Pyrex ampoule, sealed, and stored until analysis by continuous-flow isotope ratio mass spectrometry.

Analytical methods.

Nitrate [NO₃⁻] and nitrite [NO₂⁻] concentrations were measured by the spongy cadmium reduction method (22). The ammonia [NH₃ + NH_4⁺] concentration was determined by the colorimetric method of Strickland and Parsons (45). The δ¹⁵N of (NO₃⁻ + NO₂⁻) was measured by the ammonia diffusion method as modified by Sigman et al. (43).

The N in bacterial cells was quantified by acridine orange direct counting (18) and a conversion factor for cell N concentration, which was found by performing cell counting and direct cell N measurement on the same samples over a range of cell densities. Direct measurements of cell N were made with a Europa elemental analyzer. To prepare a sample containing 2 to 6 μmol of N, approximately 1.0 ml of cell suspension was filtered onto a precombusted 25-mm-diameter GF/F filter. The filters were dried at 55°C and packed in tin boats before analysis. Cell N was calculated from the following regression (r² = 0.8835, n = 4):

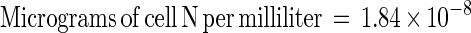9

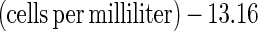N₂O production was monitored by using a Hewlett-Packard 5890A gas chromatograph equipped with an electron capture detector (23). A 1/8-in.-diameter stainless steel column packed with Hayesep Q 80/100 mesh was used at 40°C. The injector and detector temperatures were 100 and 350°C, respectively, and the carrier gas was 5% methane in argon at 30 ml min⁻¹. Under these conditions, N₂O eluted approximately 1.9 min after sample injection. Calibration curves were prepared daily by using a standard gas mixture of 101 ppm (volume) N₂O in N₂ (Scott Specialty Gases, Reading, Mass.). Standard injections were performed periodically to check for signal drift.

The δ¹⁵N of N₂O, N₂, and (NO₃⁻ + NO₂⁻) and the δ¹⁸O of N₂O were measured on a Finnigan Mat 251 mass spectrometer (55). Samples were conducted by helium carrier flow (50 ml min⁻¹) through Carbosorb and magnesium perchlorate traps to remove trace CO₂ and H₂O, respectively. The mass 29/28 ratio was measured for N₂ samples and expressed as δ¹⁵N relative to atmospheric N₂. Injections of working standard N₂ were made through a septum port in line with the carrier flow. For N₂O samples, the mass 45/44 ratio, yielding δ¹⁵N values, and the mass 46/44 ratio, yielding δ¹⁸O values, were both measured for each sample. The mass spectrometer was calibrated with a standard of pure N₂O gas kindly provided by T. Yoshinari, New York State Department of Health. N₂O δ values were expressed relative to the δ¹⁵N and δ¹⁸O of atmospheric N₂ and O₂, respectively.

Budget calculations.

Dissimilatory (denitrification) and assimilatory N budgets were calculated for the reactor system. Each term in the dissimilatory budget was expressed as a percentage of the NO₃⁻ supplied in the medium:

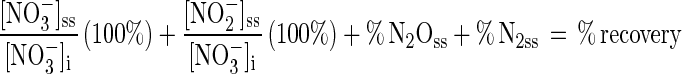10

The NO₃⁻ and NO₂⁻ terms were their respective steady-state concentrations (denoted by the subscript “ss”) expressed as percentages of the initial NO₃⁻ concentration. The N₂O term (%N₂O_ss) was the N₂O-N production rate expressed as a percentage of the NO₃⁻ supply rate:

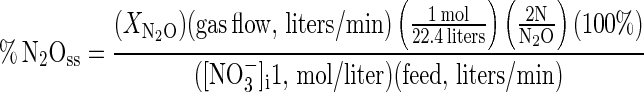11

where X_N2O is the mole fraction of N₂O in growth chamber headspace gas. The analogous N₂ term, %N_2ss, is shown below. The first factor in this equation was the amount of N contained in a 34-ml grab sample, which was measured manometrically during cryogenic distillation:

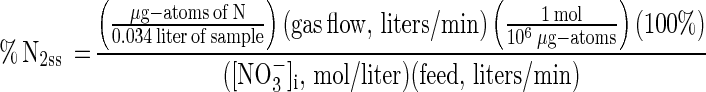(12)

The assimilatory N budget consisted of the sum of cell N and NH₃-N present at steady state, expressed as a percentage of NH₃ supplied in the medium. Dissolved organic nitrogen compounds which may have been synthesized from NH₃ were not included in the budget.

RESULTS

Reactor performance.

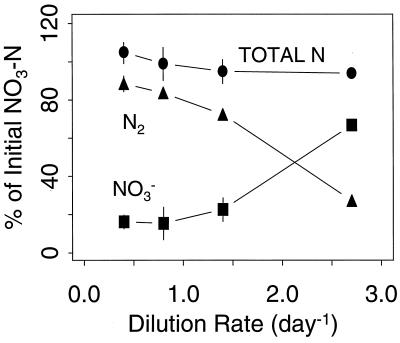
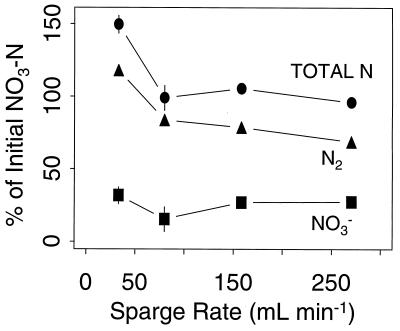
Dissimilatory N recovery from the reactor system was near 100% over a range of dilution rates (Fig. 2). Residual [NO₃⁻] increased with increasing dilution rate, but the cultures were not in danger of washout at the rates tested (39). Dissimilatory N recovery varied as a function of the sparging rate (Fig. 3), with particularly high N₂ recovery at the lowest sparging rate, as discussed below. Assimilatory N recovery was 80 to 100% over the same range of dilution and sparging rates (data not shown). Dilution and sparging rates of 0.7 day⁻¹ and 100 ml min⁻¹, respectively, were held constant in further experiments, in which variable [dO₂] was the sole experimental treatment.

FIG. 2.

Dissimilatory N budget as a function of the dilution rate. Symbols are means and SE (n = 3). NO₂⁻ and N₂O made up less than 1% of total N.

FIG. 3.

Dissimilatory N budget as a function of the sparge rate. Symbols are means and SE (n = 3).

Experimental results.

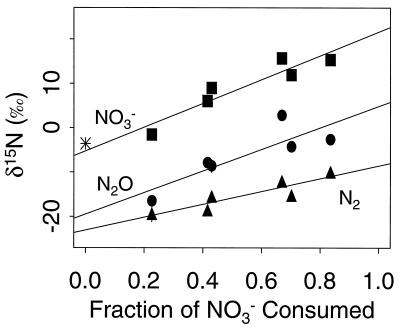
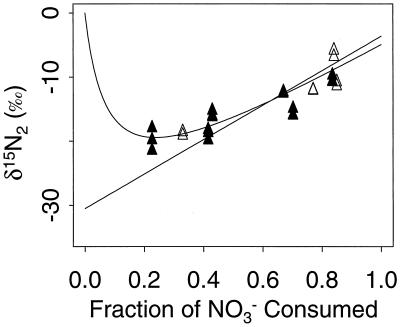
Under anoxic steady-state conditions, the reactor system yielded δ¹⁵NO₃⁻, δ¹⁵N₂O, and δ¹⁵N₂ of 15.5‰ ± 0.3‰, 0.08‰ ± 3.1‰, and −11.0‰ ± 1.2‰, respectively (mean ± standard error [SE], n = 6). According to these data and equations, 5a and 5b, ɛ₁ = 26.5‰ ± 1.2‰ and ɛ₂ = 11.1‰ ± 3.1‰ under anoxic conditions. The independent estimates of ɛ₁ calculated from equation 8b and δ¹⁵NO₃⁻ and δ¹⁵N₂O from multiple steady states at different [dO₂] are similar although more variable (26.9‰ ± 2.6‰ and 24.3‰ ± 4.0‰, respectively). However, ɛ₁ calculated from δ¹⁵N₂ from multiple steady states was ≈14‰ (Fig. 4). This discrepancy and the high N₂ recovery at the low sparging rate (Fig. 3) suggested that significant isotopic signal from atmospheric N₂ had biased the δ¹⁵N₂ values. To quantify this bias, the response of measured δ¹⁵N₂ to systematic air contamination, or a “handling blank,” was simulated (Fig. 5). The expected δ¹⁵N₂ was first calculated by subtracting the slope of the NO₃⁻ regression in Fig. 4 (i.e., ɛ₁) from points on that regression. The resulting straight line represents the δ¹⁵N₂ expected from constant fractionation and no air contamination. The expected δ¹⁵N₂ for the highest [dO₂] treatment (the least biogenic N₂) was compared to the measured value, and the amount of atmospheric N₂ necessary to create the difference was calculated by mass balance. Simulation of constant fractionation with constant air contamination was then made by adding the isotopic contribution of this amount (0.5 μmol) of atmospheric N₂ to the constant-fractionation values. The resulting curve fits very closely with the measured δ¹⁵N₂, suggesting that both ɛ₁ and the amount of air contamination during sampling were constant for all [dO₂] treatments.

FIG. 4.

δ¹⁵N as a function of NO₃⁻ consumption (f). Different fractions of f were caused by dO₂ treatments. Symbols are means and SE (n = 3). The slope and SE of the slope of regressions of δ¹⁵N versus f are 14.5 and 2.0 (N₂), 24.3 and 4.0 (N₂O), and 26.9 and 2.6 (NO₃⁻). The asterisk represents the δ¹⁵N of the NO₃⁻ supplied (−3.6‰).

FIG. 5.

Comparison between measured δ¹⁵N₂ from dO₂ experiments (solid triangles) and dilution rate experiments (open triangles), the expected δ¹⁵N₂ given constant fractionation (straight line), and the expected value with a constant amount of air contamination (curved line). See the text for details.

To compare ɛ₁ and ɛ₂ between dO₂ treatments, the δ¹⁵N₂ data were transformed to eliminate the effect of air contamination. This was done by subtracting from each measured δ¹⁵N₂ value the isotopic bias inherent in 0.5 μmol of atmospheric N₂. The corrected δ¹⁵N₂ values for each treatment were then averaged, and these means were subtracted from the corresponding mean values of δ¹⁵NO₃⁻ and δ¹⁵N₂O to yield ɛ₁ and ɛ₂, respectively (Table 1). Analysis of variance indicates that ɛ₁ and ɛ₂ varied more between reactor runs than between [dO₂] treatments. The 1.2 μM dO₂ treatment was excluded from the analysis of variance because low biogenic N₂ production made δ¹⁵N₂ very sensitive to the mass balance correction (Table 1). Given the supporting evidence for systematic air contamination and the good agreement between intratreatment estimates of ɛ₁ and regression models of intertreatment δ¹⁵N₂O and δ¹⁵NO₃⁻ (Fig. 4), ɛ₁ and ɛ₂ were assumed to be constant over the range of dO₂ treatments and are reported as the grand means 28.6‰ ± 1.9‰ and 12.9‰ ± 2.6‰, respectively (means ± SE, n = 5).

TABLE 1.

Isotope effects for different dO₂ treatments, calculated from transformed δ¹⁵N₂

ɛ	ɛa at dO2 of:
	0 μM	0 μM	0.1 μM	0.1 μM	0.3 μM	1.2 μM
ɛ1	29.3 ± 0.2a	26.6 ± 0.8b	28.8 ± 1.0a	29.7 ± 0.7a	28.7 ± 1.1a	12.4 ± 54b
ɛ2	16.5 ± 0.8c	8.6 ± 0.9d	11.3 ± 1.7e	13.5 ± 0.9f	14.7 ± 1.3cf	−2.6 ± 54b

^aData are means ± SE (n = 3). Means sharing a letter are not significantly different (P < 0.05) according to the least-significant-difference test for planned comparisons (44). Columns of data with the same dO₂ concentration represent results of different runs. 

^bSee the text. 

DISCUSSION

The value of ɛ₁ reported here falls well within the range in the literature (Table 2). All estimates of biological fractionation are well below 90‰, the maximum theoretical fractionation of N—O bond rupture (50). The precision of ɛ₁ as measured in our steady-state system compares favorably with that measured by batch culture experiments (5, 13), in which ɛ₁ was calculated by using the classic Rayleigh equation, which is sensitive to error in f (46). The value and precision of ɛ₁ are similar to those reported by Mariotti et al. (27), who measured f by the acetylene block technique.

TABLE 2.

Measured values of the overall isotope effect of denitrification

Experimental system	ɛ (‰)	Reference
Pseudomonas denitrificans	13–21	13
Pseudomonas stutzeri	20–30	50
Nitrosomonas europaeaa	35–36	52
Soil (amended with glucose)	14–23	5
Soil (unamended) at 20°C	29.4 ± 2.4	27
Soil (unamended) at 30°C	24.6 ± 0.9	27
Eastern tropical North Pacific	30–40	10
Groundwater	15.9	6

^aAssumes that N₂O is formed by NO₂⁻ reduction. 

The results reported here indicate that ɛ₁ in P. denitrificans is constant over a range of dO₂ concentrations. This constancy supports the validity of using ɛ₁ to quantify, identify, or rule out denitrification fluxes in environments containing [dO₂] gradients. However, other work indicates that biological kinetic fractionation can vary with environmental conditions. For sulfate reduction, Rees (38) hypothesized that the greater fractionation often measured in situ is due to slower growth in the field than in pure culture, but our dilution rate experiments indicated that the growth rate per se did not cause ɛ₁ to vary (Fig. 5). Bryan et al. (8) showed that the overall ɛ of denitrification does vary with the denitrification rate in whole cells and cell extracts of Pseudomonas stutzeri limited by [NO₂⁻], increasing to a maximum value of 25‰ ± 3.2‰ at initial [NO₂⁻] > 2.5 mM. The electron acceptor concentrations in our experiments were well within the asymptotic range reported by Bryan et al. (above 2.5 mM). These authors also found a negative correlation between ɛ and denitrification rate when the rate was increased by higher electron donor concentrations.

The isotopic composition of N₂O in our experiments was quite distinct from both δ¹⁵N₂ and δ¹⁵NO₃⁻. The combined effects of ɛ₁ and ɛ₂ resulted in δ¹⁵N₂O being about 13‰ heavier and 15‰ lighter than δ¹⁵N₂ and δ¹⁵NO₃⁻, respectively, at steady state. To our knowledge, this is the first report of an isotope effect for nitrous oxide reduction in a denitrifying system. Yoshida et al. (53) cited unpublished data which yielded a value of 27‰ for ɛ₂ in P. denitrificans, but they did not specify whether the bacteria were supplied with N₂O, NO₂⁻, or NO₃⁻. Yamazaki et al. (51) reported a maximum ɛ of 39‰ for N₂O reduction by the N₂ fixer Azotobacter vinelandii, but nitrogenase, not nitrous oxide reductase, appeared to be responsible for the observed activity.

The expression of isotopic fractionation by P. denitrificans was strongly influenced by [dO₂]. Within the narrow range between 0 and slightly more than 1.2 μM dO₂, the δ¹⁵N of NO₃⁻ and N₂O varied up to 26‰ and δ¹⁵N₂ probably varied to an equal extent (Fig. 4). However, the usefulness of [dO₂] as a predictor of δ¹⁵N in denitrifying environments may be limited to the extent to which it controls NO₃⁻ consumption. The expression of ɛ in natural and applied systems will also depend on the distribution of denitrifiers. P. denitrificans is very sensitive to dO₂ in comparison to some denitrifiers, such as Comamonas sp. (35) and Thiosphaera pantotropha (39), which under aerobic conditions maintain 40 and 25% of their anaerobic denitrifying activity, respectively. However, Pseudomonas fluorescens, which frequently dominates denitrifying environments, denitrifies over approximately the same range of [dO₂] as P. denitrificans (28). Chemostat studies of P. halodenitrificans revealed only slightly higher tolerance to dO₂, to ∼2 μM (20).

It is hoped that ɛ₁ and ɛ₂ may be used to help distinguish between denitrification and nitrification as sources of N₂O and may serve as in situ tracers of both processes. For example, if the δ¹⁵N of the substrate pools for both processes, NO₃⁻ and NH₄⁺, respectively, are assumed to be zero, then denitrification- and nitrification-produced δ¹⁵N₂O in open systems at steady state would be ca. −15‰ and −65‰, respectively (52). However, the isotopic composition of substrate pools in real systems could obscure this distinction. The δ¹⁵N of NO₃⁻ and NH₄⁺ vary from −23 to +43‰ and −20 to +50‰, respectively, depending upon the source and the combined fractionation effects of redox reactions in the environment (49). Given these ranges, it is possible that denitrification- and nitrification-produced δ¹⁵N₂O would be indistinguishable. Linked nitrification-denitrification may also confuse δ¹⁵N₂O signatures by increasing the range of potential substrate molecules, which may in turn may have variable δ¹⁵N (56). In environments such as sediments and biofilms, spatial linkage between nitrification and denitrification is on the order of 1 mm or less, and in single microorganisms such as Thiosphaera pantotropha, which both denitrifies and nitrifies in aerobic environments, N₂O may be produced from NH₄⁺, NO₃⁻, and NO₂⁻ (3). However, in many environments (9, 19, 29, 31), the δ¹⁵N of substrate pools can be constrained within a range of 10‰ (see, e.g., references 1, 32, and 42), and the isotopic composition of N₂O could provide a simple index to the relative contribution of the two processes producing N₂O.