Bacterivory Rate Estimates and Fraction of Active Bacterivores in Natural Protist Assemblages from Aquatic Systems

Abstract

Unlike the fraction of active bacterioplankton, the fraction of active bacterivores (i.e., those involved in grazing) during a specified time period has not been studied yet. Fractions of protists actively involved in bacterivory were estimated assuming that the distributions of bacteria and fluorescently labeled bacteria (FLB) ingested by protists follow Poisson distributions. Estimates were compared with experimental data obtained from FLB uptake experiments. The percentages of protists with ingested FLB (experimental) and the estimates obtained from Poisson distributions were similar for both flagellates and ciliates. Thus, the fraction of protists actively grazing on natural bacteria during a given time period could be estimated. The fraction of protists with ingested bacteria depends on the incubation time and reaches a saturating value. Aquatic systems with very different characteristics were analyzed; estimates of the fraction of protists actively grazing on bacteria ranged from 7 to 100% in the studied samples. Some nanoflagellates appeared to be grazing on specific bacterial sizes. Evidence indicated that there was no discrimination for or against bacterial surrogates (i.e., FLB); also, bacteria were randomly encountered by bacterivorous protists during these short-term uptake experiments. These analyses made it possible to estimate the ingestion rates from FLB uptake experiments by counting the number of flagellates containing ingested FLB. These results represent the first reported estimates of active bacterivores in natural aquatic systems; also, a proposed protocol for estimating in situ ingestion rates by protists represents a significant improvement and simplification to the current protocol and avoids the tedious work of counting the number of ingested FLB per protist.

Bacteria and their protistan grazers are of major importance to the functioning of pelagic foodwebs and biogeochemical cycles (1, 28). A highly significant fraction of the production by primary producers (20 to 50%) is channeled through bacteria (1, 5). Phagotrophic protists, including flagellates and ciliates, have been reported as the dominant bacterivores in most aquatic ecosystems (1, 19, 27, 38). The importance of bacterivores in regulating bacterial abundance, nutrient cycling, and linking lower and upper trophic levels in aquatic food webs has been emphasized in several recent studies (1, 30, 33).

Many studies have shown that only a fraction of bacteria are actually active during a determined time period (6, 11). However, these studies have not reported on the fraction of protists actually grazing on bacteria. McManus and Okubo (20) indicated that, during uptake experiments of surrogate food particles performed with natural populations, there are always protists that do not ingest bacterial surrogates; some of them may not graze on the offered surrogates, and some others may not have encountered any surrogate food particle. Thus, it is not correct to consider protists not containing ingested surrogates to be nongrazers (20). However, active grazers would be those protists ingesting bacteria (total bacteria = natural bacteria + surrogate particles) during the uptake experiments.

Recently, the importance of nanoprotists as herbivores has been reported (39). The question of which protists are bacterivores, herbivores, or both has yet to be answered. In addition, some flagellates are autotrophs, while some others are exclusively phagotrophs; still others are mixotrophs and can behave as either autotrophs or heterotrophs (2, 31, 32). The actual percentages of bacterivorous protists, herbivores, autotrophs, and mixotrophs are still unknown. Besides, it has been reported that some protists might be preferentially ingesting specific prey types (12–14, 22). How many protists are actually preying on a determined species, prey type, or prey size? The fraction of protists actually ingesting bacteria or specific prey during a given time period is still unknown.

Various methods have been used to estimate in situ bacterivory. Some techniques rely on monitoring changes in bacterial numbers during long-term incubations (12 to 48 h) after manipulations, e.g., size fractionation or dilution of water samples, or the addition of metabolic inhibitors, to reduce or eliminate protistan grazing (17, 35, 45). Another approach has been to quantify the protistan ingestion of labeled analogues of bacterioplankton, either bacterium-sized fluorescent microspheres, fluorescently labeled bacteria (FLB), or radiolabeled bacterial cells (18, 25, 36, 44). Labeled bacterial analogues have been used in short-term uptake assays (36, 38) or long-term disappearance experiments (18, 25). There are problems associated with each of these approaches: significant changes in the original microbial assemblage during long-term incubations (10), experimental artifacts due to manipulation (10), and the discriminatory feeding on added bacterial analogues (14, 26, 36, 38). Radiolabeled prey tracer experiments have additional problems due to difficulties in separating the labeled grazers from the labeled prey biomass. Short-term uptake of FLB by natural assemblages of protists is, at present, the most commonly used technique for estimating in situ bacterivory (3, 8, 34, 38), and the possibility of discriminatory feeding is minimized by labeling natural bacterial assemblages.

This study was designed to investigate the fraction of protists in natural assemblages which are actually involved in bacterivory during a specific time period. Uptake estimates were compared to experimental results in order to check the accuracy of these estimates. The number of protists with ingested FLB were used for these comparisons. Both natural flagellate and ciliate assemblages were analyzed. The results suggested that a significant fraction of the heterotrophic nanoflagellates in natural assemblages might not be feeding on bacteria during a given time period. In addition, a new protocol for estimating ingestion rates by natural assemblages of protists is proposed. This protocol may significantly simplify the tedious work of counting ingested labeled bacterial surrogates (e.g., FLB) in protist grazers.

MATERIALS AND METHODS

Experimental protocol.

Protistan grazing was studied by uptake experiments with monodispersed FLB as bacterial analogues according to the method of Sherr et al. (36).

FLB were prepared from natural bacterioplankton assemblages stained with 5-([4,6-dichlorotriazin-2-yl]amino)fluorescein (DTAF), as described by Sherr et al. (36).

FLB uptake experiments were carried out in 400-ml Whirl-Pak bags presoaked in 10% (vol/vol) HCl and copiously rinsed with deionized water (36). Experiments were run in the dark at the in situ temperature of the sample. Aliquots were taken at determined time periods and preserved by the Lugol-Formalin decoloration technique (40). For ingestion rate estimates only those samples in the linear portion of the uptake curve were considered; during this period the incubation time is shorter than the digestion time for FLB (13, 14, 37). Per-cell ingestion rates of bacteria (bacteria protist⁻¹ minute⁻¹) were calculated by multiplying the average FLB number protist⁻¹ by the inverse of the fraction of FLB to total bacteria in the sample and dividing it by the incubation time (36).

Protists were enumerated by DAPI staining according to the method of Porter and Feig (29) as modified by Sherr et al. (36). FLB were counted on unstained 0.2-μm polycarbonate filters. FLB in protist food vacuoles were visualized in DAPI (4′,6-diamidino-2-phenylindole)-stained preparations as previously described (36). Bacterial abundance was estimated by the acridine orange direct count method (15). A minimum of 200 cells was inspected per slide.

The fraction of FLB with respect to the total bacterial number in the sample was kept as low as possible according to the recommendations of McManus and Okubo (20). In this study, the effects of FLB/total bacteria ratios on grazing rates were not analyzed. The aim of this study was to focus on standard FLB uptake experiments where the sample should be minimally perturbed. During the experiments shown in this study, the final concentrations of FLB were <15% of the nonlabeled bacterial density.

Natural samples used in this study were collected from several locations in different countries as previously described: a salt marsh estuary (Sapelo Island, Ga.) (12, 36), the Butrón River and La Salvaje Beach (Basque Country, Spain) (13), and a cruise off the Oregon coast (14).

Active versus inactive protists.

During this study, actively grazing protists were considered those ingesting any bacteria (total bacteria = natural bacteria + FLB) during uptake experiments (i.e., during a limited time period). Protists ingesting no bacteria (total bacteria) during the determined time period were considered inactive protists during that period. Herein, the term grazer and the terms bacterivore and nongrazer will be used to mean actively grazing protists and nonactive protists, respectively, as described above.

Estimates of the fraction of active grazers.

The distribution of ingested bacteria (total bacteria = natural bacteria + FLB) per protist was assumed to follow a Poisson distribution. Thus, the fraction of active grazers over a time period could be estimated as:

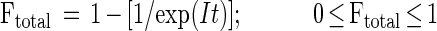1

where F_total is the fraction of protists ingesting bacteria during the incubation time, I is the ingestion rate on total bacteria by protists, and t is the incubation time. F_total represents the fraction of protists ingesting any bacteria, including both natural bacteria and FLB. Thus, F_total is the fraction of active bacterivores during that experimental time period.

During short-term FLB uptake experiments, FLB represent a small fraction of the total bacteria during the experiments. The fraction of protists ingesting FLB during an FLB uptake experiment could also be estimated from a Poisson distribution as:

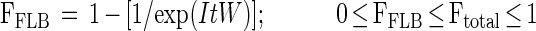2

where F_FLB is the fraction of protists ingesting FLB during the incubation time and W is the FLB/total bacteria ratio. Those protists with ingested FLB are included in the set of protists with ingested bacteria (total bacteria). The above assumptions were verified by comparing F_FLB estimates and the fraction of protists with ingested FLB from protist counts during short-term FLB uptake experiments.

Equation 2 can be transformed in order to calculate ingestion rates as follows:

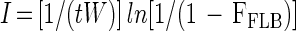3

where ln represents the natural logarithm. Thus, ingestion rates can be estimated from equation 3 if the fraction of protists with ingested FLB is obtained from short-term FLB uptake experiments.

Statistical and data analysis.

Statistics were performed as described by Sokal and Rohlf (43) unless otherwise indicated. Analysis of variance was used to compare fractions of grazers from different ecosystems. Two-way analysis of variance was used for paired comparisons among fractions of grazers in experiments with FLB from <0.6-μm-diameter bacteria and whole bacterial assemblages. When values from several orders of magnitude were analyzed, logarithmic transformations were performed in order to avoid biases (43). Model II regression analysis (41) was used as a criterion to compare experimental data and estimates (21). Confidence limits of 95% were used to establish the existence of significant differences among slopes. Nonlinear regressions were performed by using the package LSTSQ (4) in order to fit the data to nonlinear equations (i.e., Monod-like equations). Monod-like equations (24) were as follows: F = F_m t/(K_t + t), where t is the time (in minutes), F is the fraction of active grazers during t, F_m is the maximum fraction of grazers (F_m ≤ 1) in the analyzed protist assemblage, and K_t is a half-saturating constant. Since the number of prey (FLB and bacteria) remains practically constant during short-term uptake experiments, time is directly proportional to encountered bacterial prey (i.e., the prey available for ingestion); thus, Monod-like equations represent a good model for the fraction of active grazers versus time.

RESULTS

Testing estimated results.

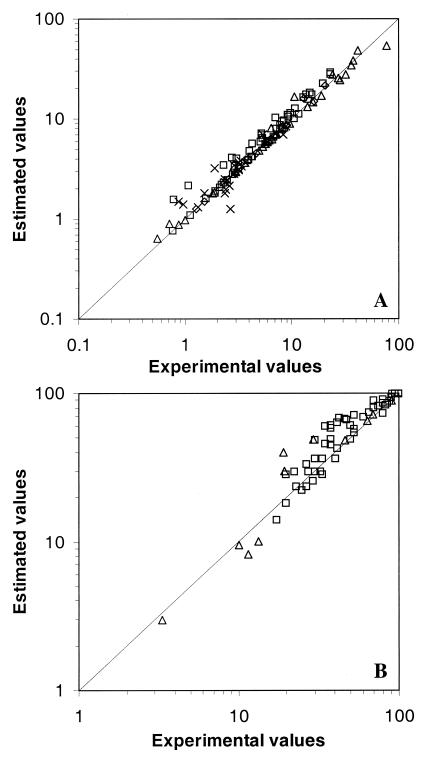
The number of protists with ingested FLB was the parameter used for comparing experimental data and estimates. Figure 1 compares the percentage of protists with ingested FLB from field experiments with the estimated values (equation 2). Results from four different environments are shown: a salt marsh estuary, river water, and marine samples from two different origins. Comparisons indicate tight relationships (P ≪ 0.001) between the experimental fraction of protists with ingested FLB and that fraction estimated from equation 2 (Fig. 1). For heterotrophic flagellates (Fig. 1A), the regression coefficient was 1.01 (95% confidence limits were 0.97 and 1.05). For ciliates (Fig. 1B), the regression coefficient was 1.00 (95% confidence limits were 0.90 and 1.10). These results indicate no significant differences between these regression coefficients and the optimum correspondence at the 1:1 slope. These results validated the above estimates and allowed the use of these data for further analyses.

FIG. 1.

Comparison of the percentage of protists, both flagellates (A) and ciliates (B), with ingested FLB obtained experimentally and estimated from Poisson distributions (equation 2 [see Materials and Methods]). Data are from different aquatic systems: Sapelo Island (□), Butron River (▵), Salvaje Beach (◊), and off the Oregon coast (×). Lines represent the 1:1 slope.

Fraction of active grazers.

Equation 1 estimates of the fractions of active bacterivores in natural assemblages of heterotrophic nanoflagellates from different aquatic systems varied from 7 to 100% of the total number of heterotrophic nanoflagellates (Table 1). These fractions depended on the bacterial surrogates used in the experiment and the ecosystem under study. The experiments performed with FLB from a <0.6-μm-diameter natural bacterial assemblage (12) are an example; these experiments led to lower (P < 0.01) fractions of active nanoflagellate grazers than the same samples incubated with FLB prepared from the whole natural bacterial assemblage (Table 1). Significant differences between the aquatic systems analyzed in this study were also observed (Table 1). Oligotrophic systems appeared to present lower (P < 0.01) percentages of actively grazing nanoflagellates (i.e., the Oregon samples, with an average of 24%) than richer systems (i.e., the La Salvaje, Sapelo, and Butron samples, with averages of 42, 54, and 60%, respectively).

TABLE 1.

Fractions of active bacterivorous nanoflagellates and ciliates from different aquatic systems

Ecosystem	Protist assemblage	Fraction of active bacterivoresa
		Avg (SD)	Range
Sapelo Island (United States)	Flagellates	0.54 (0.27)	0.12–0.99
	Flagellatesb	0.21 (0.11)	0.07–0.34
	Flagellatesc	0.44 (0.16)	0.16–0.63
	Cilates	1.00 (0.00)
Butron River (Spain)	Flagellates	0.60 (0.32)	0.10–1.00
	Ciliates	0.90 (0.19)	0.44–1.00
Salvaje Beach (Spain)	Flagellates	0.42 (0.19)	0.17–0.82
Off Oregon coast (United States)	Flagellates	0.24 (0.10)	0.11–0.45

^aFraction of active bacterivorous nanoflagellates in the protist assemblage under study for each location. Protist assemblages were visually differentiated during counting. 

^bExperiments performed with FLB of <0.6 μm in diameter from the natural bacterial population. 

^cExperiments corresponding to those of footnote b, but with FLB from the whole natural bacterial community. 

The fraction of active bacterivorous ciliates appeared to be higher than the one of flagellates. Most of ciliates found in the samples appeared to be actively grazing on bacteria (Table 1). This is probably because ciliates can be in most cases distinguished in morphological groups during counting; thus, some nonbacterivorous species can be left out during the counting procedure. Heterotrophic nanoflagellates have mostly nondistinctive morphotypes under epifluorescence illumination. Bacterivorous ciliates from the Sapelo samples were mostly oligotrichids (12), and approximately 100% of them were active bacterivores (Table 1). Ciliates from the Butron samples were almost exclusively scuticociliates (13), and between 44 and 100% (average, 90%) were actively grazing on bacteria (Table 1) during these experiments.

Fraction of grazers over time.

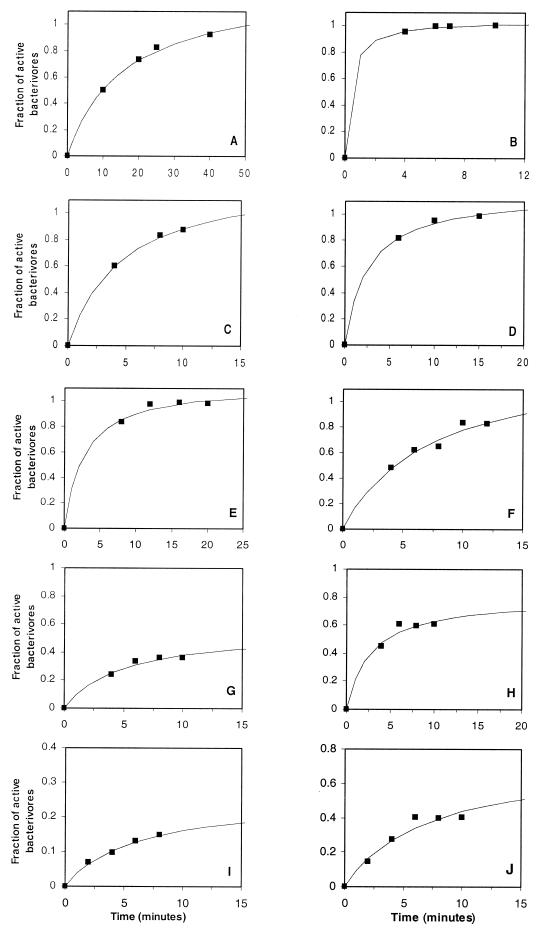
The fraction of active grazers was related to the incubation time during short-term FLB uptake experiments. The fraction of predator protists showed a saturation over time and could be represented by a Monod equation. Figure 2 shows some representative examples and the saturating curves fitting these data.

FIG. 2.

Ten representative examples of the fraction of active bacterivorous nanoflagellates versus incubation time. Saturating curves fitting the plotted data are also shown. Samples of experiments were from the Butron River (Spain) (panels A to D) and from Sapelo Island (panels E to J). Results presented in panels G and I were from samples inoculated with <0.6-μm-diameter FLB, and panels H and J represent the corresponding experiments with FLB made from the whole natural bacterial community. All other experiments were performed with FLB prepared from the whole natural bacterial population.

Ingestion rate estimates from short-term uptake experiments.

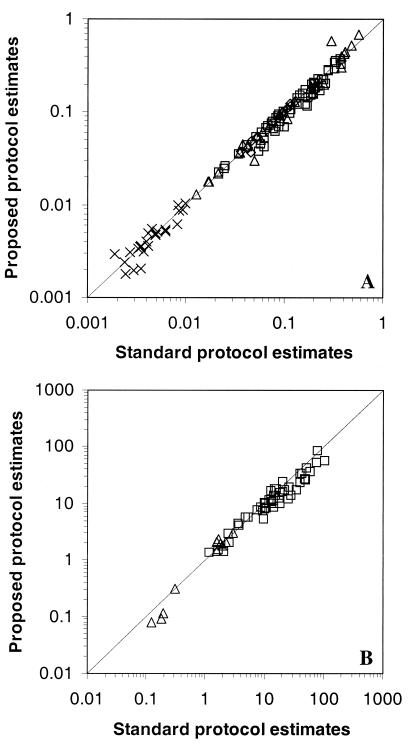
Bacterivory rate estimates were computed by using equation 3. Bacterivory rates were also calculated by following the standard protocol described by Sherr et al. (36). Rates obtained from both procedures were compared (Fig. 3). The results showed that the standard values and the equation 3 estimates were highly correlated: r² = 0.98 (n = 124, P ≪ 0.001) for flagellates and r² = 0.94 (n = 62, P ≪ 0.001) for ciliates. Regression analysis showed no significant differences from the 1:1 slope (Fig. 3). Regression coefficients were 0.99 (95% confidence limits were 0.97 and 1.01) for flagellates and 1.02 (95% confidence limits were 0.96 and 1.08) for ciliates.

FIG. 3.

Comparison of bacterivory rates obtained from the standard protocol (according to the method of Sherr et al. [36]) and from estimates from equation 3 (proposed protocol). Data are from flagellates (A) and ciliates (B) and from different aquatic systems as follows: Sapelo Island (□), Butron River (▵), Salvaje Beach (◊), and off the Oregon coast (×). Lines represent the 1:1 slope. Bacterivory rates are expressed in bacteria protist⁻¹ minute⁻¹.

DISCUSSION

Phagotrophic protists, including flagellates and ciliates, are the dominant bacterivores in most aquatic ecosystems (1, 19, 27, 38). Although there are numerous studies on ingestion rates by bacterivores, the actual number of flagellates and ciliates actively grazing during a given time period has not been studied. Although tedious, determination of the short-term uptake of FLB is the most commonly used technique to estimate in situ bacterivory rates. This study attempts to estimate the fraction of active grazers in natural protistan communities during short-term FLB uptake experiments. These estimates were performed by assuming that the ingested bacteria per protist follows a Poisson distribution. Estimates obtained in this way closely matched experimental results for both flagellates and ciliates (Fig. 1), thus corroborating the validity of the working hypothesis: the number of ingested bacteria and FLB per protist are distributed according to a Poisson distribution. These estimates can be a useful tool to better understand the behavior of natural protist assemblages in aquatic systems.

If bacteria are distributed and encountered randomly, at any given time the number of bacteria per protist should follow a Poisson distribution. According to a previous study (20) the number of ingested labeled surrogates per protist did not follow a Poisson distribution. The number of protists with no labeled surrogates ingested highly exceeded expectations based on the Poisson distribution (20). McManus and Okubo (20) used fluorescent beads as labeled bacterial surrogates. This could explain the excess of protists without ingested surrogates in their experiments, since lower grazing rates on beads than on FLB have been reported (24, 34, 36). As an example, the present study has shown (Table 1) the effect of using different bacterial assemblages on the estimates of the fraction of actively grazing protists.

In this study, the number of bacteria ingested per protist follows a Poisson distribution, and no discrimination for or against the labeled surrogates (i.e., FLB) by grazers was detected. In this scenario, protists with no ingested bacteria (total bacteria) should be either nongrazers or inactive bacterivores. Since the total bacterial population (nonlabeled and labeled bacterial particles) was considered during the analyses, protists with no ingested bacteria could be considered inactive grazers over a given time period. Those protists without FLB ingested cannot be considered nongrazers (20). Nongrazing protists could also be, for instance, autotrophs (1, 33). Inactive bacterivores could be, for instance, protists grazing during the incubation period on other available prey types, such as phytoplanktonic cells (33, 39). Those protists in resting stages of their life cycle could also be classified as inactive grazers. Temporal nonfeeders can also be those protists in reproductive stages during which they do not eat (9). At bacterial abundances found in most aquatic systems (>10⁵ bacteria ml⁻¹) a common phagotrophic nanoflagellate actively grazing is likely to encounter at least several bacteria during a typical short-term FLB uptake experiment (9, 11a, 42). More likely, protists might not encounter a labeled bacterial surrogate during that incubation period (20), since FLB represent only a small fraction of the total bacterial population during FLB uptake experiments. However, the lack of ingested FLB has not been used for characterizing inactive grazers. Active grazers are those protists ingesting bacteria during a short-term uptake experiment; they might or might not ingest labeled surrogates.

In natural aquatic systems, a significant percentage of protists may not be grazing on bacteria. From 7 to 100% (Table 1) of heterotrophic nanoflagellates and from 44 to 100% of ciliates were estimated to be actively grazing on bacteria during specific time periods. These wide percentage ranges suggest that some factors might be affecting whether or not a protist is actively grazing on bacteria. Environmental factors influence bacterivory (9, 33), so they certainly affect the number of active bacterivores in a protist assemblage. In this study, distinct ecosystems showed significant differences in percentages of active grazers (Table 1). At present, however, the effect of environmental factors on active grazers is unknown, and further work is needed. For example, bacterial prey can affect the percentage of active grazers in natural assemblages of protists. Previous publications have reported selective grazing by bacterivores as a result of size and other prey characteristics (12, 14, 22, 27, 40). Thus, labeled surrogates (i.e., FLB) should be prepared from unmodified natural bacterial assemblages (20, 34) in order to estimate the fraction of actively grazing protists in natural samples. In this study, only natural assemblages of FLB were used. Estimates of active grazers vary depending on the available bacterial sizes (Table 1), suggesting that some flagellates might be exclusively grazing on specific bacterial size classes.

Equation 1 can be used to estimate the percentage of protists grazing on specific preys. It can be used to assess, for example, the fraction of herbivores (i.e., by using fluorescently labeled algae) (40), the percentage of mixotrophs (i.e., active grazers containing photosynthetic pigments) (31), or the percentage of protists grazing on specific types or sizes of both prokaryotic or eukaryotic prey.

The fraction of active grazers is time dependent; it increases over the incubation time, reaching a saturation point. These kinetics were well represented by Monod-like equations (Fig. 2). From a practical point of view, the interesting parameter is the fraction of active grazers during a specific time period. This period should correspond to the duration of the FLB uptake experiments. Short-term uptake experiments with bacterial surrogates require that bacterivory rates are measured over the linear portion of the uptake curve (36). These experiments are from a few minutes to up to a few hours long depending on the samples and environmental conditions (34, 36). The present study focused on the percentage of protists actively grazing on bacteria during FLB uptake experiments. For this practical approach, in situ bacterivory rates should correspond to in situ fractions of active grazers over the same time period.

If the number of bacteria per protist does, in fact, follow a Poisson distribution, then the number of ingested bacteria, and thus the bacterivory rates, could be computed from the fraction of protists with ingested bacteria. As seen above, by using equation 3 one can successfully estimate the rates of bacterivory during short-term FLB uptake experiments (Fig. 3). Thus, rates of bacterivory can be obtained by simply counting the number of protists containing FLB ingested (or by counting the number of protists with no FLB ingested and subtracting this value from the total number of protists). By doing so one would save considerable time in microscopy.

Validation of equation 3 estimates of bacterivory rates by comparison with standard estimates showed a perfect agreement between both procedures (Fig. 3). Therefore, the proposed equations can be applied to field estimates of bacterivory rates. Due to the low bacterial abundances found in oligotrophic systems (i.e., most oceanic samples), short-term FLB uptake experiments often lack sensitivity; the protocol proposed in this study appears to be especially useful under these circumstances since it provides increased sensitivity compared to standard calculations of bacterivory rates (following, for instance, the method of Sherr et al. [36]). This protocol (equation 3) could also be used in similar uptake experiments involving any type of labeled prey surrogates; the assumptions of this protocol should be previously verified. This procedure also allows partial automatization; for instance, it could be extremely useful in flow cytometry, allowing fast and sensitive screenings of natural samples (7). In addition, the proposed protocol would favor lowering the concentration of labeled surrogates added to the natural samples as a result of increased sensitivity. It would also save time and work.

In this study, estimates of the percentages of active bacterivores in natural aquatic systems have been shown. Results were validated by comparing estimates with experimental data. The number of total bacteria and FLB ingested per protist are assumed to follow a Poisson distribution, and no discrimination for or against labeled surrogates was detected. Some nanoflagellates appear to be exclusively grazing on specific bacterial size classes. A novel protocol for estimating bacterivory rates from short-term FLB uptake experiments is proposed; it saves time, since counting every ingested FLB per protist is no longer necessary. Also, it is more sensitive and offers the possibility of automatization by flow cytometry. This novel procedure can be applied to uptake experiments with any type or size of prey (examples include bacteria, cyanobacteria, phytoplankton, and nanoflagellates) in order to estimate fractions of active grazers and ingestion rates on specific preys.