Understanding base excess (BE): merits and pitfalls

Base excess (BE) was introduced by Siggaard-Andersen in 1960 as an answer to the forty-year-long quest for a reliable, stand-alone marker of metabolic acidosis/alkalosis, independent from co-existing respiratory derangements, and able to quantify the severity of the disorder [1]. Previously, several parameters had been examined. The first was actual bicarbonate (HCO₃⁻) [2], which was quickly discarded due to its known dependency on the partial pressure of CO₂ (PCO₂). To eliminate the respiratory component, standard bicarbonate (HCO₃⁻(st)) was introduced, representing the plasma bicarbonate concentration after equilibration at a PCO₂ of 40 mmHg [3]. Although this was certainly a step forward, HCO₃⁻(st) does not take into account the buffer effect of weak non-carbonic acids, i.e. proteins, which normally contribute to buffering with 14–16 negative charges (A⁻) per liter. Indeed, when a strong acid is added to blood, both HCO₃⁻ and A⁻ concentrations will be reduced. In an open system, which is the case of a subject properly regulating PCO₂ through breathing, carbonic buffers have a predominant role (about 75–80%), however, non-carbonic buffers cannot be disregarded completely. Therefore, the difference between HCO₃⁻(st) and the ideal, “normal” bicarbonate value, slightly underestimates the acid/base added to the system (e.g. the addition of 10 mmol/L of a strong acid to blood with HCO₃⁻ of 24 mmol/L could result in an HCO₃⁻(st) of 16 mmol/L, instead of 24–10 = 14 mmol/L). To overcome this problem, Singer and Hastings introduced the buffer base (BB), the sum of all buffer anions [4].

$$\text{BB}={\text{HCO}}_3^{-}+{\text{A}}^{-}$$

The BB considers the non-carbonic buffers and is theoretically CO₂-independent. Unfortunately, a physiologic inter-subject variability due to different non-carbonic buffer concentrations was noticed [5]. To overcome this limitation, Siggaard-Andersen introduced the base excess (BE), i.e. the “excess” (either positive or negative) of the actual BB as compared to the Normal BB (NBB).

$$\text{BE}=\mathrm{\Delta }\text{BB}=\text{BB}-\text{NBB}$$

The NBB is the BB experimentally obtained through equilibration and titration procedures aimed to achieve normal conditions of pH of 7.40 and PCO₂ of 40 mmHg. Consequently, BE is the amount of acid/base (mmol/L) that must be added to the blood sample to reach pH of 7.40 in standardized conditions (PCO₂ 40 mmHg, 37 °C) [6].

As direct titration is obviously impossible in a clinical scenario, nomograms and formulae were developed to estimate BE. The most common equation, which is still in use, is the following:

$$\text{BE}=\left({\text{HCO}}_3^{-}-24.8\right)+\beta ·\left(\text{pH}-7.40\right)$$

where 24.8 and 7.40 are the reference, ideal HCO₃⁻ (mmol/L) and pH values, and β is the buffer power (mmol/L) of non-carbonic weak acids [7, 8], which can either be a constant value (16.2 mmol/L) or computed as a function of hemoglobin concentration (assuming a constant protein concentration of 70 g/L) [9, 10]. The β value, multiplied by the variation in pH, provides an estimate of the change in weak negative charges due to non-carbonic buffers. Of note, from a clinical point of view, the variation in β value has a minor impact on the BE computation.

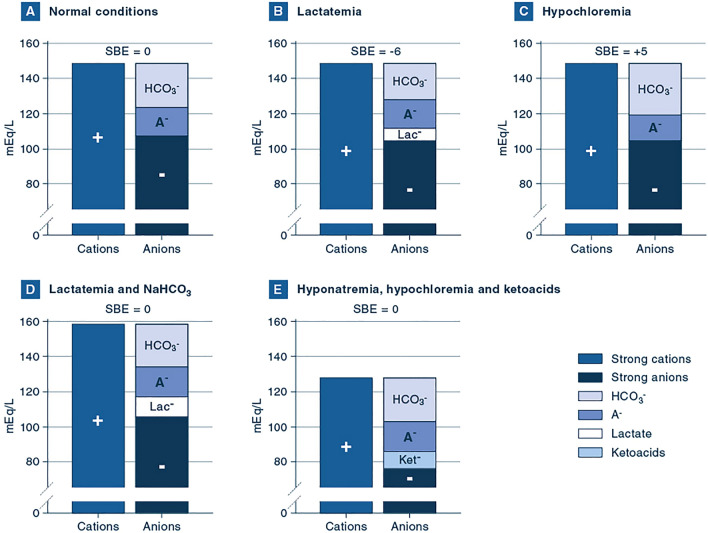
Generally, BE range between − 2 and + 2 mmol/L is considered normal. Clinical examples of abnormal BE values are reported in Fig. 1.

Fig. 1.

Gamblegrams of different acid–base conditions. A Normal conditions. This Gamblegram, i.e. the graph summarizing the electrical charges and the concept of electrical neutrality, represents a normal situation, with standard concentrations of electrolytes and normal acid–base parameters (HCO₃⁻ 24 mmol/L, PCO₂ 40 mmHg and pH of 7.40), leading to a SBE of 0 mmol/L. B Lactatemia. In this situation, lactate concentration is increased by 6 mmol/L and pH is 7.31. Other electrolytes and PCO₂ have normal values. HCO₃⁻ concentration is decreased to 20 mmol/L and BE is − 6 mmol/L. This is an example of isolated metabolic acidosis. C Hypochloremia. In this situation, chloride concentration is decreased by 5 mmol/L and pH is 7.47. Other electrolytes and PCO₂ have normal values. HCO₃⁻ concentration is increased to 29 mmol/L and BE is + 5 mmol/L. This is an example of isolated metabolic alkalosis, which can frequently be observed in case of persistent vomiting or after administration of loop diuretics. D Lactatemia and sodium bicarbonate (NaHCO₃). In this situation, lactate concentration is increased by 10 mmol/L and sodium concentration is increased by 10 mmol/L due to the administration of NaHCO₃. Other electrolytes and PCO₂ have normal values. As the increase in cations is equal to the increase in anions, no other change in electrolytes is required to fulfill the electroneutrality principle, i.e. HCO₃⁻ concentration is unaffected (24 mmol/L) and pH is 7.40. Therefore, two acid–base disorders co-exist and cancel each other out. Consequently, the calculated SBE is 0 mmol/L. Of note, the columns are higher than normal, underlining the fact that sodium-bicarbonate administration causes an increase in osmolarity. E Hyponatremia, hypochloremia and ketoacids. In this situation, sodium and chloride concentrations are reduced by 20 and 30 mmol/L, respectively. At the same time, 10 mmol/L of ketoacids are present. Also in this case, variations in strong cations and anions cancel out each other and, assuming a normal PCO₂ of 40 mmHg, normal values of pH and HCO₃⁻ are expected, leading to a normal SBE of 0 mmol/L. This acid–base derangement can be observed in infants with hypertrophic pyloric stenosis, where electrolyte losses due to persistent vomiting can coexist with hypovolemia and starvation ketoacidosis. HCO₃⁻, bicarbonate; A⁻, dissociated non-carbonic weak acids; Lac⁻, lactate; Ket⁻, ketoacids

Base excess can either be expressed for whole blood—BE(B), which does not consider the interaction of blood with the interstitial fluid, or for the entire extracellular fluid—BE(ecf), also called standard BE (SBE). The SBE calculation uses the same equation but considers a lower concentration of hemoglobin (either 1/3 of the actually measured hemoglobin or a constant concentration) for the estimation of β. Despite this conceptual difference, even in the presence of extreme anemia or polycythemia, it is hard to imagine conditions in which BE(B) and BE(ecf) would lead to markedly different results, diagnoses and therapeutic interventions. Nevertheless, SBE is generally considered a better and more reliable parameter and should therefore be preferred in clinical practice [11, 12].

Not all that glitters is gold

We have highlighted the usefulness and strengths of SBE, but some limitations exist and should be kept in mind in our daily practice. First, while SBE is a valuable quantitative estimate of metabolic acid–base derangements, it does not provide any information about the underlying mechanisms. Indeed, to identify the underlying pathologic mechanism we have to evaluate other variables, such as electrolytes, lactate, proteins, phosphates and clinical history. Second, SBE is a composite marker, i.e. several components contribute to its final value (e.g. chloride, lactate, albumin, ketoacids) [13, 14]. Therefore, different components could operate in opposite directions, potentially cancelling out each other and making the interpretation of the isolated SBE value very difficult. Let us provide an example. A patient could have hyperchloremia, therefore an increase in strong anions, together with hypoalbuminemia, leading to a reduction in weak negative charges. If the reduction in negative charges due to hypoalbuminemia is equal to the increase in chloride, HCO₃⁻ concentration will be normal. The patient could therefore have normal acid–base parameters (pH 7.40, pCO₂ 40 mmHg, HCO₃⁻ 24 mmol/L) yielding a perfectly normal SBE of 0 mmol/L. Is the value of SBE in this context wrong? No, it is not. The SBE correctly states that we do not need to add strong acids/bases to the sample to reach a pH of 7.40 with a PCO₂ of 40 mmHg. We are already there! However, two pathologic processes (with opposite sign) are present and would be missed if SBE was assessed on its own. Gamblegrams [15] of other examples of multiple acid–base derangements leading to normal SBE values are summarized in Fig. 1.

SBE is a useful parameter to assist in the diagnosis of metabolic acid–base disorders and to assess the metabolic displacement quantitatively. It is a calculated value, that relies on several measured variables (pH, PCO₂, hemoglobin concentration) and assumes normal plasma proteins. Importantly, SBE by itself does not provide information about the underlying condition and could be perfectly normal in the case of multiple conditions acting in opposite directions. Therefore, while an abnormal SBE is a reliable marker of an active metabolic issue, a normal SBE is not enough to exclude it. For this reason, SBE cannot be considered a stand-alone parameter. Indeed, it is fundamental to integrate its values with other information to identify complex acid–base disorders.

Funding

Open Access funding enabled and organized by Projekt DEAL.

Declarations

Conflicts of interest

The authors certify that they have no affiliations with or involvement in any organization or entity with any financial or non-financial interest in the subject matter discussed in this manuscript.