Abstract
The paper aims to use Modern Dimensional Analysis (MDA) to study the polymers additive manufacturing optimization. The original part of the work is represented by the application of this nonconventional method in the field of polymers additive manufacturing. The laws of the model provide the complete sets of dimensionless variables, which cannot be offered by any of the classical methods (such as Geometric Analogy, Theory of Similarity, and Classical Dimensional Analysis). The validation of the method was performed experimentally. The original part of the work is represented by the application of this nonconventional method in the field of polymers additive manufacturing optimization. An application is presented and the necessary steps are analyzed one by one.
Keywords: polymers, additive manufacturing, 3D printing, dimensional analysis
1. Introduction
Dimensional analysis is a method used since the beginning of the development of sciences to analyze the relationships that exist between different physical quantities. With the help of this method, the correctness of some relationships proposed by different researchers was checked, from a logical point of view, but it also allowed obtaining some dependency relationships between different physical quantities. Historically, it is difficult to determine the precise moment of the launch of this theory, but the concept of physical dimension, which is the basis of dimensional analysis, was introduced by Fourier in 1822 [1]. In the beginning, the method was mainly used for verifications and research on the plausibility of some hypotheses introduced by different researchers in their works. However, with the development of the technique, the field of applicability of this method has diversified a lot, and the engineering applications have caused the fields in which it proved its usefulness to be very varied [2]. The mathematical and logical bases of dimensional analysis are presented in many works. I mentioned some of them [3,4,5,6]. Dimensional Analysis can be used to formulate pertinent hypotheses about physical phenomena that can then be verified experimentally or using more advanced models [7,8,9]. At the same time, the specificity of the various possible applications forced the researchers to develop the method and improve it, to be able to obtain relevant results, in a short time and with minimal costs [3,10,11,12,13,14,15]. All these results led to the formulation of the Modern Dimensional Analysis, which better covers all cases that may appear in engineering practice [16,17,18].
The study of complex phenomena on models (usually reduced to scale) instead of prototypes has become a widespread practice in the field of engineering due to the simplicity, lower cost price and to the repeatability of experimental investigations under rigorously controllable conditions and with less numerous qualified personnel. The first approaches to the model–prototype correlation were aimed at the implementation of the Geometric Analogy, where through the proportionality of the dimensions and the equality of the angles, homologous points, homologous lines, homologous surfaces, homologous volumes of the model and the prototype could be defined, respectively [19]. Later, by means of the Theory of Similarity, the sphere of comparison also extended to some problems of functional similarity, establishing similar processes, which take place both in the prototype and in the model, carried out in homologous points and in homologous times in the two structures.
Another means, more efficient, but not ideal, is the Classical Dimensional Analysis (CDA) [20,21], when dimensionless groups πj will be constituted, the number of which is obtained by applying Buckingham’s theorem. However, there are also a number of shortcomings, such as: the rather arbitrary and difficult way of establishing dimensionless groups; imposing deep knowledge of the basic physical phenomenon; the complete sets of these dimensionless groups πj can only be obtained in certain special cases. An effective solution is offered by the version developed by the author of the works [16,17], hereinafter referred to as Modern Dimensional Analysis (MDA). MDA provides an original, unitary, easy and efficient strategy in determining complete sets of dimensionless groups πj. The complete set of the Model Law (ML) helps us to establish (depending on the dimensions adopted a priori for the prototype) the dimensions of the model, respectively, for the sought dependent variable of the prototype: its predictable size according to the results of the measurements made on the model [22,23]. Another great advantage of the MDA lies in the fact that if a variable is insignificant (irrelevant), either from a physical or dimensional point of view, it can be eliminated, without the ML undergoing significant changes (of essence) regarding the characterization of the studied phenomenon. Another important aspect is that the set of relationships contained in the ML do not represent calculation relationships in the usual sense, but only firm correlations, established between the scale factors of the variables involved in the description of the analyzed phenomenon [24,25]. A number of additional applications are presented in references [26,27,28].
Dimensional analysis has been developed and applied in numerous fields, where it has proven its usefulness and quasi-universal applicability in Civil Engineering [29,30,31,32], Aeronautical Science [33,34], Automotive Engineering [35,36,37], Mechanical Engineering [38,39], Medicine [40,41,42] and other interesting applications.
The use of polymer composites as raw material in additive manufacturing (AM) allowed a robust production and the production of parts with better mechanical properties than by using unreinforced polymers. Following the studies carried out, it was found that in general, AM available commercially can benefit from the advantage offered by reinforced fibers through different techniques [43].
Fiber-reinforced polymers could also be used for 4D printers to control shape change after 3D printing. There are also problems created by the use of these materials such as sleeping of voids, poor adhesion between the fibers and the matrix, blocking of the printer due to the inclusion of filler, increased curing time, and modeling and simulation being made much more difficult. The works in the field try to solve these mentioned problems [44,45,46,47].
The advantages and disadvantages of the procedure are presented in [48]. Comparisons are made with other technologies such as injection molding or the use of cutting machines. There is very wide use of the method, and achievements in the field of electronics, aerospace engineering and biomedical engineering are highlighted [49,50,51,52,53]. Important benefits and limitations are identified to clarify and motivate future work in this area [54,55].
In parallel with the manufacturing, aspects of the design, the additives used and the processing parameters must be addressed in relation to increasing the construction speed and improving the precision, functionality, surface finish, stability, mechanical properties and porosity [56,57]. Applications are currently being developed in light engineering, architecture, food processing, optics, energy technology, dentistry, drug delivery and personalized medicine [58,59,60]. Recent research in the field can be found in [61,62].
The modern approach to the problem of real structures Is based on establishing as precise correlations as possible between their behavior and those of scaled structures. The initial structure is called the prototype, and the one made to scale (usually reduced scale) is the model. Based on the above-mentioned references, the following shortcomings can be mentioned among others:
The Geometric Analogy can only be applied with the strict observance of the existence of well-defined ratios between all dimensions (proportions) of the compared structures (prototype and model) or in the case of a very small number of variables;
Similitude Theory already operates with dimensionless variables, but their number is relatively small, and their identification method requires solid knowledge in the field;
CDA widely uses dimensionless variables, also called quantities , but with certain shortcomings, such as [63,64,65,66,67,68,69]: their establishment is non-unitary, sometimes even chaotic, and depends mainly on the ingenuity of the one who applies it; CDA requires solid/deep knowledge in the field, both for choosing the most eloquent analytical relationships in describing the analyzed phenomenon, and in grouping the terms from these relationships, in order to establish the desired dimensionless variables; only in special cases it allows highlighting the complete set of dimensionless variables and consequently the Model Law that will result from them; it is not an easy and accessible method for the average researcher, being especially a method intended for established theoreticians.
However, in a series of works, these principles of Geometric Analogy, Similitude Theory, and CDA, are applied to thermal phenomena [70,71,72] and to aspects of engineering [41,73,74], respectively. Many researches have presented the advantages of Modern Dimensional Analysis (MDA)applied to this type of problems [75,76,77,78,79,80,81,82,83,84].
Faced with these shortcomings, the improved method, hereinafter referred to as MDA, [16,17,85,86,87,88,89,90,91], comes to offer a solution, which practically eliminates all these shortcomings, becoming a unitary, safe and particularly accessible method for any researcher. The net advantages of MDA, successfully applied over the years and by the authors in the works [18,22,23,26,28,92,93], were presented in other work [16,17,22,26].
The application of MDA principles to new phenomena, such as the one analyzed below, proves once again that it represents a method with indisputable perspectives, therefore of the future, for a wide range of aspects of engineering.
In the desire to increase savings, cost price, material, labor, etc., the reduced-scale models, on which the expected qualities of the respective product can be tested in advance (i.e., the prototype), becomes a desire. Therefore, MDA, which provides important information on the full-scale part (prototype) through thorough experimental investigations on models (parts made to a certain scale, favorably chosen), complements and improves AM technology. Thus, for example, if it is desired to obtain a relatively voluminous piece of plastic material with certain qualities (stiffness, weight, bearing capacity, etc.), then it becomes useful to test these qualities on a model, which by means of firm correlations with the initial piece (which at MDA is the Model Law) will be able to ensure this desired response of the prototype.
The advantages of combining AM with MDA becomes even more eloquent in the case of parts made of two or three different materials, some of which have the role of ensuring wear resistance, others rigidity or resistance to chemical agents, weathering, etc.
This is why the authors initiated this somewhat unusual approach to AM through the lens of MDA. They express the hope that these results/investigations can and will be extended to obtain the maximum benefits of AM technology. AM has the advantage of allowing the manufacture of customized objects on demand, thus avoiding the storage of parts. There is currently a continuous transition from rapid prototyping to rapid manufacturing. This brings new challenges for engineers. From this brief analysis, one can highlight (even if only partially) the flexibility offered by MDA over CDA and the rest of the methods that use correlation between prototype and model.
2. Model Law for Polymers Beams
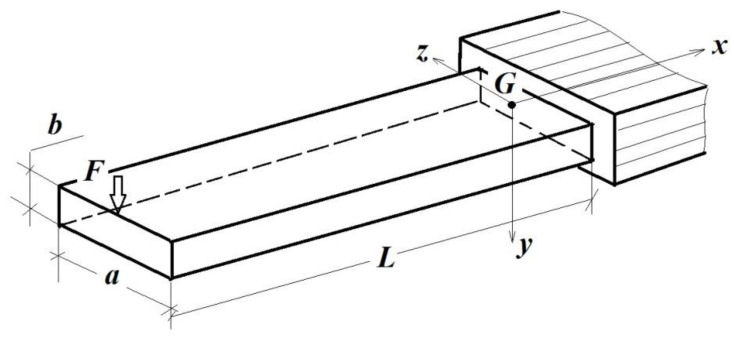
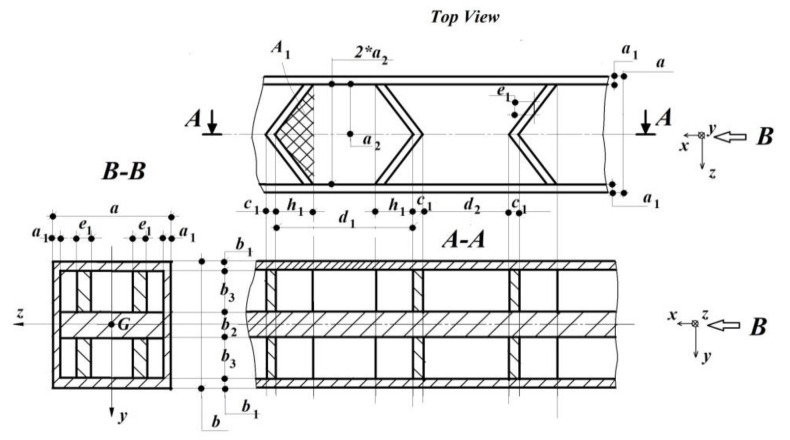
In order to develop and validate the ML in the case of these additive technologies through 3D printing, the authors started from the case of the beam (cantilever) subjected to simple bending. In Figure 1, the dimensions , the reference system and the applied force are given. In Figure 2, compared to the options offered by the software of 3D printers with the help of which the element is obtained by addition, another way of filling the nominal volume of the part is presented, namely, with the help of ribs, located on two levels, in a desired order and imposed by the desired mechanical response of that part.
Figure 1.
The testing beam.
Figure 2.
A possible version of filling the volume of the beam.
There are a number of detailed studies in which different researchers have investigated basic aspects of the influence of direction of filament deposition, type of filling degree, deposition speed, etc., on obtaining an optimal final product. This optimum refers not only to the achievement of certain mechanical properties of the final product, such as maximum rigidity, minimum weight, minimum degree of wear, resistance to the action of the environment, the weather or corrosive agents, but also to reduce manufacturing time, competitive shapes and design, etc. Modern machines (3D printers) are provided with particularly complex software, which allows the provision of some parameters, which will precisely ensure the achievement of the previously mentioned desires, not only by arranging the filament according to a certain geometry, but also by combining two or more types of filaments (either all plastic, or even some metallic). Thus, for example, it becomes possible to obtain molds with a metallic interior and the rest from plastic material, necessary for the manufacture of unique spare parts at a particularly advantageous cost price, without waste and with minimal time consumption.
In order to illustrate the flexibility of the MDA, regarding the cross-sections, which can be modelled, a relatively complex example was given, where in addition to reducing the volume of the final piece, it was also desired to preserve a certain degree of rigidity; these sections can be easily made with current 3D printers, but modelling them using other methods (Geometric Analogy, Similarity Theory or Classical Dimensional Analysis) is very unlikely; MDA in this regard provides the facilities required by a competitive AM industry.
The arrangement of these ribs, although at first sight chosen arbitrarily, leads to an optimal structure from the point of view of a beam (a bar subjected to bending). Obviously, depending on the main demands of the elements of the new product, achievable through AM technology, these ribs will have different layouts, which a good engineer, with adequate knowledge in the field of Material Strength and Elasticity Theory, will notice and impose in their configuration (of the disposition of the nerves). In this sense, even a preliminary numerical analysis can be of great use to the less initiated in order to highlight/identify the location of the isostatic lines (lines of maximum stress, along which the principal normal stresses are arranged), along which to arrange these ribs. As is well known, these principal normal stresses are either tensile or compressive, and the shape of the cross-section of the rib which is to take them will differ accordingly.
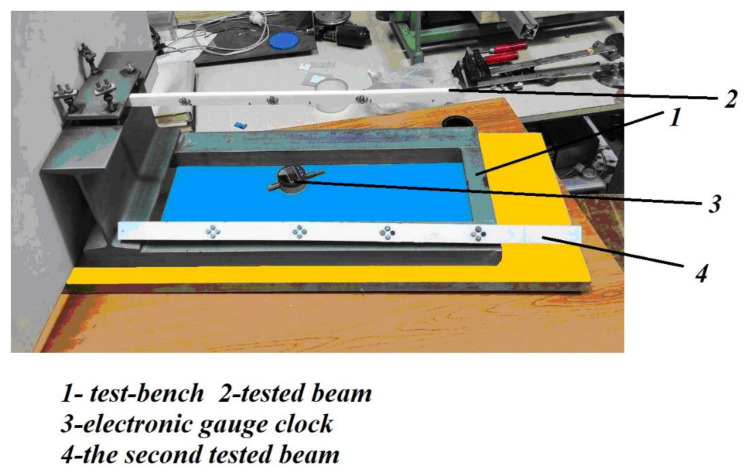
Figure 3 shows the two bars, considered as prototype and model. The plastic material used (PLA-Polylactic Acid), based on data from the literature, respectively, from the manufacturing company, had the modulus of elasticity of
and the scale factor results:
Figure 3.
The experimental setup.
From the sixth element of the Model Law, that is, from
the magnitude of force F2 will result, according to relation (18).
In order to obtain higher stiffnesses, the two beams were rotated by 90° from the position in Figure 1. Thus, even if the applied forces were relatively high, the displacements obtained were within acceptable limits. After applying the force F2 to the model, the vertical displacement at the free end of the bar v2 was measured.
From element no. 1 of the ML, i.e., from
resulted in the predicted displacement value v1 for the prototype. In order to verify the correctness of this quantity, a load with the predicted force F1 was carried out on the prototype, obtaining a value v1M, which differed only by from that predicted by the ML. Thus, the ML was found to be valid.
Based on those summarized in the works [16,17,18,22,23,26,28,92,93], the deduction of the Model Law involves the following basic steps:
- The choice of variables, which can influence to a certain extent the analyzed phenomenon; here is the vertical displacement of the beam at the level of the applied force , namely:
- The dimensions of the beam , but in the general case the area defined by the ribs and, respectively:
(1) - The applied force ;
- Longitudinal modulus of elasticity (Young) ;
-
The useful volume (which also defines the degree of filling) of the piece .In this sense, combinations of variables are also allowed, such as:
- The axial moment of inertia , if it is desired to replace the dimensions and thereby attach a more flexible model (not necessarily a rectangular section!) to the studied prototype;
- The stiffness module , if the original/traditional material used in the prototype is abandoned and only the size of their product will matter, without imposing distinct restrictions on the material and the axial moment of inertia.
The creation of the matrix A (see Table 1), formed by the exponents of the dimensions of the variables considered to be independent, i.e., those variables, the size of which is chosen a priori independently, both in the prototype and in the model; this matrix must be invertible, i.e., ; with the help of this set of variables, particularly flexible models can be obtained, which will lead to as simple, cheap and repeatable experimental investigations as possible;
The creation of the matrix B, formed by the exponents of the dimensions of the variables considered to be dependent, i.e., those variables whose size is chosen a priori independently only for the prototype, while for the model, they will necessarily result only by applying an element of the ML, which is to be deduced; among these dependent variables is the vertical displacement sought at the prototype , which will result exclusively by applying an element of the ML that will be deduced, depending on the displacement actually measured on the model;
To this set of matrices B-A are attached the matrices respectively; , which, together with the matrices A and B, will constitute the Dimensional Set (DS) in the form
Table 1.
Dimensional Set.
| B | A |
| D | C |
It is mentioned above that we have the inverse of the matrix A, that is , the transpose of the product and the number of lines from A and B being equal to the number of basic dimensions involved, while for C and D, we have the number (n) of the dimensionless variables , which helps to fully define the ML, as will be illustrated next. The matrix D represents the appropriate unit matrix .
-
5.A Scale factor
is defined for each variable (dependent, independent, respectively), where the index “1” refers to the prototype, and “2” to the model;(2) -
6.Based on a unique protocol [18,22,23,26,28,92,93],:
- All elements are extracted, i.e., dimensionless variables , which will actually be products of independent variables at certain powers (results from Dimensional Set) and a dependent variable at the first power;
- Each dimensionless variable obtained in this way is equal to unity;
- The initial variables are replaced with their Scale Factors;
- Each Scale Factor of the dependent variable from the respective equality will be expressed by ;
- By applying that relationship , the desired size will finally result, which is further illustrated in the first relationships related to the different approaches.
Next, some of these ML, deduced according to other dependent variables, will be presented.
The first approach assumes that the independent variables (which can be freely chosen a priori for both the prototype and the model) are the longitudinal modulus of elasticity and the axial moment of inertia , and the dependent variables are the rest of the variables. Within this approach, the authors also wanted to highlight the fact that the parameter can be divided into others, such as: ; ; , without affecting the essence of the ML.
At the same time, in order to correctly merge the sizes in , they (that is, and ) can no longer appear in other elements, as they are here .
The Dimensional Set had the following elements (Table 2):
Table 2.
First approach. The explicit Dimensional Set.
| Dimensions | B | A | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| v | F | L | a* | b* | c* | A1 | Vutil | E | Iz | |
| m | 1 | 0 | 1 | 1 | 1 | 1 | 2 | 3 | −2 | 4 |
| N | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 |
| π1 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | −0.25 |
| π2 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | −1 | −0.5 |
| π3 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | −0.25 |
| π4 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | −0.25 |
| π5 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | −0.25 |
| π6 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | −0.25 |
| π7 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | −0.5 |
| π8 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | −0.75 |
By applying the unique protocol, the following resulted in turn:
- From the first line, i.e., of , the exponents of the variables led to the following product, which was equal to unity, and subsequently led to the variables being substituted with their Scale Factors, finally resulting in the first ML:
(3) - From this law, based on the experimental measurements made on the model, the size is known, and consequently, from will finally result in the size of , that is:
(4) - The rest of the elements of the ML will be interpreted in a similar way, such as for example the one necessary to establish the size of the force applied to the model, knowing a certain amount of force, which would require the prototype:
so:(5) (6)
A second approach involved a merging of the modulus of elasticity and the axial moment of inertia into the new independent variable of the stiffness modulus , thus allowing both a more favorable choice of material type in combination with an equally preferable cross-section and the release of an independent variable, allowing the introduction of the length of the beams in this free place (thus the free choice of the two lengths in the prototype and in the model).
The Dimensional Set had the following elements (Table 3):
Table 3.
Second approach. Dimensional Set.
| Dimensions | B | A | |||||||
|---|---|---|---|---|---|---|---|---|---|
| v | a* | b* | c* | A1 | F | Vutil | L | E × Iz | |
| m | 1 | 1 | 1 | 1 | 2 | 0 | 3 | 1 | 2 |
| N | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 1 |
| π1 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | −1 | 0 |
| π2 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | −1 | 0 |
| π3 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | −1 | 0 |
| π4 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | −1 | 0 |
| π5 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | −2 | 0 |
| π6 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 3 | −1 |
| π7 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | −3 | 0 |
Following the application of the above protocol, the seven elements of the Model Law resulted:
| (7) |
| (8) |
| (9) |
| (10) |
| (11) |
| (12) |
| (13) |
Of these, for the concrete application, those related to and , were of particular interest.
A third approach assumed the introduction as an independent variable of the useful volume (therefore a parameter of the degree of filling) in addition to the stiffness modulus . Consequently, both the force applied to the model and its length resulted as dependent variables, thus obtained based on the ML. The DS had the following elements (Table 4):
Table 4.
Third approach. New Dimensional Set.
| Dimensions | B | A | |||||||
|---|---|---|---|---|---|---|---|---|---|
| v | a* | b* | c* | A1 | F | L | Vutil | E × Iz | |
| m | 1 | 1 | 1 | 1 | 2 | 0 | 1 | 3 | 2 |
| N | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | 1 |
| π1 | 1 | 0 | 0 | 0 | 0 | 0 | 0 | −0.33333 | 0 |
| π2 | 0 | 1 | 0 | 0 | 0 | 0 | 0 | −0.33333 | 0 |
| π3 | 0 | 0 | 1 | 0 | 0 | 0 | 0 | −0.33333 | 0 |
| π4 | 0 | 0 | 0 | 1 | 0 | 0 | 0 | −0.33333 | 0 |
| π5 | 0 | 0 | 0 | 0 | 1 | 0 | 0 | −0.66667 | 0 |
| π6 | 0 | 0 | 0 | 0 | 0 | 1 | 0 | 0.666667 | −1 |
| π7 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | −0.33333 | 0 |
In this case, only the elements related to the vertical displacement of the free end of the prototype , the force that must be applied to the model , and the required length of the model are presented, that is:
| (14) |
| (15) |
| (16) |
The following paragraph presents the way to obtain the desired values, including displacement of the free end of the prototype .
3. Experimental Validation of the ML
To present the experimental validation of the Model Law, a prototype and a model in the form of cantilevers (beams embedded at one end and loaded at the free end with a vertically concentrated force) of solid rectangular sections were chosen, as in Figure 1, but placed on the small sides of the rectangle (in order to obtain greater stiffness).
The material was the usual plastic PLA (Polylactic Acid), the machine (the 3D printer) was of the Creality Ender-3 type, and the software used was Cura [94].
It was proposed to determine, with the help of the ML from case b), the displacement of the free end of the length cross-section prototype under the action of the force , if the attached model was of length and cross-section .
The axial moments of inertia are determined
| (17) |
We have , and the scale factor results:
and therefore, .
Based on the lengths (chosen a priori independently), it results that .
Thus, from the sixth element of the ML, i.e.,
they will result in turn the magnitude of force F2:
| (18) |
Carrying out the measurements on the model with the force , the displacement resulted and from the first element of the ML
we have obtained the predictable displacement of the prototype :
| (19) |
After carrying out actual measurements on the prototype, was obtained, so an error of , with respect to the predictable value , which is acceptable from an engineering point of view.
Consequently, the ML provided by version (b) is valid for the analyzed case.
4. Discussions
It could be noted that MDA presents great flexibility, and thus, the model can be made in optimal conditions (manufacturing, testing, number and qualification of personnel, etc.);
Addition represents a very promising current trend, and the implementation of MDA in the design of models, which will facilitate the creation of the final product, i.e., prototypes, represents an area that deserves to be deepened.
Elements of the ML are not proper physical relations in the usual sense; they represent only correlations between the scale factors of the variables involved in describing the behavior of the prototype in relation to that of the model;
Depending on the purpose of new models, it will be possible to apply the other two laws of the model deduced and analyzed in Section 2;
If an emphasis will be placed on the involvement of different materials for the model and prototype, or/and on the use of different cross-sections related to them, then the different degrees of filling can be explored as an optimization parameter (through the variables , as well as );
If different degrees of filling will be imposed (with the help of ), or/and stiffness modules chosen a priori, then by means of the dependent variables and , respectively, new solutions can be found, with new forms of stiffening of the cross section, respectively, the length of the model involved in experimental investigations.
5. Conclusions
The advantages of MDA can be summarized as follows:
It does not require deep knowledge in the field, only the review of all variables, which can to a certain extent influence the studied phenomenon;
Insignificant variables (either from a physical point of view, or from the point of view of the weight in the unfolding of the respective physical phenomenon) are automatically eliminated by the protocol developed by Szirtes [16,17];
The methodology is unitary, easy and allows obtaining all the dimensionless variables related to the phenomenon, so it provides the complete version of the Model Law (ML), which can be achieved through the previously mentioned methods only in completely and completely particular cases;
MDA is a flexible method, as was illustrated in Section 3, allowing us, from the approach of the general case, to obtain a series of particular versions without any difficulty;
The MDA protocol allows the grouping of variables in an optimal manner, i.e., to highlight those variables, which depend on the testing of the model under optimal conditions and thus obtain the most reliable and repeatable information that will allow the description of the behavior of the analyzed prototype.
Among the future objectives of the authors is the expansion of these theoretical and experimental investigations, which would make available to specialists new laws of the model, developed with the help of MDA, which would be able to take into account other important aspects of the additive manufacturing of complex parts, having internal structures different from the current ones.
The ML deduced in Section 2 and validated in Section 3 serves as an illustration of how MDA can provide solutions for testing models at a low cost price and under rigorously reproducible conditions in order to complete the intended real product, i.e., the prototype, an example serving to validate the proposed method in polymers additive manufacturing optimization.
As seen, the presented MDA methodology is unitary, simple and safe and accessible to any researcher and can represent an effective way to optimize the finished products made with AM technology.
Taking into account the importance of AM in practice, numerous works have addressed different aspects identified in the study of this procedure. AM allows the use in different industrial branches, allowing the manufacturing of materials.
The importance of the work lies in the fact that it demonstrates the possibility of applicability in AM a field that is at the beginning of its development and which, alone, will come with numerous problems that can be solved with the method proposed and validated in the work.
Nomenclature
| A | Area (m2); |
| F | Force (N); |
| g | Gravitational acceleration (m/s2); |
| a, b, a*, b*, c*, l, L | Length (m); |
| V | volume (m3); |
| w | velocity (m/s); |
| v | vertical displacement (m) |
|
|
scale factor corresponding to the sizes indicated in the index. |
| Greek symbols | |
| Density (kg/m3); | |
|
|
Nabla operator; Dimensionless variables; Specific gravity (N/m3). |
| Subscripts | |
| x, y, z | Directions. |
Author Contributions
Conceptualization, Z.A. and I.S.; methodology, Z.A. and I.S.; software, Z.A.; validation, Z.A., I.S., S.V. and R.-I.S.; formal analysis, Z.A., I.S., S.V. and R.-I.S.; investigation, Z.A., I.S. and R.-I.S.; resources, I.S.; data curation, Z.A., I.S. and R.-I.S.; writing—original draft preparation, I.S.; writing—review and editing, I.S. and S.V.; visualization, Z.A., I.S., S.V. and R.-I.S.; supervision, Z.A., I.S., S.V. and R.-I.S.; project administration, I.S.; funding acquisition, Z.A., I.S., S.V. and R.-I.S. All authors have read and agreed to the published version of the manuscript.
Institutional Review Board Statement
Not applicable.
Informed Consent Statement
Not applicable.
Data Availability Statement
Not applicable.
Conflicts of Interest
The authors declare no conflict of interest.
Funding Statement
The APC was funded by Transilvania University of Brasov in 2022.
Footnotes
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.
References
- 1.Fourier J. Theorie Analytique de la Chaleur. Firmin Didot; Paris, France: 1822. [Google Scholar]
- 2.Bridgeman P.W. Dimensional Analysis. Yale University Press; New Haven, CT, USA: 1922. [Google Scholar]
- 3.Coyle R.G., Ballicolay B. Concepts and Software for Dimensional Analysis in Modeling. IEEE Trans. Syst. Man Cybern. 1984;14:478–487. doi: 10.1109/TSMC.1984.6313242. [DOI] [Google Scholar]
- 4.Remillard W.J. Applying Dimensional Analysis. Am. J. Phys. 1983;51:137–140. doi: 10.1119/1.13468. [DOI] [Google Scholar]
- 5.Martins R.D.A. The Origin of Dimensional Analysis. J. Frankl. Inst. 1981;311:331–337. doi: 10.1016/0016-0032(81)90475-0. [DOI] [Google Scholar]
- 6.Gibbings J.C. A Logic of Dimensional Analysis. J. Physiscs A-Math. Gen. 1982;15:1991–2002. doi: 10.1088/0305-4470/15/7/011. [DOI] [Google Scholar]
- 7.Szekeres P. Mathematical Foundations of Dimensional Analysis and the Question of Fundamental Units. Int. J. Theor. Phys. 1978;17:957–974. doi: 10.1007/BF00678423. [DOI] [Google Scholar]
- 8.Carlson D.E. Some New Results in Dimensional Analysis. Arch. Ration. Mech. Anal. 1978;68:191–210. doi: 10.1007/BF00247739. [DOI] [Google Scholar]
- 9.Gibbings J.C. Dimensional Analysis. J. Phys. A-Math. Gen. 1980;13:75–89. doi: 10.1088/0305-4470/13/1/010. [DOI] [Google Scholar]
- 10.Schnittger J.R. Dimensional Analysis in Design. J. Vib. Acoust. Stress Reliab. 1988;110:401–407. doi: 10.1115/1.3269533. [DOI] [Google Scholar]
- 11.Carinena J.F., Santander M. Dimensional Analysis. Adv. Electron. Electron Phys. 1988;72:181–258. doi: 10.1016/S0065-2539(08)60559-4. [DOI] [Google Scholar]
- 12.Canagaratna S.G. Is dimensional analysis the best we have to offer? J. Chem. Educ. 1993;70:40–43. doi: 10.1021/ed070p40. [DOI] [Google Scholar]
- 13.Bhaskar R., Nigam A. Qualitative Physics using Dimensional Analysis. Artif. Intell. 1990;45:73–111. doi: 10.1016/0004-3702(90)90038-2. [DOI] [Google Scholar]
- 14.Romberg G. Contribution to Dimensional Analysis. Ing. Arch. 1985;55:401–412. doi: 10.1007/BF00537647. [DOI] [Google Scholar]
- 15.Barr D.I.H. Consolidation of Basics of Dimensional Analysis. J. Eng. Mech.-ASCE. 1984;110:1357–1376. doi: 10.1061/(ASCE)0733-9399(1984)110:9(1357). [DOI] [Google Scholar]
- 16.Szirtes T. The Fine Art of Modelling, SPAR. J. Eng. Technol. 1992;1:37. [Google Scholar]
- 17.Szirtes T. Applied Dimensional Analysis and Modelling. McGraw-Hill; Toronto, ON, Canada: 1998. [Google Scholar]
- 18.Trif I., Asztalos Z., Kiss I., Élesztős P., Száva I., Popa G. Implementation of the Modern Dimensional Analysis in Engineering Problems; Basic Theoretical Layouts. Ann. Fac. Eng. Hunedoara Tome. 2019;17:73–76. [Google Scholar]
- 19.Westine P.S., Dodge F., Baker W. Similarity Methods in Engineering Dynamics. Elsevier; Amsterdam, The Netherlands: 1991. [Google Scholar]
- 20.Chen W.K. Algebraic Theory of Dimensional Analysis. J. Frankl. Inst. 1971;292:403–422. doi: 10.1016/0016-0032(71)90161-X. [DOI] [Google Scholar]
- 21.Buckingham E. On Physically Similar Systems. Phys. Rev. 1914;4:345. doi: 10.1103/PhysRev.4.345. [DOI] [Google Scholar]
- 22.Gálfi B.-P., Száva I., Șova D., Vlase S. Thermal Scaling of Transient Heat Transfer in a Round Cladded Rod with Modern Dimensional Analysis. Mathematics. 2021;9:1875. doi: 10.3390/math9161875. [DOI] [Google Scholar]
- 23.Száva I., Szirtes T., Dani P. An Application of Dimensional Model Theory in The Determination of the Deformation of a Structure. Eng. Mech. 2006;13:31–39. [Google Scholar]
- 24.Langhaar H.L. Dimensional Analysis and Theory of Models. John Wiley & Sons Ltd.; Hoboken, NJ, USA: 1951. [Google Scholar]
- 25.Pankhurst R.C. Dimensional Analysis and Scale Factor. Chapman & Hall Ltd.; London, UK: 1964. [Google Scholar]
- 26.Șova D., Száva I.R., Jármai K., Száva I., Vlase S. Heat transfer analysis in a beam with rectangular-hole using Modern Dimensional Analysis. Mathematics. 2022;10:409. [Google Scholar]
- 27.Han X., Sun X., Li G., Huang S., Zhu P., Shi C., Zhang T. A Repair Method for Damage in Aluminum Alloy Structures with the Cold Spray Process. Materials. 2021;14:6957. doi: 10.3390/ma14226957. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 28.Munteanu (Száva) I.R. Ph.D. Thesis. Transilvania University of Brasov; Brașov, Romania: 2018. Investigation Concerning Temperature Field Propagation along Reduced Scale Modelled Metal Structures. [Google Scholar]
- 29.Nikou N.S.R., Ziaei A.N., Dalir M. Study of Effective Parameters on Performance of Vortex Settling Basins Using Taguchi Method. J. Irrig. Drain. Eng. 2022;148:04021070. doi: 10.1061/(ASCE)IR.1943-4774.0001648. [DOI] [Google Scholar]
- 30.Ravazdezh F., Seok S., Haikal G., Ramirez J.A. Effect of Nonstructural Elements on Lateral Load Distribution and Rating of Slab and T-Beam Bridges. J. Bridge Eng. 2021;26:04021063. doi: 10.1061/(ASCE)BE.1943-5592.0001766. [DOI] [Google Scholar]
- 31.Mahmoudi-Rad M., Najafzadeh M. Air Entrainment Mechanism in the Vortex Structure: Experimental Study. J. Irrig. Drain. Eng. 2021;147:04021007. doi: 10.1061/(ASCE)IR.1943-4774.0001545. [DOI] [Google Scholar]
- 32.Wu Q.Y., Wang T., Ge H.B., Zhu H.P. Dimensional Analysis of Pounding Response of an Oscillator Based on Modified Kelvin Pounding Model. J. Aerosp. Eng. 2019;32:04019039. doi: 10.1061/(ASCE)AS.1943-5525.0001018. [DOI] [Google Scholar]
- 33.Maugin G.A. Continuum Mechanics through the Ages—From the Renaissance to the Twentieth Century: From Hydraulics to Plasticity. Volume 223. Springer; Berlin/Heidelberg, Germany: 2016. pp. 81–105. [DOI] [Google Scholar]
- 34.Flouros M., Cottier F., Hirschmann M., Kutz J., Jocher A. Ejector Scavenging of Bearing Chambers. A Numer. Exp. Investig. 2013;135:081602. doi: 10.1115/1.4024259. [DOI] [Google Scholar]
- 35.Milani S., Marzbani H., Azad N.L., Melek W., Jazar R.N. The importance of equation eta = mu n(2) in dimensional analysis and scaled vehicle experiments in vehicle dynamics. Veh. Syst. Dyn. 2022;60:2511–2540. doi: 10.1080/00423114.2021.1909736. [DOI] [Google Scholar]
- 36.Keeratiyadathanapat N., Sriveerakul T., Suvarnakuta N., Pianthong K. Experimental and Theoretical Investigation of a Hybrid Compressor and Ejector Refrigeration System for Automotive Air Conditioning Application. Eng. J.-Thail. 2017;21:105–123. doi: 10.4186/ej.2017.21.5.105. [DOI] [Google Scholar]
- 37.Bruckner S., Rudolph S. Data Mining and Knowledge Discovery: Theory, Tools, and Technology III. Volume 4384. SPIE; Bellingham, DC, USA: 2001. Knowledge discovery in scientific data using hierarchical modeling in dimensional analysis. Book Series; pp. 208–217. [Google Scholar]
- 38.Cheng H.Y., Fan K.K., Feng S., Seliverstov N.D., Makarova D.A. Optimization of mixing chamber parameters of pavement recycling machine under engineered particle model analysis. J. Chin. Inst. Eng. 2022;45:521–531. doi: 10.1080/02533839.2022.2078419. [DOI] [Google Scholar]
- 39.Carpinteri A., Accornero F. Dimensional Analysis of Critical Phenomena: Self-Weight Failure, Turbulence, Resonance, Fracture. Phys. Mesomech. 2021;24:459–463. doi: 10.1134/S102995992104010X. [DOI] [Google Scholar]
- 40.Zhang P., Ma Q.H., Nie Z.F., Li X.D. A Conflict Solving Process Based on Mapping between Physical Parameters and Engineering Parameters. Machines. 2022;10:323. doi: 10.3390/machines10050323. [DOI] [Google Scholar]
- 41.Ullah T., Afzal M., Sarwar H., Hanif A., Gilani S.A., Tariq A. Measuring the Effect of Dimensional Analysis on Nurses’ Level of Self-Efficacy in Medication Calculation Errors. Pak. J. Med. Health Sci. 2021;15:3579–3582. doi: 10.53350/pjmhs2115123579. [DOI] [Google Scholar]
- 42.Alhadhira A., Molloy M., Casasola M., Sarin R.R., Massey M., Voskanyan A., Ciottone G.R. Use of Dimensional Analysis in the X-, Y-, and Z-Axis to Predict Occurrence of Injury in Human Stampede. Disaster Med. Public Health Prep. 2020;14:248–255. doi: 10.1017/dmp.2019.47. [DOI] [PubMed] [Google Scholar]
- 43.Parandoush P., Lin D. A review on additive manufacturing of polymer-fiber composites. Compos. Struct. 2017;182:36–53. doi: 10.1016/j.compstruct.2017.08.088. [DOI] [Google Scholar]
- 44.Sing S.L., Yeong W.Y. Recent Progress in Research of Additive Manufacturing for Polymers. Polymers. 2022;14:2267. doi: 10.3390/polym14112267. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 45.Osswald T.A., Jack D., Thompson M.S. Polymer composites: Additive manufacturing of composites. Polym. Compos. 2022;43:3496–3497. doi: 10.1002/pc.26631. [DOI] [Google Scholar]
- 46.Sing S.L., Yeong W.Y. Process-Structure-Properties in Polymer Additive Manufacturing. Polymers. 2021;13:1098. doi: 10.3390/polym13071098. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 47.Aw J., Parikh N., Zhang X., Moore J., Geubelle P., Sottos N. Additive manufacturing of thermosetting polymers using frontal polymerization. Abstracts of Papers of the American Chemical Society; Proceedings of the 257th National Meeting of the American-Chemical-Society (ACS); Orlando, FL, USA. 31 March–4 April 2019; p. 91. [Google Scholar]
- 48.El Moumen A., Tarfaoui M., Lafdi K. Additive manufacturing of polymer composites: Processing and modeling Approaches. Composites. Part B-Eng. 2019;171:166–182. doi: 10.1016/j.compositesb.2019.04.029. [DOI] [Google Scholar]
- 49.Walia K., Khan A., Breedon P. Polymer-Based Additive Manufacturing: Process Optimisation for Low-Cost Industrial Robotics Manufacture. Polymers. 2021;13:2809. doi: 10.3390/polym13162809. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 50.Sinkora E. New Polymer Applications in Additive Manufacturing. Manuf. Eng. 2020;164:56–66. [Google Scholar]
- 51.Park S., Fu K. Polymer-based filament feedstock for additive manufacturing. Compos. Sci. Technol. 2021;213:108876. doi: 10.1016/j.compscitech.2021.108876. [DOI] [Google Scholar]
- 52.Hofer R., Hinrichs K. Additives for the Manufacture and Processing of Polymers. Polymers–Opportunities and Risks II: Sustenability, Product Design and Processing. Handb. Environ. Chem. Ser. 2010;12:97–145. doi: 10.1007/978-3-642-02797-0. [DOI] [Google Scholar]
- 53.Paesano A. Polymers for Additive Manufacturing: Present and Future along with their future properties and process requirements. Sample J. 2014;50:34–43. [Google Scholar]
- 54.Tan L.J.Y., Zhu W., Zhou K. Recent Progress on Polymer Materials for Additive Manufacturing. Adv. Funct. Mater. 2020;30:2003062. doi: 10.1002/adfm.202003062. [DOI] [Google Scholar]
- 55.Ligon S.C., Liska R., Stampfl J., Gurr M., Mulhaupt R. Polymers for 3D Printing and Customized Additive Manufacturing. Chem. Rev. 2017;117:10212–10290. doi: 10.1021/acs.chemrev.7b00074. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 56.Dickson A.N., Ross K.A., Dowling D.P. Additive manufacturing of woven carbon fibre polymer composites. Compos. Struct. 2018;206:637–643. doi: 10.1016/j.compstruct.2018.08.091. [DOI] [Google Scholar]
- 57.Aliheidari N., Ameli A. Composites and Nanocomposites: Thermoplastic Polymers for Additive Manufacturing. Encycl. Polym. Appl. VOLS I-III. 2019:486–500. doi: 10.1201/9781351019422-140000505. [DOI] [Google Scholar]
- 58.Wu H., Fahy W.P., Kim S., Kim H., Zhao N., Pilato L., Kafi A., Bateman S., Koo J.H. Recent developments in polymers/polymer nanocomposites for additive manufacturing. Prog. Mater. Sci. 2020;111:100638. doi: 10.1016/j.pmatsci.2020.100638. [DOI] [Google Scholar]
- 59.Zhang P., Mao Y.Q., Shu X. Polymer-Based Additive Manufacturing. Springer; Berlin/Heidelberg, Germany: 2019. Mechanics Modeling of Additive Manufactured Polymers; pp. 51–71. [DOI] [Google Scholar]
- 60.Jasiuk I., Abueidda D.W., Kozuch C., Pang S.Y., Su F.Y., McKittrick J. An Overview on Additive Manufacturing of Polymers. JOM. 2018;70:275–283. doi: 10.1007/s11837-017-2730-y. [DOI] [Google Scholar]
- 61.Yaragatti N., Patnaik A. A review on additive manufacturing of polymers composites. Mater. Today-Proc. 2021;44:4150–4157. doi: 10.1016/j.matpr.2020.10.490. [DOI] [Google Scholar]
- 62.Jofre L., del Rosario Z.R., Iaccarino G. Data-driven dimensional analysis of heat transfer in irradiated particle-laden turbulent flow. Int. J. Multiph. Flow. 2020;125:103198. doi: 10.1016/j.ijmultiphaseflow.2019.103198. [DOI] [Google Scholar]
- 63.Alshqirate A.A.Z.S., Tarawneh M., Hammad M. Dimensional Analysis and Empirical Correlations for Heat Transfer and Pressure Drop in Condensation and Evaporation Processes of Flow Inside Micropipes: Case Study with Carbon Dioxide (CO2) J. Braz. Soc. Mech. Sci. Eng. 2012;34:89–96. doi: 10.1590/S1678-58782012000100012. [DOI] [Google Scholar]
- 64.Levac M.L.J., Soliman H.M., Ormiston S.J. Three-dimensional analysis of fluid flow and heat transfer in single- and two-layered micro-channel heat sinks. Heat Mass Transf. 2011;47:1375–1383. doi: 10.1007/s00231-011-0795-7. [DOI] [Google Scholar]
- 65.Nakla M. On fluid-to-fluid modeling of film boiling heat transfer using dimensional analysis. Int. J. Multiph. Flow. 2011;37:229–234. doi: 10.1016/j.ijmultiphaseflow.2010.09.004. [DOI] [Google Scholar]
- 66.Illan F., Viedma A. Experimental study on pressure drop and heat transfer in pipelines for brine based ice slurry Part II: Dimensional analysis and rheological Model. Int. J. Refrig.-Rev. Int. Du Froid. 2009;32:1024–1031. doi: 10.1016/j.ijrefrig.2008.10.004. [DOI] [Google Scholar]
- 67.Nezhad A.H., Shamsoddini R. Numerical Three-Dimensional Analysis of the Mechanism of Flow and Heat Transfer in a Vortex Tube. Therm. Sci. 2009;13:183–196. doi: 10.2298/TSCI0904183N. [DOI] [Google Scholar]
- 68.Asgari O., Saidi M. Three-dimensional analysis of fluid flow and heat transfer in the microchannel heat sink using additive-correction multigrid technique; Proceedings of the Micro/Nanoscale Heat Transfer International Conference, 2008, PTS A AND B. 1st ASME Micro/Nanoscale Heat Transfer International Conference; Tainan, Taiwan. 6–9 January 2008; pp. 679–689. [Google Scholar]
- 69.Carabogdan G.I., Badea A., Bratianu C., Musatescu V. Methods of Analysis of Thermal Energy Processes and Systems. Tehn; Bucharest, Romania: 1989. [Google Scholar]
- 70.Șova M., Șova D. Thermotechnics, Part II. Transilvania University Press; Braşov, Romania: 2001. [Google Scholar]
- 71.Quintier G.J. Fundamentals of Fire Phenomena. John Willey & Sons; Hoboken, NJ, USA: 2006. [Google Scholar]
- 72.Ferro V. Assessing flow resistance law in vegetated channels by dimensional analysis and self-similarity. Flow Meas. Instrum. 2019;69:101610. doi: 10.1016/j.flowmeasinst.2019.101610. [DOI] [Google Scholar]
- 73.Khan M.A., Shah I.A., Rizvi Z., Ahmad J. A numerical study on the validation of thermal formulations towards the behaviors of RC beams. Sci. Mater. Today Proc. 2019;17:227–234. doi: 10.1016/j.matpr.2019.06.423. [DOI] [Google Scholar]
- 74.Lawson R.M. Fire engineering design of steel and composite Buildings. J. Constr. Steel Res. 2001;57:1233–1247. doi: 10.1016/S0143-974X(01)00051-7. [DOI] [Google Scholar]
- 75.Al-Homoud M.S. Performance characteristics and practical applications of common building thermal insulation materials. Build. Environ. 2005;40:353–366. doi: 10.1016/j.buildenv.2004.05.013. [DOI] [Google Scholar]
- 76.Papadopoulos A.M. State of the art in thermal insulation materials and aims for future developments. Energy Build. 2005;37:77–86. doi: 10.1016/j.enbuild.2004.05.006. [DOI] [Google Scholar]
- 77.Wong M.B., Ghojel J.I. Sensitivity analysis of heat transfer formulations for insulated structural steel components. Fire Saf. J. 2003;38:187–201. doi: 10.1016/S0379-7112(02)00057-7. [DOI] [Google Scholar]
- 78.Noack J., Rolfes R., Tessmer J. New layerwise theories and finite elements for efficient thermal analysis of hybrid structures. Comput. Struct. 2003;81:2525–2538. doi: 10.1016/S0045-7949(03)00300-6. [DOI] [Google Scholar]
- 79.Tafreshi A.M., di Marzo M. Foams and gels as temperature protection agents. Fire Saf. J. 1999;33:295–305. doi: 10.1016/S0379-7112(99)00031-4. [DOI] [Google Scholar]
- 80.Bailey C. Indicative fire tests to investigate the behavior of cellular beams protected with intumescent coatings. Fire Saf. J. 2004;39:689–709. doi: 10.1016/j.firesaf.2004.06.007. [DOI] [Google Scholar]
- 81.Yang K.-C., Chen S.-J., Lin C.C., Lee H.H. Experimental study on local buckling of fire-resisting steel columns under fire load. J. Constr. Steel Res. 2005;61:553–565. doi: 10.1016/j.jcsr.2004.07.001. [DOI] [Google Scholar]
- 82.Yao S., Yan K., Lu S., Xu P. Prediction and application of energy absorption characteristics of thinwalled circular tubes based on dimensional analysis. Thin-Walled Struct. 2018;130:505–519. doi: 10.1016/j.tws.2018.06.015. [DOI] [Google Scholar]
- 83.Kivade S.B., Murthy C.S.N., Vardhan H. The use of Dimensional Analysis and Optimisation of Pneumatic Drilling Operations and Operating Parameters. J. Inst. Eng. India Ser. D. 2012;93:31–36. doi: 10.1007/s40033-012-0001-1. [DOI] [Google Scholar]
- 84.Yen P.H., Wang J.C. Power generation and electrical charge density with temperature effect of alumina nanofluids using dimensional analysis Elsevier. Energy Convers. Manag. 2009;186:546–555. doi: 10.1016/j.enconman.2019.03.005. [DOI] [Google Scholar]
- 85.Bahrami A., Mousavi Anijdan S.H., Ekrami A. Prediction of mechanical properties of DP steels using neural network model. J. Alloys Compd. 2005;392:177–182. doi: 10.1016/j.jallcom.2004.09.014. [DOI] [Google Scholar]
- 86.Dai S.J., Zhu B.C., Chen Q. Applied Mechanics and Materials. Trans Tech Publications Ltd.; Freienbach, Switzerland: 2013. Analysis of bending strength of the rectangular hole honeycomb beam; pp. 993–999. [Google Scholar]
- 87.Deshwal P.S., Nandal J.S. On Torsion of Rectangular Beams with Holes at the Center. Indian J. Pure Appl. Math. 1991;22:425–438. [Google Scholar]
- 88.Aglan A.A., Redwood R.G. Strain-Hardening Analysis of Beams with 2 WEB- Rectangular Holes. Arab. J. Sci. Eng. 1987;12:37–45. [Google Scholar]
- 89.Vlase S., Nastac, Marin M., Mihalcica M. A Method for the Study of the Vibration of Mechanical Bars Systems with Symmetries. Acta Tech. Napocensis. Ser.-Appl. Math. Mech. Eng. 2017;60:539–544. [Google Scholar]
- 90.Fazakas-Anca I.S., Modrea A., Vlase S. Using the Stochastic Gradient Descent Optimization Algorithm on Estimating of Reactivity Ratios. Materials. 2021;14:4764. doi: 10.3390/ma14164764. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 91.Száva I.R., Șova D., Dani P., Élesztős P., Száva I., Vlase S. Experimental Validation of Model Heat Transfer in Rectangular Hole Beams Using Modern Dimensional Analysis. Mathematics. 2022;10:409. doi: 10.3390/math10030409. [DOI] [Google Scholar]
- 92.Dani P., Száva I.R., Kiss I., Száva I., Popa G. Principle schema of an original full-, and reduced-scale testing bench, destined to fire protection investigations. Ann. Fac. Eng. Hunedoara. 2018;16:149–152. [Google Scholar]
- 93.Creality, Cura4.7—Software and Machine Description. 2018.
- 94.Asztalos Z. Master’s Thesis. Transilvania University of Brasov; Brașov, Romania: 2021. MDA Implemented in Spare Parts’ Analysis Obtained by Rapid Prototyping. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.
Data Availability Statement
Not applicable.