Assessment of the Kochak-Benet Equation for Hepatic Clearance for the Parallel-Tube Model: Relevance of Classic Clearance Concepts in PK and PBPK

Abstract

This report reviews concepts related to operation of the classic parallel-tube model (PTM) for hepatic disposition and examines two recent proposals of a newly derived equation to describe hepatic clearance (CL_H). It is demonstrated that the proposed equation is identical to a re-arrangement of an earlier relationship from Pang and Rowland and provides a means of calculation of intrinsic clearance (CL_int,PTM) rather than CL_H as posed. We further demonstrate how classic hepatic clearance models with an assumed CL_int, while subject to numerous limitations, remain highly useful and necessary in both traditional pharmacokinetics (PK) and physiologically based pharmacokinetic (PBPK) modeling.

INTRODUCTION

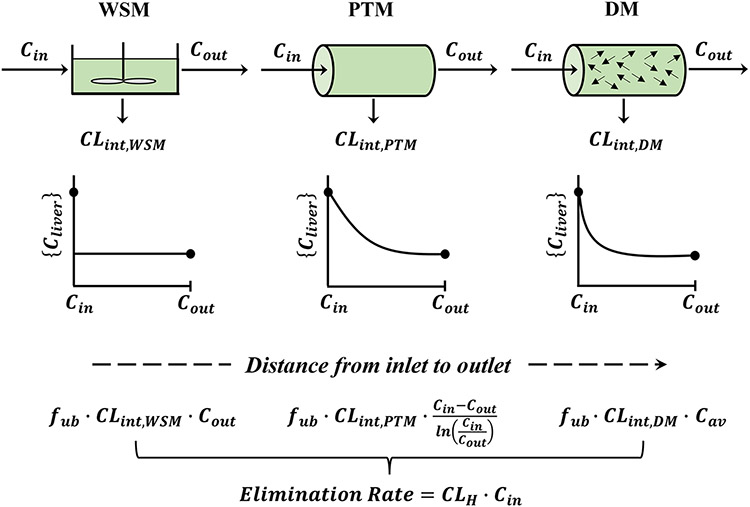
The primary models used in pharmacokinetics to describe hepatic clearance of many drugs are the well-stirred model (WSM), the parallel-tube model (PTM), and the dispersion model (DM) as shown in Fig. 1. The WSM, while greatly simplifying the structure of the liver and other organs, is easy to operate and is extensively used to generally describe hepatic drug disposition. It is primarily the basis of applying physiologically based pharmacokinetic (PBPK) models (1) and for utilizing in vitro metabolic data obtained from hepatocytes and microsomes for making in vivo extrapolations (IVIVE) (2).

Fig. 1.

Basic hepatic models. Representation of the well-stirred model (WSM), parallel-tube model (PTM), and dispersion model (DM) showing the same steady-state input (C_in) and outflow (C_out) drug concentrations in blood. The internal hepatic blood concentrations {C_liver} differ according to model assumptions. In turn, the systemically determined elimination rate calculated from CL_H × C_in and dose/AUC will be accompanied by differing intrinsic clearances {CL_int} as designated for the three models with fu_b as the fraction unbound in blood. The complex C_av for the DM falls between C_out and (C_in − C_out)/(ln(C_in/C_out)), and thus, the rank order of expected CL_int values is as follows: WSM > DM > PTM. It can be noted that the spatial distribution and exponential decline in blood concentrations in the PTM can be calculated from: $C_{blood,fx}=C_{in}•e^{\frac{-C{L}_{int,PTM•}fx}{Q_H}}$ where fx is the fractional distance between the inlet and outlet of the liver model

The WSM assumes that the liver is a single, well-stirred compartment and that the concentration of unbound drug in the emergent blood is in equilibrium with the unbound drug within the liver with elimination activity described as intrinsic clearance (3, 4). The PTM assumes that the liver is comprised of an array of identical and parallel tubes with enzymes distributed evenly in each cross-section of the sinusoidal vascular and perivascular space. This model was proposed by Winkler et al. (5, 6). The WSM assumes that the drug concentration in the liver is constant and equal to the emergent drug concentration (C_out), while the PTM assumes that there is an exponentially declining drug concentration from the inlet (C_in) to the outlet C_out. The properties and comparisons of the two models have been well described by Pang and Rowland (7, 8).

The model construct by Winkler et al. (5, 6) described the PTM with convective transport and Michaelis–Menten enzymatic metabolism that, for steady-state linear conditions, was written as follows:

$$Velocity=Q_H•C_{in}•(1-e^{-\frac{V_{max}}{K_{m•}{Q}_H}})$$ (1)

where Q_H is hepatic blood flow and V_max/K_m are capacity/equilibrium constants for metabolism. Pang and Rowland (7) recognized that Velocity/substrate concentration (C_in) is hepatic clearance (CL_H), V_max/K_m is intrinsic clearance (CL_int,PTM) for the PTM, and assumed that only fractional unbound drug in blood (fu_b) is subject to metabolism (viz. the “free drug hypothesis”) as applied in PK for the WSM to write the currently operative steady-state equation for the PTM as follows:

$$C{L}_H=Q_H•(1-e^{-\frac{f{u}_b•C{L}_{int,PTM}}{Q_H}})$$ (2)

They provided an array of ancillary equations to describe functioning of the PTM compared to the WSM (7) including the relationship:

$$C{L}_H=Q_H•E_H$$ (3)

where CL_H can be calculated from perfused organ measurements using E_H as the Extraction Ratio = (C_in − C_out)/C_in. They then compared the application of the two models to experimental data for several compounds (8).

ASSESSMENT OF THE KOCHAK-BENET EQUATION

This commentary partly addresses the recent derivations and equations that claim to describe a method of calculation of CL_H for the PTM. As developed by Kochak (9) based on an “advection mass transport” paradigm and by Benet et al. (10) based on a flow reactor perspective, it was posed that hepatic clearance (“CL_H”) can be calculated from:

$${}^{\prime }C{L}_H^{\prime }=Q_H•ln\left(\frac{C_{in}}{C_{out}}\right)$$ (4)

Furthermore, Kochak stated that Eq. 4 = Q_H x E with E described as “a new Extraction Factor” (9) for data obtained from typical organ perfusion experiments where steady-state C_in and C_out are measured. The two sets of authors used different assumptions and derivations to arrive at this equation. The Kochak E creates some confusion as it looks like the traditional E_H, but it is not. Neither author compared this equation to the expectations from the classic equations for the PTM (Eq. 2) nor assessed its relevance to in vivo PK or to PBPK models.

The following calculations were first performed to make such comparison. An array of Q_H and CL_int,PTM values were employed to calculate CL_H using Eq. 2 with fu_b assumed = 1. Pang and Rowland (7) showed for the PTM that for fu_b = 1:

$$C_{out}=C_{in}•e^{-\frac{C{L}_{int,PTM}}{Q_H}}$$ (5)

Thus, for assumed values of C_in, expected values of C_out can be obtained. In turn, the Kochak-Benet equation was applied to compare values of their “CL_H” (Eq. 4) for each pair of CL_int,PTM and Q_H values. Such calculations are provided in Table I with the values for the established PTM bolded in the denominators. The classic PTM functions as expected with highest CL_H values that are limited by Q_H.¹ It can be readily seen that the calculations for the “CL_H” from Eq. 4 do not agree with differences as great as 20-fold. Thus, it is evident that the Kochak-Benet equation is not equivalent to the classical PTM for calculating CL_H. Moreover, this equation produced “CL_H” values that matched the assumed CL_int,PTM values, many of which were assigned high values consistent with drugs producing large E_H values. Also, it can be observed that when C_in/C_out is larger than 3, then Eq. 4 predicts a “CL_H” that exceeds Q_H, which can only happen for CL_int.

Table I.

Comparison of Kochak-Benet (Eq. 4, Numerator) and Classical PTM (Eq. 2, Denominator) Equations for Hepatic Clearance (CL_H)

CLint,PTM	Assigned values of QH
	300	600	900	1200	1500
→ 0	CLint,PTM	CLint,PTM	CLint,PTM	CLint,PTM	CLint,PTM
300	300/190	300/236	300/255	300/265	300/272
600	600/259	600/379	600/438	600/472	600/495
900	900/285	900/466	900/569	900/633	900/677
1200	1200/295	1200/519	1200/663	1200/759	1200/826
1500	1500/298	1500/551	1500/730	1500/856	1500/948
3000	3000/300	3000/596	3000/868	3000/1101	3000/1297
6000	6000/300	6000/600	6000/899	6000/1192	6000/1470

Numbers have arbitrary values with units of volume/time

Upon further assessment, it can be readily shown that rearrangement of Eq. 5 taking ln(exp(-CL_int,PTM/Q_H)) produces Eq. 4. It is difficult to follow the original derivations for either model that led to Eq. 4 to determine why they produced an outcome that Eq. 4 reflects an intrinsic clearance rather than a systemic clearance (traditional CL_H). However, the simple adjustment of the Pang and Rowland equation easily produces Eq. 4. Also, Kochak’s “CL_H” value was also shown to serve in place of CL_int,PTM for calculation of the bioavailable fraction (F) escaping first-pass effect found previously for the PTM (7).

ADDITIONAL CONSIDERATIONS

There are some incidental concerns with the two publications as well. Kochak’s applications of Eq. 4 to the published data set for chromic phosphate colloid (and other compounds) from hepatic perfusion studies do not provide validation as claimed that Eq. 4 is superior to the Q_H x E_H relationship for CL_H. He appears to be indirectly comparing his model’s CL_int,PTM with the other’s CL_H and an ambiguous correction factor (a) was needed. The fittings of E_H versus Q_H used meaningless quadratic functions instead of the WSM E_H = CL_int/(Q_H + CL_int) or E_H from the PTM exponential in Eq. 2. Preferable is application of such full mechanistic equations for the WSM and PTM that compare E_H fittings as carried out by Pang and Rowland (8) who conceded that both models fitted some of these same data equally well (although the WSM was preferred for lidocaine). Such more proper comparisons have been done numerous times since (11). Kochak uses strong language to condemn the structure and operation of the WSM in advocating his version of the misinterpreted PTM. However, the two models have been compared more appropriately in numerous publications since that of Pang and Rowland (7, 8). Sodlii et al. (11) recently demonstrated the frequent similarity in fittings of both models for a diverse array of published data, and they opined at the time that “the simple but unphysiologic well-stirred model is the only model that can describe the trustworthy published available data.”

The Benet publication (10) primarily serves to remind readers that there are many definitions and approaches to assessment of clearance (CL) with the focus on differentiating the well-known calculation of CL_H from the elimination rate/plasma concentration ratio versus the various intrinsic clearances (that he redefines) that require operation of the various hepatic models as also shown here (Fig. 1). The former (“one valid definition of clearance”²) allows calculation of CL from Dose/AUC for a fully bioavailable drug. Invoking CL_int involves the need for a model for the liver and includes drug binding in blood (fu_b) and other assumptions if one seeks to describe or connect CL_H to enzymatic activity (V_max/K_m or CL_int,PTM). Benet et al. (10) compare some of the features of the WSM, PTM, and DM, which have well-elaborated structures and mathematical properties (12). As also shown previously (12), he points out with visualization (similar to Fig. 1) that the models display expected drug concentrations in hepatic blood that can differ considerably. In turn, this indicates that different intrinsic clearances will be needed to produce the same dose/AUC values and Q_H x E_H values. Benet et al. (10) convey that in vitro assessments of CL_int are commonly being scaled to in vivo CL_H based on the WSM. However, IVIVE has also been performed with the PTM (3) and both models offer similar rough approximations with underprediction of in vivo CL_H from in vitro CL_int data, particularly for high clearance drugs.

Other concerns with the Benet et al. article (10), besides misrecognition that Eq. 4 is the PTM intrinsic clearance, are that their Eq. 5 is the traditional CL_H = Q_H x E_H and they provide a “CL_H” equation for the DM with an unsupported configuration in place of the E_H term. Thus, posing that “all the models of organ elimination will yield different mechanistic liver clearance values (Eq. 5-7),” while correct in words, offers a mix of systemic, intrinsic, and conjured clearance equations for comparison. If one gives an IV dose of a drug cleared only by the liver, dose/AUC will yield one CL_H that is universal (i.e., “the one valid definition”). It is well appreciated (12) that application of the three hepatic models will yield differing intrinsic clearances owing to assumptions for the differing internal and unknowable hepatic drug concentrations {C_liver} and operative structures of the models. The mass balance for steady-state rate of drug elimination by hepatic metabolism is as follows:

$$EliminationRate=C{L}_H•C_{in}=\{C{L}_{int}\}•\{C_{liver}\}$$ (6)

with both the generic {CL_int} and {C_liver} terms being dependent on the assumed model, while CL_H is usually model-independent for a drug fully cleared by the liver (omitting consideration of fu_b). For example, {C_liver} is C_out for the WSM, while for the PTM:

$$\{C_{liver}\}=\frac{C_{in}-C_{out}}{ln(\frac{C_{in}}{C_{out}})}$$ (7)

as shown by Winkler et al. (5, 6) and depicted in Fig. 1. Note that all these concentrations refer to blood concentrations that equilibrate within the clearance organ.

RELEVANCE OF HEPATIC MODELS TO IN VIVO PHARMACOKINETICS

The classic hepatic models have been extensively applied to numerous drugs including in vitro and in vivo PK data for propranolol PK in rat and man, primarily using the WSM. This compound has been found to be well absorbed and subject to metabolism only by the liver. After IV doses in rats, the reported values for systemic blood clearance (viz. CL_H) that could be calculated from dose/AUC were 84.7 and 63.2 (13), 71.2 (plasma) (14), and 65.7 mL/min/kg (15). The typical blood/plasma ratio (R) is 1.16 (15) and fraction unbound in plasma (fu_p) is 0.13 (16).

Several direct assessments of hepatic extraction allow comparisons of systemic versus hepatic versus intrinsic clearances of propranolol in rats. Suzuki et al. (17) measured propranolol in femoral and hepatic vein blood during constant rate infusion of propranolol in rats and found E_H = 0.93. Hung et al. (18) found a similar E_H of 0.95 in perfused rat livers using an indicator dilution technique. Singh et al. (16) carried out IV and portal vein infusions of propranolol in rats to determine a steady-state E_H of 0.73. The mean oral bioavailability (F) of propranolol reported in rats by Shibasaki et al. (15) was 0.228, giving a mean E_H value (from E_H = 1 − F) of 0.772 (7). With their assessed Q_H value of 85 mL/min/kg, the CL_H can be calculated from Q_H × E_H as 65.6 mL/min/kg, similar to the above listed IV dose/AUC or CL_H values and consistent with propranolol being entirely metabolized by the liver. Furthermore, the results from the Shibasaki et al. (15) study allows (2) calculation of CL_int values from:

$$C{L}_{int,WSM}=\frac{C{L}_H}{1-\frac{C{L}_H}{Q_H}}$$ (8)

$$C{L}_{int,PTM}=Q_H•[-ln\left(\frac{Q_H-C{L}_H}{Q_H}\right)]$$ (9)

The CL_int,WSM value with E_H = 0.772 is 288 mL/min/kg and the corresponding CL_int,PTM value is 126 mL/min/kg. The larger value for the WSM is expected (Fig. 1) as well as both values being larger than CL_H and Q_H. Use of the E_H of 0.93 (17) or 0.95 (18) will produce even larger CL_int values.

Of particular note, application of the Kochak-Benet equation (using C_out = 0.228 C_in) to the E_H value for propranolol reported in the Shibasaki study (15) also yields a “CL_H” value of 126 mL/min/kg, matching CL_int,PTM rather than any of the other clearance values. It can be noted that including fu_b = 0.167 as applied in vivo (16) would produce much higher apparent CL_int values. As another means of calculation, the relationship for the PTM that F = exp(−CL_int,PTM/Q_H) described by Pang and Rowland (7) that was also offered by Kochak (9) produces the same PTM clearance value of 126 mL/min/kg for the Shibasaki et al. data (15).

The further relevance of classic clearance concepts can be demonstrated with propranolol studies in man. The E_H of propranolol was reported as 0.72 in man at oral doses at and above 30 mg based on PK data from oral and IV dosing (19), producing similar expectations that intrinsic clearances will be larger than Q_H. Indeed, the in vivo predicted CL_int value is reported as 267 for the WSM and 154 for the PTM, with 13.2 for CL_H and 21.9 mL/min/kg for Q_H for propranolol in humans (2, 19). Human PK data for propranolol were used by Gibaldi et al. (20) to first demonstrate how an IV dose/AUC value could be used with the WSM to estimate the first-pass availability (F) of an oral dose of drug. This approach was confirmed for propranolol and has since served as a simple method of using IV data to anticipate oral dose F if there are no product release issues. The value of oral dose/AUC reflects CL_int,WSM for drugs such as propranolol while a more complicated equation is needed to calculate CL_int,PTM(7). It is common practice to call such dose/AUC the “Oral Dose Clearance” with symbol CL/F in consideration that F may be less than 1 for multiple reasons.

As is common practice in human IVIVE today (2), an early study by Singh et al. (16) used rat hepatocytes to assess in vitro metabolism of propranolol and related it to in vivo results. They obtained an in vitro CL_int value based on disappearance of propranolol during an incubation experiment (substrate depletion method), scaled their hepatocyte number to the whole rat liver, used measured in vivo R (0.78) and fu_p (0.13) values, and applied the WSM with Q_H = 60 mL/min/kg to calculate the expected in vitro E_H. Their value (0.90) was larger than their own in vivo measurement of 0.73, but was closer to that (0.93) of Suzuki et al. (17) and (0.95) from Hung et al. (18). This difference in E_H values was attributed to nonlinear metabolism of propranolol (16). Similar approaches are currently used with human hepatocytes and microsomes to predict in vivo clearances (2).

As pointed out by Benet et al. (10), in vivo systemic clearance values measure CL_H for a drug fully metabolized by the liver, while in vitro assessments of CL_int using hepatocytes or microsomes require a model such as the WSM or PTM for scaling to CL_H (2) The use of IVIVE for human PK generally correlates well in terms of spanning low to high clearances over five orders of magnitude. However, using in vitro measurements with both the WSM and PTM systematically underpredicts in vivo clearances by about five-fold (2), perhaps for some of the reasons described in the next section. Nevertheless, there is considerable value in making such predictions with the expectation that they will only be approximate.

RELEVANCE OF MODEL-DEPENDENT CLEARANCES IN PBPK

It can be observed that commonly applied basic PBPK models usually follow the principles of Bischoff and Dedrick (21) who added a V_max/K_m metabolic function acting on C_out to Fick’s Law of Perfusion to describe hepatic distribution and elimination of thiopental. They subsequently invoked clearance terminology to describe various drug elimination processes in a PBPK model for methotrexate (22). Their relationships were predecessors to the simple PBPK differential equation (DE):

$$\frac{d{C}_{liver}}{dt}=\left(Q_H•\left(C_{in}-C_{out}\right)-C{L}_{int,WSM}•C_{out}\right)∕V_{liver}$$ (10)

where C_liver is the measured and modeled total hepatic drug concentration and V_liver is actual liver volume. When C_in and C_out are plasma concentrations, it is commonplace to substitute C_out = C_liver/K_p, where K_p is an equilibrium tissue/plasma partition coefficient. In addition, the CL_int term is usually operated with fraction unbound in plasma as fu_p × CL_int in assuming that the free drug hypothesis applies. The liver and all other organs and tissues in the body are thus essentially described in simple PBPK models by the WSM, which may not be generally appreciated (10). However, PBPK models provide fitted or predicted C_in versus time profiles from which the systemic clearance can be readily calculated as a secondary parameter from dose/AUC with operation of the DE, dAUC/dt = C_in. Such values will be slightly smaller than conventionally calculated clearance values when the initial condition of the plasma concentration DE is dose/blood volume (23). This systemic or whole body clearance is helpful in providing a summation of all clearance processes in the body.

It is readily possible to test various added complexities, including applying Eq. 7 for the PTM, to create and assess alternative liver (and other tissue) models in PBPK modeling. It can be pointed out that PBPK models for organs described partly by Fick’s Law of Perfusion require addition of tissue concentrations as a model-assigned variable with specified assumptions to operate the DE. Since C_out is seldom or even impossible to measure in vivo for individual organs and tissues (how could this be done for bone, skin, muscle, and fat?), the C_liver term reduces the number of variables in the DE. A model-independent CL_H × C_in may not even operate in PBPK models to describe hepatic drug concentrations. The fact that PBPK models have been applied successfully and extensively for numerous drugs, often with commercial software, indicates the considerable utility of the WSM in the field. This simple organ model is a major cornerstone of PK that can be augmented when either various complexities are encountered or assumptions are called for.

SOME LIMITATIONS OF THE HEPATIC AND PBPK MODELS

The three basic hepatic models, of course, only apply to drugs that are fully bioavailable and subject to hepatic metabolism and/or biliary excretion and without transporter involvement (24). For the latter situation, both influx and efflux clearances can be added to the basic models (12). Within PBPK models, the hepatic models operate with C_in reflecting about 80% input of blood from the portal vein and the remaining from the hepatic artery thus allowing for first-pass effects for oral or intraperitoneal doses and helping to inform (CL_in values. Drugs that undergo breakdown by hydrolysis, esterases, or proteolysis in blood will have CL = Dose/AUC values that are not rate-limited by any blood flow. Numerous drugs undergo reversible metabolism (futile cycling) where dose/AUC reflects a combination of loss and return clearances (25, 26). Similar considerations apply when intestinal secretion and/or biliary excretion occur with enterohepatic cycling (27). Some drugs may efflux slowly from red blood cells (RBC) where the models should either account for such diffusion or employ a Q_H that is less than blood flow (28). Compounds with low permeability (PS) require model adjustments that account for this property (29).

Perhaps, the greatest uncertainty in operation of hepatic and PBPK models, as well as in pharmacodynamics, is the application of the free drug hypothesis with the use of fu_p in the WSM or PTM. Albumin-mediated hepatic drug uptake (30) and rapid dissociation of protein-bound drug (31) have been demonstrated. Both the RBC and albumin have been shown with indicator dilution studies to traverse the rat liver in about 1 min (32). Drugs typically bind to their targets with equilibrium dissociation constants (K_D) ranging from 10⁻⁸ to 10⁻¹² M with a dissociation half-life for k_off as small as minutes (33). Drug binding to plasma proteins is much weaker and k_off values may be too fast to measure. Also, proteins, perhaps carrying drugs, can slowly enter tissues by convection as recognized in PBPK models for monoclonal antibodies (34). An insightful review by Bowman and Benet (35) observed, “…some highly bound ligands have more efficient [hepatic] uptake than can be explained by their unbound fraction.” Attempts in IVIVE and PBPK to utilize in vitro metabolism data using the WSM or PTM may have additional limitations owing to similar considerations for in vitro nonspecific cell or tissue binding where only the free drug is assumed to be metabolized and the need to extrapolate tissue dilution binding values to the whole organ (36).

In spite of these complexities and uncertainties, the basic hepatic models offer highly useful starting points in PK and PBPK in considering tissue distribution and clearance processes for drugs. Any of these complexities, if supported by experimental data, can be built into these foundational hepatic models.

CONCLUSIONS

The recently developed Kochak-Benet equation reflects CL_int,PTM (rather than CL_H) for the PTM as confirmed by simulations, re-arrangement of an early equation from Pang and Rowland (7), and assessment of published in vivo PK data for propranolol. Their Q_H x E equation may find utility as a quick method of calculation of CL_int,PTM when typical organ extraction-type data are available. Considerations of the model-dependency of {CL_int} as well as {C_liver} provide insights into the assumptions, requirements, and limitations of IVIVE, PK, and PBPK modeling.