Comprehensive understanding of multiple resonance thermally activated delayed fluorescence through quantum chemistry calculations

Abstract

Molecules that exhibit multiple resonance (MR) type thermally activated delayed fluorescence (TADF) are highly efficient electroluminescent materials with narrow emission spectra. Despite their importance in various applications, the emission mechanism is still controversial. Here, a comprehensive understanding of the mechanism for a representative MR-TADF molecule (5,9-diphenyl-5,9-diaza-13b-boranaphtho[3,2,1-de]anthracene, DABNA-1) is presented. Using the equation-of-motion coupled-cluster singles and doubles method and Fermi’s golden rule, we quantitatively reproduced all rate constants relevant to the emission mechanism; prompt and delayed fluorescence, internal conversion (IC), intersystem crossing, and reverse intersystem crossing (RISC). In addition, the photoluminescence quantum yield and its prompt and delayed contributions were quantified by calculating the population kinetics of excited states and the transient photoluminescence decay curve. The calculations also revealed that TADF occurred via a stepwise process of 1) thermally activated IC from the electronically excited lowest triplet state T₁ to the second-lowest triplet state T₂, 2) RISC from T₂ to the lowest excited singlet state S₁, and 3) fluorescence from S₁.

Molecules that exhibit multiple resonance thermally activated delayed fluorescence (MR-TADF) are highly efficient electroluminescent materials, but their emission mechanism is still not fully understood. Here, the emission mechanism of a representative MR-TADF molecule is studied through quantum chemistry calculations, with two consecutive decay processes unveiled.

Introduction

Since highly efficient thermally activated delayed fluorescence (TADF) was used in organic light-emitting diodes (OLEDs)¹, many TADF molecules containing various electron donor and acceptor groups have been developed^2–9. An important factor for TADF efficiency is the energy difference between the lowest triplet state (T₁) and the lowest excited singlet state (S₁), ∆E(T₁ → S₁); a small ∆E(T₁ → S₁) (<200 meV) is required to induce T₁ → S₁ reverse intersystem crossing (RISC). Another important factor is a large transition-dipole moment between S₁ and ground-state S₀ to accelerate the rate of S₁ → S₀ fluorescence. Experimentally, efficient RISC and high photoluminescence quantum yields (PLQYs) have been simultaneously realized by combining suitable donor and acceptor units that control the spatial overlap between the highest-occupied molecular orbitals (HOMOs) and the lowest-unoccupied molecular orbitals (LUMOs)^1–9. It is important to note that the fluorescence spectra were broadened because of the charge-transfer character of S₁, which was a significant drawback for OLED applications in displays.

In 2016, Hatakeyama et al. developed a new class of TADF molecules¹⁰. Using a triphenylboron core possessing two nitrogen atoms (5,9-diphenyl-5,9-diaza-13b-boranaphtho[3,2,1-de]anthracene, DABNA-1, Fig. 1a), they observed a HOMO–LUMO separation without a conventional donor–acceptor structure, providing efficient TADF with a narrow emission spectrum. The HOMO–LUMO separation resulted from a multiple resonance (MR) effect, that is an opposite resonance effect induced by the boron and nitrogen atoms. MR–TADF molecules emitting blue-to-red fluorescence have been reported^11–39.

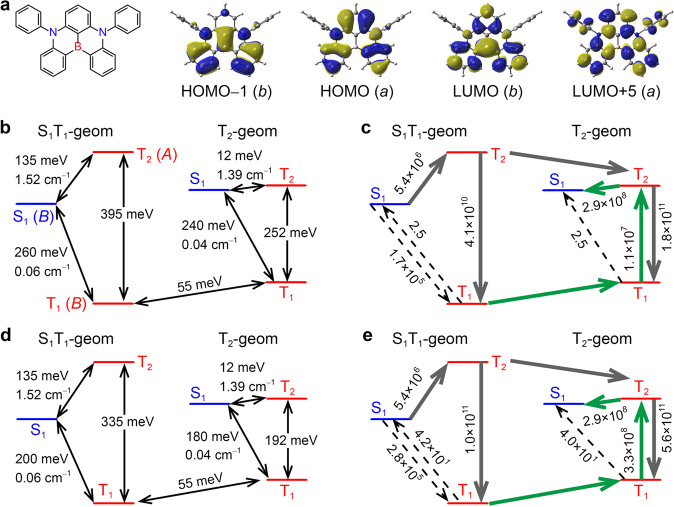
Fig. 1. Molecular orbitals and electronic transitions of DABNA-1.

a Structure of DABNA-1 and HOMO-1, HOMO, LUMO, and LUMO+5 distributions calculated at the RHF/6-31 G level (HOMO = highest-occupied molecular orbital, LUMO = lowest-unoccupied molecular orbital). The orbital symmetry is shown in parentheses. b Energy differences (meV) between S₁, T₁, and T₂. S₁–T₁ and S₁–T₂ spin–orbit couplings (cm⁻¹). The state symmetry is shown in parentheses. c The stepwise S₁ → T₂ → T₁ and T₁ → T₂ → S₁ processes of DABNA-1. The gray solid arrow depicts stepwise S₁ → T₂ → T₁; the green solid arrow depicts stepwise T₁ → T₂ → S₁; the dotted arrows show minor decay and upconversion processes. The values are calculated rate constants for one-step transitions. d The energy differences calculated using the corrected T₁ energy. e Calculated rate constants for one-step transitions using the corrected T₁ energy.

Several theoretical studies have attempted to reveal the TADF mechanism in MR molecules. Northey et al. investigated an intersystem-crossing (ISC) mechanism in DABNA-1 using quantum dynamics and time-dependent density-functional theory (TD-DFT) at the PBE0/6-31 G(d) level⁴⁰. There was only a 0.02-eV energy difference between T₂ and S₁, ∆E(T₂ → S₁). However, ∆E(T₁ → S₁) and ∆E(T₁ → T₂) were large, 0.59 eV and 0.61 eV, respectively, which could not explain efficient RISC from T₁ to S₁. Gao et al. examined the density-functional dependence of ∆E(T₁ → S₁) within the framework of TD-DFT⁴¹. The MPWK1CIS functional reproduced the experimental ∆E(T₁ → S₁)⁴¹, as reviewed by Suresh et al.²¹. However, the RISC rate constant (k_RISC) was not calculated and the TADF mechanism was unclear. Also, they considered TADF in terms of direct (one-step) T₁ → S₁ RISC, which differed from the work of Northey et al⁴⁰. Pershin et al. reported that TD-DFT methods overestimated ∆E(T₁ → S₁) for MR molecules, and that the spin-component-scaling second-order approximate coupled-cluster (SCS-CC2) method outperformed TD-DFT for predicting ∆E(T₁ → S₁)^42,43. The partial inclusion of double excitations within the SCS-CC2 method was responsible for the improved accuracy in predicting ∆E(T₁ → S₁). The SCS-CC2 calculation of DABNA-1-based molecules revealed the relationship between its molecular structure and electronic properties, ∆E(T₁ → S₁), and the fluorescence rate constant. However, the TADF mechanism was still unclear because the k_RISC was not calculated. Lin et al. calculated rate constants for fluorescence (k_F), ISC (k_ISC), k_RISC, and internal conversion (IC) (k_IC) for DABNA-1⁴⁴. However, the calculated k_RISC was 6.7 × 10²s⁻¹, which was much less than the experimental value of 1.0 × 10⁴ s⁻¹¹⁰. The calculated k_ISC of 1.4 × 10⁴s⁻¹ was two orders of magnitude less than the experimental value of 4.5 × 10⁶ s⁻¹¹⁰. In addition, the calculated nonradiative decay, k_IC(S₁ → S₀), was greater than the k_F(S₁ → S₀), suggesting that the PLQY of DABNA-1 was less than 50%, in contrast with the 88% experimental value¹⁰. All these previous studies^40–44 only partially explained the photophysical properties of MR-TADF mainly because crucial RISC was not elucidated.

Here, we report a comprehensive understanding of the TADF mechanism using the representative MR molecule, DABNA-1. So far, ∆E(T₁ → S₁) (and spin–orbit coupling (SOC) in some cases) and oscillator strength have been considered to understand the TADF mechanism and to design TADF molecules. However, these are not sufficient for the above aim; quantifications of all types of rate constants and those of energy levels, including higher-lying states relevant to the emission process, are required for the complete understanding of the emission mechanism. Recently, we reported rate-constant predictions enabled by a proposed cost-effective calculation method based on Fermi’s golden rule⁴⁵. Using the method, we calculated k_IC, k_ISC, k_RISC, k_F, and the rate constant for phosphorescence (k_Phos), of benzophenone as an example. The calculated rate constants agreed well with experimental values. Here, we applied the same method to DABNA-1 and determined all relevant rate constants. We also determined the lifetime of the delayed component in transient PL (trPL), the rate constant of TADF (k_TADF), and the PLQY, by calculating the population kinetics of the excited states and the trPL decay curve. The calculations also indicated that, after photoexcitation, T₁ was first generated via S₁ → T₂ ISC and T₂ → T₁ IC. Then, triplet-to-singlet conversion occurred via thermally activated T₁ → T₂ IC and T₂ → S₁ RISC.

Results and discussion

Excited states of DABNA-1

S₀, S₁, T₁, and T₂ geometries of DABNA-1 were obtained at the TPSSh/6-31+G(d) level. Then, excited states were computed using the equation-of-motion coupled-cluster singles and doubles (EOM-CCSD) method (see “Methods” section). At the TD-TPSSh level, S₁ and T₁ local-energy minima were located at the S₁- and T₁-optimized geometries, respectively. However, as shown in Supplementary Table 10–12 and Supplementary Fig. 3, the lowest S₁ and T₁ energy levels calculated at the EOM-CCSD level were both located at the T₁ geometry (3.37 and 3.12 eV, respectively). In addition, the S₁ and T₁ structures were nearly the same, including the dihedral angles (Supplementary Table 5), hence, the structure was denoted the S₁T₁ geometry. Because the Stokes shift was experimentally observed for DABNA-1 in solution and in solid films¹⁰, it was reasonably assumed that fluorescence occurs in  the S₁ structure at the adiabatic energy minimum. The S₀, S₁, T₁, and T₂ electronic states and the S₁T₁ and T₂ equilibrium geometries were used to model TADF for DABNA-1 (eight electronic states in all). Because S₂ states were 0.89 eV higher in energy than the S₁ states, their contributions were neglected (Supplementary Fig. 3). The T₃ states were only 0.2 eV higher than S₁, but were neglected because the electronic-transition rate constants from S₁ to T₃ were far smaller than those of the competing processes (S₁–T₁ and S₁–T₂) and that from T₂ to T₃ was smaller than that from T₂ to T₁ (Supplementary Fig. 3). In the EOM-CCSD calculations, S₁ and T₁ involved predominantly HOMO → LUMO transitions, whereas T₂ involved predominantly a linear combination of HOMO → LUMO + 5 and HOMO − 1 → LUMO transitions (Fig. 1a, Supplementary Fig. 2, and Supplementary Tables 6–9). Figure 1b shows a calculated energy-level diagram of DABNA-1. The adiabatic T₁ → S₁ energy difference calculated with the EOM-CCSD/6-31 G method (S₁T₁ geometry) was only 0.06 eV larger than the experimentally obtained 0.2 eV. Thus, EOM-CCSD significantly improved the overestimation of ∆E(T₁ → S₁), relative to those by previously reported TD-DFT methods^40,42–44.

Calculation of rate constants and luminescence quantum efficiencies

We examined triplet formation from S₁. Figure 1b shows calculated energy differences and SOCs, and Table 1 lists calculated and experimental values of k_IC, k_ISC, k_RISC, k_F, and k_Phos. The raw rate constants in Table 1 were calculated using excitation energies calculated via EOM-CCSD. Corrected values are discussed below. Equations for k_IC, k_ISC, k_RISC, k_F, and k_Phos are given in Supplementary Equations S1–S9 and Supplementary Fig. 4.

Table 1.

Calculated and experimental photophysical properties of DABNA-1.

	Calc.		Expt.
	Raw*	Corrected**
∆E(T1 → S1)	240	180
∆E(T2 → S1)	−12	−12
∆E(T1 → T2)	252	192	196
kF(S1 → S0)	1.4 × 108 s−1	1.4 × 108 s−1	1.0 × 108 s−1
kIC(S1 → S0)	1.2 × 107 s−1	1.2 × 107 s−1	1.3 × 107 s−1
kISC(S1 → T1)	1.7 × 105 s−1	2.8 × 105 s−1
kISC(S1 → T2)	5.4 × 106 s−1	5.4 × 106 s−1
kIC(T2 →T1)	1.8 × 1011 s−1	5.6 × 1011 s−1
kRISC(T2 → S1)	2.9 × 108 s−1	2.9 × 108 s−1
kPhos(T1 → S0)	9.4 × 10−1 s−1	1.9 × 10−1 s−1
kIC(T1 → T2)	1.1 × 107 s−1	3.3 × 108 s−1
kRISC(T1 → S1)	2.5 s−1	4.2 × 101 s−1
Φ	0.92	0.92	0.88
Φp	0.89	0.89	0.85
ΦTADF	2.9 × 10−2	3.2 × 10−2	3.5 × 10−2
ΦISC	3.5 × 10−2	3.5 × 10−2	4.0 × 10−2
τTADF	5.2 × 10−4 s	5.1 × 10−5 s	9.4 × 10−5 s
kTADF	0.16 × 104 s−1	1.8 × 104 s−1	0.94 × 104 s−1
k(T1 → T2 → S1)	0.17 × 104 s−1	1.9 × 104 s−1	1.0 × 104 s−1
k(S1 → T2 → T1)	5.4 × 106 s−1	5.4 × 106 s−1	4.5 × 106 s−1

Experimental values are from Hatakeyama et al.¹⁰.

^*Energy differences were calculated using the EOM-CCSD/6-31 G method (Fig. 1b,c).

^**Corrected energy differences were used (Fig. 1d,e).

Calculated values of k_F(S₁ → S₀) (1.4 × 10⁸s⁻¹) and k_IC(S₁ → S₀) (1.2 × 10⁷s⁻¹) agreed quantitatively with experimental results (1.0 × 10⁸s⁻¹ and 1.3 × 10⁷s⁻¹, respectively) determined by Hatakeyama et al.¹⁰ from PL decay curves of a 1-wt% DABNA-1: 9,9′-biphenyl-3,3′-diylbis-9H-carbazole film at 300 K (Table 1). The experimentally obtained k_ISC value (4.5 × 10⁶s⁻¹) agreed with k_ISC(S₁ → T₂) (5.4 × 10⁶s⁻¹), rather than k_ISC(S₁ → T₁) (1.7 × 10⁵s⁻¹), suggesting that the experimental k_ISC should be assigned to k_ISC(S₁ → T₂). The k_ISC(S₁ → T₂) was ten times greater than k_ISC(S₁ → T₁), because of the larger S₁–T₂ SOC (1.52 cm⁻¹) relative to that of S₁–T₁ (0.06 cm⁻¹). The large S₁ → T₂ SOC enhanced S₁ → T₂ ISC, despite the uphill transition from S₁ to T₂ (the Franck–Condon energy difference was 135 meV, Fig. 1b and Supplementary Table 13), compared with the downhill transition from S₁ to T₁ (260 meV). The small S₁–T₁ SOC resulted from very similar S₁ and T₁ orbital configurations (HOMO → LUMO transition). These results suggested that the ISC (S₁ → T₁ conversion) of DABNA-1 occurred via the stepwise S₁ → T₂ → T₁ process, rather than by the one-step S₁ → T₁ ISC, irrespective of the geometry relaxation (gray arrows in Fig. 1c). Such T₂-supported ISC is experimentally observed for a TADF system when ∆E(S₁ → T₂) is sufficiently small⁴⁶. Because T₂ → T₁ IC was much faster than S₁ → T₂ ISC, the latter was the rate-determining step of the S₁ → T₂ → T₁ process (Fig. 1c). Hence, the net rate constant k(S₁ → T₂ → T₁) was approximately equal to k_ISC (S₁ → T₂) = 5.4 × 10⁶s⁻¹ (Table 1).

For RISC from T₁, the calculated k_RISC(T₁ → S₁) (2.5 s⁻¹) was considerably less than the experimental value (1 × 10⁴s⁻¹)¹⁰, indicating that T₁ → S₁ conversion did not occur in one-step but via a more effective process. Because the S₂ and T₃ energy levels, as well as higher singlet and triplet states, could not contribute to the RISC from T₁, as discussed above, and T₂ was close to S₁ (see Supplementary Fig. 3), stepwise T₁ → T₂ → S₁ was the most promising mechanism (green arrows in Fig. 1c). To determine k(T₁ → T₂ → S₁), we calculated the trPL decay curve (Fig. 2) via kinetic equations (Supplementary Equations S10–S17, Supplementary Table 15, and Supplementary Note 1). The total PLQY (Φ) and the TADF lifetime (τ_TADF) were then obtained from the trPL decay curve (Fig. 2). The prompt and delayed components of Φ (Φ_P and Φ_TADF, respectively) were calculated from

$$\begin{array}{c}{\mathrm{\Phi }}_{\mathrm{P}}=\hfill \\ \frac{k_{\mathrm{F}}\left({\mathrm{S}}_1\to {\mathrm{S}}_0\right)}{k_{\mathrm{F}}\left({\mathrm{S}}_1\to {\mathrm{S}}_0\right)+k_{\mathrm{IC}}\left({\mathrm{S}}_1\to {\mathrm{S}}_0\right)+k_{\mathrm{ISC}}\left({\mathrm{S}}_1\to {\mathrm{T}}_2\right)+k_{\mathrm{ISC}}\left({\mathrm{S}}_1\to {\mathrm{T}}_1\right)}\hfill \end{array}$$ 1

$${\mathrm{\Phi }}_{\mathrm{TADF}}=\mathrm{\Phi }-{\mathrm{\Phi }}_{\mathrm{P}}$$ 2

and the ISC quantum yield (Φ_ISC) was calculated from

$$\begin{array}{c}{\mathrm{\Phi }}_{\mathrm{ISC}}=\hfill \\ \frac{k_{\mathrm{ISC}}\left({\mathrm{S}}_1\to {\mathrm{T}}_2\right)+k_{\mathrm{ISC}}\left({\mathrm{S}}_1\to {\mathrm{T}}_1\right)}{k_{\mathrm{F}}\left({\mathrm{S}}_1\to {\mathrm{S}}_0\right)+k_{\mathrm{IC}}\left({\mathrm{S}}_1\to {\mathrm{S}}_0\right)+k_{\mathrm{ISC}}\left({\mathrm{S}}_1\to {\mathrm{T}}_2\right)+k_{\mathrm{ISC}}\left({\mathrm{S}}_1\to {\mathrm{T}}_1\right)}\hfill \end{array}$$ 3

Fig. 2. Calculated transient photoluminescence (trPL) decay curve.

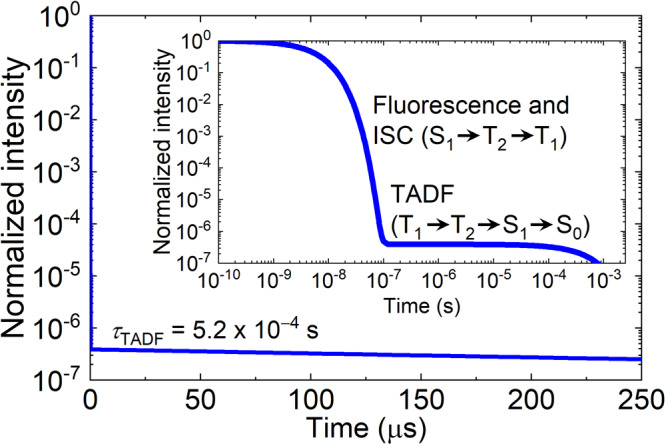
The inset is a log–log plot of the decay curve.

Then, k_TADF was determined from¹⁰

$$k_{\mathrm{TADF}}=\frac{{\mathrm{\Phi }}_{\mathrm{TADF}}}{{\mathrm{\Phi }}_{\mathrm{ISC}}{\tau }_{\mathrm{TADF}}}$$ 4

Finally, k(T₁ → T₂ → S₁) was calculated from

$$k\left({\mathrm{T}}_1\to {\mathrm{T}}_2\to {\mathrm{S}}_1\right)=\frac{k_{\mathrm{F}}\left({\mathrm{S}}_1\to {\mathrm{S}}_0\right)\times k_{\mathrm{TADF}}}{k_{\mathrm{F}}\left({\mathrm{S}}_1\to {\mathrm{S}}_0\right)-k\left({\mathrm{S}}_1\to {\mathrm{T}}_2\to {\mathrm{T}}_1\right)}$$ 5

Table 1 also lists calculated Φ, Φ_P, Φ_TADF, Φ_ISC, τ_TADF, k_TADF, k(T₁ → T₂ → S₁), and k(S₁ → T₂ → T₁) values. The Φ, Φ_P, Φ_TADF, and Φ_ISC values were quantitatively consistent with the experimental values because of the quantitative predictions for k_F(S₁ → S₀), k_IC(S₁ → S₀), k_ISC(S₁ → T₂), and k_ISC(S₁ → T₁). The calculated conversion rate constant k(S₁ → T₂ → T₁) was consistent with the experimental $k_{\mathrm{ISC}}$, suggesting that the experimental ISC should be assigned to S₁ → T₂ → T₁ ISC rather than direct S₁ → T₁ ISC. All the calculated single-step rate constants and k(S₁ → T₂ → T₁) agreed well with the experimental values. However, the agreements of the calculated k_TADF and k(T₁ → T₂ → S₁) (0.16 × 10⁴s⁻¹ and 0.17 × 10⁴s⁻¹, respectively) and the experiments (0.94 × 10⁴s⁻¹ and 1.0 × 10⁴s⁻¹, respectively) were not as good as we expected, compared with the case of k(S₁ → T₂ → T₁). Considering that they included the uphill-energy T₁ → T₂ IC, there was a possibility that the overestimation of ∆E(T₁ → T₂) leads to an underestimation of k_IC(T₁ → T₂), k_TADF, and k(T₁ → T₂ → S₁).

To examine the effect of ∆E(T₁ → T₂) on k_IC(T₁ → T₂), k_TADF, and k(T₁ → T₂ → S₁), we recalculated these rate constants using a corrected ∆E(T₁ → T₂). The rate-determining step for the T₂-mediated RISC was T₁ → T₂ IC; hence, ∆E(T₁ → T₂) could be viewed as the activation energy for DABNA-1 TADF and RISC. As discussed above, the energy difference between the calculated and experimental T₁ → S₁ was 60 meV. Thus, 60 meV was subtracted from the EOM-CCSD-calculated ∆E(T₁ → T₂) value of 252 meV. The energy levels and rate constants calculated with the corrected ∆E(T₁ → T₂) are also listed in Table 1. The value of k(S₁ → T₂ → T₁) was unchanged, suggesting that the T₁ energy correction had little effect on the ISC. In contrast, k_IC(T₁ → T₂) increased from 1.1 × 10⁷s⁻¹ to 3.3 × 10⁸s⁻¹. As a result, k_TADF and k(T₁ → T₂ → S₁) increased to 1.8 × 10⁴s⁻¹ and 1.9 × 10⁴s⁻¹, respectively, which were in much better agreements with the experiments. These results suggested that the ∆E(T₁ → T₂) overestimation was responsible for the underestimation of k_TADF and k(T₁ → T₂ → S₁). Thus, k_TADF and k(T₁ → T₂ → S₁) depended largely on k_IC(T₁ → T₂), which was the sum of IC rate constants for individual molecular vibrations, k_IC,α(T₁ → T₂), where α denoted the αth vibrational mode (Supplementary Equations S5–S7). A combined in-plane and out-of-plane bending mode (743 cm⁻¹) had the largest k_IC,α(T₁ → T₂) value (Supplementary Table 14 and Supplementary Fig. 5), suggesting that the T₁ → T₂ IC was predominantly accelerated by the bending mode.

Summary

In this work, we calculated the IC, ISC, RISC, fluorescence, phosphorescence, and TADF rate constants for DABNA-1, using EOM-CCSD wave functions and Fermi’s golden rule. The values quantitatively reproduced those from experiments, and thus validated the calculations. Various quantum yields, including PLQY, were also predicted and we revealed the DABNA-1 decay mechanism. The calculated population kinetics and the trPL decay curve indicated that TADF from DABNA-1 occurs via consecutive two processes, T₁ → T₂ IC, T₂ → S₁ RISC, and S₁ → S₀ radiative decay. Our proposed method here will be useful for accurate prediction of all rate constants and quantum yields relevant to OLED phenomena for various compounds with a wide range of structures.

Methods

Calculation of matrix elements between vibronic states

Geometry optimization and frequency analysis of S₀ for DABNA-1 were performed using the TPSSh/6-31+G(d) method, whereas those of S₁, T₁, and T₂ were performed using the TD-TPSSh/6-31+G(d) method with spin multiplicity = 1 (Supplementary Table 1–5 and Supplementary Fig. 1). The polarizable continuum model (PCM) of a CH₂Cl₂ solvent was used to consider the effects on vibronic states. We used the functional and the PCM conditions for ground- and excited-state geometry optimizations because Gao et al. reported that the TPSSh/6-31+G(d)-PCM model reproduced experimental DABNA-1 emission and absorption wavelengths in CH₂Cl₂⁴¹. The geometry optimization and frequency analysis were performed with the Gaussian 16 program package⁴⁷. For MR molecules, EOM-CCSD method and algebraic diagrammatic construction of the second order, have been shown to be suitable for calculating singlet–triplet energy differences^42,48–50. Here, excited-state calculations were performed using the EOM-CCSD/6-31 G method implemented in the Q-Chem program package⁵¹. Excitation energies, SOCs, vibronic couplings, transition-dipole moments, and permanent dipole moments were calculated using EOM-CCSD wave functions. Examples of Q-Chem input files for running SOC calculations are shown in the Supplementary Methods.

Calculation of IC rate constant

Mathematical formulation of a method of calculating vibronic couplings and k_IC is described elsewhere^45,52 and reviewed in Supplementary Equation S5–S9. First, for each vibrational mode α and each ${\mathrm{S}}_m\text{-}{\mathrm{S}}_n$ transition, $\mathrm{\Delta }{E}_{\mathrm{FC}}\left({\mathrm{S}}_m\text{-}{\mathrm{S}}_n\right)$ and $V_{\alpha }$ were obtained from the EOM-CCSD calculations (Supplementary Equation S7) and ${\mathrm{LSF}}_{\mathrm{IC},\alpha }$ was obtained from the frequency analyses performed using the TPSSh/6-31+G(d)-PCM model (Supplementary Equations S8 and S9). Then, $k_{\mathrm{IC},\alpha }\left({\mathrm{S}}_m\text{-}{\mathrm{S}}_n\right)$ was calculated (Supplementary Equation S6). Finally, $k_{\mathrm{IC},\alpha }\left({\mathrm{S}}_m\text{-}{\mathrm{S}}_n\right)$ was calculated by summing $k_{\mathrm{IC},\alpha }\left({\mathrm{S}}_m\text{-}{\mathrm{S}}_n\right)$ over α (Supplementary Equation S5). This protocol was also used for calculating the IC rate constants for ${\mathrm{T}}_m\text{-}{\mathrm{T}}_n$ transitions.

Author contributions

K.S. performed the theoretical calculations. H.K. planned and supervised the project. All authors contributed to the writing of this paper and have approved the final version.

Peer review

Peer-review information

Communications Chemistry thanks Jianzhong Fan and the other, anonymous, reviewer(s) for their contribution to the peer review of this work. Peer-reviewer reports are available.

Data availability

All relevant data are available from the authors upon request.

Code availability

The code used to generate Fig. 2 is available from the authors upon request.

Competing interests

The authors have no competing interests.

Supplementary information

The online version contains supplementary material available at 10.1038/s42004-022-00668-6.